Skeletal Rearrangements of the C240 Fullerene: Efficient Topological Descriptors for Monitoring Stone–Wales Transformations
Abstract
1. Introduction
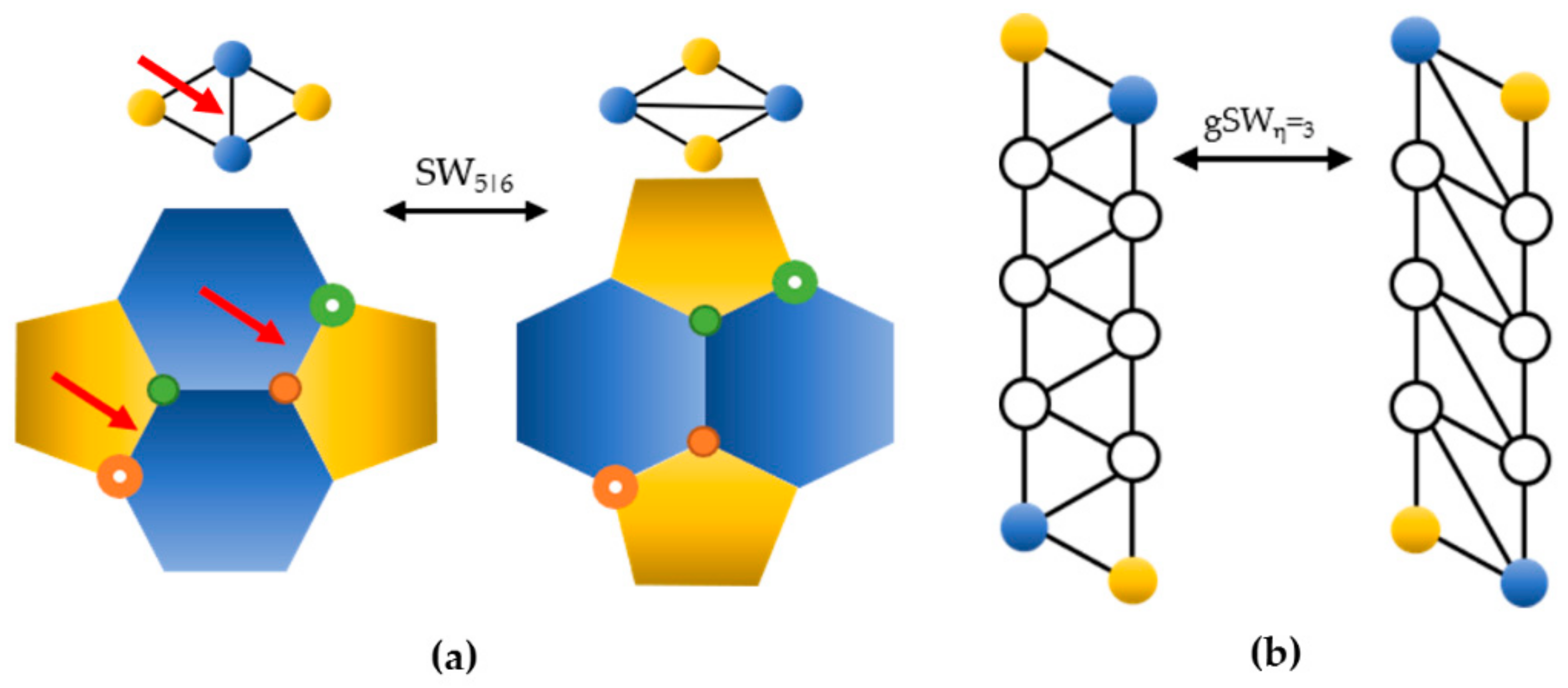
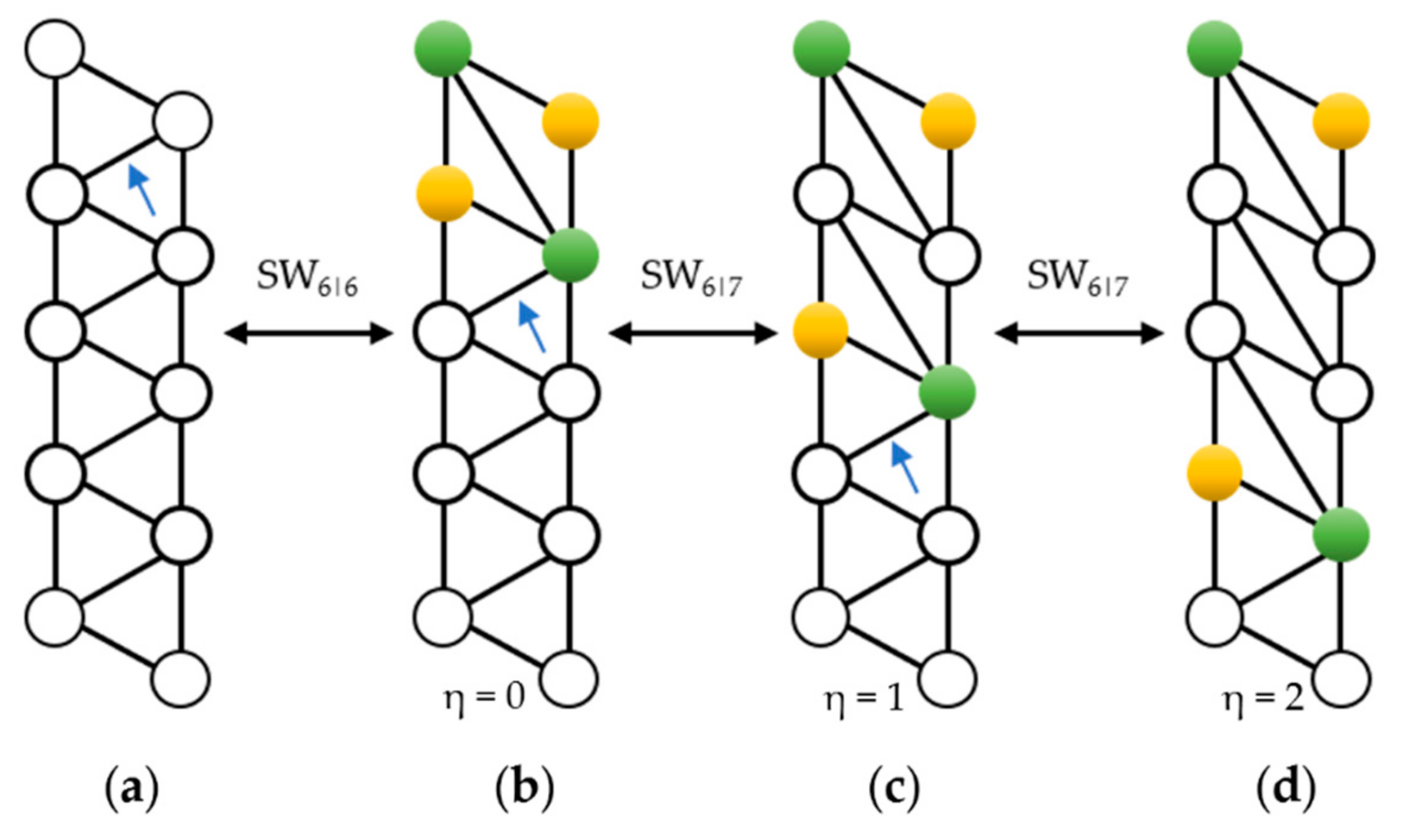
- In the dual space, a SW rotation consists in the reversible rotation of just one dual bond.
- The gSW size η corresponds to the number of pairs of internal faces (white circles) included between the two 5|6 pairs.
- In which ways is the fullerene surface modified by SWw topological defects?
- Is the creation of SWw defects energetically favored?
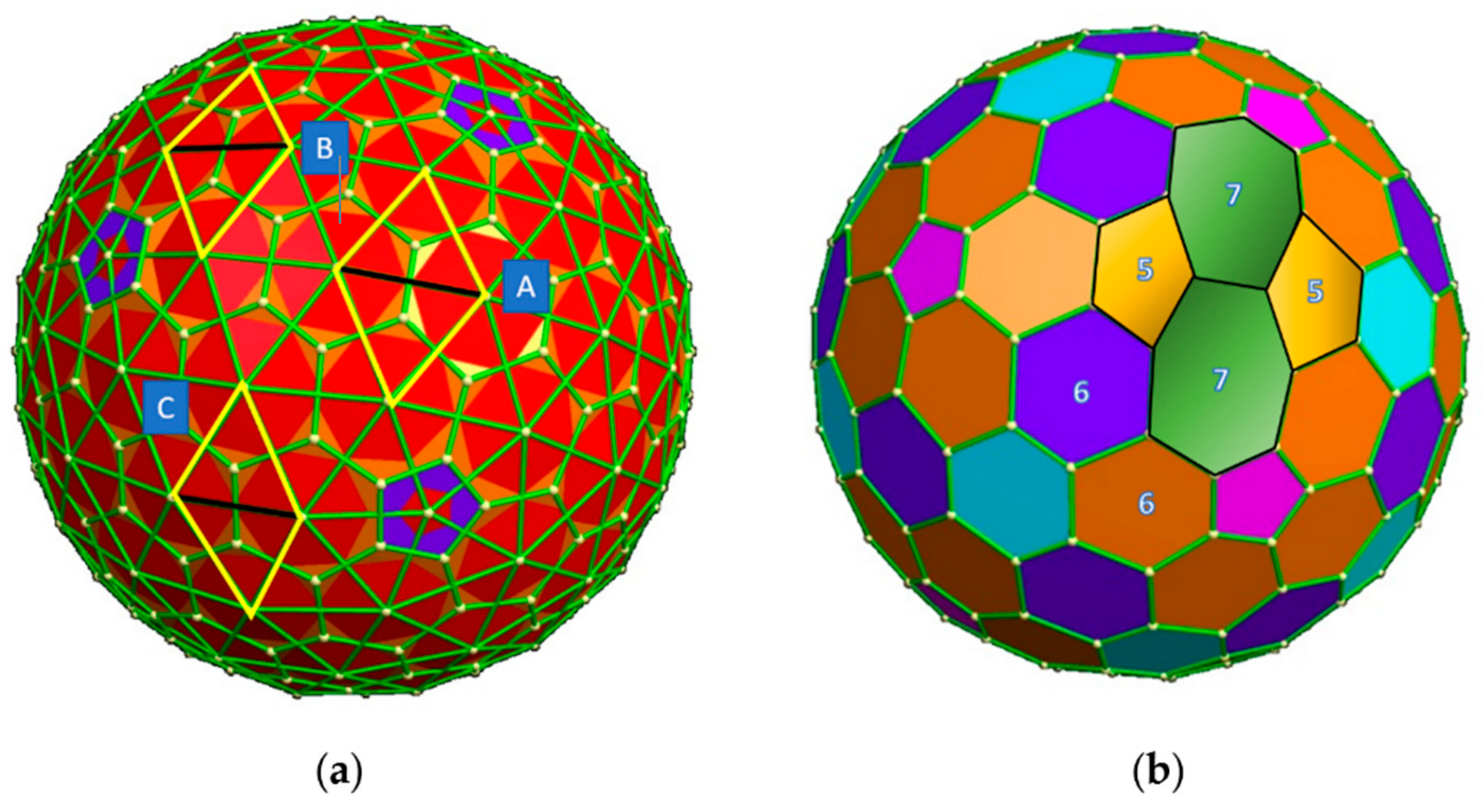
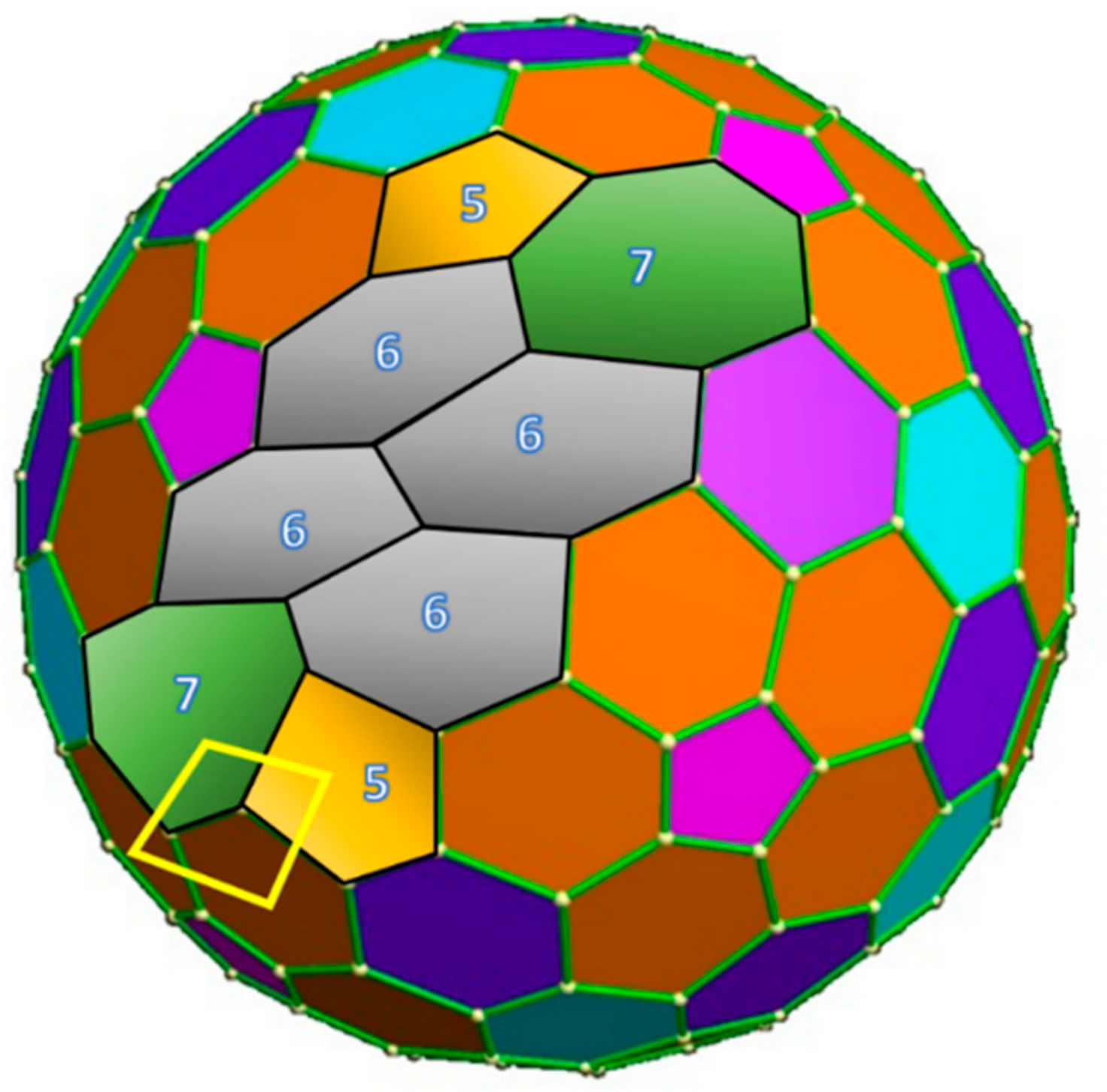
2. SW Waves on the C240 (Ih) Fullerene
3. Topological Simulations and Electronic Structure
3.1. Topological Modeling
3.2. DFT Simulations on Energetic and Molecular Parameters of the Defected C240 Cages
4. Discussion
5. Conclusions and Prospective
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Details of Quantum Chemical and Auxiliary Calculations
References
- Lu, X.; Chen, Z. Curved pi-conjugation, aromaticity, and the related chemistry of small fullerenes (<C60) and single-walled carbon nanotubes. Chem. Rev. 2005, 105, 3643–3696. [Google Scholar] [CrossRef]
- Kovalenko, V.I.; Khamatgalimov, A.R. Regularities in the molecular structures of stable fullerenes. Russ. Chem. Rev. 2006, 75, 981–988. [Google Scholar] [CrossRef]
- Goldberg, M. A class of multi-symmetric polyhedra. Tohoku Math. J. First Ser. 1937, 43, 104–108. [Google Scholar]
- Schewerdtfeger, P.; Wirz, L.N.; Avery, J.E. The topology of fullerenes. WIREs Comput. Mol. Sci. 2015, 5, 96–145. [Google Scholar] [CrossRef]
- Yoshida, M.; Ōsawa, E. Molecular mechanics calculations of giant- and hyperfullerenes with eicosahedral symmetry. Fullerene Sci. Tech. 1992, 1, 55–74. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ōsawa, E. Information entropy of fullerenes. J. Chem. Inf. Model. 2015, 55, 1576–1584. [Google Scholar] [CrossRef]
- Suyetin, M.V.; Vakhrushev, A.V. Guided carbon nanocapsules for hydrogen storage. J. Phys. Chem. C 2011, 115, 5485–5491. [Google Scholar] [CrossRef]
- Zope, R.R. Electronic structure and static dipole polarizability of C60@C240. J. Phys. B: At. Mol. Opt. Phys. 2008, 41, 085101. [Google Scholar] [CrossRef]
- Hirsch, A.; Brettreich, M. Fullerenes: Chemistry and Reactions; John Wiley & Sons: New York, NY, USA, 2006. [Google Scholar]
- Taylor, R. Lecture Notes on Fullerene Chemistry: A Handbook for Chemists; Imperial College Press: London, UK, 1999. [Google Scholar]
- Mordkovich, V.Z.; Umnov, A.G.; Inoshita, T.; Endo, M. The observation of multiwall fullerenes in thermally treated laser pyrolysis carbon blacks. Carbon 1999, 37, 1855–1858. [Google Scholar] [CrossRef]
- Mordkovich, V.Z.; Shiratori, Y.; Hiraoka, H.; Umnov, A.G.; Takeuchi, Y. A path to larger yields of multishell fullerenes. Carbon 2001, 39, 1929–1941. [Google Scholar] [CrossRef]
- Mordkovich, V.Z.; Takeuchi, Y. Multishell fullerenes by laser vaporization of composite carbon–metal targets. Chem. Phys. Lett. 2002, 355, 133–138. [Google Scholar] [CrossRef]
- Mordkovich, V.Z.; Shiratori, Y.; Hiraoka, H.; Takeuchi, Y. Synthesis of multishell fullerenes by laser vaporization of composite carbon targets. Phys. Solid State 2002, 44, 603–606. [Google Scholar] [CrossRef]
- Noël, Y.; De La Pierre, M.; Zicovich-Wilson, C.M.; Orlando, R.; Dovesi, R. Structural, electronic and energetic properties of giant icosahedral fullerenes up to C6000: Insights from an ab initio hybrid DFT study. Phys. Chem. Chem. Phys. 2014, 16, 13390–13404. [Google Scholar] [CrossRef] [PubMed]
- Pankratyev, E.Y.; Khatymov, R.V.; Sabirov, D.S.; Yuldashev, A.V. On the upper bound of the thermodynamic stability of fullerenes from small to giant. Physica E 2018, 101, 265–272. [Google Scholar] [CrossRef]
- Zope, R.R.; Baruah, T.; Pederson, M.R.; Dunlap, B.I. Static dielectric response of icosahedral fullerenes from C60 to C2160 characterized by an all-electron density functional theory. Phys. Rev. B 2008, 77, 115452. [Google Scholar] [CrossRef]
- Langlet, R.; Mayer, A.; Geuquet, N.; Amara, H.; Vandescuren, M.; Henrard, L.; Maksimenko, S.; Lambin, P. Study of the polarizability of fullerenes with a monopole–dipole interaction model. Diamond Relat. Mater. 2007, 16, 2145–2149. [Google Scholar] [CrossRef]
- Calaminici, P.; Carmona-Espindola, J.; Geudtner, G.; Köster, A.M. Static and dynamic polarizability of C540 fullerene. Int. J. Quantum Chem. 2012, 112, 3252–3255. [Google Scholar] [CrossRef]
- Irle, S.; Zheng, G.; Wang, Z.; Morokuma, K. The C60 formation puzzle “solved”: QM/MD simulations reveal the shrinking hot giant road of the dynamic fullerene self-assembly mechanism. J. Phys. Chem. B 2006, 110, 14531–14545. [Google Scholar] [CrossRef]
- Ōsawa, E. Formation mechanism of C60 under nonequilibrium and irreversible conditions—An annotation. Fuller. Nanotub. Carbon Nanostruct. 2012, 20, 299–309. [Google Scholar] [CrossRef]
- Stone, A.J.; Wales, D.J. Theoretical studies of icosahedral C60 and some related species. Chem. Phys. Lett. 1986, 128, 501–503. [Google Scholar] [CrossRef]
- Monthioux, M.; Charlier, J.C. Giving credit where credit is due: The Stone–(Thrower)–Wales designation revisited. Carbon 2014, 75, 1–4. [Google Scholar] [CrossRef]
- Kumeda, Y.; Wales, D.J. Ab initio study of rearrangements between C60 fullerenes. Chem. Phys. Lett. 2003, 374, 125–131. [Google Scholar] [CrossRef]
- Babić, D.; Bassoli, S.; Casartelli, M.; Cataldo, F.; Graovac, A.; Ori, O.; York, B. Generalized Stone–Wales transformations. Mol. Simul. 1995, 14, 395–401. [Google Scholar] [CrossRef]
- Ori, O.; Cataldo, F.; Putz, M.V. Topological anisotropy of Stone–Wales waves in graphenic fragments. Int. J. Mol. Sci. 2011, 12, 7934–7949. [Google Scholar] [CrossRef] [PubMed]
- Ori, O.; Putz, M.V.; Gutman, I.; Schwerdtfeger, P. Generalized Stone–Wales transformations for fullerene graphs derived from Berge’s switching theorem. In Ante Graovac—Life and Works; Gutman, I., Pokrić, B., Vukičević, D., Eds.; University of Kragujevac: Kragujevac, Serbiba, 2014; pp. 259–272. [Google Scholar]
- Liu, Y.; Yakobson, B.I. Cones, pringles, and grain boundary landscapes in graphene topology. Nano Lett. 2010, 10, 2178–2183. [Google Scholar] [CrossRef] [PubMed]
- Carpio, A.; Bonilla, L.L.; de Juan, F.; Vozmediano, M.A.H. Dislocations in graphene. New J. Phys. 2008, 10, 053021. [Google Scholar] [CrossRef]
- Zhoua, L.G.; Shib, S.Q. Formation energy of Stone–Wales defects in carbon nanotubes. Appl. Phys. Lett. 2003, 83, 1222–1224. [Google Scholar] [CrossRef]
- Collins, P.G. Defects and disorder in carbon nanotubes. In Oxford Handbook of Nanoscience and Technology: Frontiers and Advances; Narlikar, A.V., Fu, Y.Y., Eds.; Oxford University Press: Oxford, UK, 2011; Volume 2. [Google Scholar] [CrossRef]
- Ewels, C.P.; Heggie, M.I.; Briddon, P.R. Adatoms and nanoengineering of carbon. Chem. Phys. Lett. 2002, 351, 178–182. [Google Scholar] [CrossRef]
- Samsonidze, G.G.; Samsonidze, G.G.; Yakobson, B.I. Energetics of Stone–Wales defects in deformations of monoatomic hexagonal layers. Comput. Mater. Sci. 2002, 23, 62–72. [Google Scholar] [CrossRef]
- Nordlund, K.; Keinonen, J.; Mattila, T. Formation of ion irradiation induced small-scale defects on graphite surfaces. Phys. Rev. Lett. 1996, 77, 699–702. [Google Scholar] [CrossRef]
- Krasheninnikov, A.V.; Nordlund, K.; Sirviö, M.; Salonen, E.; Keinonen, J. Formation of ion-irradiation-induced atomic-scale defects on walls of carbon nanotubes. Phys. Rev. B 2001, 63, 245405. [Google Scholar] [CrossRef]
- Hashimoto, A.; Suenaga, K.; Gloter, A.; Urita, K.; Iijima, S. Direct evidence for atomic defects in graphene layers. Nature 2004, 430, 870–873. [Google Scholar] [CrossRef]
- Kotakoski, J.; Krasheninnikov, A.V.; Kaiser, U.; Meyer, J.C. From point defects in graphene to two-dimensional amorphous carbon. Phys. Rev. Lett. 2011, 106, 105505. [Google Scholar] [CrossRef] [PubMed]
- RobertLovesPi: Polyhedra, tessellations, and more. Available online: https://robertlovespi.net/c240-fullerene-1/ (accessed on 27 April 2020).
- Pyshnov, M.B. Topological solution for cell proliferation in intestinal crypt. I. Elastic growth without cell loss. J. Theor. Biol. 1980, 87, 189–200. [Google Scholar] [CrossRef]
- Branden, C.I.; Tooze, J. Introduction to Protein Structure, 2nd ed.; Garland Science: New York, NY, USA, 1999; pp. 1–302. [Google Scholar]
- Twarock, R. Mathematical virology: A novel approach to the structure and assembly of viruses. Philos. Trans. R. Soc. A 2006, 364, 335–3373. [Google Scholar] [CrossRef]
- Dechant, P.-P.; Wardman, J.; Keef, T.; Twarock, R. Viruses and fullerenes – symmetry as a common thread? Acta Crystallogr. A 2014, 70, 162–167. [Google Scholar] [CrossRef]
- Iranmanesh, A.; Ashrafi, A.R.; Graovac, A.; Cataldo, F.; Ori, O. Wiener index role in topological modeling of hexagonal systems-from fullerenes to graphene. In Distance in Molecular Graphs – Applications; Gutman, I., Furtula, B., Eds.; University of Kragujevac: Kragujevac, Serbia, 2012; pp. 135–155. [Google Scholar]
- Ori, O.; D’Mello, M. A topological study of the structure of the C76 fullerene. Chem. Phys. Lett. 1992, 197, 49–54. [Google Scholar] [CrossRef]
- Babić, D.; Klein, D.J.; Sah, C.H. Symmetry of fullerenes. Chem. Phys. Lett. 1993, 211, 235–241. [Google Scholar] [CrossRef]
- Vukicevic, D.; Cataldo, F.; Ori, O.; Graovac, A. Topological efficiency of C66 fullerene. Chem. Phys. Lett. 2011, 501, 442–445. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ori, O.; László, I. Isomers of the C84 fullerene: A theoretical consideration within energetic, structural, and topological approaches. Fuller. Nanotub. Carbon Nanostruct. 2018, 26, 100–110. [Google Scholar] [CrossRef]
- Dobrynin, A.A.; Ori, O.; Putz, M.V.; Vesnin, A.Y. Generalized topological efficiency – case study with C84 fullerene. Fuller. Nanotub. Carbon Nanostruct. 2020, 28, 545–550. [Google Scholar] [CrossRef]
- Chen, Q.; Robertson, A.W.; He, K.; Gong, C.; Yoon, E.; Lee, G.D.; Warner, J.H. Atomic level distributed strain within graphene divacancies from bond rotations. ACS Nano 2015, 9, 8599–8608. [Google Scholar] [CrossRef] [PubMed]
- Haddon, R.C. Chemistry of the fullerenes: The manifestation of strain in a class of continuous aromatic molecules. Science 1993, 261, 1545–1550. [Google Scholar] [CrossRef] [PubMed]
- Astakhova, T.Y.; Vinogradov, G.A.; Gurin, O.D.; Menon, M. Effect of local strain on the reactivity of carbon nanotubes. Russ. Chem. Bull. 2002, 51, 764–769. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Bulgakov, R.G.; Khursan, S.L. Indices of the fullerene reactivity. ARKIVOC 2011, 2011, 200–224. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Bulgakov, R.G. Reactivity of fullerene derivatives C60O and C60F18 (C3v) in terms of local curvature and polarizability. Fuller. Nanotub. Carbon Nanostruct. 2010, 18, 455–457. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Garipova, R.R. The increase in the fullerene cage volume upon its chemical functionalization. Fuller. Nanotub. Carbon Nanostruct. 2019, 27, 702–709. [Google Scholar] [CrossRef]
- Fowler, P.W.; Manolopoulos, D.E. An Atlas of Fullerenes; Dover Publications Inc.: Mineola, NY, USA, 1995; pp. 1–392. [Google Scholar]
- Sure, R.; Hansen, A.; Schwerdtfeger, P.; Grimme, S. Comprehensive theoretical study of all 1812 C60 isomers. Phys. Chem. Chem. Phys. 2017, 19, 14296–14305. [Google Scholar] [CrossRef]
- Klyavlina, A.I.; Rysaeva, L.K.; Murzaev, R.T. Dislocation dipole in graphene at finite temperatures. J. Phys. Conf. Ser. 2020, 1435, 012063. [Google Scholar] [CrossRef]
- Sabirov, D.S. Rules of fullerene polarizability. Fuller. Nanotub. Carbon Nanostruct. 2020, 28, 71–77. [Google Scholar] [CrossRef]
- Plonska-Brzezinska, M.E. Carbon nano-onions: A review of recent progress in synthesis and applications. ChemNanoMat. 2019, 5, 568–580. [Google Scholar] [CrossRef]
- Bartkowski, M.; Giordani, S. Supramolecular chemistry of carbon nano-onions. Nanoscale 2020, 12, 9352–9358. [Google Scholar] [CrossRef] [PubMed]
- Laikov, D.N.; Ustynyuk, Y.A. PRIRODA-04: A quantum-chemical program suite. New possibilities in the study of molecular systems with the application of parallel computing. Russ. Chem. Bull. 2005, 54, 820–826. [Google Scholar] [CrossRef]
- Shestakov, A.F. Reactivity of fullerene C60. Russ. J. Gen. Chem. 2008, 78, 811–821. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Khursan, S.L.; Bulgakov, R.G. 1,3-Dipolar addition reactions to fullerenes: The role of the local curvature of carbon surface. Russ. Chem. Bull. 2008, 57, 2520–2525. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Zakirova, A.D.; Tukhbatullina, A.A.; Gubaydullin, I.M.; Bulgakov, R.G. Influence of the charge on the volumes of nanoscale cages (carbon and boron-nitride fullerenes, Ge9z− Zintl ions, and cubic Fe4S4 clusters). RSC Adv. 2013, 3, 1818–1824. [Google Scholar] [CrossRef]
- Tulyabaev, A.R.; Kiryanov, I.I.; Samigullin, I.S.; Khalilov, L.M. Are there reliable DFT approaches for 13C NMR chemical shift predictions of fullerene C60 derivatives? Int. J. Quant. Chem. 2017, 117, 7–14. [Google Scholar] [CrossRef]
- Pankratyev, E.Y.; Tulyabaev, A.R.; Khalilov, L.M. How reliable are GIAO calculations of 1H and 13C NMR chemical shifts? A statistical analysis and empirical corrections at DFT (PBE/3z) level? J. Comput. Chem. 2011, 32, 1993–1997. [Google Scholar] [CrossRef] [PubMed]
- Sabirov, D.S.; Kinzyabaeva, Z.S. Sonochemical synthesis of novel C60 fullerene 1,4-oxathiane derivative through the intermediate fullerene radical anion. Ultrason. Sonochem. 2020, 67, 105169. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Garipova, R.R.; Cataldo, F. Polarizability of isomeric and related interstellar compounds in the aspect of their abundance. Mol. Astrophys. 2018, 12, 10–12. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Terentyev, A.O.; Shepelevich, I.S.; Bulgakov, R.G. Inverted thermochemistry of “norbornadiene–quadricyclane” molecular system inside fullerene nanocages. Comput. Theor. Chem. 2014, 1045, 86–92. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Tukhbatullina, A.A.; Bulgakov, R.G. Compression of methane endofullerene CH4@C60 as a potential route to endohedral covalent fullerene derivatives: A DFT study. Fuller. Nanotub. Carbon Nanostruct. 2015, 23, 835–842. [Google Scholar] [CrossRef]
- Zakirova, A.D.; Sabirov, D.S. Volume of the fullerene cages of endofullerenes and hydrogenated endofullerenes with encapsulated atoms of noble gases and nonadditivity of their polarizability. Russ. J. Phys. Chem. A 2020, 94, 963–971. [Google Scholar] [CrossRef]
- Shestakov, A.F. Role of fullerene–nitrogen complexes of alkali metals in C60-catalyzed nitrogen fixation. Russ. J. Phys. Chem. A 2020, 94, 919–924. [Google Scholar] [CrossRef]
- Pimenova, A.S.; Kozlov, A.A.; Goryunkov, A.A.; Markov, V.Y.; Khaverl, P.A.; Avdoshenko, S.M.; Vorobiev, V.A.; Ioffe, I.N.; Sakharov, S.G.; Troyanov, S.I.; et al. Preparation and structures of [6,6]-open difluoromethylene [60]fullerenes: C60(CF2) and C60(CF2)2. Dalton Trans. 2007, 5322–5328. [Google Scholar] [CrossRef]
- Ignat’eva, D.V.; Goryunkov, A.A.; Tamm, N.B.; Ioffe, I.N.; Sidorov, L.N.; Troyanov, S.I. Isolation and structural characterization of the most highly trifluoromethylated C70 fullerenes: C70(CF3)18 and C70(CF3)20. New J. Chem. 2013, 37, 299–302. [Google Scholar] [CrossRef]
- Lukonina, N.S.; Semivrazhskaya, O.O.; Apenova, M.G.; Belov, N.M.; Troyanov, S.I.; Goryunkov, A.A. CF2-functionalized trifluoromethylated fullerene C70(CF3)8(CF2): Structure, electronic properties, and spontaneous oxidation at the bridgehead carbon atoms. Asian J. Org. Chem. 2019, 8, 1924–1932. [Google Scholar] [CrossRef]
- Diniakhmetova, D.R.; Friesen, A.K.; Kolesov, S.V. Quantum chemical analysis of the mechanism of the participation of C60 fullerene in the radical polymerization of styrene and mma initiated by benzoyl peroxide or azobisisobutyronitrile. Russ. J. Phys. Chem. B 2017, 11, 492–498. [Google Scholar] [CrossRef]
- Diniakhmetova, D.R.; Friesen, A.K.; Kolesov, S.V. Quantum chemical modeling of the addition reactions of 1-n-phenylpropyl radicals to C60 fullerene. Int. J. Quant. Chem. 2016, 116, 489–496. [Google Scholar] [CrossRef]
- Diniakhmetova, D.R.; Friesen, A.K.; Yumagulova, R.K.; Kolesov, S.V. Simulation of potentially possible reactions at the initial stages of free-radical polymerization of styrene and methyl methacrylate in the presence of fullerene C60. Polymer Sci. B 2018, 60, 414–420. [Google Scholar] [CrossRef]
- Khatymov, R.V.; Muftakhov, M.V.; Tuktarov, R.F.; Raitman, O.A.; Shokurov, A.V.; Pankratyev, E.Y. Fragmentation and slow autoneutralization of isolated negative molecular ions of phthalocyanine and tetraphenylporphyrin. J. Chem. Phys. 2019, 150, 134301. [Google Scholar] [CrossRef] [PubMed]
- Khatymov, R.V.; Shchukin, P.V.; Muftakhov, M.V.; Yakushchenko, I.K.; Yarmolenko, O.V.; Pankratyev, E.Y. A unified statistical RRKM approach to the fragmentation and autoneutralization of metastable molecular negative ions of hexaazatrinaphthylenes. Phys. Chem. Chem. Phys. 2020, 22, 3073–3088. [Google Scholar] [CrossRef] [PubMed]
- Kuznetsov, V.V. Stereochemistry of simple molecules inside nanotubes and fullerenes: Unusual behavior of usual systems. Molecules 2020, 25, 2437. [Google Scholar] [CrossRef] [PubMed]
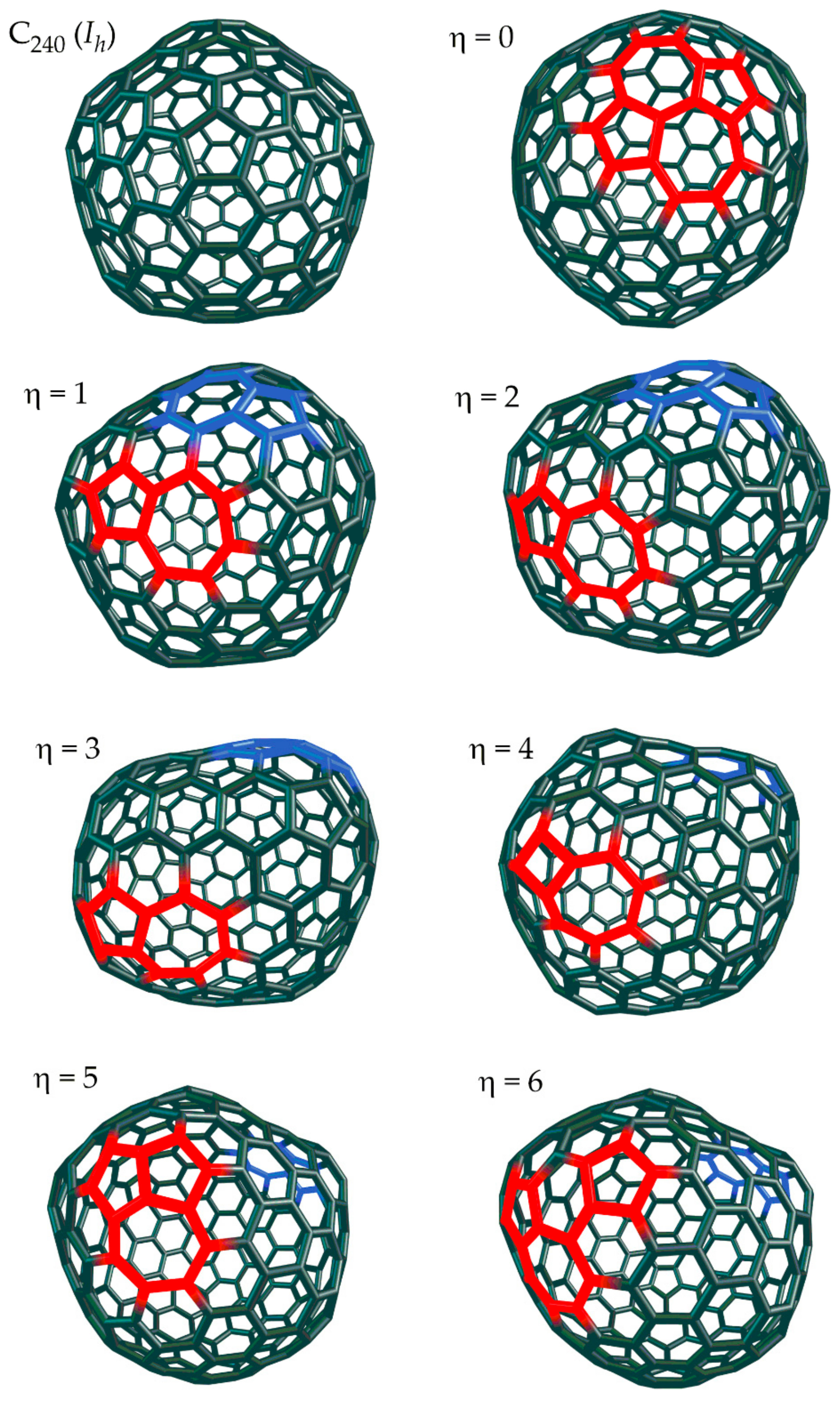
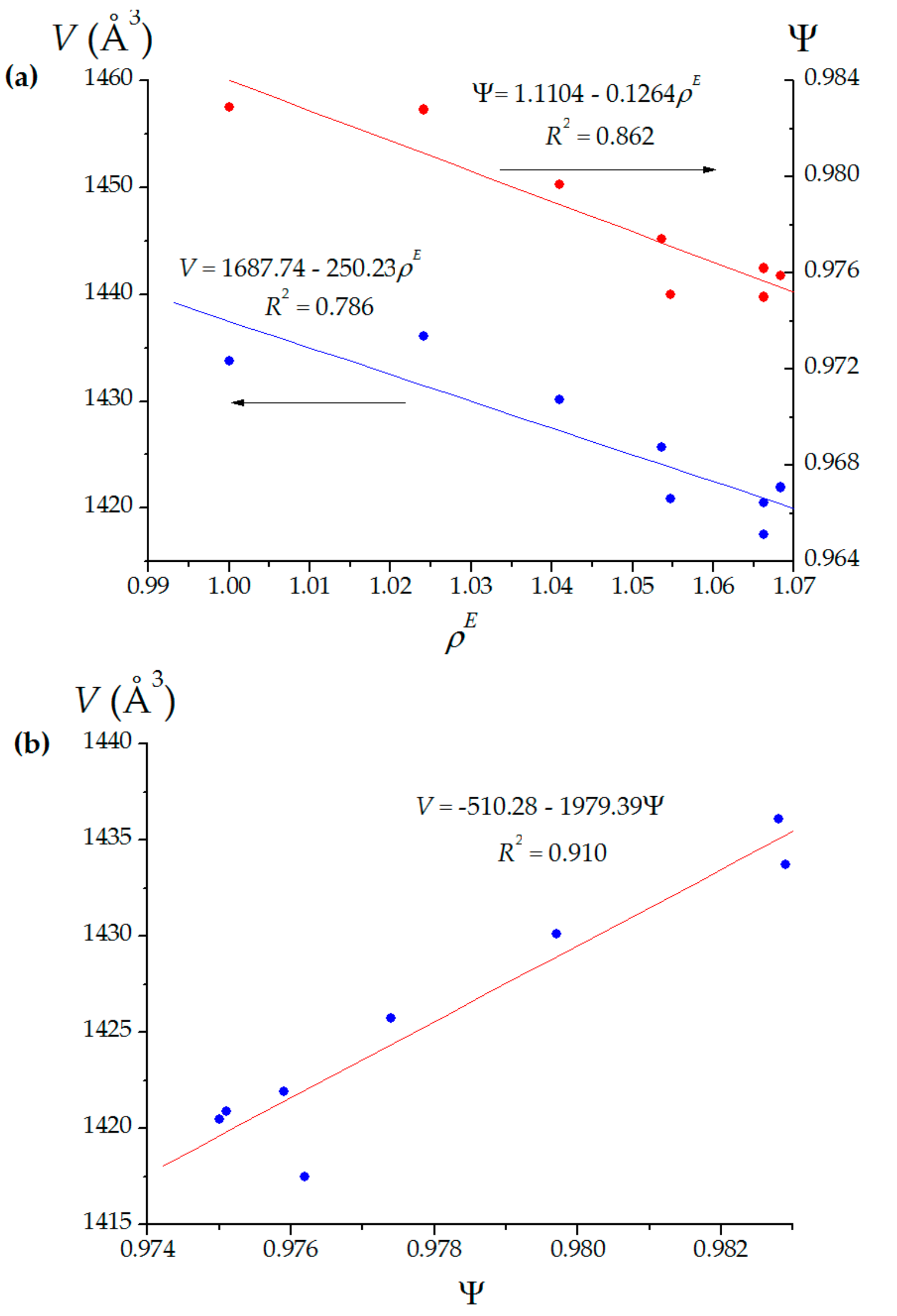
| Fullerene Cage | Number of Polygons 1 | Volume (Å 3) | Sphericity, Ψ | Curvatures of Original Pentagons (Å–1) | Curvatures of Defected Pentagons (Å–1) | |
|---|---|---|---|---|---|---|
| Static | Migrating | |||||
| C240 (Ih) | 12 110 0 0 | 1433.73 | 0.9829 | 0.2365 | none | none |
| 14 106 2 0 | 1436.10 | 0.9828 | 0.1850–0.2475 | 0.1641 | 0.1257 | |
| 14 106 2 0 | 1430.11 | 0.9797 | 0.1763–0.2586 | 0.2112 | 0.1537 | |
| 14 106 2 0 | 1425.73 | 0.9774 | 0.1763–0.2502 | 0.2171 | 0.1915 | |
| 14 106 2 0 | 1420.88 | 0.9751 | 0.1763–0.2680 | 0.2151 | 0.2722 | |
| 12 107 2 1 | 1417.49 | 0.9762 | 0.1754–0.3277 | 0.2135 | 0.3932 2 (tetragon) | |
| 14 106 2 0 | 1420.49 | 0.9750 | 0.1765–0.2589 | 0.2140 (0.2679) | 0.2754 | |
| 14 106 2 0 | 1421.94 | 0.9759 | 0.1578–0.2588 | 0.2140 (0.2383) | 0.1939 | |
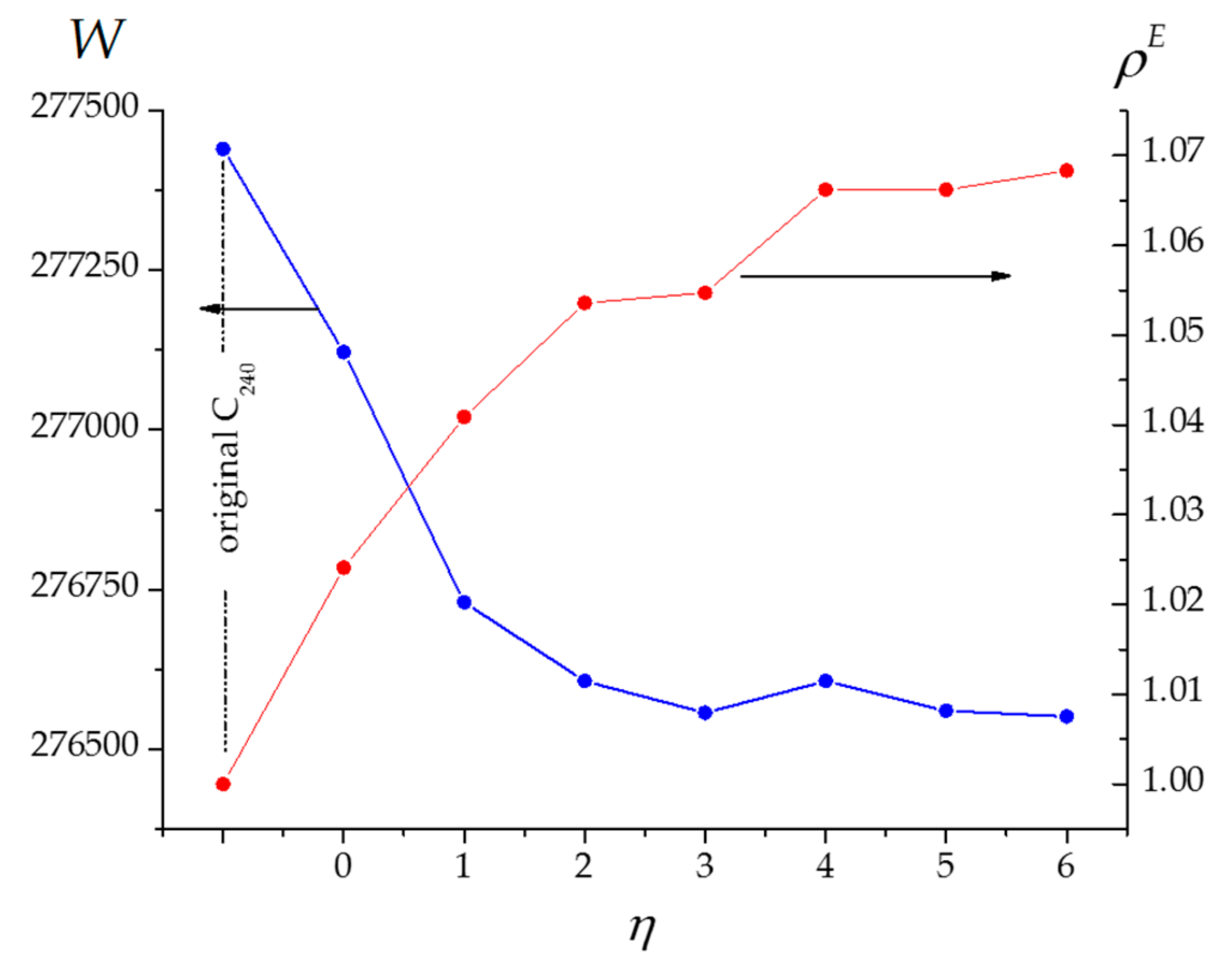
| Fullerene Cage | W | M 1 | |||
|---|---|---|---|---|---|
| C240 (Ih) | 277,440 | 19 | 1156 | 1156 | 1 |
| 277,122 | 19 | 1140 | 1167.5 | 1.0241 | |
| 276,730 | 19 | 1125.5 | 1171.5 | 1.0409 | |
| 276,607 | 19 | 1118.5 | 1178.5 | 1.0536 | |
| 276,557 | 19 | 1124 | 1185.5 | 1.0547 | |
| 276,607 | 19 | 1118.5 | 1192.5 | 1.0662 | |
| 276,560 | 19 | 1125 | 1199.5 | 1.0662 | |
| 276,551 | 19 | 1120 | 1196.5 | 1.0683 |
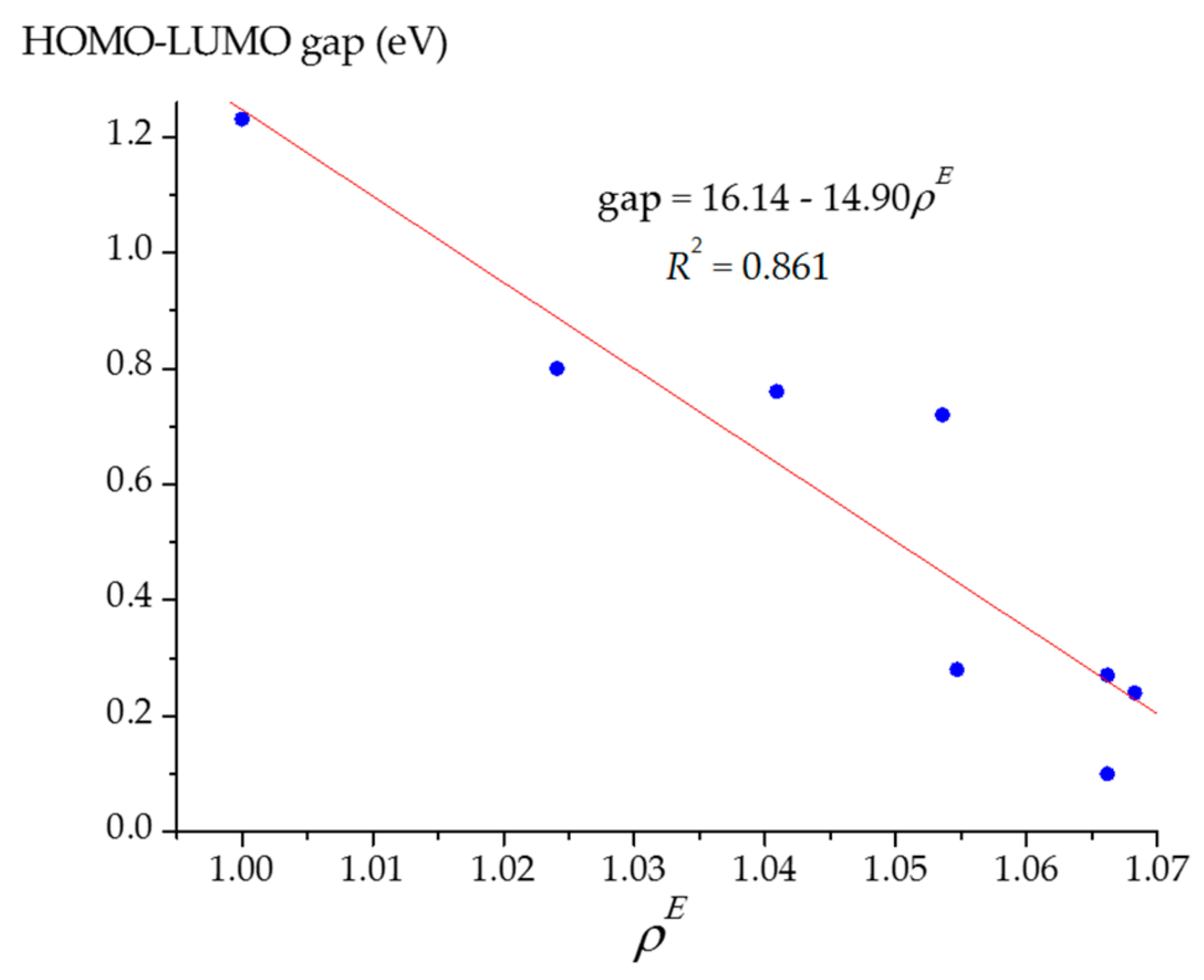
| Fullerene Cage | Relative Energy, Erel (kJ/mol) 1 | Energy Effect, ΔE (kJ/mol) 2 | Dipole Moment, μ (D) | εHOMO (eV) | εLUMO (eV) | HOMO–LUMO Gap (eV) |
|---|---|---|---|---|---|---|
| C240 (Ih) | 0 | n/a | 0 | –5.53 | –4.30 | 1.23 |
| 147.8 | 147.8 | 0.86 | –5.26 | –4.46 | 0.80 | |
| 315.5 | 167.7 | 0.51 | –5.18 | –4.41 | 0.76 | |
| 430.4 | 114.9 | 1.57 | –5.30 | –4.57 | 0.72 | |
| 510.5 | 80.1 | 2.53 | –4.98 | –4.70 | 0.28 | |
| 642.6 | 132.1 | 0.83 | –4.89 | –4.61 | 0.27 | |
| 489.2 | –153.5 | 4.62 | –4.94 | –4.83 | 0.10 | |
| 384.0 | –105.2 | 3.57 | –5.03 | –4.79 | 0.24 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sabirov, D.S.; Ori, O. Skeletal Rearrangements of the C240 Fullerene: Efficient Topological Descriptors for Monitoring Stone–Wales Transformations. Mathematics 2020, 8, 968. https://doi.org/10.3390/math8060968
Sabirov DS, Ori O. Skeletal Rearrangements of the C240 Fullerene: Efficient Topological Descriptors for Monitoring Stone–Wales Transformations. Mathematics. 2020; 8(6):968. https://doi.org/10.3390/math8060968
Chicago/Turabian StyleSabirov, Denis Sh., and Ottorino Ori. 2020. "Skeletal Rearrangements of the C240 Fullerene: Efficient Topological Descriptors for Monitoring Stone–Wales Transformations" Mathematics 8, no. 6: 968. https://doi.org/10.3390/math8060968
APA StyleSabirov, D. S., & Ori, O. (2020). Skeletal Rearrangements of the C240 Fullerene: Efficient Topological Descriptors for Monitoring Stone–Wales Transformations. Mathematics, 8(6), 968. https://doi.org/10.3390/math8060968







