A New Wavelet Tool to Quantify Non-Periodicity of Non-Stationary Economic Time Series
Abstract
1. Introduction
2. The Scale Index Revisited
2.1. Basic Concepts of Wavelets
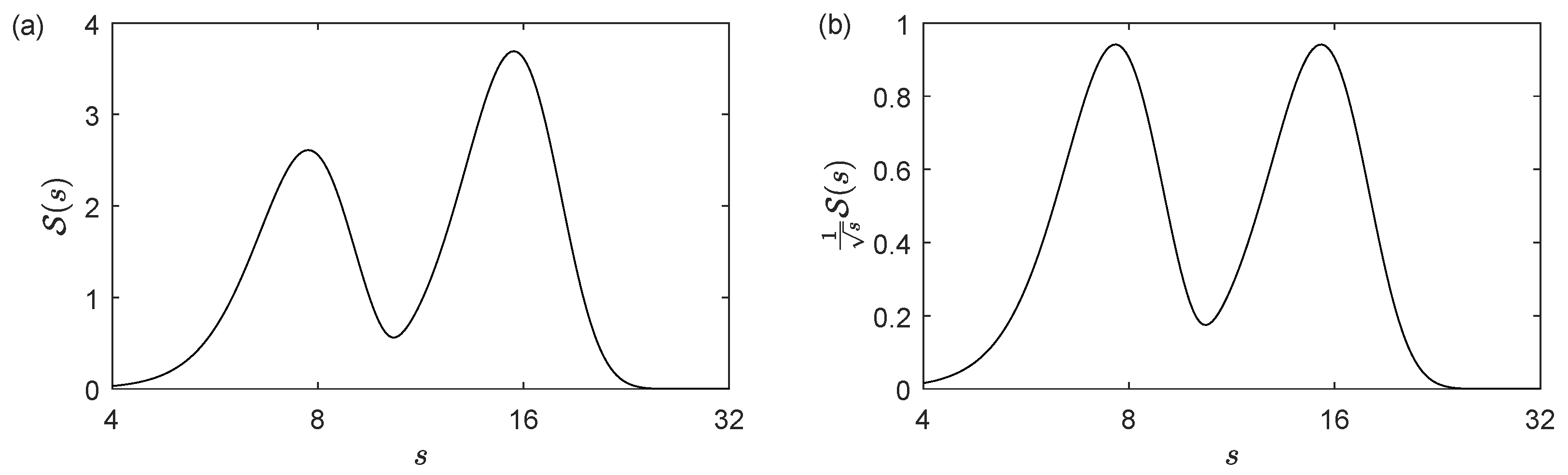
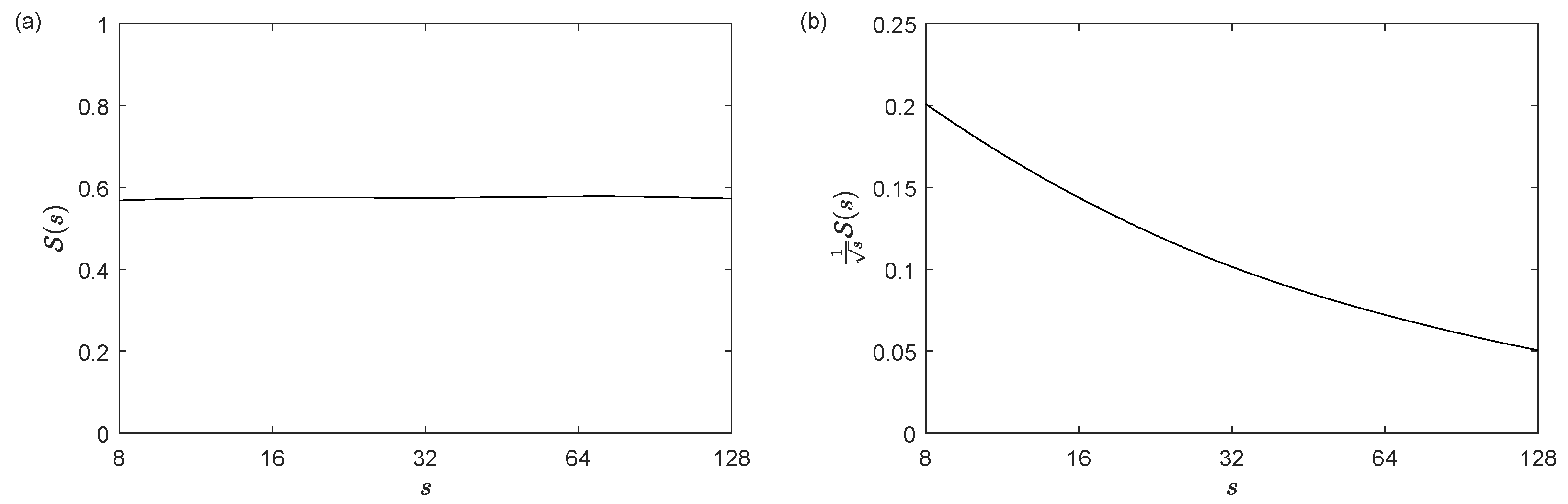
2.2. The Scale Index
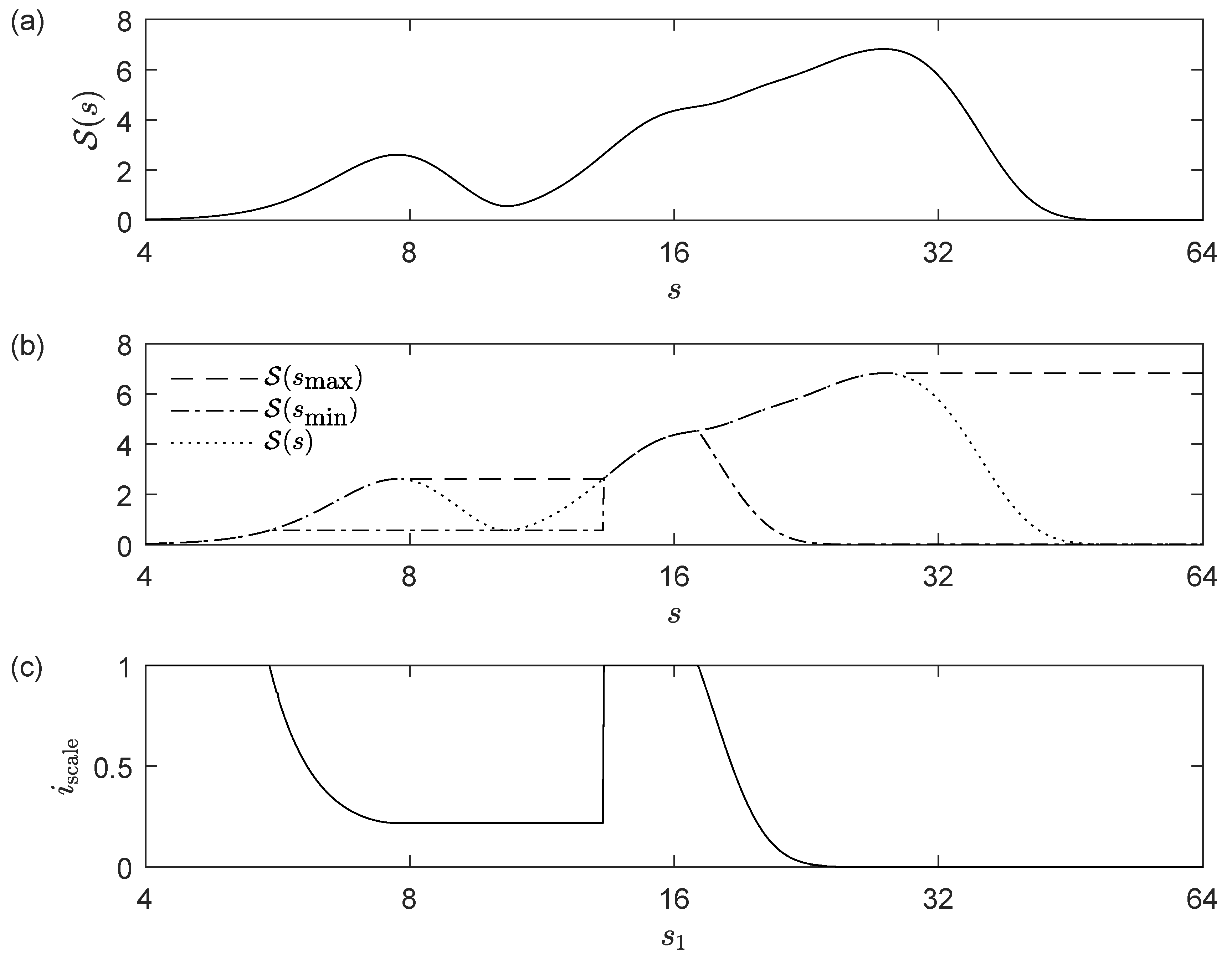
3. The Windowed Scale Index
4. Examples and Applications
4.1. The Bonhoeffer-van der Pol Oscillator
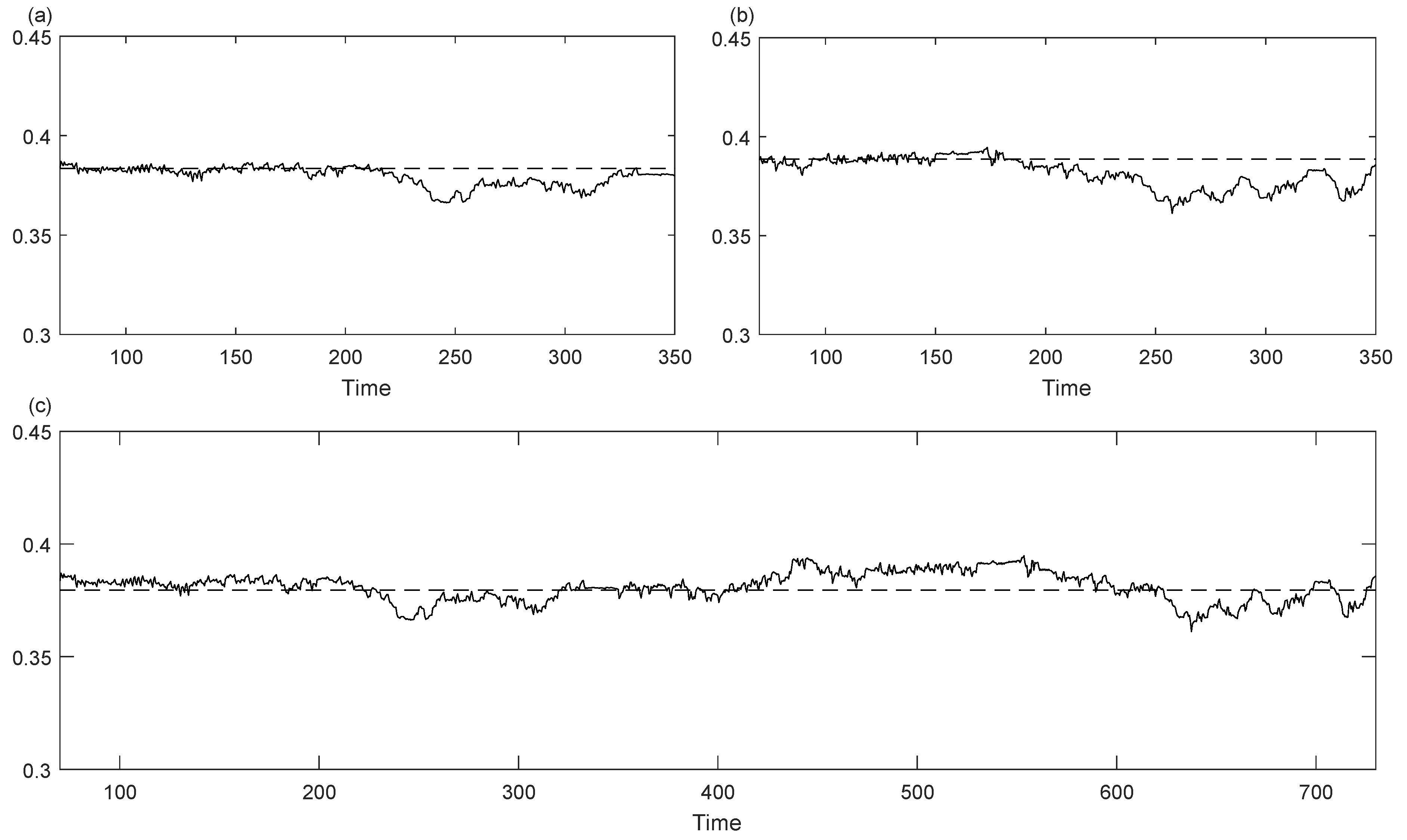
4.2. A Signal with Increasing Noise
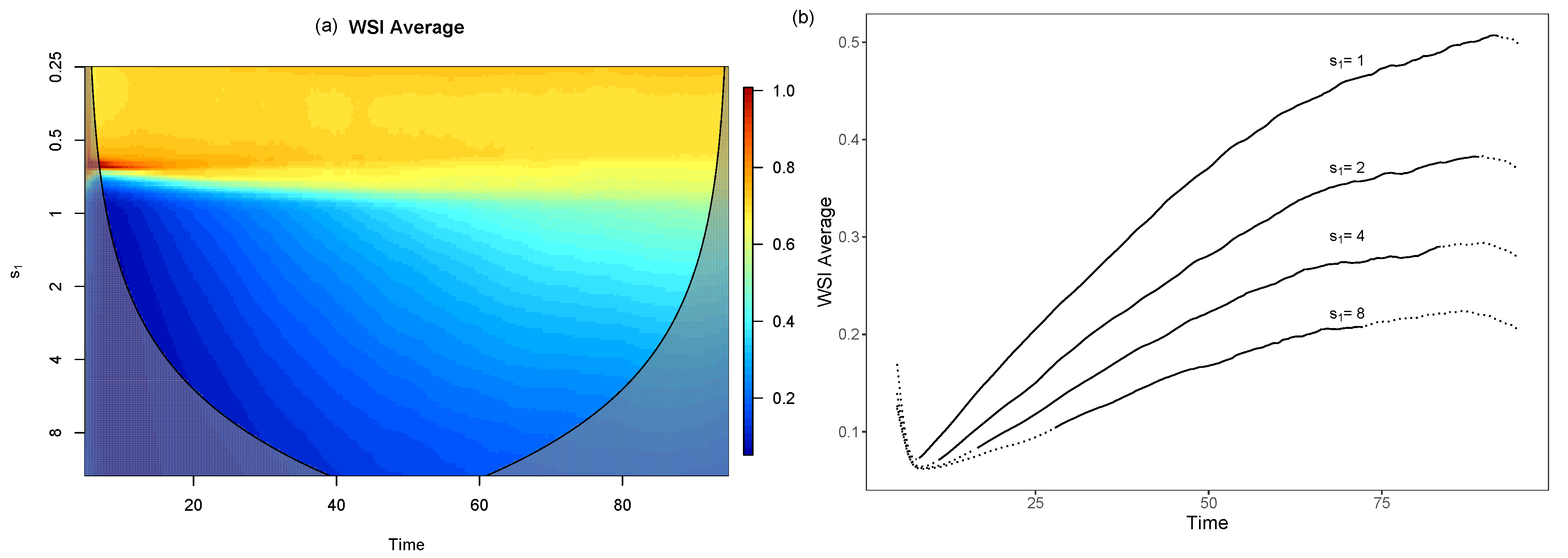
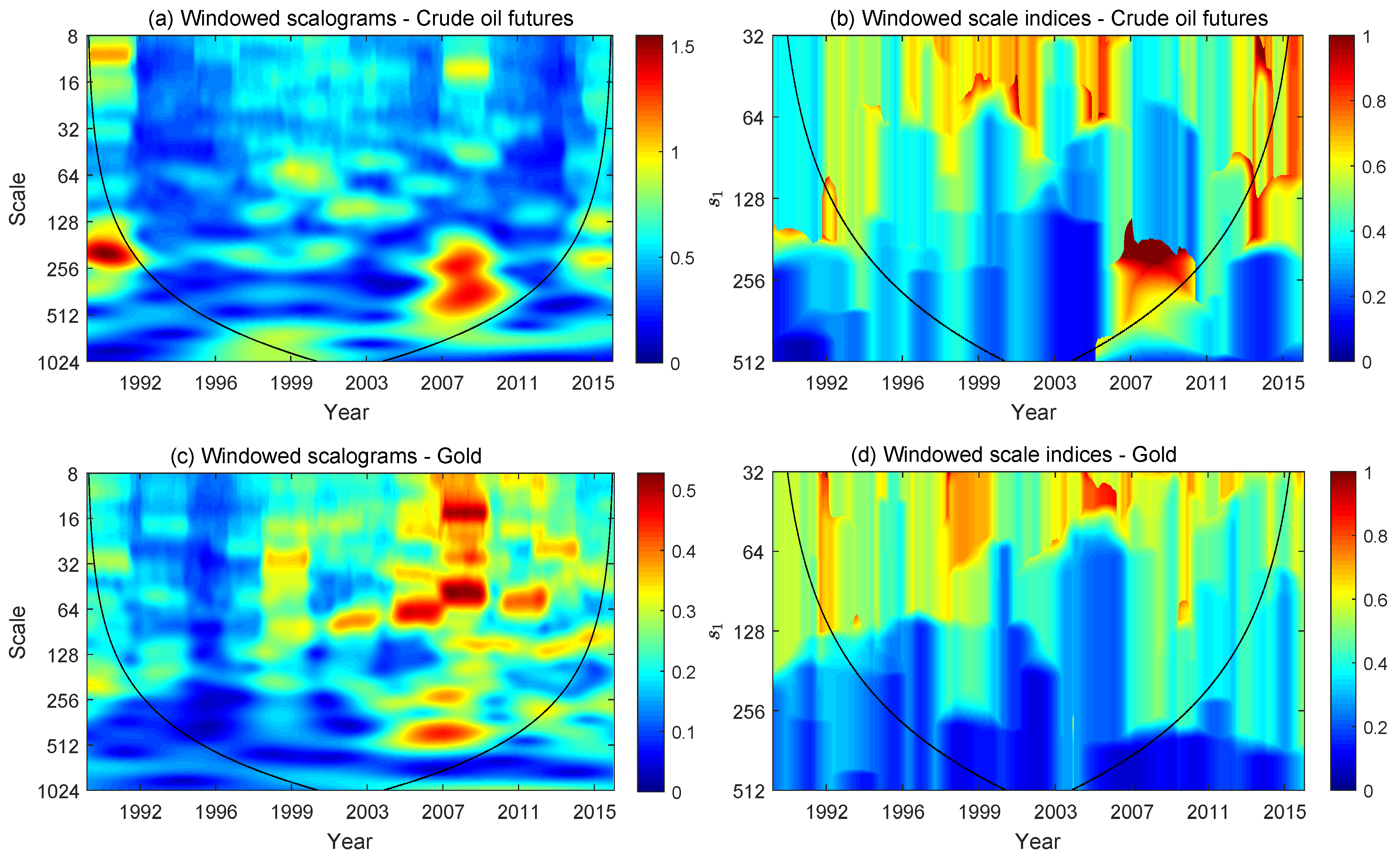
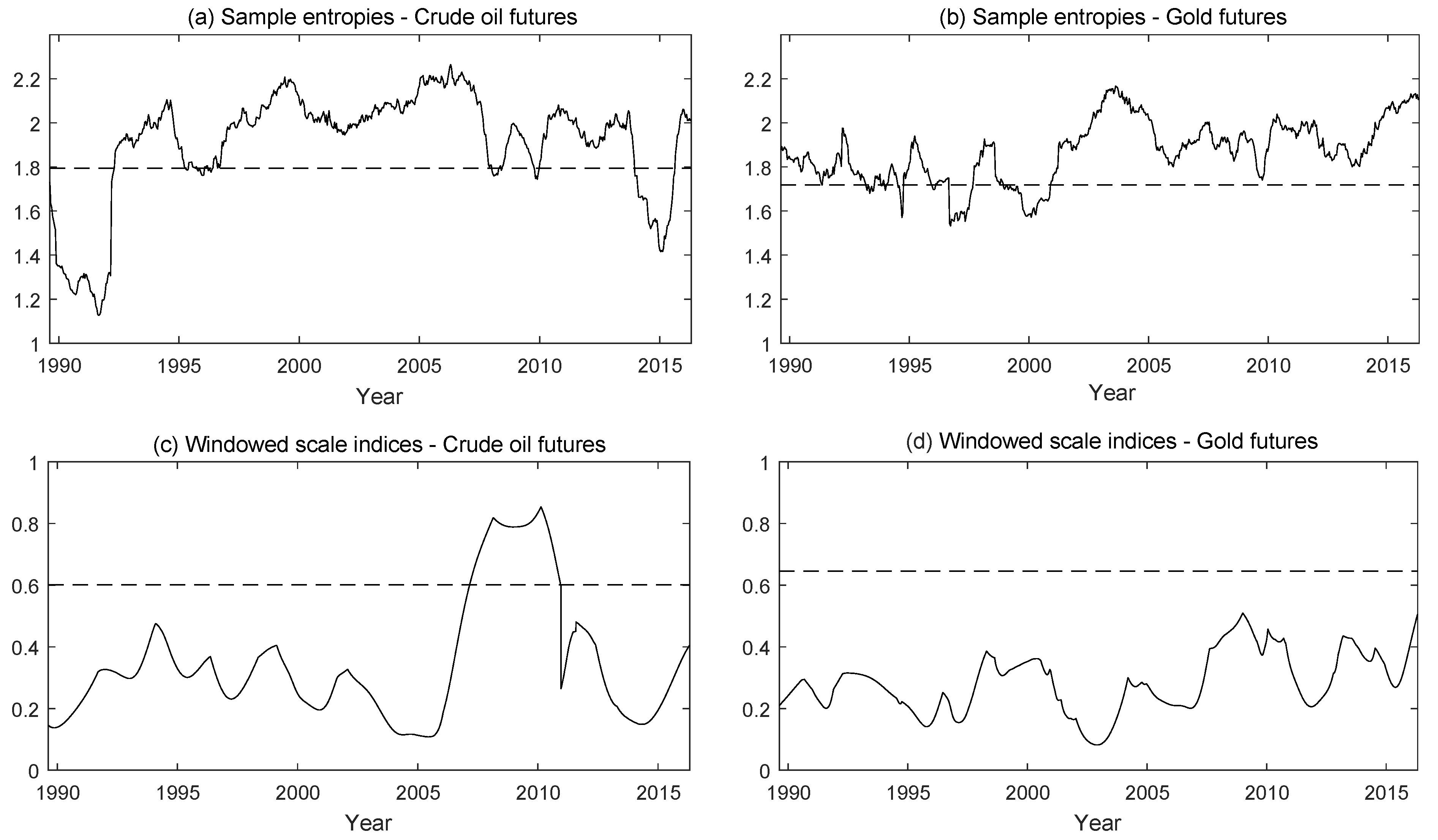
4.3. An Economic Application: Crude Oil and Gold Prices
4.4. Non-Periodicity and Unpredictability
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Pincus, S.M.; Gladstone, I.M.; Ehrenkranz, R.A. A regularity statistic for medical data analysis. J. Clin. Monit. 1991, 7, 335–345. [Google Scholar] [CrossRef] [PubMed]
- Richman, J.S.; Moorman, J.R. Physiological time-series analysis using approximate entropy and sample entropy. Am. J. Physiol.-Heart Circ. Physiol. 2000, 278, H2039–H2049. [Google Scholar] [CrossRef] [PubMed]
- Bandt, C.; Pompe, B. Permutation Entropy: A Natural Complexity Measure for Time Series. Phys. Rev. Lett. 2002, 88, 174102. [Google Scholar] [CrossRef] [PubMed]
- Henry, M.; Judge, G. Permutation Entropy and Information Recovery in Nonlinear Dynamic Economic Time Series. Econometrics 2019, 7, 10. [Google Scholar] [CrossRef]
- Benítez, R.; Bolós, V.J.; Ramírez, M.E. A wavelet-based tool for studying non-periodicity. Comput. Math. Appl. 2010, 60, 634–641. [Google Scholar] [CrossRef]
- Hesham, M. Wavelet-scalogram based study of non-periodicity in speech signals as a complementary measure of chaotic content. Int. J. Speech Technol. 2013, 16, 353–361. [Google Scholar] [CrossRef]
- Akhshani, A.; Akhavan, A.; Mobaraki, A.; Lim, S.C.; Hassan, Z. Pseudo random number generator based on quantum chaotic map. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 101–111. [Google Scholar] [CrossRef]
- Avaroğlu, E.; Tuncer, T.; Özer, A.B.; Ergen, B.; Türk, M. A novel chaos-based post-processing for TRNG. Nonlinear Dyn. 2015, 81, 189–199. [Google Scholar] [CrossRef]
- Yang, Y.G.; Xu, P.; Yang, R.; Zhou, Y.H.; Shi, W.M. Quantum Hash function and its application to privacy amplification in quantum key distribution, pseudo-random number generation and image encryption. Sci. Rep. 2016, 6, srep19788. [Google Scholar] [CrossRef]
- Yang, Y.G.; Zhao, Q.Q. Novel pseudo-random number generator based on quantum random walks. Sci. Rep. 2016, 6, srep20362. [Google Scholar] [CrossRef]
- Tuncer, T. The implementation of chaos-based PUF designs in field programmable gate array. Nonlinear Dyn. 2016, 86, 975–986. [Google Scholar] [CrossRef]
- Murillo-Escobar, M.A.; Cruz-Hernández, C.; Cardoza-Avendaño, L.; Méndez-Ramírez, R. A novel pseudorandom number generator based on pseudorandomly enhanced logistic map. Nonlinear Dyn. 2017, 87, 407–425. [Google Scholar] [CrossRef]
- Yang, Y.G.; Pan, Q.X.; Sun, S.J.; Xu, P. Novel Image Encryption based on Quantum Walks. Sci. Rep. 2015, 5, srep07784. [Google Scholar] [CrossRef] [PubMed]
- Fan, Q.; Wang, Y.; Zhu, L. Complexity analysis of spatial–temporal precipitation system by PCA and SDLE. Appl. Math. Model. 2013, 37, 4059–4066. [Google Scholar] [CrossRef]
- Behnia, S.; Ziaei, J.; Ghiassi, M.; Yahyavi, M. Comprehensive Chaotic Description of Heartbeat Dynamics Using Scale Index and Lyapunov Exponent. In Proceedings of the 6th Chaotic Modeling and Simulation International Conference, Istanbul, Turkey, 11–14 June 2013; Volume 500, pp. 1–5. [Google Scholar]
- Felix, J.L.P.; Balthazar, J.M.; Tusset, A.M.; Piccirillo, V.; Bueno, A.M.; Brasil, R.M.L.R.F. On Optimal Control of a Nonlinear Robotic Mechanism Using the Saturation Phenomenon. In Structural Nonlinear Dynamics and Diagnosis; Springer Proceedings in Physics; Springer: Cham, Switzerland, 2015; pp. 145–165. [Google Scholar] [CrossRef]
- Piccirillo, V.; Balthazar, J.M.; Tusset, A.M.; Bernardini, D.; Rega, G. Characterizing the nonlinear behavior of a pseudoelastic oscillator via the wavelet transform. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2016, 230, 120–132. [Google Scholar] [CrossRef]
- Jiménez, D.S.; Stahl, P.; Terminel, O. Mechanical fault identification using Wavelet Transform and Labview. Nova Sci. 2015, 7, 162–177. [Google Scholar] [CrossRef][Green Version]
- Bolós, V.J.; Benítez, R.; Ferrer, R.; Jammazi, R. The windowed scalogram difference: A novel wavelet tool for comparing time series. Appl. Math. Comput. 2017, 312, 49–65. [Google Scholar] [CrossRef]
- Mallat, S. A Wavelet Tour of Signal Processing: The Sparse Way; Academic Press: Cambridge, MA, USA, 2008. [Google Scholar]
- Torrence, C.; Compo, G.P. A Practical Guide to Wavelet Analysis. Bull. Am. Meteorol. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]
- Liu, Y.; San Liang, X.; Weisberg, R.H. Rectification of the Bias in the Wavelet Power Spectrum. J. Atmos. Ocean. Technol. 2007, 24, 2093–2102. [Google Scholar] [CrossRef]
- Torrence, C.; Webster, P.J. Interdecadal Changes in the ENSO–Monsoon System. J. Clim. 1999, 12, 2679–2690. [Google Scholar] [CrossRef]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2017. [Google Scholar]
- Bolós, V.J.; Benítez, R. wavScalogram: Wavelet Scalogram Tools for Time Series Analysis; R Package Version 1.0.0; 2019; Available online: https://CRAN.R-project.org/package=wavScalogram (accessed on 1 May 2020).
- Scott, A. Neurophysics; Wiley: New York, NY, USA, 1977. [Google Scholar]
- Aguilera, R.F.; Radetzki, M. The synchronized and exceptional price performance of oil and gold: Explanations and prospects. Resour. Policy 2017, 54, 81–87. [Google Scholar] [CrossRef]
- Baur, D.G.; Lucey, B.M. Is gold a hedge or a safe haven? An analysis of stocks, bonds and gold. Financ. Rev. 2010, 45, 217–229. [Google Scholar] [CrossRef]
- Baur, D.G.; McDermott, T.K. Is gold a safe haven? International evidence. J. Bank. Financ. 2010, 24, 1886–1898. [Google Scholar] [CrossRef]
- Ciner, C.; Gurdgiev, C.; Lucey, B.M. Hedges and safe havens: An examination of stocks, bonds, gold, oil and exchange rates. Int. Rev. Financ. Anal. 2013, 29, 202–211. [Google Scholar] [CrossRef]
- Ratti, R.A.; Vespignani, J.L. Why are crude oil prices high when global activity is weak? Econ. Lett. 2013, 21, 133–136. [Google Scholar] [CrossRef]
- Sinai, Y.G. On the notion of entropy for a dynamic system. Dokl. Russ. Acad. Sci. 1959, 124, 768–771. [Google Scholar]
- Borchers, H.W. pracma: Practical Numerical Math Functions; R Package Version 2.2.9; 2019; Available online: https://CRAN.R-project.org/package=pracma (accessed on 1 May 2020).



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bolós, V.J.; Benítez, R.; Ferrer, R. A New Wavelet Tool to Quantify Non-Periodicity of Non-Stationary Economic Time Series. Mathematics 2020, 8, 844. https://doi.org/10.3390/math8050844
Bolós VJ, Benítez R, Ferrer R. A New Wavelet Tool to Quantify Non-Periodicity of Non-Stationary Economic Time Series. Mathematics. 2020; 8(5):844. https://doi.org/10.3390/math8050844
Chicago/Turabian StyleBolós, Vicente J., Rafael Benítez, and Román Ferrer. 2020. "A New Wavelet Tool to Quantify Non-Periodicity of Non-Stationary Economic Time Series" Mathematics 8, no. 5: 844. https://doi.org/10.3390/math8050844
APA StyleBolós, V. J., Benítez, R., & Ferrer, R. (2020). A New Wavelet Tool to Quantify Non-Periodicity of Non-Stationary Economic Time Series. Mathematics, 8(5), 844. https://doi.org/10.3390/math8050844





