Unsteady Three-Dimensional MHD Non-Axisymmetric Homann Stagnation Point Flow of a Hybrid Nanofluid with Stability Analysis
Abstract
1. Introduction
2. Mathematical Model
3. Stability Analysis
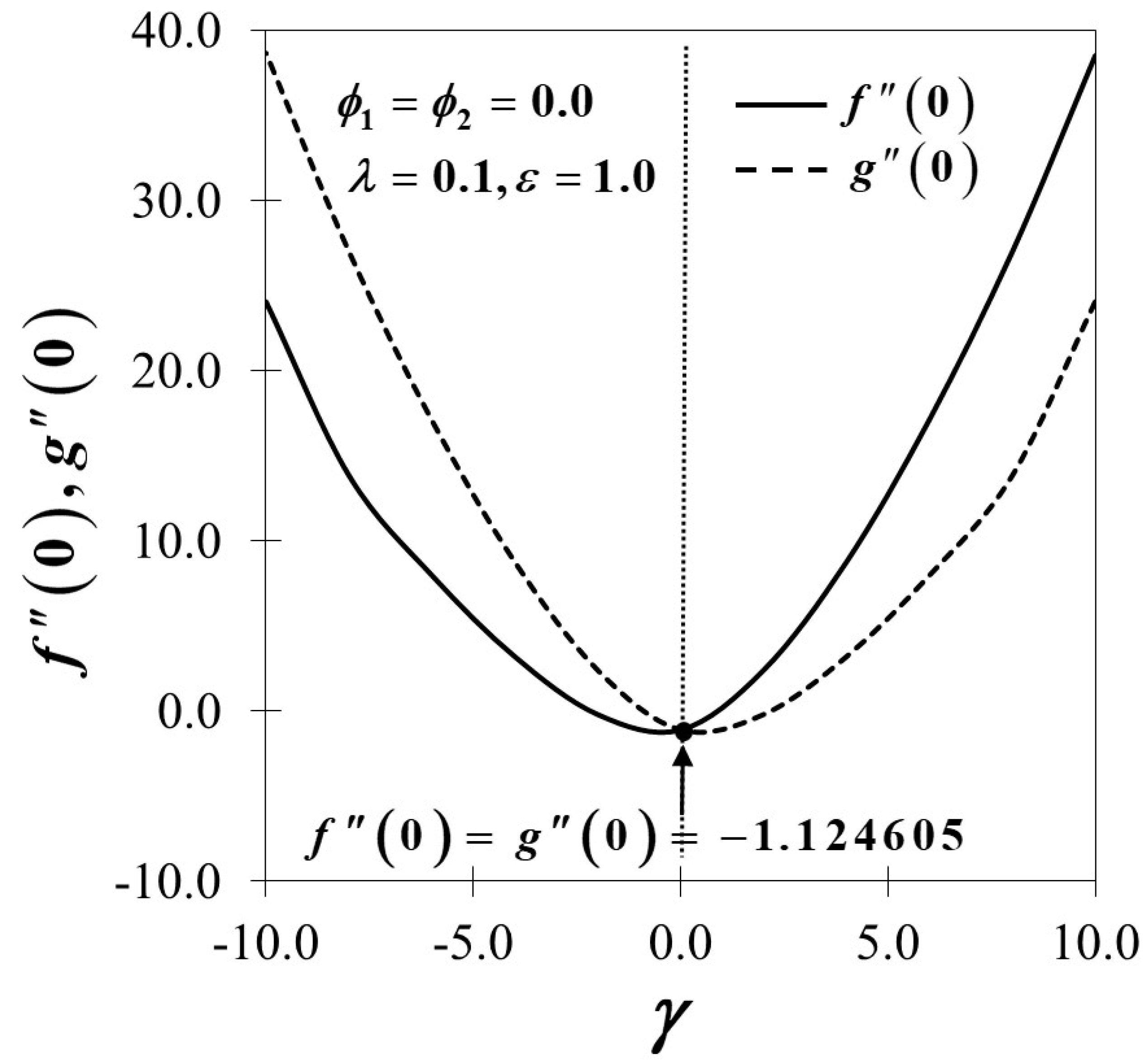
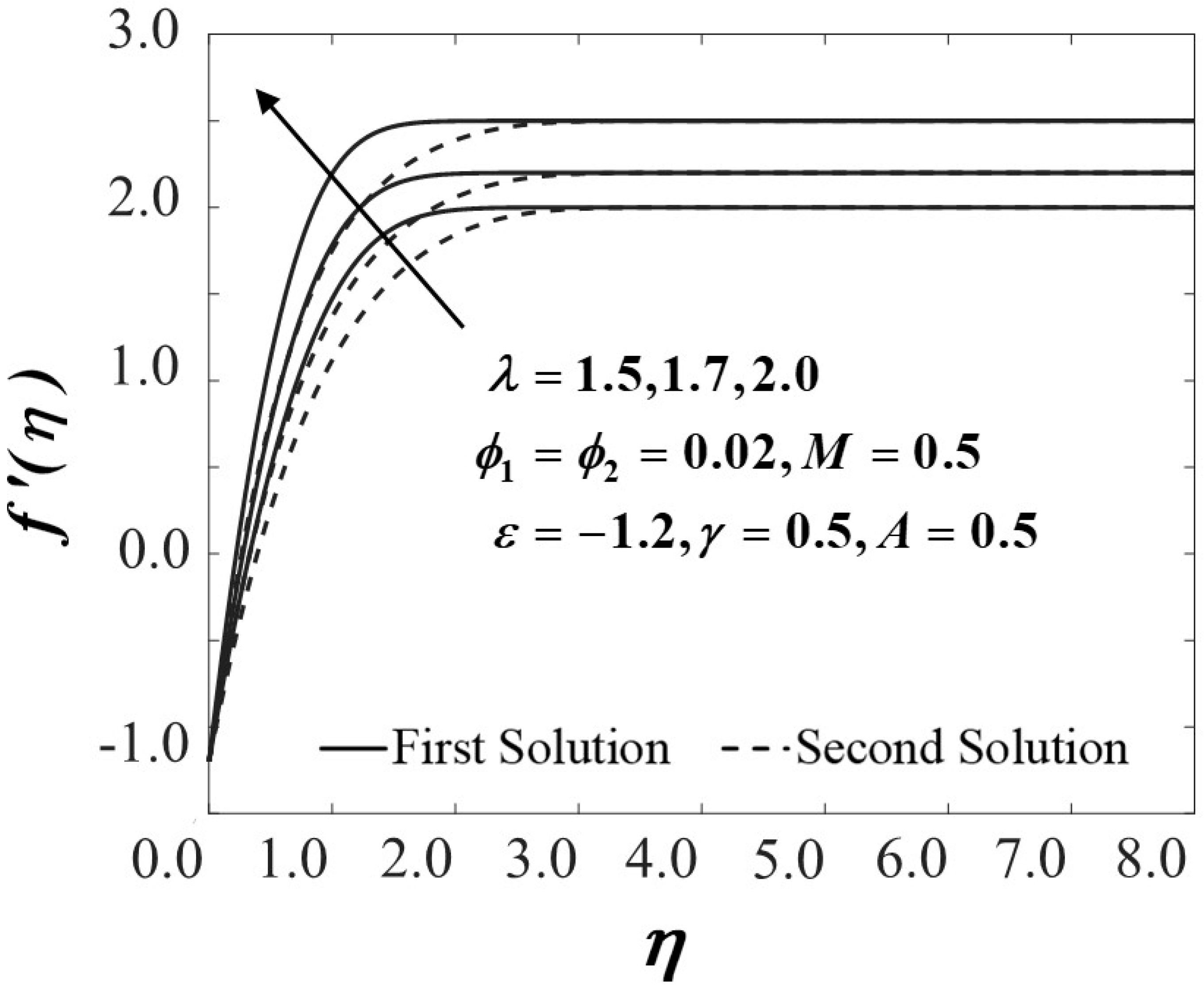
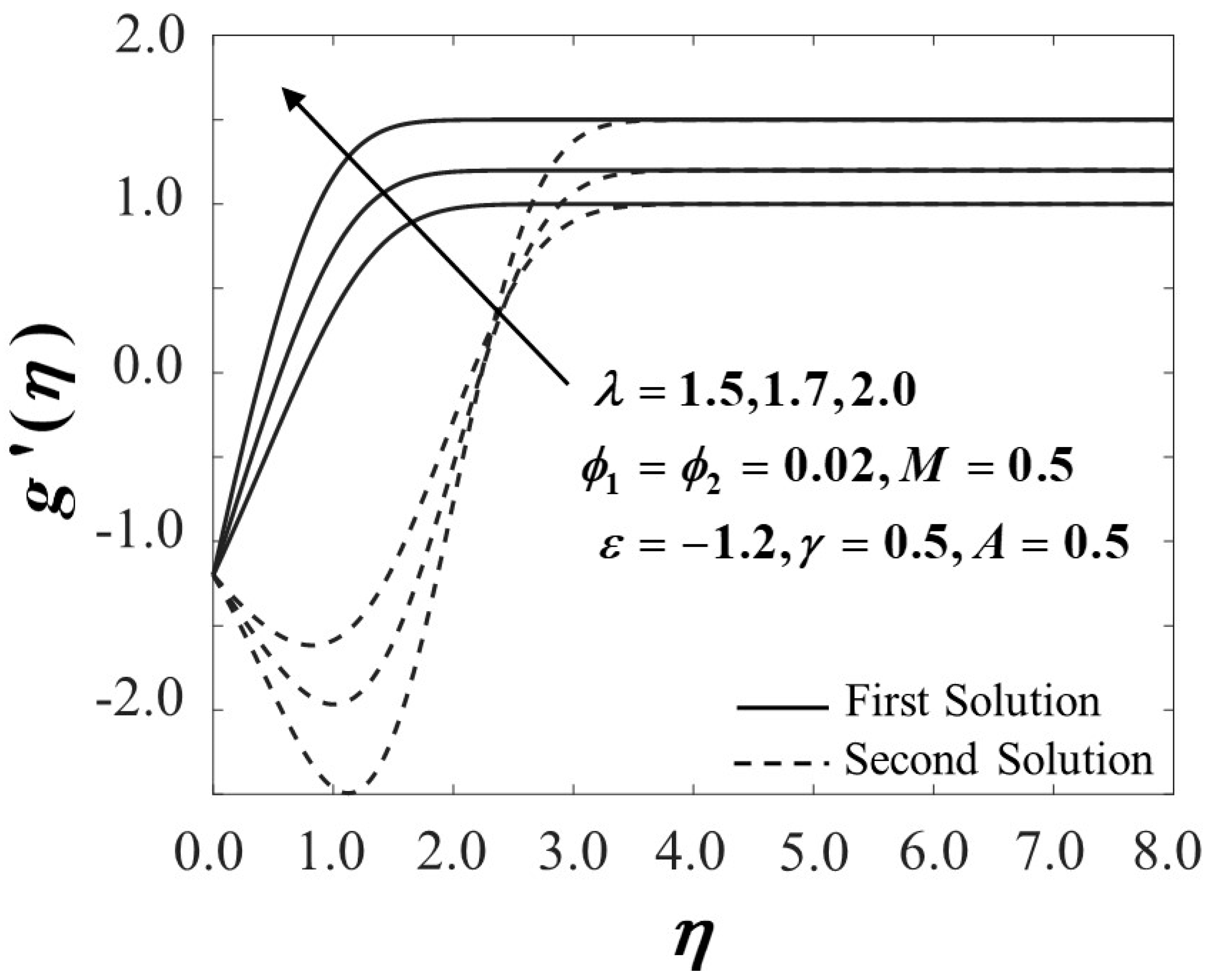
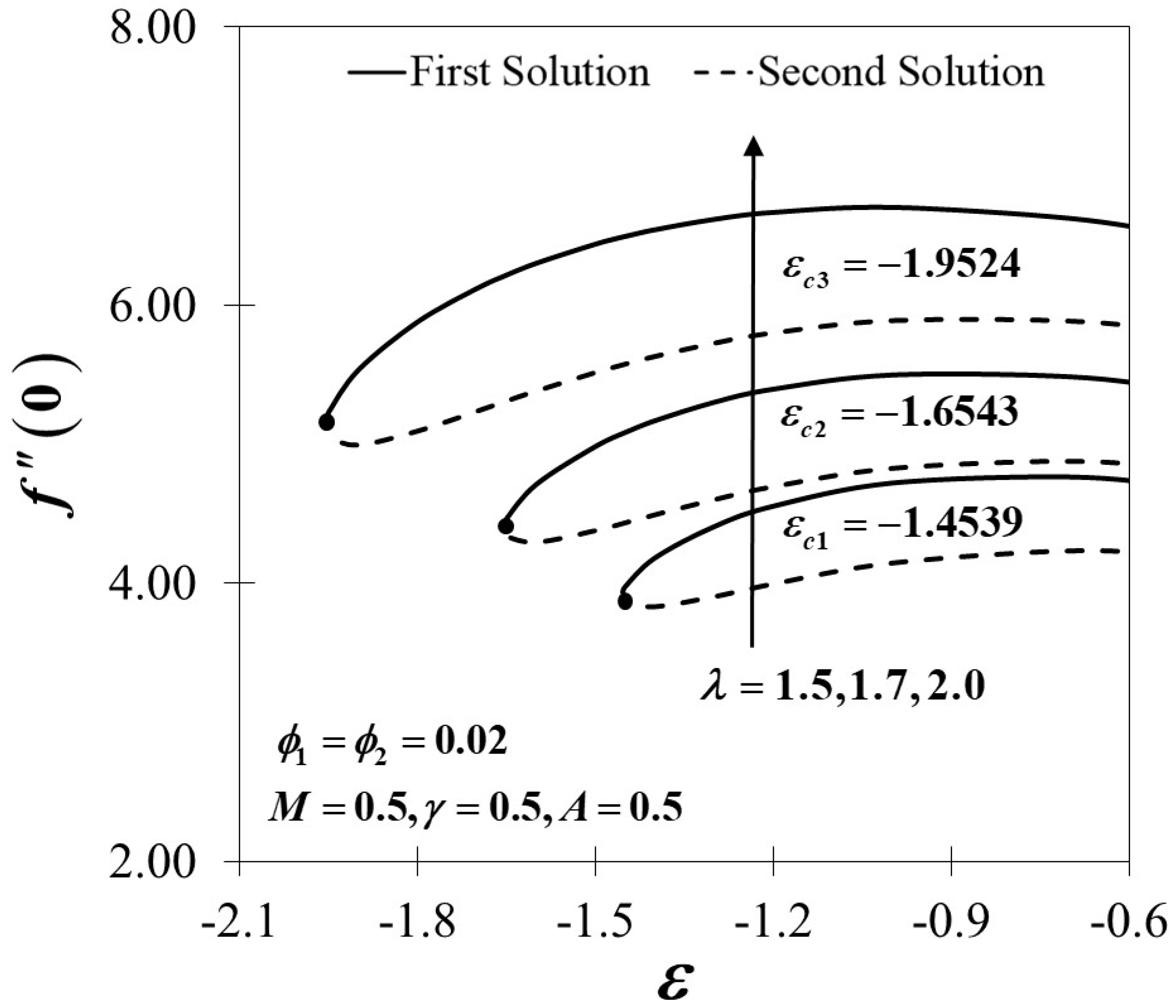
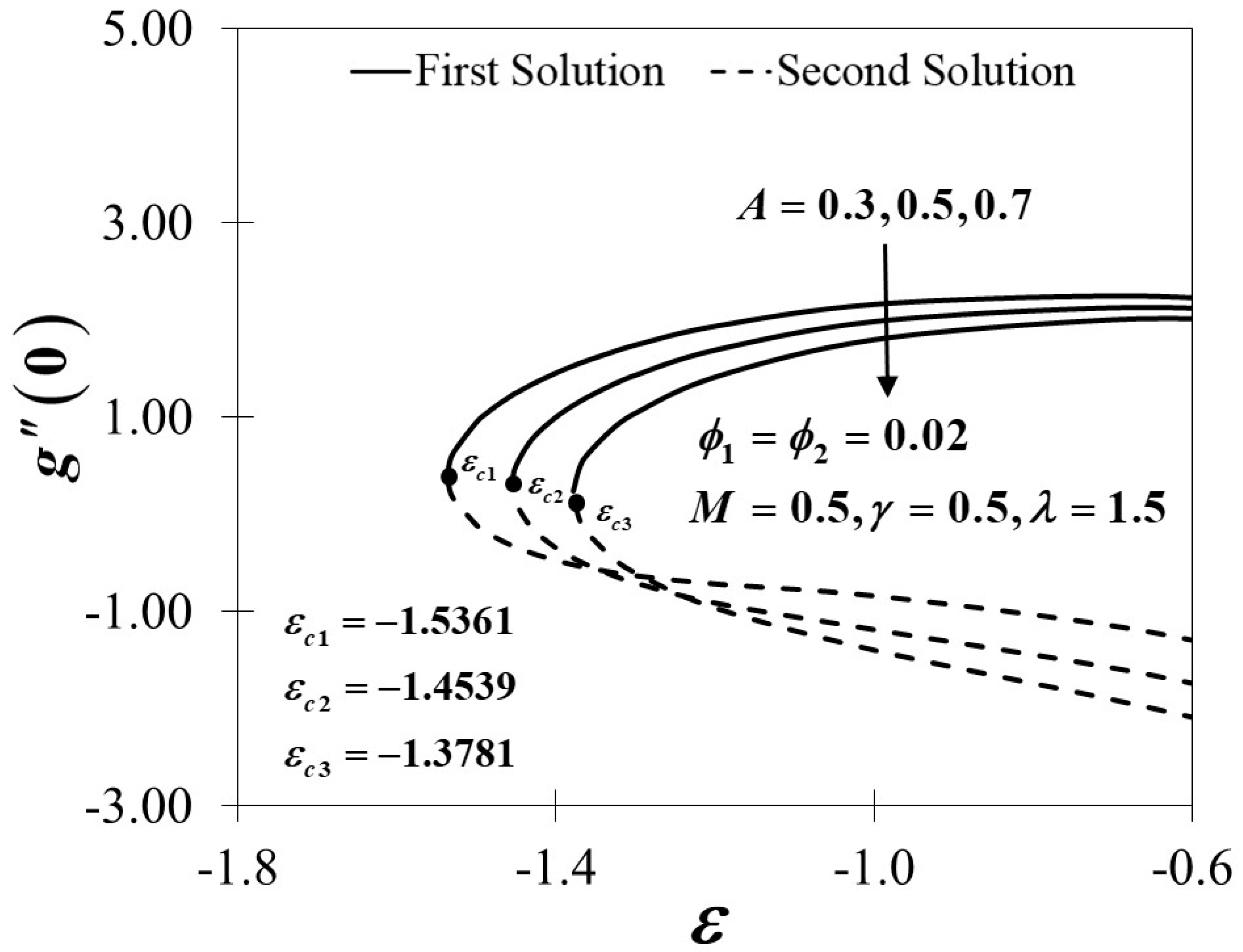
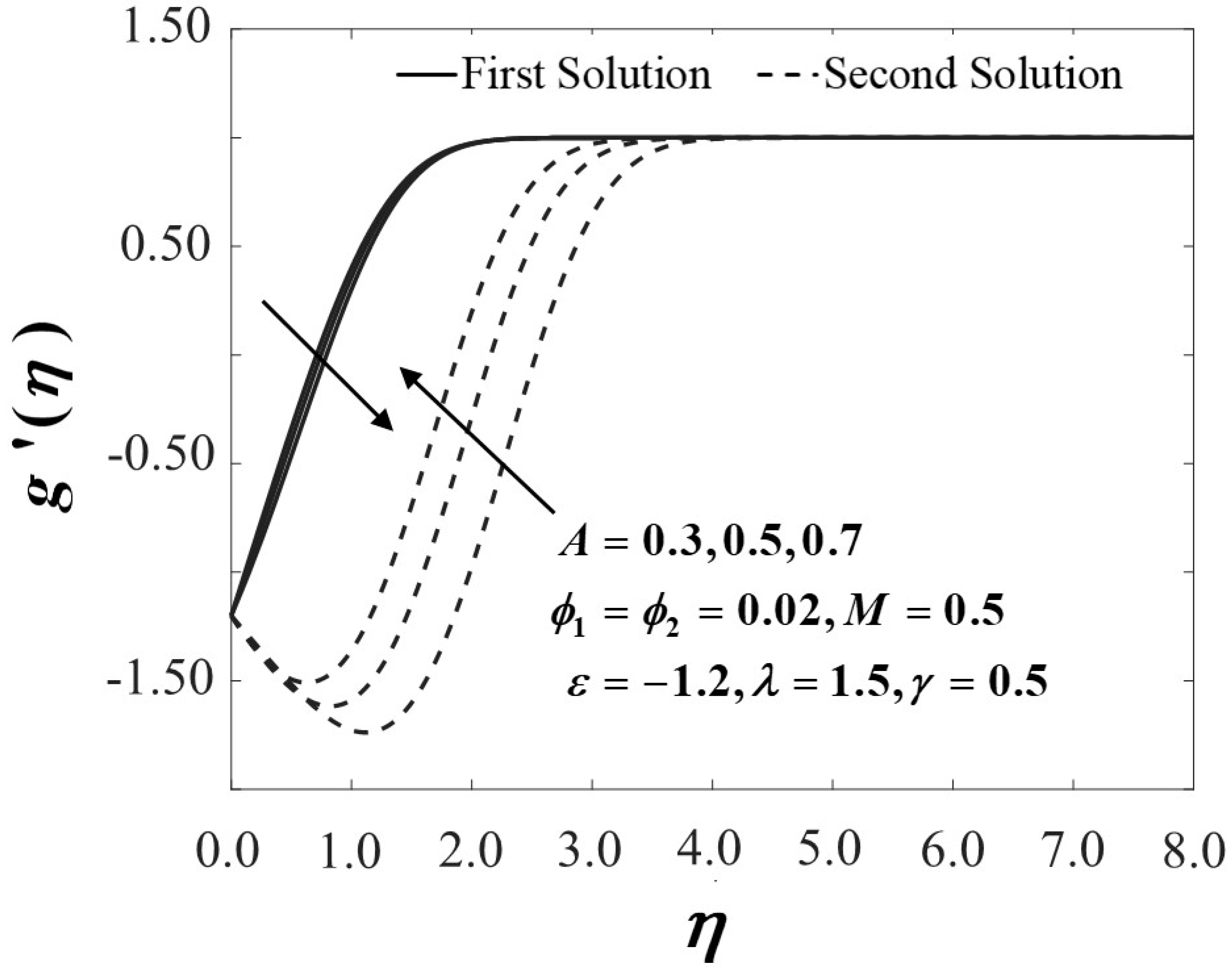
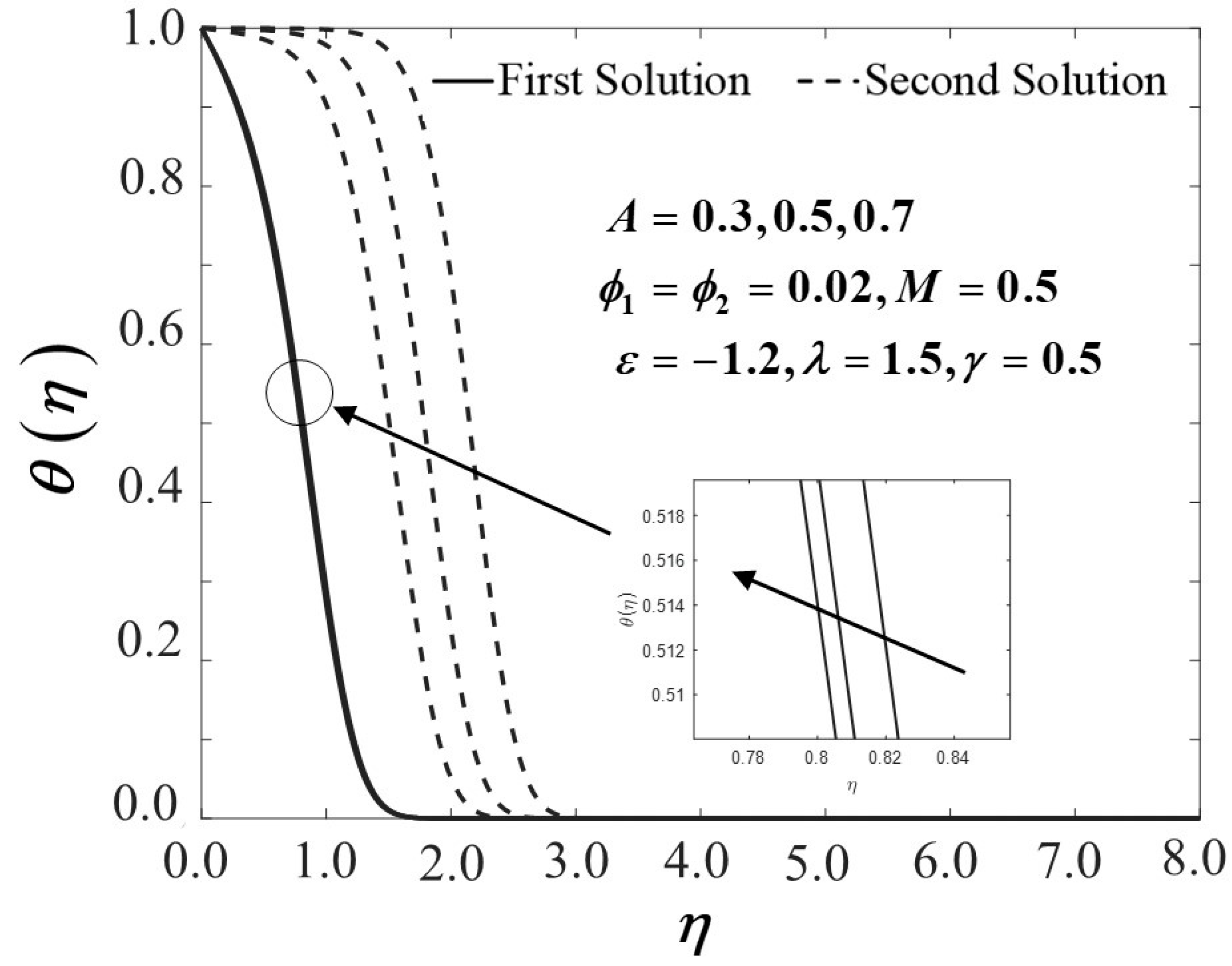
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Roman letters | |
| positive constants | |
| unsteadiness parameter | |
| transverse magnetic field | |
| skin friction coefficient along the direction | |
| skin friction coefficient along the direction | |
| specific heat at constant pressure | |
| heat capacitance of the fluid | |
| dimensionless stream function in the direction | |
| dimensionless stream function in the direction | |
| thermal conductivity of the fluid | |
| magnetic parameter | |
| local Nusselt number | |
| Prandtl number | |
| local Reynolds number in the and directions, respectively | |
| time | |
| fluid temperature | |
| surface temperature | |
| ambient temperature | |
| velocities component in the and directions, respectively | |
| velocities of the free stream in the and directions | |
| velocities of the stretching/shrinking surface in the and directions | |
| Cartesian coordinates | |
| Greek symbols | |
| positive constant | |
| fluid thermal diffusivity | |
| thermal expansion coefficient | |
| ratio of the ambient fluid shear rate to the plate strain rate | |
| stretching/shrinking parameter | |
| estimated relative error | |
| similarity variable | |
| dimensionless temperature | |
| ratio of the ambient fluid strain rate to the plate strain rate | |
| dynamic viscosity | |
| kinematic viscosity | |
| density | |
| electrical conductivity | |
| dimensionless time variable | |
| wall shear stress along the and directions | |
| nanoparticle volume fractions for Al2O3 (alumina) | |
| nanoparticle volume fractions for Cu (copper) | |
| eigenvalue | |
| smallest eigenvalue | |
| Subscripts | |
| base fluid | |
| nanofluid | |
| hybrid nanofluid | |
| solid component for Al2O3 (alumina) | |
| solid component for Cu (copper) | |
| Superscript | |
| differentiation with respect to | |
References
- Fisher, E. Extrusion of Plastics; Wiley: New York, NY, USA, 1976. [Google Scholar]
- Rauwendaal, C. Polymer Extrusion; Hanser Publication: Munich, Germany, 1985. [Google Scholar]
- Ibrahim, W.; Shankar, B.; Nandeppanavar, M.M. MHD stagnation point flow and heat transfer due to nanofluid towards a stretching sheet. Int. J. Heat Mass Transf. 2013, 56, 1–9. [Google Scholar] [CrossRef]
- Hiemenz, K. Die Grenzschicht an einem in den gleichfÖrmingen Flüssigkeitsstrom eingetauchten geraden Kreiszylinder. Dinglers Polytech J. 1911, 326, 321–324. [Google Scholar]
- Homann, F. Der Einfluss grosser Zähigkeit bei der Strömung um den Zylinder und um die Kugel. Z. Angew. Math. Mech. 1936, 16, 153–164. [Google Scholar] [CrossRef]
- Howarth, L. CXLIV. The boundary layer in three-dimensional flow—Part II. The flow near a stagnation point. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1951, 42, 1433–1440. [Google Scholar] [CrossRef]
- Davey, A. Boundary-layer flow at a saddle point of attachment. J. Fluid Mech. 1961, 10, 593–610. [Google Scholar] [CrossRef]
- Davey, A.; Schofield, D. Three-dimensional flow near a two-dimensional stagnation point. J. Fluid Mech. 1967, 28, 149–151. [Google Scholar] [CrossRef]
- Weidman, P.D. Non-axisymmetric Homann stagnation-point flows. J. Fluid Mech. 2012, 702, 460–469. [Google Scholar] [CrossRef]
- Mahapatra, T.R.; Sidui, S. Unsteady heat transfer in non-axisymmetric Homann stagnation-point flows. Z. Angew. Math. Phys. 2017, 68, 32. [Google Scholar] [CrossRef]
- Mahapatra, T.R.; Sidui, S. Non-axisymmetric Homann stagnation-point flow of a viscoelastic fluid towards a fixed plate. Eur. J. Mech. B/Fluids 2020, 79, 38–43. [Google Scholar] [CrossRef]
- Wang, C.Y. Stagnation flow towards a shrinking sheet. Int. J. Non. Linear Mech. 2008, 43, 377–382. [Google Scholar] [CrossRef]
- Mahapatra, T.R.; Sidui, S. Unsteady heat transfer in non-axisymmetric Homann stagnation-point flows towards a stretching/shrinking sheet. Eur. J. Mech. B/Fluids 2019, 75, 199–208. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Pop, I.; Nazar, R.; Hafidzuddin, E.H.; Wahi, N. Non-axisymmetric Homann stagnation point flow and heat transfer past a stretching/shrinking sheet using hybrid nanofluid. Int. J. Numer. Methods Heat Fluid Flow 2020. [Google Scholar] [CrossRef]
- Zaimi, K.; Ishak, A. Stagnation-point flow towards a stretching vertical sheet with slip effects. Mathematics 2016, 4, 27. [Google Scholar] [CrossRef]
- Arif Ullah Khan, S.; Saleem, S.; Nadeem, A.A.A. Analysis of unsteady non-axisymmetric Homann stagnation point flow of nanofluid and possible existence of multiple solutions. Physica A 2019, 137567. [Google Scholar] [CrossRef]
- Jusoh, R.; Nazar, R.; Pop, I. Impact of heat generation/absorption on the unsteady magnetohydrodynamic stagnation point flow and heat transfer of nanofluids. Int. J. Numer. Methods Heat Fluid Flow 2019, 30, 557–574. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.; Hafidzuddin, E.H.; Wahi, N. MHD mixed convective stagnation point flow with heat generation past a shrinking sheet. ASM Sci. J. 2019, 71–81. [Google Scholar]
- Ali, F.M.; Naganthran, K.; Nazar, R.; Pop, I. MHD mixed convection boundary layer stagnation-point flow on a vertical surface with induced magnetic field: A stability analysis. Int. J. Numer. Methods Heat Fluid Flow 2019. [Google Scholar] [CrossRef]
- Shehzad, S.A.; Abdullah, Z.; Alsaedi, A.; Abbasi, F.M.; Hayat, T. Thermally radiative three-dimensional flow of Jeffrey nanofluid with internal heat generation and magnetic field. J. Magn. Magn. Mater. 2016, 397, 108–114. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Double stratification effects on unsteady electrical MHD mixed convection flow of nanofluid with viscous dissipation and Joule heating. J. Appl. Res. Technol. 2017, 15, 464–476. [Google Scholar] [CrossRef]
- Pavlov, K.B. Magnetohydrodynamic flow of an incompressible viscous fluid caused by deformation of a plane surface. Magn. Gidrodin. 1974, 4, 146–147. [Google Scholar]
- Chakrabarti, A.; Gupta, A.S. Hydromagnetic flow and heat transfer over a stretching sheet. Q. Appl. Math. 1979, 37, 73–78. [Google Scholar] [CrossRef]
- Vajravelu, K. Hydromagnetic flow and heat transfer over a continuous, moving, porous, flat surface. Acta Mech. 1986, 185, 179–185. [Google Scholar] [CrossRef]
- Andersson, H.I. An exact solution of the Navier-Stokes equations for magnetohydrodynamic flow. Acta Mech. 1995, 113, 241–244. [Google Scholar] [CrossRef]
- Lok, Y.Y.; Ishak, A.; Pop, I. MHD stagnation-point flow towards a shrinking sheet. Int. J. Numer. Methods Heat Fluid Flow 2011, 21, 61–72. [Google Scholar] [CrossRef]
- Almutairi, F.; Khaled, S.M.; Ebaid, A. MHD flow of nanofluid with homogeneous-heterogeneous reactions in a porous medium under the influence of second-order velocity slip. Mathematics 2019, 7, 220. [Google Scholar] [CrossRef]
- Zhu, J.; Xu, Y.; Han, X. A non-newtonian magnetohydrodynamics (MHD) nanofluid flow and heat transfer with nonlinear slip and temperature jump. Mathematics 2019, 7, 1199. [Google Scholar] [CrossRef]
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. ASME Publ. Fed. 1995, 231, 99–103. [Google Scholar]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Mahian, O.; Kianifar, A.; Kalogirou, S.A.; Pop, I.; Wongwises, S. A review of the applications of nanofluids in solar energy. Heat Mass Transf. 2013, 57, 582–594. [Google Scholar] [CrossRef]
- Saidur, R.; Leong, K.Y.; Mohammed, H.A. A review on applications and challenges of nanofluids. Renew. Sustain. Energy Rev. 2011, 15, 1646–1668. [Google Scholar] [CrossRef]
- Thaker, R.; Patel, J.R. A review on application of nanofluids in solar energy. Am. J. Nano Res. Appl. 2015, 2, 53–61. [Google Scholar]
- Sarkar, J.; Ghosh, P.; Adil, A. A review on hybrid nanofluids: Recent research, development and applications. Renew. Sustain. Energy Rev. 2015, 43, 164–177. [Google Scholar] [CrossRef]
- Sidik, N.A.C.; Mahmud Jamil, M.; Aziz Japar, W.M.A.; Muhammad Adamu, I. A review on preparation methods, stability and applications of hybrid nanofluids. Renew. Sustain. Energy Rev. 2017, 80, 1112–1122. [Google Scholar] [CrossRef]
- Huminic, G.; Huminic, A. Hybrid nanofluids for heat transfer applications—A state-of-the-art review. Int. J. Heat Mass Transf. 2018, 125, 82–103. [Google Scholar] [CrossRef]
- Turcu, R.; Darabont, A.; Nan, A.; Aldea, N.; Macovei, D.; Bica, D.; Vekas, L.; Pana, O.; Soran, M.L.; Koos, A.A.; et al. New polypyrrole-multiwall carbon nanotubes hybrid materials. J Optoelectron. Adv. Mater. 2006, 8, 643–647. [Google Scholar]
- Yen, T.H.; Soong, C.Y.; Tzeng, P.Y. Erratum: Hybrid molecular dynamics-continuum simulation for nano/mesoscale channel flows (Microfluid Nanofluid). Microfluid. Nanofluidics 2007, 3, 729. [Google Scholar] [CrossRef]
- Devi, S.S.U.; Devi, S.P.A. Numerical investigation of three-dimensional hybrid Cu–Al2O3/water nanofluid flow over a stretching sheet with effecting Lorentz force subject to Newtonian heating. Can. J. Phys. 2016, 94, 490–496. [Google Scholar] [CrossRef]
- Devi, S.U.; Devi, S.P.A. Heat transfer enhancement of Cu−Al2O3/water hybrid nanofluid flow over a stretching sheet. J. Niger. Math. Soc. 2017, 36, 419–433. [Google Scholar]
- Yousefi, M.; Dinarvand, S.; Eftekhari Yazdi, M.; Pop, I. Stagnation-point flow of an aqueous titania-copper hybrid nanofluid toward a wavy cylinder. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 1716–1735. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Nazar, R.; Hafidzuddin, E.H.; Wahi, N.; Pop, I. Magnetohydrodynamics (MHD) axisymmetric flow and heat transfer of a hybrid nanofluid past a radially permeable stretching/shrinking sheet with Joule heating. Chin. J. Phys. 2020, 64, 251–263. [Google Scholar] [CrossRef]
- Hayat, T.; Nadeem, S. Heat transfer enhancement with Ag–CuO/water hybrid nanofluid. Results Phys. 2017, 7, 2317–2324. [Google Scholar] [CrossRef]
- Dinarvand, S.; Rostami, M.N.; Pop, I. A novel hybridity model for TiO2-CuO/water hybrid nanofluid flow over a static/moving wedge or corner. Sci. Rep. 2019, 9, 16290. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Unsteady flow and heat transfer past a stretching/shrinking sheet in a hybrid nanofluid. Int. J. Heat Mass Transf. 2019, 136, 288–297. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Flow and heat transfer along a permeable stretching/shrinking curved surface in a hybrid nanofluid. Phys. Scr. 2019, 94, 105219. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow and heat transfer over a nonlinear permeable stretching/shrinking surface. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 3110–3127. [Google Scholar] [CrossRef]
- Cai, W.; Su, N.; Liu, X. Unsteady convection flow and heat transfer over a vertical stretching surface. PLoS ONE 2014, 9, e107229. [Google Scholar] [CrossRef]
- Hayat, T.; Nadeem, S.; Khan, A.U. Rotating flow of Ag-CuO/H2O hybrid nanofluid with radiation and partial slip boundary effects. Eur. Phys. J. E 2018, 41, 75. [Google Scholar] [CrossRef]
- Devi, S.P.A.; Devi, S.S.U. Numerical investigation of hydromagnetic hybrid Cu—Al2O3/water nanofluid flow over a permeable stretching sheet with suction. Int. J. Nonlinear Sci. Numer. Simul. 2016, 17, 249–257. [Google Scholar] [CrossRef]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Merrill, K.; Beauchesne, M.; Previte, J.; Paullet, J.; Weidman, P. Final steady flow near a stagnation point on a vertical surface in a porous medium. Int. J. Heat Mass Transf. 2006, 49, 4681–4686. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Nawaz, M.; Hayat, T. Axisymmetric stagnation-point flow of nanofluid over a stretching surface. Adv. Appl. Math. Mech. 2014, 6, 220–232. [Google Scholar] [CrossRef]
- Yuan, J.; Piomelli, U. Roughness effects on the Reynolds stress budgets in near-wall turbulence. J. Fluid Mech. 2014, 760, 1–12. [Google Scholar] [CrossRef]
- Ghodke, C.D.; Apte, S.V. DNS study of particle-bed-turbulence interactions in an oscillatory wall-bounded flow. J. Fluid Mech. 2016, 792, 232–251. [Google Scholar] [CrossRef]

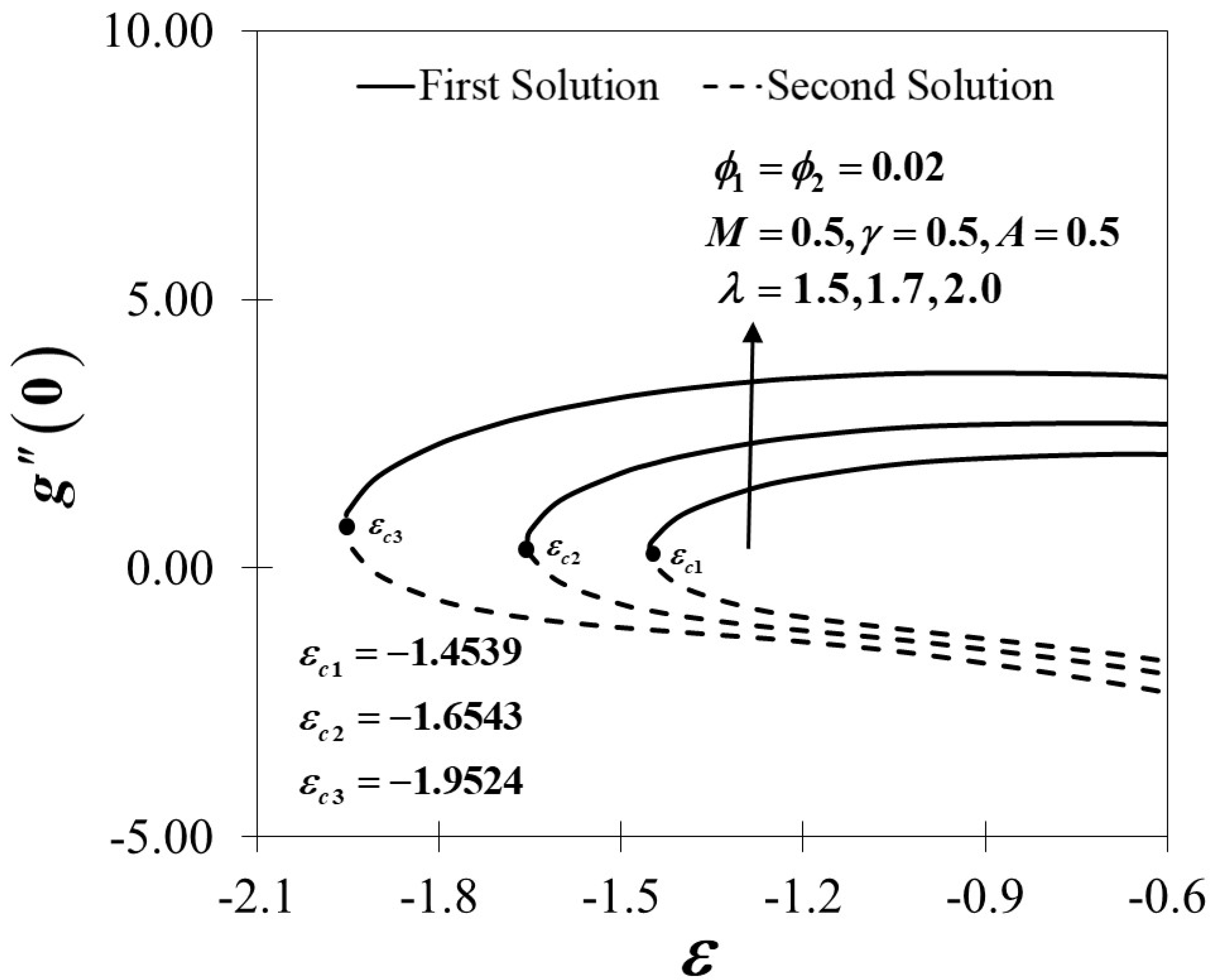
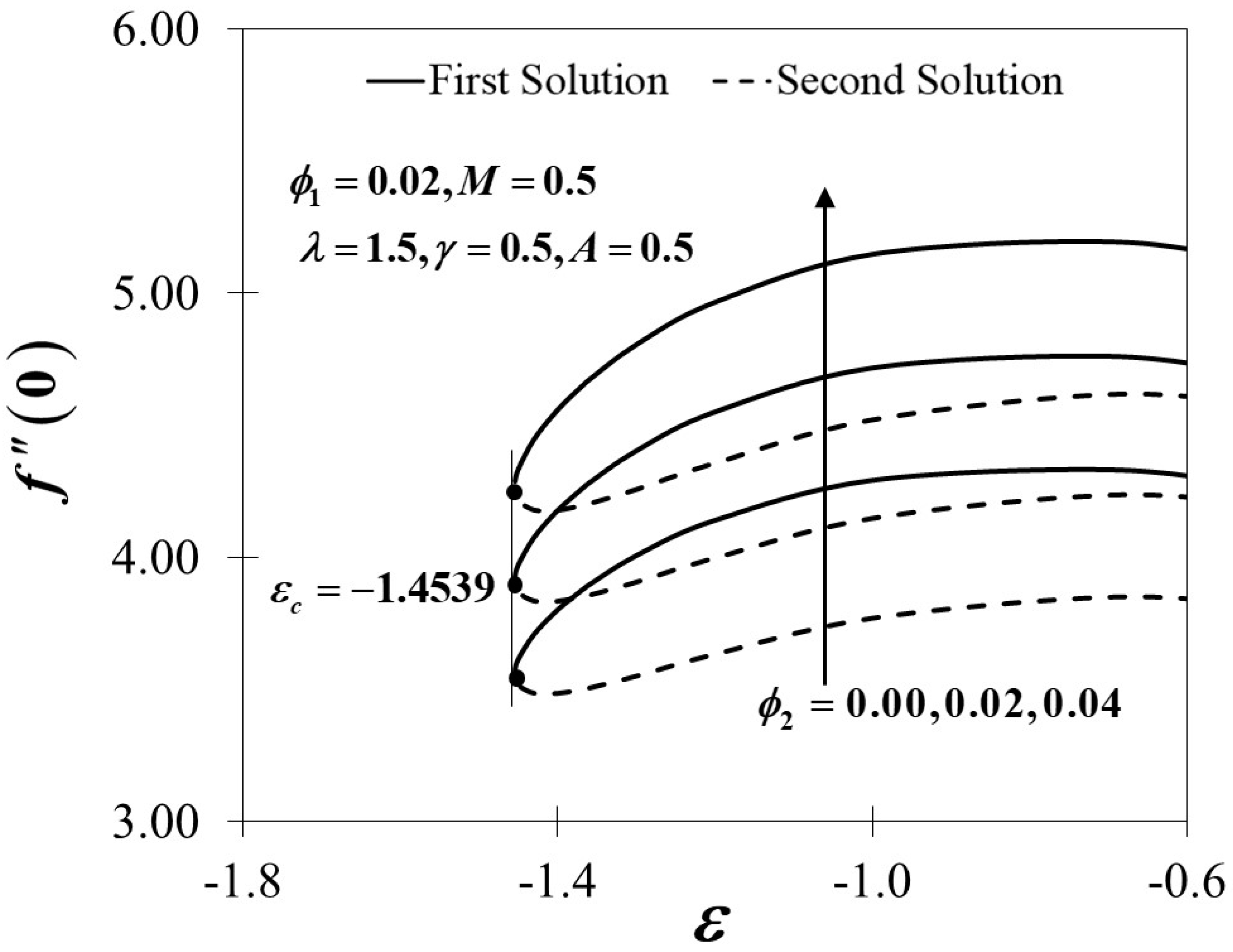
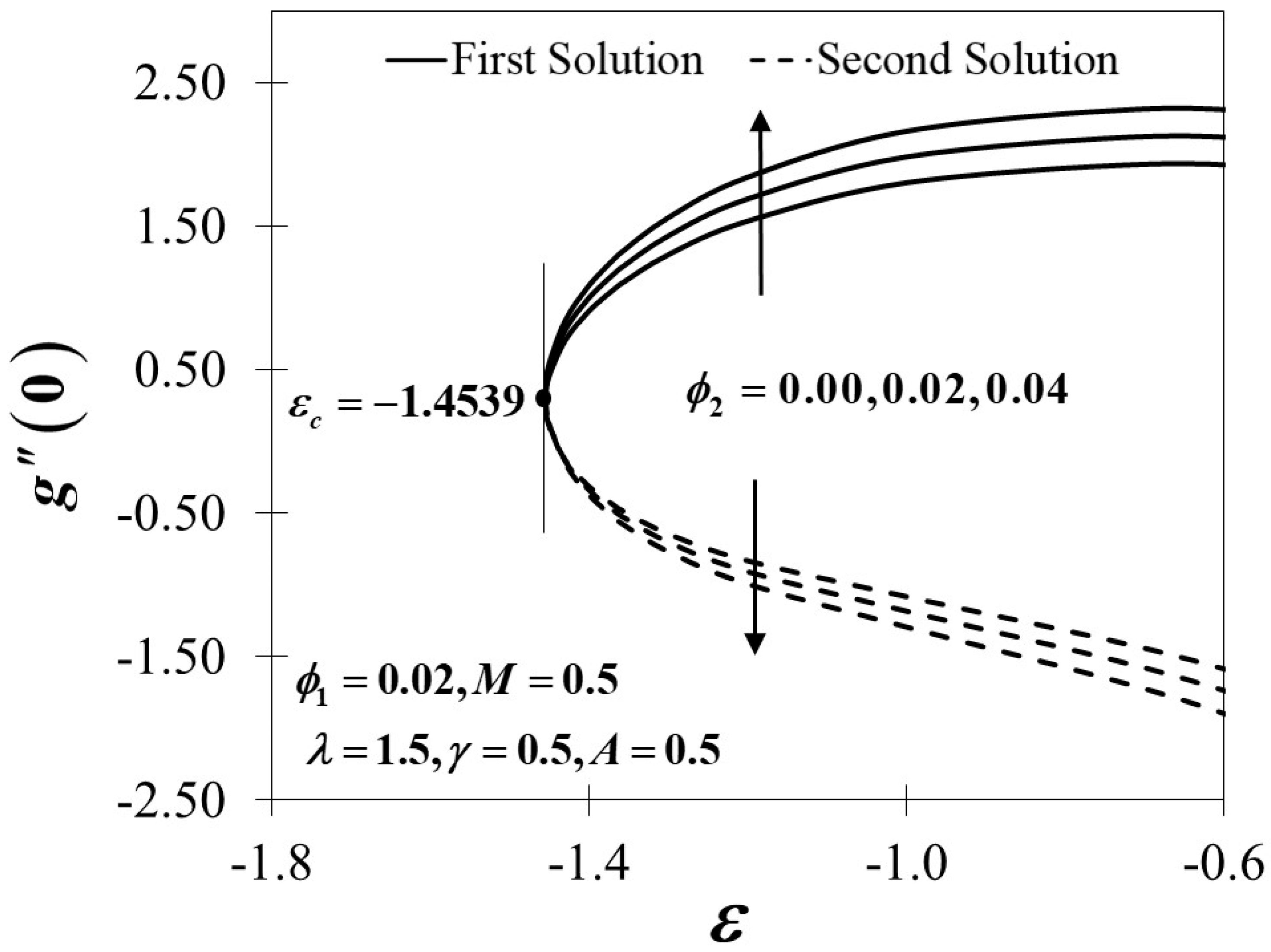
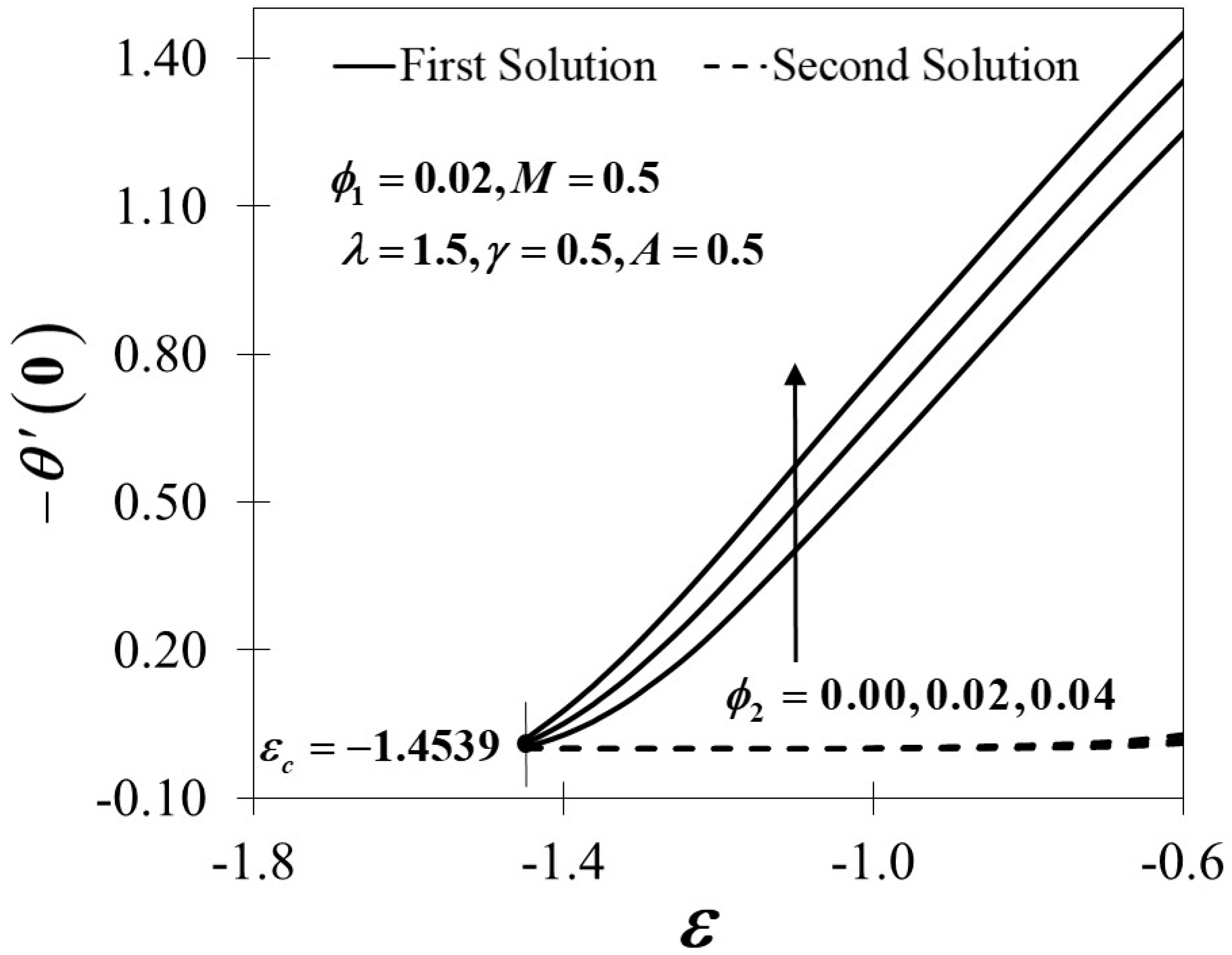
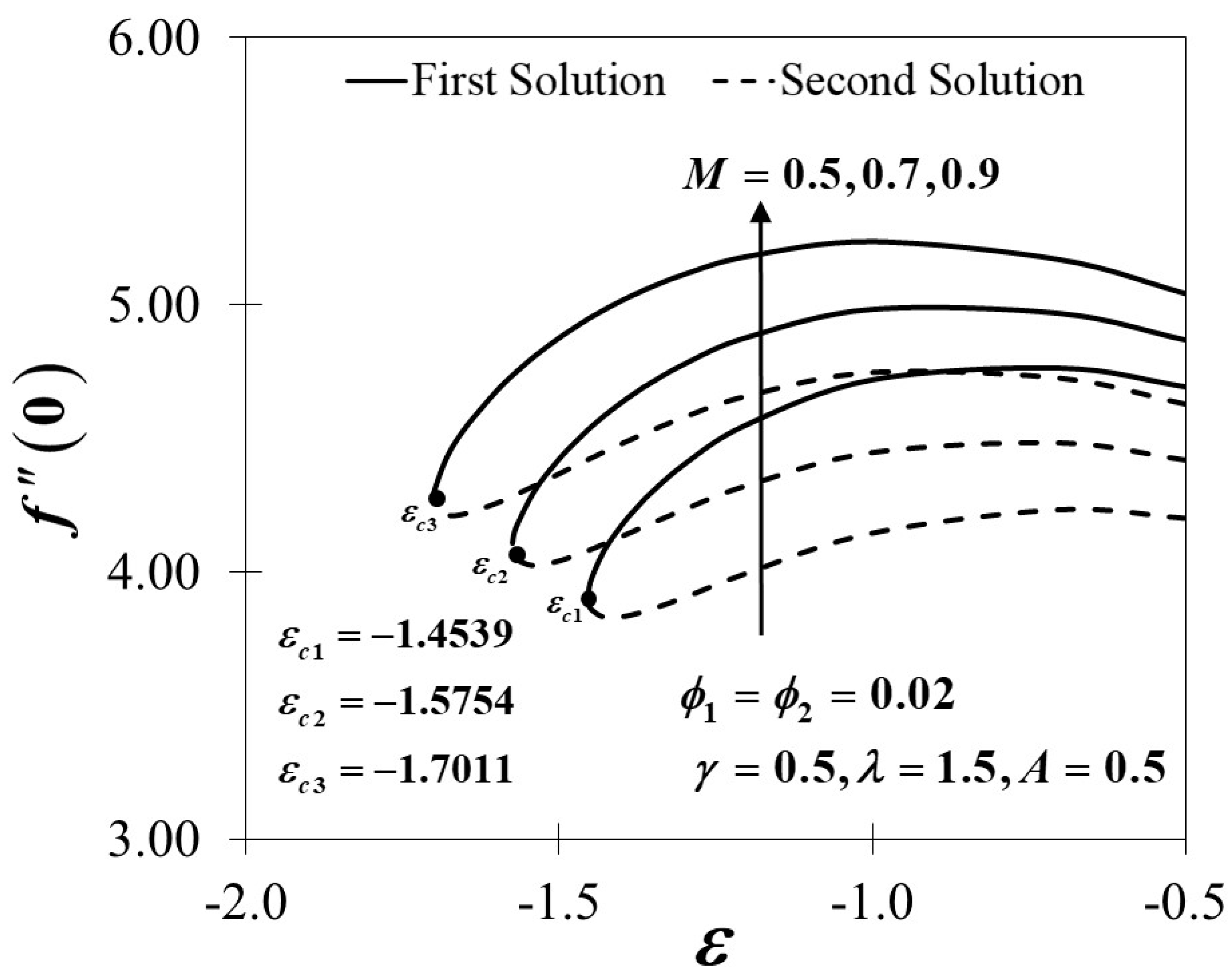
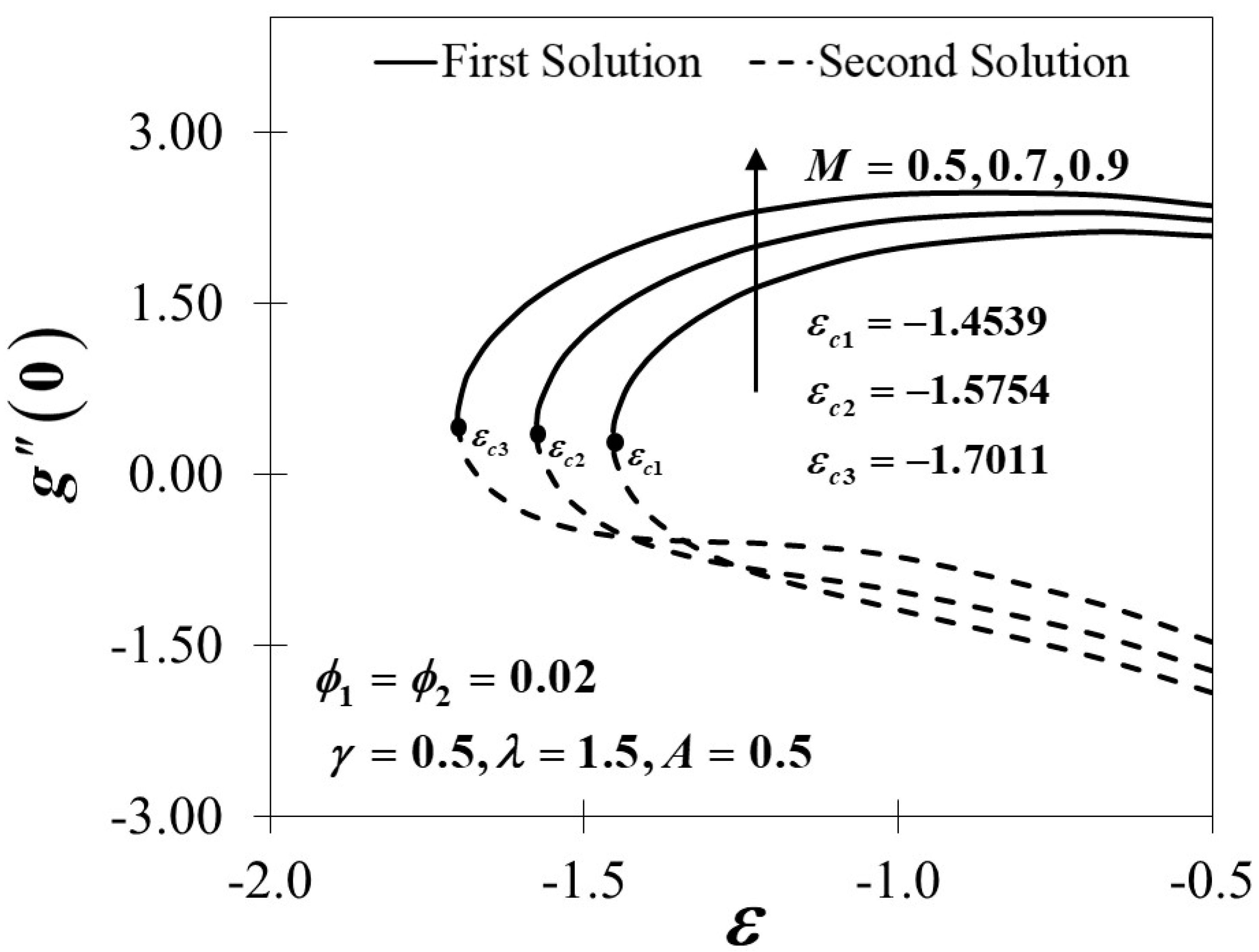

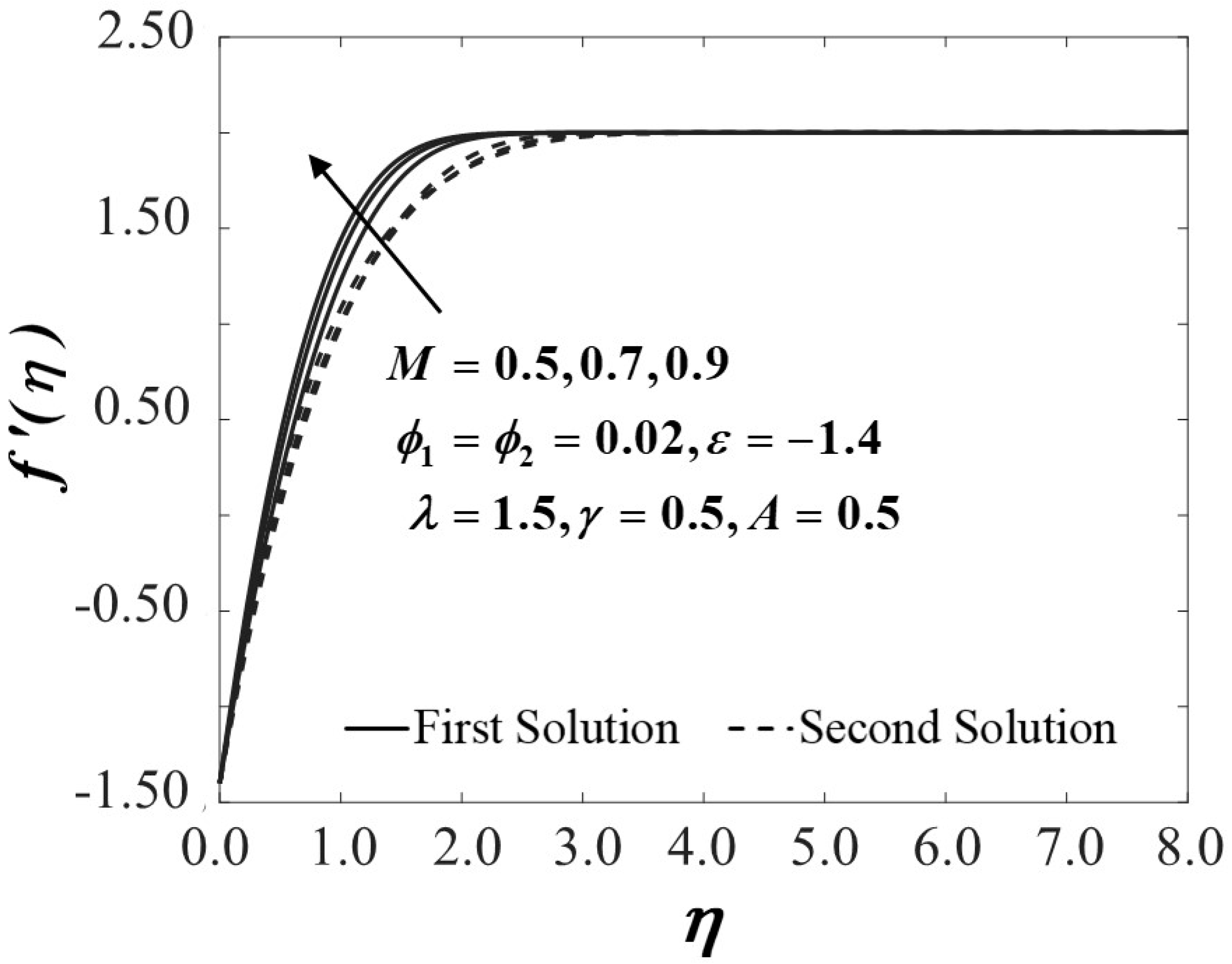


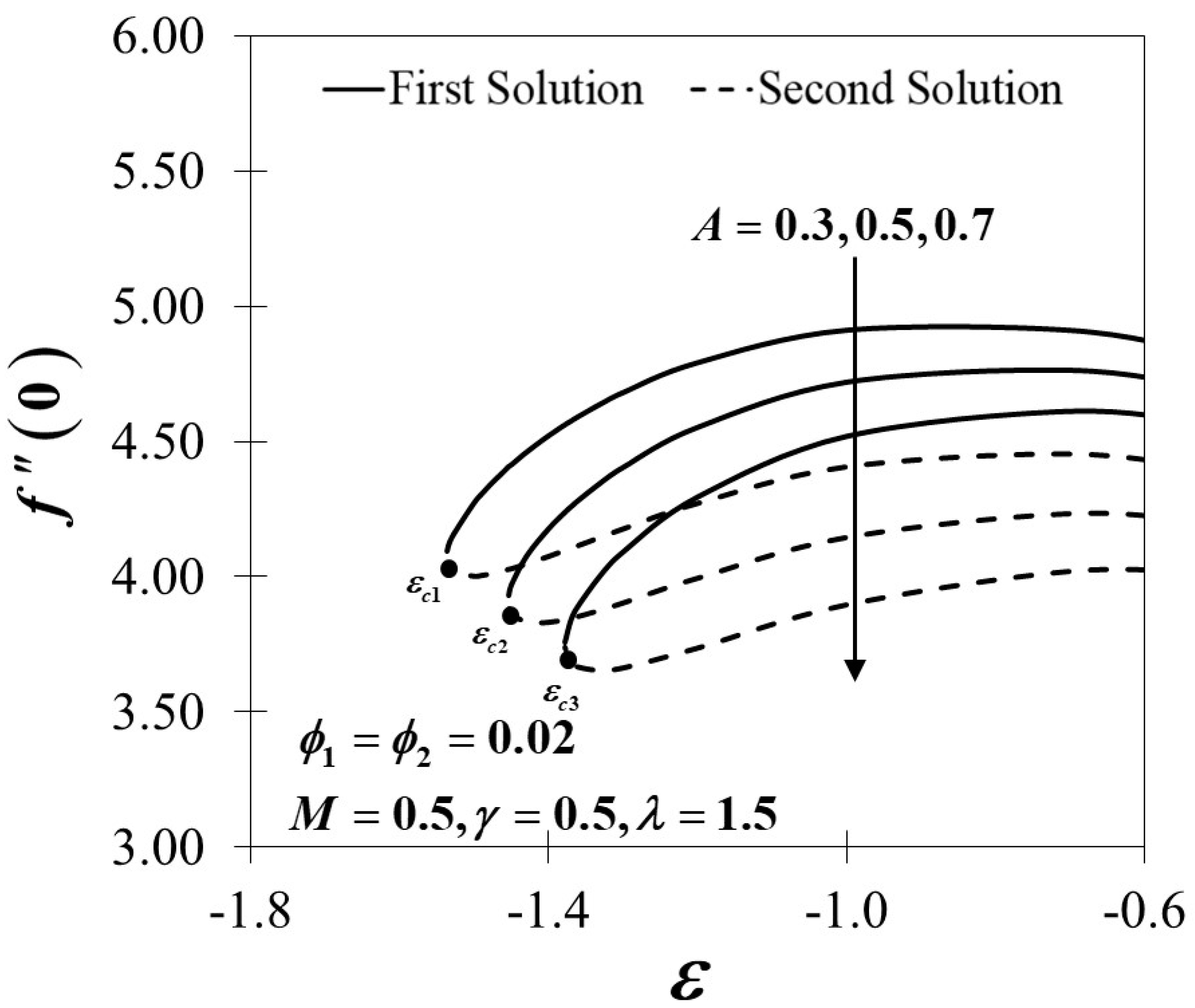


| Properties | Hybrid Nanofluid |
|---|---|
| Density | |
| Heat capacity | |
| Dynamic viscosity | |
| Thermal conductivity | where, |
| Properties | Al2O3 | Cu | H2O |
|---|---|---|---|
| 3970 | 8933 | 997.1 | |
| 765 | 385 | 4179 | |
| 40 | 400 | 0.613 | |
| 0.85 | 1.67 | 21 |
| λ | Present Result | Mahapatra and Sidui [10] | Nawaz and Hayat [57] | ||
|---|---|---|---|---|---|
| 1.4 | 0.9181 | 0.3952 |
| 1.452 | 0.1712 | 0.1469 |
| 1.4532 | 0.1158 | 0.0979 |
| 1.4534 | 0.1040 | 0.0869 |
| 1.4536 | 0.0902 | 0.0744 |
| 1.4538 | 0.0741 | 0.0593 |
| 1.45388 | 0.0665 | 0.0521 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Unsteady Three-Dimensional MHD Non-Axisymmetric Homann Stagnation Point Flow of a Hybrid Nanofluid with Stability Analysis. Mathematics 2020, 8, 784. https://doi.org/10.3390/math8050784
Zainal NA, Nazar R, Naganthran K, Pop I. Unsteady Three-Dimensional MHD Non-Axisymmetric Homann Stagnation Point Flow of a Hybrid Nanofluid with Stability Analysis. Mathematics. 2020; 8(5):784. https://doi.org/10.3390/math8050784
Chicago/Turabian StyleZainal, Nurul Amira, Roslinda Nazar, Kohilavani Naganthran, and Ioan Pop. 2020. "Unsteady Three-Dimensional MHD Non-Axisymmetric Homann Stagnation Point Flow of a Hybrid Nanofluid with Stability Analysis" Mathematics 8, no. 5: 784. https://doi.org/10.3390/math8050784
APA StyleZainal, N. A., Nazar, R., Naganthran, K., & Pop, I. (2020). Unsteady Three-Dimensional MHD Non-Axisymmetric Homann Stagnation Point Flow of a Hybrid Nanofluid with Stability Analysis. Mathematics, 8(5), 784. https://doi.org/10.3390/math8050784






