Regarding New Wave Patterns of the Newly Extended Nonlinear (2+1)-Dimensional Boussinesq Equation with Fourth Order
Abstract
1. Introduction
2. General Facts of SGEM
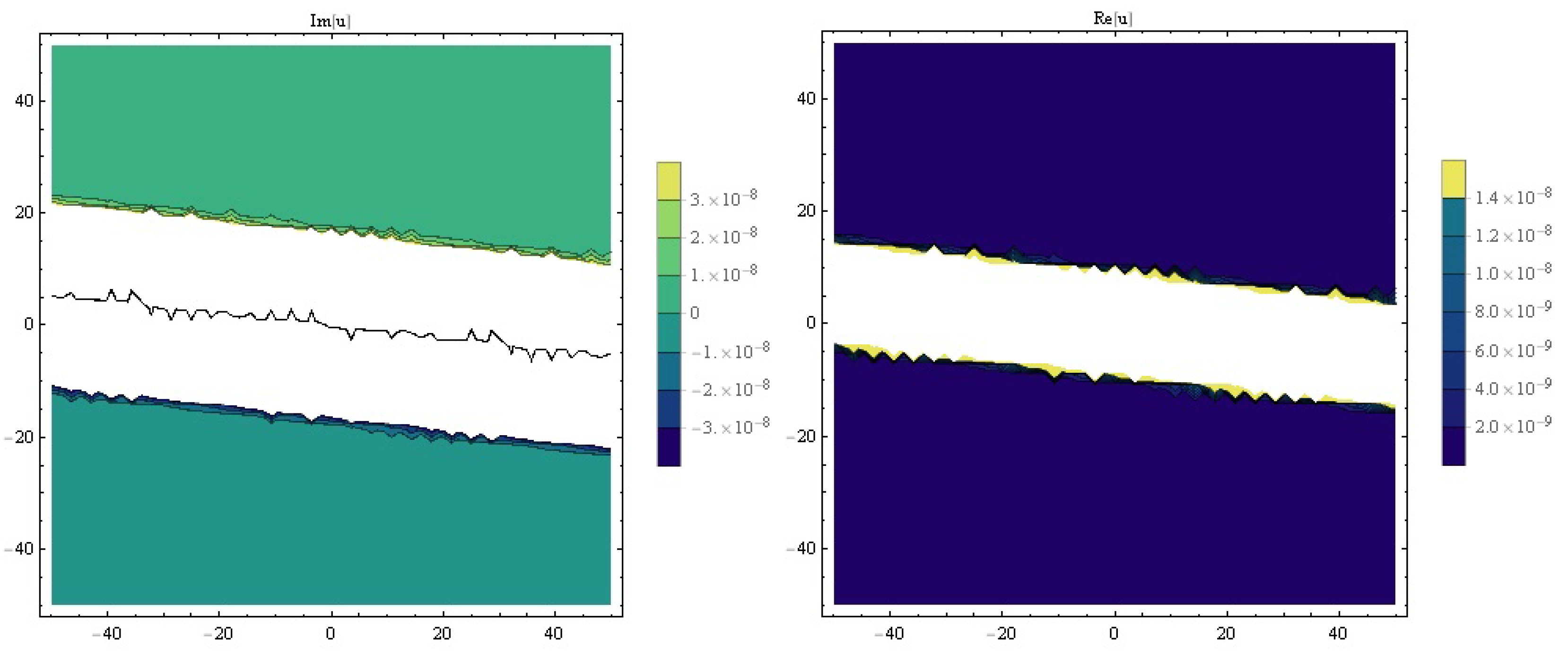
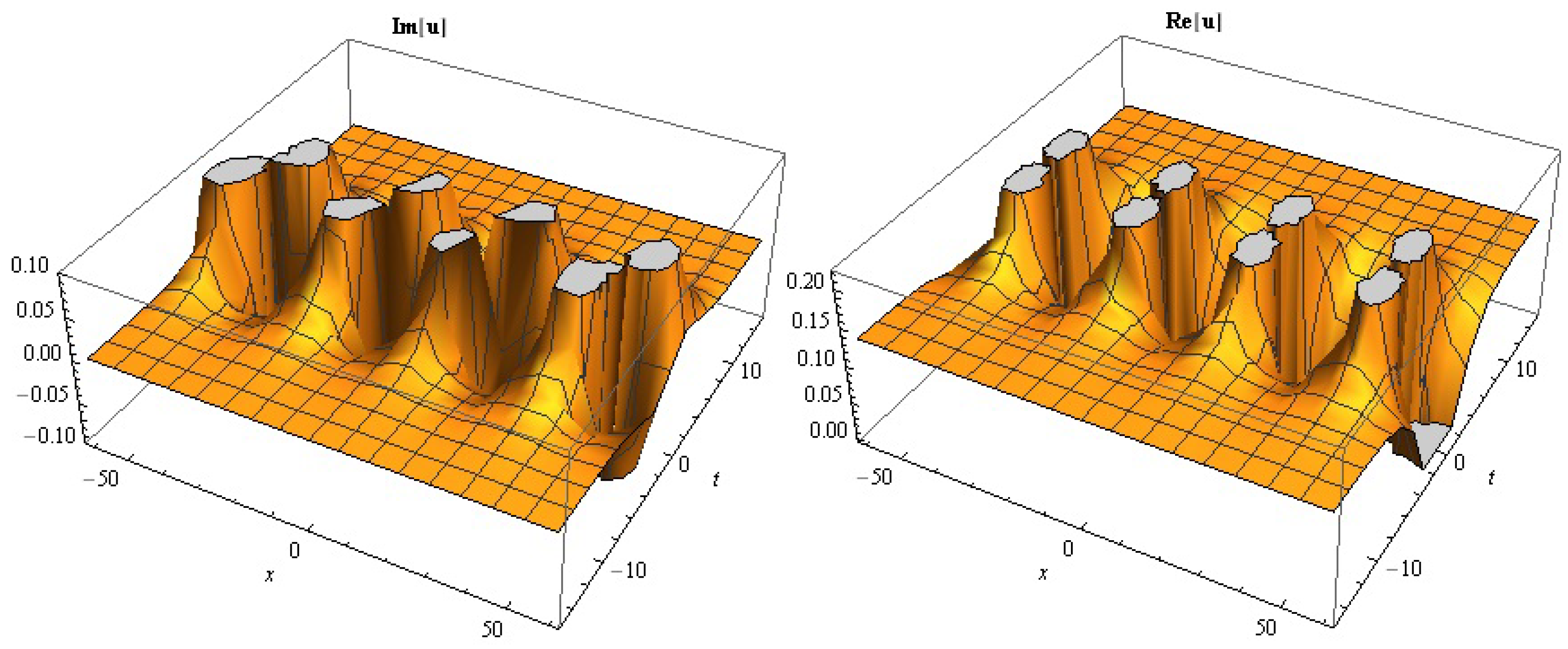
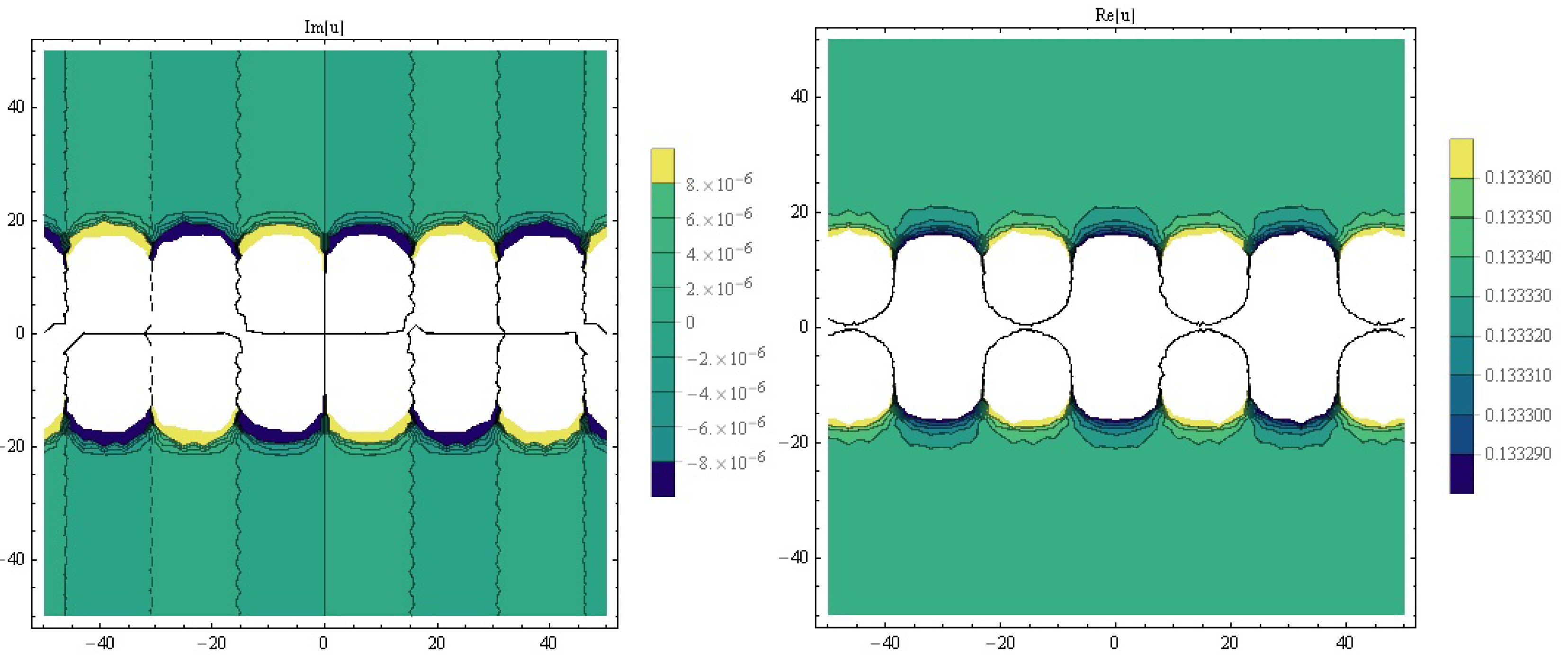
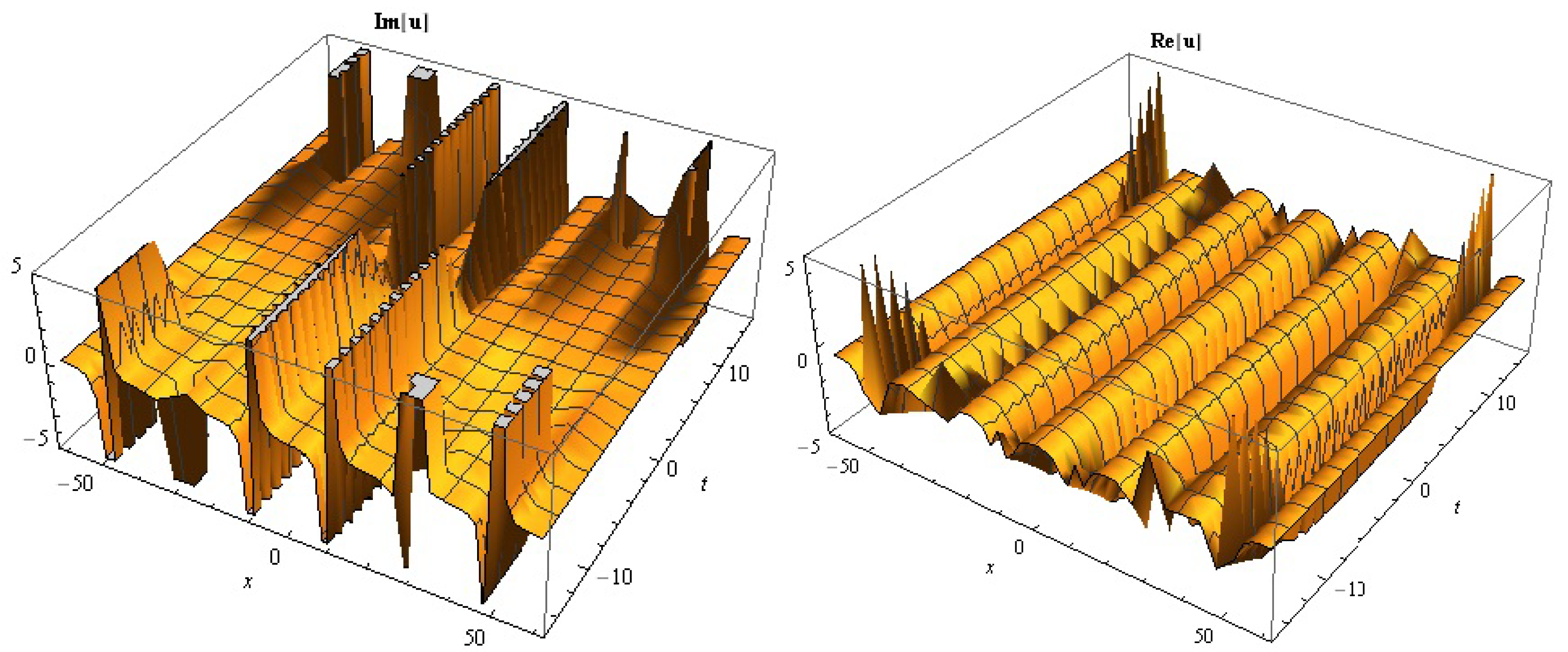
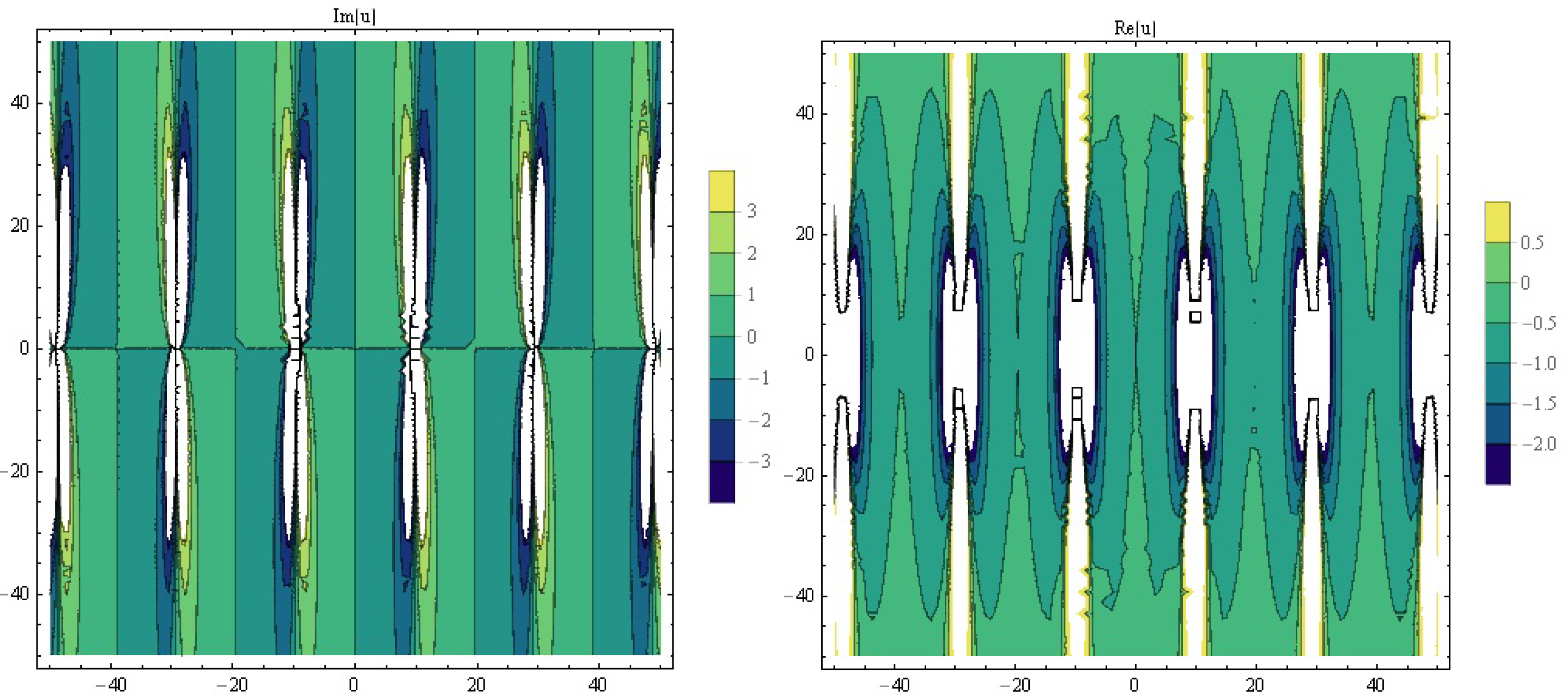
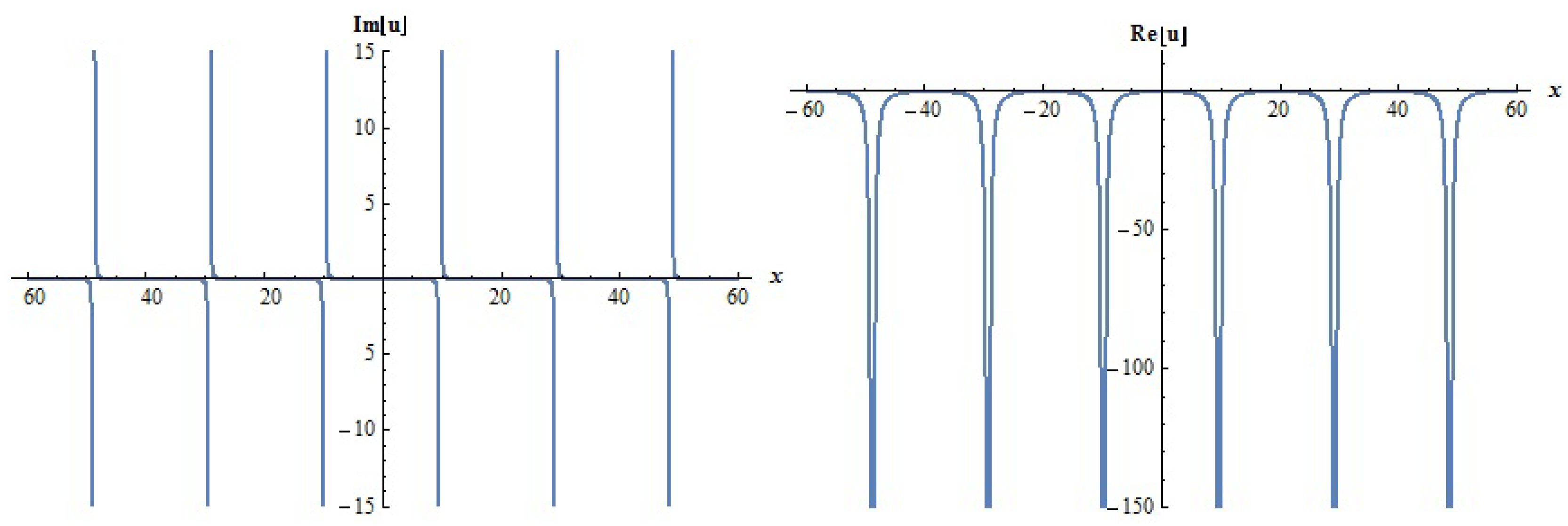
3. Application of SGEM
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wazwaz, A.M. Bright and dark optical solitons for (2+1)-dimensional Schrodinger (NLS) equations in the anomalous dispersion regimes and the normal dispersive regimes. Optik 2019, 192, 162948. [Google Scholar] [CrossRef]
- Biswas, A.; Yaard, E.; Zhoue, Q.; Seithuti, P.; Belic, M.M. Optical soliton solutions to Fokas-lenells equation using some different methods. Optik 2018, 173, 21–31. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Multiple complex and multiple real soliton solutions for the integrable sine Gordon equation. Optik 2018, 172, 622–627. [Google Scholar] [CrossRef]
- Abdullahi, A. Symbolic computation on exact solutions of a coupled KadomtsevPetviashvili equation: Lie symmetry analysis and extended tanh method. Comput. Math. Appl. 2017, 74, 1897–1902. [Google Scholar]
- Wazwaz, A.M. New (3+1)-dimensional nonlinear evolution equations with mKdV equation constituting its main part: Multiple soliton solutions. Chaos Solitons Fractals 2015, 76, 93–97. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The tanh method and the sine cosine method for solving the KP-MEW equation. Int. J. Comput. Math. 2007, 82, 235–246. [Google Scholar] [CrossRef]
- Vakhnenko, V.O.; Parkes, E.J.; Morrison, A.J. A Backlund transformation and the inverse scattering transform method for the generalised Vakhnenko equation. Chaos Solitons Fractals 2003, 17, 683–692. [Google Scholar] [CrossRef]
- Hu, C.C.; Deng, Y.S.; Tian, B.; Sun, Y.; Zhang, C.R. Rational and semi-rational solutions for the (3+1)-dimensional B-type Kadomtsev Petviashvili Boussinesq equation. Mod. Phys. Lett. B 2019, 33, 1950296. [Google Scholar]
- Eskitascioglu, E.I.; Aktas, M.B.; Baskonus, H.M. New Complex and Hyperbolic Forms for Ablowitz-Kaup-Newell-Segur Wave Equation with Fourth Order. Appl. Math. Nonlinear Sci. 2019, 4, 105–112. [Google Scholar]
- Khalique, C.M.; Mhlanga, I.E. Travelling waves and conservation laws of a (2+1)-dimensional coupling system with Korteweg-de Vries equation. Appl. Math. Nonlinear Sci. 2018, 3, 241–254. [Google Scholar] [CrossRef]
- Bibi, S.; Mohyud-Din, S.T. New traveling wave solutions of Drinfeld Sokolov Wilson Equation using Tanh and Extended Tanh methods. J. Egypt. Math. Soc. 2014, 22, 517–523. [Google Scholar] [CrossRef]
- Zayed, E.M.; Tala-Tebue, E. New Jacobi elliptic function solutions, solitons and other solutions for the (2+1)-dimensional nonlinear electrical transmission line equation. Eur. Phys. J. Plus 2018, 133, 314. [Google Scholar]
- Fan, E. Extended tanh-function method and its applications to nonlinear equations. Phys. Lett. A 2000, 277, 212–218. [Google Scholar] [CrossRef]
- Qingling, G. A Generalized Tanh Method and its Application. Appl. Math. Sci. 2011, 5, 3789–3800. [Google Scholar]
- Willy, H.; Malfliet, W. The tanh method: II. Perturbation technique for conservative systems. Phys. Scr. 1996, 54, 569–575. [Google Scholar]
- Zhang, J.L.; Mingliang, X.W. The () expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A 2008, 372, 417–423. [Google Scholar]
- Kudryashov, N.A. Traveling wave reduction of the modified KdV hierarchy: The Lax pair and the first integrals. Commun. Nonlinear Sci. Numer. Simul. 2019, 73, 472–480. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Solitary and periodic waves of the hierarchy for propagation pulse in optical fiber. Optik 2019, 194, 163060. [Google Scholar] [CrossRef]
- Pandey, P.K. Solution of two point boundary value problems, a numerical approach: Parametric difference method. Appl. Math. Nonlinear Sci. 2018, 3, 649–658. [Google Scholar] [CrossRef]
- Pandey, P.K.; Jaboob, S.S.A. A finite difference method for a numerical solution of elliptic boundary value problems. Appl. Math. Nonlinear Sci. 2018, 3, 311–320. [Google Scholar] [CrossRef]
- Raslan, R.K.; Evans, J.D. The tanh function method for solving some important non-linear partial differential equations. Int. J. Comput. Math. 2005, 82, 897–905. [Google Scholar]
- Raslan, K.R.; Ali, K.K.; Shallal, M.A. The modified extended tanh method with the Riccati equation for solving the space-time fractional EW and MEW equations. Chaos Solitons Fractals 2017, 103, 404409. [Google Scholar] [CrossRef]
- Zhong, X.; Wu, B.; Sheng, J.; Cheng, K. Generation of bright-dark soliton trains with a central wide dip in optical fibers. Optik 2018, 162, 54–60. [Google Scholar] [CrossRef]
- Yao, Y.; Ma, G.; Zhang, X.; Liu, W. M-typed dark soliton generation in optical fibers. Optik 2019, 193, 162997. [Google Scholar] [CrossRef]
- Yao, Y.; Ma, G.; Zhang, X.; Liu, W. Interactions between M-typed dark solitons in nonlinear optics. Optik 2019, 198, 163170. [Google Scholar] [CrossRef]
- Gao, Y.T.; Tian, B. Generalized hyperbolic-function method with computerized symbolic computation to construct the solitonic solutions to nonlinear equations of mathematical physics. Comput. Phys. Commun. 2001, 133, 158–164. [Google Scholar] [CrossRef]
- Zhao, Z.; Han, B. Lump solutions of a (3+1)-dimensional B-type KP equation and its dimensionally reduced equations. Anal. Math. Phys. 2019, 9, 119–130. [Google Scholar] [CrossRef]
- Zhao, Z.; He, L. Multiple lump solutions of the (3+1)-dimensional potential Yu-Toda-Sasa-Fukuyama equation. Appl. Math. Lett. 2019, 95, 114–121. [Google Scholar] [CrossRef]
- Xu, Z.W.; Yu, G.F.; Zhu, Z.N. Bright-dark soliton solutions of the multi-component AB system. Wave Motion 2018, 83, 134–147. [Google Scholar] [CrossRef]
- Hirota, R. Exact N-soliton solutions of the wave equation of long waves in shallow water and in nonlinear lattices. J. Math. Phys. 1973, 14, 810–814. [Google Scholar] [CrossRef]
- Nguyen, L.T.K. Wronskian Formulation and Ansatz Method for Bad Boussinesq Equation. Vietnam J. Math. 2016, 44, 449–462. [Google Scholar] [CrossRef]
- Nguyen, L.T.K. Soliton Solution of Good Boussinesq Equation. Vietnam J. Math. 2016, 44, 375–385. [Google Scholar] [CrossRef]
- Xia, L.C.; Ma, W.X.; Liu, X.J.; Zeng, Y.B. Wronskian solutions of the Boussinesq equation solitons, negatons, postions and complexitons. Inverse Probl. 2007, 23, 279–296. [Google Scholar]
- Nguyen, L.T.K. Modified homogeneous balance method: Applications and new solutions. Chaos Solitons Fractals 2015, 73, 148–155. [Google Scholar] [CrossRef]
- Tariq, K.U.H.; Seadawy, A.R. Bistable Bright-Dark solitary wave solutions of the (3+1)-dimensional Breaking soliton, Boussinesq equation with dual dispersion and modified Korteweg-de Vries-Kadomtsev-Petviashvili equations and their applications. Results Phys. 2017, 7, 1143–1149. [Google Scholar] [CrossRef]
- Cao, Y.; He, J.; Mihalache, D. Families of exact solutions of a new extended (2+1)-dimensional Boussinesq equation. Nonlinear Dyn. 2018, 91, 2593–2605. [Google Scholar] [CrossRef]
- Moleleki, L.D.; Khalique, C.M. Solutions and Conservation Laws of a (2+1)-Dimensional Boussinesq Equation. Abstr. Appl. Anal. 2003, 2013, 1–8. [Google Scholar] [CrossRef]
- Lu, D.; Seadawy, A.R.; Ahmed, I. Applications of mixed lump-solitons solutions and multi-peaks solitons for newly extended (2+1)-dimensional Boussinesq wave equation. Mod. Phys. Lett. B 2019, 33, 1950363. [Google Scholar] [CrossRef]
- Gao, W.; Ismael, H.F.; Husien, A.M.; Bulut, H.; Baskonus, H.M. Optical Soliton solutions of the Nonlinear Schrödinger and Resonant Nonlinear Schrödinger Equation with Parabolic Law. Appl. Sci. 2020, 10, 219. [Google Scholar] [CrossRef]
- Guirao, J.L.G.; Baskonus, H.M.; Kumar, A.; Rawat, M.S.; Yel, G. Complex Soliton Solutions to the (3+1)-Dimensional B-type Kadomtsev-Petviashvili-Boussinesq Equation. Symmetry 2020, 12, 17. [Google Scholar] [CrossRef]
- Ismael, H.F.; Bulut, H.; Baskonus, H.M. Optical soliton solutions to the Fokas-Lenells equation via sine-Gordon expansion method and (m+G’/G)-expansion method. Pramana J. Phys. 2020, 94, 35. [Google Scholar] [CrossRef]
- Weisstein, E.W. Concise Encyclopedia of Mathematics, 2nd ed.; CRC: New York, NY, USA, 2002. [Google Scholar]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
García Guirao, J.L.; Baskonus, H.M.; Kumar, A. Regarding New Wave Patterns of the Newly Extended Nonlinear (2+1)-Dimensional Boussinesq Equation with Fourth Order. Mathematics 2020, 8, 341. https://doi.org/10.3390/math8030341
García Guirao JL, Baskonus HM, Kumar A. Regarding New Wave Patterns of the Newly Extended Nonlinear (2+1)-Dimensional Boussinesq Equation with Fourth Order. Mathematics. 2020; 8(3):341. https://doi.org/10.3390/math8030341
Chicago/Turabian StyleGarcía Guirao, Juan Luis, Haci Mehmet Baskonus, and Ajay Kumar. 2020. "Regarding New Wave Patterns of the Newly Extended Nonlinear (2+1)-Dimensional Boussinesq Equation with Fourth Order" Mathematics 8, no. 3: 341. https://doi.org/10.3390/math8030341
APA StyleGarcía Guirao, J. L., Baskonus, H. M., & Kumar, A. (2020). Regarding New Wave Patterns of the Newly Extended Nonlinear (2+1)-Dimensional Boussinesq Equation with Fourth Order. Mathematics, 8(3), 341. https://doi.org/10.3390/math8030341






