All Pairs of Pentagons in Leapfrog Fullerenes Are Nice
Abstract
1. Introduction
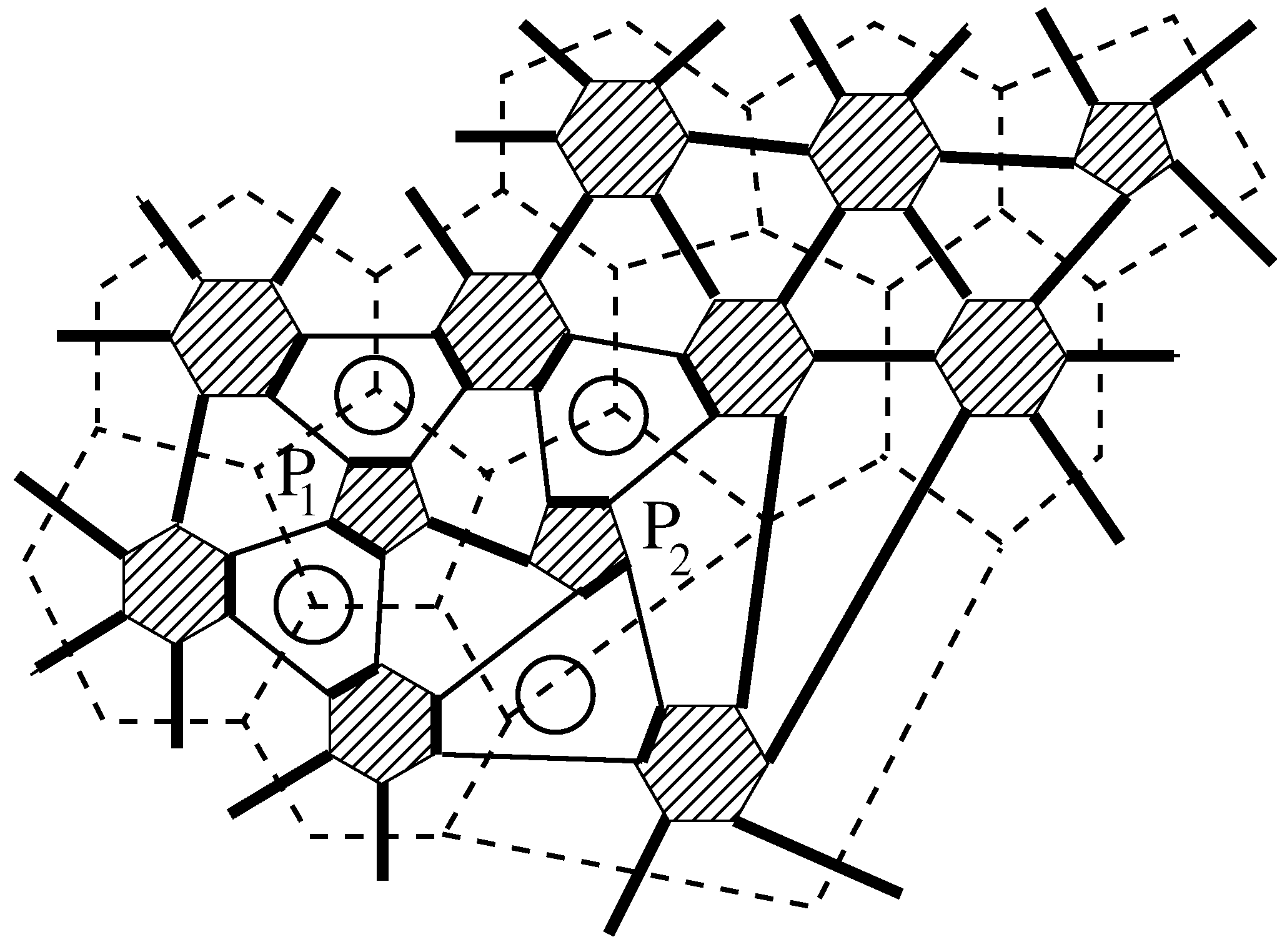
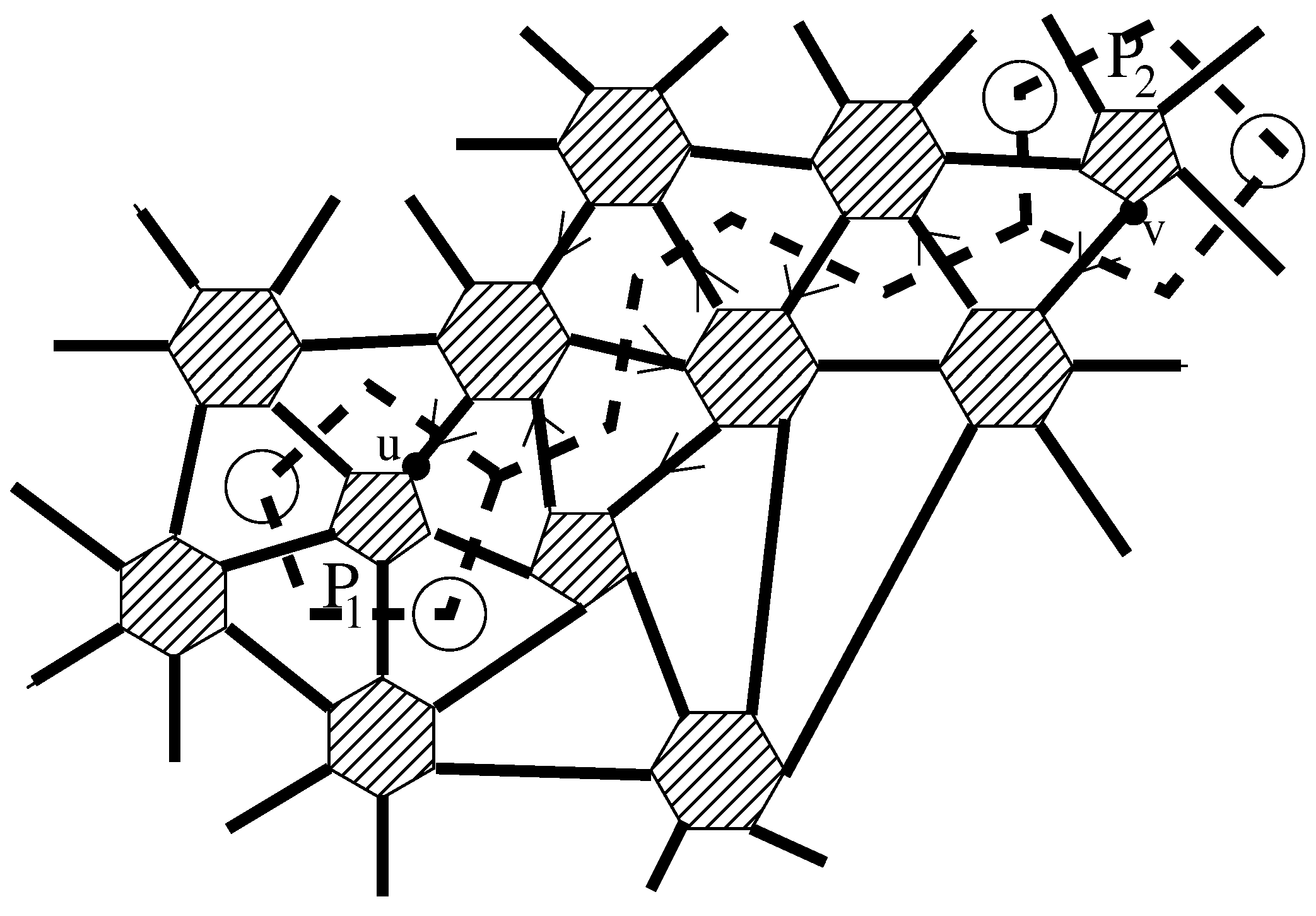
2. Definitions and Preliminary Results
3. Main Results
4. Open Questions and Concluding Remarks
Funding
Conflicts of Interest
References
- Austin, S.J.; Fowler, P.W.; Hansen, P.; Monolopoulos, D.E.; Zheng, M. Fullerene isomers of C60. Kekulé counts versus stability. Chem. Phys. Lett. 1994, 228, 478–484. [Google Scholar] [CrossRef]
- Došlić, T. Cyclical edge-connectivity of fullerene graphs and (k,6)-cages. J. Math. Chem. 2003, 33, 103–112. [Google Scholar] [CrossRef]
- Kardoš, F.; Škrekovski, R. Cyclic edge-cuts in fullerene graphs. J. Math. Chem. 2008, 44, 121–132. [Google Scholar] [CrossRef]
- Kutnar, K.; Marušič, D. On cyclic edge-connectivity of fullerenes. Discrete Appl. Math. 2008, 156, 1661–1669. [Google Scholar] [CrossRef][Green Version]
- Qi, Z.; Zhang, H. A note on the cyclical edge-connectivity of fullerene graphs. J. Math. Chem. 2008, 43, 134–140. [Google Scholar] [CrossRef]
- Došlić, T. Nice pairs of odd cycles in fullerene graphs. J. Math. Chem. 2020, 58, 2204–2222. [Google Scholar] [CrossRef]
- de Carvalho, M.H.; Lucchesi, C.L.; Murty, U.S.R. Optimal ear decomposition of matching covered graphs. J. Combin. Theory Ser. B 2002, 85, 59–93. [Google Scholar] [CrossRef]
- de Carvalho, M.H.; Lucchesi, C.L.; Murty, U.S.R. On a conjecture of Lovász concerning bricks I. The Characteristic of a Matching Covered Graph. J. Combin. Theory Ser. B 2002, 85, 94–136. [Google Scholar] [CrossRef]
- de Carvalho, M.H.; Lucchesi, C.L.; Murty, U.S.R. On a conjecture of Lovász concerning bricks II. Bricks of finite characteristic. J. Combin. Theory Ser. B 2002, 85, 137–180. [Google Scholar] [CrossRef][Green Version]
- de Carvalho, M.H.; Lucchesi, C.L.; Murty, U.S.R. The perfect matching polytope and solid bricks. J. Combin. Theory Ser. B 2004, 92, 319–324. [Google Scholar] [CrossRef]
- de Carvalho, M.H.; Lucchesi, C.L.; Murty, U.S.R. Graphs with independent perfect matchings. J. Graph Theory 2005, 48, 19–50. [Google Scholar] [CrossRef]
- de Carvalho, M.H.; Lucchesi, C.L.; Murty, U.S.R. How to build a brick. Discrete Math. 2006, 306, 2383–2410. [Google Scholar] [CrossRef]
- Harary, F. Graph Theory; Addison-Wesley: Reading, MA, USA, 1969. [Google Scholar]
- Lovász, L.; Plummer, M.D. Matching Theory; North-Holland: Amsterdam, The Netherlands, 1986. [Google Scholar]
- Grünbaum, B.; Motzkin, T.S. The number of hexagons and the simplicity of geodesics on certain polyhedra. Can. J. Math. 1963, 15, 744–751. [Google Scholar] [CrossRef]
- Fowler, P.W.; Manolopoulos, D.E. An Atlas of Fullerenes; Clarendon Press: Oxford, UK, 1995. [Google Scholar]
- Schwerdtfeger, P.; Wirz, L.N.; Avery, J. The topology of fullerenes. WIRE: Comput. Mol. Sci. 2015, 5, 96–145. [Google Scholar] [CrossRef] [PubMed]
- Petersen, J. Die Theorie der regulären graphs. Acta Math. 1891, 15, 193–220. [Google Scholar] [CrossRef]
- Došlić, T. On some structural properties of fullerene graphs. J. Math. Chem. 2002, 31, 187–195. [Google Scholar] [CrossRef]
- Li, H.; Zhang, H. The isolated-pentagon rule and nice substructures in fullerenes. Ars Math. Contemp. 2018, 15, 487–497. [Google Scholar] [CrossRef]
- Ye, D.; Zhang, H. On k-resonant fullerene graphs. SIAM J. Discrete Math. 2009, 23, 1023–1044. [Google Scholar] [CrossRef]
- Diudea, M.V.; Stefu, M.; John, P.E.; Graovac, A. Generalized operations on maps. Croat. Chem. Acta 2006, 79, 355–362. [Google Scholar]
- Došlić, T. Leapfrog fullerenes have many perfect matchings. J. Math. Chem. 2008, 44, 1–4. [Google Scholar] [CrossRef]
- Došlić, T. Finding more perfect matchings in leapfrog fullerenes. J. Math. Chem. 2009, 45, 1130–1136. [Google Scholar] [CrossRef]
- King, R.B.; Diudea, M.V. The chirality of icosahedral fullerenes: A comparison of the tripling, (leapfrog), quadrupling (chamfering) and septupling (capra) transformations. J. Math. Chem. 2006, 39, 597–604. [Google Scholar] [CrossRef]
- Thurston, W.P. Shapes of polyhedra and triangulations of the sphere. Geom. Topol. Mono. 1998, 1, 511–549. [Google Scholar]
- Cioslowski, J. Note on the asymptotic isomer count of large fullerenes. J. Math. Chem. 2014, 52, 1–5. [Google Scholar] [CrossRef]
- Došlić, T.; Dehkordi, M.T.; Fath-Tabar, G.H. Packing stars in fullerenes. J. Math. Chem. 2020, 58, 2223–2244. [Google Scholar] [CrossRef]
- Nagy, P.; Ehlich, R.; Biró, L.P.; Gyulai, J. Y-branching of single walled carbon nanotubes. Appl. Phys. A 2000, 70, 481–483. [Google Scholar] [CrossRef]
- Astakhova, T.Y.; Vinogradov, G.A. Fullerene notation and isomerization operations. Fullerene Sci. Tech. 1997, 5, 1545–1562. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ori, O. Skeletal rearrangements of the C240 fullerene: Efficient topological descriptors for monitoring Stone-Wales transformations. Mathematics 2020, 8, 968. [Google Scholar] [CrossRef]
- Ori, O.; Cataldo, F. Moving pentagons on nanocones. Fuller. Nanotub. Carbon Nanostruct. 2020, 28, 732–736. [Google Scholar] [CrossRef]
- Diudea, M.V.; Nagy, C.L.; Ursu, O.; Balaban, T.S. C60 dimers revisited. Fuller. Nanotub. Carbon Nanostruct. 2003, 11, 245–255. [Google Scholar] [CrossRef]
- Gadomski, A. Three types of computational soft-matter problems revisited, an own-selection-based opinion. Front. Phys. 2014, 2, 36. [Google Scholar] [CrossRef][Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Došlić, T. All Pairs of Pentagons in Leapfrog Fullerenes Are Nice. Mathematics 2020, 8, 2135. https://doi.org/10.3390/math8122135
Došlić T. All Pairs of Pentagons in Leapfrog Fullerenes Are Nice. Mathematics. 2020; 8(12):2135. https://doi.org/10.3390/math8122135
Chicago/Turabian StyleDošlić, Tomislav. 2020. "All Pairs of Pentagons in Leapfrog Fullerenes Are Nice" Mathematics 8, no. 12: 2135. https://doi.org/10.3390/math8122135
APA StyleDošlić, T. (2020). All Pairs of Pentagons in Leapfrog Fullerenes Are Nice. Mathematics, 8(12), 2135. https://doi.org/10.3390/math8122135




