Designing Limit-Cycle Suppressor Using Dithering and Dual-Input Describing Function Methods
Abstract
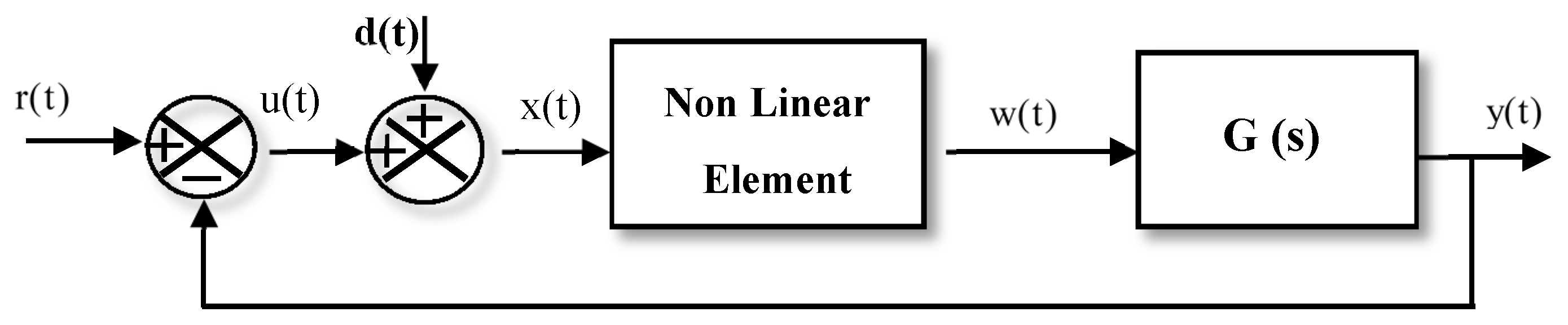
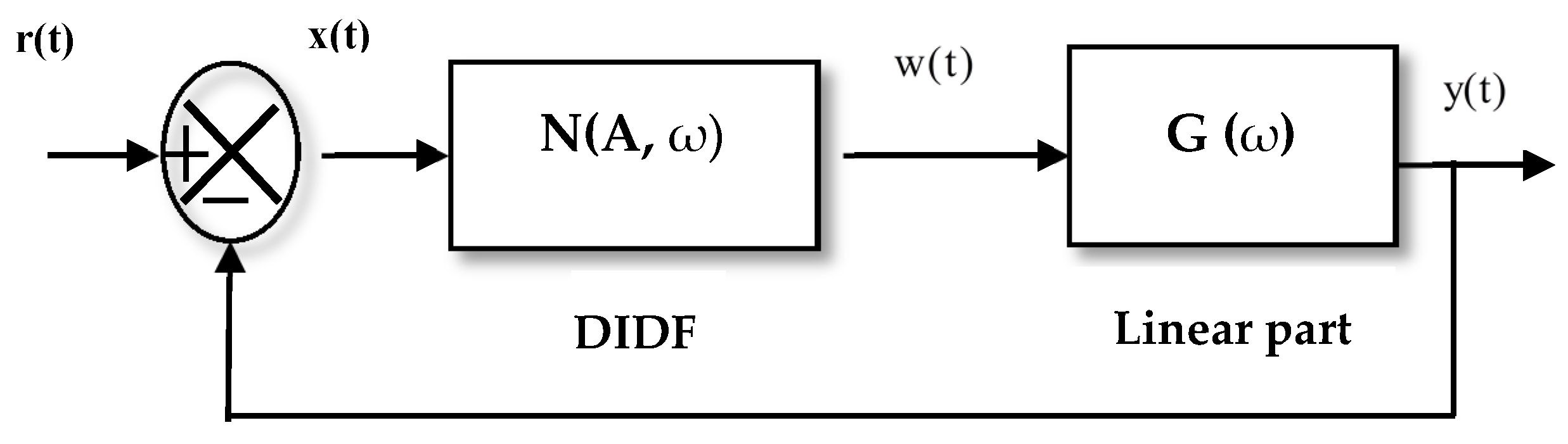
1. Introduction
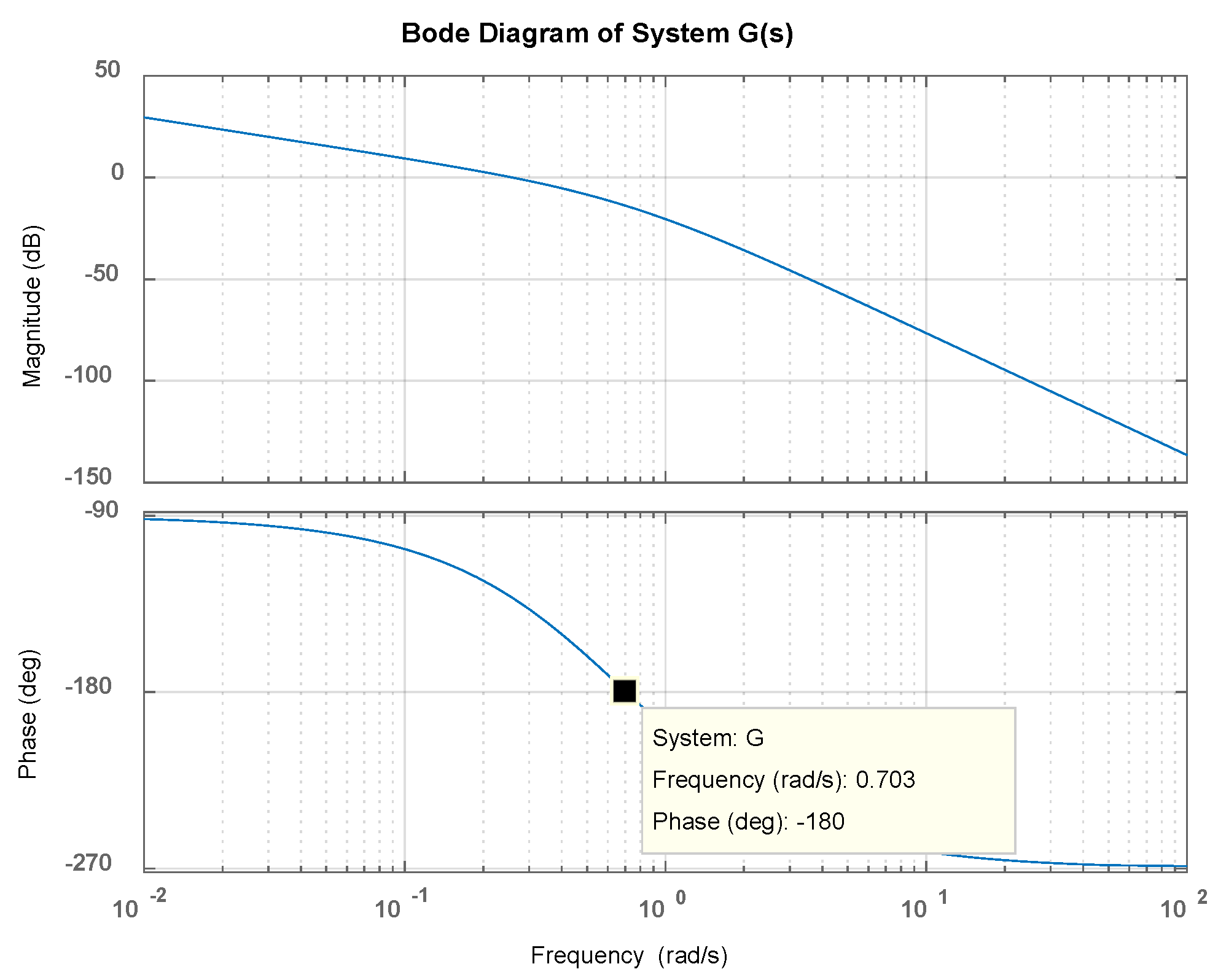
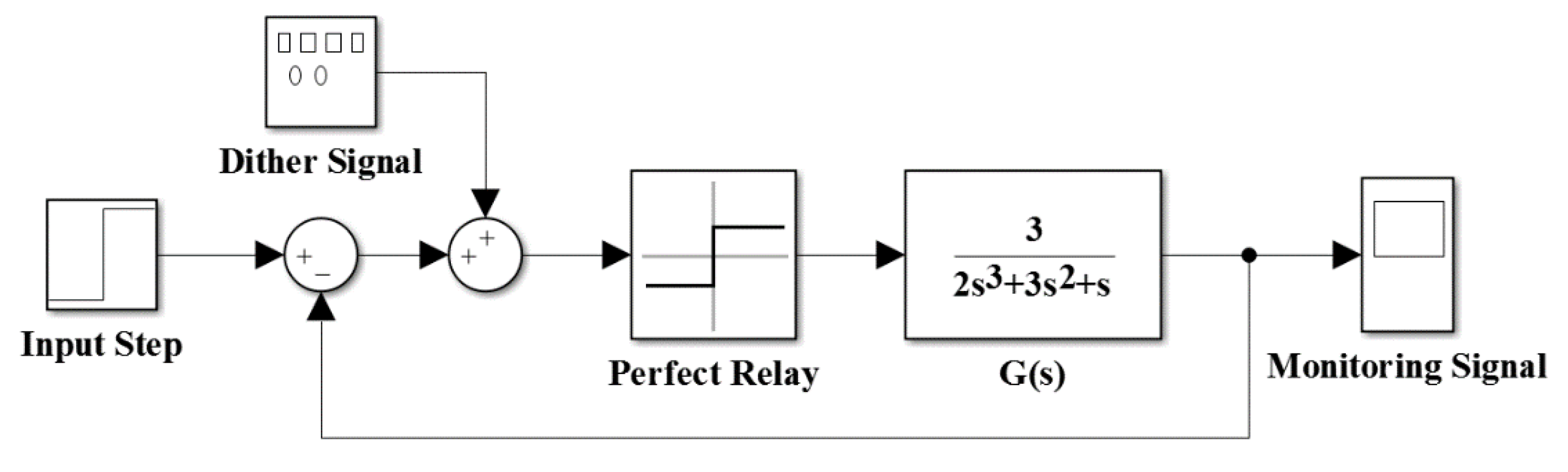
2. Method
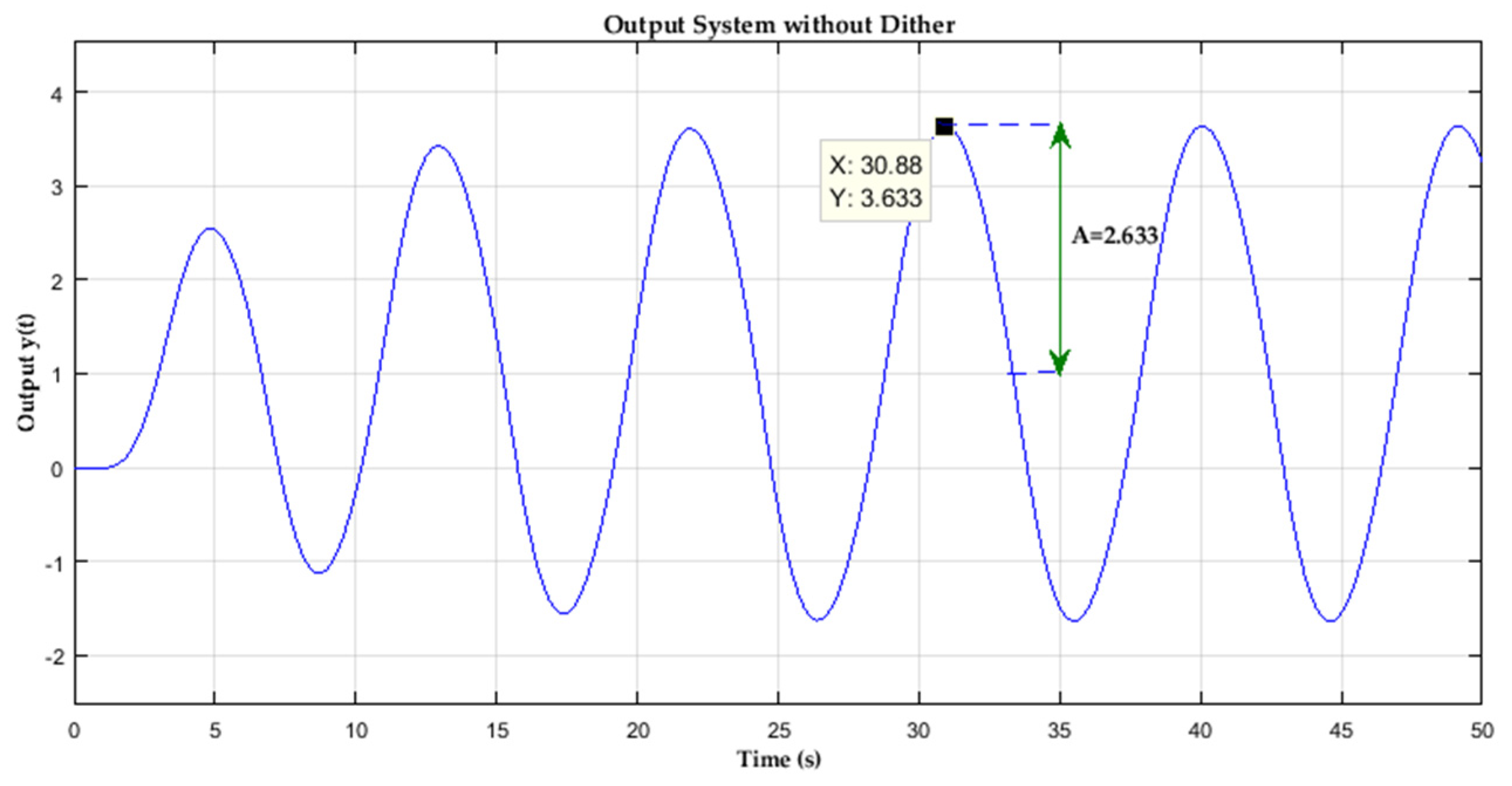
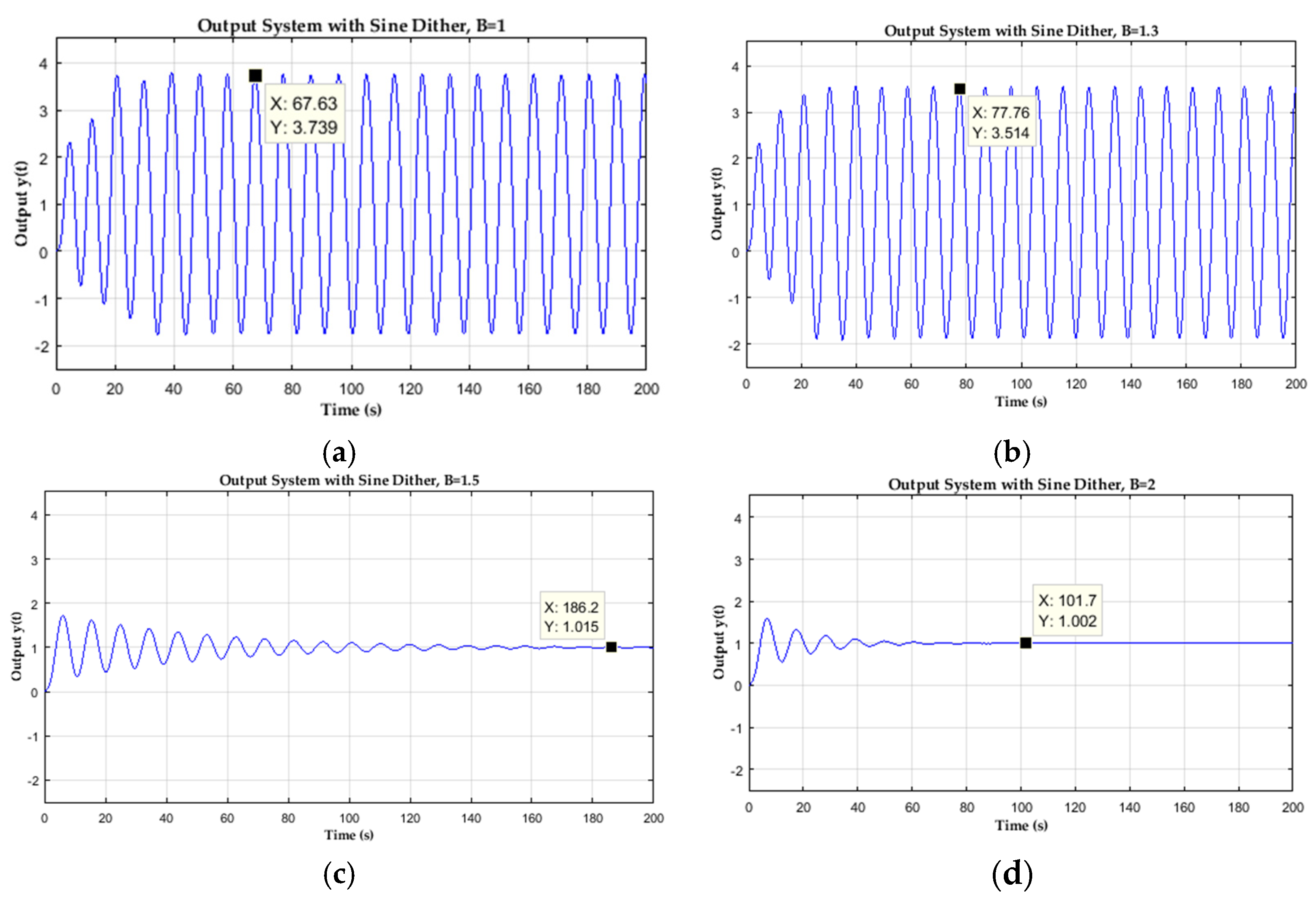
3. Results and Discussion
4. Conclusions and Future Works
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| e | Input to a nonlinear element |
| k | The modulus in elliptic integrals |
| t | time |
| y | Output of nonlinear element |
| ω | Frequency of limit cycle |
| φ | Phase angles |
| A | Amplitude of the limit cycle |
| B | Amplitude of dither signal |
| Bmin | The minimum of dither amplitude |
| E | Complete elliptic integral of the second kind |
| F | Complete elliptic integral of the first kind |
| M | Parameter associated with nonlinear element |
| N(A,B) | DIDF |
| N(A,B)|c | Critical line of DIDF |
| N(A,B)|max | The peak value of DIDF curve |
References
- Huang, B.; Yap, C. An algorithmic approach to small limit cycles of nonlinear differential systems: The averaging method revisited. J. Symb. Comput. 2020. [Google Scholar] [CrossRef]
- Yang, J. Limit cycles appearing from the perturbation of differential systems with multiple switching curves. Chaos Solitons Fractals 2020, 135, 109764. [Google Scholar] [CrossRef]
- Balajewicz, M.; Dowell, E. Reduced-order modeling of flutter and limit-cycle oscillations using the sparse Volterra series. J. Aircr. 2012, 49, 1803–1812. [Google Scholar] [CrossRef]
- Slotine, J.-J.E.; Li, W. Nonlinear system analysis. In Applied Nonlinear Control; Prentice Hall: Englewood Cliffs, NJ, USA, 1991; Volume 199. [Google Scholar]
- Hayes, R.; Marques, S.P. Prediction of limit cycle oscillations under uncertainty using a harmonic balance method. Comput. Struct. 2015, 148, 1–13. [Google Scholar] [CrossRef]
- Shukla, H.; Patil, M.J. Controlling limit cycle oscillation amplitudes in nonlinear aeroelastic systems. J. Aircr. 2017, 54, 1921–1932. [Google Scholar] [CrossRef]
- Zhang, G.; Wu, Z. Approximate limit cycles of coupled nonlinear oscillators with fractional derivatives. Appl. Math. Model. 2020, 77, 1294–1309. [Google Scholar] [CrossRef]
- Al-Moqbali, M.K.; Al-Salti, N.S.; Elmojtaba, I.M. Prey-predator models with variable carrying capacity. Mathematics 2018, 6, 102. [Google Scholar] [CrossRef]
- Denimal, E.; Sinou, J.-J.; Nacivet, S. Generalized Modal Amplitude Stability Analysis for the prediction of the nonlinear dynamic response of mechanical systems subjected to friction-induced vibrations. Nonlinear Dyn. 2020, 100, 1–24. [Google Scholar] [CrossRef]
- Taylor, J.H. Describing functions. In Electrical Engineering Encyclopedia; John Wiley & Sons: New York, NY, USA, 1999. [Google Scholar]
- Zare, S.; Tavakolpour-Saleh, A.; Sangdani, M. Investigating limit cycle in a free piston Stirling engine using describing function technique and genetic algorithm. Energy Convers. Manag. 2020, 210, 112706. [Google Scholar] [CrossRef]
- Xia, Y.; Laera, D.; Jones, W.P.; Morgans, A.S. Numerical prediction of the Flame Describing Function and thermoacoustic limit cycle for a pressurised gas turbine combustor. Combust. Sci. Technol. 2019, 191, 979–1002. [Google Scholar] [CrossRef]
- Maraini, D.; Nataraj, C. Nonlinear analysis of a rotor-bearing system using describing functions. J. Sound Vib. 2018, 420, 227–241. [Google Scholar] [CrossRef]
- Yang, Z.; Li, H.; Liu, C.; Ding, Y.; Zhang, B. Accurate modeling and stability analysis for chaotic PWM boost converters based on describing function method. In Proceedings of the IECON 2019—45th Annual Conference of the IEEE Industrial Electronics Society, Lisbon, Portugal, 14–17 September 2019; pp. 1585–1590. [Google Scholar]
- Namadchian, Z.; Zare, A. Stability analysis of dynamic nonlinear interval type-2 TSK fuzzy control systems based on describing function. Soft Comput. 2020, 24, 1–14. [Google Scholar] [CrossRef]
- Shukla, H.; Patil, M.J. Nonlinear state feedback control design to eliminate subcritical limit cycle oscillations in aeroelastic systems. Nonlinear Dyn. 2017, 88, 1599–1614. [Google Scholar] [CrossRef]
- Girija, S.; Narayanasamy, S.; Kurian, T. Method to eliminate the limit cycle oscillation for digitally controlled DC–DC converter using reduced state Kalman filter. IET Power Electron. 2016, 9, 2445–2452. [Google Scholar] [CrossRef]
- Kumar, A.; Ali, S.F.; Friswell, M.I.; Arockiarajan, A. Creation and stabilization of limit cycles in chaotic attractors through closure of orbits. In Proceedings of the 11th Asian Control Conference (ASCC), Gold Coast, Australia, 17–20 December 2017; pp. 653–658. [Google Scholar]
- Antonio-Cruz, M.; Hernández-Guzmán, V.M.; Silva-Ortigoza, R.; Silva-Ortigoza, G. Implementation of a controller to eliminate the limit cycle in the inverted pendulum on a cart. Complex 2019, 2019, 13. [Google Scholar] [CrossRef]
- Oldenburger, R.; Nakada, T. Signal stabilization of self-oscillating systems. IRE Trans. Autom. Control 1961, 6, 319–325. [Google Scholar] [CrossRef]
- Yang, H.; Tao, W.; Zhang, Z.; Zhao, S.; Yin, X.; Zhao, H. Reduction of the influence of laser beam directional dithering in a laser triangulation displacement probe. Sensors 2017, 17, 1126. [Google Scholar] [CrossRef]
- Duan, Y.; Chen, T.; Chen, D. Low-cost dithering generator for accurate ADC linearity test. In Proceedings of the 2016 IEEE International Symposium on Circuits and Systems (ISCAS), Montreal, QC, Canada, 22–25 May 2016; pp. 1474–1477. [Google Scholar]
- Tao, Y.; Li, S.; Zhou, G.; Lin, J. Mechanical dither control optimization for laser gyro with total reflection prisms. In Proceedings of the 2017 24th Saint Petersburg International Conference on Integrated Navigation Systems (ICINS), Saint Petersburg, Russia, 29–31 May 2017; pp. 1–7. [Google Scholar]
- Zhang, S.; Li, T.; Jin, L.; Yang, J.; He, L.; Lin, F. A 11-bit 1.2 V 40.3 μW SAR ADC with Self-Dithering Technique, 2016 IEEE MTT-S International Wireless Symposium (IWS); IEEE: New York, NJ, USA, 2016; pp. 1–4. [Google Scholar]
- Zhu, H.; Fujimoto, H. Suppression of current quantization effects for precise current control of SPMSM using dithering techniques and Kalman filter. IEEE Trans. Ind. Inform. 2014, 10, 1361–1371. [Google Scholar]
- Wang, X.; Suh, C.S. Nonlinear time-frequency control of PM synchronous motor instability applicable to electric vehicle application. Int. J. Dyn. Control 2016, 4, 400–412. [Google Scholar] [CrossRef]
- Behera, R.K.; Das, S.P. High performance induction motor drive: A dither injection technique. In Proceedings of the 2011 International Conference on Energy, Automation and Signal, Bhubaneswar, India, 28–30 December 2011; pp. 1–6. [Google Scholar]
- Behera, R.; Das, S. Improved direct torque control of induction motor with dither injection. Sadhana 2008, 33, 551–564. [Google Scholar] [CrossRef]
- Kadjoudj, M.; Taibi, S.; Golea, N.; Benbouzid, M. Modified direct torque control of PMSM drives using dither signal injection and non-hysteresis controllers. Electromotion 2006, 13, 262–270. [Google Scholar]
- Chang, S.-C. Quenching chaos of a magnetically levitated system based on dither signals. Appl. Math. Comput. 2013, 222, 539–547. [Google Scholar] [CrossRef]
- Chang, S.-C. Stability analysis, routes to chaos, and quenching chaos in electromechanical valve actuators. Math. Comput. Simul. 2020, 177, 140–151. [Google Scholar] [CrossRef]
- Lue, Y.-F.; Chang, S.-C. Non-linear dynamics and control of an automotive suspension system based on local and global bifurcation analysis. Int. J. Veh. Auton. Syst. 2017, 13, 340–359. [Google Scholar] [CrossRef]
- Gibson, J.; Sridhar, R. A new dual-input describing function and an application to the stability of forced nonlinear systems. IEEE Trans. Appl. Ind. 1963, 82, 65–70. [Google Scholar] [CrossRef]
- Chen, S.-C.; Tsai, H.-H. The Performance of different dither signals in nonlinear systems. Mod. Phys. Lett. B 2009, 23, 2507–2520. [Google Scholar] [CrossRef]
- Raafat, S.M.; Ali, S.S. The selection of dither signal in extremum seeking control of 3 DOF helicopter system. In Proceedings of the Zaytoonah University International Engineering Conference on Design and Innovation in Sustainability, Amman, Jordan, 13–15 May 2014; pp. 13–15. [Google Scholar]
- West, J.; Douce, J.; Livesley, R. The dual-input describing function and its use in the analysis of non-linear feedback systems. Proc. IEEE Part B Radio Electron. Eng. 1956, 103, 463–473. [Google Scholar] [CrossRef]
- Kasi, V.R.; Majhi, S.; Gogoi, A.K. Estimation of stable FOPDT and SOPDT process using dual input describing function. In Proceedings of the 11th International Conference on Industrial and Information Systems (ICIIS), Roorkee, India, 3–4 December 2016; pp. 810–814. [Google Scholar]
- Choe, Y.W. Performance Improvement for PID Controllers by using Dual-Input Describing Function (DIDF) Method. Trans. Korean Inst. Electr. Eng. 2011, 60, 1741–1747. [Google Scholar] [CrossRef][Green Version]
- Choe, Y.W. Tuning PID controllers for unstable systems with dead time based on dual-input describing function method. In AETA 2015: Recent Advances in Electrical Engineering and Related Sciences; Springer: Berlin, Germany, 2016; pp. 427–439. [Google Scholar]
- Brito, A.G. Computation of multiple limit cycles in nonlinear control systems-a describing function approach. J. Aerosp. Technol. Manag. 2011, 3, 21–28. [Google Scholar] [CrossRef]
- Welford, G.D. Dual input describing functions. In Army Missile Research Development and Engineering Lab Redstone Arsenal al Guidance and Control Directorate; Army Missile Command: Redstone Arsenal, AL, USA, 1969. [Google Scholar]
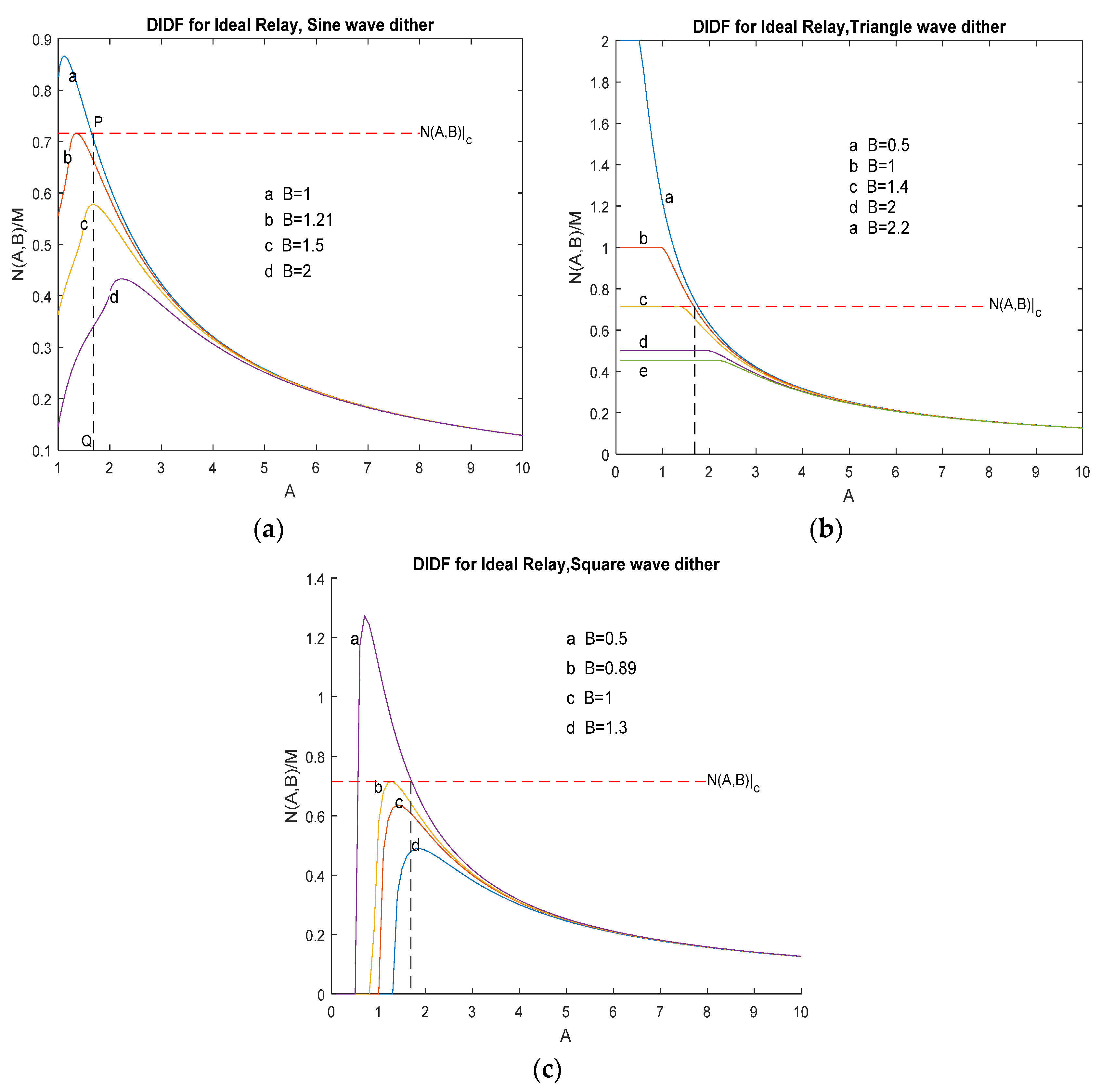


| Dither Signal | |
|---|---|
| Sine | 0.85 |
| Triangle | 1 |
| Square | 0.64 |
| Dither Signal | |
|---|---|
| Sine | >1.2 |
| Triangle | >1.4 |
| Square | >0.89 |
| Dither Shape | Predicted | Simulation | ||
|---|---|---|---|---|
| A | A | |||
| Sine | 1.69 | >1.22 | 2.739 | ≥1.5 |
| Triangle | 1.69 | >1.4 | 2.753 | ≥2.4 |
| Square | 1.69 | >0.89 | 2.234 | ≥1.065 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mbitu, E.T.; Chen, S.-C. Designing Limit-Cycle Suppressor Using Dithering and Dual-Input Describing Function Methods. Mathematics 2020, 8, 1978. https://doi.org/10.3390/math8111978
Mbitu ET, Chen S-C. Designing Limit-Cycle Suppressor Using Dithering and Dual-Input Describing Function Methods. Mathematics. 2020; 8(11):1978. https://doi.org/10.3390/math8111978
Chicago/Turabian StyleMbitu, Elisabeth Tansiana, and Seng-Chi Chen. 2020. "Designing Limit-Cycle Suppressor Using Dithering and Dual-Input Describing Function Methods" Mathematics 8, no. 11: 1978. https://doi.org/10.3390/math8111978
APA StyleMbitu, E. T., & Chen, S.-C. (2020). Designing Limit-Cycle Suppressor Using Dithering and Dual-Input Describing Function Methods. Mathematics, 8(11), 1978. https://doi.org/10.3390/math8111978




