Scale Mixture of Rayleigh Distribution
Abstract
1. Introduction
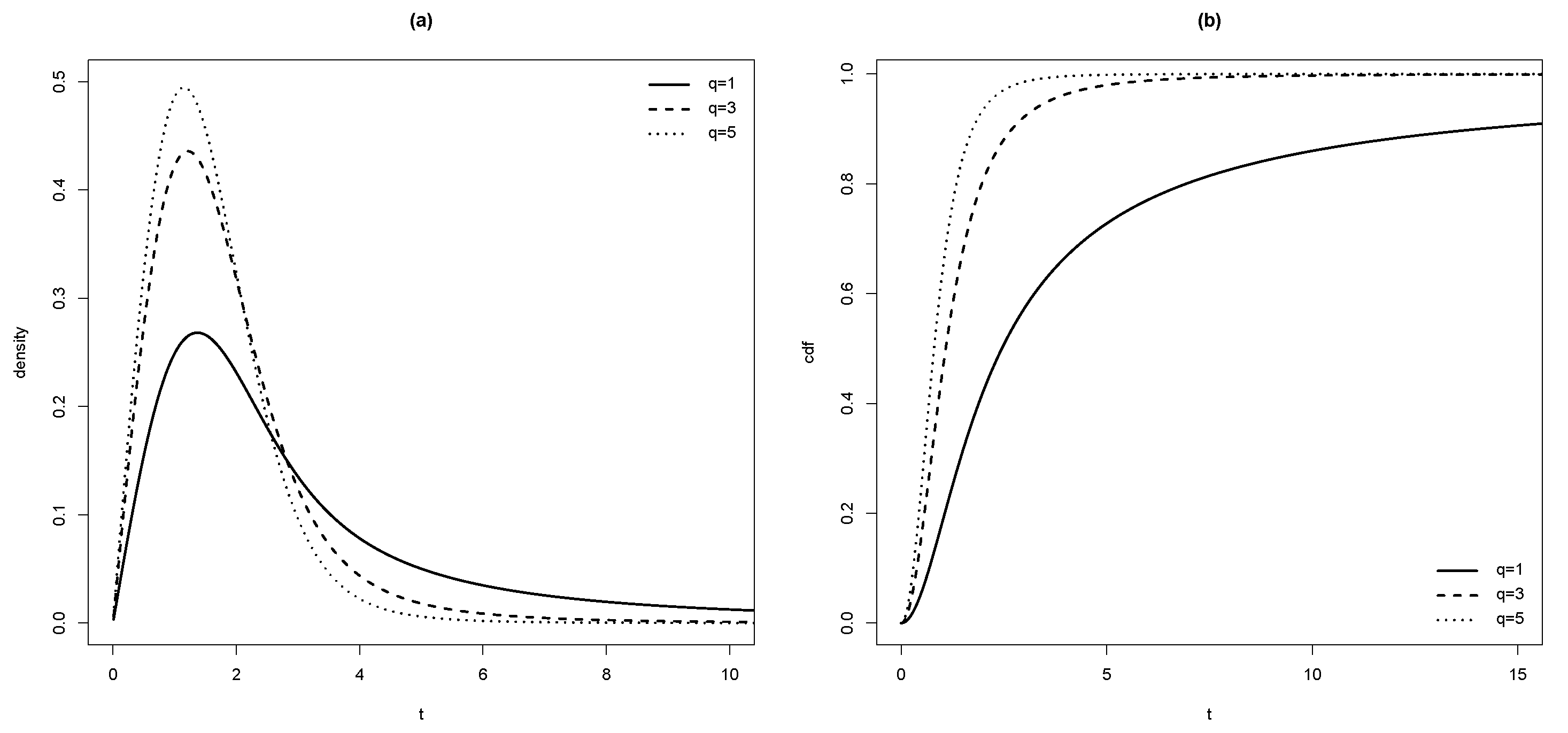
2. Definition and Properties
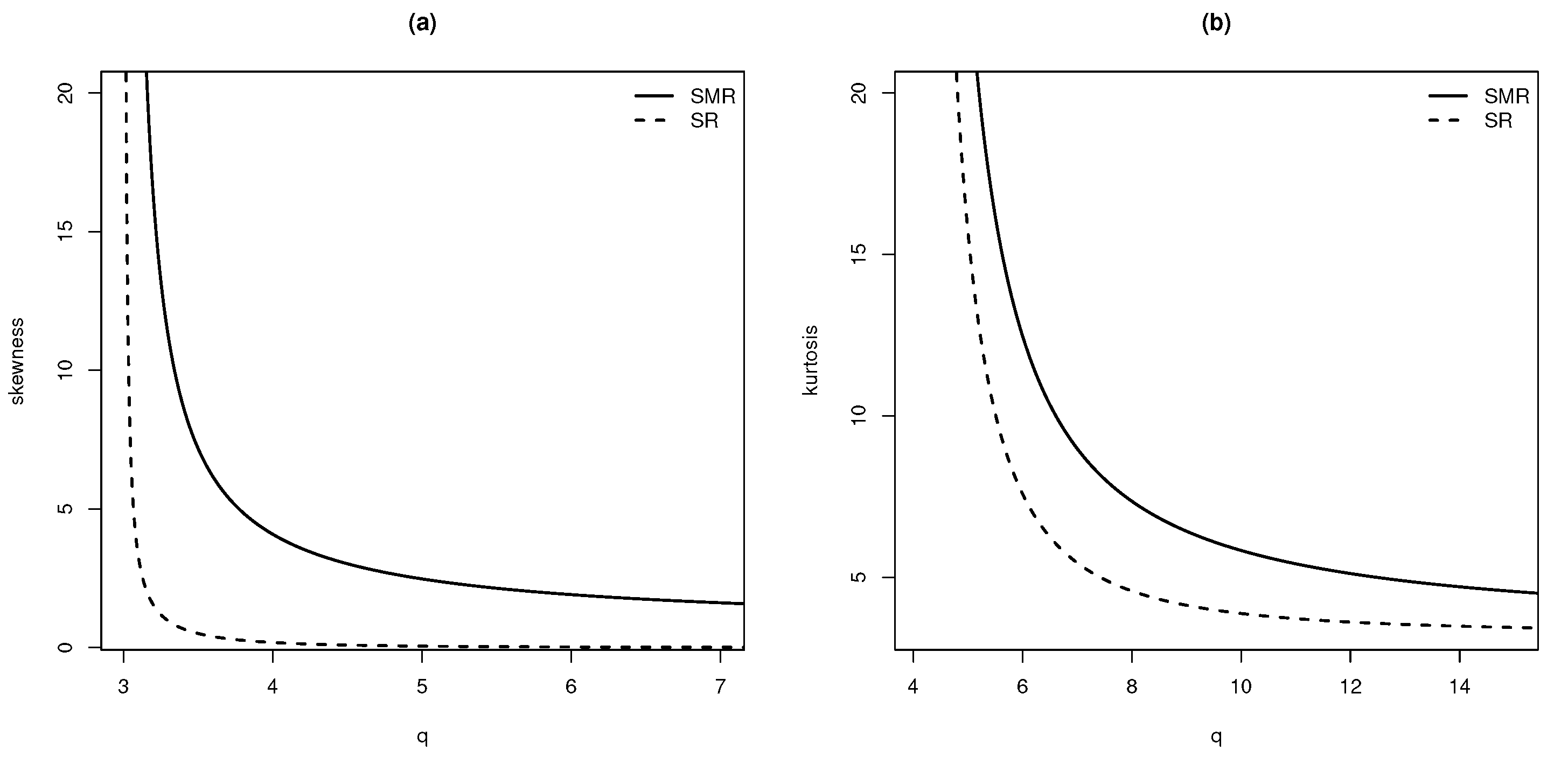
Moments
3. Lifetime Analysis
- 1.
- The survival function is , .
- 2.
- The hazard function is
Mean Residual Life
Order Statistics
4. Entropy
5. Inference
5.1. Moment Method Estimators
5.2. ML Estimation
5.3. ML Estimation Using EM-Algorithm
- 1.
- , , with pdf given in (2).
- 2.
- where denotes the digamma function.
- E-step: For compute
- M-step: Update the vector of parameters
- E and M steps are repeated until a suitable convergence is reached.
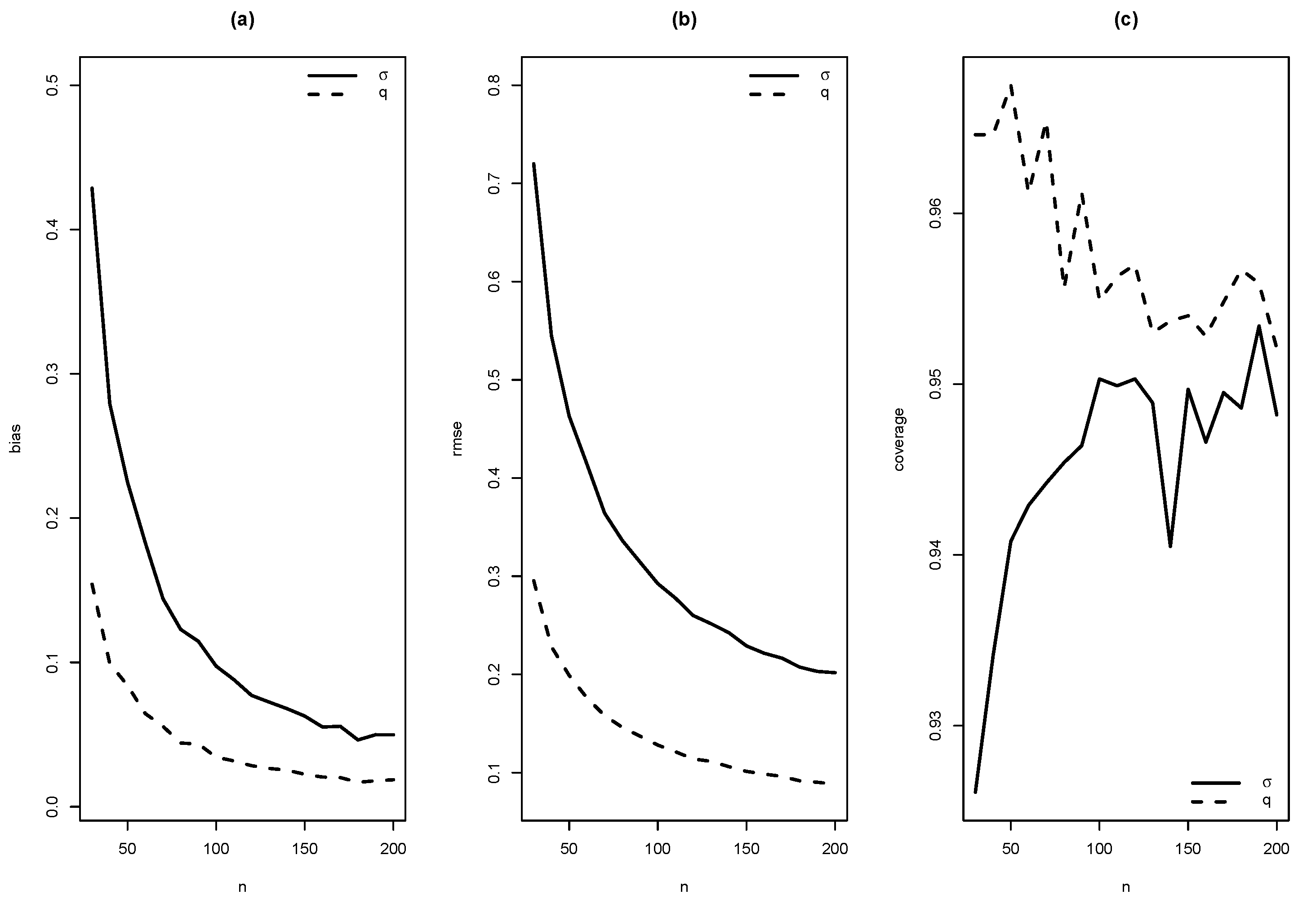
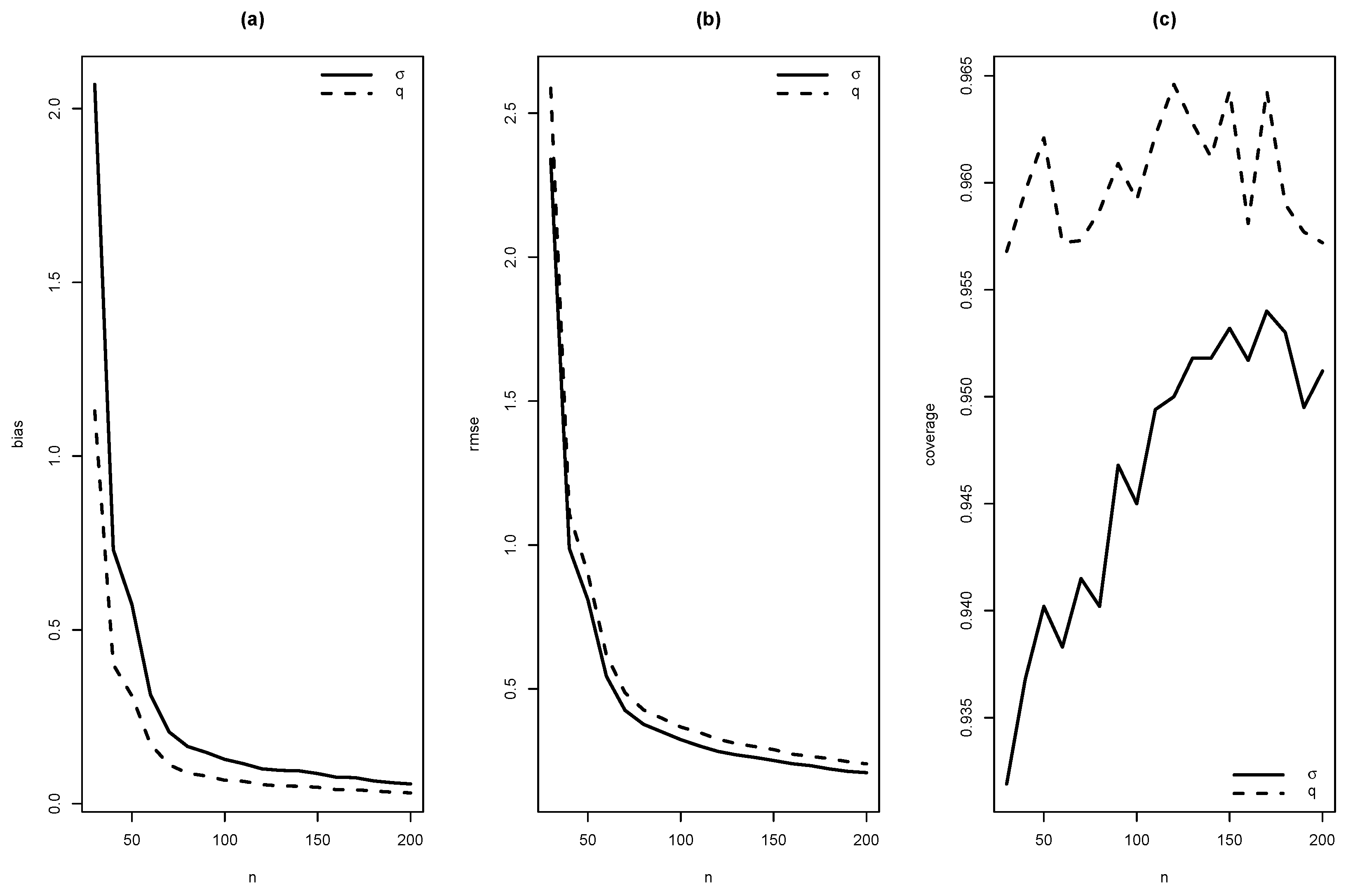
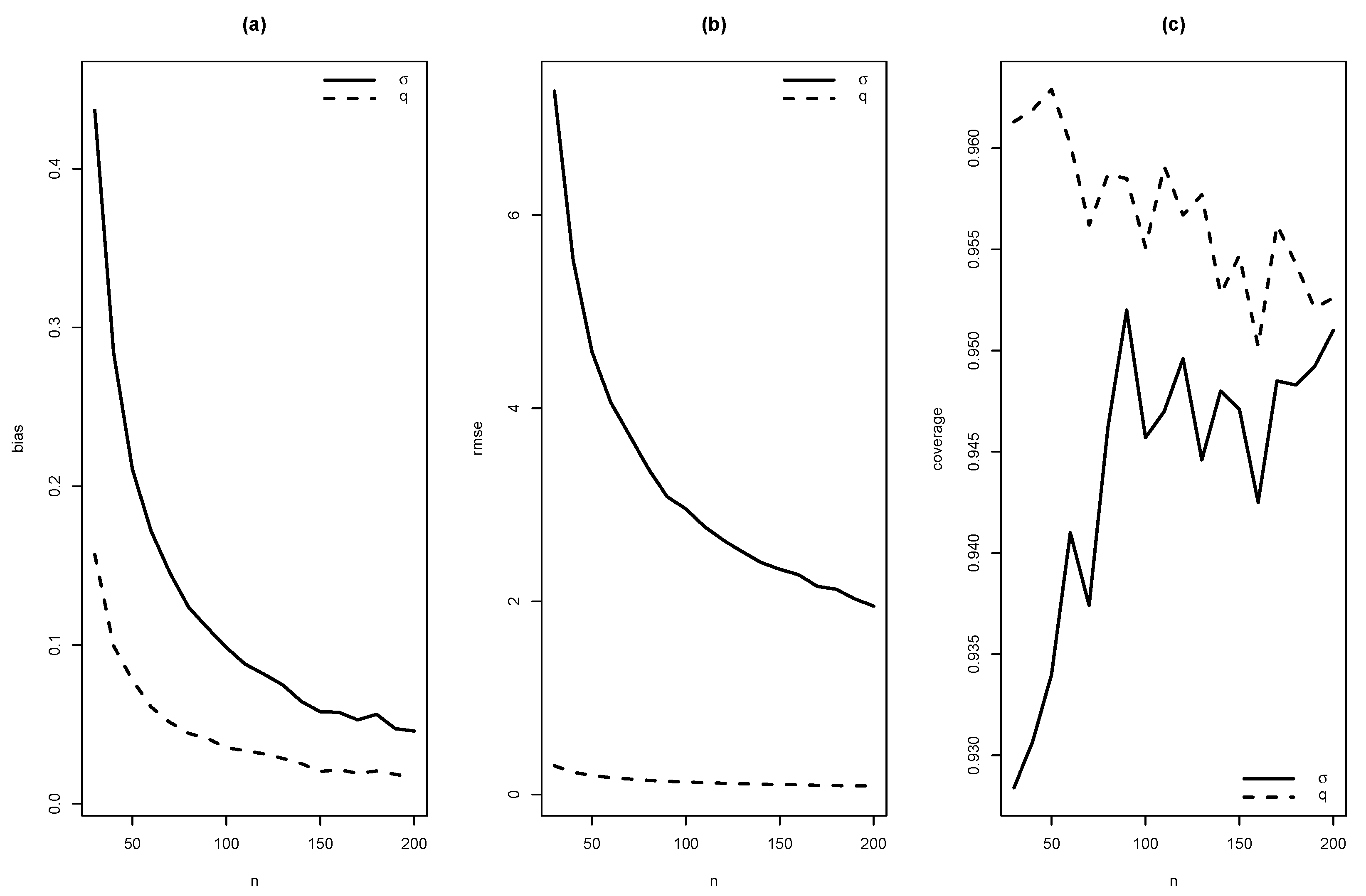
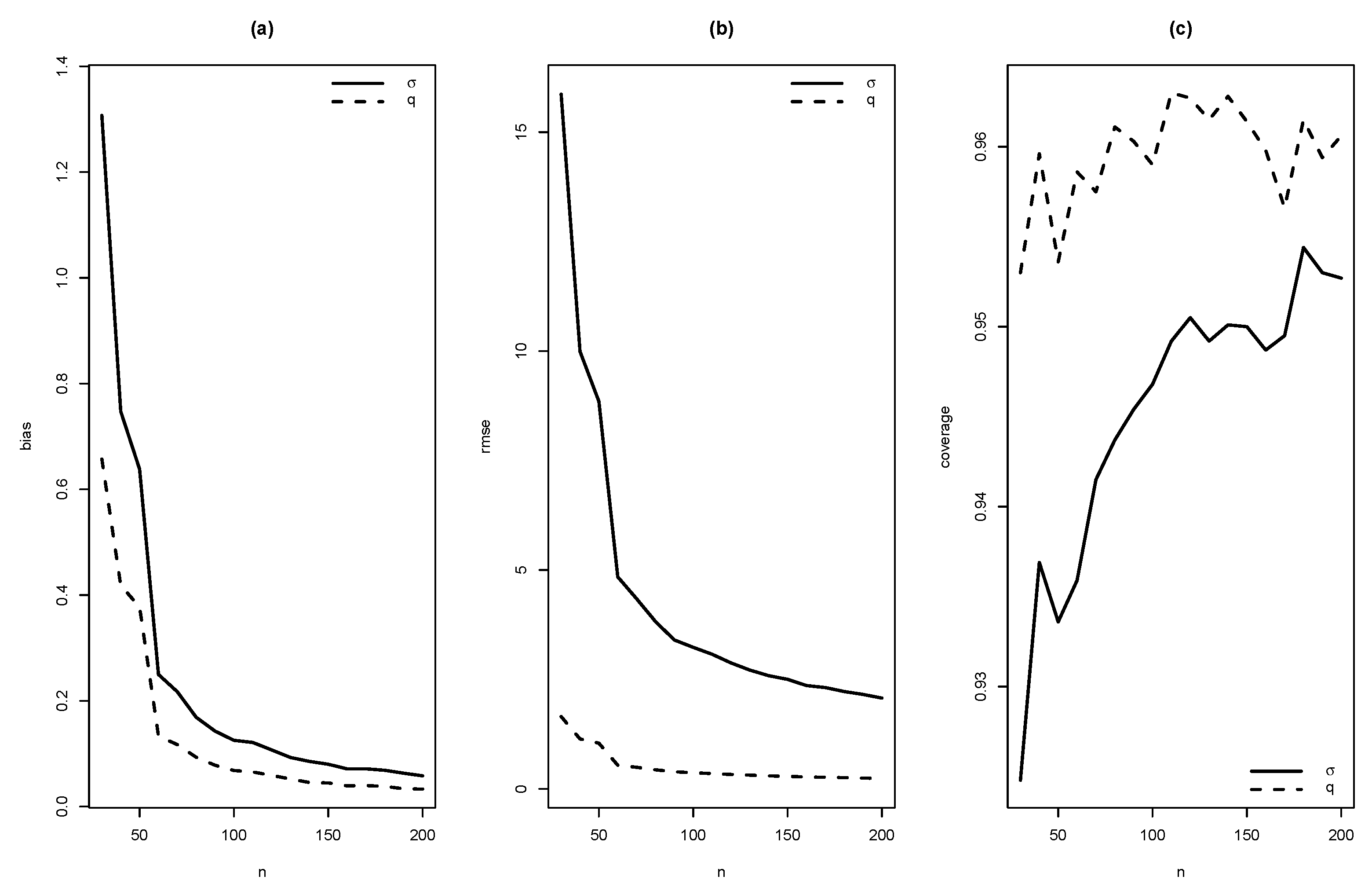
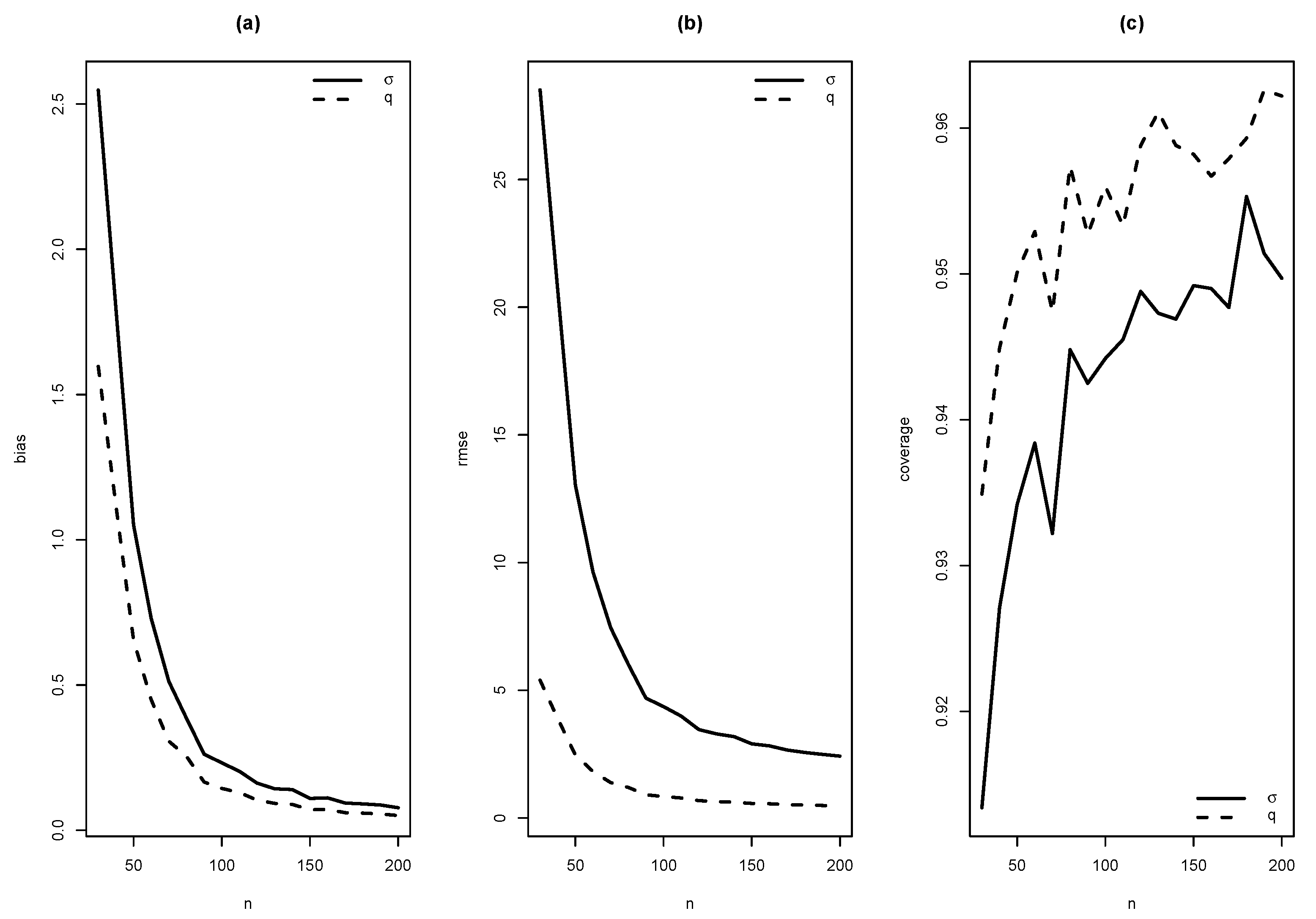
6. Simulation Study
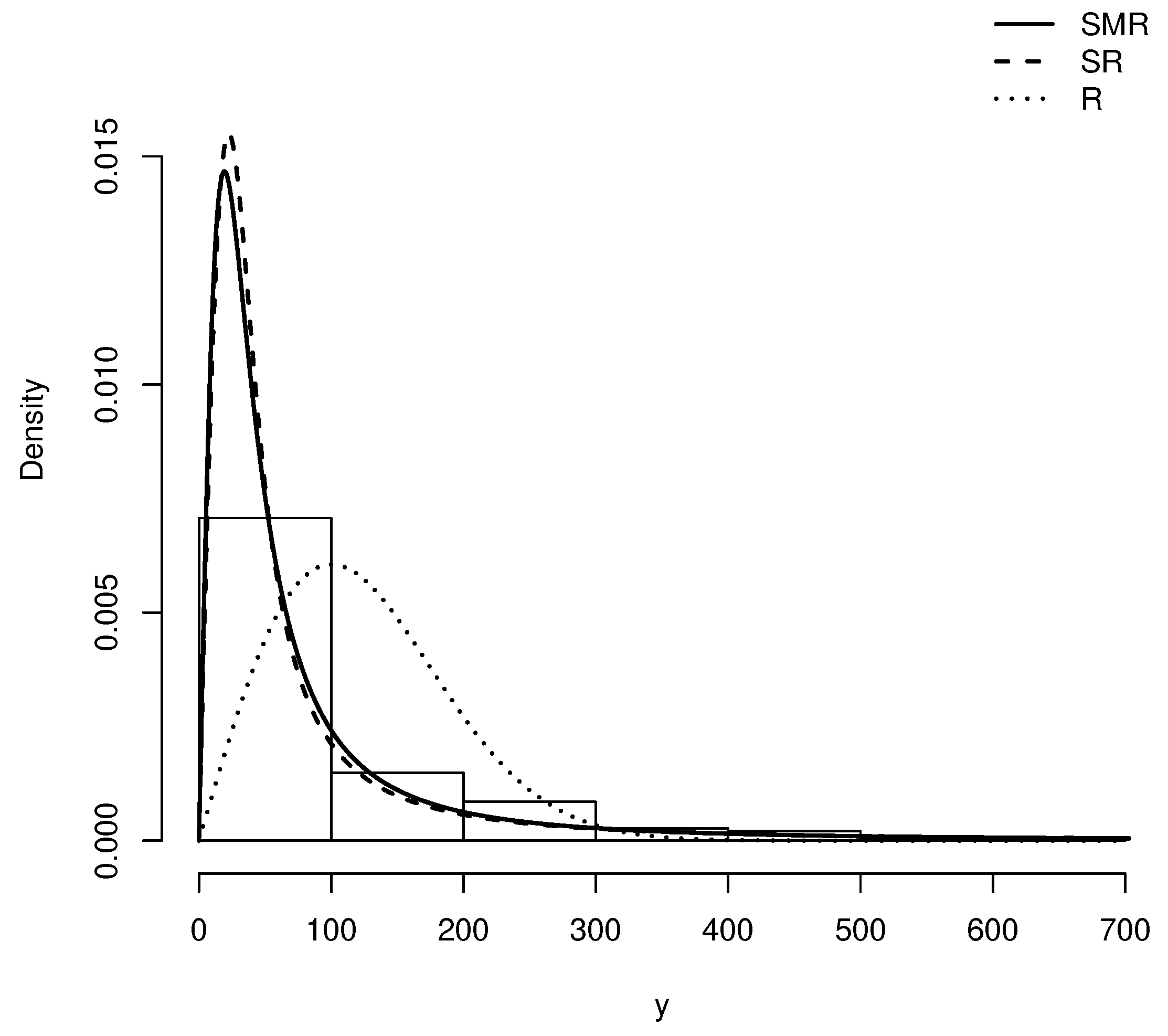
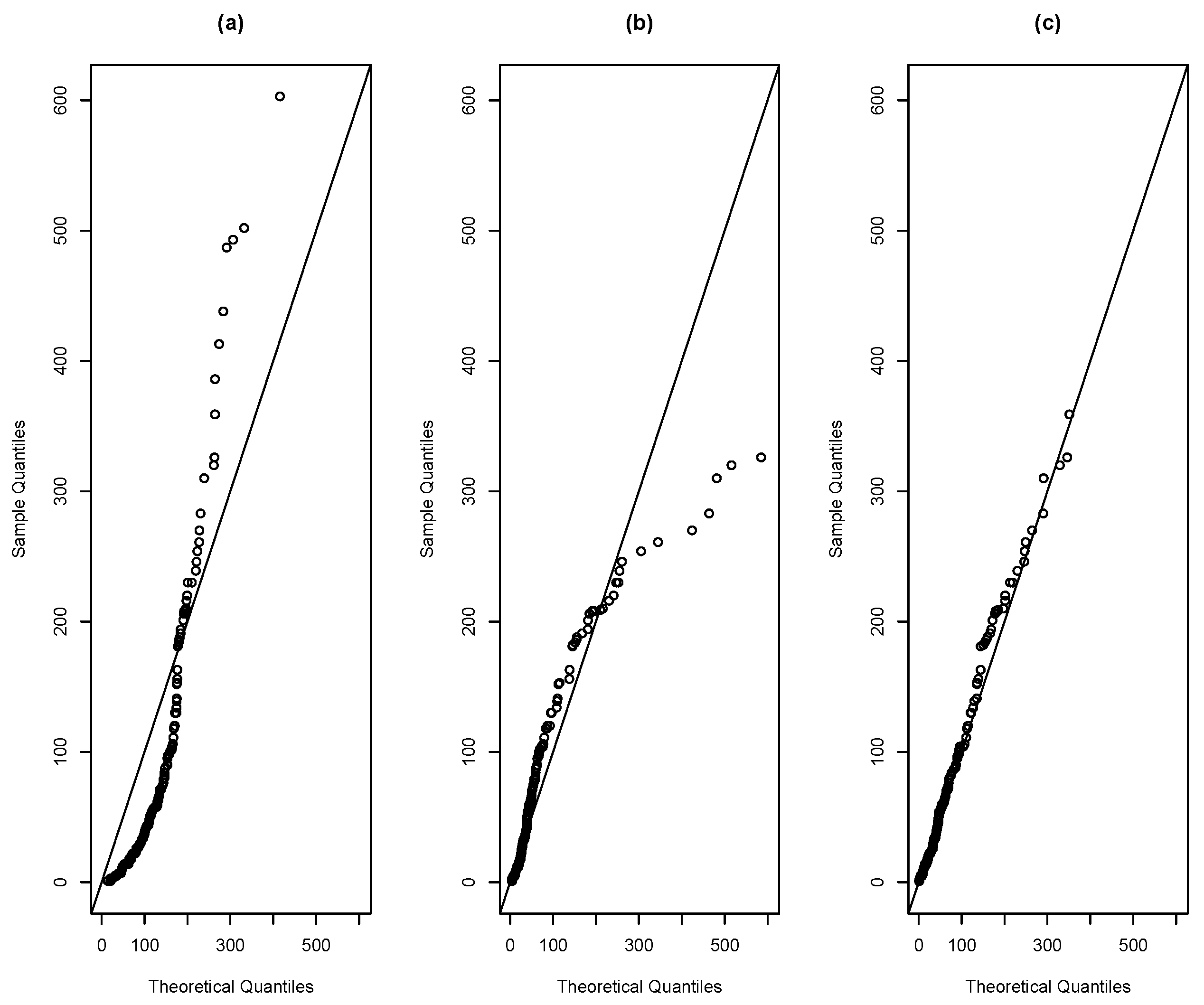
7. Real Data Illustration
7.1. Application to Patients with Bladder Cancer
7.2. Application to Number of Failures of an Air Conditioning System
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Siddiqui, M.M. Some problems connected with Rayleigh distributions. J. Res. Natl. Bureu Stand. Ser. D 1962, 66, 167–174. [Google Scholar] [CrossRef]
- Miller, K.S. Multidimensional Gaussian Distributions; Wiley: New York, NY, USA, 1964. [Google Scholar]
- Polovko, A.M. Fundamentals of Reliability Theory; Academic Press: San Diego, CA, USA, 1968. [Google Scholar]
- Hirano, K. Rayleigh distribution, In Encyclopedia of Statistical Sciences; Kotz, S., Johnson, N.L., Read, C.B., Eds.; Wiley: New York, NY, USA, 1986; pp. 647–649. [Google Scholar]
- Lopez-Blazquez, J.F.; Barranco-Chamorro, I.; Moreno-Rebollo, J.L. Umvu estimation for certain family of exponential distributions. Commun. Stat.-Theory Methods 1997, 26, 469–482. [Google Scholar] [CrossRef]
- Ahmed, A.; Ahmad, S.P.; Reshi, J.A. Bayesian Analysis of Rayleigh Distribution. Int. J. Sci. Res. Publ. 2013, 3, 217–225. [Google Scholar]
- Sarti, A.; Corsi, C.; Mazzini, E.; Lamberti, C. Maximum likelihood segmentation of ultrasound images with Rayleigh distribution. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2005, 52, 947–960. [Google Scholar] [CrossRef] [PubMed]
- Kalaiselvi, S.; Loganathan, A.; Vijayaraghavan, R. Bayesian Reliability Sampling Plans under the Conditions of Rayleigh-Inverse-Rayleigh Distribution. Econ. Qual. Control 2014, 29, 29–38. [Google Scholar] [CrossRef]
- Dhaundiyal, A.; Singh, S.B. Approximations to the Non-Isothermal Distributed Activation Energy Model for Biomass Pyrolysis Using the Rayleigh Distribution. Acta Technol. Agric. 2017, 20, 78–84. [Google Scholar] [CrossRef]
- Iriarte, Y.A.; Gómez, H.W.; Varela, H.; Bolfarine, H. Slashed Rayleigh Distribution. Colomb. J. Stat. 2015, 38, 31–44. [Google Scholar] [CrossRef]
- Reyes, J.; Barranco-Chamorro, I.; Gallardo, D.; Gómez, H.W. Generalized modified slash Birnbaum-Saunders distribution. Symmetry 2018, 10, 724. [Google Scholar] [CrossRef]
- Reyes, J.; Barranco-Chamorro, I.; Gómez, H.W. Generalized modified slash distribution with applications. Commun. Stat.-Theory Methods 2020, 49, 2025–2048. [Google Scholar] [CrossRef]
- Iriarte, Y.A.; Varela, H.; Gómez, H.J.; Gómez, H.W. A Gamma-Type Distribution with Applications. Symmetry 2020, 12, 870. [Google Scholar] [CrossRef]
- Segovia, F.A.; Gómez, Y.M.; Venegas, O.; Gómez, H.W. A Power Maxwell Distribution with Heavy Tails and Applications. Mathematics 2020, 8, 1116. [Google Scholar] [CrossRef]
- Stacy, E.W. A generalization of the gamma distribution. Ann. Math. Stat. 1962, 33, 1187–1192. [Google Scholar] [CrossRef]
- Casella, G.; Berger, R.L. Statistical lnference; Duxbury Advanced Series; Thomson Learning: Pacific Grove, CA, USA, 2002. [Google Scholar]
- Johnson, N.L.; Kotz, S.; Balakrishnan, N. Continuous Univariate Distributions, 2nd ed.; Wiley Series in Probability and Statistics: New York, NY, USA, 1995; Volume 2. [Google Scholar]
- Dempster, A.P.; Laird, N.M.; Rubim, D.B. Maximum likelihood from incomplete data via the EM algorithm (with discussion). J. R. Stat. Soc. Ser. B 1977, 39, 1–38. [Google Scholar]
- Lee, E.T.; Wang, J. Statistical Methods for Survival Data Analysis; John Wiley & Sons: New York, NY, USA, 2003; Volume 476. [Google Scholar]
- Akaike, H. A new look at the statistical model identification. IEEE Trans. Autom. Control. 1974, 19, 716–723. [Google Scholar] [CrossRef]
- Schwarz, G. Estimating the dimension of a model. Ann. Stat. 1978, 6, 461–464. [Google Scholar] [CrossRef]
- Proschan, F. Theoretical explanation of observed decreasing failure rate. Technometrics 1963, 5, 375–383. [Google Scholar] [CrossRef]




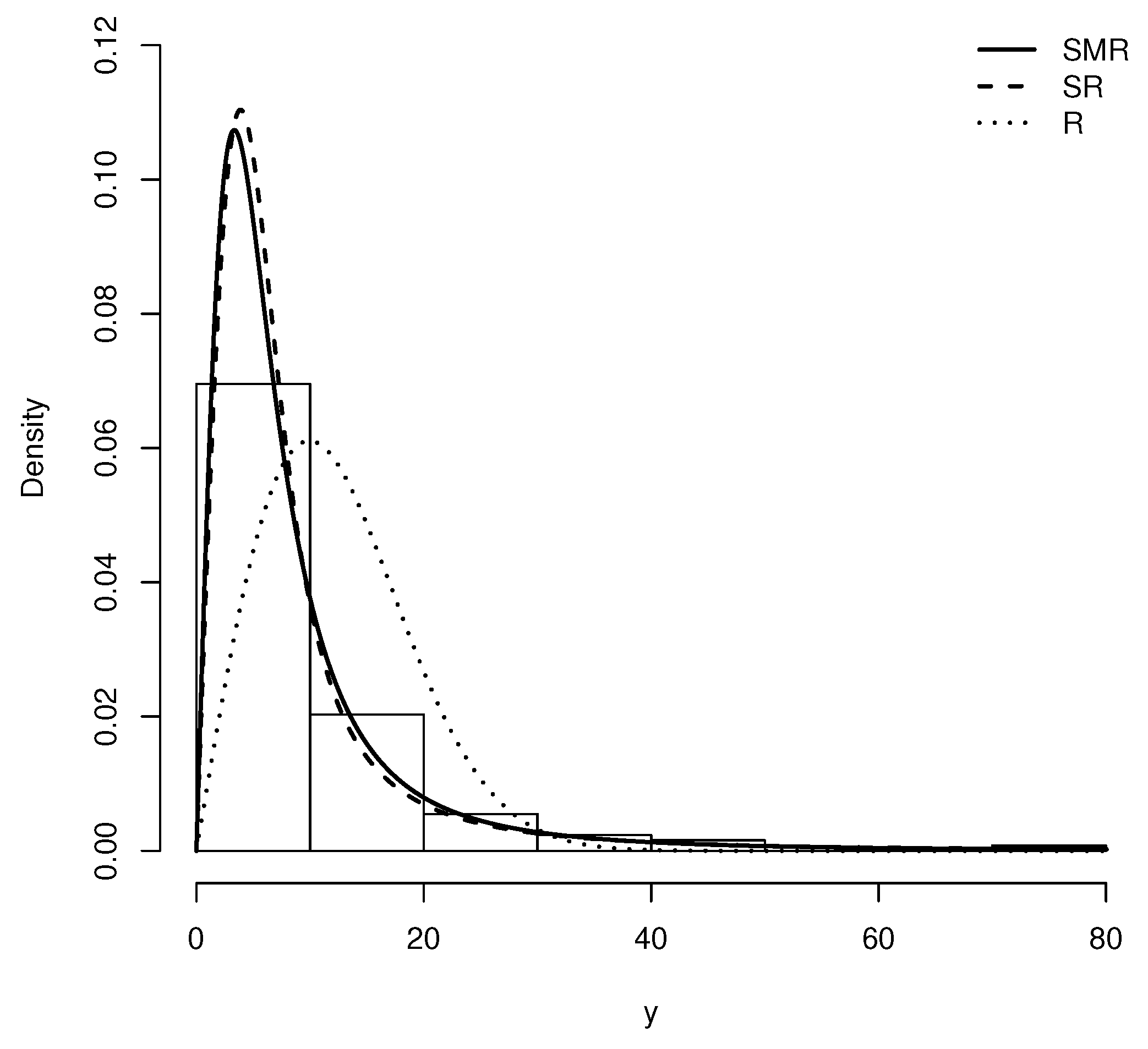

| n | S | |||||
|---|---|---|---|---|---|---|
| 128 | 9.366 | 10.508 | 3.287 | 18.483 | 0.08 | 79.05 |
| Estimaciones | R (SE) | SR (SE) | SMR (SE) |
|---|---|---|---|
| 98.639 (8.718) | 8.647 (2.051) | 15.369 (5.108) | |
| - | 1.424 (0.224) | 1.772 (0.318) | |
| log-likelihood | −491.266 | −415.815 | −413.339 |
| AIC | 984.531 | 835.631 | 830.677 |
| BIC | 987.383 | 841.335 | 836.381 |
| n | S | |||||
|---|---|---|---|---|---|---|
| 188 | 92.074 | 107.916 | 2.139 | 8.023 | 1 | 603 |
| Estimaciones | R (SE) | SR (SE) | SMR (SE) |
|---|---|---|---|
| 10,030.83 (730.135) | 264.611 (68.021) | 382.761 (113.843) | |
| - | 0.902 (0.107) | 1.069 (0.136) | |
| log-likelihood | −1191.275 | −1053.503 | −1046.549 |
| AIC | 2384.550 | 2111.006 | 2097.097 |
| BIC | 2387.787 | 2117.479 | 2103.570 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rivera, P.A.; Barranco-Chamorro, I.; Gallardo, D.I.; Gómez, H.W. Scale Mixture of Rayleigh Distribution. Mathematics 2020, 8, 1842. https://doi.org/10.3390/math8101842
Rivera PA, Barranco-Chamorro I, Gallardo DI, Gómez HW. Scale Mixture of Rayleigh Distribution. Mathematics. 2020; 8(10):1842. https://doi.org/10.3390/math8101842
Chicago/Turabian StyleRivera, Pilar A., Inmaculada Barranco-Chamorro, Diego I. Gallardo, and Héctor W. Gómez. 2020. "Scale Mixture of Rayleigh Distribution" Mathematics 8, no. 10: 1842. https://doi.org/10.3390/math8101842
APA StyleRivera, P. A., Barranco-Chamorro, I., Gallardo, D. I., & Gómez, H. W. (2020). Scale Mixture of Rayleigh Distribution. Mathematics, 8(10), 1842. https://doi.org/10.3390/math8101842







