A New Family of Chaotic Systems with Different Closed Curve Equilibrium
Abstract
1. Introduction
2. Chaotic Behavior of the Proposed System
3. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dudkowski, D.; Kapitaniak, T.; Kuznetsov, N.V.; Leonov, G.A.; Prasad, A. Hidden attractors in dynamical systems. Phys. Rep. 2016, 637, 1–50. [Google Scholar] [CrossRef]
- Kuznetsov, N.I. Hidden attractors in fundamental problems and engineering models: A short survey. In AETA 2015: Recent Advances in Electrical Engineering and Related Sciences; Lecture Notes in Electrical Engineering; Duy, V., Dao, T., Zelinka, I., Choi, H.S., Chadli, M., Eds.; Springer: Cham, Switzerland, 2015; Volume 371, pp. 13–25. [Google Scholar]
- Kapitaniak, T.; Leonov, G.A. Multistability: uncovering hidden attractors. Eur. Phys. J. Spec. Top. 2015, 224, 1405–1408. [Google Scholar] [CrossRef]
- Pham, V.-T.; Jafari, S.; Volos, C.; Gotthans, T.; Wang, X.; Hoang, D.V. A chatoic system with rounded square equilibrium and with no-equilibrium. Optik 2017, 130, 365–371. [Google Scholar] [CrossRef]
- Wang, X.; Chen, G. Constructing a chaotic system with any number of equilibria. Nonlinear Dyn. 2013, 71, 429–436. [Google Scholar] [CrossRef]
- Wei, Z. Dynamical behaviors of a chaotic system with no equilibria. Phys. Lett. 2011, 376, 102–108. [Google Scholar] [CrossRef]
- Wei, Z.; Wang, R.; Liu, A. A new finding of the existence of hidden hyperchaotic attractor with no equilibria. Math. Comput. Simul. 2014, 100, 13–23. [Google Scholar] [CrossRef]
- Pham, V.-T.; Volos, C.K.; Jafari, S.; Wei, Z.; Wang, X. Constructing a novel no-equilibrium chaotic system. Int. J. Bifurc. Chaos 2014, 24, 1450073. [Google Scholar] [CrossRef]
- Tahir, F.R.; Jafari, S.; Pham, V.-T.; Wang, X. A novel no-equilibrium chaotic system with multiwing butterfly attractors. Int. J. Bifurc. Chaos 2015, 25, 1550056. [Google Scholar] [CrossRef]
- Pham, V.-T.; Vaidyanathan, S.; Volos, C.K.; Jafari, S.; Kingni, S.T. A no-equilibrium hyperchaotic system with a cubic nonlinear term. Optik 2016, 127, 3259–3265. [Google Scholar] [CrossRef]
- Molaie, M.; Jafari, S.; Sprott, J.C.; Golpayegani, S. Simple chaotic flows with one stable equilibrium. Int. J. Bifurc. Chaos 2013, 23, 1350188. [Google Scholar] [CrossRef]
- Wang, X.; Chen, G. A chaotic system with only one stable equilibrium. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 1264–1272. [Google Scholar] [CrossRef]
- Jafari, S.; Sprott, J.C. Simple chaotic flows with a line equilibrium. Chaos Solitons Fractals 2013, 57, 79–84. [Google Scholar] [CrossRef]
- Gotthans, T.; Petržela, J. New class of chaotic systems with circular equilibrium. Nonlinear Dyn. 2015, 73, 429–436. [Google Scholar] [CrossRef]
- Gotthans, T.; Sprott, J.C.; Petržela, J. Simple chaotic flow with circle and square equilibrium. Int. J. Bifurc. Chaos 2016, 26, 1650137. [Google Scholar] [CrossRef]
- Pham, V.-T.; Volos, C.; Jafari, S.; Vaidyanathan, S.; Kapitaniak, T.; Wang, X. A chaotic systems with different families of hidden attractors. Int. J. Bifurc. Chaos 2016, 26, 1650139. [Google Scholar] [CrossRef]
- Wiggins, S. Linearization. In Introduction to Applied Nonlinear Dynamical Systems and Chaos, 2nd ed.; Marsden, J.E., Sirovich, L., Antman, S.S., Eds.; Springer: New York, NY, USA, 2002; pp. 10–11. [Google Scholar]
- Lynch, S. Three-Dimensional Autonomous Systems and Chaos. In Dynamical Systems With Applications Using Matlab, 2nd ed.; Marsden, J.E., Sirovich, L., Antman, S.S., Eds.; Springer International Publishing: Dordrecht, Switzerland, 2014; pp. 294–296. [Google Scholar]
- Cicek, S.; Ferikoglu, A.; Pehlivan, I. A new 3D chaotic system: dynamical analysis, electronic circuit design, active control synchronization and chaotic masking communication application. Optik 2016, 127, 4024–4030. [Google Scholar] [CrossRef]
- Volos, C.; Kyprianidis, I.; Stouboulos, I. A chaotic path planning generator for autonomous mobile robots. Robot. Auton. Syst. 2012, 60, 651–656. [Google Scholar] [CrossRef]
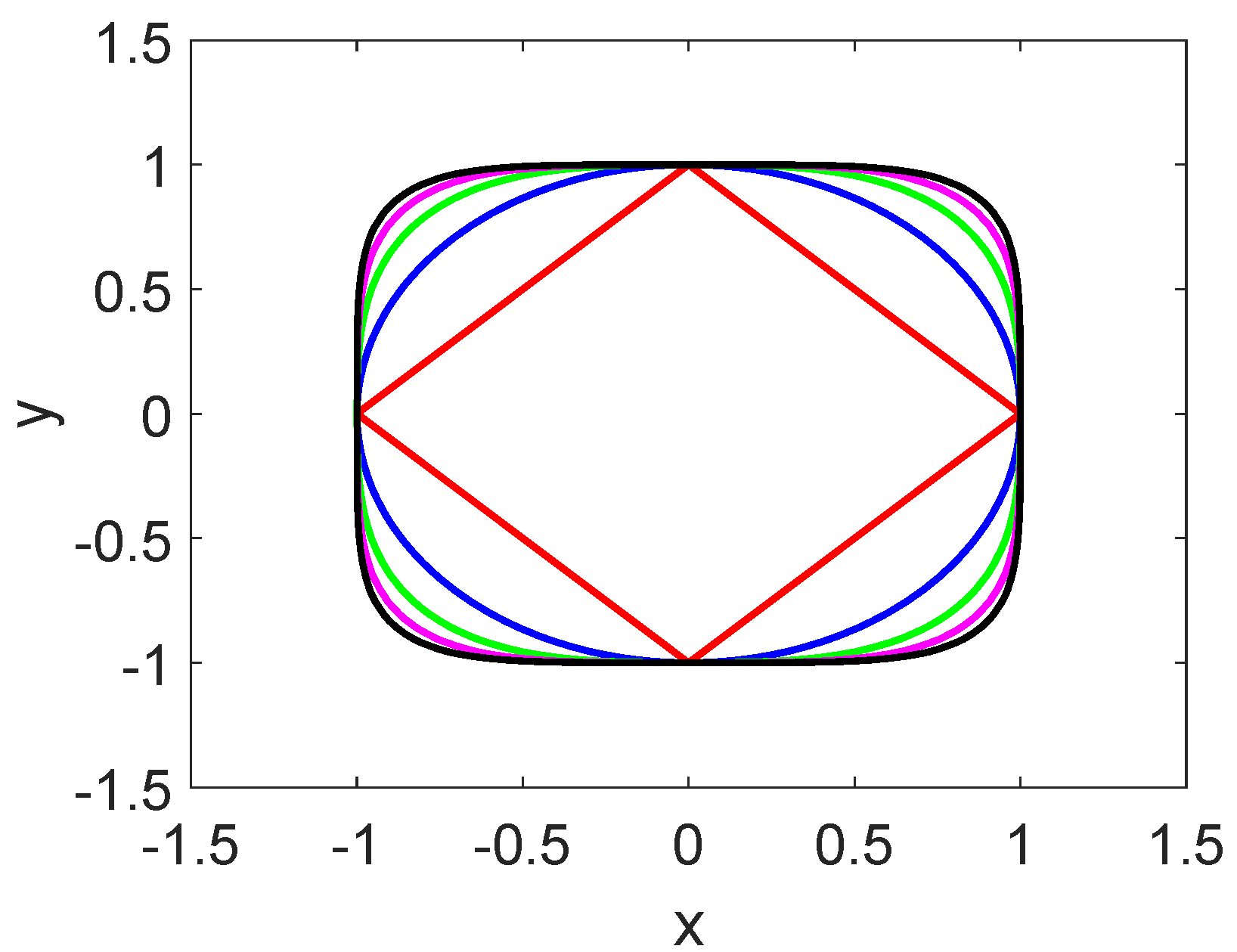
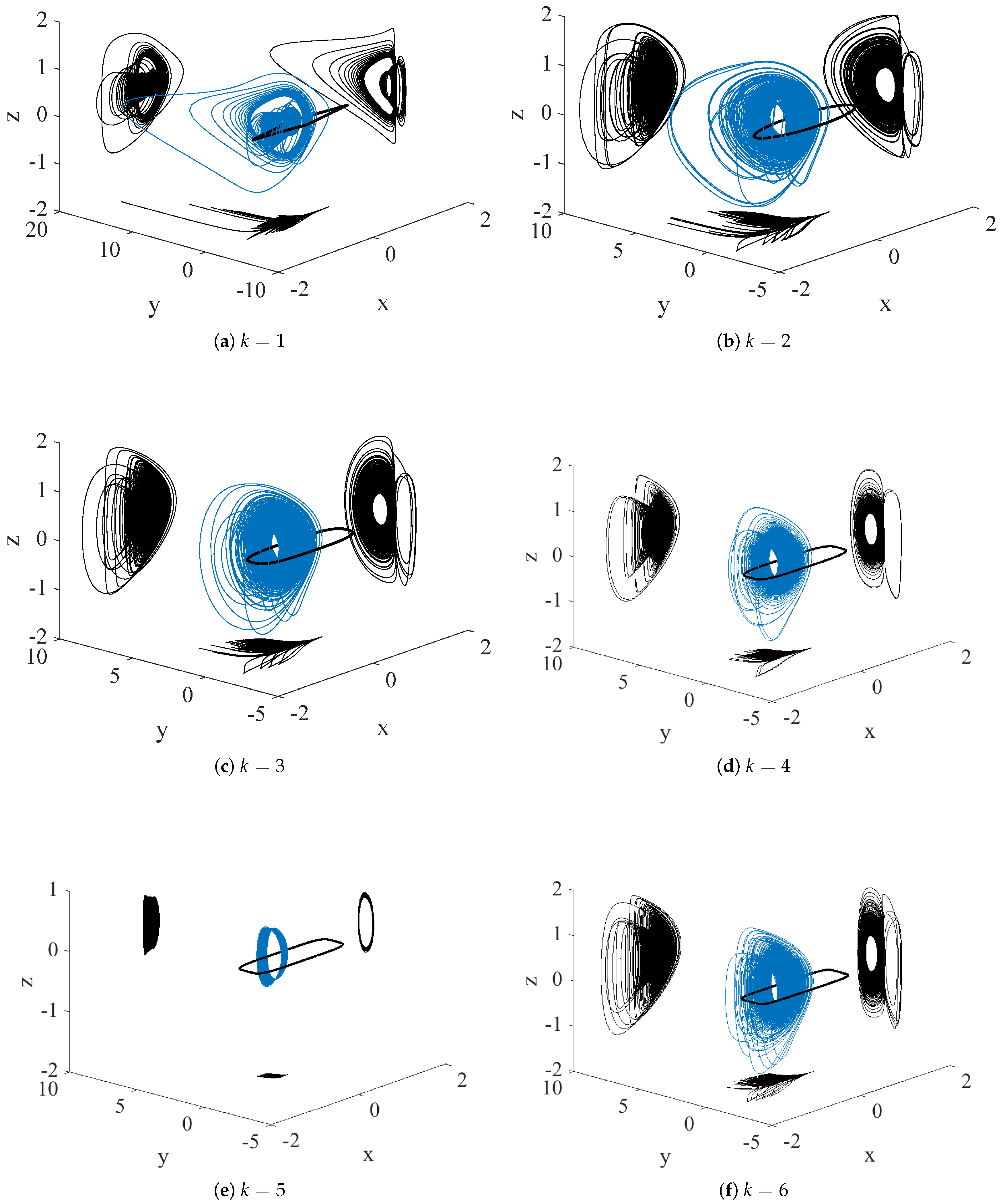
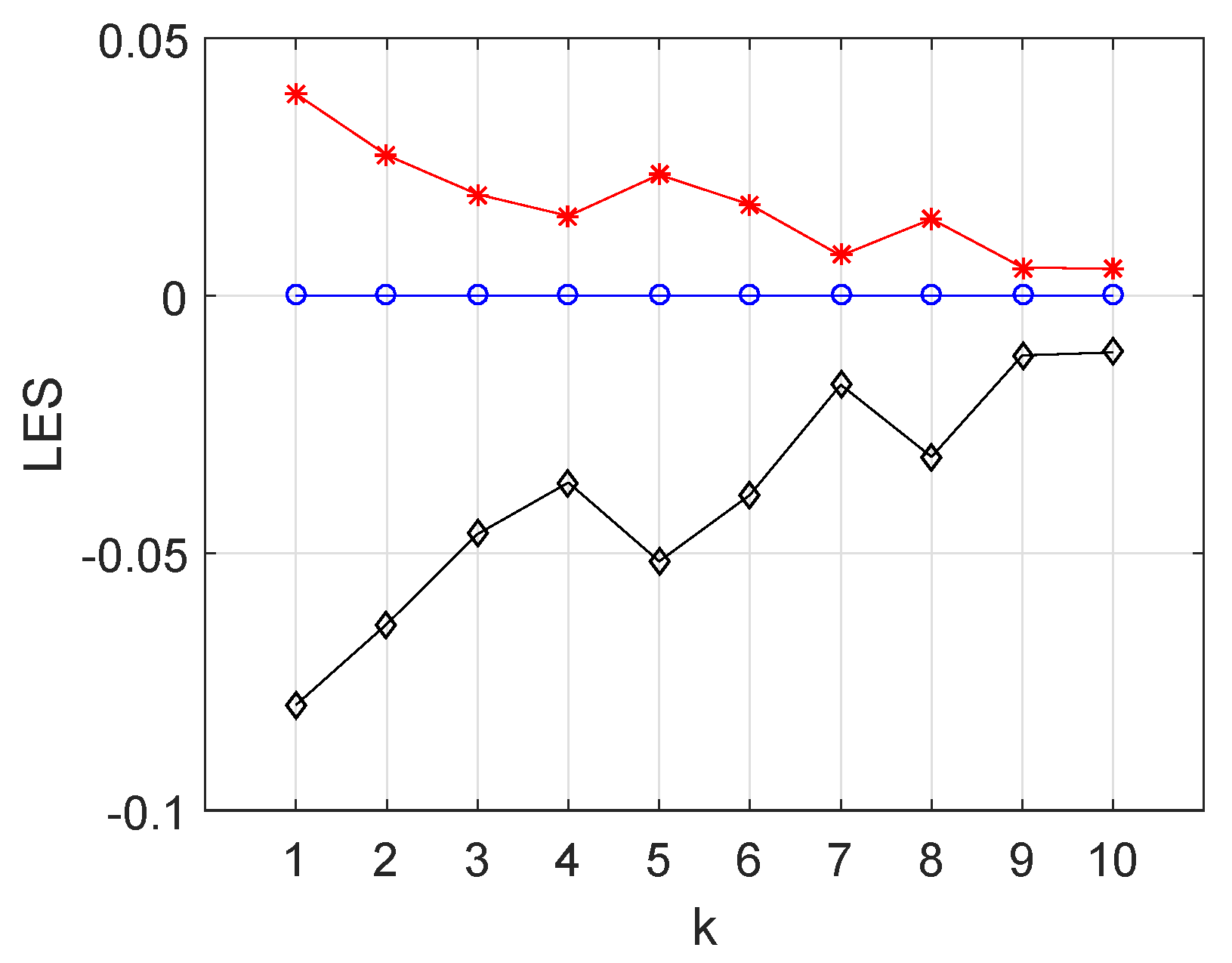
| Equations | Equilibrium | LEs | |
|---|---|---|---|
| Case | |
|---|---|
| I | one positve real, one negative real |
| I | a pair of purely imaginary |
| a pair of purely imaginary | |
| a pair of purely imaginary | |
| a pair of purely imaginary | |
| one positve real, one negative real | |
| Case | |
|---|---|
| I | one positve real, one negative real |
| I | a pair of purely imaginary |
| a pair of purely imaginary | |
| a pair of purely imaginary | |
| a pair of purely imaginary | |
| one positve real, one negative real | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, X.; Du, W.-S. A New Family of Chaotic Systems with Different Closed Curve Equilibrium. Mathematics 2019, 7, 94. https://doi.org/10.3390/math7010094
Zhu X, Du W-S. A New Family of Chaotic Systems with Different Closed Curve Equilibrium. Mathematics. 2019; 7(1):94. https://doi.org/10.3390/math7010094
Chicago/Turabian StyleZhu, Xinhe, and Wei-Shih Du. 2019. "A New Family of Chaotic Systems with Different Closed Curve Equilibrium" Mathematics 7, no. 1: 94. https://doi.org/10.3390/math7010094
APA StyleZhu, X., & Du, W.-S. (2019). A New Family of Chaotic Systems with Different Closed Curve Equilibrium. Mathematics, 7(1), 94. https://doi.org/10.3390/math7010094






