1. Introduction
A sequence of measurements made on a quantum system leads to a number of random outcomes, observed by an experimentalist. In this sense, the composite system + meter(s) may be seen as following a path across a stochastic network, connecting all possible measurement results. The same can be said about a purely classical stochastic system, and the quantum nature of the experiment reveals itself only in the manner in which the transition probabilities are calculated. While a classical theory allows for non-invasive observations, a quantum meter plays an active role in “shaping” the network. In the simplest case of von Neumann (vN) measurements [
1], the transition probabilities, as well as the observed events themselves are determined by the virtual paths available to the studied system, the nature of the measured quantity and the accuracy of the meter(s).
Perhaps the most studied network is produced by an accurate vN measurement of a projector on a state
(preparation or pre-selection), followed by a not necessarily accurate vN measurement of an operator
, followed by an accurate measurement of a projector on a final state
(post-selection) [
2]. Post-selection may succeed or fail, and a sub-ensemble is selected by collecting the statistics only in the case it has been successful. If
has
N distinct eigenvalues, there a
N virtual paths connecting the two states, for which quantum mechanics provides probability amplitudes, but not probabilities, if the system is considered in isolation. If the measurement of
is accurate (ideal, strong), the meter destroys the interference between the paths, which can be now equipped with probabilities (typically, squares of the moduli of the corresponding amplitudes) and become “real”. Each time the experiment is repeated, the pointer points at one of the eigenvalues of the operator
, whose mean value is then calculated using the relative frequencies of these occurrences. The probability to end up in
is not what it would be, had the meter not perturbed the system’s evolution.
More controversial is the opposite limit, where the intermediate measurement is made inaccurate (weak), in order to avoid the said perturbation. The weakness of the measurement inevitably causes the meter’s readings to be spread, covering the whole real axis in the limit when the perturbation is sent to zero [
2]. This gives an operational meaning to the uncertainty principle, which states that the value of
in a superposition of its eigenstates must be indeterminate. Indeed, trying to measure it without destroying the superposition may yield any reading at all..
Much of the controversy resides, however, in the description of the expectation of a weak meter’s reading (weak value) as a “new concept” [
2] , capable of shedding new light on quantum phenomena. In the years that followed it gave rise a number of extraordinary notions. These include
“negative kinetic energy” [
3],“negative number of particles” [
4], “having one particle in several places simultaneously” [
5], “photons disembodied from its polarisation” [
6], “electrons with disembodied charge and mass” [
6] and “an atom with the internal energy disembodied from the mass” [
6], all supported by the “evidence” of weak measurements.
Recently, we have shown [
7] that the above statements amount to over-interpretations, easily dismissed once the weak values are identified with the transition amplitudes on the paths connecting the initial and final states, and are not seen as the values of the measured quantities. In this paper, we will continue to look at the problem of consecutive quantum measurements, focusing on the classical statistical ensembles (networks) produced whenever a meter, or meters, interact with the observed quantum system. We will pay special attention to the importance of the quantum uncertainty principle and emphasize the role that quantum interference plays in the loss of information about the system’s past. Throughout the paper, we will use the simplest case of a two-level system as an example and refer the reader to [
8,
9,
10,
11,
12,
13,
14,
15] for a more general analysis.
The rest of the paper is organized as follows. In
Section 2, we compare two of the best known formulations of the uncertainty principle. In
Section 3, we briefly describe the work of a von Neumann meter. In
Section 4, we introduce a simple classical toy model, to be compared with its quantum counterparts later.
Section 5 returns to the simplest quantum network produced by a vN measurement of a quantity, with pre- and post-selection. In
Section 6, we use our approach to describe a measurement of the difference of two physical quantities, in such a way that the values themselves remain indeterminate.
Section 7 sets the rules for combining virtual paths. Accurate (strong) and inaccurate (weak) limits of the measurements are analyzed in
Section 8 and
Section 9, respectively. In
Section 10, the uncertainty principle is used to explain the properties of the weak values. Common misconceptions related to the issue are discussed in
Section 11.
Section 12 contains our conclusions.
2. Quantum Uncertainty Principle(s)
There are several formulations of the uncertainty principle (UP), covering various aspects of the problem (for a recent review, see [
16]). Perhaps best known is the one relating the respective standard deviations,
and
, of two variables, represented by non-commuting operators
and
, measured in the same state. The so-called Robertson’s uncertainty relation [
17] reads:
where
,
is the commutator, and the angular brackets indicate averaging in the pure state
that the system is supposed to be in,
. Equation (
1) is often interpreted as an indication of the fact that a measurement of
must disturb a measurement of
, and vice versa.
A somewhat different approach to the UP can be found in [
18], where one reads: “Any determination of the alternative taken by a process capable of following more that one alternative destroys the interference between alternatives”. This principle is complemented by the rule for assigning probabilities to alternative scenarios [
19]: “When an event can occur in several alternative ways, the probability amplitude for the event is the sum of the probability amplitudes for each way considered separately. If an experiment is performed which is capable of determining when one or another alternative is actually taken, the probability of the event is the sum of the probabilities of each alternative”.
From the above statements, to which we will refer as “Feynman’s uncertainty principle”, one may conclude that interfering alternatives cannot be told apart and must, therefore, form a single indivisible pathway [
20]. Another corollary to the principle is that interference must be destroyed by a physical agent, e.g., a meter coupled to the observed system. Thus, construction of probabilities by means of squaring the moduli of the corresponding amplitudes pre-supposes the existence of a suitable meter, as well as the fact that the meter has already been deployed [
15].
It is the Feynman’s UP to which we will appeal in what follows. Despite the obvious difference between the formulations, Feynman’s principle is not all that different from the UP expressed by Equation (
1). Consider, for example, a system with a zero Hamiltonian,
, prepared at
in a state
. An accurate measurement of
at
yields an eigenvalue
with the probability
, where
is the corresponding eigenstate. Suppose we also want to learn something about the value of
at
. The system must be, in some sense, in one of the eigenstates of
. To see in what sense, precisely, we insert the unity
into the amplitude
. The result is
. There are now different ways (paths, pathways, routes) to reach the final state by “passing through one of the states
”. Without a meter, we cannot determine which of them is actually taken. Thus, despite the abundance of virtual paths, there is only a single “real” one, “traveled” with the probability
. If, on the other hand, we employ a meter, capable of telling us which of the eigenvalues
has occurred at
, the virtual paths will become real, with the
-th path traveled with the probability
. We also find that the measurement of
has disturbed the result of measuring
, since:
Quantifying the disturbance would lead to estimates similar to (
1). We will not follow this matter any further, and in the next section we discuss possible realizations of quantum measurements.
3. Von Neumann Measurements and Meters
In the following, we will often refer to von Neumann (vN) measurements [
1], whose definition we will revisit in this section. A vN meter, designed to measure a variable
of a system governed by a Hamiltonian
, consists of a pointer (e.g., a massive particle on a line) whose position is
ξ and which is coupled to the observed system via (
):
If the value of
is measured at some
, the function
is chosen to be
, where
is the Dirac delta. We will assume that
has discrete eigenvalues
, and
are the corresponding eigenstates,
. Prior to the interaction, at
, the system is described by a state
, and the pointer is in the state
, such that:
is a real function (e.g., a Gaussian), peaked around
and rapidly tending to zero as
. (The choice of
as a real function ensures that both the mean momentum and the mean position of the pointer are zero. In the classical limit, a vN meter does not perturb the motion of the monitored system, provided its momentum is set exactly to zero. Quantally, this condition can be met only approximately. The spread of the momenta around the zero mean accounts, therefore, for the inevitable perturbation the meter would incur. Making
G broad in the coordinate space would minimise this perturbation, at the expense of the measurement’s accuracy.) Immediately after the interaction has taken place, the pointer becomes entangled with the system, and their wave function is given by:
where
. We assume further that the final position of the pointer can be determined precisely, leaving out the fundamental question of how exactly this is done and whether a collapse of the wave function has occurred [
1]. In one way or another, after the interaction has taken place, we may “look” at the pointer and find a meter’s reading
ξ. Perhaps the best known practical realization of a vN measurement is the Stern–Gerlach apparatus (see, for example, [
2,
21]). Other vN meters can be constructed by using the spin-orbit interaction [
22,
23,
24] or Bose–Einstein condensates trapped in double-well potentials [
25].
If nothing else is done to the system, the probability (density) to find a reading
is (
stands for the trace of the operator inside the brackets):
where
. Alternatively, at some
, we may try to find (post-select) the system in some state
, and keep the meter’s readings only if the post-selection is successful. With this additional condition, the probability to find a reading
becomes:
Thus, apart from the preparation (pre-selection) of the system in a state
, two other events have occurred: the pointer was found pointing at
, and the system was later found in a final state
. Quantum mechanics provides the probabilities of such occurrences by means of expressions similar to Equations (
6) and (
7). We can imagine, for example, performing more measurements between
and
, thus creating a network of scenarios, each of which would occur with certain frequency if the experiment is repeated many times. Before discussing such “quantum” networks, we briefly turn to their classical counterparts.
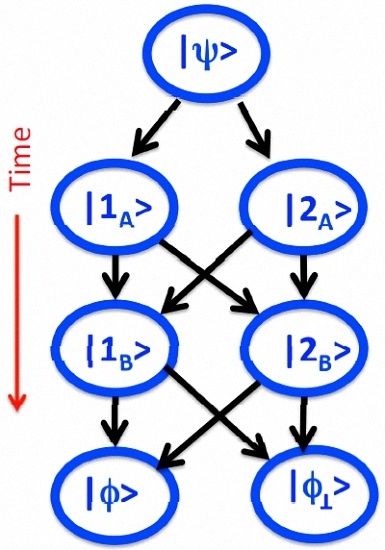
4. Classical Networks and Functionals
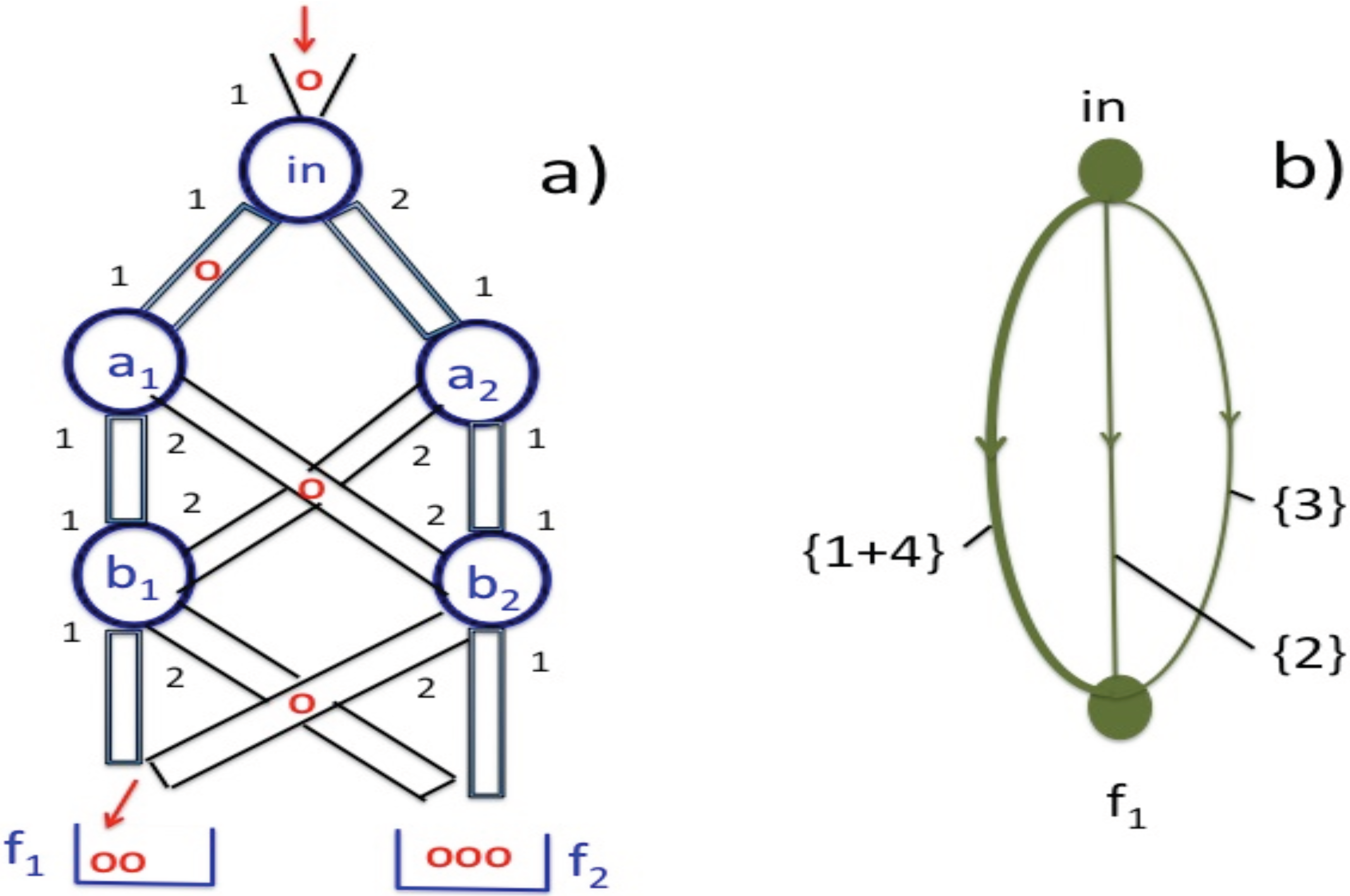
We start with a simple toy model, which, we hope, will be helpful for the discussion of the following sections. Suppose one has at his/her disposal a kit containing purely classical elements, little balls, tubes and connectors, which can be joined together. A connector
x has two entrances, and
is the probability to enter via the inlet
j and subsequently leave via the outlet
i. If one of the outlets is blocked, the probability to exit via the remaining one is unity for both entrances. Connecting the elements as shown in
Figure 1, one can “engineer” a network of paths, which a ball inserted at the top will travel at random, until ending up in one of the final states
and
. Ascribing variables (numbers)
,
,
,
to each of the connectors, one can also associate numbers (functionals) with each path. For example, in
Figure 1a, there are eight paths
,
. Four of them end in the final state
,
: , traveled with the probability ,
: , traveled with the probability ,
: , traveled with the probability ,
: , traveled with the probability ,
while four more paths,
,
, end in
. It is always possible to consider sub-networks containing only few paths, regardless of what is happening at later times, or elsewhere in the diagram.
For example, we may limit our attention only to the cases where the ball goes to . As a result of this “post-selection”, there are only two paths: through and through , traveled with probabilities and , respectively. There is a single random variable, a functional taking the values , , and whose mean value, evaluated over many runs by counting the times the ball passes through and , is: .
Alternatively, we may focus only on those cases where the ball ends up in
, and ask for the difference between the values of
and
,
, which is recorded for each run. Let
and
. Now, the said difference is a functional
defined on the four paths introduced above and taking the values
,
and
. Since two of the values of
are the same, we can reduce the number of paths by one, by combining
and
into a single pathway,
, traveled with a probability
, and on which
is understood to be zero. The resulting equivalent system is shown in
Figure 1b, and for
, evaluated by writing down the value of
for each run of the ball, adding up the results and dividing by the number of runs, we find:
We have invoked the simple toy model in
Figure 1 in order to emphasize its resemblances to and differences from the quantum case outlined at the end of the previous section. As in the classical case, a set of vN measurements creates sequences of events occurring with certain probabilities, which can be seen as classical pathways “traveled” with certain frequencies, if the the experiment is repeated many times.
The quantum origin of the experiment becomes evident in the way the probabilities of the events are calculated, as well as in the nature of these events. A meter recombines and divides the system’s virtual paths, endowed with probability amplitudes only, thereby producing “real” ones, to which probabilities can be assigned. In particular, two interfering paths are combined into a single one in a much stronger sense, than in the classical case. For example, in
Figure 1b, the paths
and
are combined into
, since we chose to be interested only in the difference
of
and not in their actual values. We will show that in the quantum case, these actual values may not even exist. Finally, in the classical case, it is understood that two different setups built from the elements of the same kit can be completely different and need not share any statistical properties. In the quantum case, this is less clear, and we will return to the problem of “contextuality” later.
5. A Simple Quantum Network: Measuring a Quantity with Post-Selection
As the first example, we consider a vN measurement of an operator
, for a two-level system, pre- and post-selected in the states
and
, at
and
, respectively. For simplicity, we put the system’s Hamiltonian to zero,
, so that nothing happens between
and
, as well as between
and
. Our purpose is to show that this creates a classical statistical ensemble not too dissimilar from the one built from the classical elements in the previous section. To see how this is done, we consider the two virtual paths connecting
and
,
,
. These are
: , with an amplitude , and
: , with an amplitude .
We also have .
The value of
at
is a functional
defined on the two paths and, obviously, taking the values
and
:
Without a meter, we may define an amplitude distribution
for the value
f taken by
,
but cannot say what is the probability for having, e.g., the value
.
With a meter (
3) coupled to the system, the final pointer’s state
is easily found to be given by:
and the meter reads
with a (unnormalized) probability:
Equation (
12) may be interpreted in the following way. By turning the meter on, we have created a continuum of real paths, all labeled by
ξ. These connect the three observed events,
←
←
, assuming the meter is read before the post-selection is performed.
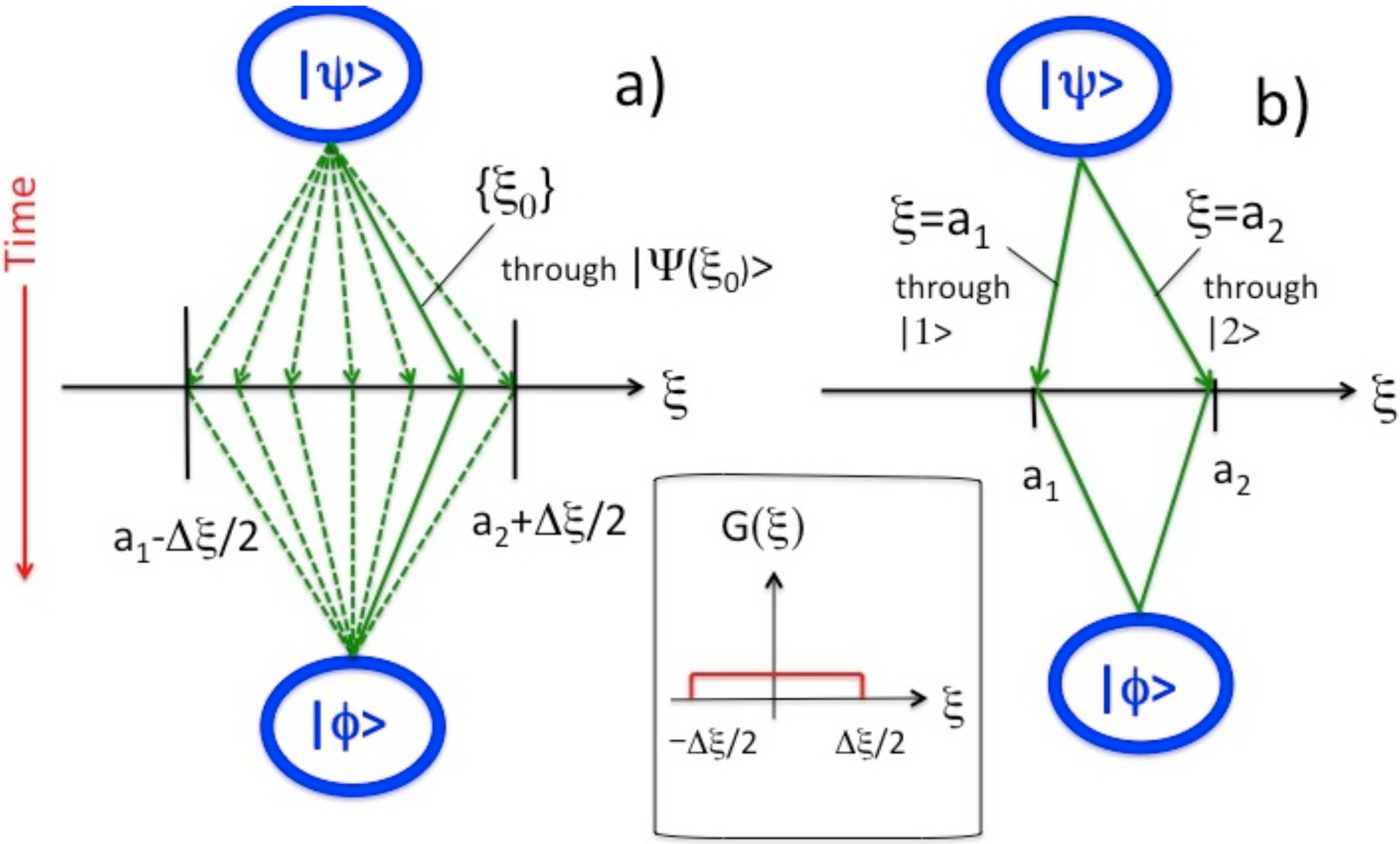
The range of possible scenarios is determined by the width of the initial pointer’s state
in Equation (
4), which also determines the accuracy of the measurement. For example, if
is chosen to be:
the possible values of
ξ would lie inside the the interval
, assuming
(see
Figure 2). The path labeled
ξ is traveled with a probability (density)
. Far from passively observing the motion of the system, a meter actively participates in shaping the observed statistical ensemble. Accordingly, the probabilities in Equation (
12) contain both the amplitudes
, which characterize the unobserved system, and the meter’s own
.
In the general case, we may learn little about the quantity of interest,
. Instead of extracting its value, we can only conclude that if the meter reading is
, the system’s state after interaction with the meter was (the next Equation follows upon multiplication of the pure state
in Equation (
5) by a projector
, and tracing out the pointer’s variable):
for which the precise value of
remains indeterminate. It is, however, possible to say that the measurement “drives” the system through a state
, with a probability
. To see that this is, indeed, the case, we could make an accurate measurement of the projector onto
just after
. Repeating the procedure many times will show that whenever the reading of the first meter lies in a narrow interval around
, the additional projection will also succeed. Thus, while every attempt to measure
leads to a particular stochastic network, not every measurement allows us to determine the value of
. We will return to the question of the accuracy shortly after considering yet another example.
6. A More Elaborate Network: Measuring the Difference of Two Quantities
Suppose that, as in the previous section, we have a two-level system pre- and post-selected in the states and at and , respectively. Now, we want to learn something about the difference of the quantities represented by operators and , which may or may not commute, at and , such that (classically, we could consider, for example, the x-and y-components of the momentum and ask for ). Quantally, there is more than one way to approach this task.
- (i)
We could employ two vN meters, set to measure and separately, and evaluate the difference of their readings.
- (ii)
We could also construct a Hermitian operator formally representing the difference of interest,
, and use a single vN meter to measure it at
. As discussed in [
26], a classical analog of this procedure is a predictive measurement, which uses the fact that knowing the system’s coordinates and momenta at
allows one to restore the system’s subsequent trajectory and, with it, all future values of all quantities.
- (iii)
A procedure unique to quantum mechanics allows one to evaluate the difference of interest, leaving the actual values of and indeterminate.
In general, all three methods give different results [
26], and here, we are only interested in the option (iii). With this in mind, we couple a vN pointer (position
ξ), to
and
at
and
, respectively, so that the interaction with the meter is given by:
and the pointer’s initial state,
, has, as before, a Gaussian shape, centered at
. The measurement works as follows: after the first coupling, the pointer is shifted by one of the eigenvalues of
and after the second one, by an eigenvalue of
, albeit in the opposite sense. The resulting shift is, thus, the difference of the two values. This is not exactly the scheme used by von Neumann [
1], and we will call this meter von Neumann-like [
12].
Without a meter, there are four virtual paths,
,
, which connect
and
and pass through different intermediate states. For example, the path going through
and then through
has a probability amplitude
. Putting, as before,
, we, therefore, have the paths
: , with an amplitude ,
: , with an amplitude ,
: , with an amplitude ,
: , with an amplitude
with the amplitudes satisfying a condition
. The four paths are easily identified in the diagram in
Figure 3a.
The difference between
and
is a functional
defined on the four paths, where it may take four different values:
Let
and
represent the components of spin-1/2 (without a factor of 1/2) along two different axes, so that
and
. Now,
takes three different values,
, 0 and 2. With no meter employed, we cannot say what is the chance of having, e.g.,
, but, as before, may write down an amplitude distribution
for the value
f of the functional
,
normalized by the condition
.
As in the previous section, this so far purely cosmetic re-arrangement of the virtual paths is useful for describing the effects of a meter, once it is coupled to the system as prescribed by Equation (
15). After a successful post-selection, the pointer is in a pure state
, and it is a simple matter to check that:
We can now look at Equation (
18) from the point of view adopted in the previous section. As in
Section 4, by external manipulation (connecting the system to a meter), we have created a classical statistical ensemble, where the system can reach the final state via a continuum of real paths, labeled by the the variable
ξ. Again, the meter plays an active role, and the (unnormalized) probability to travel the
-th path is determined by the shape of
G, which is the property of the pointer, as well as the amplitudes of the virtual paths, which represent the system “on its own”,
With this, the mean reading of the meter over many trials is readily seen to be given by:
As in the previous example, the value of
remains indeterminate, if there is more than one term in the r.h.s. of Equation (
19). To establish a good correlation between the pointer readings
ξ and the values of
, we must, therefore, try to avoid the overlap between different
G’s in (
19). We will do so after discussing the general rules for combining virtual paths in the next section.
7. Superposition Principle for Virtual Paths
Feynman’s uncertainty principle, cited in
Section 2, implies that virtual paths can be combined and recombined into new sets of paths, with interference being responsible for the loss of information about certain aspects of quantum motion. Next, we briefly discuss the rules for creating such combinations.
We start with the well-known case of changing the basis functions in which a state of the system can be expanded at a given time. Let a two-state system be in the state , where , and is the amplitude with which enters in . Consider a different basis, , , which is related to the first basis by a unitary transformation, . Now, the amplitude , with which enters in , is , . These equations can be read as follows: if a new basis state is a weighted sum of the old ones, the corresponding amplitude is the weighted sum of the corresponding amplitudes.
The same rules can be applied to virtual paths, and we can describe the action of the meter in
Section 5 in a similar manner. If the pointer reading is
, the meter combines virtual paths
and
with amplitudes
and
, into a single real path
with an amplitude
. The path is travelled with the probability
.
The same can be said about the measurement in Section VI. There the meter reading
combines four available virtual paths into a single real one,
with the amplitude
and
gives the probability to obtain a meter reading
if the measurement is repeated many times. In general, it is understood that if paths
and
with the amplitudes
and
are combined into a new path,
, with complex valued weights
α and
β, then the amplitude for the new pathway is:
If a functional
takes different definite values on
and
,
, its value on
is indeterminate (we will elaborate on this statement in
Section 10).
That the width of
,
, determines the resolution, or the accuracy, of a measurement is readily seen if
is chosen to have the form of a “rectangular window” (
13). Inserting (
13) in Equation (
18) yields:
and we can be sure that if a reading is
, the value of the measured functional,
f, is no smaller than
and no greater than
. We are, however, “banned” from looking inside the interval
, where all information about
f is lost to the interference, which the meter has failed to destroy. Next, we look at the question of accuracy in more detail.
8. Most Accurate Measurements, Real Pathways and Contextuality
Suppose we can change the width
of the initial pointer state, e.g., by choosing:
where the factor
ensures that the state is properly normalized,
. Since, by assumption, the pointer’s final position is evaluated accurately, it is the uncertainty of its initial setting that determines the precision of the measurement. For a highly accurate measurement, we should choose
as small as possible, thus requiring:
If so, in the example of
Section 5, Equation (
12) reduces to:
and of all real paths, only two survive. Equivalently, we can say that the action of the meter has destroyed all interference between the virtual paths
and
, which can now be equipped with the probabilities
and
, respectively. The paths are “real” in the sense that each time the experiment is repeated, the pointer will indicate which of the two paths has been traveled, with
and
giving the relative frequencies of each occurrence. Note that the appearance of
, later interpreted as probabilities, is not postulated, but is a consequence of the change in the physical state of the system, caused by its interaction with the meter [
15].
Recalling that whenever post-selection in the state
fails, the system ends up in its orthogonal compliment,
,
, we can add two new paths,
and
, connecting
with
, and traveled with probabilities:
and:
respectively. We now have an exact equivalent of the (upper part of the) model in
Figure 1. The only difference is that this time, it is built from quantum “elements”, rather than from pieces of a classical kit. The rest is a simple exercise in classical statistics. In both cases, we can calculate the averages of the functional
. For example, conditioned by successful post-selection in
, the mean value of
is given by
. We can also choose the operator
to be the projector on the state
, so that its eigenvalues are one and zero, and we have
and
. Now, the mean value of
yields precisely the probability to travel the first path,
Classically, we would arrive at the same result by writing down one whenever the ball passes through , zero if it passes through , add up what is written and divide by the number of balls we used.
The case of measuring the difference of operators
and
, discussed in
Section 6, can be analyzed in a similar manner. There, an accurate meter creates three real paths connecting
and
. The three pathways are
, traveled with a probability ,
, traveled with a probability , and
, traveled with a probability .
Adding three more paths connecting
with
, we obtain a classical stochastic system shown in
Figure 3, similar to that built from the tubes and connectors in
Figure 1. The mean value of the functional
defined in Equation (
16), conditioned by arriving in
, is now twice the difference of the relative frequencies with which the paths
and
are traveled,
To conclude, we return to the remark made at the end of
Section 4. Joining the connectors and tubes in different ways, one may construct completely different statistical networks, which have nothing in common, except their constituent parts. In a similar way, by applying different measurement schemes and measuring different operators to different accuracies, one may engineer equally different stochastic ensembles, having nothing in common except their parent quantum system. Ignoring this fact may lead to various counterfactual paradoxes, some of which disappear once the above remark is taken into account (for more detail, see [
7] and the references therein).
9. Most Inaccurate Measurements and the Meaning of “Indeterminate”
Next, we look at what happens in the opposite limit,
There are several reasons for doing this. Firstly, as in the case of the accurate limit considered in the previous section, the result is universal in the sense that it no longer depends on the particular choice of
and appears to represent some “intrinsic” property of the studied system. Secondly, the observation of the system’s history changes, in general, the probability to end up in the state
, which, when an accurate meter is employed, is different from that without a meter,
This is a well-known example of “one measurement perturbing the other”, and it is tempting to try to get rid of the perturbation and to see what will then occur.
The perturbation disappears if, and and only if, all
G’s in Equation (
18) have approximately the same value,
, so that
in Equation (
19) is
. This can only happen in the limit (
32), when
is made very broad. One immediate consequence of taking the limit (
32) is that the range of possible meter’s readings has widened to occupy the whole real axis,
This gives an operational meaning to the word “indeterminate” that we have often used above. If one wishes to keep interference between paths intact, he/she must choose the meter so inaccurate, that its reading will be arbitrary, leaving the researcher completely ignorant of the value of the functional in question.
It appears that not much can be learned from an individual inaccurate measurement, and we follow [
2] to look instead at the average meter reading,
, obtained as the number of trials
N tends to infinity. Apparently,
must be expressed in terms of the path amplitudes
since, in the absence of probabilities, these are the only quantities quantum mechanics provides for the description of the transition. The authors of [
2] have shown that in the case of
Section 5, the correct expression is:
where:
is the relative amplitude for the path number
i. In the special case of
being the projector onto the state
,
, we also have:
In [
12], the result was extended to any real functional for which, in the absence of a meter, the amplitude for taking a value
f is
,
For example, the average reading of a very inaccurate meter measuring the difference of
and
in the example of
Section 6 is given by:
In general, an average reading of a very inaccurate meter is expressed in terms of the relative amplitudes for the virtual paths connecting the initial and final states. Equations (
37) and (
39) bear uncanny resemblance to Equations (
30) and (
31), in which the probabilities
have been replaced by the corresponding probability amplitudes
, and the real part was chosen to make the result real valued. This may be taken as a hint that the quantity under the
sign, the weak value of a functional
F,
has the universal importance of its accurate (“strong”) counterpart in Equation (
31),
in Equations (
30) and (
31). In the next section, we will show that this cannot be the case and for a good reason.
10. Weak Values and the Uncertainty Principle
As discussed in
Section 8, an accurately measured mean value of the projector onto an intermediate state gives the relative frequency with which the path created by the meter is traveled and, so, serves to distinguish it from other real paths present. What would the result of such measurement mean in the most inaccurate limit, where the path in question exists only as a virtual one? The uncertainty principle states that two virtual paths cannot be distinguished by physical means inside the single real pathway into which they are combined.
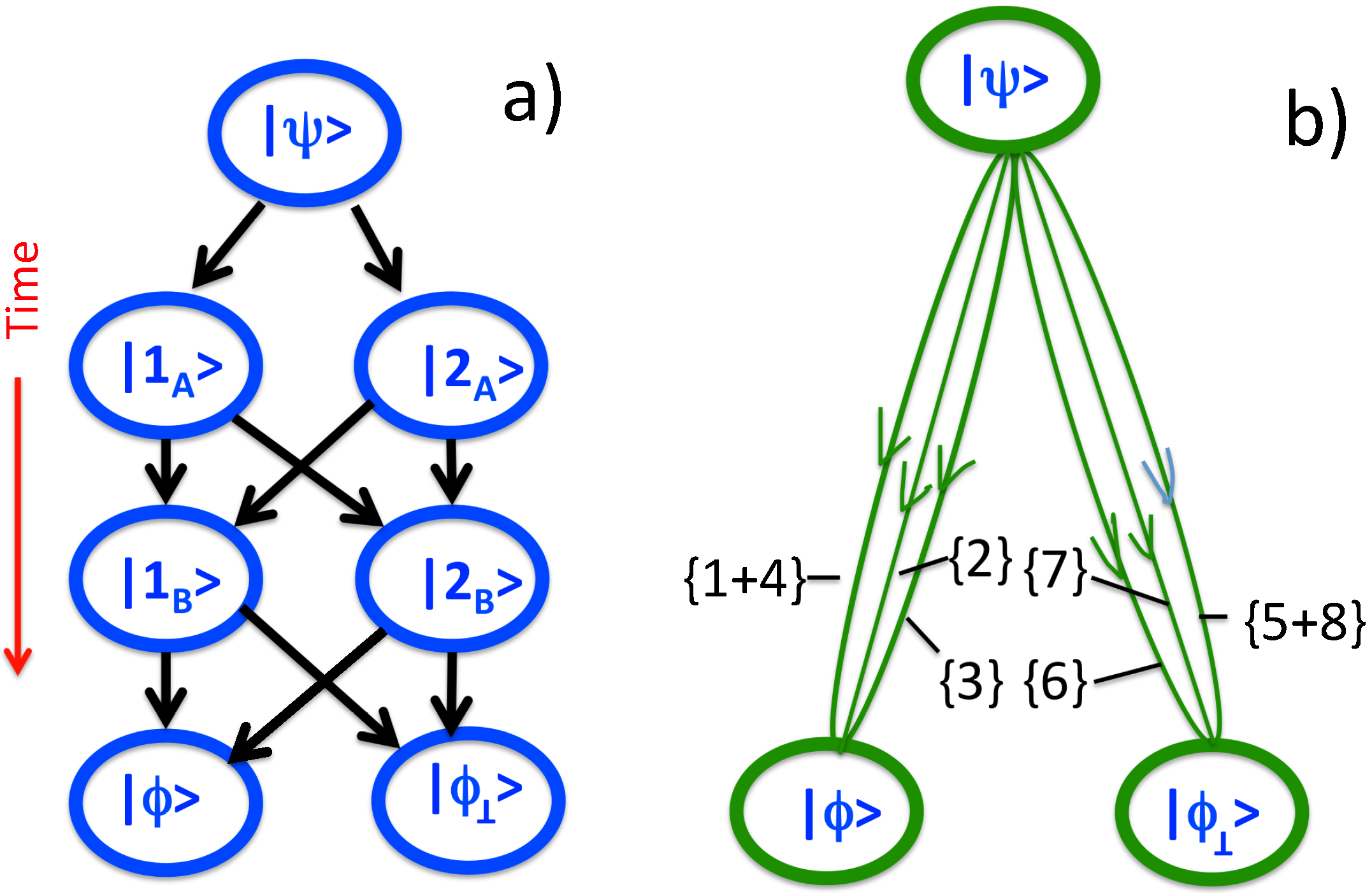
There appears to be a logical rather than a mathematical difficulty. To address it, consider a purely classical conundrum. Suppose there are two drops of water on a glass surface, and we put a grain of sand in Drop Number 1. The drops move closer to each other, and coalesce. In which of the two drops is the sand grain now? If asked, one would certainly reject the question as meaningless, given the new circumstances. However, if an answer is required under pain of death, the individual might reply “inside the Water Drop Number 100, for all I care”. The answer to a meaningless question may be “anything at all”.
The above passage, illustrated in
Figure 4, is not entirely out of place in the present discussion. With two real paths created by an accurate meter, in each trial, the pointer would point at zero or one, indicating the realization of one of the two real possibilities. If the meter is highly inaccurate and the interference between two virtual paths is not destroyed, the pointer is equally likely to point at any number. If asked “was the first or the second virtual path traveled in this transition?”, the meter, unable to politely decline to answer a meaningless question, points at, say, 100. Importantly, this behavior is a necessity, rather than a paradox. It is the Uncertainty Principle that makes the question meaningless, and any other kind of answer would lead to a conflict with the Principle.
Fully aware of the randomness of an individual inaccurate result, the authors of [
2] focused on the meter’s average reading, which, given the same initial and final states, is a uniquely defined quantity, which should come up the same in every long series of trials. This is given by the real part (or the imaginary part, if a slightly different meter is used [
2,
7]) of what looks like an average (
31), but obtained with a complex valued distribution, constructed from the path amplitudes
. We wish to examine its meaning, bearing in mind the restrictions imposed by the UP.
Firstly, it should be remembered that Equation (
38) expresses the average
defined in a standard way by Equation (
20), in terms of the system’s amplitudes
. Secondly, as discussed in
Section 8, the mean pointer reading in an accurate measurement of a projector yields the relative frequency with which the real path in question is traveled. Thus, with
trials performed,
gives the number of trials in which the system has chosen the path. The result makes sense, since
and
. The same cannot be said about the amplitudes
. Choosing
and
and inserting them into Equation (
40), we obtain
. An attempt to interpret
as the number of cases where the first virtual path is traveled in a series of
trials gives a bizarre value of
, another arbitrary answer to a meaningless question.
We need not limit ourselves to measuring projectors. Every functional taking the values can be written as a sum , where takes the values . In an accurate measurement, the mean value of gives the relative frequency with which the j-th path is traveled. In an inaccurate measurement, its value will remain indeterminate and with it, also the value of .
Suppose a group of researchers measure the z-components of the spin of an electron, , at , in a setup where the spin is pre- and post-selected at and . Moreover, all researchers have different initial and final states and . If the measurement is accurate, all researchers will be obtaining the results and , albeit in different proportions. They can all agree that the quantity they measure may take only these two values and use this knowledge to develop theories of spin-1/2 particles, if needed. If the measurement is weak, they can only agree that the spin they measure is able to take any real value.
Suppose further that the equipment is such that each researcher only has access to the average values and not to the distributions. If the measurements are accurate, all choices of
and
would lie between
and
. This gives the knowledge of a useful fact that
represents a quantity whose value cannot be larger than one or less than minus one, although its discreet nature must be learned from some other source. If the measurement is weak, it can be shown [
27] that there is always a choice of
and
which would give
any real value, positive, negative or zero. Thus, after trying all possible
and
, the experimentators will remain in the state of maximal ignorance regarding the properties of the measured quantity.
The mathematical trick that allows the UP to conceal the information about individual virtual paths is based on replacing the path probabilities with path amplitudes when calculating a weak value. It is not so much the fact that
are in general complex valued, but rather the absence of restrictions on the signs of their real and imaginary parts, which can cause the weak value of a projector to be
[
12]. The mean and variance of a non-negative distribution give the estimates for the center and the width of its region of support. The mean and variance of a distribution that can change sign do not have this property, allowing
to lie anywhere on the real axis or obtain a zero variance even when the distribution is not a Dirac delta [
12].
11. Weak Values: Over-Interpretations and Misconceptions
The conclusions of the previous section can be summarized as follows [
7]. A highly inaccurate “weak” measurement (WM) on a pre- and post-selected system is a perturbative scheme, in which an additional system (a vN meter) is weakly coupled to the observed one (e.g., a spin). The coupling is such that the mean value of one of the meter’s variables (pointer position
ξ) coincides with the real (or imaginary, if necessary [
2,
7]) part of a weighted sum of the relative amplitudes on the virtual paths connecting the system’s initial and final states,
In Equation (
42), the paths
,
and the weights
are determined by the nature of the measured quantities. If it is possible (it may not be) to measure directly a functional
, whose values are zero on all paths except the path number
j,
, the mean reading of the weak pointer will yield precisely the real (imaginary) part of the amplitude
. In short, weak measurements measure probability amplitudes.
A certain amount of confusion, which led to a plethora of recent publications on this still fashionable subject (for a recent review, see [
28] and references therein), has its roots in [
2], where the weak values, defined in [
28] as “...complex numbers that one can assign to the powers of a quantum observable operator
using two states, an initial state
called the preparation or preselection, and a final state
called the postselection”, were first introduced. The authors of [
2] considered a weak measurement of the
z-component of a spin-1/2, similar to that described in
Section 5, and concluded that “... the usual measurement procedure for preselected and postselected ensembles of quantum systems gives unusual results.” The title question of the [
2], “How the result of measurement of a component of the spin of a spin-1/2 particle can turn out to be 100?”, has, however, a simple answer. This would happen if the meter measures the difference of relative amplitudes
, for an improbable transition, for which
is small. Surprise at the “unusual results” is also misplaced: the authors of [
2] might as well obtain any other value, large or small, negative, positive or zero. Indeed, the opposite would be surprising, as finding only the values between
and one would contradict the uncertainty principle, as discussed in the previous section.
With weak values described only vaguely as a “new concept” [
2], some authors proposed to use them as a tool for resolving “counterfactual paradoxes”. One simple example is the so-called “three-box case” [
5,
20,
29] (for a path analysis of the so-called Hardy’s paradox [
4] see, for example, [
30]). Here, the final state can be reached via three virtual pathways with amplitudes:
where
C is a complex number. An accurate measurement of a functional
,
always finds the system taking the first path. On the other hand, an accurate measurement of a functional
,
always finds the system taking the third path, and the authors of [
5] alert the reader to “... the peculiarity of having one particle in several places simultaneously even in the stronger sense than in the double slit experiment...”. Admitting that the two results were obtained in different physical circumstances, the authors of [
4] rely on the WM “... to test... the assertions otherwise regarded ascounterfactual”. Their reasoning is as follows. Since WM perturb the system only slightly, it is possible to conduct several of them at the same time [
4] (or, we add, even perform full tomography of the studied transition [
7]). Simultaneous WM of
and
by two meters yield
and
and seemingly confirm the particle’s presence in two places at the same time.
A closer look suggests a far simpler explanation of this “peculiarity”. Different strong measurements “engineer” two completely different classical ensembles (cf. the passage at the end of
Section 8). This becomes clear if one adds all remaining final states and the real pathways leading to them [
20]. The fact that in two different setups the same final state is always reached via paths of a different kind is not surprising at all (we would not give it a second thought if the setups were built from the tubes and connectors of
Section 4). Therefore, what is tested by the simultaneous WM of
and
? We have seen that their results would be:
Since the relative amplitudes always add up to one,
, we also learn that
. Far from asserting that the particle is in “two places”, the weak values give us the values of the real parts of the relative amplitudes. This information is not yet sufficient even for predicting the results of the two accurate measurements, since for this, we also need the imaginary parts of
. Suppose that by making a WM of a different kind, we established that
,
. Now, we know that all
α’s are real, and
and the standard rules of quantum mechanics tell us what would happen in two mutually-exclusive situations, where the paths
and
are combined into a real pathway
or
and
are combined into
, as happens in the two accurate measurements mentioned above. The last shred of mystery, if there were one, disappears when we recall that the perturbation theory typically gives information about both the moduli and the phases of amplitudes and the wave function, and the WM scheme is the rule rather than the exception [
7].
Finally, in a much criticized [
27,
31,
32,
33,
34,
35] paper entitled “How the result of a single coin toss can turn out to be 100 heads” [
36], the authors argue that “weak values are not inherently quantum but rather a purely statistical feature of pre- and postselection with disturbance”. Without going into the details of the author’s reasoning or into the arguments of their critics, one sees that the claim of [
36] must be wrong. To obtain a mean value that lies outside the region of support of a distribution, the distribution must change sign [
12]. Such distributions naturally appear in quantum mechanics, whose basic building blocks are complex valued probability amplitudes [
19]. They may not, however, appear in a classical theory where all physically meaningful averages are taken with non-negative probabilities.
The parallel between the titles of [
2] and [
36], which followed it twenty six years on, are not coincidental. The fallacy of weak classical values in [
36] stems naturally from the suggestion [
2] that the combinations of the transitions amplitudes, which arise when a measurement is extremely inaccurate, amount to a “new concept” of a quantum variable, capable of shedding light on a new physical reality.
12. Conclusions
In summary, we have shown that the application of a sequence of quantum measurements creates a stochastic network. A network is classical in the sense that the probabilities for all observable events have, of course, all the usual properties of probabilities in a classical theory. A network is quantum in its origin, and the action of a meter, or meters, can be seen as a way of “engineering” real scenarios, endowed with probabilities, from the virtual paths of the system, for which only probability amplitudes are available. A network is conveniently described in terms of functionals, which take numerical values on the virtual paths and whose values the measurements are set to determine. In the case of von Neumann and von Neumann-like meters considered here, the real pathways are determined by:
- (i)
the set of virtual paths available for the system if no meter is present.
- (ii)
the values of the measured functional. Two paths sharing the same value cannot be distinguished by the meter(s).
- (iii)
the form and width of the initial state of the pointer, , which determine the accuracy of the measurement.
Highly accurate measurements turn all virtual paths, that can be distinguished, into the real ones, traveled with the probabilities proportional to the squared moduli of the corresponding probability amplitudes. If a functional whose value is 1 on one of the virtual paths and 0 on all others is measured accurately, its mean value yields the relative frequency with which the path is traveled.
If the same functional is measured in a highly inaccurate manner, its mean (weak) value coincides with the real (or imaginary) part of the relative amplitude, corresponding to the selected path. Since there are no a priori restrictions on the values of the relative amplitudes, it is always possible to find a transition in which the result of a weak measurement would be any desired number, large, small, positive or negative. This is a necessary consequence of the uncertainty principle, and the “unusual” weak values lying outside the spectrum of the operator
in the example given in
Section 5 are by no means unusual. Many of exotic interpretations of the weak measurements’ results stem from the failure to identify the weak values with the path amplitudes, or their linear combinations, and are easily dismissed once the problem is reformulated in the language of standard quantum mechanics.