Birkhoff Normal Forms, KAM Theory and Time Reversal Symmetry for Certain Rational Map
Abstract
:1. Introduction
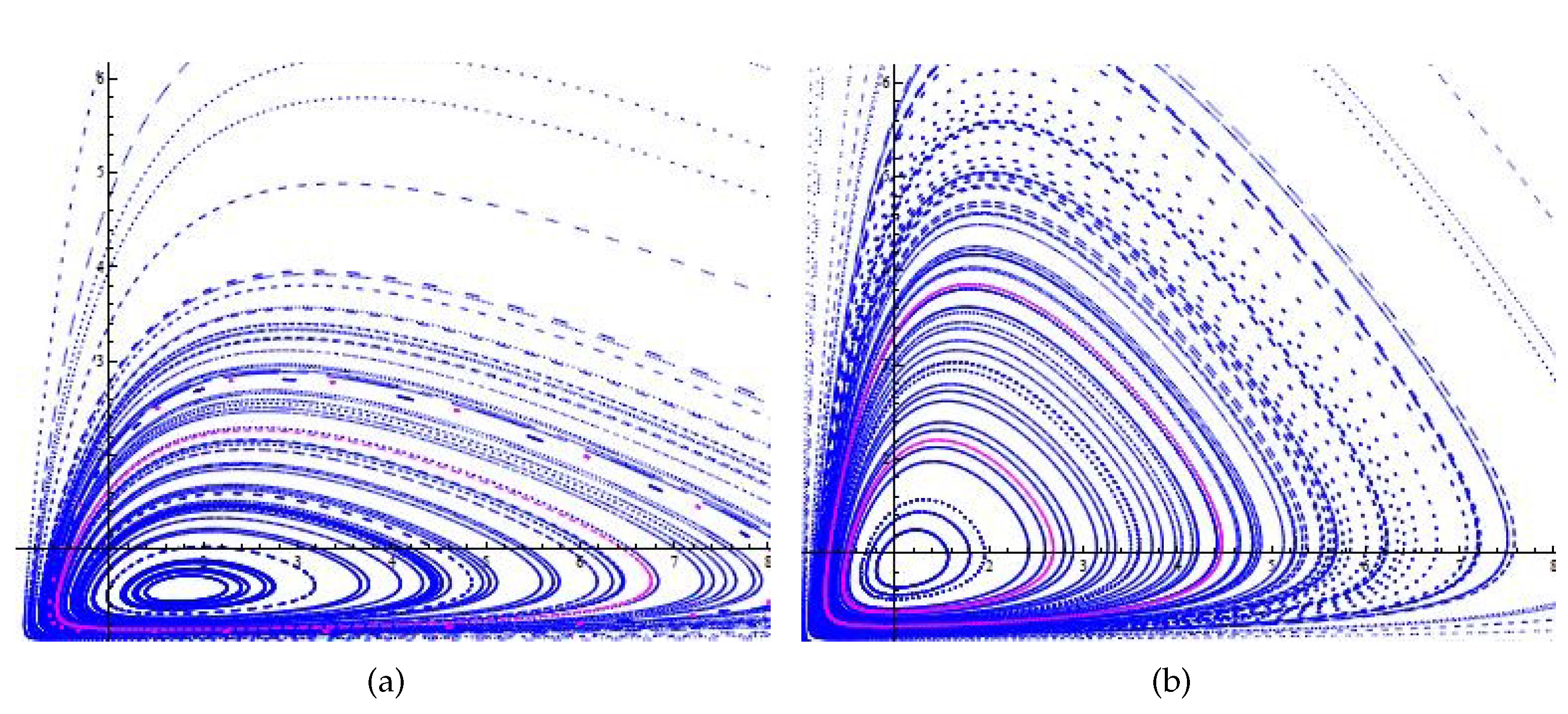
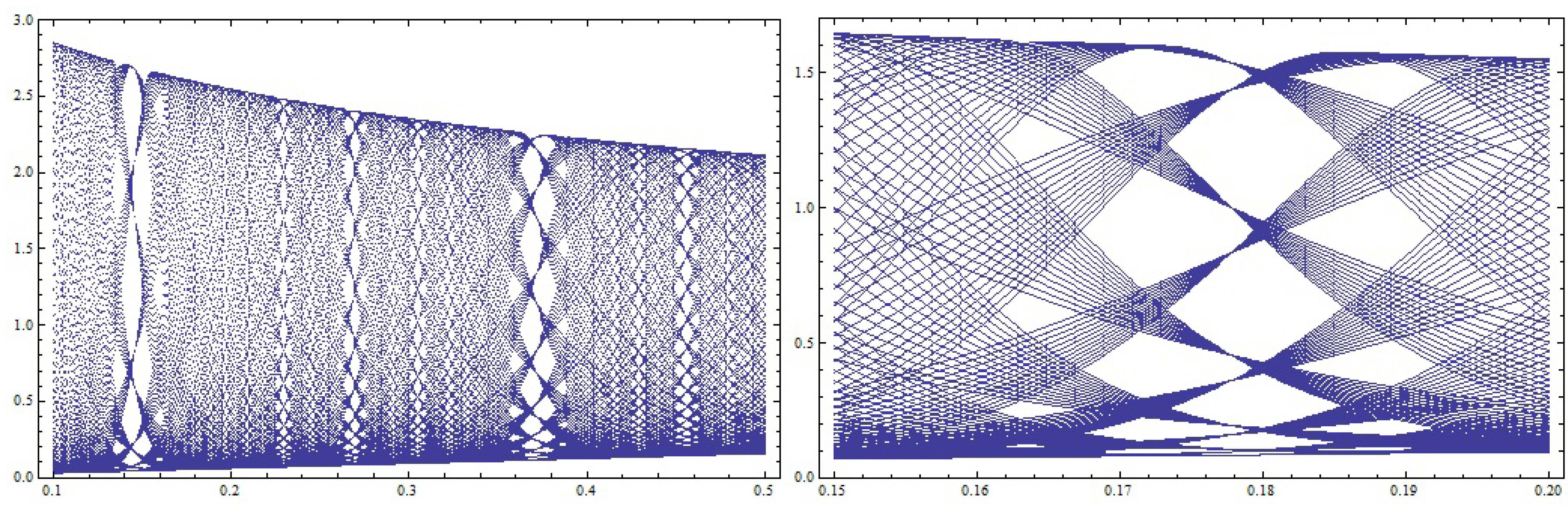
2. The KAM Theory and Birkhoff Normal Form
3. Invariant
4. Symmetries
5. Conclusions
Author Contributions
Conflicts of Interest
References
- Amleh, A.M.; Camouzis, E.; Ladas, G. On the Dynamics of a Rational Difference Equation, Part I. Int. J. Differ. Equ. 2008, 3, 1–35. [Google Scholar]
- Drymmonis, E.; Camouzis, E.; Ladas, G.; Tikjha, G.W. Patterns of boundedness of the rational system and . J. Differ. Equ. Appl. 2012, 18, 89–110. [Google Scholar]
- Feuer, J.; Janowski, E.; Ladas, G. Invariants for some rational recursive sequences with periodic coefficients. J. Differ. Equ. Appl. 1996, 2, 167–174. [Google Scholar] [CrossRef]
- Grove, E.A.; Janowski, E.J.; Kent, C.M.; Ladas, G. On the rational recursive sequence . Comm. Appl. Nonlinear Anal. 1994, 1, 61–72. [Google Scholar]
- Kulenović, M.R.S. Invariants and related Liapunov functions for difference equations. Appl. Math. Lett. 2000, 13, 1–8. [Google Scholar] [CrossRef]
- Kulenović, M.R.S.; Merino, O. Discrete Dynamical Systems and Difference Equations with Mathematica; Chapman and Hall/CRC: Boca Raton, FL, USA; London, UK, 2002. [Google Scholar]
- Chan, D.M.; Kent, C.M.; Ortiz-Robinson, N.L. Convergence results on a second-order rational difference equation with quadratic terms. Adv. Differ. Equ. 2009. [Google Scholar] [CrossRef]
- Dehghan, M.; Kent, C.M.; Mazrooei-Sebdani, R.; Ortiz, N.L.; Sedaghat, H. Monotone and oscillatory solutions of a rational difference equation containing quadratic terms. J. Differ. Equ. Appl. 2008, 14, 1045–1058. [Google Scholar] [CrossRef]
- Dehghan, M.; Mazrooei-Sebdani, R.; Sedaghat, H. Global behaviour of the Riccati difference equation of order two. J. Differ. Equ. Appl. 2011, 17, 467–477. [Google Scholar] [CrossRef]
- Kulenović, M.R.S.; Moranjkić, S.; Nurkanović, Z. Naimark-Sacker bifurcation of second order rational difference equation with quadratic terms. J. Nonlinear Sci. Appl. 2016, in press. [Google Scholar]
- Cima, A.; Gasull, A.; Manosa, V. Non-integrability of measure preserving maps via Lie symmetries. J. Differ. Equ. 2015, 259, 5115–5136. [Google Scholar] [CrossRef]
- Kulenović, M.R.S.; Nurkanović, Z.; Pilav, E. Birkhoff Normal Forms and KAM theory for Gumowski-Mira Equation. Sci. World J. Math. Anal. 2014. [Google Scholar] [CrossRef] [PubMed]
- Gidea, M.; Meiss, J.D.; Ugarcovici, I.; Weiss, H. Applications of KAM Theory to Population Dynamics. J. Biol. Dyn. 2011, 5, 44–63. [Google Scholar] [CrossRef] [PubMed]
- Kocic, V.L.; Ladas, G.; Tzanetopoulos, G.; Thomas, E. On the stability of Lyness’ equation. Dynam. Contin. Discrete Impuls. Syst. 1995, 1, 245–254. [Google Scholar]
- Kulenović, M.R.S.; Nurkanović, Z. Stability of Lyness’ Equation with Period-Two Coefficient via KAM Theory. J. Concr. Appl. Math. 2008, 6, 229–245. [Google Scholar]
- Ladas, G.; Tzanetopoulos, G.; Tovbis, A. On May’s host parasitoid model. J. Differ. Equ. Appl. 1996, 2, 195–204. [Google Scholar] [CrossRef]
- Hrustić, S.J.; Kulenović, M.R.S.; Nurkanović, Z.; Pilav, E. Birkhoff Normal Forms, KAM theory and Symmetries for Certain Second Order Rational Difference Equation with Quadratic Term. Int. J. Differ. Equ. 2015, 10, 181–199. [Google Scholar]
- Hale, J.K.; Kocak, H. Dynamics and Bifurcation; Springer-Verlag: New York, NY, USA, 1991. [Google Scholar]
- Tabor, M. Chaos and Integrability in Nonlinear Dynamics: An introduction; A Wiley-Interscience Publication; John Wiley & Sons, Inc.: New York, NY, USA, 1989. [Google Scholar]
- Del-Castillo-Negrete, D.; Greene, J.M.; Morrison, E.J. Area preserving nontwist maps: Periodic orbits and transition to chaos. Phys. D 1996, 91, 1–23. [Google Scholar] [CrossRef]
- Wan, Y.H. Computation of the stability condition for the Hopf bifurcation of diffeomorphisms on . SIAM J. Appl. Math. 1978, 34, 167–175. [Google Scholar] [CrossRef]
- Moeckel, R. Generic bifurcations of the twist coefficient. Ergodic Theory Dyn. Syst. 1990, 10, 185–195. [Google Scholar] [CrossRef]
- MacKay, R.S. Renormalization in Area-Preserving Maps; World Scientific: River Edge, NJ, USA, 1993. [Google Scholar]
- Siegel, C.; Moser, J. Lectures on Celestial Mechanics; Springer-Varlag: New York, NY, USA, 1971. [Google Scholar]

© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Denette, E.; Kulenović, M.R.S.; Pilav, E. Birkhoff Normal Forms, KAM Theory and Time Reversal Symmetry for Certain Rational Map. Mathematics 2016, 4, 20. https://doi.org/10.3390/math4010020
Denette E, Kulenović MRS, Pilav E. Birkhoff Normal Forms, KAM Theory and Time Reversal Symmetry for Certain Rational Map. Mathematics. 2016; 4(1):20. https://doi.org/10.3390/math4010020
Chicago/Turabian StyleDenette, Erin, Mustafa R. S. Kulenović, and Esmir Pilav. 2016. "Birkhoff Normal Forms, KAM Theory and Time Reversal Symmetry for Certain Rational Map" Mathematics 4, no. 1: 20. https://doi.org/10.3390/math4010020
APA StyleDenette, E., Kulenović, M. R. S., & Pilav, E. (2016). Birkhoff Normal Forms, KAM Theory and Time Reversal Symmetry for Certain Rational Map. Mathematics, 4(1), 20. https://doi.org/10.3390/math4010020





