Limit Theorems for the Non-Convex Multispecies Curie–Weiss Model
Abstract
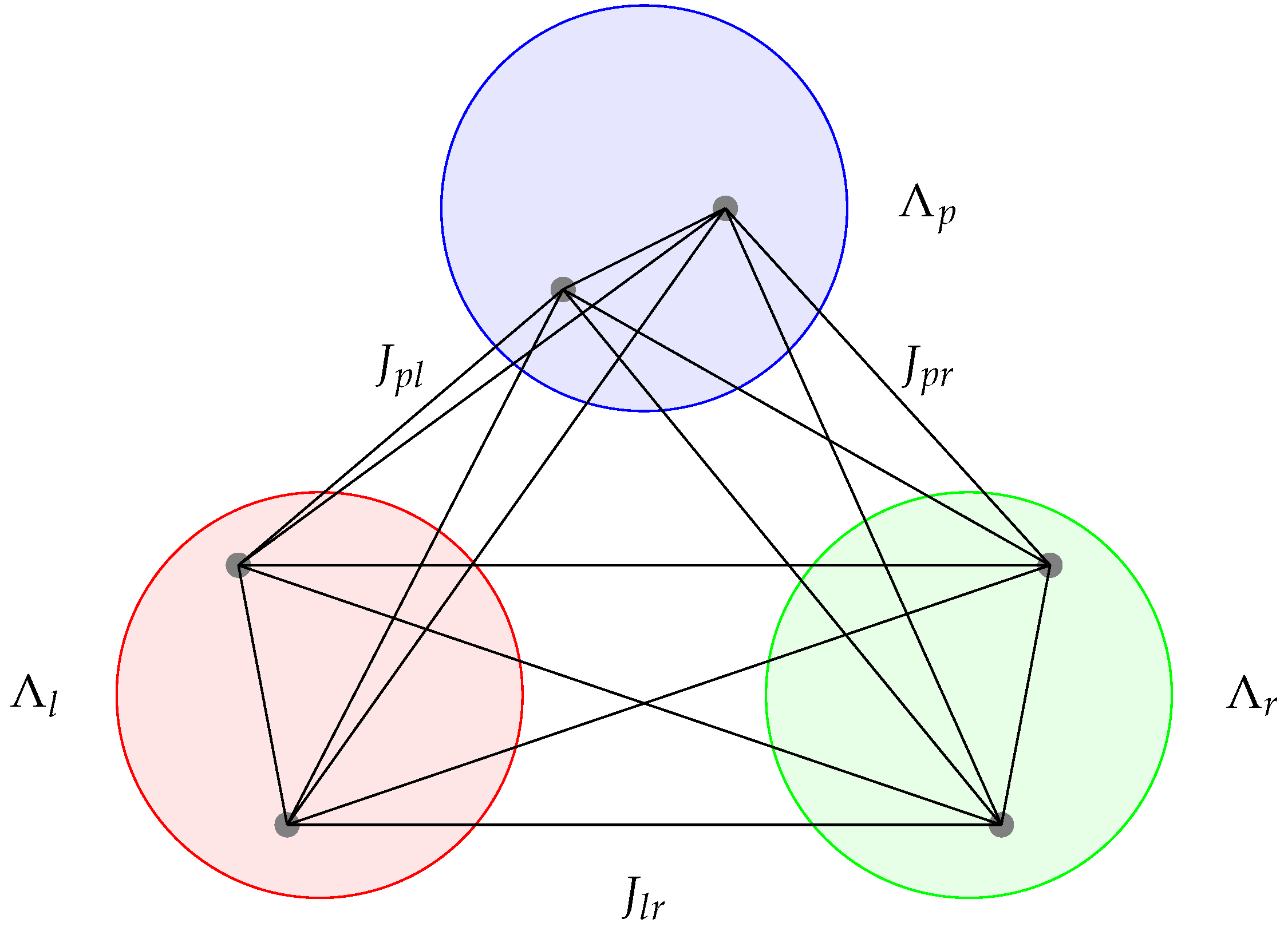
1. Introduction
2. Model Description and Definitions
3. Main Results
3.1. Thermodynamic Limit of the Generating Functional
3.2. Fluctuations of the Magnetization
4. Proofs
4.1. Proof of Theorem 1
4.2. Proof of Theorem 2
4.3. Proof of Theorem 3
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Technical Tools
Appendix B. Approximation Lemmas
References
- Kac, M. MATHEMATICAL MECHANISMS OF PHASE TRANSITIONS. In Statistical Physics, Phase Transitions, and Superfluidity; Chretien, M., Gross, E.P., Deser, S., Eds.; Gordon and Breach, Science Publishers: New York, NY, USA, 1968; Volume I, pp. 241–305. [Google Scholar]
- Kirsch, W.; Langner, J. The fate of the square root law for correlated voting. In Studies in Choice and Welfare; Springer International Publishing: Cham, Switzerland, 2014; pp. 147–158. [Google Scholar]
- Kirsch, W. A mathematical view on voting and power. In Mathematics and Society; EMS Press: Zuerich, Switzerland, 2016; pp. 251–279. [Google Scholar]
- Gsänger, M.; Hösel, V.; Mohamad-Klotzbach, C.; Müller, J. Opinion models, election data, and political theory. Entropy 2024, 26, 212. [Google Scholar] [CrossRef] [PubMed]
- Brock, W.A.; Durlauf, S.N. Discrete choice with social interactions. Rev. Econ. Stud. 2001, 68, 235–260. [Google Scholar] [CrossRef]
- Blume, L.; Durlauf, S. Equilibrium concepts for social interaction models. Int. Game Theory Rev. 2003, 5, 193–209. [Google Scholar] [CrossRef]
- Marsman, M.; Tanis, C.C.; Bechger, T.M.; Waldorp, L.J. Network psychometrics in educational practice: Maximum likelihood estimation of the Curie-Weiss model. In Theoretical and Practical Advances in Computer-based Educational Measurement; Springer International Publishing: Cham, Switzerland, 2019; pp. 93–120. [Google Scholar]
- Contucci, P.; Ghirlanda, S. Modelling society with statistical mechanics: An application to cultural contact and immigration. Qual. Quant. 2007, 41, 569–578. [Google Scholar] [CrossRef]
- Burioni, R.; Contucci, P.; Fedele, M.; Vernia, C.; Vezzani, A. Enhancing participation to health screening campaigns by group interactions. Sci. Rep. 2015, 5, 9904. [Google Scholar] [CrossRef]
- Opoku, A.A.; Osabutey, G.; Kwofie, C. Parameter evaluation for a statistical mechanical model for binary choice with social interaction. J. Probab. Stat. 2019, 2019, 3435626. [Google Scholar] [CrossRef]
- Frøyen, S.; Sudbø, A.S.; Hemmer, P. Ising models with two-and three-spin interactions: Mean field equation of state. Phys. A Stat. Mech. Its Appl. 1976, 85, 399–408. [Google Scholar] [CrossRef]
- Bidaux, R.; Boccara, N.; Forgàcs, G. Three-spin interaction Ising model with a nondegenerate ground state at zero applied field. J. Stat. Phys. 1986, 45, 113–134. [Google Scholar] [CrossRef]
- Kincaid, J.M.; Cohen, E.G.D. Phase diagrams of liquid helium mixtures and metamagnets: Experiment and mean field theory. Phys. Rep. 1975, 22, 57–143. [Google Scholar] [CrossRef]
- Galam, S.; Yokoi, C.S.O.; Salinas, S.R. Metamagnets in uniform and random fields. Phys. Rev. B 1998, 57, 8370–8374. [Google Scholar] [CrossRef]
- Gallo, I.; Contucci, P. Bipartite mean field spin systems. Existence and solution. Math. Phys. Electron. J. 2008, 14, 1–21. [Google Scholar]
- Genovese, G.; Barra, A. A certain class of Curie-Weiss models. arXiv 2009, arXiv:0906.4673. [Google Scholar]
- Barra, A.; Genovese, G.; Guerra, F. Equilibrium statistical mechanics of bipartite spin systems. J. Phys. A Math. Theor. 2011, 44, 245002. [Google Scholar] [CrossRef]
- Genovese, G.; Tantari, D. Non-convex multipartite ferromagnets. J. Stat. Phys. 2016, 163, 492–513. [Google Scholar] [CrossRef]
- Fedele, M.; Contucci, P. Scaling limits for multi-species statistical mechanics mean-field models. J. Stat. Phys. 2011, 144, 1186–1205. [Google Scholar] [CrossRef]
- Ellis, R.S.; Newman, C.M. The statistics of Curie-Weiss models. J. Stat. Phys. 1978, 19, 149–161. [Google Scholar] [CrossRef]
- Löwe, M.; Schubert, K. Fluctuations for block spin Ising models. Electron. Commun. Probab. 2018, 23, 1–12. [Google Scholar] [CrossRef]
- Knöpfel, H.; Löwe, M.; Schubert, K.; Sinulis, A. Fluctuation results for general block spin Ising models. J. Stat. Phys. 2020, 178, 1175–1200. [Google Scholar] [CrossRef]
- Kirsch, W.; Toth, G. Two groups in a Curie-Weiss model. Math. Phys. Anal. Geom. 2020, 23, 17. [Google Scholar] [CrossRef]
- Fleermann, M.; Kirsch, W.; Toth, G. Local central limit theorem for multi-group Curie–Weiss models. J. Theor. Probab. 2022, 35, 2009–2019. [Google Scholar] [CrossRef]
- Kirsch, W.; Toth, G. Limit theorems for multi-group Curie–Weiss models via the method of moments. Math. Phys. Anal. Geom. 2022, 25, 24. [Google Scholar] [CrossRef]
- Barra, A.; Contucci, P.; Mingione, E.; Tantari, D. Multi-species mean field spin glasses. Rigorous results. In Proceedings of the Annales Henri Poincaré; Springer: Basel, Switzerland, 2015; Volume 16, pp. 691–708. [Google Scholar] [CrossRef]
- Panchenko, D. The free energy in a multi-species Sherrington–Kirkpatrick model. Ann. Probab. 2015, 43, 3494–3513. [Google Scholar] [CrossRef]
- Baik, J.; Lee, J.O. Free energy of bipartite spherical Sherrington–Kirkpatrick model. Ann. Inst. Henri Poincaré 2020, 56, 2897–2934. [Google Scholar] [CrossRef]
- Subag, E. TAP approach for multispecies spherical spin glasses II: The free energy of the pure models. Ann. Probab. 2023, 51, 1004–1024. [Google Scholar] [CrossRef]
- Alberici, D.; Camilli, F.; Contucci, P.; Mingione, E. The Multi-species Mean-Field Spin-Glass on the Nishimori Line. J. Stat. Phys. 2021, 182, 2. [Google Scholar] [CrossRef]
- Alberici, D.; Camilli, F.; Contucci, P.; Mingione, E. The Solution of the Deep Boltzmann Machine on the Nishimori Line. Commun. Math. Phys. 2021, 387, 1191–1214. [Google Scholar] [CrossRef]
- Mourrat, J.C. Nonconvex interactions in mean-field spin glasses. arXiv 2020, arXiv:2004.01679. [Google Scholar]
- Hong-Bin Chen, J.C.M. On the free energy of vector spin glasses with non-convex interactions. arXiv 2023, arXiv:2311.08980. [Google Scholar]
- Guerra, F.; Lucio Toninelli, F. Central limit theorem for fluctuations in the high temperature region of the Sherrington–Kirkpatrick spin glass model. J. Math. Phys. 2002, 43, 6224–6237. [Google Scholar] [CrossRef]
- Guerra, F.; Toninelli, F.L. Quadratic replica coupling in the Sherrington–Kirkpatrick mean field spin glass model. J. Math. Phys. 2002, 43, 3704–3716. [Google Scholar] [CrossRef]
- Talagrand, M. Spin Glasses: A Challenge for Mathematicians: Cavity and Mean Field Models; Springer: Berlin/Heidelberg, Germany; Springer Science & Business Media: New York, NY, USA, 2003; Volume 46. [Google Scholar]
- Camilli, F.; Contucci, P.; Mingione, E. Central limit theorem for the overlaps on the Nishimori line. arXiv 2023, arXiv:2305.19943. [Google Scholar]
- Dey, P.; Wu, Q. Fluctuation Results for Multi-species Sherrington-Kirkpatrick Model in the Replica Symmetric Regime. J. Stat. Phys. 2021, 185. [Google Scholar] [CrossRef]
- Zimmaro, F.; Galam, S.; Javarone, M.A. Asymmetric games on networks: Mapping to Ising models and bounded rationality. Chaos Solitons Fractals 2024, 181, 114666. [Google Scholar] [CrossRef]
- Agliari, E.; Migliozzi, D.; Tantari, D. Non-convex multi-species Hopfield models. J. Stat. Phys. 2018, 172, 1247–1269. [Google Scholar] [CrossRef]
- Contucci, P.; Vernia, C. On a statistical mechanics approach to some problems of the social sciences. Front. Phys. 2020, 8, 585383. [Google Scholar] [CrossRef]
- Ignacio, G.; Barra, A.; Contucci, P. Parameter evaluation of a simple mean-field model of social interaction. Math. Models Methods Appl. Sci 2009, 19, 1427–1439. [Google Scholar]
- Mukherjee, S.; Son, J.; Bhattacharya, B.B. Fluctuations of the magnetization in the p-spin Curie-Weiss model. Comm. Math. Phys. 2021, 387, 681–728. [Google Scholar] [CrossRef]
- Contucci, P.; Mingione, E.; Osabutey, G. Limit theorems for the cubic mean-field Ising model. Ann. Henri Poincare 2024, 25, 5019–5044. [Google Scholar] [CrossRef]
- Dembo, A.; Zeitouni, O. Large Deviations Techniques and Applications; Springer: Berlin, Germany, 2010. [Google Scholar]
- Opoku, A.A.; Osabutey, G. Multipopulation spin models: A view from large deviations theoretic window. J. Math. 2018, 2018, 1–13. [Google Scholar] [CrossRef]
- Eisele, T.; Ellis, R.S. Multiple phase transitions in the generalized Curie-Weiss model. J. Stat. Phys. 1988, 52, 161–202. [Google Scholar] [CrossRef]
- Collet, F. Macroscopic limit of a bipartite Curie–Weiss model: A dynamical approach. J. Stat. Phys. 2014, 157, 1301–1319. [Google Scholar] [CrossRef]
- Fedele, M.; Unguendoli, F. Rigorous results on the bipartite mean-field model. J. Phys. A Math. Theor. 2012, 45, 385001. [Google Scholar] [CrossRef]
- Zhao, K.; Bianconi, G. Percolation on interdependent networks with a fraction of antagonistic interactions. J. Stat. Phys. 2013, 152, 1069–1083. [Google Scholar] [CrossRef]
- Andreis, L.; Tovazzi, D. Coexistence of stable limit cycles in a generalized Curie–Weiss model with dissipation. J. Stat. Phys. 2018, 173, 163–181. [Google Scholar] [CrossRef]
- Ayi, N.; Pouradier Duteil, N. Mean-field and graph limits for collective dynamics models with time-varying weights. J. Differ. Equations 2021, 299, 65–110. [Google Scholar] [CrossRef]
- Giacomin, G.; Poquet, C. Noise, interaction, nonlinear dynamics and the origin of rhythmic behaviors. Brazilian Jounal of Probab. Stat 2015, 29, 460–493. [Google Scholar]
- Collet, F.; Formentin, M.; Tovazzi, D. Rhythmic behavior in a two-population mean-field Ising model. Phys. Rev. E 2016, 94, 042139. [Google Scholar] [CrossRef]
- Salwinski, D. The Continuous Binomial Coefficient: An Elementary Approach. Am. Math. Mon. 2018, 125, 231–244. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Camilli, F.; Mingione, E.; Osabutey, G. Limit Theorems for the Non-Convex Multispecies Curie–Weiss Model. Mathematics 2025, 13, 1343. https://doi.org/10.3390/math13081343
Camilli F, Mingione E, Osabutey G. Limit Theorems for the Non-Convex Multispecies Curie–Weiss Model. Mathematics. 2025; 13(8):1343. https://doi.org/10.3390/math13081343
Chicago/Turabian StyleCamilli, Francesco, Emanuele Mingione, and Godwin Osabutey. 2025. "Limit Theorems for the Non-Convex Multispecies Curie–Weiss Model" Mathematics 13, no. 8: 1343. https://doi.org/10.3390/math13081343
APA StyleCamilli, F., Mingione, E., & Osabutey, G. (2025). Limit Theorems for the Non-Convex Multispecies Curie–Weiss Model. Mathematics, 13(8), 1343. https://doi.org/10.3390/math13081343





