Analysis of Mild Extremal Solutions in Nonlinear Caputo-Type Fractional Delay Difference Equations

Abstract

This study investigates extremal solutions for fractional-order delayed difference equations, utilizing the Caputo nabla operator to establish mild lower and upper approximations via discrete fractional calculus. A new approach is employed to demonstrate the uniform convergence of the sequences of lower and upper approximations within the monotone iterative scheme using the summation representation of the solutions, which serves as a discrete analogue to Volterra integral equations. This research highlights practical applications through numerical simulations in discrete bidirectional associative memory neural networks.

1. Introduction

Fractional derivatives offer precise modeling of diverse natural phenomena [1,2]. Despite limited exploration, fractional difference equations—discrete analogs to continuous fractional dynamical systems—are increasingly being studied, particularly for delay components. The existence and uniqueness of time-delayed nabla fractional systems are established in [3,4]. For a detailed overview of discrete fractional calculus, refer to monograph [5]. In engineering applications like missile guidance and robotics, maintaining system trajectories within limits prevents control loss or threshold breaches [6]. The study of dynamic behaviors under external influences is detailed in generalized oscillator models [7]. The monograph [8] thoroughly examines the existence and stability of solutions for fractional difference equations, addressing delays and impulsive effects using fixed point theorems and upper–lower approximations. The study [9] investigates Ulam stability in delay fractional difference equations using Gronwall’s discrete inequality, enhancing understanding in neural networks. In the research [10], memory effects in neural networks are addressed through variable-order fractional discrete-time recurrent neural networks, including applications of fractional discrete-time neural network associated with standard Hopfield-type fractional neural networks:

$${}_{t_0}{}^{C}D_t^{\alpha }{x}_i\left(t\right)=-c_i{x}_i\left(t\right)+\sum _{j=1}^n{a}_{ij}{f}_j\left(x_j\left(t\right)\right)+I_i,i=1,2,\dots ,n$$

or

$${}_{t_0}{}^{C}D_t^{\alpha }x\left(t\right)=-Cx\left(t\right)+Af\left(x\left(t\right)\right)+I,$$

where $x={(x_1,x_2,\dots ,x_n)}^T\in {\mathbb{R}}^n$ represents the state vector; $C=\mathrm{diag}(c_1,c_2,\dots ,c_n)$, with $0<c_i$, denotes the state feedback coefficients; $A={\left(a_{ij}\right)}_{n\times n}$ is the network’s interconnection matrix; $f\left(x\right)$ is the activation function; and I indicates the constant external input. Short memory models are proposed with stability analysis via fixed point techniques, and the applications extend to chaos control and diffusion modeling.

The aforementioned studies emphasize the pivotal role of fractional calculus in modeling neural network memory, serving as a primary motivation for this research aimed at identifying discrete analogs for these systems. Previous numerical solutions have encountered challenges due to cumulative errors from infinite-memory effects, numerical truncation, and convergence issues. To address these challenges effectively within applications like bidirectional associative memory neural networks, this study proposes utilizing discrete fractional calculus on isolated time scales.

Recent studies on Caputo, Riemann–Liouville, and impulsive differential equations with generalized proportional derivatives utilize upper–lower solutions as well as monotone iterative techniques [11,12,13,14,15] and for Caputo-type fractional nabla difference equations [16]. The Banach–Schauder fixed point theorem confirms solution existence under nonlinear delta scenarios with delays/impulses [17]. Challenges in fractional differential/difference equations arise from non-linearity and delays. Various methods combine upper–lower solutions with monotone iterative techniques. One approach uses explicit formulas for linear inhomogeneous equation approximation [12]; supposing Lipschitz condition, another selects analytic properties of functions within problem statements specific to difference contexts [13,16]. This article examines a Caputo-type fractional nabla difference equation ($\mathcal{CFNDE}$) using the sum of two monotonic functions on the right-hand side. Approximation results utilize summation equations, defining mild extremal solutions according to the methods of Agarwal et al. [13], establishing sufficient conditions for monotonicity and uniform convergence. In related work, [18] presents key insights into the overconvergence of functional series.

In the present study, a monotone iterative method utilizing coupled mild lower and upper solutions is employed to approximate the exact solution of nabla fractional delayed difference equations. The impact of fractional operator orders is examined through numerical simulations. Integral representations are essential for analyzing solution behavior and stability in fractional differential equations or systems with delays [19,20]. Discrete counterparts are utilized within iterative algorithms in this study. Instability often arises from undetected disturbances and ineffective management due to time delays. Consequently, significant efforts have been dedicated by scientists and engineers to studying the stability of fractional neural networks with time delays. For asymptotic stability in nonlinear nabla fractional difference equations with bounded time delays, refer to [21]. Applications for variable-order systems are discussed in [22]. In [23], sufficient conditions for existence, uniqueness, and uniform stability of non-trivial solutions in discrete fractional-order neural networks with leakage delay were established using discrete fractional calculus along with mathematical inequalities and fixed point theorems.

This research addresses the following aspects:

(I)

The introduction of an iterative method employing mild upper and lower solution approximations for Caputo fractional nabla delayed difference equations.

(II)

The establishment of a novel analytical framework demonstrating uniform convergence of successive approximation sequences, grounded in fundamental real and functional analysis principles.

(III)

The demonstration of the algorithm’s practical utility within Bidirectional Associative Memory (BAM) neural networks, demonstrating its effectiveness in improving network performance through innovative applications.

The subsequent sections of this paper are structured as follows: Section 2 explains foundational concepts and definitions related to discrete fractional calculus relevant to our study. Section 3 is dedicated to applying the outlined methodology for deriving the main results. In Section 4, illustrative examples with computer simulations are presented.

2. Background of the Main Results

2.1. Fractional Difference Calculus: Definitions

This section explains the basic concepts of the $\vartheta$-order Caputo-type nabla fractional operator. Let $t_0$ and T be integers. The following sets will be utilized: ${\mathbb{Z}}_{t_0}=\{t_0,t_0+1,\dots \},{\mathbb{Z}}_{t_0}^T=\left\{t_0,t_0+1,\dots ,T\right\}.$

Definition 1

(Def. 2.2., [24]). Let $\rho \left(t\right):=t-1$ denote the backward jump operator. Then, the (nabla) left fractional sum of order $\vartheta >0$ is defined by

$$\left({\nabla }_{t_0}^{-\vartheta }x\right)\left(t\right)={\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{s=t_0+1}^t{\left(t-\rho \left(s\right)\right)}^{\overline{\vartheta -1}}x\left(s\right),t\in {\mathbb{Z}}_{t_0+1}$$

where $t^{\overline{\vartheta }}={\displaystyle \frac{\Gamma (t+\vartheta )}{\Gamma \left(t\right)}},t\in \mathbb{R}\{\dots ,-2,-1,0\}$ is the (generalized) rising function with $0^{\overline{\vartheta }}=0$, and $\left({\nabla }_{t_0}^{-\vartheta }x\right)\left(t_0\right):=0.$

Lemma 1

(Lemma 2.2., [25]). Assume that the following properties of the rising function are well defined:

(i) 

$t^{\overline{\vartheta }}{(t+\vartheta )}^{\overline{\mu }}=t^{\overline{\vartheta +\mu }};$

(ii) 

If $t\le s$ then $t^{\overline{\vartheta }}\le s^{\overline{\vartheta }};$

(iii) 

If $\vartheta <t\le s$ then $s^{\overline{-\vartheta }}\le t^{\overline{-\vartheta }}.$

Theorem 1

(Th. 2.4., [25]). Consider $\vartheta >0$ and $\mu >-1.$ It can be concluded that

$${\nabla }_{t_0}^{-\vartheta }{(t-t_0)}^{\overline{\mu }}={\displaystyle \frac{\Gamma (\mu +1)}{\Gamma (\mu +\vartheta +1)}}{(t-t_0)}^{\overline{\mu +\vartheta }},t\in {\mathbb{Z}}_{t_0}.$$

Definition 2

(Def. 3.117, [5]). Let $x:{\mathbb{Z}}_{t_0}\to \mathbb{R}.$ The Caputo fractional nabla difference for $0<\vartheta \le 1$ is defined as follows:

$$\left({\nabla }_{t_0^{\ast }}^{\vartheta }x\right)\left(t\right)=\left({\nabla }_{t_0}^{-(1-\vartheta )}\nabla x\right)\left(t\right), t\in {\mathbb{Z}}_{t_0},$$

by convention $\left({\nabla }_{t_0^{\ast }}^{\vartheta }x\right)\left(t_0\right):=0$.

Theorem 2

(Th. 3.119, [5]). Let $x:{\mathbb{Z}}_{t_0}\to \mathbb{R}$. For $0<\vartheta \le 1$, it can be concluded that

$$x\left(t\right)=x\left(t_0\right)+\left({\nabla }_{t_0}^{-\vartheta }{\nabla }_{t_0^{\ast }}^{\vartheta }x\right)\left(t\right), t{\in \mathbb{Z}}_{t_0}$$

2.2. Problem Set Up and Preliminaries

We examine the initial value problem ($\mathcal{IVP}$) for nonlinear $\mathcal{CFNDE}$ with a constant delay, expressed as follows:

$$\begin{array}{cc}\hfill & \left({\nabla }_{0\ast }^{\vartheta }x\right)\left(t\right)=u\left(t,x\left(t\right),x(t-\tau )\right)+w\left(t,x\left(t\right),x(t-\tau )\right),t\in {\mathbb{Z}}_0^T\hfill \\ \hfill & x\left(t\right)=\varphi \left(t\right),t\in {\mathsf{\Omega }}_0,\tau \ge 0,\hfill \end{array}$$

(1)

where $u,w:{\mathbb{Z}}_0^T\times \mathbb{R}\times \mathbb{R}\to \mathbb{R},$ are continuous in their second and third variables, with u being non-decreasing and w non-increasing, with respect to these variables on ${\mathbb{Z}}_0^T$. Additionally, $\varphi :{\mathsf{\Omega }}_0\to \mathbb{R}$, where ${\mathsf{\Omega }}_0={\mathbb{Z}}_{-\tau }^0,\tau \ge 0,$ and $\left({\nabla }_{0\ast }^{\vartheta }x\right)$ denotes the Caputo fractional difference of order $\vartheta \in (0,1)$.

Lemma 2

(Lemma 1, [26]). A function $x:{\mathbb{Z}}_{-\tau }^T\to \mathbb{R}$ is a solution to Equation (1) if, and only if, the following representation holds

$$x\left(t\right)=\left\{\begin{array}{cc}\varphi \left(t\right),& t\in {\mathsf{\Omega }}_0,\\ \varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}{\displaystyle \sum _{j=1}^t}{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u(j,x(j),x(j-\tau \left)\right)+w(j,x(j),x(j-\tau \left)\right)\right),& t\in {\mathbb{Z}}_0^T.\end{array}\right.$$

(2)

Definition 3.

The function $x:{\mathbb{Z}}_{-\tau }^T\to \mathbb{R}$ is termed a mild solution of the $\mathcal{IVP}$ for $\mathcal{CFNDE}$ (1) if it satisfies (2).

Remark 1.

Previous studies, specifically [26,27] have provided the representation denoted by (2). It serves as a fundamental basis for our main results, exploring cases without guaranteed unique solutions. Such inquiries into equations similar to Equation (1), with arbitrary nonlinear functions on the right-hand side, have been examined in [26] for the discrete domains and in [11,27] for the continuous domains.

The exposition below requires definitions from real and functional analysis [28,29].

Definition 4.

Suppose that ${\left\{y_n\right\}}_{n=0}^{\infty }$ is a sequence of functions $y_n:S\to \mathbb{R}$.

(i) 

Pointwise Convergence: A sequence is said to converge pointwise on S if, for every $t\in S$, the limit

$$\underset{n\to \infty }{lim}{y}_n\left(t\right)=y\left(t\right).$$

(ii) 

Uniformly Cauchy: The sequence is uniformly Cauchy if, for every $ϵ>0$, there exists $N\in {\mathbb{Z}}_1$ such that $|y\left(t_1\right)-y\left(t_2\right)| <ϵ$ whenever $t_1,t_2\in {\mathbb{Z}}_{N+1}$ for any $y=\left\{y_n\left(t\right)\right\}$ in $S.$

Let $S^{\infty }$ represent the space of all real sequences defined over the positive integers, each bounded with respect to the supremum norm. It is evident that $S^{\infty }$ is a Banach space under this norm.

Define the set

$$\mathcal{B}=\{y=\left\{y_n\right\}\in S^{\infty }:\parallel y\parallel \le \rho \}.$$

Theorem 3.

Let $S\subseteq \mathbb{R}.$ The sequence of real function $\left\{y_n\right\}$ is uniformly Cauchy on S if, and only if, it converges uniformly on S.

Remark 2.

An analogy exists between uniform and pointwise convergence, similar to the relationship between continuous and uniformly continuous functions. Uniform versions require a single parameter $(N$ or $\delta )$ that applies universally across the domain, while pointwise definitions allow parameters to vary with each specific point. This distinction highlights a fundamental difference in controlling variation across different types of convergence and continuity.

2.3. Initial Assessments on Mild Extremal Solutions

This section delineates mild upper and lower solutions for $\mathcal{CFNDE}$ (1). For detailed information on mild solution techniques, see [12,13]. The authors examine scalar differential equations with Riemann–Liouville derivatives, addressing both delayed and non-delayed cases. Known for initial point discontinuities, these derivatives pose challenges that mild solutions mitigate. It is established that a mild solution may lack an existing fractional derivative, paralleling discrete case observations [30], warranting further study of Riemann–Liouville difference equations. For insights on Caputo-type equations, see [31].

Definition 5.

Let ${\alpha }^0,{\beta }^0:{\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0\to \mathbb{R}$. The functions ${\alpha }^{0}and{\beta }^0$ are termed coupled mild lower–upper solutions ($\mathcal{CMLU}$) of Type I for Equation (1) if they satisfy

$$\begin{array}{cc}\hfill & {\alpha }^0\left(t\right)\le \varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)+w\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)\right),t\in {\mathbb{Z}}_0^T,\hfill \\ \hfill & {\beta }^0\left(t\right)\ge \varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)+w\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)\right),t\in {\mathbb{Z}}_0^T,\hfill \\ \hfill & {\alpha }^0\left(t\right)\le \varphi \left(t\right)\le {\beta }^0\left(t\right),t\in {\mathsf{\Omega }}_0.\hfill \end{array}$$

(3)

Remark 3.

For a variety of lower/upper solutions of (1), see Def. 5 in [11], and for a mild lower/upper solutions, refer to Def 4.7 in [13]. The current work focuses on a $\mathcal{CMLU}$ of Type I.

Definition 6.

A function $x:{\mathbb{Z}}_{-\tau }^T\to \mathbb{R}$ is called a mild minimal (or maximal) solution of the $\mathcal{IVP}$ for $\mathcal{CFNDE}$ (1) if it is a mild solution such that, for any other mild solution $x^{\ast }\left(t\right)$, we have $x\left(t\right)\le x^{\ast }\left(t\right)$ (or $x\left(t\right)\ge x^{\ast }\left(t\right)$) over ${\mathbb{Z}}_{-\tau }^T.$

3. Main Results

3.1. Theoretical Foundation of the Algorithm

The following theorem requires $\mathcal{CMLU}$ of Type I for $\mathcal{CFNDE}$ (1). We will establish that sequences converge uniformly and approach both the minimal and maximal coupled mild solutions of Equation (1). This study examines cases where $\mathcal{CMLU}$ originate from a common point, specifically at the initial time of the given problem, or alternatively explores case 2 as detailed in [31].

To establish our main results, we consider these assumptions:

$\mathrm{(𝒜}$1)

The functions ${\alpha }^0\left(t\right),{\beta }^0\left(t\right)$ are defined as $\mathcal{CMLU}$ of Type I for the Equation (1), satisfying ${\alpha }^0\left(t\right)\le {\beta }^0\left(t\right)$ over $Z_0^T\bigcup {\mathsf{\Omega }}_0.$

$\mathrm{(𝒜}$2)

The functions $u,w:{\mathbb{Z}}_0^T\times \left[{\alpha }^0\left(t\right),{\beta }^0\left(t\right)\right]\times \left[{\alpha }^0(t-\tau ),{\beta }^0(t-\tau )\right]\to \mathbb{R}$, associated with Equation (1), are continuous in their second and third variables on ${\mathbb{Z}}_0^T.$ Additionally, u is non-decreasing in these variables, while w is non-increasing.

Let us define the following sequences of functions.

$$\begin{array}{cc}\hfill & {\alpha }^{n+1}\left(t\right)=\varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\eta \left(j\right)\right)}^{\overline{\vartheta -1}}(u\left(j,{\alpha }^n\left(j\right),{\alpha }^n(j-\tau )\right)+w\left(j,{\beta }^n\left(j\right),{\beta }^n(j-\tau )\right)),t\in {\mathbb{Z}}_0^T\hfill \\ \hfill & {\beta }^{n+1}\left(t\right)=\varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\eta \left(j\right)\right)}^{\overline{\vartheta -1}}(u\left(j,{\beta }^n\left(j\right),{\beta }^n(j-\tau )\right)+w\left(j,{\alpha }^n\left(j\right),{\alpha }^n(j-\tau )\right)),t\in {\mathbb{Z}}_0^T\hfill \\ \hfill & {\alpha }^{n+1}\left(t\right)=\varphi \left(t\right)={\beta }^{n+1}\left(t\right),t\in {\mathsf{\Omega }}_0.\hfill \end{array}$$

(4)

Theorem 4.

Let the conditions $\left(\mathcal{A}1\right),\left(\mathcal{A}2\right)$ be satisfied. Then, if $x\left(t\right)$ is a solution of (1), such that ${\alpha }^0\left(t\right)\le x\left(t\right)\le {\beta }^0\left(t\right)$ for all $t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0$, the sequences defined by (4) are:

($𝒯$1) 

monotonically non-decreasing/non-increasing over the set ${\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0$, that is ${\alpha }^0\le {\alpha }^1\le \cdots \le {\alpha }^{n+1}\le x\le {\beta }^{n+1}\le {\beta }^n\le \cdots \le {\beta }^0,$ and the inequalities ${\alpha }^n\left(t\right)\le {\beta }^n\left(t\right),t\in {\mathbb{Z}}_0^T,n\in {\mathbb{Z}}_0^T$ are satisfied.

($𝒯$2) 

converge uniformly on ${\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0$, specifically as $n\to \infty$ we have ${\alpha }_n\to \alpha \mathrm{and}{\beta }_n\to \beta ,$ where α and β represent the minimal and maximal mild solutions of Equation (1) and $\alpha \le x\le \beta .$

Proof. 

Initially, we verify statement ($𝒯$1). Given that ${\alpha }^0\le x\le {\beta }^0$, we aim to show that ${\alpha }^0\le {\alpha }^1\le x\le {\beta }^1\le {\beta }^0.$ From assumption $\mathrm{(𝒜}$1), it is concordant with Definition 5 that both ${\alpha }^0$ and ${\beta }^0$ satisfies the inequalities outlined in (3). Consequently, from the representations (4), we obtain for $t\in {\mathbb{Z}}_0^T$:

$$\begin{array}{cc}\hfill & {\alpha }^1\left(t\right)=\varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\eta \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)+w\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)\right)\hfill \\ \hfill & {\beta }^1\left(t\right)=\varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\eta \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)+w\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)\right)\hfill \\ \hfill & {\alpha }^1\left(t\right)=\varphi \left(t\right)={\beta }^1\left(t\right),t\in {\mathsf{\Omega }}_0\hfill \end{array}$$

(5)

Let us define $m\left(t\right)={\alpha }^1\left(t\right)-{\alpha }^0\left(t\right),t\in {\mathbb{Z}}_0^T.$ Thus, by ${\alpha }^1\left(t\right)=\varphi \left(t\right)\ge {\alpha }^0\left(t\right),t\in {\mathsf{\Omega }}_0$ we have $m\left(t\right)\ge 0,t\in {\mathsf{\Omega }}_0.$

Additionally, based on assumption $\mathrm{(𝒜}$1), since ${\alpha }_0$ serves as a mild lower solution for Equation (1), by Definition 5, we derive the following results from representations (3) and (5)

$$\begin{array}{cc}& m\left(t\right)={\alpha }^1\left(t\right)-{\alpha }^0\left(t\right)\hfill \\ & ={\alpha }^1\left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)+w\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)\right)-{\alpha }^0\left(t\right)\hfill \\ & \ge {\alpha }^1\left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)+w\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)\right)\hfill \\ & -{\alpha }^0\left(0\right)-{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)+w\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)\right)\hfill \\ & ={\alpha }^1\left(0\right)-{\alpha }^0\left(0\right)\ge 0,\mathrm{from}{\alpha }^1\left(t\right)\ge {\alpha }^0\left(t\right),t\in {\mathsf{\Omega }}_0\hfill \end{array}$$

Consequently, this implies $m\left(t\right)\ge 0$ and ${\alpha }^0\left(t\right)\le {\alpha }^1\left(t\right)$ in ${\mathsf{\Omega }}_0\bigcup {\mathbb{Z}}_0^T$. Assume that the solution x of Equation (1) satisfies

$${\alpha }^0\left(t\right)\le x\left(t\right)\le {\beta }^0\left(t\right)in{\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0.$$

(6)

Setting $m\left(t\right)={x\left(t\right)-\alpha }^1\left(t\right),$ since ${\alpha }^0\left(t\right)\le \varphi \left(s\right),{\beta }^0\left(t\right)\ge \varphi \left(s\right)$ and $x\left(t\right)=\varphi \left(t\right),t\in {\mathsf{\Omega }}_0,$ we find that $m\left(t\right)=x\left(t\right)-{\alpha }^1\left(t\right)=\varphi \left(t\right)-\varphi \left(t\right)=0,t\in {\mathsf{\Omega }}_0.$

Given that x is a solution to Equation (1), using Lemma 2, Definition 3, assumption $\mathrm{(𝒜}$2), and representations (2), (5) and (6), we obtain the following

$$\begin{array}{cc}& m\left(t\right)=x\left(t\right)-{\alpha }^1\left(t\right)=\varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,x\left(j\right),x(j-\tau )\right)+w\left(j,x\left(j\right),x(j-\tau )\right)\right)\hfill \\ & -\varphi \left(0\right)-{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)+w\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)\right)\ge 0.\hfill \end{array}$$

It follows that $m\left(t\right)\ge 0,$ and ${\alpha }^1\left(t\right)\le x\left(t\right)$ in ${\mathsf{\Omega }}_0\bigcup {\mathbb{Z}}_0^T.$ Using similar arguments, we show that $x\left(t\right)\le {\beta }^1\left(t\right)$ and ${\beta }^1\left(t\right)\le {\beta }^0\left(t\right).$ Thus, it follows that ${\alpha }^0\left(t\right)\le {\alpha }^1\left(t\right)\le x\left(t\right)\le {\beta }^1\left(t\right)\le {\beta }^0\left(t\right)$ in ${\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0.$

Next, we prove the inequality ${\alpha }^k\left(t\right)\le {\alpha }^{k+1}\left(t\right),$ for $k\ge 1$ and $t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0.$

Suppose that

$${\alpha }^{k-1}\left(t\right)\le {\alpha }^k\left(t\right)\le x\left(t\right)\le {\beta }^k\left(t\right)\le {\beta }^{k-1}\left(t\right), t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0$$

(7)

for $k>1.$ Since ${\alpha }^k\left(t\right)={\beta }^k\left(t\right)=\varphi \left(t\right),t\in {\mathsf{\Omega }}_0$ it follows ${\alpha }^{k-1}\left(t\right)={\alpha }^k\left(t\right)=x\left(t\right)={\beta }^k\left(t\right)={\beta }^{k-1}\left(t\right)=\varphi \left(t\right), t\in {\mathsf{\Omega }}_0$ for $k>1.$

Consequently, letting $m\left(t\right)$ be defined as ${\alpha }_{k+1}\left(t\right)-{\alpha }_k\left(t\right)$, then ${\alpha }^k\left(t\right)=\varphi \left(t\right)={\alpha }^{k+1}\left(t\right),t\in {\mathsf{\Omega }}_0$ and using representation (4) from assumption $\mathrm{(𝒜}$2) along with (7) as the inductive hypothesis, we establish

$$\begin{array}{cc}& m\left(t\right)={\alpha }^{k+1}\left(t\right)-{\alpha }^k\left(t\right)\hfill \\ & ={\alpha }^k\left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^k\left(j\right),{\alpha }^k(j-\tau )\right)+w\left(j,{\beta }^k\left(j\right),{\beta }^k(j-\tau )\right)\right)\hfill \\ & -{\alpha }^{k-1}\left(0\right)-{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^{k-1}\left(j\right),{\alpha }^{k-1}(j-\tau )\right)+w\left(j,{\beta }^{k-1}\left(j\right),{\beta }^{k-1}(j-\tau )\right)\right)\hfill \\ & \ge \varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^{k-1}\left(j\right),{\alpha }^{k-1}(j-\tau )\right)+w\left(j,{\beta }^{k-1}\left(j\right),{\beta }^{k-1}(j-\tau )\right)\right)\hfill \\ & -\varphi \left(0\right)-{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^{k-1}\left(j\right),{\alpha }^{k-1}(j-\tau )\right)+w\left(j,{\beta }^{k-1}\left(j\right),{\beta }^{k-1}(j-\tau )\right)\right)=0.\hfill \end{array}$$

It follows that $m\left(t\right)\ge 0,t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0.$ Therefore, we conclude that ${\alpha }^k\left(t\right)\le {\alpha }^{k+1}\left(t\right),$ $t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0.$

Analogously, considering $m\left(t\right)=x\left(t\right)-{\alpha }^{k+1}\left(t\right)$ and by representations (2) and (4), along with assumption $\mathrm{(𝒜}$2) and inequalities (7), we have $x\left(t\right)=\varphi \left(t\right)={\alpha }^{k+1}\left(t\right),$ $t\in {\mathsf{\Omega }}_0$ and

$$\begin{array}{cc}& m\left(t\right)=x\left(t\right)-{\alpha }^{k+1}\left(t\right)\hfill \\ & =\varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,x\left(j\right),x(j-\tau )\right)+w\left(j,x\left(j\right),x(j-\tau )\right)\right)\hfill \\ & -{\alpha }^k\left(0\right)-{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^k\left(j\right),{\alpha }^k(j-\tau )\right)+w\left(j,{\beta }^k\left(j\right),{\beta }^k(j-\tau )\right)\right)\ge 0.\hfill \end{array}$$

Similarly, the inequalities ${\beta }^{k+1}\left(t\right)\le {\beta }^k\left(t\right)$ and $x\left(t\right)\le {\beta }^{k+1}\left(t\right)$ can be shown for $t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0$. Thus, for any positive integer n and $t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0,$

$${\alpha }^0\le {\alpha }^1\le \cdots \le {\alpha }^n\le x\le {\beta }^n\le \cdots \le {\beta }^1\le {\beta }^0.$$

From assumption $\mathrm{(𝒜}$1), it is evident that $\varphi \left(t\right)={\alpha }^0\left(t\right)\le {\beta }^0\left(t\right)=\varphi \left(t\right),t\in {\mathbb{Z}}_0^T\bigcup {\mathsf{\Omega }}_0.$ By using monotonic properties of the functions u and w per condition $\mathrm{(𝒜}$2), it transpires that

$$\begin{array}{cc}\hfill {\alpha }^1\left(t\right)& =\varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)+w\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)\right)\hfill \\ & \le \varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^t{\left(t-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\left(u\left(j,{\beta }^0\left(j\right),{\beta }^0(j-\tau )\right)+w\left(j,{\alpha }^0\left(j\right),{\alpha }^0(j-\tau )\right)\right)\hfill \\ & ={\beta }^1\left(t\right),t\in Z_0^T.\hfill \end{array}$$

By induction, this inequality holds for any $n\ge 1$ and the proof of ($𝒯$1) concludes.

We shall now address the proof of ($𝒯$2). One approach to establishing uniform convergence, as discussed in [16,25,26], involves demonstrating both uniform boundedness and equicontinuity. In this context, our methodology utilizes well-established principles from real analysis, as detailed in Section 2. According to ($𝒯$1), the sequences, $\left\{{\alpha }^n\left(t\right)\right\}$ and $\left\{{\beta }^n\left(t\right)\right\}$ are monotonic and bounded on ${\mathbb{Z}}_0^T$. Let us denote their pointwise limits by $\alpha$ for $\left\{{\alpha }^n\right\}$ and $\beta$ for $\left\{{\beta }^n\right\}$. Thus, it becomes necessary to verify that these sequences satisfy the uniformly Cauchy criterion according to Definition 4.

Let $0\le t_1\le t_2\le T.$ For an arbitrary $n,$ we have:

$$\begin{array}{cc}& |{\alpha }^n\left(t_2\right)-{\alpha }^n\left(t_1\right)|={\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\left|\sum _{j=1}^{t_2}{\left(t_2-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\right(u(j,{\alpha }^{n-1}\left(j\right),{\alpha }^{n-1}(j-\tau ))+w(j,{\beta }^{n-1}\left(j\right),{\beta }^{n-1}(j-\tau )))\hfill \\ & -{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^{t_1}{\left(t_1-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}(u(j,{\alpha }^{n-1}\left(j\right),{\alpha }^{n-1}(j-\tau ))+w(j,{\beta }^{n-1}\left(j\right),{\beta }^{n-1}(j-\tau )))|\hfill \\ & \le {\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^{t_1}|{\left(t_2-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}-{\left(t_1-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}||u(j,{\alpha }^{n-1}\left(j\right),{\alpha }^{n-1}(j-\tau ))+w(j,{\beta }^{n-1}\left(j\right),{\beta }^{n-1}(j-\tau ))|\hfill \\ & +{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=t_1+1}^{t_2}\left|{\left(t_2-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\right||u(j,{\alpha }^{n-1}\left(j\right),{\alpha }^{n-1}(j-\tau ))+w(j,{\beta }^{n-1}\left(j\right),{\beta }^{n-1}(j-\tau ))|\hfill \end{array}$$

Invoking condition $\mathrm{(𝒜}$2) and the continuity of the real-valued functions u and w on $[{\alpha }_0,{\beta }_0]$, with respect to their second and third arguments, along with the uniform boundedness of sequences on ${\mathbb{Z}}_0^T$, it follows that both functions u and w attain maximum values over ${\mathbb{Z}}_0^T$. Let us denote these maxima as $M_u=maxu$ and $M_w=maxw$. Then,

$$\begin{array}{cc}& |{\alpha }^n\left(t_2\right)-{\alpha }^n\left(t_1\right)|\le (M_u+M_w)({\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^{t_1}{\left(t_2-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}-{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=1}^{t_1}{\left(t_1-\rho \left(j\right)\right)}^{\overline{\vartheta -1}}\hfill \\ & +{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}\sum _{j=t_1+1}^{t_2}{\left(t_2-\rho \left(j\right)\right)}^{\overline{\vartheta -1}})\le (M_u+M_w)({\nabla }_0^{-\vartheta }{(t_2-0)}^{\overline{0}}-{\nabla }_0^{-\vartheta }{(t_1-0)}^{\overline{0}}+{\nabla }_{t_1}^{-\vartheta }{(t_2-t_1)}^{\overline{0}})\hfill \\ & ={\displaystyle \frac{(M_u+M_w)}{\Gamma (\vartheta +1)}}((t_2^{\overline{0}}-t_1^{\overline{0}})+{(t_2-t_1)}^{\overline{0}})\le {\displaystyle \frac{(M_u+M_w)}{\Gamma (\vartheta +1)}}{(t_2-t_1)}^{\overline{0}}\le {\displaystyle \frac{(M_u+M_w)}{\Gamma (\vartheta +1)}}K\left(\vartheta \right).\hfill \end{array}$$

Utilizing ${(t-0)}^{\overline{0}}=1$ alongside the sum ${\displaystyle \sum _{j=1}^t}{(t-\rho \left(j\right))}^{\overline{\vartheta -1}}{(t-0)}^{\overline{0}}=\Gamma \left(\vartheta \right){\nabla }_0^{-\vartheta }{(t-0)}^{\overline{0}}={\displaystyle \frac{\Gamma (t+\vartheta )}{\vartheta \Gamma \left(t\right)}}$, where $K\left(\vartheta \right)={\displaystyle \frac{\Gamma (T^{\ast }+\vartheta )}{\vartheta \Gamma \left(T^{\ast }\right)}},T^{\ast }\le T$ is a constant depending on $\vartheta$, this confirms that the sequence ${\{\alpha }^{\left(n\right)}\left(t\right)\}$ is uniformly Cauchy on ${\mathbb{Z}}_0^T$. Applying similar reasoning establishes ${\{\beta }^{\left(n\right)}\left(t\right)\}$ as uniformly Cauchy on ${\mathbb{Z}}_0^T$ as well. Consequently, both ${\{\alpha }^n\}$ and ${\{\beta }^n\}$ converge uniformly to $\alpha$ and $\beta$ on ${\mathbb{Z}}_0^T$.

To confirm that $\alpha$ and $\beta$ are a couple of mild minimal and maximal solutions of (1), note that since ${\alpha }^n$ and ${\beta }^n$ are constructed by (4), it follows that as $n\to \infty$, by the Lebesgue Dominated Convergence Theorem, we obtain the limits

$$\alpha \left(t\right)=\left\{\begin{array}{cc}\varphi \left(t\right),& t\in {\mathsf{\Omega }}_0,\\ \varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}{\displaystyle \sum _{j=t_0+1}^t}{\left(t-\eta \left(j\right)\right)}^{\overline{\vartheta -1}}(u\left(j,\alpha \left(j\right),\alpha (j-\tau )\right)+w\left(j,\beta \left(j\right),\beta (j-\tau )\right)),& t\in {\mathbb{Z}}_0^T\end{array}\right.$$

and

$$\beta \left(t\right)=\left\{\begin{array}{cc}\varphi \left(t\right),& t\in {\mathsf{\Omega }}_0,\\ \varphi \left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\vartheta \right)}}{\displaystyle \sum _{j=t_0+1}^t}{\left(t-\eta \left(j\right)\right)}^{\overline{\vartheta -1}}(u\left(j,\beta \left(j\right),\beta (j-\tau )\right)+w\left(j,\alpha \left(j\right),\alpha (j-\tau )\right)),& t\in {\mathbb{Z}}_0^T.\end{array}\right.$$

Therefore, they constitute a couple of mild solutions of (1) per Definition 5.

Furthermore, if x is the solution of (1), then $x\left(t\right)\in \left[{\alpha }^n\left(t\right),{\beta }^n\left(t\right)\right]on{\mathbb{Z}}_0^T$ within each iteration, $n,$ by induction via statement ($𝒯$1) yields $x\left(t\right)\in \left[\alpha \right(t),\beta (t\left)\right]$ on ${\mathbb{Z}}_0^T$. Therefore, $\alpha$ and $\beta$ satisfy Definition 6 and form a couple of mild minimal and maximal solution of (1), and the proof is complete. □

Remark 4.

We can establish solution existence conditions by replacing hypothesis $\left(\mathcal{A}2\right)$ in Theorem 4 with one-sided Lipschitz criteria. This method is a standard approach in proving analogous results.

3.2. Algorithm for Approximation

Step 1. Select initial functions ${\alpha }^{\left(0\right)}\left(t\right)$ and ${\beta }^{\left(0\right)}\left(t\right)$ that satisfy condition a of Theorem 4.

Step 2. Verify the monotonicity and boundedness of functions u and w, as per condition $\mathrm{(𝒜}$2) in Theorem 4.

Step 3. Construct $\mathcal{CMLU}$ ${\alpha }^{\left(n\right)}\left(t\right)$ and ${\beta }^{\left(n\right)}\left(t\right)$ by (4).

Step 4. Compute the mild solution $x\left(t\right),t\in {\mathbb{Z}}_0^T$ of (1) using a Formula (2).

Step 5. Determine the absolute error ($\mathcal{AE}$) between the approximate values from Steps 3–4 to achieve desired accuracy.

Remark 5.

In our Applications section, Wolfram Mathematica (version 13.3) was used for efficient algorithm implementation. Additionally, MATLAB^® (version R2020b) was employed for visual data representation, enhancing clarity through continuous line graphs.

4. Applications

4.1. Numerical Techniques: Advantages and Methodological Insights

The primary objective of this section is to examine the operational dynamics of the employed methodology while deriving analytical insights into its efficacy. Specifically, it seeks to establish and validate a $\mathcal{CMLU}$ framework within delayed BAM neural networks, utilizing Caputo fractional-order nabla operators. In-depth analysis by [23] addresses critical aspects such as existence, uniqueness, and uniform stability of nontrivial solutions in this context. Notably, refs. [32,33] offer significant applicability for analogous mathematical frameworks. Works [34,35] explore fractional order influences within Caputo-type fractional–differential SIR models, including analyses into a fractional-order delayed model for tuberculosis where constant delay denotes recovery time.

The methodology employed in this study, utilizing a coupled mild upper and lower solution technique, presents distinct advantages. It facilitates the application to complex problems while offering enhanced analytical perspectives. In contexts involving difference equations on discrete sets such as integers, traditional methods like Adams and Predictor–Corrector are not typically utilized. Similarly, Euler and Runge–Kutta methods, designed for continuous differential equations, may not be directly applicable. Instead, finite difference methods or specifically tailored discrete algorithms are effectively employed. Within this framework lies the method of upper and lower solutions, by iteratively employing mild lower solutions to approximate exact solutions, this approach ensures improved accuracy while maintaining computational efficiency. Consequently, current research is directed towards developing and refining these techniques to augment applicability and efficiency with a particular emphasis on fractional orders of the nabla operator. However, this study does not prioritize efficiency comparisons among different classes of numerical methods or technical aspects such as relative error and parameter dependency. These comparisons can be misleading due to reliance on limited test problems or specific method implementations. Thus, assessing relative efficiencies across various classes remains technically challenging and falls outside our primary objectives. A predictor–corrector approach specifically tailored for fractional systems enhances accuracy through the Adams–Bashforth–Moulton scheme [36]. Adaptations of Runge–Kutta methods effectively address fractional derivatives [37], while insights into stability considerations are crucial when applying algorithms like Euler or Runge–Kutta in fractional contexts [38]. Additionally, linear multistep methods applicable to Abel integral equations, diffusion problems, and special function computations have been extensively examined [39].

4.2. BAM Neural Networks with Constant Delay

We examine a discrete model of fractional-order BAM neural networks with two neuron layers [23]:

$$\begin{array}{cc}\hfill & \left({\nabla }_{0\ast }^{\lambda }{x}_i\right)\left(t\right)=-c_i{x}_i(t-\tau )+\sum _{j=1}^m{a}_{ij}{f}_j\left(y_j\left(t\right)\right)+I_i,i=1,2,\dots ,n,\hfill \\ \hfill & \left({\nabla }_{0\ast }^{\mu }{y}_j\right)\left(t\right)=-d_j{y}_j(t-\tau )+\sum _{i=1}^n{b}_{ji}{g}_i\left(x_i\left(t\right)\right)+J_j,j=1,2,\dots ,m,\hfill \end{array}$$

(8)

where $t\in Z_0^T,0<\lambda ,\mu <1,{\nabla }^{\lambda }$ and ${\nabla }^{\mu }$ are the Caputo fractional difference operators of order $\lambda$ and $\mu$, respectively.

The parameters are explained as follows: n and m denote neuron counts; $x_i\left(t\right)$ and $y_j\left(t\right)$ represent membrane potentials at time t; functions $f_j$ and $g_i$ are non-decreasing activations; $I_i$ and $J_j$ denote the ith and the jth component of an external input sources; constants like $c_i$, $d_j$, etc., define synaptic strengths or delays.

The $\mathcal{IVP}$ for this system employs Caputo-type fractional nabla difference system ($\mathcal{CFNDS}$) (8) is

$$\begin{array}{cc}\hfill & x_i\left(s\right)={\phi }_i\left(s\right),y_j\left(s\right)={\psi }_j\left(s\right),s\in Z_{-\tau }^0,\hfill \\ \hfill & i=1,2,\dots ,n,j=1,2,\dots ,m.\hfill \end{array}$$

(9)

We derive mild solutions of $\mathcal{IVP}$ (8) and (9), using (2), essential for our analysis.

$$\begin{array}{cc}\hfill & x_i\left(t\right)={\varphi }_i\left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\lambda \right)}}\sum _{k=1}^t{\left(t-\rho \left(k\right)\right)}^{\overline{\lambda -1}}\left(-c_i{x}_i(k-\tau )+\sum _{j=1}^m{a}_{ij}{f}_j\left(y_j\left(k\right)\right)+I_i\right),\hfill \\ \hfill & y_j\left(t\right)={\psi }_j\left(0\right)+{\displaystyle \frac{1}{\Gamma \left(\mu \right)}}\sum _{k=1}^t{\left(t-\rho \left(k\right)\right)}^{\overline{\mu -1}}\left(-d_j{y}_j(k-\tau )+\sum _{i=1}^n{b}_{ji}{g}_i\left(x_i\left(k\right)\right)+J_j\right),\hfill \end{array}$$

(10)

Example 1.

Consider a two-state Caputo fractional-order BAM neural network with constant integer delay

$$\begin{array}{cc}\hfill & \left({\nabla }_{0\ast }^{\vartheta }{x}_1\right)\left(t\right)=-{\lambda }_1{x}_1(t-\tau )+{\eta }_1{f}_1\left(y_1\left(t\right)\right)-{\xi }_1{f}_2\left(y_2\left(t\right)\right)+{\zeta }_1\hfill \\ \hfill & \left({\nabla }_{0\ast }^{\vartheta }{x}_2\right)\left(t\right)=-{\lambda }_2{x}_2(t-\tau )-{\eta }_2{f}_1\left(y_1\left(t\right)\right)+{\xi }_2{f}_2\left(y_2\left(t\right)\right)-{\zeta }_2\hfill \\ \hfill & \left({\nabla }_{0\ast }^{\mu }{y}_1\right)\left(t\right)=-{\lambda }_3{y}_1(t-\tau )+{\eta }_3{g}_1\left(x_1\left(t\right)\right)+{\xi }_3{g}_2\left(x_2\left(t\right)\right)+{\zeta }_3\hfill \\ \hfill & \left({\nabla }_{0\ast }^{\mu }{y}_2\right)\left(t\right)=-{\lambda }_4{y}_2(t-\tau )+{\eta }_4{g}_1\left(x_1\left(t\right)\right)+{\xi }_4{g}_2\left(x_2\left(t\right)\right)+{\zeta }_4\hfill \\ \hfill & x_j\left(t\right)={\varphi }_j\left(t\right),y_j\left(t\right)={\psi }_j\left(t\right),t\in {\mathsf{\Omega }}_0,j=1,2.\hfill \end{array}$$

(11)

Activation functions are hyperbolic tangents with specific coefficients for each state variable pair $(x_1,x_2)$ or $(y_1,y_2)$ under given conditions ensuring $\mathcal{CMLU}$ Type I compliance per Theorem 4 requirements—allowing further numerical simulation exploration, presented below.

• the activation functions $f_j\left(y_j\right)=0.2tanh\left(y_j\right),$ $g_i\left(x_i\right)=0.2tanh\left(x_j\right),$ $j=1,2$;
• the fractional orders $\vartheta =0.1, 0.9\mu =0.1, 0.9;$ the delay $\tau =1,{\mathsf{\Omega }}_0=\{-1,0\};$
• the initial functions ${\varphi }_1\equiv 0.5,{\varphi }_2\equiv 1.5,{\psi }_1\equiv 5.5,{\psi }_2\equiv 9;$
• the parameters ${\lambda }_1=0.2,{\lambda }_2=0.03,{\lambda }_3=0.05,{\lambda }_4=0.04,$ ${\eta }_1=0.2,{\eta }_2=0.02,{\eta }_3=0.2,{\eta }_4=0.1,$ ${\xi }_1=0.01,{\xi }_2=0.3,{\xi }_3=0.1,{\xi }_4=0.2,$ ${\zeta }_1=0.1,{\zeta }_2=0.1,{\zeta }_3=0.3,{\zeta }_4=0.4;$
• the initial $\mathcal{CMLU}$ ${\alpha }_{x_1}^0\equiv 0$ and ${\beta }_{x_1}^0\left(t\right)\equiv 1,$ ${\alpha }_{x_2}^0\left(t\right)\equiv 1$ and ${\beta }_{x_2}^0\left(t\right)\equiv 2,$ ${\alpha }_{y_1}^0\equiv 5$ and ${\beta }_{y_1}^0\left(t\right)\equiv 6,$ ${\alpha }_{y_2}^0\left(t\right)\equiv 8$ and ${\beta }_{y_2}^0\left(t\right)\equiv 10,t\in {\mathbb{Z}}_0^7.$

Numerical simulation results are detailed below; see Appendix A for tables on absolute errors and exact approximations, and Appendix B for graphical representations.

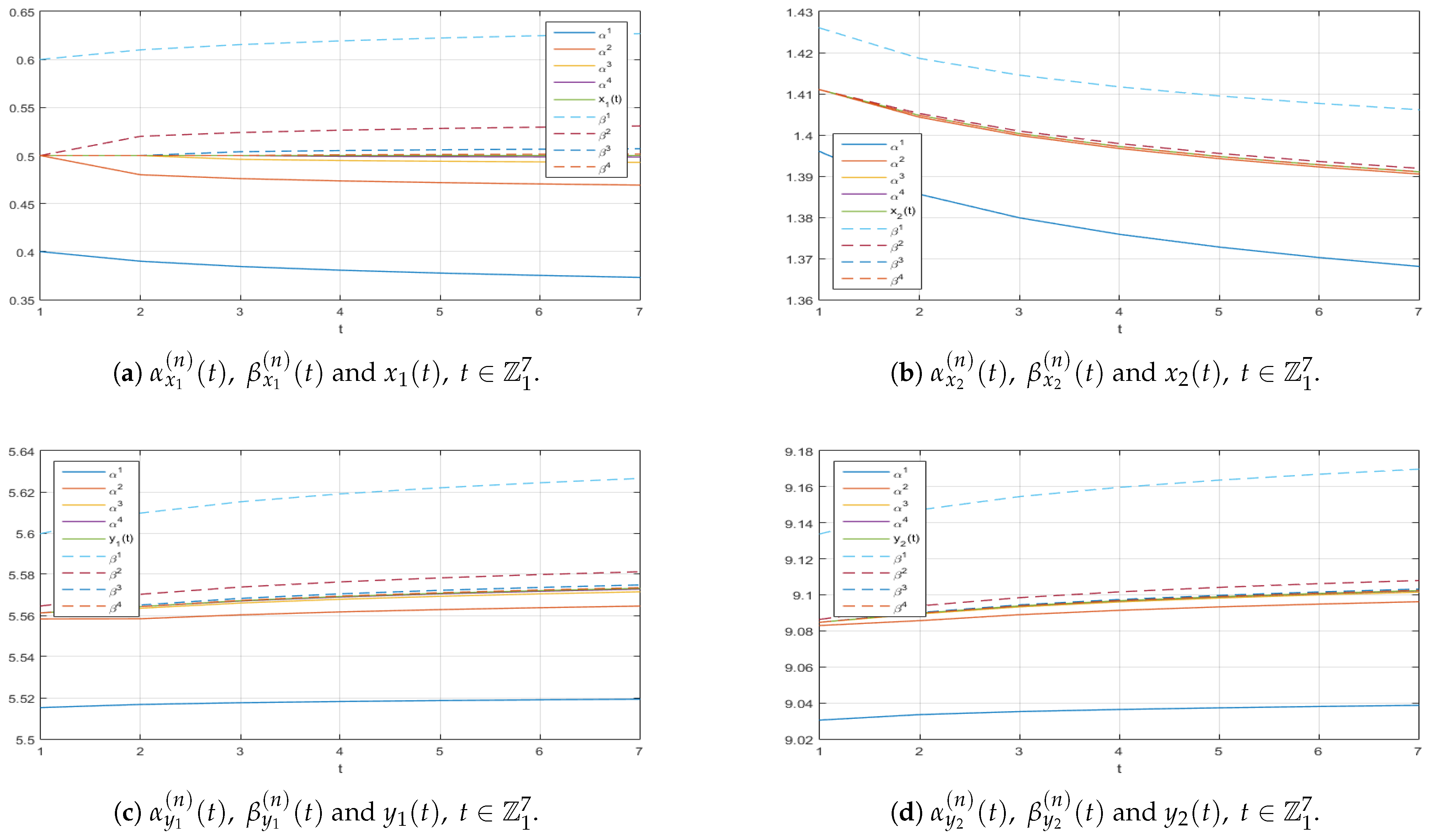
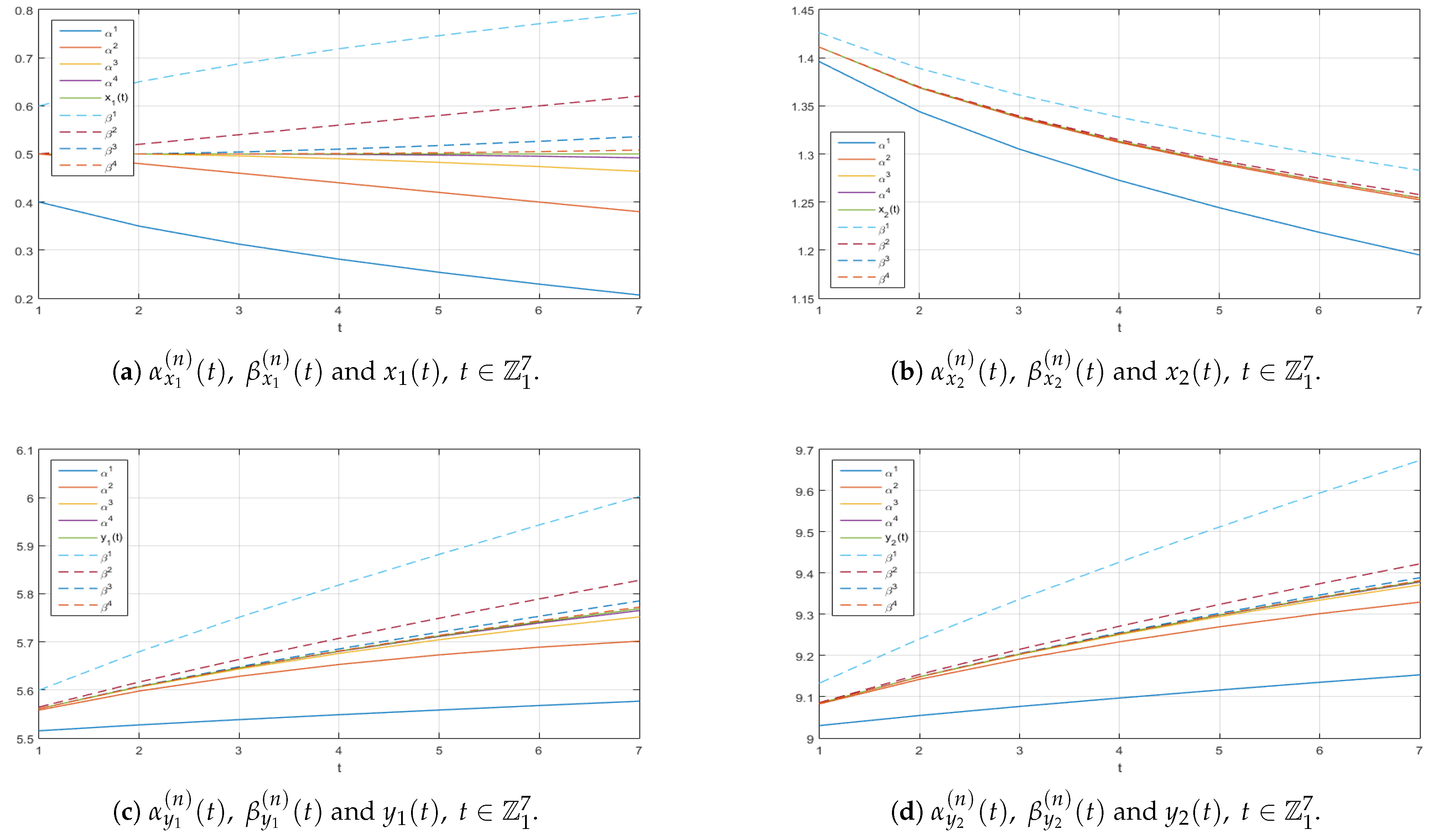
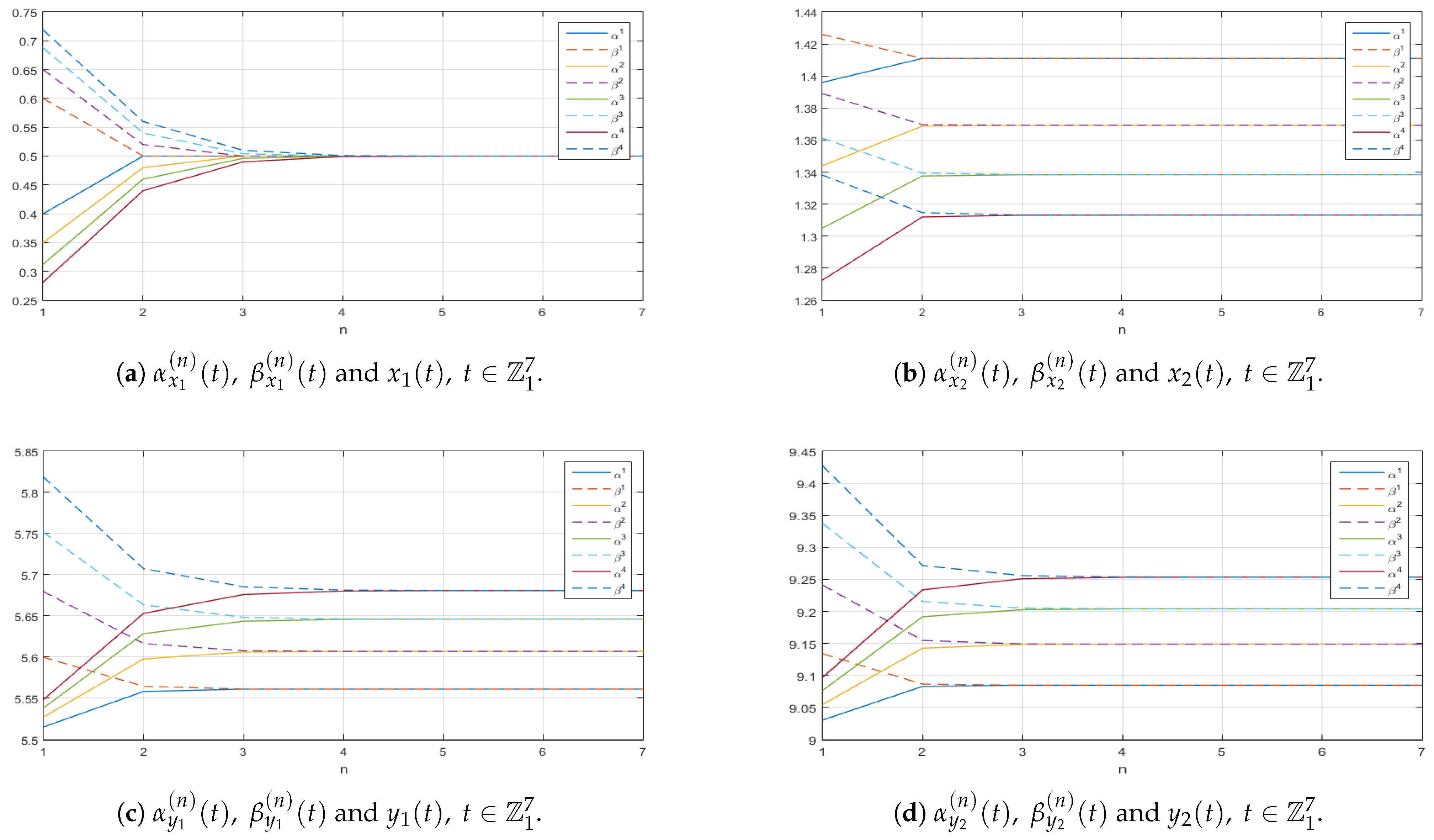
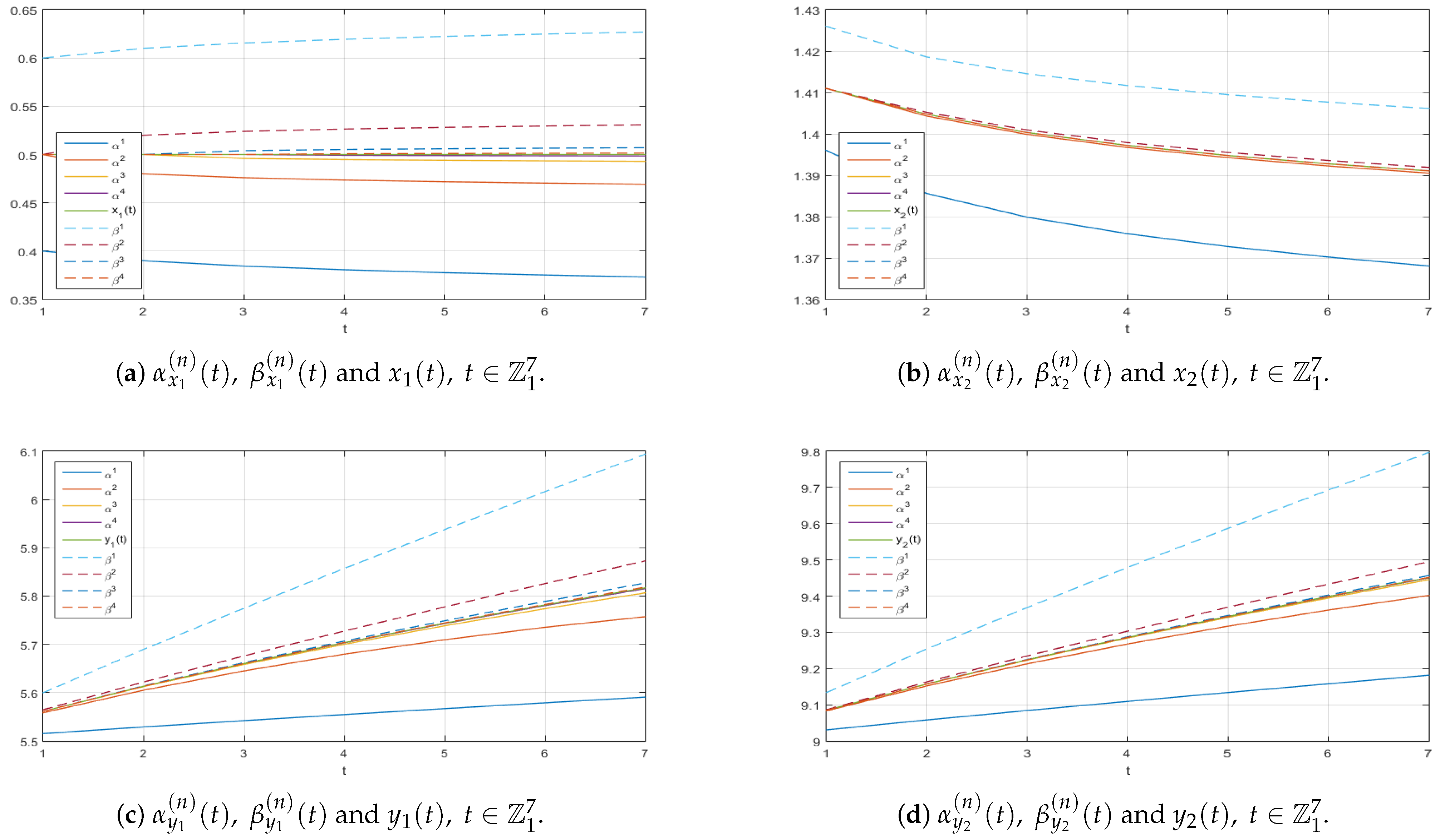
4.3. Analysis of Proposed Graphs

The comprehensive analysis reveals several key observations, detailed below.

-

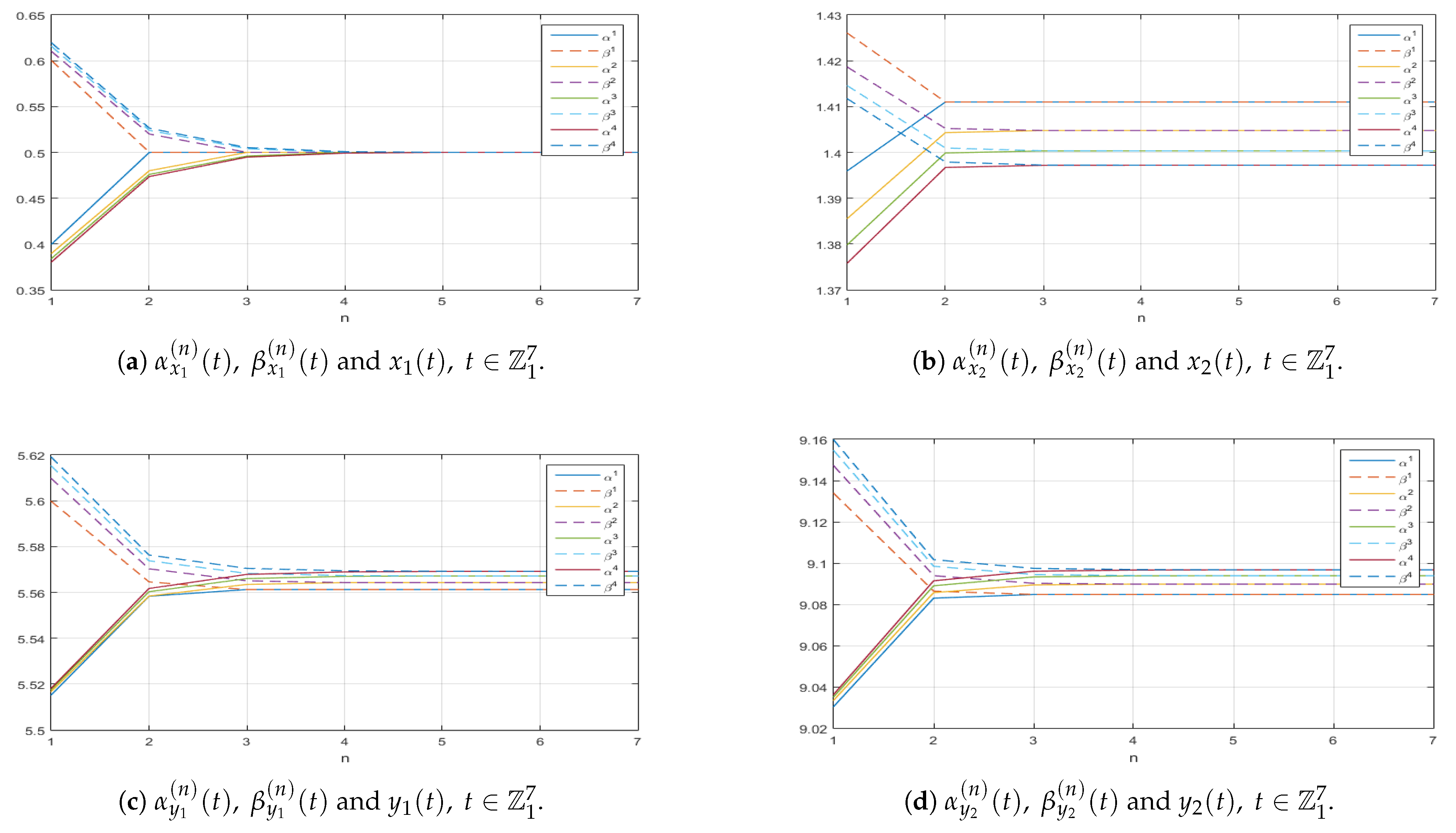
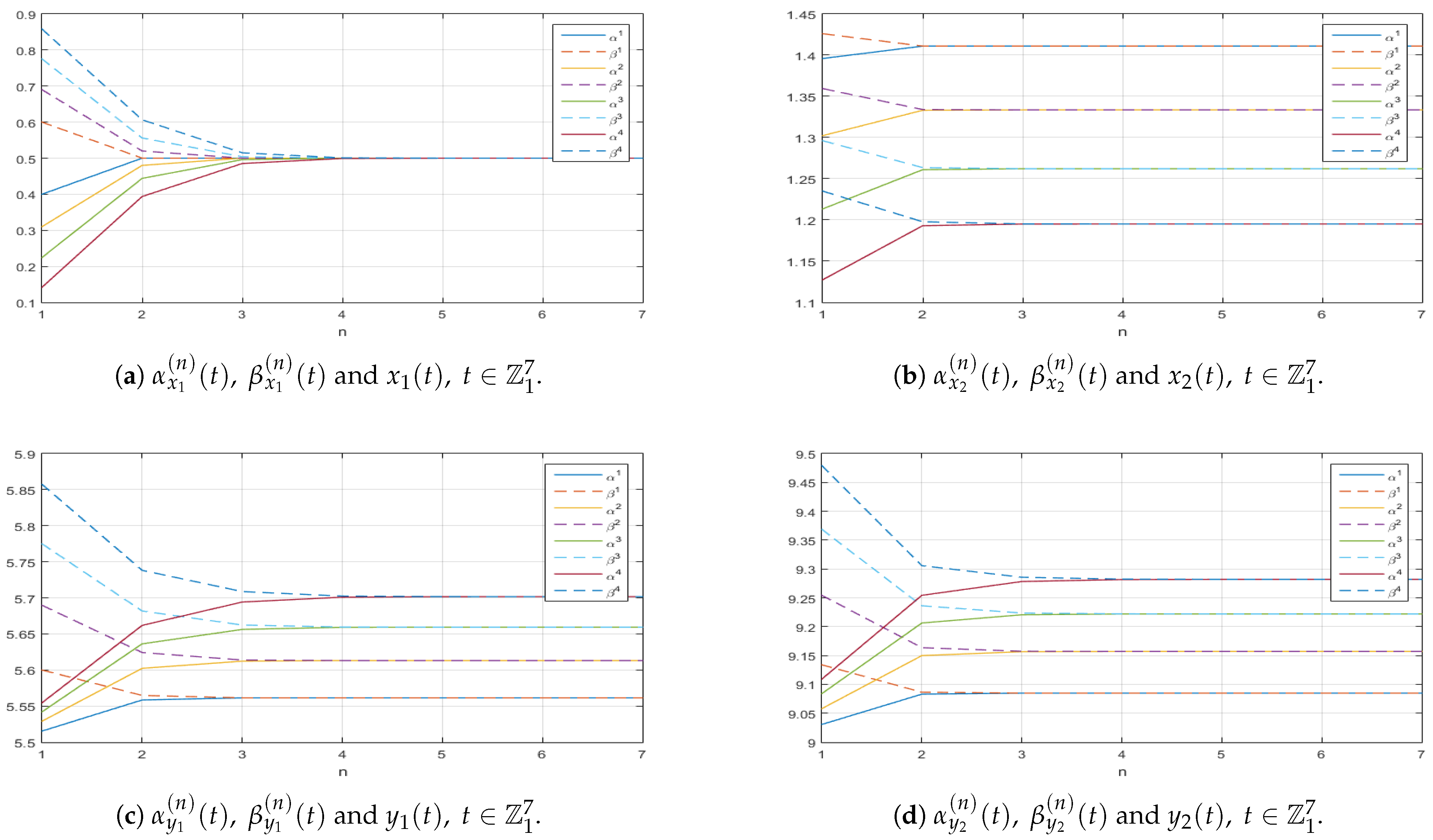
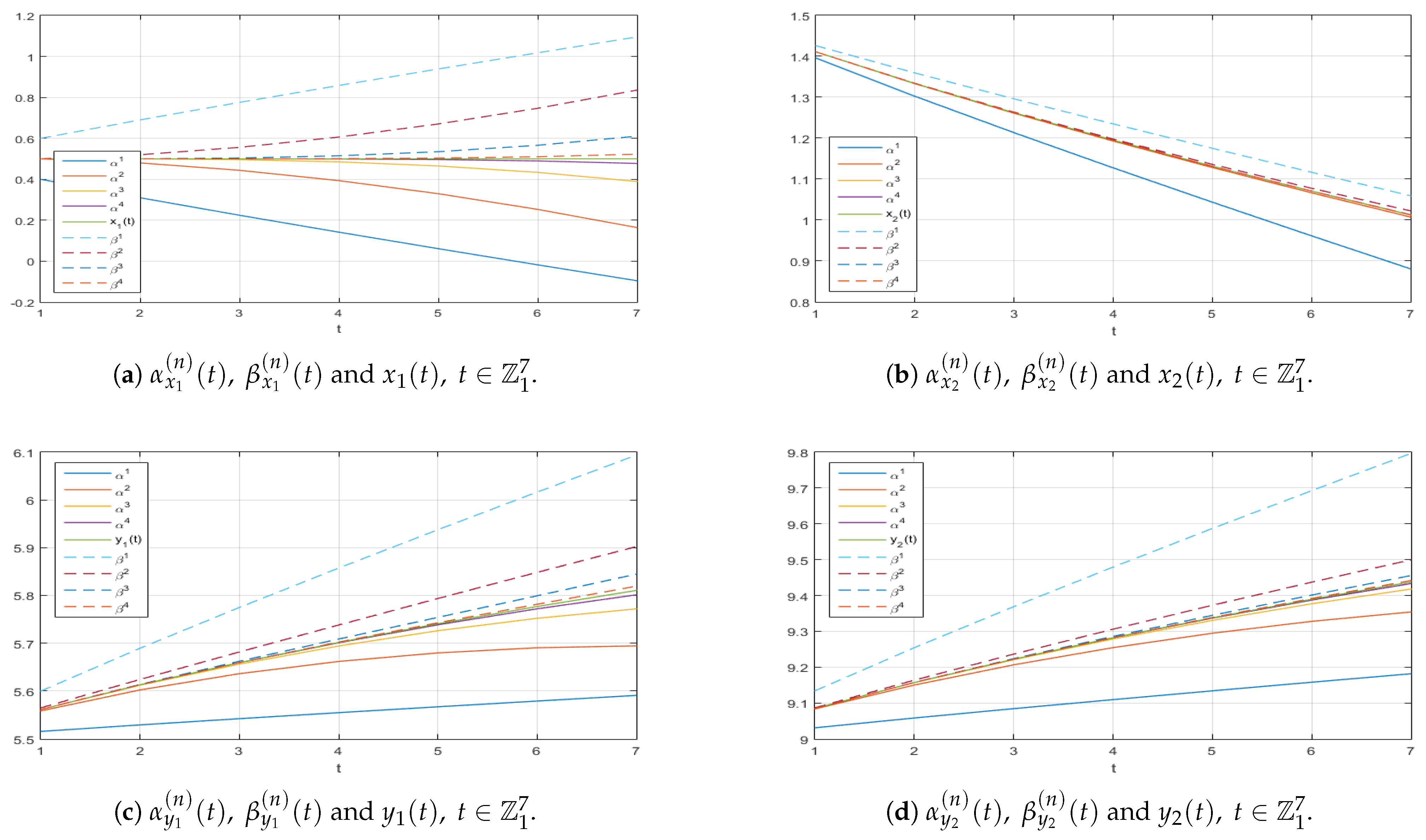
The sequences $\left\{{\alpha }^{\left(n\right)}\left(t\right)\right\}$ and $\left\{{\beta }^{\left(n\right)}\left(t\right)\right\}$, representing mild lower and upper solutions, respectively, demonstrate distinct monotonic behaviors. Specifically, the sequence $\left\{{\alpha }^{\left(n\right)}\left(t\right)\right\}$ is non-decreasing while the sequence $\left\{{\beta }^{\left(n\right)}\left(t\right)\right\}$ is non-increasing. This behavior is clearly illustrated in Figure 1, Figure 2 and Figure 3. Further supporting evidence is presented in Figure A1, Figure A2, Figure A3, Figure A4, Figure A5, Figure A6 and Figure A7 within the Appendix B. Additionally,

Table 1. Values of the mild lower solutions ${\alpha }_{x_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_1\left(t\right),$ $t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\alpha }}_{{\mathit{x}}_1}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^6\left(\mathit{t}\right)$	$\mathit{x}\left(\mathit{t}\right)$	$\mathcal{AE}$
1	0.437996368	0.537998704	0.537998814	0.537998821	0.537998821	0.537998821	0.537998821	1.11022 $\times {10}^{-15}$
2	0.431796005	0.514198677	0.534198915	0.534198942	0.534198946	0.534198946	0.534198946	8.88178 $\times {10}^{-16}$
3	0.428385805	0.510768628	0.532288749	0.536288876	0.536288886	0.536288887	0.536288887	6.66134 $\times {10}^{-16}$
4	0.425998665	0.508919591	0.532095687	0.536191851	0.536991867	0.53699187	0.53699187	2.83572 $\times {10}^{-10}$
5	0.424148632	0.507624588	0.532077068	0.536511655	0.53753247	0.537692476	0.537692477	7.09731 $\times {10}^{-10}$
6	0.422631605	0.506611261	0.53210097	0.536824455	0.538019669	0.538215518	0.538247519	3.20012 $\times {10}^{-5}$
7	0.421342131	0.505769363	0.532135907	0.53710546	0.538442674	0.538672029	0.538725663	5.36335 $\times {10}^{-5}$

and

Table 2. Values of the mild lower solutions ${\alpha }_{x_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_1\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\alpha }}_{{\mathit{x}}_1}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_1}^6\left(\mathit{t}\right)$	${\mathit{x}}_1\left(\mathit{t}\right)$	$\mathcal{AE}$
1	0.437996368	0.537998704	0.537998814	0.537998821	0.537998821	0.537998821	0.537998821	1.11022 $\times {10}^{-15}$
2	0.3821931	0.544597671	0.56459807	0.564598112	0.564598115	0.564598115	0.564598115	1.11022 $\times {10}^{-15}$
3	0.329179994	0.527406804	0.580927442	0.584927647	0.584927665	0.584927666	0.584927666	4.10783 $\times {10}^{-15}$
4	0.277933993	0.48926107	0.586485747	0.600182317	0.600982377	0.600982382	0.600982382	2.37896 $\times {10}^{-10}$
5	0.227969141	0.431783077	0.580459874	0.610162675	0.613743615	0.613903635	0.613903637	1.66748 $\times {10}^{-9}$
6	0.179003586	0.356072913	0.561949768	0.613814406	0.623573066	0.624408968	0.624440974	3.20067 $\times {10}^{-5}$
7	0.130854124	0.262947644	0.530033248	0.609441152	0.630337161	0.632914689	0.633121941	0.000207252

, as well as all tables included in Appendix A, provide data that reinforce these findings.

-

Figure 3 and Figure A1 show that iterative processes converge towards the exact solution regardless of fractional order variations, with further validation in Figure A2, Figure A4 and Figure A6.

-

Figure 1 and Figure 2 illustrate the convergence of lower $\alpha$ iterations towards their corresponding upper $\beta$ counterparts, as further detailed in Figure A3, Figure A5, and Figure A7.

-

Smaller fractional orders (refer to specific Figure 1 and Figure A1, and Table 1 and

Table A9. Values of the mild upper solutions ${\beta }_{x_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_1\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\beta }}_{{\mathit{x}}_1}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^6\left(\mathit{t}\right)$	${\mathit{x}}_1\left(\mathit{t}\right)$	$\mathcal{AE}$
1	0.637999509	0.537998906	0.537998828	0.537998821	0.537998821	0.537998821	0.537998821	1.11022 $\times {10}^{-15}$
2	0.65179946	0.554199544	0.534198984	0.534198949	0.534198946	0.534198946	0.534198946	1.55431 $\times {10}^{-15}$
3	0.659389433	0.558769643	0.540288961	0.536288896	0.536288888	0.536288887	0.536288887	2.9976 $\times {10}^{-15}$
4	0.664702414	0.561720699	0.542495948	0.537791901	0.536991873	0.53699187	0.53699187	2.72584 $\times {10}^{-10}$
5	0.668819974	0.563945763	0.544037363	0.53875172	0.537852482	0.537692477	0.537692477	6.06242 $\times {10}^{-10}$
6	0.672196374	0.565748491	0.545257291	0.53951253	0.538499685	0.538279521	0.538247519	3.20016 $\times {10}^{-5}$
7	0.675066313	0.56727208	0.546278949	0.540151944	0.539042693	0.538774433	0.538725663	4.87704 $\times {10}^{-5}$

,

Table A10. Values of the mild lower solutions ${\alpha }_{x_2}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_2\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\alpha }}_{{\mathit{x}}_2}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^6\left(\mathit{t}\right)$	${\mathit{x}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	1.396000036	1.411000108	1.411000116	1.411000116	1.411000116	1.411000116	1.410999999	1.1783 $\times {10}^{-7}$
2	1.385600039	1.404320105	1.404770122	1.404770124	1.404770124	1.404770124	1.404769998	1.25378 $\times {10}^{-7}$
3	1.379880041	1.399869108	1.400315527	1.400329029	1.400329029	1.400329029	1.400328898	1.30508 $\times {10}^{-7}$
4	1.375876043	1.39670901	1.39718135	1.3972009	1.397201305	1.397201305	1.397201171	1.34075 $\times {10}^{-7}$
5	1.372772944	1.394248834	1.394742523	1.394765505	1.394765988	1.394766	1.394765863	1.36826 $\times {10}^{-7}$
6	1.370228402	1.392227582	1.392738959	1.392764519	1.392765073	1.392765093	1.392764955	1.38704 $\times {10}^{-7}$
7	1.368065543	1.390507955	1.3910344	1.391062072	1.391062686	1.391062712	1.391062571	1.40447 $\times {10}^{-7}$

,

Table A11. Values of the mild upper solutions ${\beta }_{x_2}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_2\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\beta }}_{{\mathit{x}}_2}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^6\left(\mathit{t}\right)$	${\mathit{x}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	1.426000363	1.411000128	1.411000117	1.411000116	1.411000116	1.411000116	1.410999999	1.1783 $\times {10}^{-7}$
2	1.418600399	1.40522014	1.404770125	1.404770124	1.404770124	1.404770124	1.404769998	1.25378 $\times {10}^{-7}$
3	1.414530419	1.400949146	1.400342531	1.400329029	1.400329029	1.400329029	1.400328898	1.30508 $\times {10}^{-7}$
4	1.411681433	1.397897051	1.397216455	1.397201711	1.397201306	1.397201305	1.397201171	1.34093 $\times {10}^{-7}$
5	1.409473469	1.395516077	1.394782894	1.39476664	1.394766013	1.394766	1.394765863	1.36854 $\times {10}^{-7}$
6	1.407662938	1.393558187	1.392783366	1.392765881	1.39276511	1.392765094	1.392764955	1.3947 $\times {10}^{-7}$
7	1.406123987	1.391891784	1.391082138	1.391063616	1.391062732	1.391062713	1.391062571	1.41657 $\times {10}^{-7}$

,

Table A12. Values of the mild lower solutions ${\alpha }_{y_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $y_1\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\alpha }}_{{\mathit{y}}_1}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^6\left(\mathit{t}\right)$	${\mathit{y}}_1\left(\mathit{t}\right)$	$\mathcal{AE}$
1	5.515231883	5.559582255	5.562412989	5.562412992	5.562412993	5.562412993	5.562412992	4.99545 $\times {10}^{-10}$
2	5.516755071	5.559933536	5.564613122	5.565391586	5.565391587	5.565391587	5.565391586	5.62432 $\times {10}^{-10}$
3	5.517592825	5.561991456	5.567221372	5.568234381	5.568393565	5.568393565	5.568393565	5.78969 $\times {10}^{-10}$
4	5.518179253	5.56353138	5.569106674	5.570247933	5.570431954	5.570464276	5.570464275	5.60552 $\times {10}^{-10}$
5	5.518633734	5.564747011	5.570584641	5.571828226	5.572036905	5.572081596	5.572088064	6.46791 $\times {10}^{-6}$
6	5.519006408	5.565750227	5.571801776	5.57312997	5.573359197	5.573413659	5.573423525	9.86656 $\times {10}^{-6}$
7	5.519323182	5.566604573	5.572837798	5.574238658	5.574485531	5.574548204	5.574560932	1.27281 $\times {10}^{-5}$

,

Table A13. Values of the mild upper solutions ${\beta }_{y_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $y_1\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\beta }}_{{\mathit{y}}_1}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^6\left(\mathit{t}\right)$	${\mathit{y}}_1\left(\mathit{t}\right)$	$\mathcal{AE}$
1	5.599744318	5.565644401	5.562412995	5.562412993	5.562412993	5.562412993	5.562412992	4.99545 $\times {10}^{-10}$
2	5.60971875	5.571534931	5.566138125	5.565391588	5.565391587	5.565391587	5.565391586	5.62433 $\times {10}^{-10}$
3	5.615204687	5.575174147	5.569419413	5.568553867	5.568393566	5.568393565	5.568393565	6.52062 $\times {10}^{-10}$
4	5.619044843	5.577756131	5.5716654	5.570705679	5.570496523	5.570464276	5.570464275	7.62753 $\times {10}^{-10}$
5	5.622020964	5.579769748	5.573411927	5.572379322	5.572134238	5.572094536	5.572088064	6.47217 $\times {10}^{-6}$
6	5.624461384	5.581427399	5.574847942	5.573755213	5.573480935	5.573434379	5.573423525	1.08534 $\times {10}^{-5}$
7	5.62653574	5.582840358	5.576070925	5.57492671	5.574627562	5.574575135	5.574560932	1.42035 $\times {10}^{-5}$

,

Table A14. Values of the mild lower solutions ${\alpha }_{y_2}^n\left(t\right),n\in {\mathbb{Z}}_1^{6}t\in {\mathbb{Z}}_1^7$ and the exact solution $y_2\left(t\right),$ $t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\alpha }}_{{\mathit{y}}_2}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^6\left(\mathit{t}\right)$	${\mathit{y}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	9.030463766	9.083619004	9.085337684	9.085337686	9.085337686	9.085337686	9.085337685	9.99089 $\times {10}^{-10}$
2	9.033510143	9.086434568	9.089973068	9.090347081	9.090347081	9.090347081	9.09034708	1.13486 $\times {10}^{-9}$
3	9.03518565	9.089835825	9.093881243	9.094405422	9.094481404	9.094481404	9.094481403	1.22325 $\times {10}^{-9}$
4	9.036358505	9.09231546	9.096671916	9.097266809	9.097355401	9.097370586	9.097370585	1.27427 $\times {10}^{-9}$
5	9.037267468	9.094259342	9.098850207	9.099500692	9.099601414	9.099622432	9.099625466	3.03425 $\times {10}^{-6}$
6	9.038012817	9.095859784	9.10064124	9.10133736	9.101448153	9.101473773	9.101478401	4.62772 $\times {10}^{-6}$
7	9.038646364	9.097221846	9.102165037	9.102900195	9.103019626	9.103049114	9.103055083	5.96959 $\times {10}^{-6}$

and

Table A15. Values of the mild upper solutions ${\beta }_{y_2}^n\left(t\right),n\in {\mathbb{Z}}_1^{6}t\in {\mathbb{Z}}_1^7$ and the exact solution $y_2\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.1,\mu =0.1$.

t	${\mathit{\beta }}_{{\mathit{y}}_2}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^6\left(\mathit{t}\right)$	${\mathit{y}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	9.133792986	9.086904479	9.085337687	9.085337686	9.085337686	9.085337686	9.085337685	9.99089 $\times {10}^{-10}$
2	9.147172285	9.094501763	9.090721222	9.090347082	9.090347081	9.090347081	9.09034708	1.13486 $\times {10}^{-9}$
3	9.154530899	9.099117704	9.095018481	9.094557141	9.094481404	9.094481404	9.094481403	1.2593 $\times {10}^{-9}$
4	9.159681929	9.102377415	9.098003904	9.097485646	9.097385772	9.097370586	9.097370585	1.37217 $\times {10}^{-9}$
5	9.163673977	9.104912015	9.100325575	9.099764451	9.099647207	9.099628503	9.099625466	3.03658 $\times {10}^{-6}$
6	9.166947457	9.10699393	9.102232933	9.101636747	9.10150543	9.101483492	9.101478401	5.09094 $\times {10}^{-6}$
7	9.169729915	9.108765377	9.103855848	9.103229743	9.103086453	9.103061745	9.103055083	6.66205 $\times {10}^{-6}$

) enhance the convergence rates for variables $x_i$ and $y_j$, with acceleration becoming more evident as the fractional order approaches zero. Conversely, larger orders, depicted in certain Figure 2 and Figure 3 and Table 2 and

Table A16. Values of the mild upper solutions ${\beta }_{x_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_1\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\beta }}_{{\mathit{x}}_1}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_1}^6\left(\mathit{t}\right)$	$\mathit{x}\left(\mathit{t}\right)$	$\mathcal{AE}$
1	0.637999509	0.537998906	0.537998828	0.537998821	0.537998821	0.537998821	0.537998821	1.11022 $\times {10}^{-15}$
2	0.762199067	0.584598827	0.564598168	0.564598118	0.564598115	0.564598115	0.564598115	1.77636 $\times {10}^{-15}$
3	0.880188647	0.639409504	0.588927844	0.584927682	0.584927667	0.584927666	0.584927666	9.65894 $\times {10}^{-15}$
4	0.994245241	0.70206583	0.616086779	0.601782453	0.600982388	0.600982383	0.600982382	2.27345 $\times {10}^{-10}$
5	1.10545042	0.772270365	0.650021946	0.617523047	0.614063659	0.613903639	0.613903637	1.53252 $\times {10}^{-9}$
6	1.214431496	0.849779164	0.694117363	0.634423227	0.625333193	0.624472981	0.624440974	3.20066 $\times {10}^{-5}$
7	1.321596221	0.934387826	0.751413616	0.654780323	0.636057464	0.63332433	0.633121941	0.000202389

,

Table A17. Values of the mild lower solutions ${\alpha }_{x_2}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_2\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\alpha }}_{{\mathit{x}}_2}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{x}}_2}^6\left(\mathit{t}\right)$	${\mathit{x}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	1.396000036	1.411000108	1.411000116	1.411000116	1.411000116	1.411000116	1.410999999	1.1783 $\times {10}^{-7}$
2	1.302400068	1.333120176	1.333570203	1.333570206	1.333570206	1.333570206	1.333569998	2.08389 $\times {10}^{-7}$
3	1.213480098	1.261021218	1.262187671	1.262201179	1.262201179	1.262201179	1.262200897	2.82693 $\times {10}^{-7}$
4	1.127524128	1.19305714	1.195104906	1.195156867	1.195157274	1.195157274	1.195156929	3.45128 $\times {10}^{-7}$
5	1.043717056	1.128423847	1.131440519	1.131567113	1.131568896	1.131568908	1.13156851	3.98269 $\times {10}^{-7}$
6	0.961586127	1.066642179	1.070650106	1.070898897	1.070903657	1.070903727	1.070903283	4.43489 $\times {10}^{-7}$
7	0.880824046	1.007392475	1.012356153	1.012786378	1.012796358	1.012796589	1.012796108	4.80863 $\times {10}^{-7}$

,

Table A18. Values of the mild upper solutions ${\beta }_{x_2}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $x_2\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\beta }}_{{\mathit{x}}_2}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{x}}_2}^6\left(\mathit{t}\right)$	${\mathit{x}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	1.426000363	1.411000128	1.411000117	1.411000116	1.411000116	1.411000116	1.410999999	1.1783 $\times {10}^{-7}$
2	1.35940069	1.334020239	1.333570209	1.333570206	1.333570206	1.333570206	1.333569998	2.08389 $\times {10}^{-7}$
3	1.296131	1.263541341	1.262214687	1.26220118	1.262201179	1.262201179	1.262200897	2.82693 $\times {10}^{-7}$
4	1.2349703	1.197845336	1.195204837	1.195157681	1.195157274	1.195157274	1.195156929	3.45141 $\times {10}^{-7}$
5	1.175338617	1.136084928	1.131675336	1.131570848	1.131568921	1.131568909	1.13156851	3.98345 $\times {10}^{-7}$
6	1.116899568	1.077750714	1.071096239	1.070909347	1.070903793	1.070903728	1.070903283	4.4448 $\times {10}^{-7}$
7	1.059434504	1.02250005	1.013103405	1.012809357	1.012796797	1.012796594	1.012796108	4.86216 $\times {10}^{-7}$

,

Table A19. Values of the mild lower solutions ${\alpha }_{y_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $y_1\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\alpha }}_{{\mathit{y}}_1}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_1}^6\left(\mathit{t}\right)$	${\mathit{y}}_1\left(\mathit{t}\right)$	$\mathcal{AE}$
1	5.515231883	5.559582255	5.562412989	5.562412992	5.562412993	5.562412993	5.562412992	4.99545 $\times {10}^{-10}$
2	5.528940578	5.605910915	5.615148081	5.615908669	5.615908671	5.615908671	5.615908669	1.43694 $\times {10}^{-9}$
3	5.541963838	5.64348346	5.661463116	5.664261419	5.66441386	5.66441386	5.664413858	2.76659 $\times {10}^{-9}$
4	5.554552989	5.673738515	5.701981981	5.70846327	5.709120406	5.709150845	5.709150841	4.36036 $\times {10}^{-9}$
5	5.566827412	5.697390786	5.736718761	5.748842684	5.750567755	5.750729859	5.750735857	5.9987 $\times {10}^{-6}$
6	5.578856346	5.714865941	5.76542356	5.785442927	5.789004977	5.789516275	5.789553885	3.76107 $\times {10}^{-5}$
7	5.590684798	5.726444089	5.787696489	5.818135839	5.824496575	5.825740395	5.82587696	0.000136565

,

Table A20. Values of the mild upper solutions ${\beta }_{y_1}^n\left(t\right),n\in {\mathbb{Z}}_1^6,t\in {\mathbb{Z}}_1^7$ and the exact solution $y_1\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\beta }}_{{\mathit{y}}_1}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_1}^6\left(\mathit{t}\right)$	${\mathit{y}}_1\left(\mathit{t}\right)$	$\mathcal{AE}$
1	5.599744318	5.565644401	5.562412995	5.562412993	5.562412993	5.562412993	5.562412992	4.99545 $\times {10}^{-10}$
2	5.689514204	5.626830065	5.616637149	5.615908673	5.615908671	5.615908671	5.615908669	1.43694 $\times {10}^{-9}$
3	5.774795596	5.68643966	5.667107117	5.664567419	5.664413861	5.66441386	5.664413858	2.82886 $\times {10}^{-9}$
4	5.857234274	5.745069132	5.715387739	5.70986285	5.709181209	5.709150846	5.709150841	4.8081 $\times {10}^{-9}$
5	5.937611986	5.802863926	5.762338764	5.752735373	5.750898861	5.750741872	5.750735857	6.01522 $\times {10}^{-6}$
6	6.016382144	5.85983143	5.808516071	5.793946651	5.790071776	5.789592423	5.789553885	3.85376 $\times {10}^{-5}$
7	6.093839465	5.915932078	5.854306341	5.834174288	5.827143799	5.826019805	5.82587696	0.000142845

,

Table A21. Values of the mild lower solutions ${\alpha }_{y_2}^n\left(t\right),n\in {\mathbb{Z}}_1^{6}t\in {\mathbb{Z}}_1^7$ and the exact solution $y_2\left(t\right),$ $t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\alpha }}_{{\mathit{y}}_2}^1\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^2\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^3\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^4\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^5\left(\mathit{t}\right)$	${\mathit{\alpha }}_{{\mathit{y}}_2}^6\left(\mathit{t}\right)$	${\mathit{y}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	9.030463766	9.083619004	9.085337684	9.085337686	9.085337686	9.085337686	9.085337685	9.99089 $\times {10}^{-10}$
2	9.057881156	9.151691185	9.158057338	9.158422799	9.1584228	9.1584228	9.158422797	2.88387 $\times {10}^{-9}$
3	9.083927676	9.209981145	9.223217821	9.224602611	9.224675351	9.224675351	9.224675345	5.61593 $\times {10}^{-9}$
4	9.109105979	9.260271505	9.282207933	9.285470794	9.28578544	9.285799724	9.285799715	9.12941 $\times {10}^{-9}$
5	9.133654824	9.303409651	9.335568757	9.341743073	9.342572056	9.342648157	9.342650958	2.80144 $\times {10}^{-6}$
6	9.157712692	9.339870573	9.383498084	9.393778588	9.395497158	9.395737306	9.395754921	1.76152 $\times {10}^{-5}$
7	9.181369596	9.36994133	9.426014801	9.441744015	9.444826529	9.445411118	9.445475115	6.39974 $\times {10}^{-5}$

and

Table A22. Values of the mild upper solutions ${\beta }_{y_2}^n\left(t\right),n\in {\mathbb{Z}}_1^{6}t\in {\mathbb{Z}}_1^7$ and the exact solution $y_2\left(t\right),t\in {\mathbb{Z}}_1^7$ of (11) for $\vartheta =0.9,\mu =0.9$.

t	${\mathit{\beta }}_{{\mathit{y}}_2}^1\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^2\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^3\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^4\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^5\left(\mathit{t}\right)$	${\mathit{\beta }}_{{\mathit{y}}_2}^6\left(\mathit{t}\right)$	${\mathit{y}}_2\left(\mathit{t}\right)$	$\mathcal{AE}$
1	9.133792986	9.086904479	9.085337687	9.085337686	9.085337686	9.085337686	9.085337685	9.99089 $\times {10}^{-10}$
2	9.254206674	9.164893049	9.158788296	9.158422801	9.1584228	9.1584228	9.158422797	2.88387 $\times {10}^{-9}$
3	9.368599677	9.238558428	9.226053461	9.224747845	9.224675351	9.224675351	9.224675345	5.64667 $\times {10}^{-9}$
4	9.479179581	9.309143523	9.289035811	9.286136842	9.285814009	9.285799725	9.285799715	9.34908 $\times {10}^{-9}$
5	9.586994986	9.377161615	9.348741636	9.343599077	9.34272765	9.342653787	9.342650958	2.82843 $\times {10}^{-6}$
6	9.692654084	9.442861651	9.405815033	9.397838947	9.395998552	9.395772988	9.395754921	1.80672 $\times {10}^{-5}$
7	9.796552196	9.506372671	9.460713338	9.449410796	9.446070964	9.445542045	9.445475115	6.69295 $\times {10}^{-5}$

nearing one, tend to decelerate this process.

To provide clarity on these findings, Appendix A provides tables detailing absolute errors between mild solutions derived through approximations across various fractional orders. Furthermore, concerning temporal accuracy (as demonstrated in Appendix A and Appendix B), any observed decrease in convergence over time is not an inherent limitation but rather contingent upon the discretization choices, which can be adjusted according to the specific application requirements.

In conclusion, the presented graphs and tables effectively demonstrate the collective influence of iteration dynamics, time factors, and fractional order variations on convergence rates and accuracy levels.

5. Final Comments and Conclusions

This study examines nonlinear fractional difference equations with constant delay, using the Caputo-type nabla operator in discrete time over a compact interval. The order of the fractional difference operator affects interval discretization and summation representation, as discussed in [17,40]. Integer discretization is assumed for this analysis. Despite advances in continuous operators, discrete models remain essential due to progress in informatics. We have established sufficient conditions ensuring uniform convergence of two monotonous sequences of functions (specifically $\mathcal{CMLU}$), allowing for the approximation of extremal solutions through equivalent summation representations. These theoretical results have been applied to a discrete model of BAM neural networks.

Future research could explore specific models in science, engineering, or neurocomputing to further elucidate applications. By analyzing various types of fractional operators and examining scenarios where mild lower and upper solutions exist at varying initial times (see [31]), researchers can devise iterative schemes to obtain extremal mild solutions for initial value problems. Another direction involves incorporating spatial operators, as demonstrated in the study [41] on a nonlinear fractional Schrödinger–Poisson system:

$$\begin{array}{cc}& {(-\Delta )}^{s}u+V\left(x\right)u+\varphi u=f\left(u\right)\mathrm{in}{\mathbb{R}}^3,\hfill \\ & {(-\Delta )}^s\varphi =u^2\mathrm{in}{\mathbb{R}}^3,\hfill \end{array}$$

where ${(-\Delta )}^s$ is the fractional Laplacian of order $s\in ({\displaystyle \frac{3}{4}},1)$ and $V:{\mathbb{R}}^3\to \mathbb{R}$ is a potential function.

Similarly, ref. [42] discusses existence results for discrete fractional boundary value problems under nonlinear growth conditions through Schaefer’s fixed point theorem. In work [43], positive solutions for discrete delta-nabla ${\Delta }_{\nu -2}^{\beta }\left({\varphi }_p\left({}_b\nabla ^{\nu }x\left(t\right)\right)\right)$ boundary value problems with p-Laplacian operators (${\varphi }_p\left(s\right)={\left|s\right|}^{p-2}s,p>1$) can be approximated using techniques such as upper and lower solution methods combined with Schauder’s fixed point theorem. For continuous cases, sufficient conditions for the existence and uniqueness of positive solutions are presented in [44,45].