On New Generalized Mitrinović-Adamović-Type Inequalities
Abstract
1. Introduction
2. Lemmas for Bernoulli Numbers
3. Some New Generalized Mitrinović–Adamović-Type Inequalities
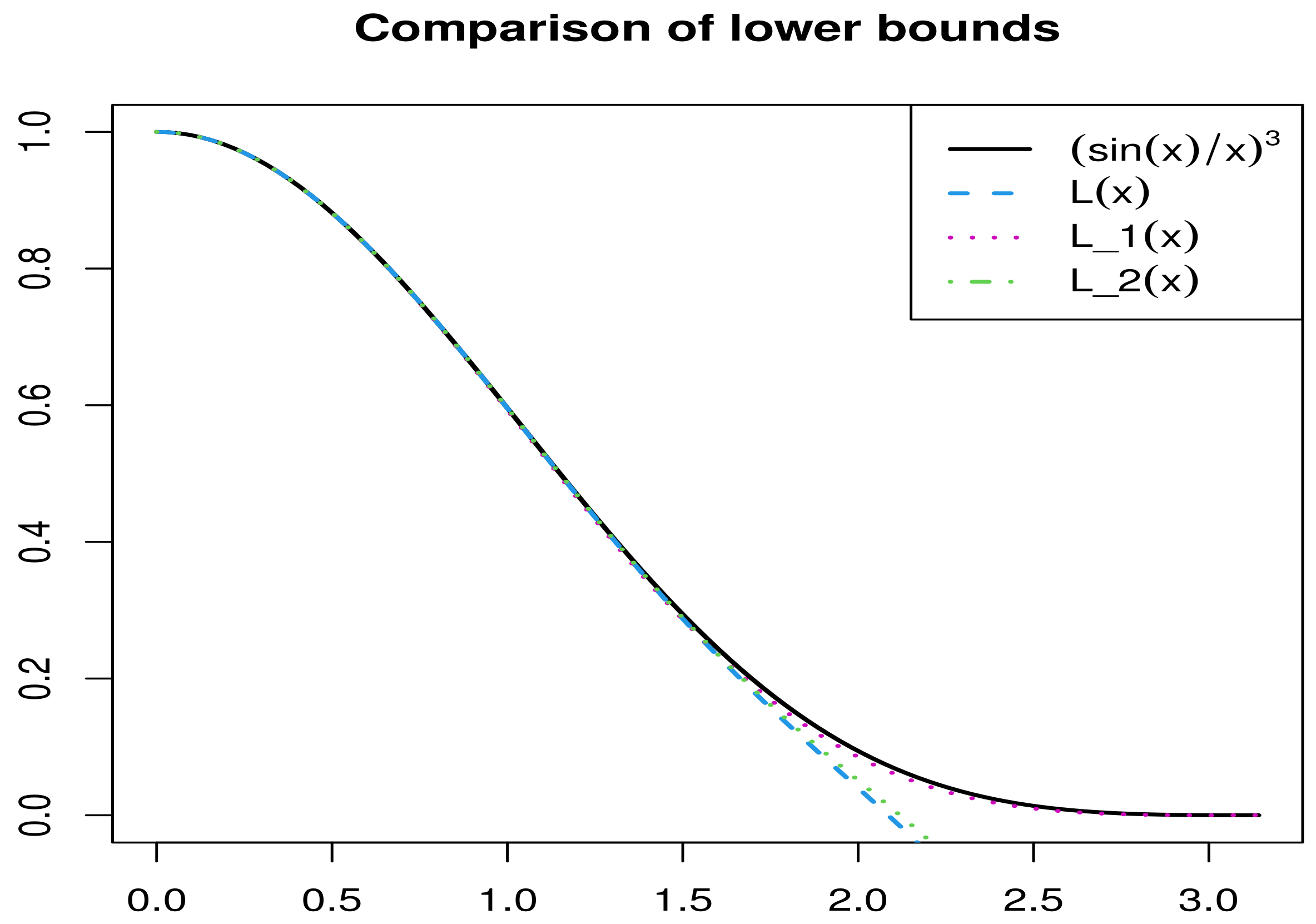
- (i)
- The double inequalityholds with the best possible constants and .
- (ii)
- The double inequalityholds with the best possible constants and .
- (i)
- The double inequalityholds with the best possible constants and
- (ii)
- The double inequalityholds with the best possible constants and
- (i)
- The double inequalityholds with the best possible constants and
- (ii)
- The double inequalityholds with the best possible constants and
4. Conclusions
- (See Theorem 2):
- (i)
- The double inequalityholds with the best possible constants and .
- (ii)
- The double inequalityholds with the best possible constants and .
- (See Theorem 4):
- (i)
- The double inequalityholds with the best possible constants and
- (ii)
- The double inequalityholds with the best possible constants and
- (See Theorem 6):
- (i)
- The double inequalityholds with the best possible constants and
- (ii)
- The double inequalityholds with the best possible constants and
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mitrinović, D.S.; Adamović, D.D. Sur une Inegalite Elementaire ou Interviennent des Fonctions Trigonometriques; Univerzitet u Beogradu: Belgrade, Serbia, 1965; pp. 23–34. [Google Scholar]
- Mitrinović, D.S.; Adamović, D.D. Complement a L’article “Sur une Inegalite Elementaire ou Interviennent des Fonctions Trigonometriques”; Univerzitet u Beogradu: Belgrade, Serbia, 1966; pp. 31–32. [Google Scholar]
- Lv, Y.-P.; Wang, G.-D.; Chu, Y.-M. A note on Jordan type inequalities for hyperbolic functions. Appl. Math. Lett. 2012, 25, 505–508. [Google Scholar] [CrossRef][Green Version]
- Neuman, E.; Sándor, J. On some inequalities involving trigonometric and hyperbolic functions with emphasis on the Cusa-Huygens, Wilker, and Huygens inequalities. Math. Inequal. Appl. 2010, 13, 715–723. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Jiang, Y.-L.; Song, Y.-Q.; Chu, Y.-M. Sharp inequalities for trigonometric functions. Abstr. Appl. Anal. 2014, 2014, 601839. [Google Scholar] [CrossRef]
- Mortici, C. The natural approach of Wilker-Cusa-Huygens inequalities. Math. Inequal. Appl. 2011, 14, 535–541. [Google Scholar] [CrossRef]
- Chouikha, A.R. New sharp inequalities related to classical trigonometric inequalities. J. Inequal. Spec. Funct. 2020, 11, 27–35. [Google Scholar]
- Zhu, L. New Mitrinović-Adamović type inequalities. RACSAM 2020, 114, 119. [Google Scholar] [CrossRef]
- Jiang, W.-D. New sharp inequalities of Mitrinović-Adamović type. Appl. Anal. Discrete Math. 2023, 17, 76–91. [Google Scholar] [CrossRef]
- Chen, C.-P.; Sándor, J. Inequality chains for Wilker, Huygens and Lazarević type inequalities. J. Math. Inequal. 2014, 8, 55–67. [Google Scholar] [CrossRef]
- Chouikha, A.R. On natural approaches related to classical trigonometric inequalities. Open J. Math. Sci. 2023, 7, 299–320. [Google Scholar] [CrossRef]
- Malešević, B.; Malešević, B. A minimax approximant in the theory of analytic inequalities. Appl. Anal. Discrete Math. 2021, 154, 486–509. [Google Scholar] [CrossRef]
- Nenezić, M.; Malešević, B.; Mortici, C. New approximations of some expressions involving trigonometric functions. Appl. Math. Comput. 2016, 283, 299–315. [Google Scholar] [CrossRef]
- Qian, G.; Chen, X.-D. Improved bounds of Mitrinović-Adamović-type inequalities by using two-parameter functions. J. Inequal. Appl. 2023, 2023, 25. [Google Scholar] [CrossRef]
- Sándor, J. Sharp Cusa-Huygens and related inequalities. Notes Number Theory Discret. Math. 2013, 19, 50–54. [Google Scholar]
- Wu, S.-H.; Yue, H.-P.; Deng, Y.-P.; Chu, Y.-M. Several improvements of Mitrinović-Adamović and Lazarević inequalities with applications to the sharpening of Wilker-type inequalities. J. Nonlinear Sci. Appl. 2016, 9, 1755–1765. [Google Scholar] [CrossRef]
- Wu, S.-H.; Li, S.-G.; Bencze, M. Sharpened versions of Mitrinović-Adamović, Lazarević and Wilker’s inequalities for trigonometric and hyperbolic functions. J. Nonlinear Sci. Appl. 2016, 9, 2688–2696. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Chu, Y.-M. A note on Jordan, Mitrinović-Adamović, and Cusa inequalities. Abstr. Appl. Anal. 2014, 2014, 364076. [Google Scholar] [CrossRef]
- Zhang, L.-N.; Ma, X.-S. Some new results of Mitrinović-Cusa’s and related inequalities based on the interpolation and approximation method. J. Math. 2021, 2021, 5595650. [Google Scholar] [CrossRef]
- Zhu, L. On Frame’s inequalities. J. Inequal. Appl. 2018, 2018, 94. [Google Scholar] [CrossRef]
- Zhu, L. Sharp inequalities of Mitrinović-Adamović type. RACSAM 2019, 113, 957–968. [Google Scholar] [CrossRef]
- Zhu, L. An unity of Mitrinović-Adamović and Cusa-Huygens inequalities and the analogue for hyperbolic functions. RACSAM 2019, 113, 3399–3412. [Google Scholar] [CrossRef]
- Zhu, L. New bounds for the ratio of two adjacent even-indexed Bernoulli numbers. RACSAM 2020, 114, 83. [Google Scholar] [CrossRef]
- Zhu, L.; Zhang, R.-J. New inequalities of Mitrinović-Adamović type. RACSAM 2022, 116, 34. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. (Eds.) Handbook of Mathematical Functions with Formulas, Graphs and Mathematical Tables. In Applied Mathematics Series, 10th Printing; National Bureau of Standards: Washington, DC, USA, 1972; Volume 55. [Google Scholar]
- D’Aniello, C. On some inequalities for the Bernoulli numbers. Rend. Circ. Mat. Palermo Ser. 2 1994, 43, 329–332. [Google Scholar] [CrossRef]
- Qi, F. A double inequality for the ratio of two non-zero neighbouring Bernoulli numbers. J. Comput. Appl. Math. 2019, 351, 1–5. [Google Scholar] [CrossRef]
- Bagul, Y.J.; Panchal, S.K. Certain inequalities of Kober and Lazarevi’c type. J. Indian Math. Soc. 2022, 89, 1–7. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bagul, Y.J.; Du, W.-S. On New Generalized Mitrinović-Adamović-Type Inequalities. Mathematics 2025, 13, 1174. https://doi.org/10.3390/math13071174
Bagul YJ, Du W-S. On New Generalized Mitrinović-Adamović-Type Inequalities. Mathematics. 2025; 13(7):1174. https://doi.org/10.3390/math13071174
Chicago/Turabian StyleBagul, Yogesh J., and Wei-Shih Du. 2025. "On New Generalized Mitrinović-Adamović-Type Inequalities" Mathematics 13, no. 7: 1174. https://doi.org/10.3390/math13071174
APA StyleBagul, Y. J., & Du, W.-S. (2025). On New Generalized Mitrinović-Adamović-Type Inequalities. Mathematics, 13(7), 1174. https://doi.org/10.3390/math13071174