Langlands Duality and Invariant Differential Operators
Abstract
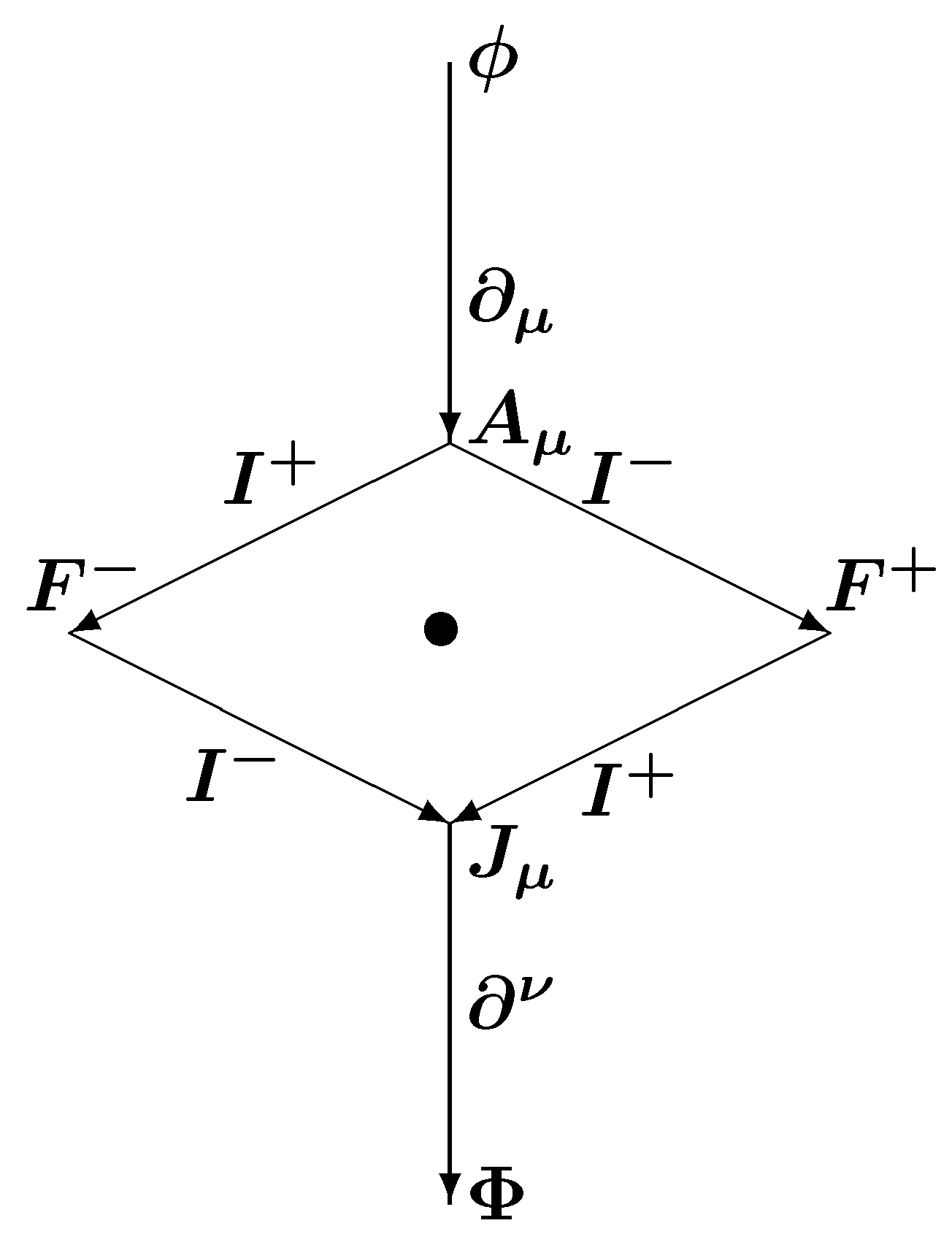
1. Introduction
2. Preliminaries
3. Main Results
3.1. Restricted Weyl Groups and Related Notions
3.2. The Case of
3.3.
3.4.
3.5. Reduced Multiplets
4. Case sl(8)
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Langlands, R.P. Problems in the theory of automorphic forms. Lecture Notes Math. 1970, 170, 18–61. [Google Scholar]
- Langlands, R.P. On the Classification of Irreducible Representations of Real Algebraic Groups. Mimeographed notes Princeton 1973. Math. Surveys Monogr. 1989, 31, 101–170. [Google Scholar]
- Adams, J.; Vogan, D.A., Jr. Contragredient representations and characterizing the local Langlands correspondence. Am. J. Math. 2016, 138, 657–682. [Google Scholar] [CrossRef]
- Aganagic, M.; Frenkel, E.; Okounkov, A. Quantum q-Langlands Correspondence. Trans. Moscow Math. Soc. 2018, 79, 1–83. [Google Scholar] [CrossRef]
- Alekseev, A.; Berenstein, A.; Hoffman, B.; Li, Y. Langlands Duality and Poisson-Lie Duality via Cluster Theory and Tropicalization. Sel. Math. New Ser. 2021, 27, 69. [Google Scholar] [CrossRef]
- Arakawa, T.; Frenkel, E. Quantum Langlands duality of representations of W-algebras. Compos. Math. 2019, 155, 2235–2262. [Google Scholar] [CrossRef]
- Balasubramanian, A.; Teschner, J. Supersymmetric field theories and geometric langlands: The other side of the coin. Proc. Symp. Pure Math. 2018, 98, 79–105. [Google Scholar]
- Beilinson, A. Langlands parameters for Heisenberg modules. In Studies in Lie Theory. Progress in Mathematics; Bernstein, J., Hinich, V., Melnikov, A., Eds.; Birkhäuser: Boston, FL, USA, 2006; Volume 243. [Google Scholar]
- Ben-Zvi, D.; Nadler, D. Loop Spaces and Langlands Parameters. arXiv 2007, arXiv:0706.0322. [Google Scholar] [CrossRef]
- Ben-Zvi, D.; Sakellaridis, Y.; Venkatesh, A. Relative Langlands Duality. arXiv 2024, arXiv:2409.04677. [Google Scholar] [CrossRef]
- Braverman, A.; Kazhdan, D. Normalized intertwining operators and nilpotent elements in the Langlands dual group. Moscow Math. J. 2002, 2, 533–553. [Google Scholar] [CrossRef]
- Campbell, J.; Raskin, S. Langlands duality on the Beilinson-Drinfeld Grassmannian. arXiv 2023, arXiv:2310.19734. [Google Scholar] [CrossRef]
- Chen, T.-H.; Nadler, D. Real groups, symmetric varieties and Langlands duality. arXiv 2024, arXiv:2403.13995. [Google Scholar] [CrossRef]
- Chen, E.Y.; Venkatesh, A. Some Singular Examples of Relative Langlands Duality. arXiv 2024, arXiv:2405.18212. [Google Scholar]
- Chua, A. Kazhdan-Lusztig map and Langlands duality. arXiv 2024, arXiv:2403.07080. [Google Scholar] [CrossRef]
- Dat, J.-F.; Helm, D.; Kurinczuk, R.; Moss, G. Local Langlands in families: The banal case. arXiv 2024, arXiv:2406.09283. [Google Scholar] [CrossRef]
- Dinh, D.; Teschner, J. Classical limit of the geometric Langlands correspondence for SL(2,C). arXiv 2023, arXiv:2312.13393. [Google Scholar] [CrossRef]
- Donagi, R.; Pantev, T. Langlands duality for Hitchin systems. Invent. Math. 2012, 189, 653–735. [Google Scholar] [CrossRef]
- Drinfeld, V.G. Proof of the global Langlands conjecture for GL(2) over a function field. Funct. Anal. Its Appl. 1977, 11, 223–225. [Google Scholar] [CrossRef]
- Espinosa, M. The Multiplicative Formula of Langlands for Orbital Integrals in GL(2). arXiv 2024, arXiv:2402.08013. [Google Scholar] [CrossRef]
- Etingof, P.; Frenkel, E.; Kazhdan, D. A general framework for the analytic Langlands correspondence. Pure Appl. Math. Quart. 2024, 20, 307–426. [Google Scholar] [CrossRef]
- Fargues, L. Cohomology of moduli spaces of p-divlsible groups and local Langlands correspondence. Asterisque 2004, 291, 1–199. [Google Scholar]
- Fargues, L.; Scholze, P. Geometrization of the local Langlands correspondence. arXiv 2021, arXiv:2102.13459. [Google Scholar] [CrossRef]
- Feigin, B.; Frenkel, E. Quantization of soliton systems and Langlands duality. In Exploration of New Structures and Natural Constructions in Mathematical Physics; Advanced Studies in Pure Mathematics; Mathematical Society of Japan: Japan, Tokyo, 2011; Volume 61, p. 185274. [Google Scholar]
- Frenkel, E. Langlands Program, Trace Formulas, and their Geometrization. Bull. AMS 2013, 50, 1–55. [Google Scholar] [CrossRef]
- Frenkel, E.; Gaiotto, D. Quantum Langlands dualities of boundary conditions, D-modules, and conformal blocks. Commun. Number Theory Phys. 2020, 14, 199–313. [Google Scholar] [CrossRef]
- Frenkel, E.; Gaitsgory, D.; Kazhdan, D.; Vilonen, K. Geometric Realization of Whittaker Functions and the Langlands Conjecture. J. AMS 1998, 11, 451–484. [Google Scholar] [CrossRef]
- Frenkel, E.; Gaitsgory, D.; Vilonen, K. On the geometric Langlands conjecture. J. AMS 2022, 15, 367–417. [Google Scholar] [CrossRef]
- Frenkel, E.; Hernandez, D. Spectra of Quantum KdV Hamiltonians, Langlands Duality, and Affine Opers. Commun. Math. Phys. 2018, 362, 361–414. [Google Scholar] [CrossRef]
- Gaiotto, D.; Teschner, J. Quantum Analytic Langlands Correspondence. arXiv 2024, arXiv:2402.00494. [Google Scholar] [CrossRef]
- Gaiotto, D.; Witten, E. Gauge Theory and the Analytic Form of the Geometric Langlands Program. Ann. Henri Poincare 2024, 25, 557–671. [Google Scholar] [CrossRef]
- Ginzburg, V. Langlands Reciprocity for Algebraic Surfaces. arXiv 1995, arXiv:q-alg/9502013. [Google Scholar] [CrossRef]
- Gukov, S.; Witten, E. Gauge Theory, Ramification, and the Geometric Langlands Program. arXiv 2006, arXiv:hep-th/0612073. [Google Scholar] [CrossRef]
- Hameister, T.; Luo, Z.; Morrissey, B. Relative Dolbeault Geometric Langlands via the Regular Quotient. arXiv 2024, arXiv:2409.15691. [Google Scholar] [CrossRef]
- Hansen, D. Beijing notes on the categorical local Langlands conjecture. arXiv 2024, arXiv:2310.04533. [Google Scholar] [CrossRef]
- Hausel, T. Enhanced mirror symmetry for Langlands dual Hitchin systems. arXiv 2022, arXiv:2112.09455. [Google Scholar] [CrossRef]
- Hitchin, N. Langlands duality and G2 spectral curves. Q. J. Math. 2007, 58, 319–344. [Google Scholar] [CrossRef]
- van den Hove, T. The stack of spherical Langlands parameters. arXiv 2024, arXiv:2409.09522. [Google Scholar] [CrossRef]
- Ikeda, K. Topological aspects of matters and Langlands program. Rev. Math. Phys. 2024, 36, 2450005. [Google Scholar] [CrossRef]
- Imai, N. On the geometrization of the local Langlands correspondence. arXiv 2024, arXiv:2408.16571. [Google Scholar] [CrossRef]
- Jeong, S.; Lee, N.; Nekrasov, N. di-Langlands correspondence and extended observables. J. High Energy Phys. 2024, 6, 105. [Google Scholar] [CrossRef]
- Kapustin, A.; Witten, E. Electric-Magnetic Duality and the Geometric Langlands Program. Commun. Num. Theor. Phys. 2007, 1, 1–236. [Google Scholar] [CrossRef]
- Kimura, T.; Noshita, G. Gauge origami and quiver W-algebras II: Vertex function and beyond quantum q-Langlands correspondence. arXiv 2024, arXiv:2404.17061. [Google Scholar] [CrossRef]
- Koroteev, P.; Sage, D.S.; Zeitlin, A.M. (SL(N),q)-opers, the q-Langlands correspondence, and quantum/classical duality. Commun. Math. Phys. 2021, 381, 641–672. [Google Scholar] [CrossRef]
- Lafforgue, V. Chtoucas for Reductive Groups and Parameterization of Global Langlands. J. AMS 2018, 31, 719–891. [Google Scholar]
- Lenart, C.; Zhao, G.; Zhong, C. Elliptic classes via the periodic Hecke module and its Langlands dual. arXiv 2023, arXiv:2309.09140. [Google Scholar] [CrossRef]
- De Martino, M.; Opdam, E. A remark on the Langlands correspondence for tori. arXiv 2024, arXiv:2410.06346. [Google Scholar] [CrossRef]
- Matringe, N. Local converse theorems and Langlands parameters. arXiv 2024, arXiv:2409.20240. [Google Scholar] [CrossRef]
- Nakajima, H. S-dual of Hamiltonian G spaces and relative Langlands duality. arXiv 2024, arXiv:2409.06303. [Google Scholar] [CrossRef]
- Suzuki, K. Rationality of the Local Jacquet-Langlands Correspondence for GL(n). arXiv 2023, arXiv:2307.06039. [Google Scholar] [CrossRef]
- Tai, T.S. Seiberg-Witten prepotential from WZNW conformal block: Langlands duality and Selberg trace formula. Mod. Phys. Lett. A 2012, 27, 1250129. [Google Scholar] [CrossRef]
- Tan, M.-C. M-Theoretic Derivations of 4d-2d Dualities: From a Geometric Langlands Duality for Surfaces, to the AGT Correspondence, to Integrable Systems. J. High Energy Phys. 2013, 7, 171. [Google Scholar] [CrossRef]
- Teschner, J. Quantization of the Hitchin moduli spaces, Liouville theory and the geometric Langlands correspondence I. Adv. Theor. Math. Phys. 2011, 15, 471–564. [Google Scholar] [CrossRef]
- Witten, E. More on gauge theory and geometric Langlands. Adv. Math. 2018, 327, 624–707. [Google Scholar] [CrossRef]
- Dobrev, V.K. Invariant Differential Operators, Volume 1: Noncompact Semisimple Lie Algebras and Groups; De Gruyter Studies in Mathematical Physics; De Gruyter: Berlin, Germany; Boston, MA, USA, 2016; Volume 35, p. 408. ISBN 978-3-11-042764-6. [Google Scholar]
- Knapp, A.W.; Zuckerman, G.J. Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 1977; Volume 587, pp. 138–159. [Google Scholar]
- Dobrev, V.K. Positive energy unitary irreducible representations of D = 6 conformal supersymmetry. J. Phys. 2002, A35, 7079–7100. [Google Scholar] [CrossRef]
- Collacciani, E. A Reduction over finite fields of the tame local Langlands correspondence for SLn. arXiv 2025, arXiv:2501.09085. [Google Scholar] [CrossRef]
- Scholze, P. Geometrization of the local Langlands correspondence, motivically. arXiv 2025, arXiv:2501.07944. [Google Scholar] [CrossRef]
- Braverman, A.; Finkelberg, M.; Kazhdan, D.; Travkin, R. Relative Langlands duality for osp(2n + 1|2n). arXiv 2024, arXiv:2412.20544. [Google Scholar] [CrossRef]
- Tong, X. p-adic Local Langlands Correspondence. arXiv 2013, arXiv:2412.12055. [Google Scholar] [CrossRef]
- Letellier, E.; Scognamiglio, T. PGL2(C) -character stacks and Langlands duality over finite fields. arXiv 2024, arXiv:2412.03234. [Google Scholar] [CrossRef]
- Kapranov, M.; Schechtman, V.; Schiffmann, O.; Yuan, J. The Langlands formula and perverse sheaves. arXiv 2024, arXiv:2412.01638. [Google Scholar] [CrossRef]
- Solleveld, M.; Xu, Y. Hecke algebras and local Langlands correspondence for non-singular depth-zero representations. arXiv 2024, arXiv:2411.19846. [Google Scholar] [CrossRef]
- Dospinescu, G.; Esteban Rodríguez Camargo, J. A Jacquet-Langlands functor for p-adic locally analytic representations. arXiv 2024, arXiv:2411.17082. [Google Scholar] [CrossRef]
- Dobrev, V.K.; Mack, G.; Petkova, V.B.; Petrova, S.G.; Todorov, I.T. Harmonic Analysis on the n-Dimensional Lorentz Group and Its Application to Conformal Quantum Field Theory. Lecture Notes Phys. 1977, 63, 1–280. [Google Scholar]
- Knapp, A.W. Lie Groups Beyond an Introduction, 2nd ed.; Progr. Math.; Birkhäuser: Boston, MA, USA; Basel, Switzerland; Stuttgart, Germany, 2002; Volume 140. [Google Scholar]
- Dobrev, V.K. Multiplet classification of the reducible elementary representations of real semisimple Lie groups: The SOe(p,q) example. Lett. Math. Phys. 1985, 9, 205–211. [Google Scholar] [CrossRef]
- Dobrev, V.K.; Petkova, V.B. On the group-theoretical approach to extended conformal supersymmetry: Classification of multiplets. Lett. Math. Phys. 1985, 9, 287–298. [Google Scholar] [CrossRef]
- Dixmier, J. Enveloping Algebras; North Holland: New York, NY, USA, 1977. [Google Scholar]
- Jacquet, H.; Rallis, S. Uniqueness of linear periods. Compos. Math. 1996, 102, 65–123. [Google Scholar]
- Knapp, A.W.; Stein, E.M. Interwining operators for semisimple groups. Ann. Math. 1971, 93, 489–578. [Google Scholar] [CrossRef]
- Gel’fand, I.M.; Graev, M.I.; Vilenkin, N.Y. Generalised Functions; Academic Press: New York, NY, USA, 1966; Volume 5. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dobrev, V. Langlands Duality and Invariant Differential Operators. Mathematics 2025, 13, 855. https://doi.org/10.3390/math13050855
Dobrev V. Langlands Duality and Invariant Differential Operators. Mathematics. 2025; 13(5):855. https://doi.org/10.3390/math13050855
Chicago/Turabian StyleDobrev, Vladimir. 2025. "Langlands Duality and Invariant Differential Operators" Mathematics 13, no. 5: 855. https://doi.org/10.3390/math13050855
APA StyleDobrev, V. (2025). Langlands Duality and Invariant Differential Operators. Mathematics, 13(5), 855. https://doi.org/10.3390/math13050855






