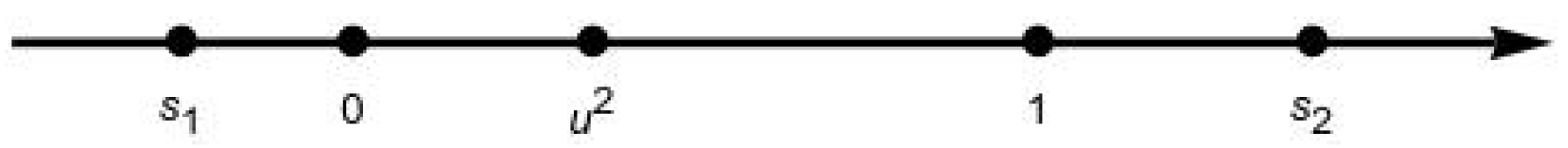1. Introduction
This work focuses on the differential equations governing the nonlinear dynamics of conservative oscillators. The current goal is to analyze the oscillator as a theoretical tool, in its mathematical aspects, leaving to future studies the physical effects, such as non-autonomous forcing perturbations, friction, or dissipation. This does not detract from the importance of this study, which represents a necessary starting model for applied systems. In this way, we complete the theoretical presentation of the oscillators treated in [
1,
2,
3,
4,
5,
6,
7] in the light of what has already been produced in [
8], adapting our solution method to the problems arising from the restoring forces (
2) and (
3) treated here and gaining notable results in terms of approximation obtained.
Specifically, we will deal with restoring forces, which, being odd functions, satisfy a precise symmetry condition, and which will be approximated with Chebyshev series arrested at the fifth order, to allow the closed-form solution of the quinticate system obtained. The term ‘quinticate’ is adopted to indicate that the solution method includes a quintication step, where the original restoring force is replaced by an equivalent expression, given as a function of quintic terms [
9,
10,
11].
We follow the approach introduced in [
8]. However, the present work does not constitute a mere replica of the procedures used therein, since a different wave configuration is considered here, which has never been addressed before. The root pattern in Theorem 1 is new and generates periodic waves different from those studied previously. As a further result of considering this new configuration, the present work demonstrates, once again, the stability of our method, when the type of wave generated by the original oscillator varies.
Overall, our procedure revises, refines, and extends earlier results on exact analytic solutions of quintic oscillators [
12,
13,
14,
15,
16] and related fifth-order Chebyshev models [
9,
10,
17]. In particular, two are the main features, induced by the nature of the restoring forces, which are of interest in our research. First of all, the class of dynamical systems examined generates, through the fifth-order truncated Chebyshev series, oscillators not yet considered in the literature: the point is that the nature of the oscillations is determined not only by the degree, as erroneously stated in [
14], but also by the sign and position of the roots of the associated potential function; in the present work, the latter indicate types of elliptic integrals different from those encountered in [
8] and therefore inverted via elliptic functions not used in [
8]. Second, the two models presented here are such that both the time-integral and related period and the motion-integral cannot be evaluated in closed form; hence, the quintic approximation provides a symbolic estimate of the period energy function, which is then compared with the asymptotic period estimate obtained using results in [
18].
This article is organized as follows. In
Section 2, we describe how to construct the quinticate form of the original oscillator, in a general case, and how to arrive at the time-integral equation associated with the quinticate oscillator. We show that the periodic solution to the latter equation is linked to the roots of a second-order polynomial, and we state and prove Theorem 1, which provides a solution and period in closed form, under a particular roots configuration. In
Section 3, our new solution process is applied to two Duffing oscillator models, and validated both qualitatively and quantitatively; further wave configurations have been considered, and the method has been validated on them, in [
8], of which this work is a completion. In
Section 4, a concise step-by-step description of our resolution procedure is provided. The conclusions are drawn in
Section 5, where some indications on future work, currently under development, are also provided.
Before ending this section, let us recall how the literature dedicated to nonlinear oscillatory problems is rich in different techniques and approaches. The introductory sections of works such as [
8,
9,
11,
16], just to name a few, contain a classification and a short discussion of many of these approaches. The class of Chebyshev Quintication methods, to which our procedure belongs, is briefly discussed in [
11], where the advantages of using the Chebyshev series expansion of the restoring force are reported, in particular with respect to the Taylor series. Further motivations for the use of orthogonal Chebyshev polynomials are given, for example, in [
8] and in the references therein, and will be highlighted in
Section 2 and
Section 3; for now, let us just recall their good numerical properties, in terms of error propagation, and the fact that they provide near-minimax approximations.
The mathematical complexity of the inversion of the elliptic integral that governs the time evolution of the quinticate system is also mentioned in [
11]: with our method, we manage to overcome this complexity. A generalization to higher-order Chebyshev polynomials will be the subject of future work, since it involves the inversion of hyperelliptic integrals and a level of mathematical sophistication still under exploration.
2. General Quintic Oscillator
We are interested in differential models configured as initial value problems (IVPs):
with odd
and positive initial displacement
a such that
without loss of generality,
can also be assumed. In particular, we consider:
which respectively represent the dimensionless form of the Duffing harmonic oscillator [
1,
2] and an instance of the generalized Duffing oscillator [
3,
4,
5,
6,
7].
The motion governed by (
1), with restoring force (
2) or (
3), is periodic, and the particle satisfies
As shown in [
8], finding
implies solving a time-integral equation
where:
The auxiliary function
with simple zeroes
in the cases of our interest, appears in the solution period too:
The inversion
often involves unknown functions and, therefore, cannot provide the explicit description of the motion.
To obtain an exactly invertible time-integral, we construct a near-minimax approximation of
in terms of fifth-order truncated Chebyshev series. For this aim, we use the change of variable
to normalize
on the interval
and associate the original problem (
1) with the following IVP:
The normalized restoring force
is then expanded in Chebyshev series, truncated (or projected) at order five:
where
are first-kind Chebyshev polynomials of integer order
while coefficients
result from integrating a weighted inner product [
19]:
Formulae (
8) and (
9), where
yield an efficient and easy-to-handle near-minimax approximation [
8]. This follows from the properties of Chebyshev polynomials, which ensure that a Lipschitz continuous function
admits, on its domain
a unique representation in terms of an absolutely and uniformly convergent Chebyshev series:
where:
Depending on the regularity of
one can assume that the coefficients
decrease in magnitude rapidly enough to imply that
equioscillates
times on
so that
is a near-minimax approximation of
[
19].
Expressing the projected force in the monomial base, a quinticate IVP replaces (
7):
where:
In more detail, the relations between the Chebyshev coefficients
and the coefficients
in quinticate form (
10) are the following, where
Applying (
4)–(
5) to the quinticate force in (
10), we can see that the squared solution of (
10) follows from evaluating and then inverting the following elliptic integral:
where
is a parabola of the form:
In detail, to arrive at (
12), we applied (
4)–(
5) to (
10), computing:
and employing the change of variable
so that
The discriminant of parabola
discarding the factor
is:
Given the physical nature of the models under investigation, coefficients
can be assumed to be such that
This scenario has already been explored in [
8] in the the following two cases:
Here, the study of oscillators of the form (
1)–(
2), or (
1)–(
3), led us to consider a third situation of a quinticate oscillator, never treated before, in which:
The configuration (
17) of the coefficients implies that the two roots
of
have opposite signs, and that the interval
where the motion takes place, is included between them, as illustrated in
Figure 1.
Within this scenario, we can describe the solution to problem (
10), and its period, in closed form: we state and prove it in Theorem 1.
Theorem 1. Under condition (17), the quinticate problem (10) has exact squared solution and exact period given by:where: being the two roots of the parabola defined in (13). denotes the complete elliptic integral of the first kind, is the elliptic modulus, and is the elliptic integral of the first kind:
is given by the Jacobi amplitude function inverse of in the sense that Finally, is the Jacobi cosine amplitude and is the Jacobi sine amplitude. Proof. The time-integral (
12) can be rewritten as follows:
The elliptic integral (
23) can be evaluated using entry 3.147–5 of [
20], which we recall here for ease of reading:
where:
Here, it is
hence,
turns out to be as in (
21), while (
23) becomes:
Through the inversion of
in equality (
25), it is possible to see that the explicit representation of the squared solution of (
10) is indeed:
with a period given by:
□
3. Application to the Duffing Oscillators
For the original oscillator (
1) with restoring force (
2) or (
3), the normalized force is respectively:
whose auxiliary function
defined in (
5) and function
in (
4) become:
where
while
respectively for (
26), (
27).
In both cases, the time-integral equation
does not admit a closed-form representation of its primitive, due to the presence of the logarithm and the powers in the term
appearing in Formula (
28).
This is also a further justification for using the Chebyshev expansion, instead of the Taylor one. The latter, in fact, gives a good approximation only at points, unlike the global approximation provided by the Chebyshev polynomials: this point-specific feature would negatively affect the Taylor approximation of the logarithm in away from zero. Furthermore, the Taylor expansion of would be limited to degree higher degrees would require the use of high-order hypergeometric functions, making the level of complexity excessive, and definitely more expensive than that implied by our method.
We, then, form the associated quinticate oscillator (
10), with
that verify (
11).
Here, the integrand in (
9), yielding the Chebyshev coefficients
takes the form
where
in the case of (
26), while
for (
27).
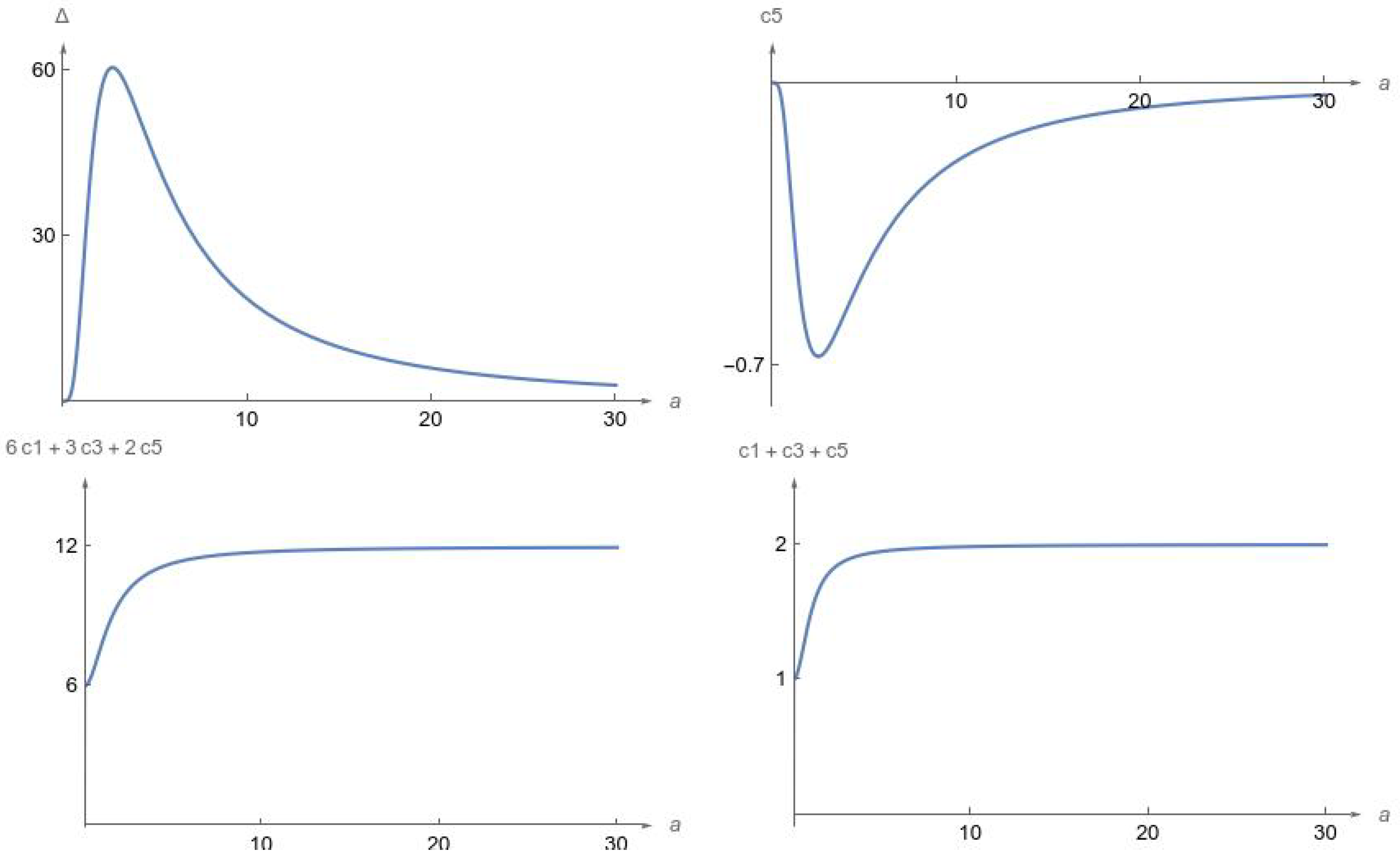
At this point, based on coefficients
and using (
14), we form the discriminant
of the second-order polynomial
and find that hypothesis (
17) of Theorem 1 holds for the quinticate oscillators under study. This is shown in
Figure 2 and
Figure 3.
To deal with the elaborated expressions involved in condition (
17), we developed our method and analysis within the
Mathematica scientific environment [
21,
22], exploiting both symbolic and numeric resources, and graphics capabilities, sometimes in a hybrid manner [
23,
24].
The sought solution is the square root of (
18), which we validated, qualitatively and quantitatively, through the differential operator:
whose behavior and maximum norm
are studied in the first quarter of the period and for various values of the displacement
The quinticate oscillator is periodic and conservative, just like the original oscillator, and its solution and period are computed exactly using (
18) and (
19). Consequently, it suffices to study the differential operator (
30) in the first quarter of the period, observing its deviation from zero, a value that would be obtained by applying (
30) to the exact solution of the original oscillator.
Integration over longer time intervals is not an usual task in the case of periodic motion, where restarting is commonly performed after observing the motion for a period. This is also the motivation for using, if we want to numerically compute the solution of the original oscillator, an LSODE approach, namely, the Livermore Solver for Ordinary Differential Equations [
25] available in
Mathematica, equipped with switching between a non-stiff Adams method and a stiff Gear Backward Differentiation Formula method [
26]; symplectic methods for long time integration, also available in
Mathematica [
27], are not needed here, and they go beyond the current scope of this research.
The period of the original oscillator can be numerically computed by integration, in which case a Gauss–Kronrod rule is used, by default, in one dimension: it consists in adaptive Gaussian quadrature, with error estimation based on evaluation at Kronrod points [
28]; other methods, also available, are not necessary in the cases considered here.
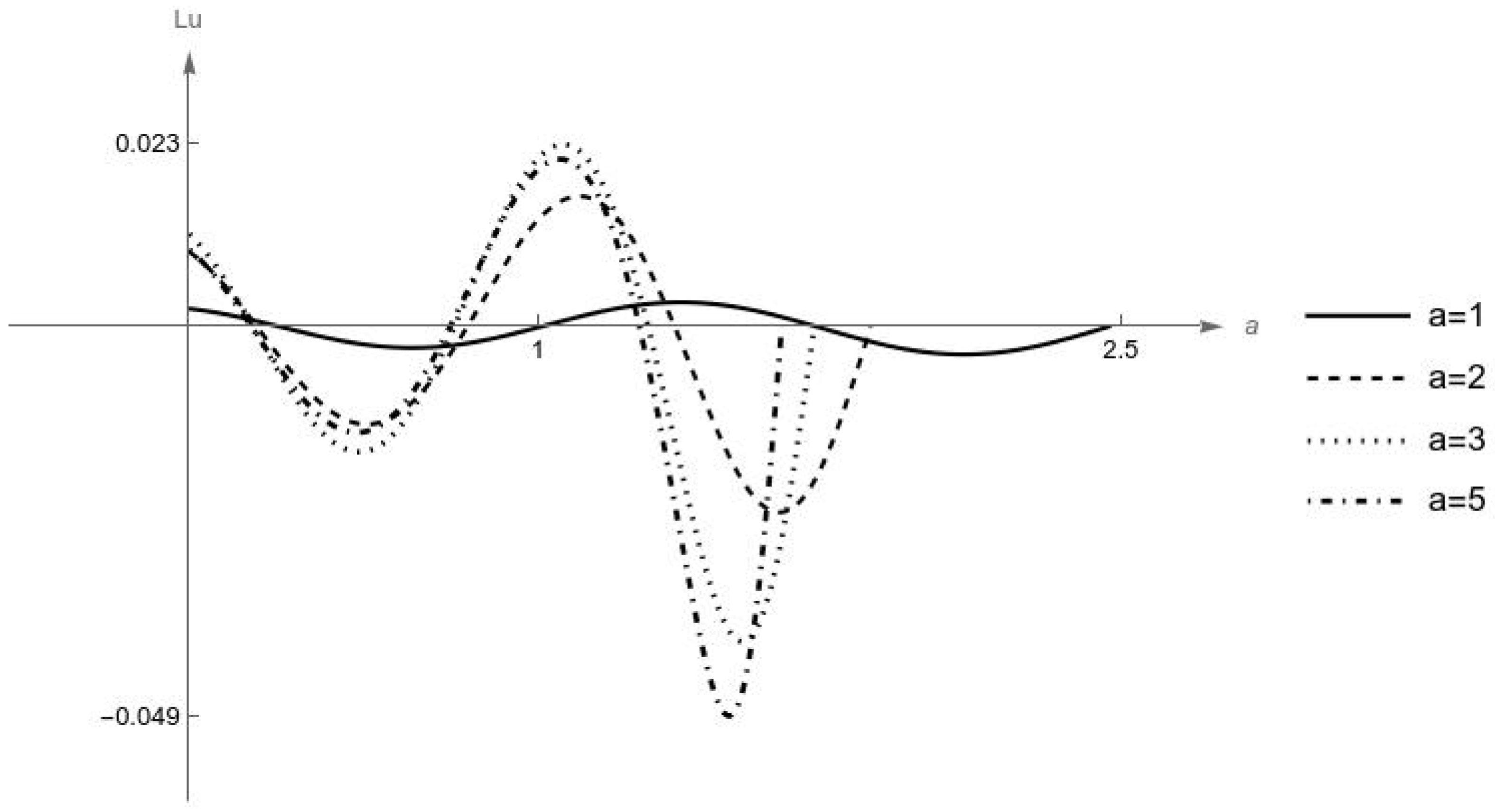
Figure 4 shows a graph of the differential operator (
30) applied to the quinticate oscillator associated with oscillator (
1)–(
2), at initial displacements
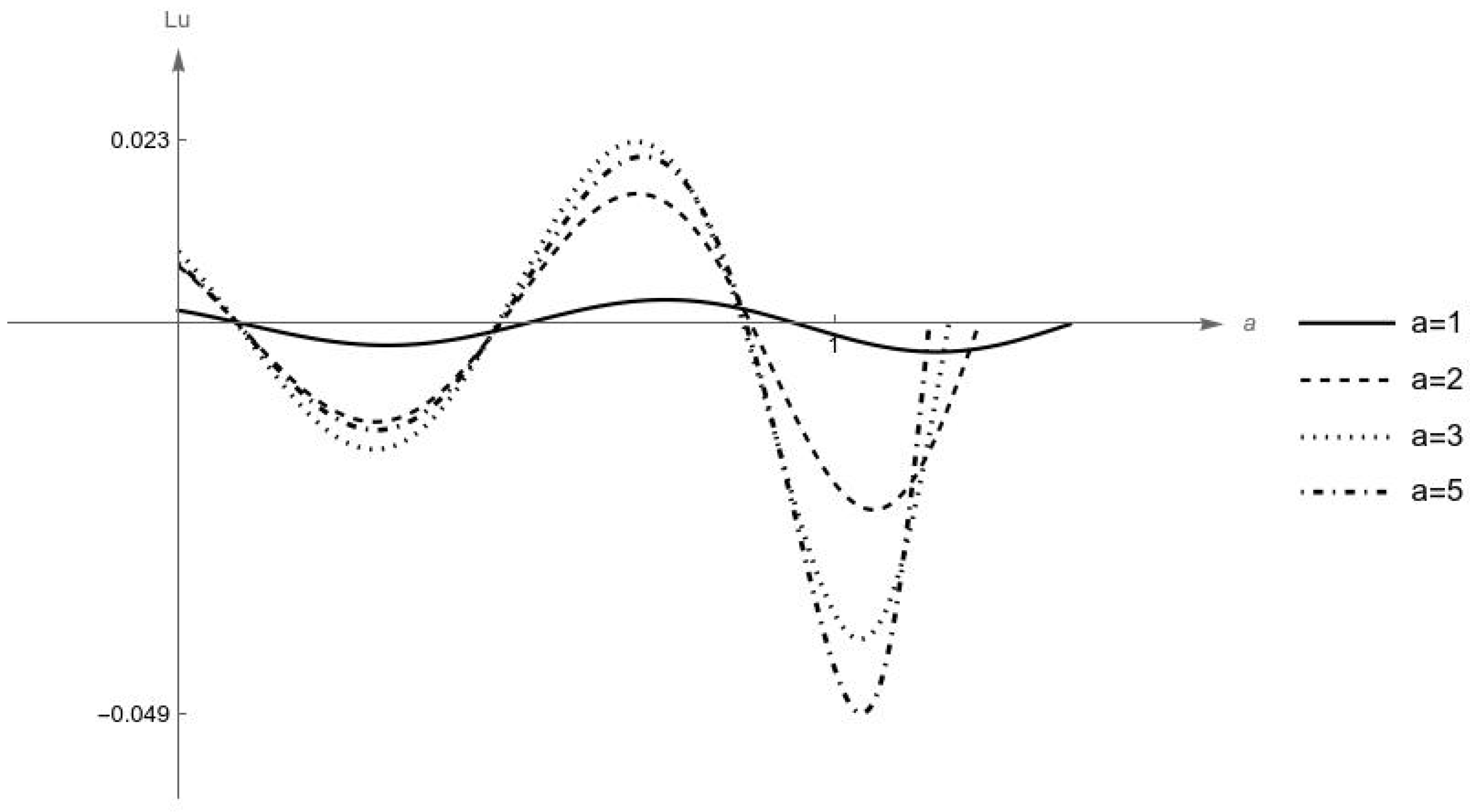
In a similar way,
Figure 5 plots the operator (
30) for the quinticate form of oscillator (
1)–(
3), again at initial displacements
As mentioned before, what is significant to observe in
Figure 4 and
Figure 5 is the deviation from zero. A small deviation indicates that the wave governed by the quinticate oscillator and the wave described by the original oscillator, although different, remain uniformly close to each other, and that both the quinticate and the original restoring forces cause the same physical effect. As expected, the behavior is worse initially due to the normalization of the integration interval, and improves with larger displacements. For any
however, the differential operator remains bounded above, approximately by the machine-precision value
This is confirmed by the computation of
the results of which are collected in
Table 1 and
Table 2, respectively, for the quinticate forms of oscillators (
1)–(
2) and (
1)–(
3), showing that the upper bound
is reached at
from where the norm of
decreases monotonically. As for
Figure 4 and
Figure 5, also in
Table 1 and
Table 2, the small deviation from zero has the physical significance that the quinticate and original waves are uniformly close to each other.
Another measure that we introduced to validate the obtained solution is the ratio between the original oscillator period (
6), computed via numerical quadrature, and the period of its quinticate form, obtained exactly from (
19). This ratio remains close to
and bounded above by a value smaller than
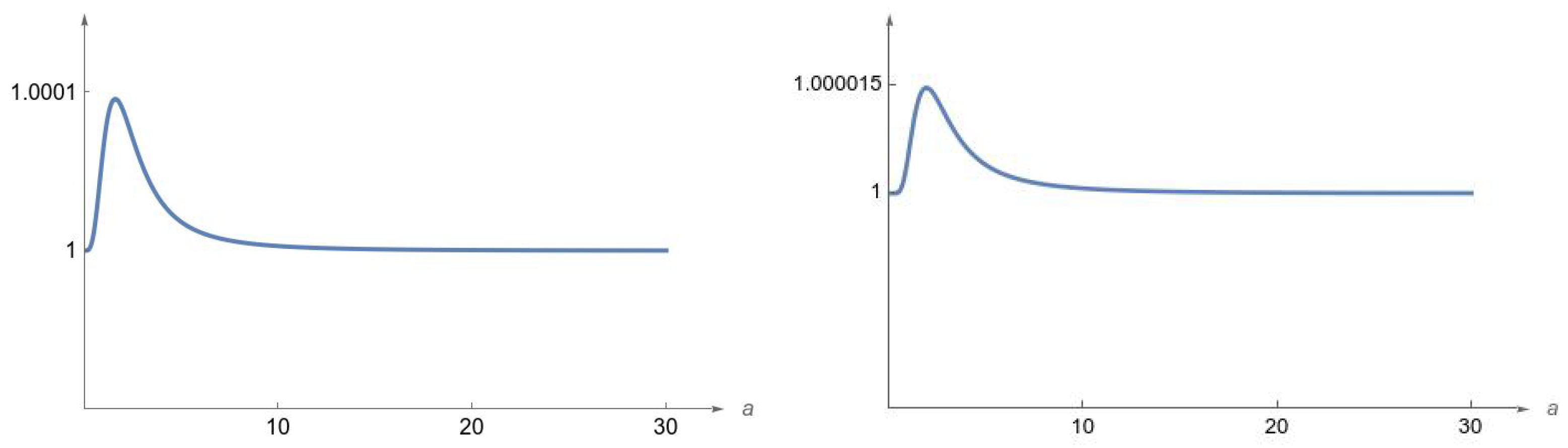
as illustrated in
Figure 6 for displacements up to
and for both oscillators investigated.
4. Pseudocode
For ease of reading and implementation, we provide here a concise description of the steps in our resolution procedure; the routines (in bold) mentioned are built-in functions available in Mathematica, version 14.0.
1– Choose the restoring force
namely, (
2) or (
3), and normalize it as in (
7) to obtain a function
of the sought solution
u and the displacement
2– Define the Chebyshev coefficients using (
9):
where
are computed exactly using Integrate, for example, and are functions of
3– Define the quinticate coefficients as functions of
using (
11):
4– Define the parabola
which is a function of
s and
as in (
13):
and compute its roots
exactly using
Solve, for example;
are functions of
5– Form the discriminant
as a function of
as shown in (
14):
and check that the conditions (
17) are met so that Theorem 1 can be applied. This may be performed exploiting
Reduce, for example, as well as the graphical capabilities of
Mathematica.
6– Define the exact period
of the quinticate oscillator, given by (
19)–(
20)–(
21), namely:
EllipticK provides the complete elliptic integral of the first kind
with the warning that it must be invoked as
that is to say, using the squared elliptic modulus
7– Define the exact solution
u of the quinticate oscillator as the square root of
given by (
18)–(
20)–(
21):
JacobiCN and
JacobiSN provide the Jacobi cosine amplitude and the Jacobi sine amplitude, which must be invoked using the squared elliptic modulus
8– Evaluate the normalized restoring force defined in step 1, at the quinticate solution u obtained in step 7.
9– Form namely, the second derivative of the quinticate solution u with respect to using D, for example. is a function of time t and the displacement
10– Form the differential operator
defined in (
30), evaluating
and
at various values of the displacement
a and for time
11– Define the period of the original oscillator as in (
6), taking into account normalization, that is to say:
where
is defined as in (
5), again taking into account normalization, and
is the normalized restoring force defined in step
1. Period
T is a function of
12– Form the period ratio as the ratio between period of step 6 and period T defined in step 11 and numerically evaluated with NIntegrate, for example, for various values of the displacement
5. Conclusions and Future Work
With this work, we extend our previous findings on modeling the dynamics of non–dissipative and nonlinear oscillators, where the restoring force stems from even-order potentials and is, therefore, an odd function. Our attention, in particular, is directed to wave configurations that have not been considered before in the relevant literature.
In the case of the Duffing-type dynamical model examined here, of which (
1)–(
2) and (
1)–(
3) represent two common instances, an exact description of the motion would require a closed-form inversion of the associated time-integral, which is not feasible.
This is, instead, possible for the quinticate form of the original oscillator, obtained by fifth-order truncation of the Chebyshev series of the restoring force.
The core of our exact solution method is the construction of a parabola, which appears in the time-integral equation of the quinticate oscillator as a function of its coefficients, and whose root configuration constitutes the hypotheses of Theorem 1: the latter is new and guarantees the closed-form description of the motion and period of the quinticate differential problem, in terms of elliptic integrals of the first kind, thus invertible in the Jacobi sense.
We validate our method by comparing the solutions of the quinticate model and the original one, the latter taken in normalized form. This is performed by studying the qualitative behavior of the differential operator (
30) and computing its uniform norm as a quantitative measure; the period ratio is also analyzed both qualitatively and quantitatively. All simulations performed confirm the efficiency of our new solution procedure.
Further work involves a generalization to truncations of order greater than five, which is currently being investigated and requires the inversion of hyperelliptic integrals, and related functionalities, that are under development although not yet available.