PSE-Based Aerodynamic Flow Transition Prediction Using Automated Unstructured CFD Integration

Abstract

The accurate, robust, and efficient prediction of transition in viscous flows is a significant challenge in computational fluid dynamics. We present a coupled high-fidelity iterative approach that leverages the FUN3D flow solver and the LASTRAC stability code to predict transition in low-disturbance environments, initiated by the linear growth of boundary-layer instability modes. Our method integrates the ability of FUN3D to compute mixed laminar–transitional–turbulent mean flows via transition-sensitized Reynolds-Averaged Navier–Stokes equations with the ability of LASTRAC to perform linear stability analysis, all within an automated framework that requires no intermediate user involvement. Unlike conventional frameworks that rely on classical stability theory or reduced-order metamodels, our approach employs parabolized stability equations to provide more accurate and reliable estimates of disturbance growth for multiple instability mechanisms, including Tollmien–Schlichting, Kelvin–Helmholtz, and crossflow modes. By accounting for the effects of mean-flow nonparallelism as well as the surface curvature, this approach lays the foundation for improved N-factor correlations for transition onset prediction in a broad class of flows. We apply this method to three distinct flow configurations: (1) flow over a zero-pressure-gradient flat plate, (2) the NLF-0416 airfoil with both natural and separation-induced transition, and (3) a 6:1 prolate spheroid, where transition is primarily driven by crossflow instability. For two-dimensional cases, a formulated intermittency distribution is used to model the transition zone between the laminar and fully turbulent flows. The results include comparisons with experimental measurements, similar numerical approaches, and transport-equation-based models, demonstrating good agreement in surface pressure coefficients, transition onset locations, and skin-friction coefficients for all three configurations. In addition to contributing a couple of new insights into boundary-layer transition in these canonical cases, this study presents a powerful tool for transition modeling in both research and design applications in aerodynamics.

1. Introduction

From the NASA Computational Fluid Dynamics (CFD) Vision 2030 Study [1], one of the most important fluid dynamics research areas that will remain a pacing item for the foreseeable future is the ability to accurately, robustly, and efficiently predict transition to turbulence in viscous flows. Laminar–turbulent boundary-layer transition has many applications to aerodynamic design that include unmanned aerial vehicles, natural laminar flow surfaces, and crewed re-entry vehicles [2]. Maximizing the laminar regions on aerodynamic surfaces by delaying or preventing the onset of transition substantially reduces drag, which increases the energy efficiency by reducing the fuel burn rate [3]. The energy efficiency of new and existing aircraft has to be significantly improved in order to reduce the negative impact that aviation has on the environment [4]. For high-speed flow on vehicle surfaces, transition to turbulence can result in a fivefold to eightfold increase in the skin-friction drag and heat flux. Uncertainties in transition prediction can have a critical impact on the safety, design, and performance of an aerospace vehicle, including weight, structure, and engine operability.

The common approaches to model and investigate boundary-layer transition are Direct Numerical Simulations (DNS), Reynolds-Averaged Navier–Stokes (RANS) equations, and Wall-Resolved Large-Eddy Simulations (WRLES). The use of DNS or WRLES to study transition requires substantial computational resources, especially if extended to high Reynolds numbers, where the required grid resolution is extremely fine. Moreover, these expensive simulations must have accurate initial and boundary conditions based on either wind-tunnel measurements or flight tests. RANS-based transition models that rely on various transport equations are less computationally expensive than DNS and WRLES but can be inaccurate for several reasons, including their inability to capture the process of laminar–turbulent boundary-layer transition, which involves complicated physics, including the receptivity, linear amplification, and nonlinear interactions and breakdown of instability waves. Furthermore, many RANS-based transition models are only accurate in the parameter space (or transition scenario) where they were originally calibrated, and, outside of this parameter space, i.e., very low freestream turbulence intensity values, very small Reynolds numbers, or very large Reynolds numbers, they do not provide good predictions, unless they are recalibrated or updated to fix certain discrepancies. A well-known example of this drawback is the 2009 version of the Langtry–Menter $\gamma$-$R{e}_{{\theta }_t}$ transition model [5] that does not behave as well at freestream turbulence intensity values below 0.1% as the correlation for $R{e}_{{\theta }_t}$ grows exponentially.

To improve the accuracy and robustness of RANS-based models, they can be paired with more physics-based linear stability analysis techniques through a modular approach that is conducted in this work since directly integrating stability analysis techniques into particular Navier–Stokes flow solvers can be difficult. Semiempirical correlation-based methods that rely on Linear Stability Theory (LST) are often employed to predict transition locations in various flow configurations. The $e^N$ method developed by Smith and Gamberoni [6] as well as van Ingen [7] is the most commonly used and cited in the literature. This method seeks to correlate the onset of transition with the location where the peak amplification factor reaches a critical value of $N_{\mathrm{crit}}$. Here, the value of $N_{\mathrm{crit}}$ is determined by experimental measurements. A well-known problem with the $e^N$ method is that receptivity and nonlinear breakdown cannot be modeled accurately with just a single value of $N_{\mathrm{crit}}$. Therefore, variable N-factor methods have been proposed by Mack [8] as well as by Crouch and Ng [9]. The Parabolized Stability Equations (PSE) [10] can also be employed to predict transition. These equations are less restrictive than LST because they do not require a parallel flow assumption. Both LST and PSE can account for the amplification of Tollmien–Schlichting (TS) waves, stationary/traveling crossflow vortices, and other types of instability mechanisms. However, the application of PSE provides a more accurate prediction of the disturbance growth characteristics by accounting for the effects of mean-flow nonparallelism as well as the surface curvature. These effects can be rather important in the context of laminar flow aircraft, as shown by Malik et al. [11].

There has been considerable past work on coupling simulation tools, primarily RANS transport-equation-based models, with various linear stability analysis methods [12,13,14,15,16,17], but they have a few shortcomings. First, the use of stability analysis includes an increased computational cost and complexity, requires a CFD user with knowledge of transition physics and hydrodynamic stability theory, and usually lacks robustness. Also, modern commercial CFD codes often rely on massive parallelization and unstructured grids, which are not compatible with the global dependence of the stability characteristics in one or more spatial directions. In some cases, less accurate surrogate models that utilize laminar flow field data are employed instead of direct calculations of the stability characteristics [18,19,20,21]. For those codes that do compute the stability characteristics directly, they are tightly coupled to specific data structures that are used within their CFD solver, which makes a number of algorithmic tasks, such as the extraction of boundary-layer profiles, difficult and costly in the context of a parallel computing framework. They also cannot be easily ported to other flow solvers. Automated initiation of PSE computations that leads to minimal initial transients is also a difficult task (refer to Kosarev et al. [22] and Halila et al. [17]), commonly resulting in the adoption of simpler approaches, such as classical LST, for predicting instability growth.

The present study implements a coupled iterative approach that uses a combination of RANS basic states computed with FUN3D, an unstructured finite-volume RANS solver [23], and local stability analysis performed with LASTRAC, a state-of-the-art LST and PSE solver [24], to model boundary-layer transition. A solver-independent module that couples FUN3D and LASTRAC automatically identifies the viscous surfaces for transition prediction, extracts wall-normal boundary-layer profiles from the CFD solution, and defines integration trajectories (typically in the form of streamlines) for stability analysis with no user involvement. This solver-independent module can be coupled to any flow solver or stability code, as outlined in Choudhari et al. [2]. We determine the locations of transition onset in the FUN3D basic states with LASTRAC stability calculations that involve computing N-factor envelopes with PSE in an iterative procedure. This approach is robust because we are taking into account the growth of several different instability waves. It also does not take long to converge the transition location (both in terms of computational time and number of iterations). Furthermore, it has the potential to leverage unique capabilities in the NASA FUN3D suite of codes, which include automated grid adaptation [25,26] and the ability to perform adjoint-based design [27,28]. A similar automated iterative transition-prediction approach that couples a structured overset-grid flow solver, i.e., OVERFLOW, and LASTRAC has been verified and validated in recent work by Venkatachari et al. [29].

The work presented here is an extension of Hildebrand et al. [30]. We apply our automated FUN3D–LASTRAC iterative approach to low-speed flow over a zero-pressure-gradient flat plate, the NLF-0416 airfoil at an angle of attack equal to 5 degrees, and the 6:1 prolate spheroid at an angle of attack equal to 24 degrees. Three different transition scenarios are investigated. Natural transition occurs for the flat-plate case, both natural and separation-bubble-induced transition happen for the NLF-0416 airfoil, and crossflow instabilities primarily cause transition away from the windward and leeward planes of symmetry on the 6:1 prolate spheroid. Comparisons are conducted between results from our approach, experimental measurements, transport-equation-based models, and results from similar numerical iterative transition-prediction approaches. This paper is structured in the following manner. Section 2 describes the Spalart–Allmaras (SA) model with and without imposed transition in FUN3D, the linear stability analysis methods in LASTRAC, and the solver-independent module. The transitional results from the flat-plate, airfoil, and spheroid cases are presented in Section 3. We conclude the paper in Section 4.

2. Methodology

The proposed methodology seeks to predict boundary-layer transition over both 2D and 3D flow configurations. Practical configurations often require unstructured mesh computations. To that end, we describe an implementation that employs the FUN3D suite of codes [23,31] to compute the RANS transitional basic states, the LASTRAC suite for stability analysis [24], and the solver-independent module written in Python 3.4.3/C++11 to convert the basic states to wall-normal boundary-layer profiles and compute the streamlines for spatially marching linear stability analysis. Our iterative FUN3D–LASTRAC approach is more computationally expensive than solely using a RANS-based transition model, e.g., the Langtry–Menter (LM) $\gamma$-${\mathrm{Re}}_{{\theta }_t}$ model [5], but it provides a better physics-based representation because it captures several different types of instability modes. It is also considerably less expensive than DNS or LES. The different parts of our approach (i.e., basic state computations, stability analysis, and the solver-independent module) are discussed in the following three subsections.

2.1. FUN3D Basic States

To compute the basic states, we utilize the suite of codes known as FUN3D, which is an implicit node-centered unstructured-grid upwind-biased flow solver [23]. FUN3D has the ability to compute a laminar, transitional, or fully turbulent basic state. Several different RANS-based turbulence and transition models can be run with the NASA FUN3D CFD solver, including the SA turbulence model [32], the LM transition model [5], and Coder’s Amplification Factor Transport (AFT) transition model [14]. The inviscid fluxes are computed at edge medians with Roe’s scheme [33]. Second-order accuracy is achieved via MUSCL reconstruction with unweighted least-squares nodal gradients. The viscous fluxes are computed with Green–Gauss element-based gradients at dual faces. A point implicit procedure is used to solve the linear system of equations at each time step. Each of the FUN3D simulations are run until the residuals have reached machine zero and leveled out. For more information on the various numerical methods in FUN3D, see the current version of the manual [31].

In this work, the SA one-equation turbulence model [32] with and without imposed transition is solved for the 2D and 3D basic states of low-speed flow over a flat plate, the NLF-0416 airfoil, and the 6:1 prolate spheroid. This particular RANS model is given by the following expression

$$\begin{array}{c}\hfill {\displaystyle \frac{\partial \widehat{\nu }}{\partial t}}+u_j{\displaystyle \frac{\partial \widehat{\nu }}{\partial x_j}}=\gamma c_{b1}(1-f_{t2})\widehat{S}\widehat{\nu }-\left(c_{w1}{f}_w-{\displaystyle \frac{c_{b1}}{{\kappa }^2}}{f}_{t2}\right){\left({\displaystyle \frac{\widehat{\nu }}{d}}\right)}^2\\ \hfill +{\displaystyle \frac{1}{\sigma }}\left\{{\displaystyle \frac{\partial }{\partial x_j}}\left[(\nu +\widehat{\nu }){\displaystyle \frac{\partial \widehat{\nu }}{\partial x_j}}\right]+c_{b2}{\left({\displaystyle \frac{\partial \widehat{\nu }}{\partial x_i}}\right)}^2\right\},\end{array}$$

(1)

where ${\mu }_t=\rho \widehat{\nu }{f}_{v1}$ is the turbulent eddy viscosity, $f_{v1}={\chi }^3/({\chi }^3+c_{v1}^3)$, $\chi =\widehat{\nu }/\nu$, $\nu =\mu /\rho$ is the molecular kinematic viscosity, $\widehat{S}=\mathsf{\Omega }+\widehat{\nu }{f}_{v2}/{\kappa }^2{d}^2$, $\mathsf{\Omega }={\left(2W_{ij}{W}_{ij}\right)}^{1/2}$ is the vorticity magnitude, d is the nearest wall distance, $f_{v2}=1-\chi /(1+\chi f_{v1})$, $f_w=g{[(1+c_{w3}^6)/(g^6+c_{w3}^6)]}^{1/6}$, $g=r+c_{w2}(r^6-r)$, $r=\min [\widehat{\nu }/\left(\widehat{S}{\kappa }^2{d}^2\right),10]$, $f_{t2}=c_{t3}\exp (-c_{t4}{\chi }^2)$, and $\gamma$ is the intermittency function. The boundary conditions are $\widehat{\nu }=0$ at the wall and $\widehat{\nu }=3{\nu }_{\infty }$ to $\widehat{\nu }=5{\nu }_{\infty }$ at the farfield. For the different transitional cases in this paper, we modify the boundary conditions in the farfield such that $\widehat{\nu }=0.1{\nu }_{\infty }$. This lower value helps to avoid contamination of the laminar boundary layers. Also, the constants are $c_{b1}=0.1355$, $\sigma =2/3$, $c_{b2}=0.622$, $\kappa =0.41$, $c_{w2}=0.3$, $c_{w3}=2$, $c_{v1}=7.1$, $c_{t3}=1.2$, $c_{t4}=0.5$, and $c_{w1}=c_{b1}/{\kappa }^2+(1+c_{b2})/\sigma$. For a more complete list of the constants, function definitions, boundary conditions, and limiters, see Ref. [32] or the NASA Turbulence Modeling Resource (https://turbmodels.larc.nasa.gov/, accessed on 20 March 2025).

The specification of transition to turbulence along a viscous boundary is possible in the FUN3D suite of codes. For the 2D basic states, an intermittency function is used that multiplies the production terms of any turbulence model equations. This intermittency function is defined as

$$\gamma (x,x_{tr})=\left\{\begin{array}{cc}1-\exp \left(-0.413{\left[{\displaystyle \frac{3.36(x-x_{tr})}{l_{tr}\left(x_{tr}\right)}}\right]}^2\right)\hfill & \mathrm{if}x\ge x_{tr}\hfill \\ 0\hfill & \mathrm{if}x<x_{tr},\hfill \end{array}\right.$$

(2)

which is taken from Dhawan and Narasimha [34]. Here, $x_{tr}$ represents the location of transition onset, and $l_{tr}$ corresponds to the transition length. For separation-induced transition, $l_{tr}=700{\left({\mathrm{Re}}_{\infty }\right)}^{-0.3}{\left({\delta }_2\right)}^{0.7}$ and ${\delta }_2=0.665\sqrt{x_{tr}/{\mathrm{Re}}_{\infty }}$ following Krumbein [35]. In the case of TS waves, $l_{tr}=2.3\sqrt{{\mathrm{Re}}_{\infty }}{\left({\delta }_1\right)}^{1.5}$ and ${\delta }_1=1.72\sqrt{x_{tr}/{\mathrm{Re}}_{\infty }}$ from Dhawan and Narasimha [34]. Note that Re_∞ is the freestream unit Reynolds number, ${\delta }_1$ is the displacement thickness, and ${\delta }_2$ is the momentum thickness.

2.2. LASTRAC Stability Analysis

In the LASTRAC stability analysis tool package [24], both LST and PSE can be run for 2D and 3D RANS basic states. The linear disturbance amplification is computed with PSE from LASTRAC [36,37] in this work. These perturbations can be assumed to be harmonic in the spanwise direction, z, and in time, t, such that

$$\tilde{\mathbf{q}}(x,y,z,t)=\breve{\mathbf{q}}(x,y)exp\left[\mathrm{i}\left(\beta z-\omega t\right)\right]+\mathrm{c}.\mathrm{c}.,$$

(3)

where $\mathrm{c}.\mathrm{c}.$ denotes the complex conjugate, $\beta$ is the spanwise wavenumber, and $\omega$ is the angular frequency. Here, $\tilde{\mathbf{q}}(x,y,z,t)={(\tilde{\rho },\tilde{u},\tilde{v},\tilde{w},\tilde{T})}^{\mathrm{T}}$ and $\breve{\mathbf{q}}(x,y)={(\breve{\rho },\breve{u},\breve{v},\breve{w},\breve{T})}^{\mathrm{T}}$. The variables $(x,y,z)$ are the streamwise, wall-normal, and spanwise coordinates, respectively, with the variables $(u,v,w)$ representing the velocity components in those coordinate directions. We employ PSE instead of LST in this work because it accounts for the streamwise variation in the boundary-layer thickness and shape of the disturbance field. PSE solves an approximate form of the Navier–Stokes equations for a slowly varying shape function and a complex streamwise wavenumber $\alpha \left(x\right)$ such that

$$\breve{\mathbf{q}}(x,y)=\widehat{\mathbf{q}}(x,y)exp\left[\mathrm{i}{\int }_{x_0}^x\alpha \left(x^{\prime }\right)\mathrm{d}{x}^{\prime }\right].$$

(4)

According to linear stability analysis, transition occurs at the streamwise location where the N-factor value of any fixed-frequency/fixed-wavelength disturbance first reaches a critical value of $N_{\mathrm{crit}}$. The N-factor is defined as

$$N\left(x\right)={\displaystyle \frac{1}{2}}ln\left[{\displaystyle \frac{K\left(x\right)}{K\left(x_{\mathrm{lb}}\right)}}\right],$$

(5)

where $x_{\mathrm{lb}}$ denotes the lower-branch location where the disturbance at any given frequency and wavelength combination first becomes unstable, and the kinetic-energy norm K is

$$K\left(x\right)={\int }_y{(\breve{u},\breve{v},\breve{w})}^{\mathrm{H}}\mathrm{diag}\left[\overline{\rho }(x,y),\overline{\rho }(x,y),\overline{\rho }(x,y)\right](\breve{u},\breve{v},\breve{w})\mathrm{d}y.$$

(6)

The superscript $\mathrm{H}$ denotes the Hermitian operator. To acquire the N-factor envelope over all selected disturbances, we calculate the maximum N-factor at each streamwise station. The location of transition onset is where the N-factor envelope first reaches $N_{\mathrm{crit}}$. In general, the value of $N_{\mathrm{crit}}$ can be specified as part of the user input. However, if we know the measured turbulent intensity $Tu$, we can use the expression $N_{\mathrm{crit}}=-8.43-2.4\ln \left(Tu\right)$ from Mack [8] for TS waves in 2D flows. The quantity $Tu$ can be obtained from experimental measurements or workshops. For more complicated 3D flows that contain both TS waves and crossflow instability, the expression $N_{\mathrm{crit}}=N_{\mathrm{TS}}+N_{\mathrm{CF}}=8$ is used to determine a transition front. The requirement of prescribing the $N_{crit}$ value forms a limitation of the N-factor methods as described by Choudhari et al. [2]; however, that requirement simply represents the sensitivity of the transition process to the external disturbance environment.

2.3. Solver-Independent Module and Iterative Approach

A diagram of the automated iterative coupling approach for transition prediction is displayed in Figure 1. It consists of the FUN3D v14.0.2 and LASTRAC v3.0 software packages that both can be run in parallel. The solver-independent module listed in Figure 1 is designed to couple any CFD simulation code with any stability analysis tool. Specifically, the solver-independent module in this work couples FUN3D with LASTRAC. The solver-independent module is written in Python/C++ and can also be run in parallel. It reads the grid and output solution data from the flow solvers (i.e., FUN3D and LASTRAC) in ASCII or binary format, extracts wall-normal boundary-layer profiles from the CFD solution, identifies viscous surfaces for transition prediction, forms integration trajectories or streamlines, and determines the stopping criteria. The iterative procedure in this work begins with loading the required FUN3D, LASTRAC, and Python/C++ modules. Next, a Python code generates the FUN3D input files, i.e., the name list and the job script, gathers the other required files, e.g., the computational grid and the boundary conditions, and runs the simulation in parallel. Once the FUN3D simulation has finished, the solver-independent module reads in the FUN3D solution from a binary volume file, extracts the boundary-layer profiles, identifies the viscous surfaces, and forms the integration trajectories for stability analysis. Afterward, the solver-independent module uses all this information to write a binary file of the mean flow that LASTRAC is able to read. Next, a Python code generates the LASTRAC input files and runs the stability analysis either in parallel or with multithreading. The stability analysis computations require a significant amount of memory. Once the stability analysis has finished, the N-factor values are interpolated onto the surface grid. Afterward, the solver-independent module reads in the N-factor distribution and determines the next transition location. This location of transition onset is enforced in the new FUN3D simulation as part of the next iteration.

We determine the location of transition onset from the fixed value of $N_{\mathrm{crit}}$ and the N-factor distribution. An under-relaxation expression is used to improve the iterative convergence and is given by the following expression

$$x_{tr,1}=x_{tr,0}+\theta (x_{\mathrm{crit}}-x_{tr,0}),$$

(7)

where the factor $\theta$ is a specified value between 0.7 and 1.0, $x_{\mathrm{crit}}$ is where $N_{\mathrm{crit}}$ intersects the N-factor envelope, $x_{tr,0}$ is the previous transition location, and $x_{tr,1}$ is the new transition location. If the new location of transition onset, intermittency field, or 3D transition front is within a given tolerance of the same quantity for the previous iteration, our automated iterative method terminates; otherwise, it continues to iterate by setting up and running the next FUN3D simulation. If a steady RANS basic state does not fully converge to machine zero due to strong unsteadiness, nonlinearities, or a poor initial approximation of a transition location/front (usually too far downstream), then stability analysis is still applied to it, and, if the appropriate unstable mode is not found, then our automated coupled approach will shift the corresponding transition location/front upstream. On the other hand, if a transition location/front is too far upstream, producing a very small region of laminar flow, and the critical N-factor value is not reached from the spatial growth of the appropriate unstable mode, then our automated coupled approach will extrapolate the spatial growth of that unstable mode downstream into the fully turbulent region to provide a best estimate of the next transition location/front downstream.

For the computations of TS waves along streamlines, the wave angle is set to zero because the most unstable TS waves in low-speed flows are usually assumed to follow the inviscid streamline for any given disturbance frequency. In terms of stationary crossflow disturbances, a nonorthogonal coordinate is utilized at each point along the streamline, where the x coordinate is along the streamline direction and the spanwise or z coordinate is along the azimuthal grid line direction. An azimuthal wavenumber is imposed for axisymmetric bodies such as the 6:1 prolate spheroid. PSE N-factor integration is carried out for each frequency. The N-factor envelope across various disturbance frequencies as a function of the arc length along each streamline is used to uniquely determine the N-factor value at each streamline point. We linearly interpolate the N-factor values across streamlines to the entire viscous surface in order to determine transition locations based on a predetermined N-factor criterion.

3. Results

To demonstrate and evaluate our automated FUN3D–LASTRAC coupling approach, we examine three different canonical flow configurations: a zero-pressure-gradient flat plate, the NLF-0416 airfoil, and the 6:1 prolate spheroid. Collectively, these cases feature transition due to TS waves, separation bubbles, and crossflow instability. The computational predictions for these cases are evaluated via comparisons with experimental measurements as well as other computational predictions, including transport-equation-based models and results from similar iterative approaches for transition prediction.

3.1. Zero-Pressure-Gradient Flat Plate

The first case we consider is low-speed flow over a two-dimensional zero-pressure-gradient flat plate with a sharp leading edge. This flat plate starts at $x=0$ m and extends to $x=2.5$ m. We select the flow conditions to match those from experiments by Schubauer and Skramstad [38]. The freestream density, velocity, and temperature are ${\rho }_{\infty }=1.2$ kg/m³, $U_{\infty }=50.1$ m/s, and $T_{\infty }=288.17$ K, respectively. This yields a freestream unit Reynolds number of $R{e}_{\infty }=3.36\times {10}^6$ $(1/$m) and Mach number of $M=0.147$. We assume a perfect gas such that $p=\rho RT$, where $R=287.15$ J/K/kg is the specific gas constant for air. Here, $\gamma =1.4$ is the constant ratio of specific heats. The Prandtl number is set to a constant value of $\mathrm{Pr}=0.72$. To compute the dynamic viscosity $\mu$, we use Sutherland’s law $\mu =c_1{T}^{1.5}/(T+T_s)$ with $c_1=1.458\times {10}^{-6}$ kg/(ms$·\sqrt{\mathrm{K}}$) and $T_s=110.4$ K. A freestream turbulence intensity of $Tu=0.18\%$ is used for this case. 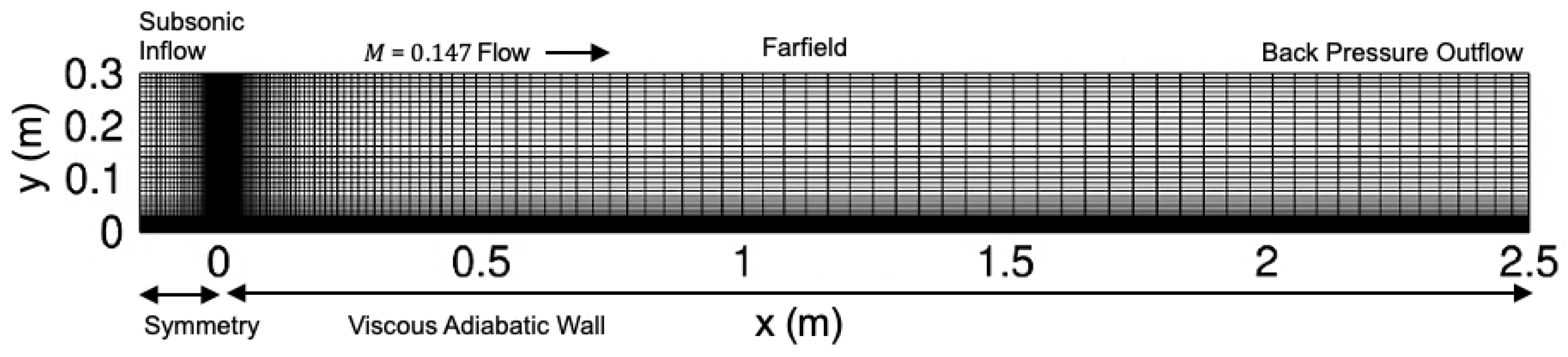The left edge of the computational domain at $x=-0.15$ m is a subsonic inflow, and the right edge is a back-pressure outflow. Furthermore, the flat plate has adiabatic and no-slip boundary conditions, and the small portion before the flat plate is set to a wall-normal-symmetry boundary condition. A farfield boundary condition is used at the top edge of the domain at $z=0.3$ m.

We use a computational grid with 961 and 513 points in the streamwise and wall-normal directions, respectively. The 961 points also include the 257 points upstream of the leading edge of the flat plate. We place the first grid point above the flat plate at a distance of $3\times {10}^{-6}$ m, which satisfies $\Delta y^{+}\le 0.25$ regardless of the boundary-layer state, and the wall-normal grid spacing increases gradually from there to the freestream. This computational grid is designed to have more than 150 points within the boundary layer. Furthermore, the streamwise grid spacing is the smallest near the leading edge of the flat plate at $x=0$ m. This exact computational grid is depicted as level 6 herein, and others in the family were used in previous work by Venkatachari et al. [39]. For grids in this family, the resolution doubles between consecutive even or odd levels. Grid level 7 has 1441 and 769 points in the streamwise and wall-normal directions, respectively, while grid level 8 has 1921 and 1025 points. 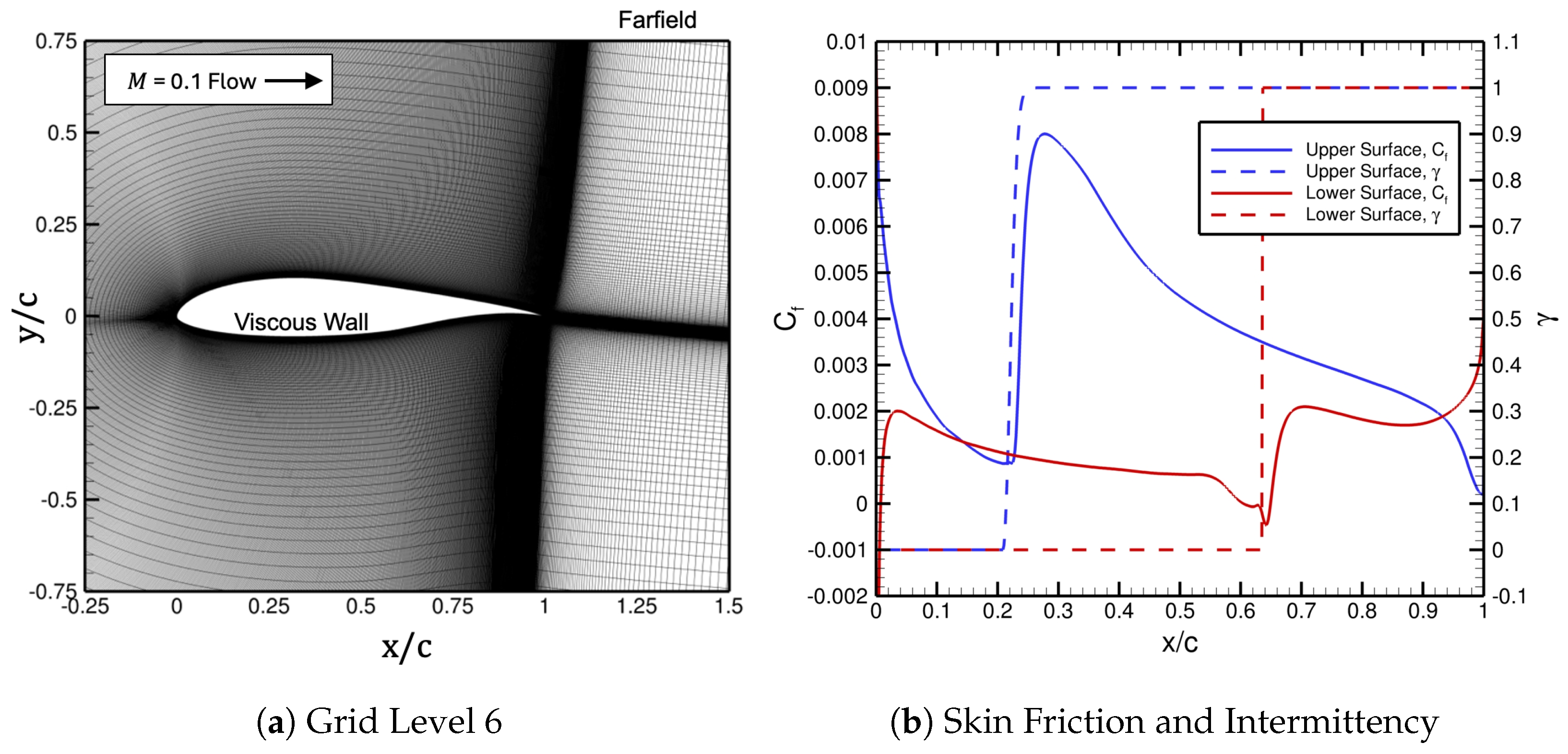Figure 2 displays grid level 1 in this family and the corresponding boundary conditions.

Figure 3 displays the streamwise distributions of the skin-friction coefficient and the intermittency along the plate for iterations 1 and 10 of the automated coupling approach with FUN3D and LASTRAC pertaining to grid level 6. There is a significant difference between the results from iterations 1 and 10 after transition occurs, but, upstream in the laminar portion, the solutions are the exact same. Moreover, the intermittency varies smoothly from 0 to 1 in the region of transition as the skin-friction coefficient also rises from approximately $4\times {10}^{-4}$ to $3.4\times {10}^{-3}$. A closeup view of both the skin-friction coefficient and the intermittency near transition to turbulence are shown in Figure 3 on the right. As indicated in Figure 3, the intermittency function helps the flow to shift from laminar to fully turbulent over a finite streamwise extent (instead of happening very abruptly) to better match the physics and the experiments. Although not shown, it also improves the iterative convergence of the automated FUN3D–LASTRAC transition-prediction method.

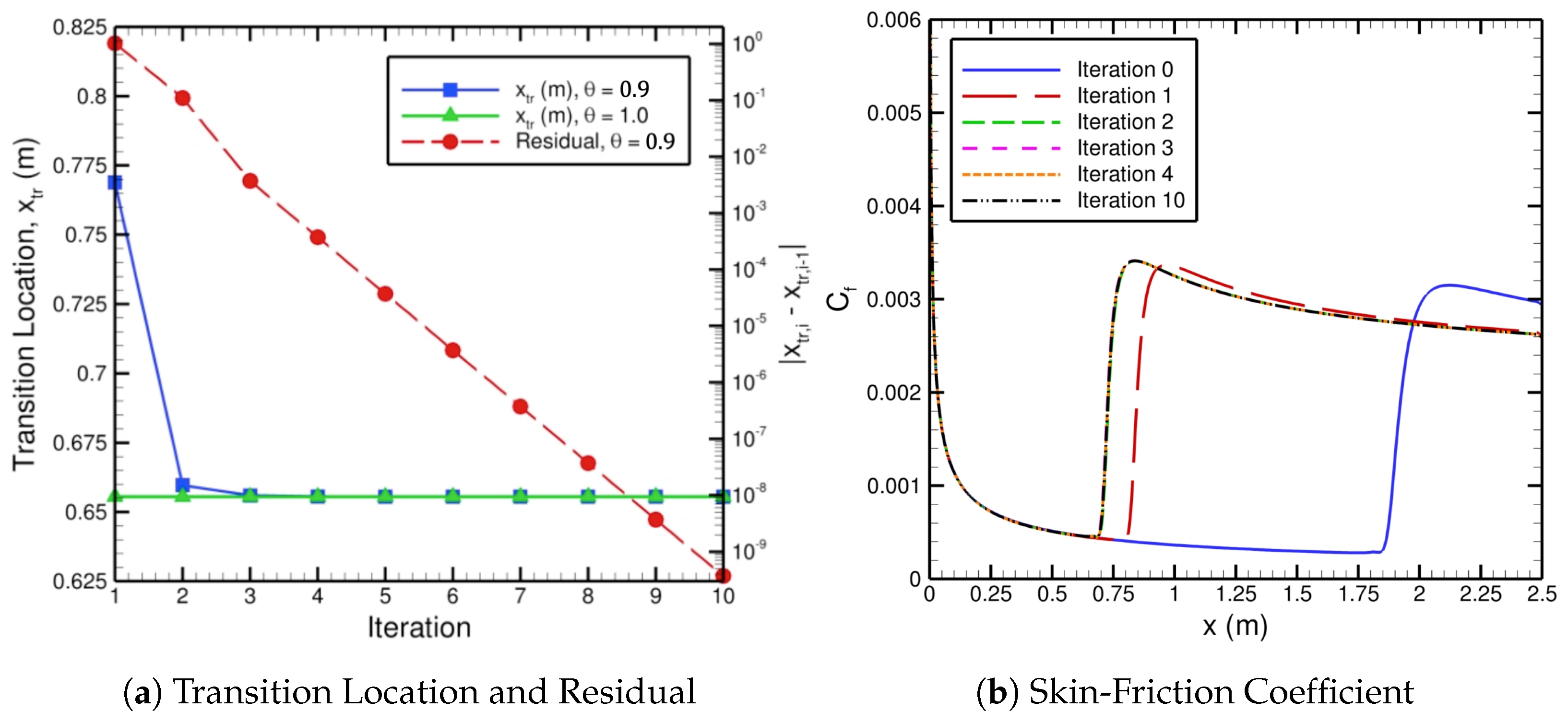
The iterative convergence of the imposed transition location with the corresponding residual and the skin-friction coefficient are displayed in Figure 4. For the initial basic state, i.e., the zeroth iteration, we imposed transition far downstream at $x=1.8$ m. The skin-friction distribution from the initial basic state is shown in Figure 4b. Once the initial FUN3D basic state has been obtained, it is converted by the solver-independent module to a format that LASTRAC is able to read. 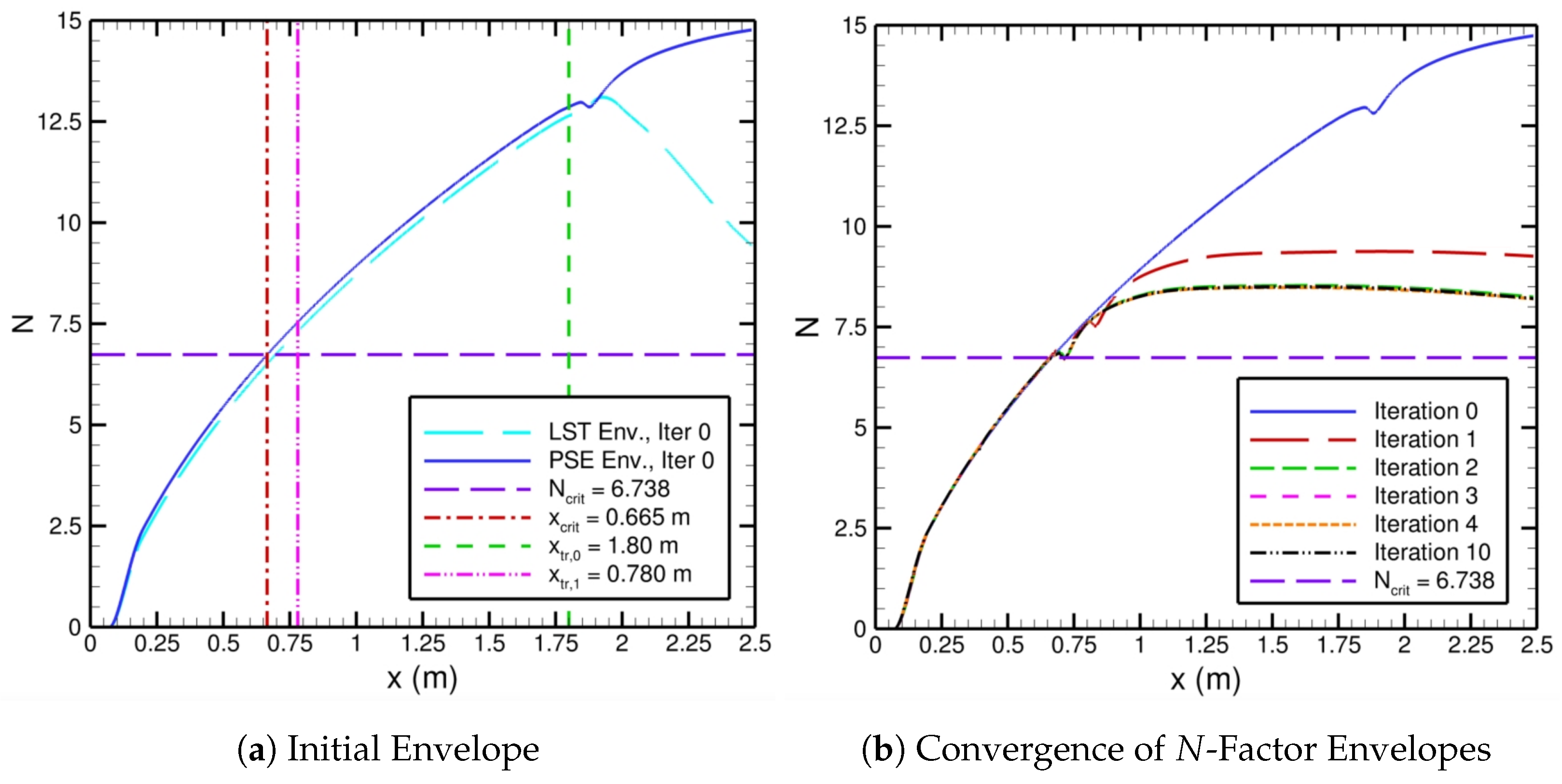That mean flow file is used to determine the N-factor envelope based on PSE computations. The N-factor envelope of the initial basic state is depicted in Figure 5, along with the N-factor envelopes pertaining to iterations 1 through 10. The value of $N_{\mathrm{crit}}=6.738$ in Figure 5 comes from the expression $N_{\mathrm{crit}}=-8.43-2.4\ln \left(Tu\right)$ via Mack [8] for TS waves in 2D flows with $Tu=0.18\%$. Note that Mack’s correlation of $N_{\mathrm{crit}}$ is based on LST results, but, since nonparallel effects for the flat plate are rather small, the same expression may be used for the PSE as well. The location where $N_{\mathrm{crit}}$ crosses the N-factor envelope yields $x_{\mathrm{crit}}$, which, in turn, is used to determine the next transition location, $x_{\mathrm{tr}},1$, with Equation (7) using $x_{\mathrm{tr}},0$ and an appropriate under-relaxation factor. This results in the values of $x_{\mathrm{crit}}=0.665$ m and $x_{\mathrm{tr}},1=0.78$ m in Figure 5 with $x_{\mathrm{tr}},0=1.8$ m and $\theta =0.9$ for iteration 0. The new transition location, $x_{\mathrm{tr}},1=0.78$ m, is prescribed in the next FUN3D basic-state computation pertaining to iteration 1. As the iteration number increases from 0 to 10, the N-factor envelopes converge in Figure 5b, and the skin-friction distributions converge in Figure 4b. The resulting change in the location of transition onset from each iteration decreases exponentially, or in a straight line on a logarithmic scale, to machine zero according to Figure 4a. Moreover, if the under-relaxation factor is set to unity (i.e., zero under-relaxation), then after just one iteration the location of transition onset converges for this flat-plate case. This means the flow does not have significant viscous–inviscid interactions and is not overly sensitive to changes in the location of transition onset. For cases with stronger viscous–inviscid interactions, under-relaxation is necessary to achieve robust convergence, especially during the early iterations, but can be increased afterwards to reduce the number of overall iterations. Similar iterative convergence of the automated FUN3D–LASTRAC method is obtained for different initially imposed transition locations of the initial basic state from $x=0.25$ m to $x=2.25$ m. If the imposed transition location is considerably upstream of the final converged transition location, then extrapolation is used to predict the next imposed transition location.

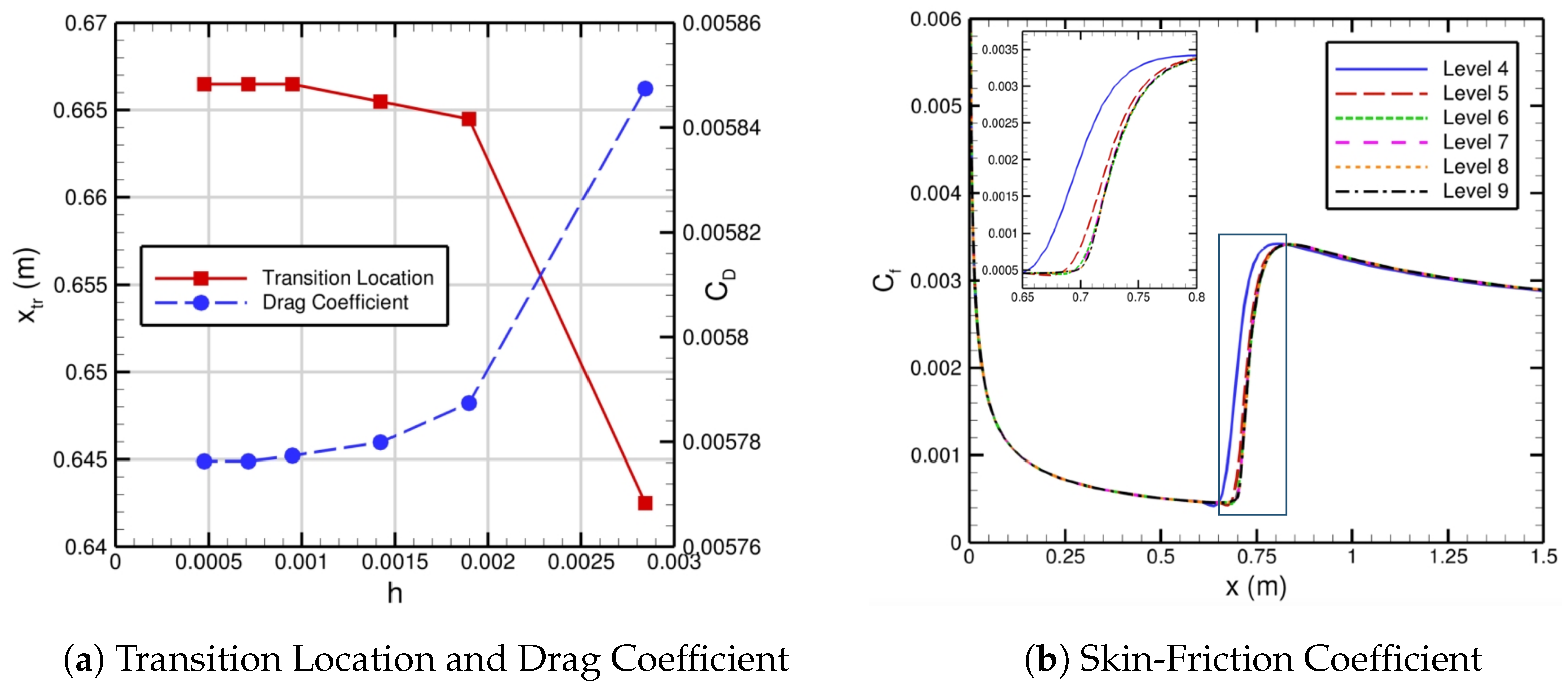
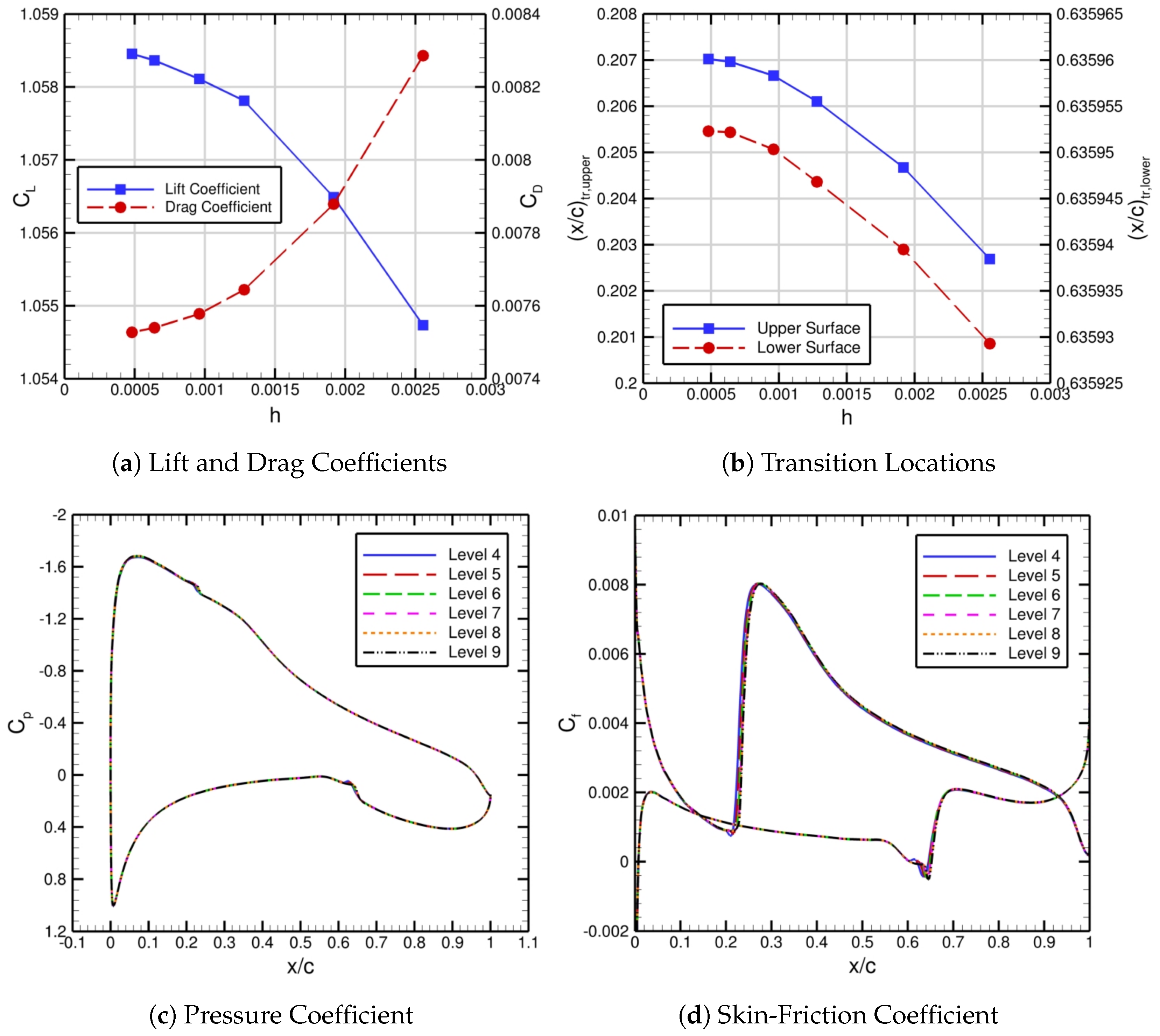
After showing iterative convergence of the automated FUN3D–LASTRAC transition-prediction method for the zero-pressure-gradient flat plate, we focus on grid convergence for the same case. Figure 6 shows the grid convergence of the drag coefficient, transition location, and skin-friction coefficient for this flat-plate case across levels 4 through 9. The level 4 grid has 123,616 points, and the level 9 grid has 4,428,097 points, where the resolution doubles along each spatial direction every other grid level. In Figure 6a, both the drag coefficient and transition location plateau for levels 8 and 9 as the grid spacing parameter $h=1/\sqrt{n_x^2+n_z^2}$ approaches zero. The skin-friction distribution also converges as the resolution increases (or the grid spacing decreases) in Figure 6b. Hence, our automated FUN3D–LASTRAC transition-prediction method converges with respect to both the iteration number and grid resolution.

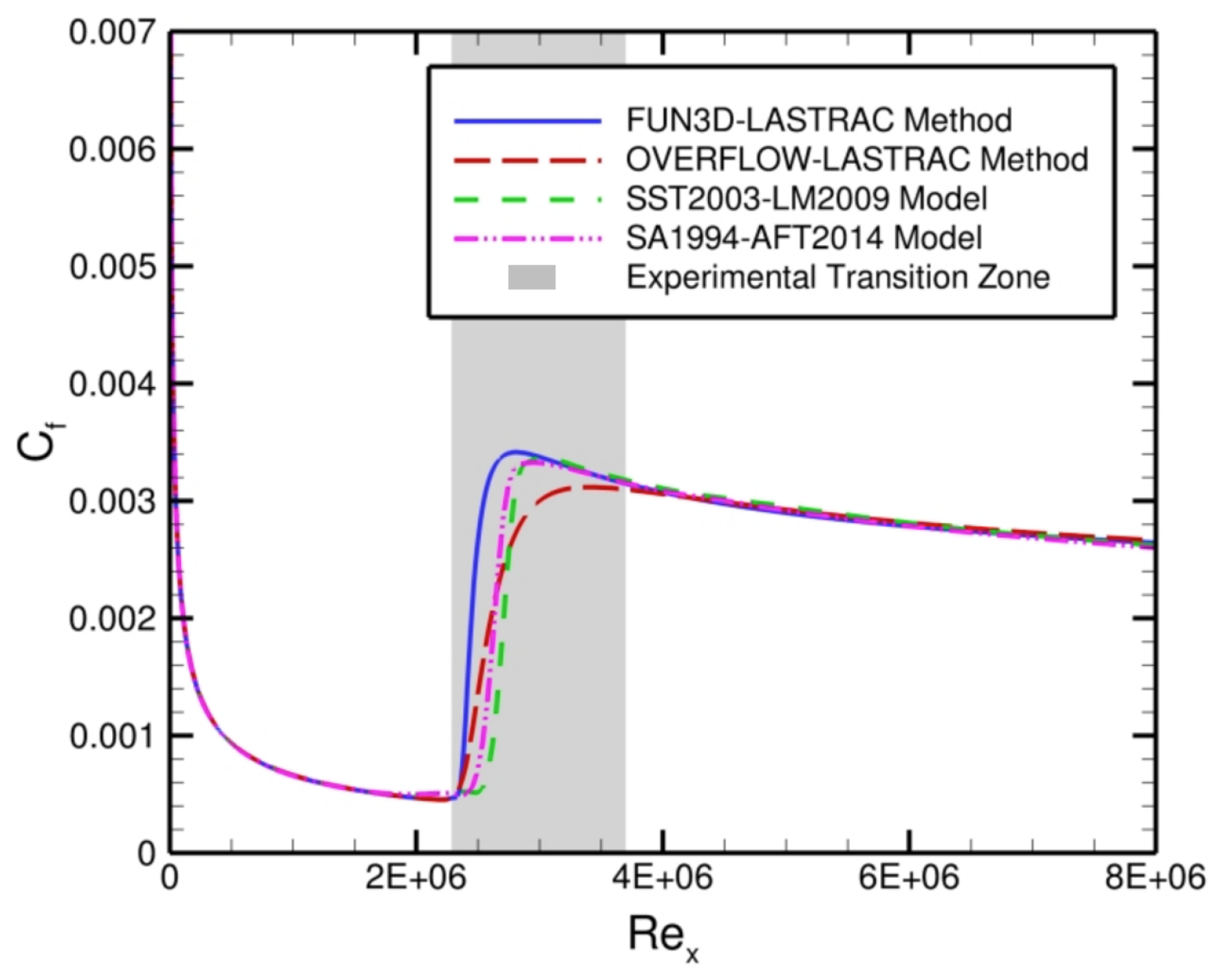
To validate our automated FUN3D–LASTRAC transition-prediction method, we compare the skin-friction distributions between our method, the OVERFLOW–LASTRAC method [29], the SST2003-LM2009 model [5], and the SA1994-AFT2014 model [14] in Figure 7. The experimentally observed transition zone [38] is also included as part of Figure 7 to validate our method. All the different computational skin-friction distributions begin to quickly rise in the experimentally observed transition zone [38], which indicates good agreement between the computations and experiments. The relative agreement between the skin-friction distributions computed with the FUN3D–LASTRAC and OVERFLOW–LASTRAC methods indicates that transition occurs at the exact same value of $R{e}_x$ (the laminar and turbulent skin-friction coefficients also match); the only deviation is limited to the intermittent region between laminar and fully turbulent flow because the coupling methods use different intermittency functions. There is also relatively good agreement between the RANS-based transition models (i.e., SST2003-LM2009 and SA1994-AFT2014) and the automated FUN3D–LASTRAC transition-prediction method in terms of the skin-friction coefficient and the location of transition onset. These successful comparisons provide verification and validation of the FUN3D–LASTRAC approach for modeling transition for this 2D zero-pressure-gradient flat-plate case.

3.2. NLF-0416 Airfoil

To help validate the automated FUN3D–LASTRAC iterative method for strong viscous–inviscid interaction and transition due to a separation bubble, we consider the NLF-0416 airfoil that is a natural-laminar-flow configuration developed at the NASA Langley Research Center by Somers [40]. This particular airfoil is designed to achieve a large value of the maximum lift coefficient and a low value of the cruise drag coefficient. An angle of attack equal to 5 degrees is considered, which leads to natural boundary-layer transition in a fully attached boundary layer over the upper surface and separation-bubble-induced transition across the lower surface. A moderate Reynolds number based on the chord length is prescribed, ${\mathrm{Re}}_c=4\times {10}^6$, and the freestream Mach number is $M_{\infty }=0.1$. The freestream density, velocity, and temperature are ${\rho }_{\infty }=2.124$ kg/m³, $U_{\infty }=34.7$ m/s, and $T_{\infty }=300$ K, respectively. A freestream turbulence intensity of $Tu=0.15\%$ is used based on the benchmark problem from the 1st AIAA Transition Modeling Workshop (https://transitionmodeling.larc.nasa.gov/workshop_i/, accessed on 20 March 2025). The farfield boundaries are placed 1000 chord lengths away from the airfoil surface. Freestream boundary conditions are enforced at the left, right, top, and bottom edges of the computational domain. Adiabatic and no-slip boundary conditions are employed at the airfoil surface. This case is significantly more challenging than the flat plate because multiple applications of stability analysis for the basic states are required to capture the strong influence of the recirculation bubble on transition due to an interaction that occurs between the separation-reattachment characteristics and the location of transition onset.

We use a C-type mesh generated with conformal mapping for the NLF-0416 airfoil. The coarser levels of this mesh are based on the grids provided by the 1st AIAA Transition Modeling Workshop. Here, the level 6 grid has 2113 and 289 points along the chordwise and wall-normal directions, respectively. There are 289 points in the wake cut of the grid. It has a near-wall spacing of $1\times {10}^{-6}$ based on the chord length that yields a nondimensional wall spacing of approximately $\Delta y^{+}=0.15$ based on turbulent flat-plate estimates at the quarter-chord location. Furthermore, this exact computational grid (level 6) and others in the family were used in the previous work by Venkatachari et al. [39]. For grids in this family, the resolution doubles with every other level. Grid level 8 has 4225 and 577 points in the chordwise and wall-normal directions, respectively. Figure 8a displays grid level 6 in this family and the corresponding boundary conditions. There is a clustering of grid points near the airfoil surface and in the wake region.

The streamwise distribution of the skin-friction coefficient and the intermittency along the upper and lower surfaces of the NLF-04146 airfoil for the final iteration of the automated FUN3D–LASTRAC method computed on grid level 6 are shown in Figure 8b. The intermittency increases smoothly from 0 to 1 in the regions of boundary-layer transition occurring on the upper and lower surfaces of the NLF-0416 airfoil, while the skin friction also rises sharply in these regions. For the lower airfoil surface, there is negative skin friction within a separation bubble that causes transition, while the boundary layer on the upper airfoil surface undergoes a natural transition.

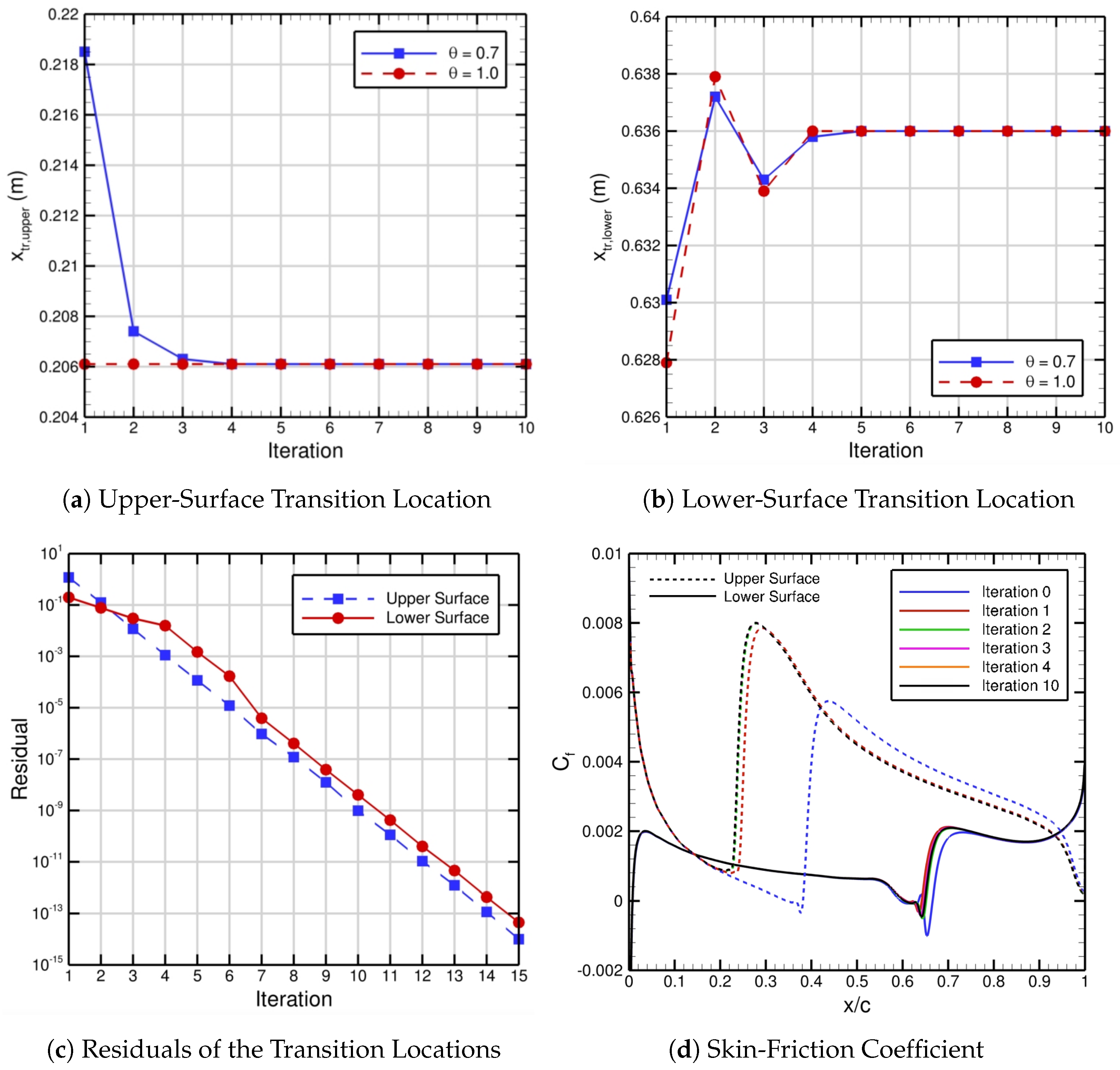
Figure 9 depicts the iterative convergence of the imposed transition locations with the associated residuals and the skin-friction distributions. For the initial basic state, i.e., the zeroth iteration, we imposed transitions at ${(x/c)}_{\mathrm{tr}},\mathrm{upper}=0.4$ and ${(x/c)}_{\mathrm{tr}},\mathrm{lower}=0.65$. Note that this choice is arbitrary, and, if we imposed transition further upstream or downstream on either the upper or lower surfaces for the zeroth iteration, the final converged transition locations would be the same. The skin friction of the initial basic state is shown in Figure 9d. After the initial FUN3D basic state has been obtained, we perform the same iterative procedure conducted for the zero-pressure-gradient flat-plate case and outlined in Figure 1. Both transition locations on the upper and lower surfaces of the NLF-0416 airfoil converge in Figure 9, with values of ${(x/c)}_{\mathrm{tr}},\mathrm{upper}=0.2085$ and ${(x/c)}_{\mathrm{tr}},\mathrm{lower}=0.636$. In this case, we consider the flow to be converged at iteration 5 because the associated change in the locations of transition onset for the upper and lower surfaces after iteration 5 is less than $0.001$ m, but we also show convergence to machine zero (i.e., $\Delta x_{\mathrm{tr}}={10}^{-13}$ m) or to iteration 15 in Figure 9c. Also, the skin friction in Figure 9d is essentially identical for iterations 4 through 10. The lower under-relaxation factor of 0.7 does not have a significant benefit in this particular case, but, for initially imposed transition locations far away from their converged values and for more complex flow configurations, it helps the automated FUN3D–LASTRAC iterative method to smoothly approach the final solution. The upper-surface transition location converges similarly to the flat-plate case, but the lower-surface transition location has oscillatory convergence because of the strong influence of the separation bubble on transition to turbulence. In other words, for the lower airfoil surface, there is an interaction that occurs between the separation-reattachment characteristics and the location of transition onset. This causes the imposed transition location for the lower surface to be upstream and downstream of the final converged transition location at different iterations of the automated FUN3D–LASTRAC process.

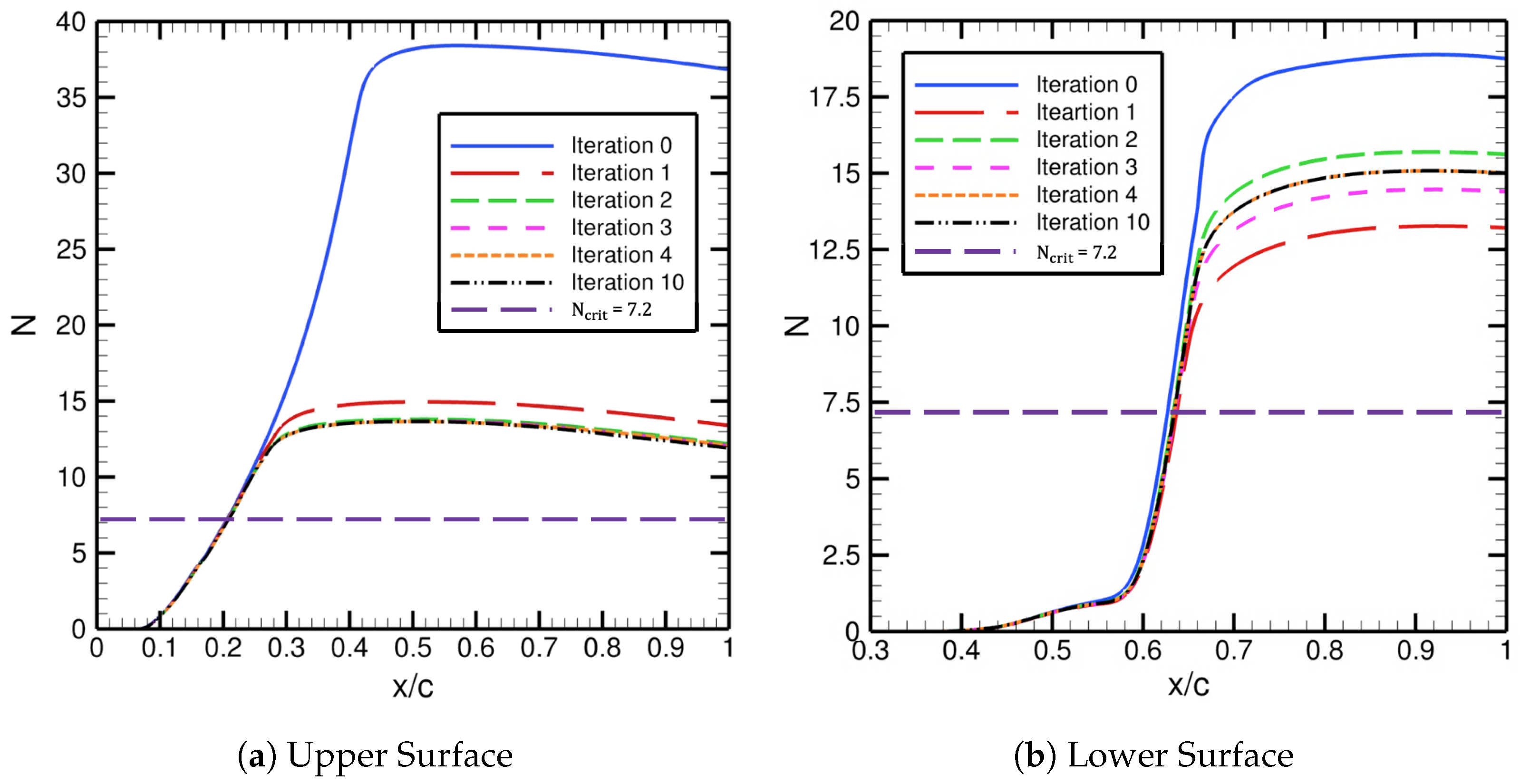
Figure 10 shows the iterative convergence of the N-factor envelopes that are computed with PSE using LASTRAC for iterations 0, 1, 3, 4, and 10. Since the value of $Tu$ is 0.15%, we obtain $N_{\mathrm{crit}}=-8.43-2.4\ln (0.15\%)=7.2$. Again, this expression from Mack [8] is based on flat-plate computations with LST (i.e., TS growth in attached boundary layers). Thus, it applies for natural transition along the upper surface, but, for the lower surface, its use for separation bubbles is somewhat arbitrary. It will be demonstrated that values based on Mack’s correlation lead to reasonable flow behavior and yield a good match with the limited available experimental measurements for this case. For the upper airfoil surface, the N-factor envelopes decrease from a maximum value of approximately 38 to 15 until convergence by about iteration 5. Moreover, for the lower airfoil surface, the maximum values of the N-factor envelopes oscillate around the final converged maximum value of 15 by iteration 4. This reaffirms the interaction that occurs between the separation-reattachment characteristics and the location of transition onset on the lower surface. Regardless of the visible influence of the separation bubble on the lower-surface transition location, the automated FUN3D–LASTRAC transition-prediction method iteratively converges quickly by iteration 4 in terms of both the skin-friction coefficient and the location of transition onset.

Similar to the flat-plate case, after demonstrating iterative convergence of the automated FUN3D–LASTRAC transition-prediction method for the NLF-0416 airfoil, we focus on showing grid convergence for the $\alpha =5^{\circ }$ case. Figure 11 shows the grid convergence of the lift and drag coefficients, transition locations, and the skin-friction coefficient for the NLF-0416 airfoil via levels 4 through 9. The level 4 grid has 153,265 points, and the level 9 grid has 4,331,777 points. In Figure 11a, the lift and drag coefficients are converging as the grid spacing parameter $h=1/\sqrt{n_c^2+n_z^2}$ approaches zero. Both the upper and lower transition locations in Figure 11b also converge with respect to grid resolution. Furthermore, the skin-friction distribution in Figure 11d is also grid-converged by level 9.

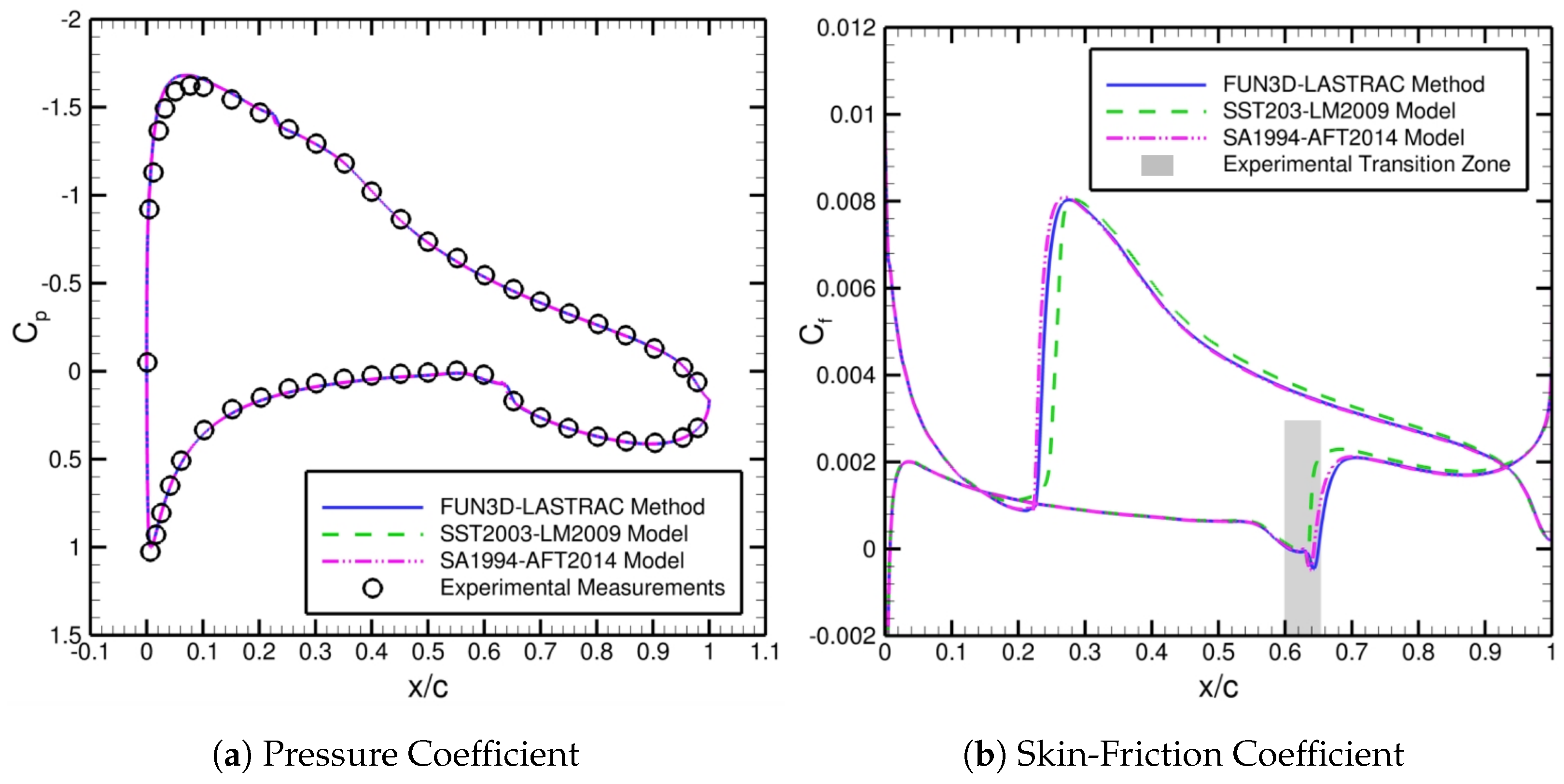
To validate our automated FUN3D–LASTRAC transition-prediction method, we compare the pressure and skin-friction coefficients between our method, the SST2003-LM2009 model [5], the SA1994-AFT2014 model [14], and experimental measurements [40] in Figure 12. There is excellent agreement between the computational and experimental results in terms of the pressure coefficient in Figure 12a. All the different computational skin-friction distributions begin to quickly rise in the experimentally observed transition zone [40] for the lower airfoil surface, which indicates good agreement between the computations and experiments in Figure 12b. Note that there are no measurements of the experimental transition zone on the upper surface. There is also good agreement between the different computational predictions (i.e., our automated FUN3D–LASTRAC transition-prediction method and two RANS-based transition models) of the skin-friction distributions and transition locations.

3.3. The 6:1 Prolate Spheroid

The last case we consider to validate our automated FUN3D–LASTRAC transition-prediction method is the 6:1 prolate spheroid. This geometry was experimentally investigated by Kreplin et al. [41]. Stability analysis for the 6:1 prolate spheroid has been performed by Spall and Malik [42], Stock [43], and Krimmelbein et al. [44]. The spheroid is representative of a simplified fuselage of a commercial aircraft. This flow configuration is a part of both the AIAA Transition Modeling Workshop and the AVT-313 Incompressible Flow Transition Comparison Workshop (http://web.tecnico.ulisboa.pt/ist12278/Workshop_AVT_313_2D_cases/Workshop_AVT_313_2D_cases.htm, accessed on 20 March 2025). We consider an angle of attack equal to 24 degrees. The freestream Mach number, temperature, and Reynolds number based on the spheroid length are 0.136, 300 K, and $6.5\times {10}^6$, respectively. A freestream turbulence intensity of $Tu=0.15\%$ is used for this case. The spheroid length is equal to 2.4 m. Transition can occur via TS waves and crossflow instability. Farfield boundary conditions are employed at the domain edges approximately 100 spheroid lengths away from the viscous surface, except for a symmetry boundary condition that is employed on the $xy$ plane at $z=0$. Adiabatic and no-slip boundary conditions are enforced on the spheroid surface. We have run a few representative cases using our coupled iterative approach for the 6:1 prolate spheroid with the entire geometry and flow field, i.e., no symmetry assumption, and the results are identical to simplified cases with the symmetry assumption.

Figure 13 depicts the lowest-resolution (i.e., level 3) computational grid on the spheroid surface and within the $y/L=0$ plane for better visibility. We use a multiblock structured grid to avoid singularities along the major axis of the spheroid model near the nose and the aft end of the geometry. Totals of 65, 65, and 257 points are used in the streamwise, azimuthal, and wall-normal directions to resolve the nose and aft blocks. For the central portion of the grid, we use 321, 193, and 257 points along the streamwise, azimuthal, and wall-normal directions, respectively. This grid is designed to contain at least 100 points within the boundary layer. Moreover, the first grid point away from the viscous surface is located at $\Delta y/L=5\times {10}^{-6}$, which yields $\Delta y^{+}=0.5$ at the location of $x/L=0.5$. For mesh level 5, totals of 97, 97, and 385 points are used in the streamwise, azimuthal, and wall-normal directions to resolve the nose and aft blocks, while 481, 289, and 385 points in the same respective directions are used to resolve the central portion of the grid.

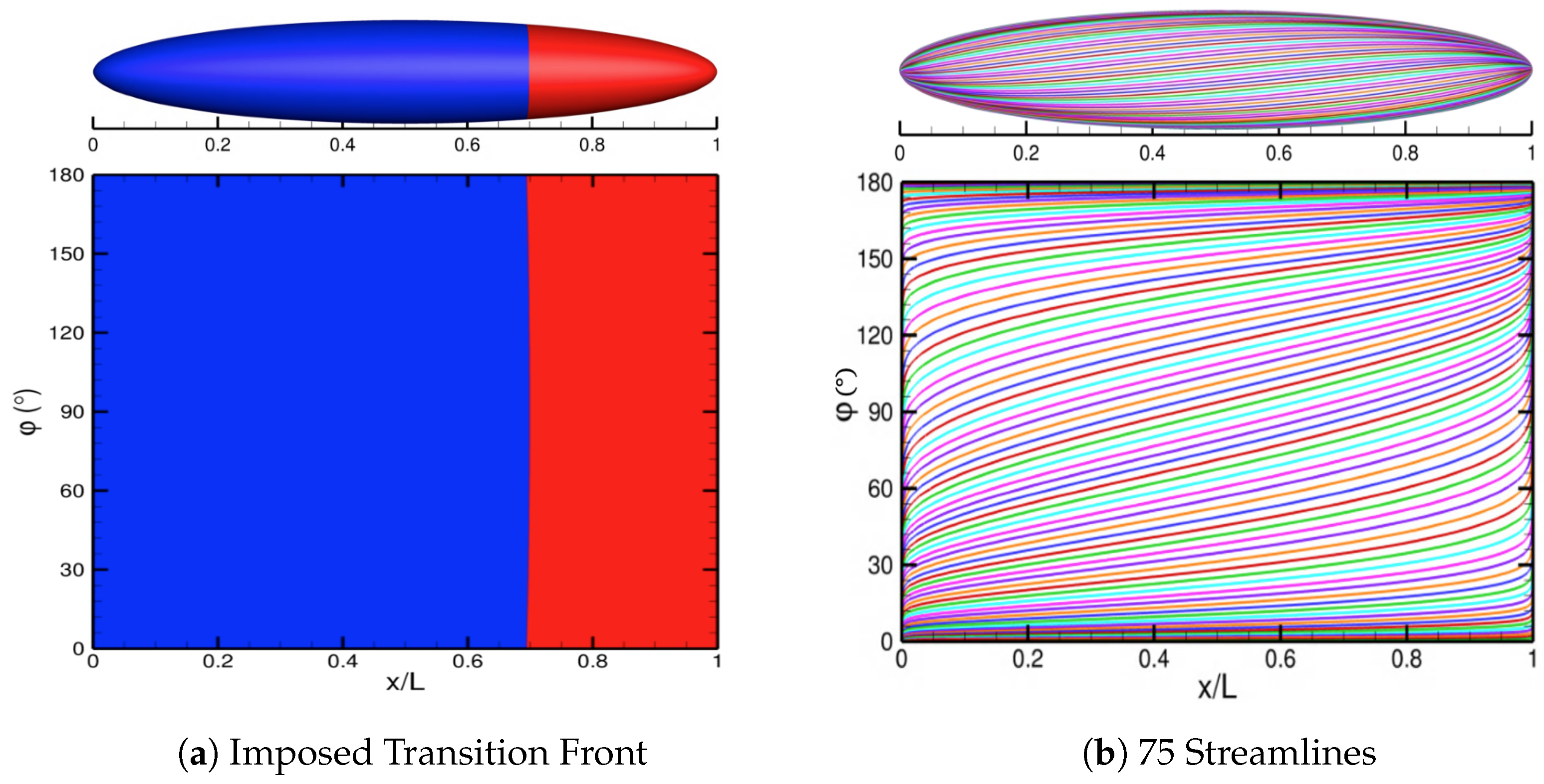
To initiate the automated FUN3D–LASTRAC method, we impose a transition front downstream at $x/L=0.7$. This initial transition front is shown in Figure 14a, where the blue contours indicate a laminar region and the red contours indicate a turbulent region. For the LASTRAC stability analysis, we use 75 vertex seeds on the spheroid surface. The solver-independent module creates the streamlines for stability analysis from these vertex seeds. Figure 14b displays the 75 streamlines on the spheroid surface. We employ under-relaxation by using Equation (7) at each point along the azimuthal or $\varphi$ plane with $\theta =0.7.$ We use the dual $N_{\mathrm{TS}}$–$N_{\mathrm{CF}}$ criterion [45,46,47] to determine where transition occurs from the TS and crossflow N-factor values. Refer to Choudhari et al. [2] for more information on calibrating the dual $N_{\mathrm{TS}}$–$N_{\mathrm{CF}}$ criterion for multiple aerodynamic configurations. To illustrate the automated transition modeling for a 3D flow configuration, we use the simple criterion $N_{\mathrm{crit}}=N_{\mathrm{TS}}+N_{\mathrm{CF}}=8$. In a real-world application, the criterion would be tuned for a specific facility using a broad range of data. Unlike for the 2D cases, we use point transition or an intermittency zone with zero length, which is a convenient option for 3D cases with mixed-mode transition where intermittency models have not been developed. 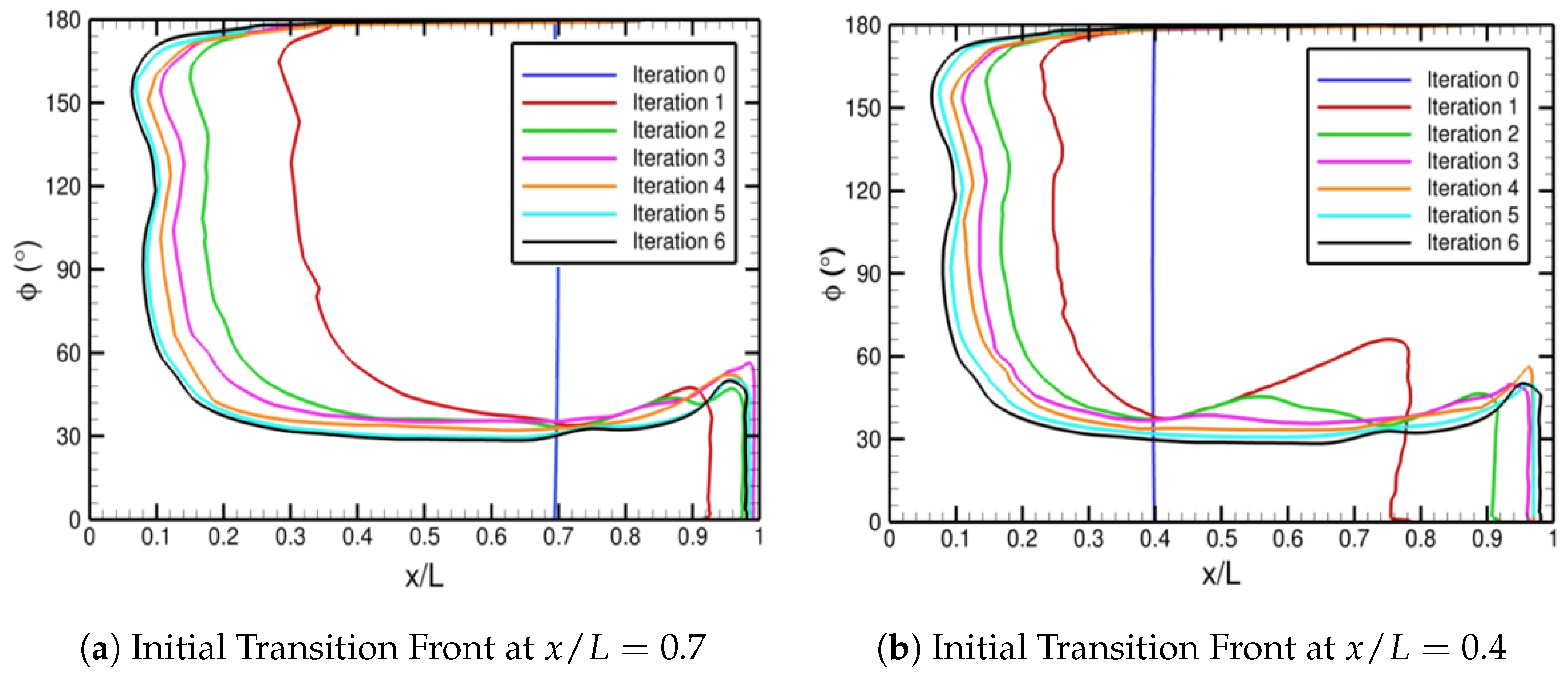Figure 15 displays the transition fronts corresponding to iterations 0 through 6 for two different initially imposed transition fronts. Notice that, by iteration 6, the automated FUN3D–LASTRAC method converges for both initial conditions.

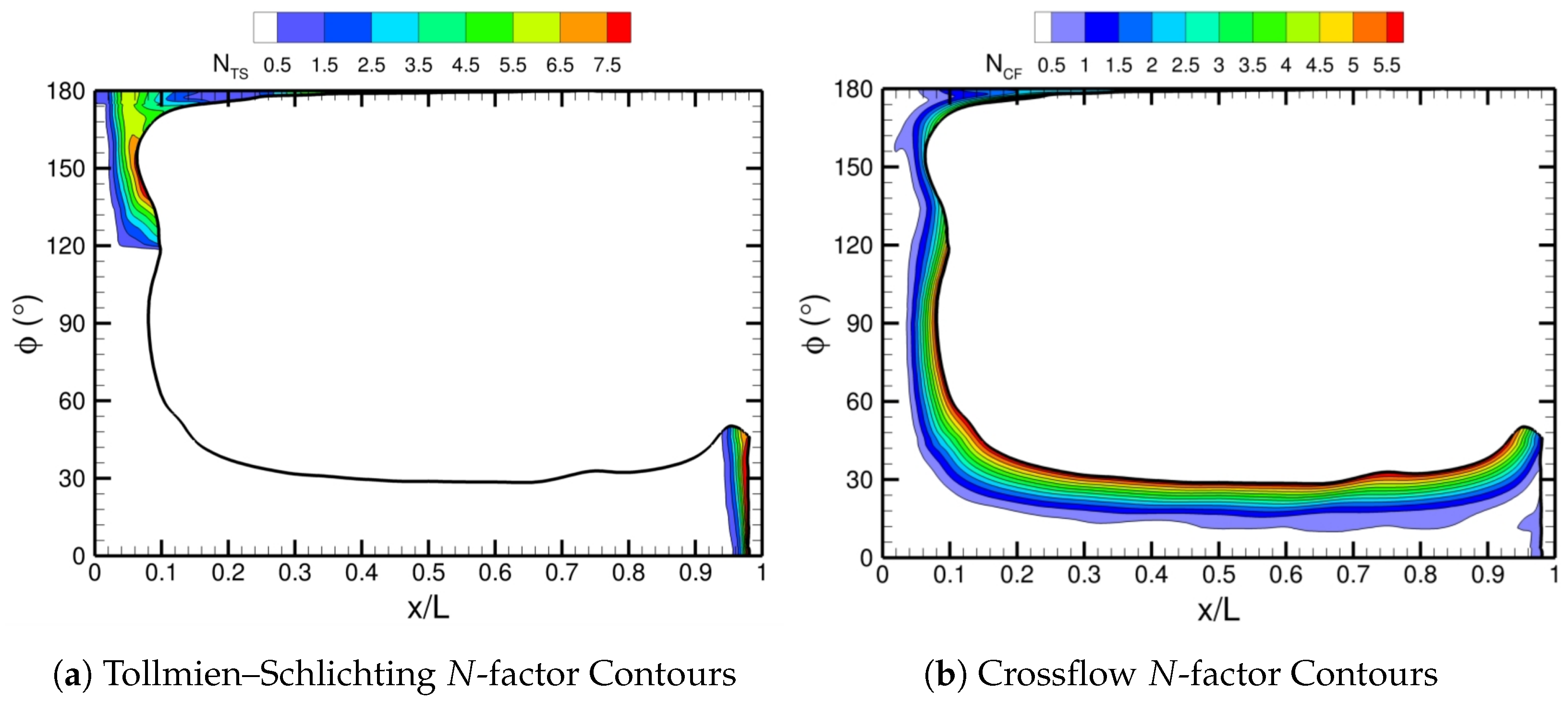
Figure 16 depicts the N-factor contours of TS and crossflow instability from PSE with LASTRAC. The final imposed transition front is also shown in Figure 16, downstream of which the N-factor contours are removed or set to zero because the flow has already transitioned to turbulence. For this case where $\alpha ={24}^{\circ }$, the N-factor values associated with TS waves are relatively small and do not significantly influence transition, except for $0\le \varphi <30$ far downstream near $x/L=0.98$ and $120<\varphi \le 180$ in Figure 16a. Crossflow vortices are important for this angle of attack because the associated N-factor values are appreciably large and are likely to dominate transition for $30\le \varphi \le 120$. 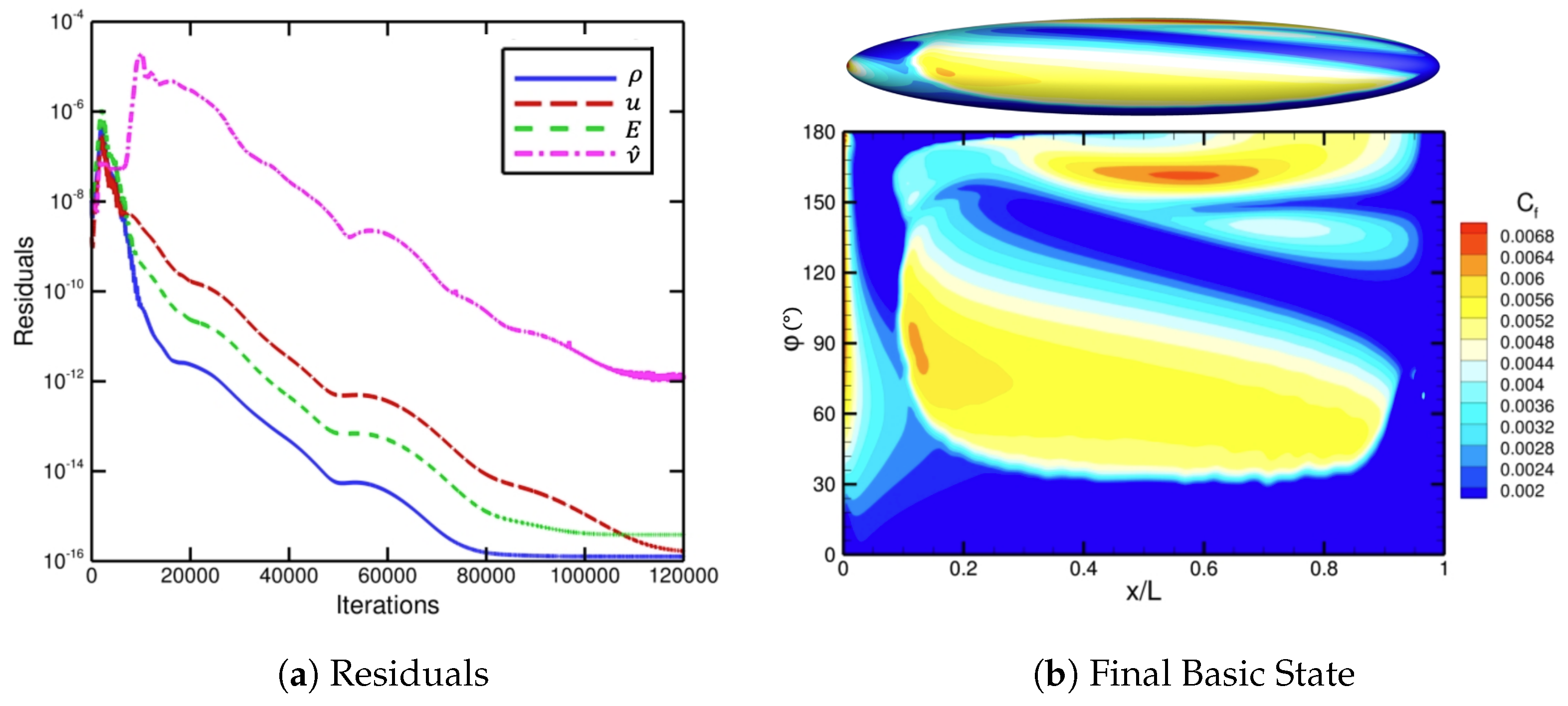We show the final solution of the automated FUN3D–LASTRAC method in Figure 17, along with the mean-flow basic-state residuals. Notice in Figure 17a that the mean-flow basic-state residuals drop approximately 10 orders of magnitude to machine zero and level out. The skin-friction contours of the final converged solution in Figure 17b are smooth and match the final transition front in Figure 15. Also, the results in Figure 17b agree with the skin-friction contours in Figure 11d of Krimmelbein et al. [44] for the 6:1 prolate spheroid at $\alpha ={24}^{\circ }$, computed using a coupled iterative approach (i.e., SST model [48] with a similar dual N-factor strategy).

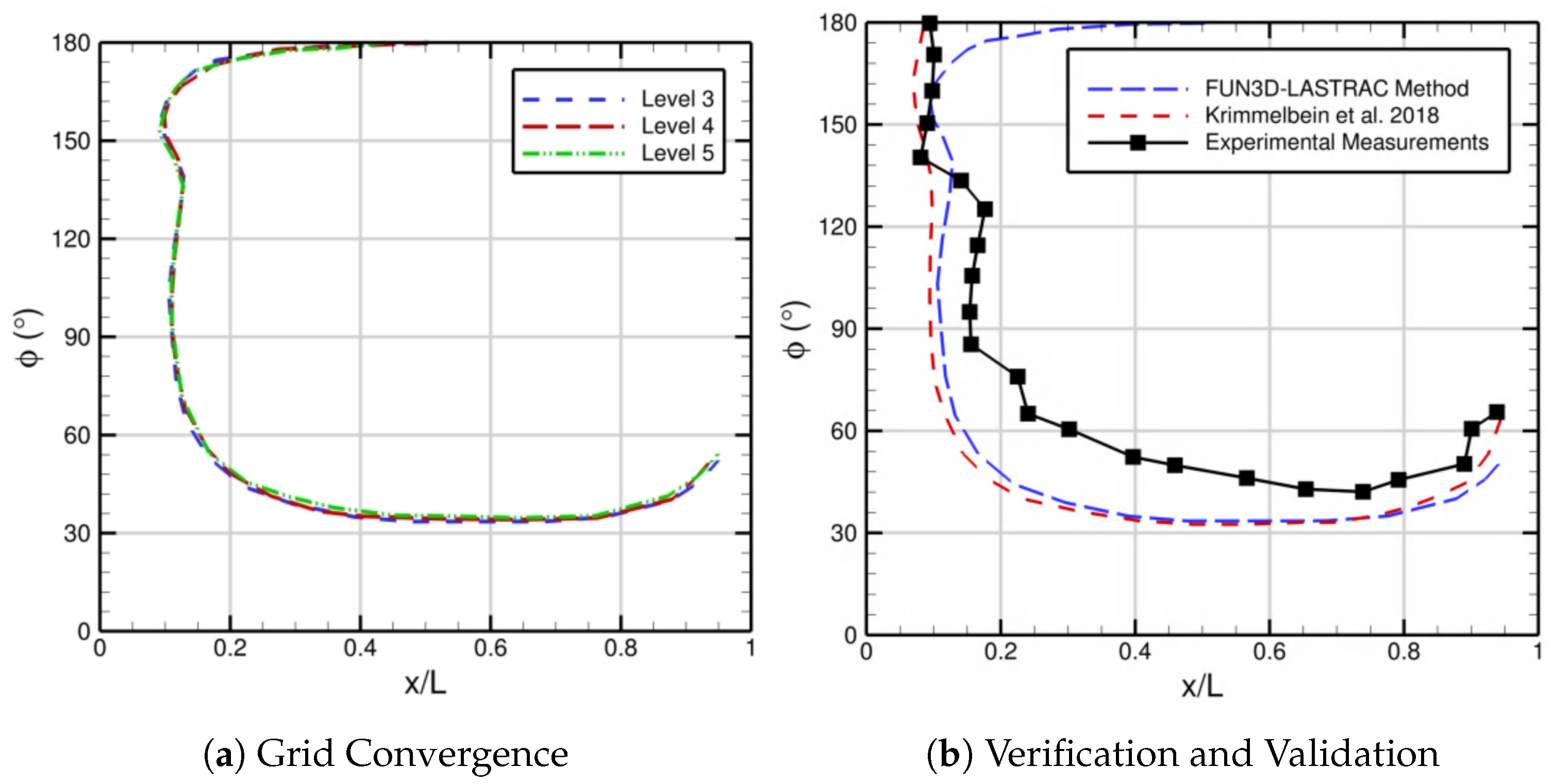
The grid convergence of the automated FUN3D–LASTRAC transition-prediction method is displayed in Figure 18a. Comparisons with the results from our method, the experimental measurements in Kreplin et al. [41], and the computational results from Krimmelbein et al. [44] based on the SST model paired with a similar dual N-factor strategy are depicted in Figure 18b. For the results in Figure 18, the extracted transition front comes from the skin-friction contours, more specifically $C_f=0.003$. This is conducted for better comparison with the measurements by Kreplin et al. [41] and Krimmelbein et al. [44]. In Figure 18a, the converged transition front is relatively insensitive to changes in the grid resolution across levels three, four, and five. The agreement between our computational results and those of Krimmelbein et al. [44] is very good. The comparison between our predictions and the experimental measurements by Kreplin et al. [41] is also reasonable, except that the predicted transition front is more upstream across the crossflow-dominated region from $30\le \varphi <130$, highlighting the need for calibrating the dual N-factor criterion in actual applications, similar to Krimmelbein et al. [44] and Choudhari et al. [2]. Overall, the sufficient iterative and grid convergence, along with the successful validation of the automated iterative coupled approach with FUN3D and LASTRAC for a 3D flow configuration, demonstrate that it can be applied to more complex problems and maintain accuracy, robustness, and efficiency.

4. Conclusions

The ability to accurately, robustly, and efficiently predict transition to turbulence in viscous flows is a key objective of the NASA CFD Vision 2030 initiative. In this article, we presented an automated iterative approach that couples two state-of-the-art capabilities: the unstructured flow solver FUN3D and the stability analysis code LASTRAC. This methodology was successfully demonstrated across a range of different transition scenarios in several canonical low-speed boundary-layer flows. These scenarios included natural transition via TS instabilities in zero-pressure-gradient flow over a flat plate, separation-bubble-induced transition over the NLF-0416 airfoil, and crossflow-dominated transition over a 6:1 prolate spheroid at a nonzero angle of incidence. The application of automated PSE-based stability analysis to these transition mechanisms in both 2D and 3D boundary layers represents a significant advancement over the traditional linear stability approaches by providing higher-fidelity modeling of the spatial evolution of the instability waves that initiate the transition process. The overall framework entails the application of three integrated software components during each iteration. First, a basic state computation is performed using steady-state RANS with the SA turbulence model in FUN3D that incorporates an estimated transition front. Second, boundary-layer stability analysis is conducted using the PSE implementation in LASTRAC to estimate the revised transition onset front. Third, a solver-independent module performs several critical functions that include viscous surface identification, the extraction of wall-normal profiles along selected disturbance growth paths, and the generation of integration trajectories (typically in the form of inviscid streamlines).

For the first test case, we simulated Mach 0.147 flow over a 2D zero-pressure-gradient flat plate, where natural boundary-layer transition occurs. The iterative approach coupling FUN3D and LASTRAC, along with a robust intermittency function, was shown to converge for two different fixed under-relaxation factors. This flat-plate case did not have significant viscous–inviscid interaction and, hence, was not significantly sensitive to iterative changes in the location of transition onset. Furthermore, grid convergence of the coupled approach with FUN3D and LASTRAC was also demonstrated for this flat-plate case, where consistent results were obtained for key metrics, including the drag coefficient, the location of transition onset, and the skin-friction coefficient. Our FUN3D–LASTRAC transition-prediction method showed good agreement with other methods that included an analogous approach coupling the overset grid flow solver OVERFLOW and LASTRAC, the SST2003-LM2009 and SA1994-AFT2014 models based on model auxiliary transport equations, and the Schubauer and Skramstad experiment [38] in terms of skin-friction evolution and the transition onset location.

The second test case involved the NLF-0416 airfoil at a five-degrees angle of attack. This configuration exhibited two distinct transition mechanisms: natural boundary-layer transition on the upper surface and separation-bubble-induced transition along the lower surface. Again, despite strong viscous–inviscid interaction due to the separation bubble that led to oscillations in the lower-surface transition location during early iterations, our coupled approach achieved successful convergence. The solution converged after five iterations, as evidenced by the convergence of the skin-friction distribution across the airfoil surface, the N-factor envelopes, and the predicted transition locations. A grid convergence study confirmed the reliability of the computed solution, demonstrating consistent predictions of the lift coefficient, drag coefficient, streamwise distribution of the skin-friction coefficient, and the transition onset locations on both upper and lower surfaces. The FUN3D–LASTRAC-based predictions of surface pressure distribution and transition onset locations showed excellent agreement with multiple verification sources, including the SST2003-LM2009 model, the SA1994-AFT2014 model, and the Somers experiment [40].

For the final test case, we simulated Mach 0.136 flow over a 6:1 prolate spheroid at an angle of attack equal to twenty-four degrees, where both TS waves and crossflow vortices had significant amplification factors. Thus, we employed a dual N-factor criterion based on PSE ($N_{\mathrm{crit}}=N_{\mathrm{TS}}+N_{\mathrm{CF}}=8$) to determine the transition front. The coupled FUN3D–LASTRAC approach converged iteratively using an under-relaxation factor of 0.7. Our analysis revealed distinct transition mechanisms across different regions of the spheroid surface. TS waves dominated for $0\le \varphi <30$ and $120<\varphi \le 180$, while crossflow instability played a dominant role across the intermediate region, $30\le \varphi \le 120$. The converged transition front demonstrated insensitivity to changes in the resolution on finer grids. The verification of the FUN3D–LASTRAC coupling approach showed excellent agreement with the transition front predicted by Krimmelbein et al. [44] using a similar approach. A comparison of the predicted transition front with the available measurements also indicated acceptable agreement with the experimental data by Kreplin et al. [41]. These three test cases demonstrate that our automated PSE-based FUN3D–LASTRAC coupling approach reliably predicts transition in viscous flows with good iterative and grid convergence. Future applications across diverse flow configurations should help to refine the N-factor calibrations, advancing the transition modeling capabilities for the design of greener aircraft.