Weakly Ricci-Symmetric Space-Times and f (R,G) Gravity
Abstract
1. Introduction
2. Proof of the Main Results
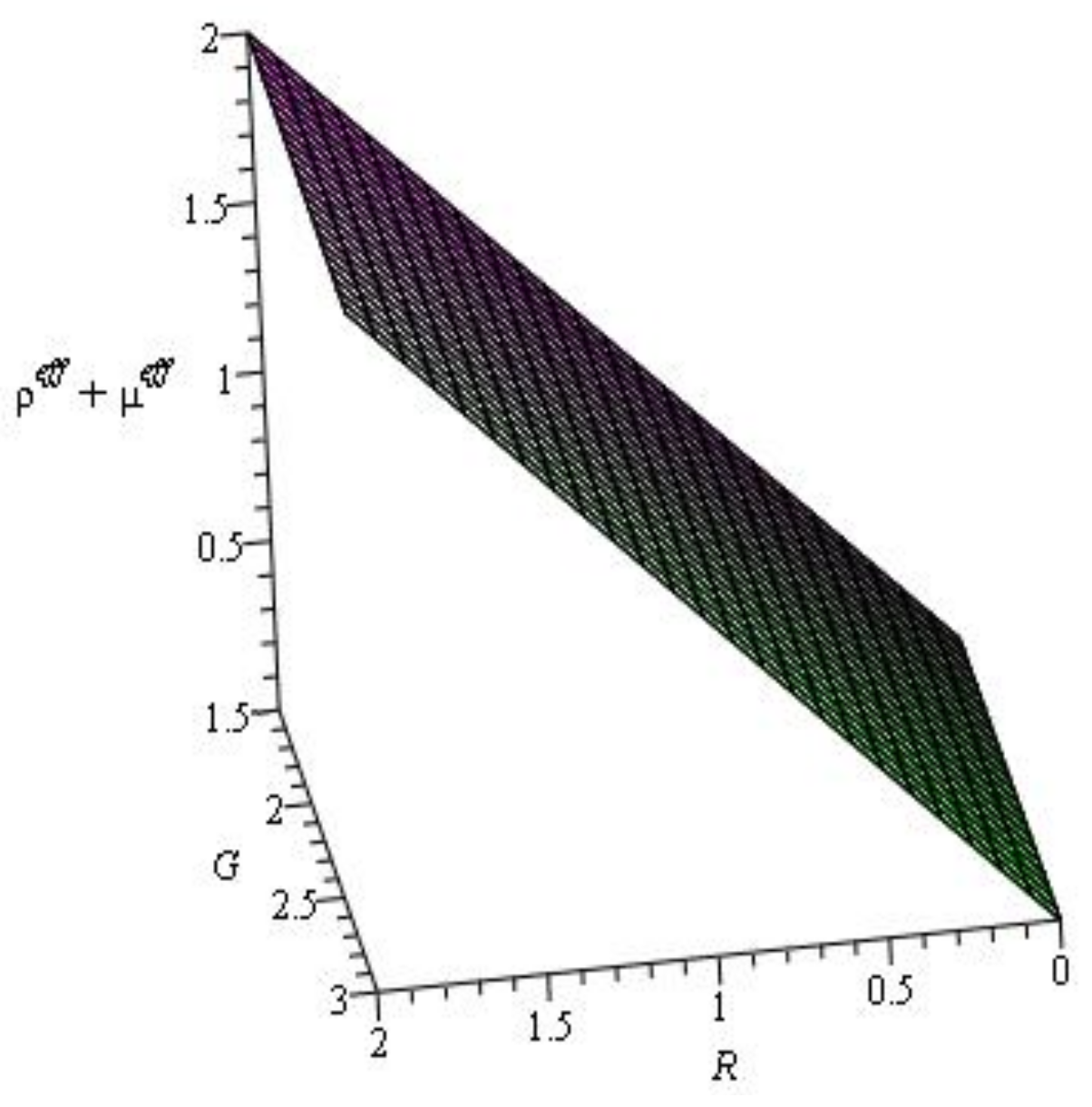
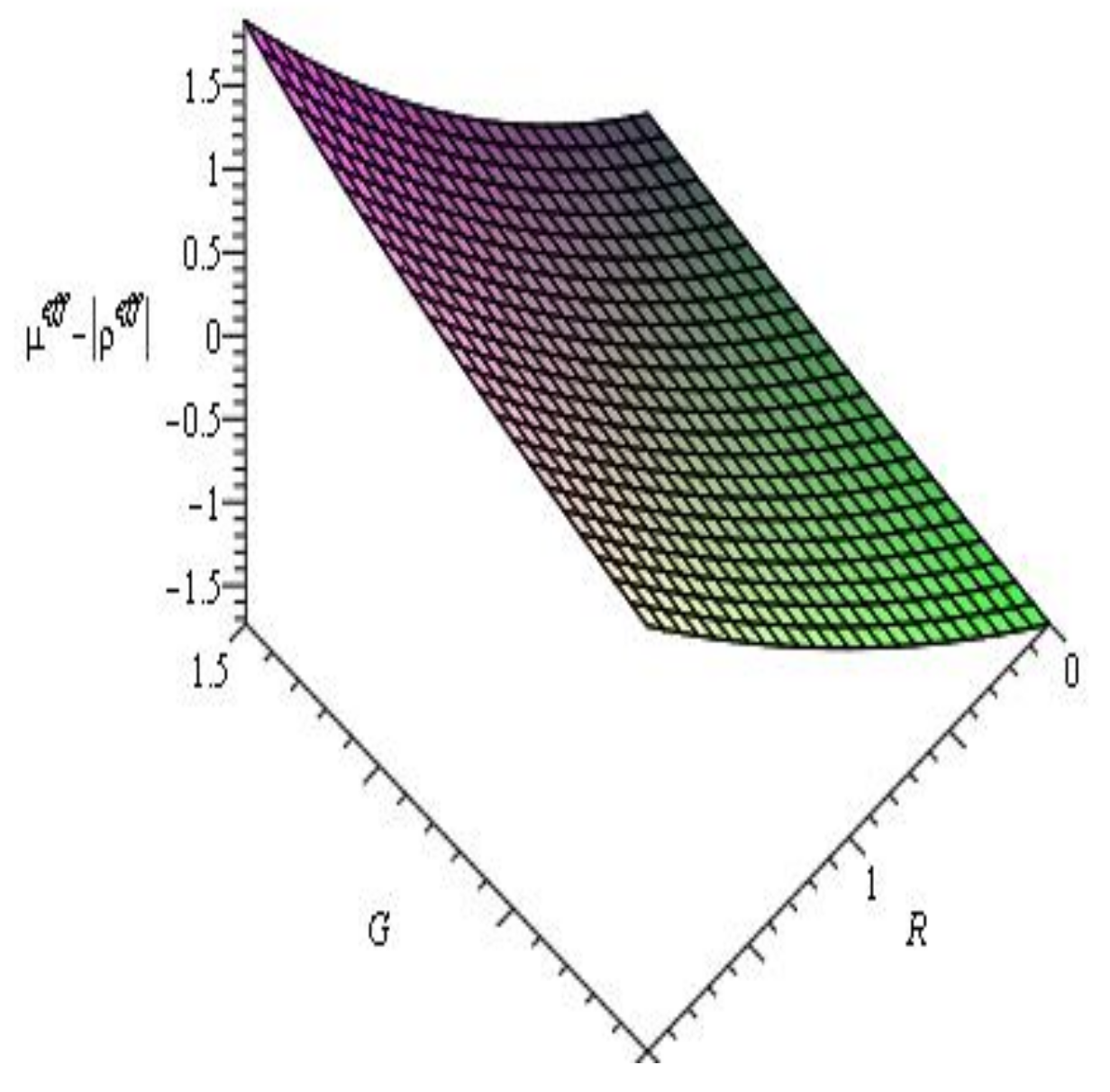
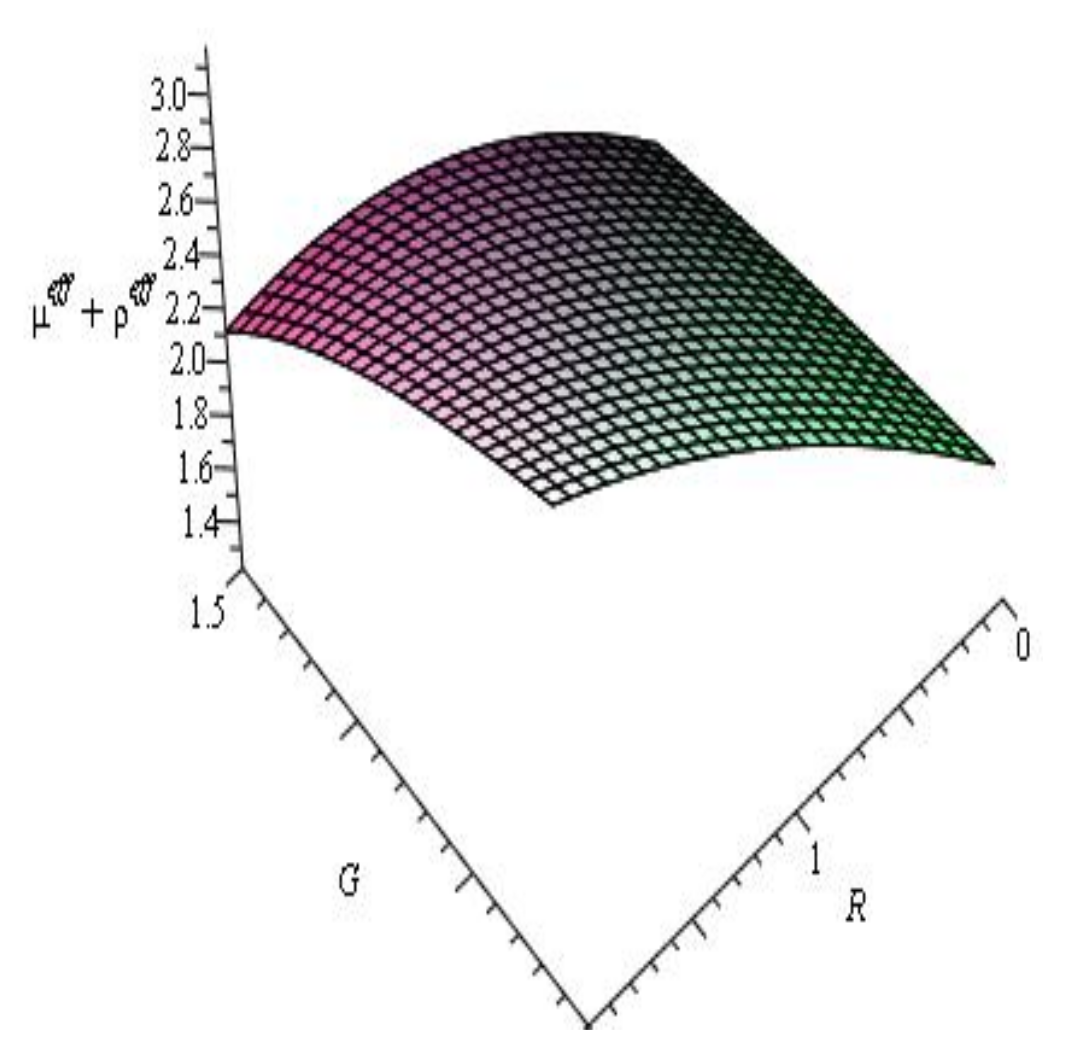
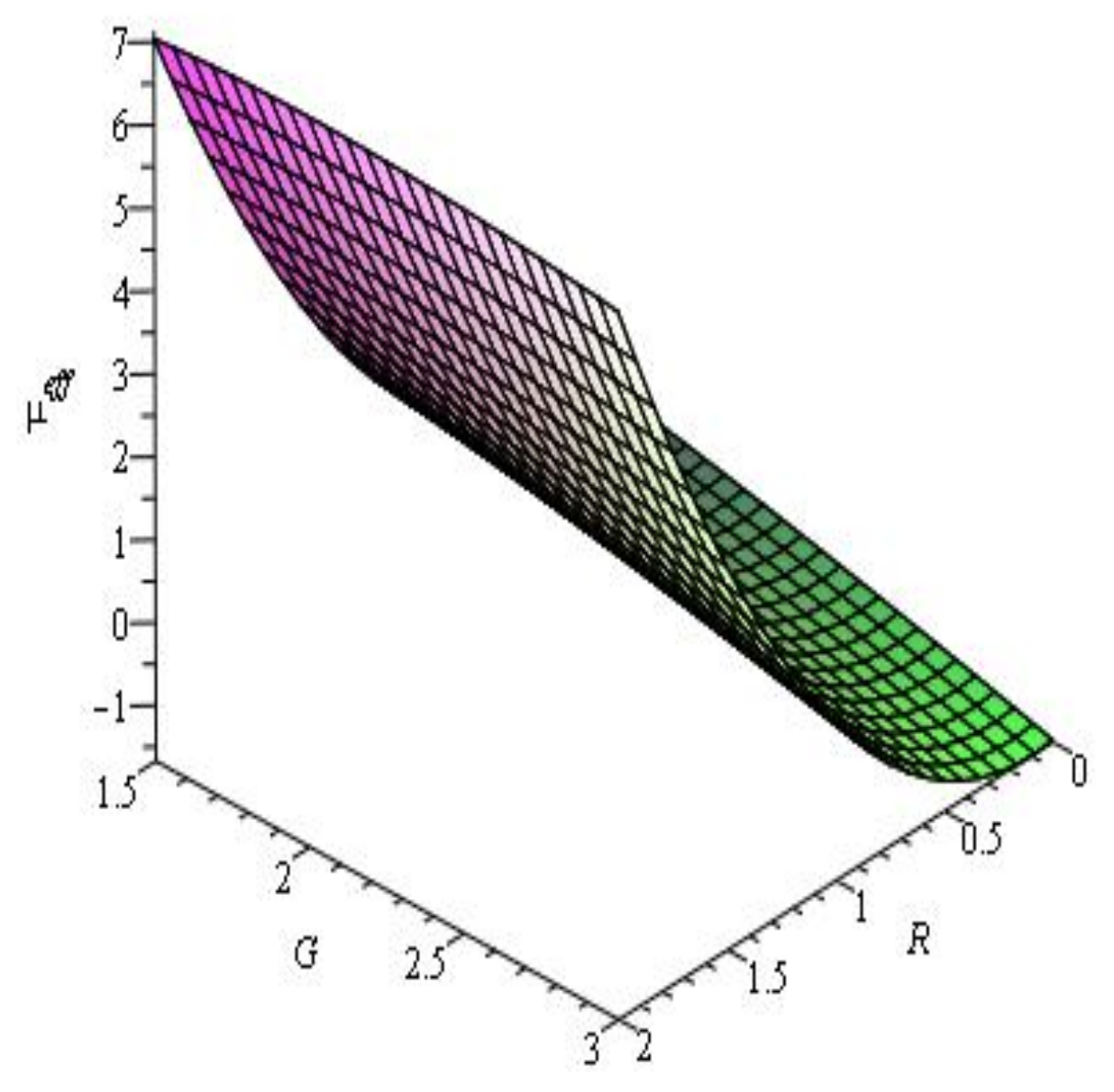
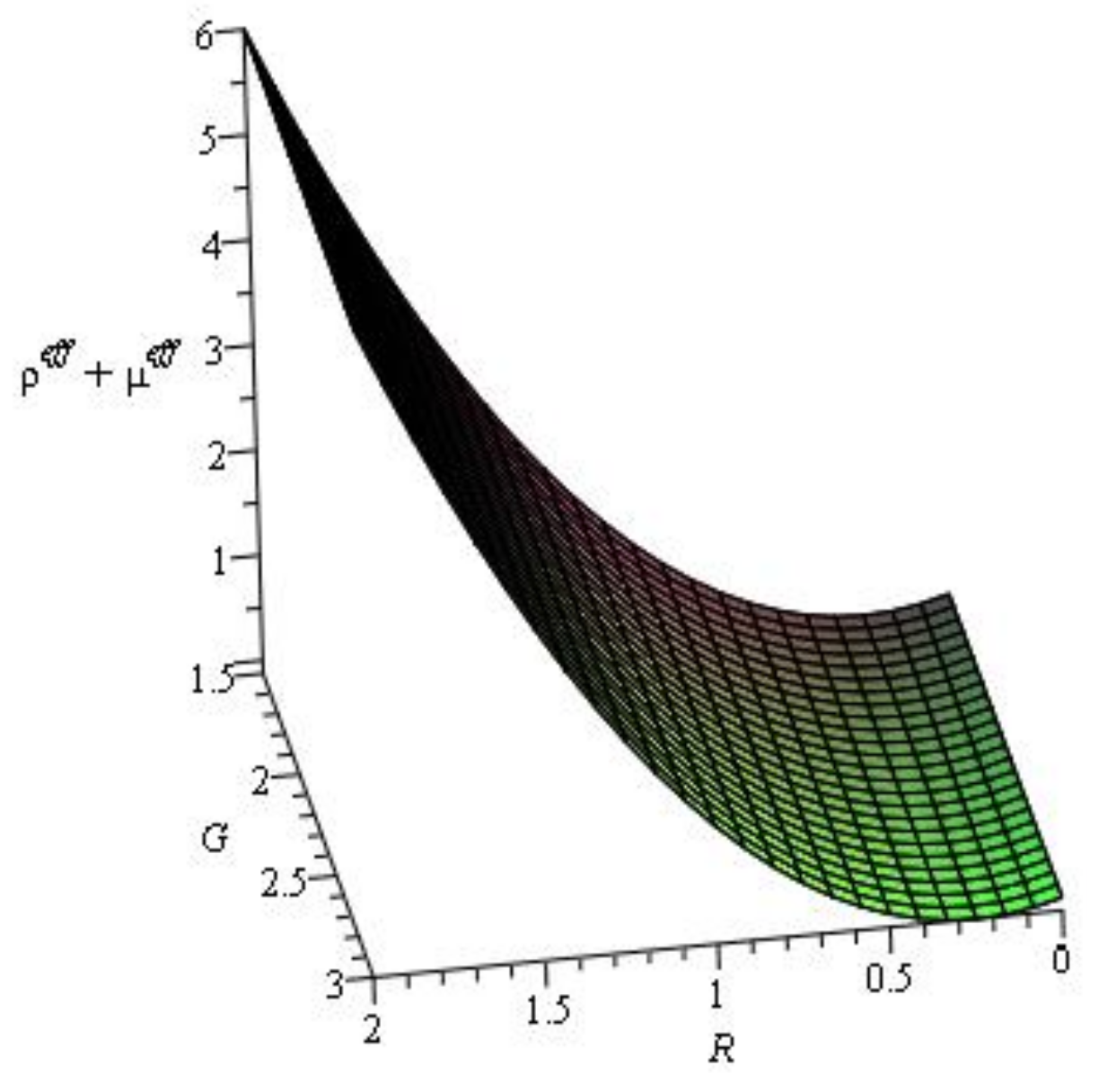
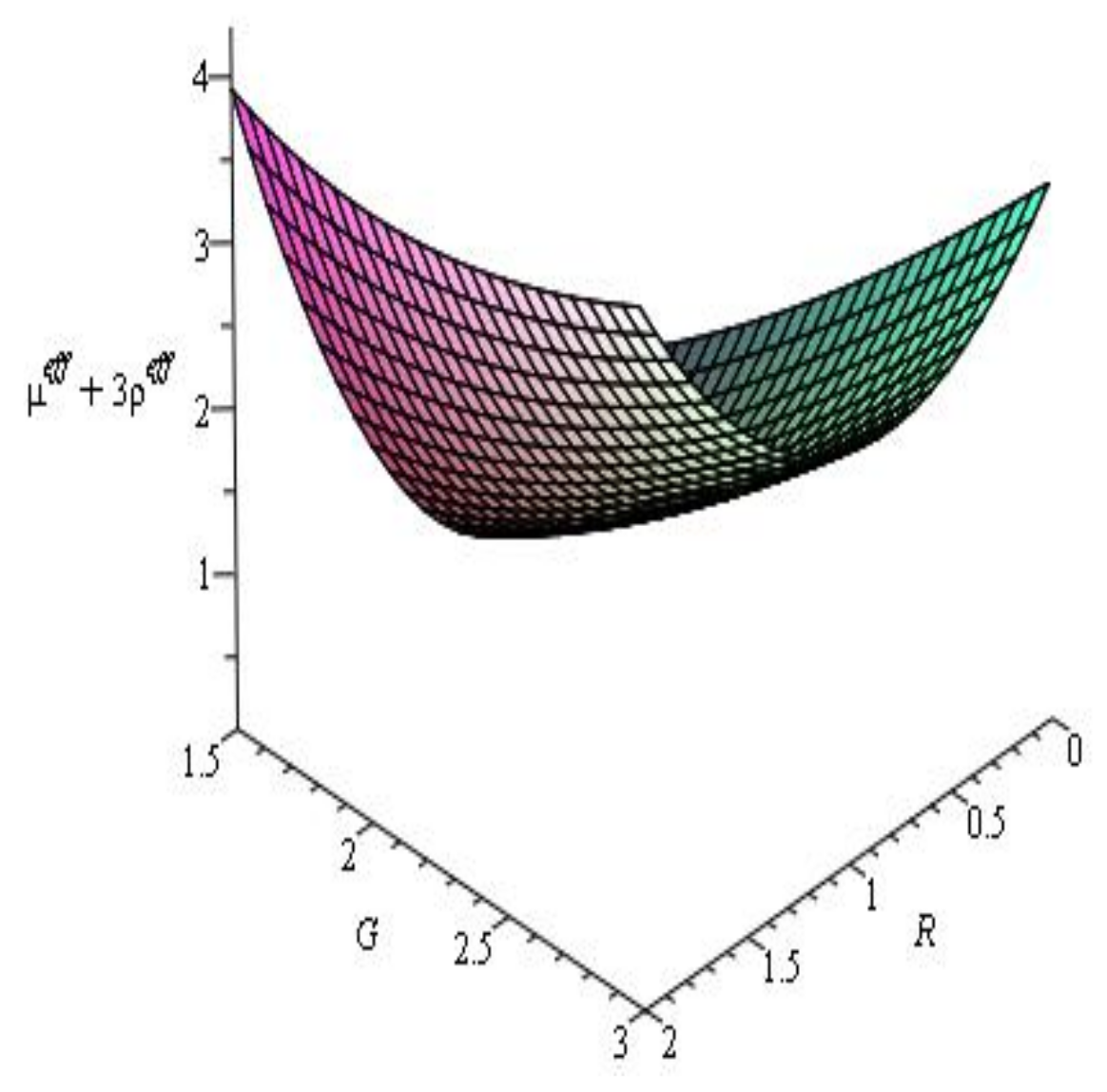
3. Conformally Flat Weakly Ricci-Symmetric Space-Time Satisfying Gravity
- A.
- B.
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- De, K.; De, U.C.; Velimirovic, L. Some curvature properties of perfect fluid spacetimes. Quaest. Math. 2024, 47, 751–764. [Google Scholar] [CrossRef]
- Guler, S.; Demirbag, S.A. A study of generalized quasi-Einstein spacetimes with applications in general relativity. Int. J. Theor. Phys. 2016, 55, 548–562. [Google Scholar] [CrossRef]
- Li, Y.; Alshehri, N.; Ali, A. Riemannian invariants for warped product submanifolds in Qϵm×R and their applications. Open Math. 2024, 22, 20240063. [Google Scholar] [CrossRef]
- Alías, L.; Romero, A.; Sánchez, M. Uniqueness of complete spacelike hypersurfaces of constant mean curvature in generalized Robertson-Walker space-times. Gen. Relativ. Gravit. 1995, 27, 71–84. [Google Scholar] [CrossRef]
- Mantica, C.A.; Molinari, L.G. Twisted Lorentzian manifolds: A characterization with torse-forming time-like unit vectors. Gen. Relativ. Gravit. 2017, 49, 51–58. [Google Scholar] [CrossRef]
- Chen, B.-Y. Totally umbilical submanifolds. Soochow J. Math. 1979, 5, 9–37. [Google Scholar]
- Chen, B.-Y. A simple characterization of generalized Robertson–Walker space-times. Gen. Relativ. Gravit. 2014, 46, 1833. [Google Scholar] [CrossRef]
- Li, Y.; Bhattacharyya, S.; Azami, S.; Hui, S. Li-Yau type estimation of a semilinear parabolic system along geometric flow. J. Inequal. Appl. 2024, 131, 2024. [Google Scholar] [CrossRef]
- Li, Y.; Siddesha, M.S.; Kumara, H.A.; Praveena, M.M. Characterization of Bach and Cotton Tensors on a Class of Lorentzian Manifolds. Mathematics 2024, 12, 3130. [Google Scholar] [CrossRef]
- Li, Y.; Mallick, A.K.; Bhattacharyya, A.; Stankovic, M.S. A Conformal η-Ricci Soliton on a Four-Dimensional Lorentzian Para-Sasakian Manifold. Axioms 2024, 13, 753. [Google Scholar] [CrossRef]
- Mandal, S.; Bhattacharjee, S.; Pacif, S.K.; Sahoo, P.K. Accelerating universe in hybrid and logarithmic teleparallel gravity. Phys. Dark Univ. 2020, 28, 100551. [Google Scholar] [CrossRef]
- Mandal, S.; Sahoo, P.K.; Santos, J.R.L. Energy conditions in f (Q) gravity. Phys. Rev. D 2020, 102, 024057. [Google Scholar] [CrossRef]
- Mantica, C.A.; Molinari, L.G. Generalized Robertson Walker space-times-A survey. Int. J. Geom. Meth. Mod. Phys. 2017, 14, 1730001. [Google Scholar] [CrossRef]
- O’Neill, B. Semi-Riemannian Geometry with Applications to the Relativity; Academic Press: New York, NY, USA; London, UK, 1983. [Google Scholar]
- Chavanis, P.H. Cosmology with a stiff matter era. Phys. Rev. D 2015, 92, 103004. [Google Scholar] [CrossRef]
- Sen, R.N.; Chaki, M.C. On curvature restriction of a certain kind of conformally flat Riemannian spaces of class-one. Proc. Nat. Inst. Sci. India Part A 1967, 33, 100–102. [Google Scholar]
- Chaki, M.C. On pseudo Ricci symmetric manifolds. Bulg. J. Phys. 1988, 15, 526–531. [Google Scholar]
- Tamássy, L.; Binh, T.Q. On weak symmetries of Einstein and Sasakian manifolds. Tensor 1993, 53, 140–148. [Google Scholar]
- Chaki, M.C.; Ray, S. Spacetimes with covariant constant energy momentum tensor. Int. J. Theor. Phys. 1996, 35, 1027–1032. [Google Scholar] [CrossRef]
- Chaki, M.C.; Koley, S. On generalized pseudo Ricci symmetric manifolds. Period. Math. Hungar. 1994, 28, 123–129. [Google Scholar] [CrossRef]
- Mantica, C.A.; Suh, Y.J.; De, U.C. A note on Generalized Robertson Walker space-times. Int. J. Geom. Meth. Mod. Phys. 2016, 13, 1650079. [Google Scholar] [CrossRef]
- Sánchez, M. On the geometry of static space-times. Nonlinear Anal. Theory Methods Appl. 2005, 63, 455–463. [Google Scholar] [CrossRef]
- Hawking, S.W.; Ellis, G.F.R. The Large Scale Structure of Spacetime; Cambridge University Press: Cambridge, UK, 1973. [Google Scholar]
- Patterson, E.M. Some theorems on Ricci-recurrent spaces. J. London Math. Soc. 1952, 27, 287–295. [Google Scholar] [CrossRef]
- Mirzoyan, V.A. Structure theorems for Riemannian Ric-semisymmetric spaces. Russian Math. (Iz. VUZ) 1992, 36, 75–83. [Google Scholar]
- Atazadeh, K.; Darabi, F. Energy conditions in f(R,G) gravity. Gen. Rel. Grav. 2013, 46, 1664. [Google Scholar] [CrossRef]
- Raychaudhuri, A.K.; Banerji, S.; Banerjee, A. General Relativity, Astrophysics, and Cosmology; Springer: New York, NY, USA, 1992. [Google Scholar]
- Duggal, K.L.; Sharma, R. Symmetries of Space-Times and Riemannian Manifolds; Springer: New York, NY, USA, 1999. [Google Scholar]
- Elizalde, E.; Myrzakulov, R.; Obukhov, V.V.; Sáez-Gómez, D. ΛCDM epoch reconstruction from F (R,G)and modified Gauss–Bonnet gravities. Class. Quantum Gravity 2010, 27, 095007. [Google Scholar] [CrossRef]
- De la Cruz-Dombriz, Á.; Sáez-Gómez, D. On the stability of the cosmological solutions in f (R,G) gravity. Class. Quantum Gravity 2012, 29, 245014. [Google Scholar] [CrossRef]
- Laurentis, M.D.; Lopez-Revelles, A.J. Newtonian, Post-Newtonian and Parametrized Post-Newtonian limits of f (R,G) gravity. Int. J. Geom. Methods Mod. Phys. 2014, 11, 1450082. [Google Scholar] [CrossRef]
- Laurentis, M.D.; Paolella, M.; Capozziello, S. Cosmological inflation in f (R,G) gravity. Phys. Rev. D 2015, 91, 083531. [Google Scholar] [CrossRef]
- Bhatti, M.Z.; Yousaf, Z.; Rehman, A.A. Gravastars in f(R,G) gravity. Phys. Dark Universe 2020, 29, 100561. [Google Scholar] [CrossRef]
- Bhatti, M.Z.; Yousaf, Z.; Rehman, A.A. Horizon thermodynamics in f(R,G) gravity. Fortschr. Phys. 2023, 71, 2200113. [Google Scholar] [CrossRef]
- Suh, Y.J.; De, K.; De, U.C. Impact of projective curvature tensor in f (R,G), f (R,T) and f (R,Lm)-gravity. Int. J. Geom. Meth. Mod. Phys. 2024, 21, 2450062. [Google Scholar] [CrossRef]
- Turki, N.B.; De, U.C.; Syied, A.A.; Vilcu, G.E. Investigation of space-times through W2-curvature tensor in f(R,G) gravity. J. Geom. Phys. 2023, 194, 104987. [Google Scholar] [CrossRef]
- Brozos-Vazquez, M.; Garcia-Rio, E.; Vazquez-Lorenzo, R. Some remarks on locally conformally flat static space–times. J. Math. Phys. 2005, 46, 022501. [Google Scholar] [CrossRef]
- Stephani, H.; Kramer, D.; Mac-Callum, M.; Hoenselaers, C.; Herlt, E. Exact Solutions of Einstein’s Field Equations; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Ehlers, J.; Kundt, W. Gravitation: An Introduction to Current Research; Witten, L., Ed.; Wiley: New York, NY, USA, 1962; p. 49. [Google Scholar]
- Myrzakulov, R.; Sebastiani, L.; Zerbini, S. Topological Static Spherically Symmetric vacuum Solutions in f(R,G) Gravity. Gen. Rel. Grav. 2013, 45, 675–690. [Google Scholar] [CrossRef]
- Bahamonde, S.; Dialektopoulos, K.; Camci, U.U. Exact spherically symmetric solutions in modified Gauss-Bonnet gravity from Noether symmetry approach. Symmetry 2020, 68, 12. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; De, U.C.; De, K. Weakly Ricci-Symmetric Space-Times and f (R,G) Gravity. Mathematics 2025, 13, 943. https://doi.org/10.3390/math13060943
Li Y, De UC, De K. Weakly Ricci-Symmetric Space-Times and f (R,G) Gravity. Mathematics. 2025; 13(6):943. https://doi.org/10.3390/math13060943
Chicago/Turabian StyleLi, Yanlin, Uday Chand De, and Krishnendu De. 2025. "Weakly Ricci-Symmetric Space-Times and f (R,G) Gravity" Mathematics 13, no. 6: 943. https://doi.org/10.3390/math13060943
APA StyleLi, Y., De, U. C., & De, K. (2025). Weakly Ricci-Symmetric Space-Times and f (R,G) Gravity. Mathematics, 13(6), 943. https://doi.org/10.3390/math13060943




