Default Priors in a Zero-Inflated Poisson Distribution: Intrinsic Versus Integral Priors
Abstract
1. Introduction
2. Default Procedures in Bayesian Testing
3. Testing for a Zero-Inflated Parameter in the ZIP
3.1. Default Bayes Testing for
3.2. Intrinsic Prior
- The proof is provided in Appendix A.
3.3. Integral Prior
- The proof is provided in Appendix A.
- The proof is provided in Appendix A.
3.4. An Encompassing Approach
4. Simulation Studies
5. Real Data Analysis
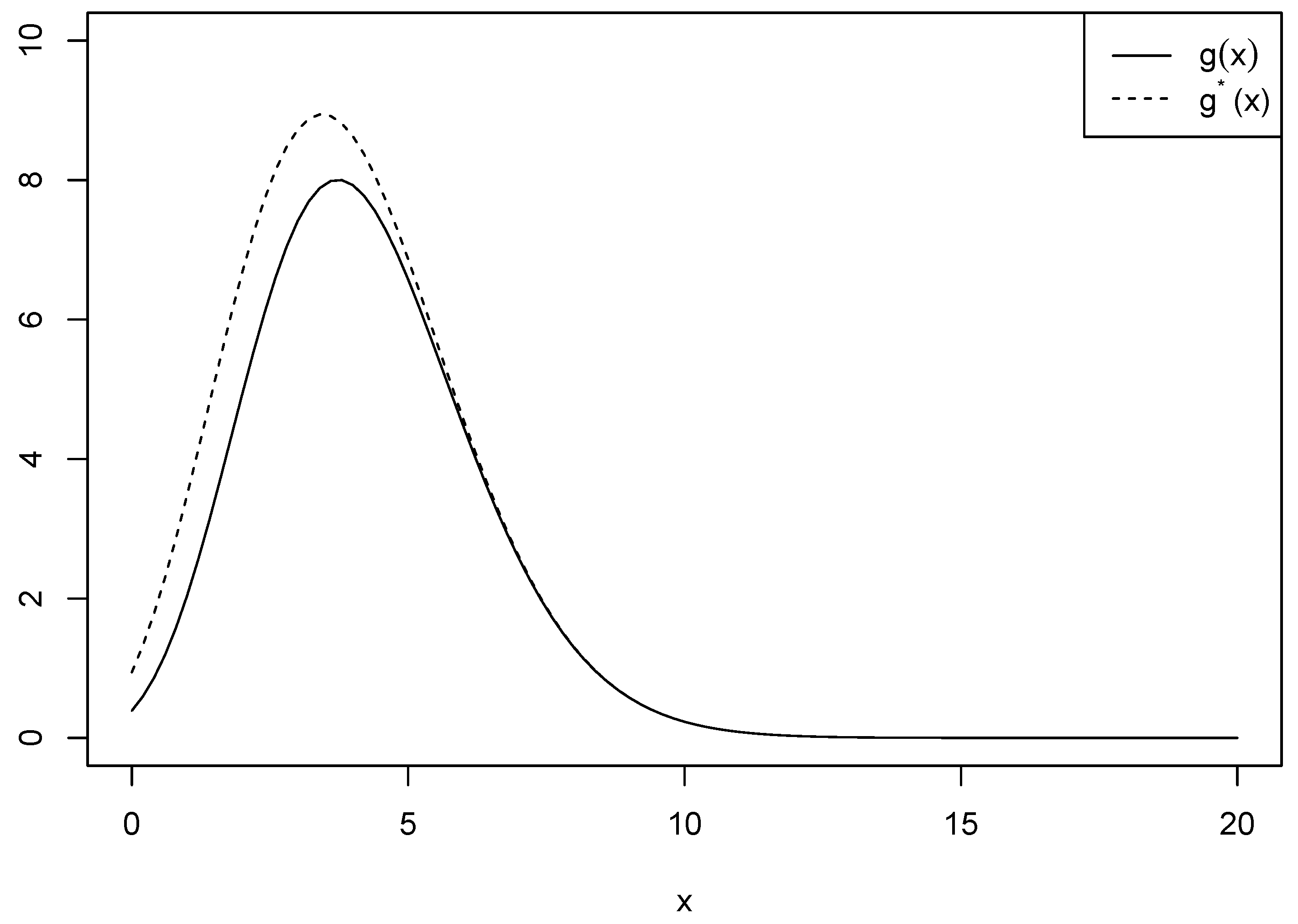
5.1. Yellow Dust Storm Data
5.2. Book Reading Data
6. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Cohen, A.C. Estimation in mixtures of two normal distributions. Technometrics 1967, 9, 15–28. [Google Scholar] [CrossRef]
- Lambert, D. Zero-inflated Poisson regression, with an application to defects in manufacturing. Technometrics 1992, 34, 1–14. [Google Scholar] [CrossRef]
- Hall, D.B. Zero-inflated Poisson and binomial regression with random effects: A case study. Biometrics 2000, 56, 1030–1039. [Google Scholar] [CrossRef] [PubMed]
- Yau, K.K.W.; Lee, A.H. Zero-inflated Poisson regression with random effects to evaluate an occupational injury prevention programme. Stat. Med. 2001, 20, 2907–2920. [Google Scholar] [CrossRef]
- Ghosh, S.K.; Mukhopadhyay, P.; Lu, J. Bayesian analysis of zero-inflated regression models. J. Stat. Plan. Inference 2006, 136, 1360–1375. [Google Scholar] [CrossRef]
- Yirdraw, B.S.; Debusho, L.K.; Samuel, A. Application of longitudinal multilevel zero inflated Poisson regression in modeling of infectious diseases among infants in Ethiopia. BMC Infect. Dis. 2024, 24, 927. [Google Scholar]
- Tshishimbi, W.M. Double robust semiparametric weighted M-estimators of a zero-inflated Poisson regression with missing data in covariates. Commun.-Stat.-Simul. Comput. 2024, 1–24. [Google Scholar] [CrossRef]
- Jeffreys, H. The Theory of Probability, 3rd ed.; Oxford University Press: New York, NY, USA, 1961; ISBN 978-01-9850-368-2. [Google Scholar]
- Berger, J.O.; Bernardo, J.M. Estimating a product of means: Bayesian analysis with reference priors. J. Am. Stat. Assoc. 1989, 84, 200–207. [Google Scholar] [CrossRef]
- Berger, J.O.; Pericchi, L.R. The intrinsic Bayes factor for model selection and prediction. J. Am. Stat. Assoc. 1996, 91, 109–122. [Google Scholar] [CrossRef]
- Lingham, R.T.; Sivaganesan, S. Intrinsic Bayes factor approach to a test for the power law process. J. Stat. Plan. Inference 1999, 77, 195–220. [Google Scholar] [CrossRef]
- Sanso, B.; Pericchi, L.R.; Moreno, E.; Racugno, W. On the robustness of the intrinsic Bayes factor for nested models [with discussion and rejoinder]. Lect. Notes-Monogr. Ser. 1996, 29, 155–173. [Google Scholar]
- Cano, J.A.; Robert, C.P.; Salmerón, D. Integral equation solutions as prior distributions for model selection. Test 2008, 17, 493–504. [Google Scholar] [CrossRef]
- Salmerón, D.; Cano, J.A.; Robert, C.P. Objective Bayesian hypothesis testing in binomial regression models with intgral prior distributions. Stat. Sin. 2015, 25, 1009–1023. [Google Scholar]
- Salmerón, D.; Cano, J.A.; Robert, C.P. On integral priors for multiple comparison in Bayesian model selection. arXiv 2024, arXiv:2406.14184. [Google Scholar]
- Bayarri, M.J.; García-Donato, G. Generalization of Jeffreys divergence-based priors for Bayesian hypothesis testing. J. R. Stat. Soc. Ser. B Stat. Methodol. 2008, 70, 981–1003. [Google Scholar] [CrossRef]
- Sivaganesan, S.; Jiang, D. Objective Bayesian testing of a Poisson mean. Commun. Stat.-Theory Methods 2010, 39, 1887–1897. [Google Scholar] [CrossRef]
- Han, Y.; Hwang, H.; Ng, H.K.T.; Kim, S.W. Default Bayesian testing for the zero-inflated Poisson distribution. Stat. Its Interface 2024, 17, 623–634. [Google Scholar] [CrossRef]
- Kass, R.E.; Raftery, A.E. Bayes factors. J. Am. Stat. Assoc. 1995, 90, 773–795. [Google Scholar] [CrossRef]
- Reid, N.; Mukerjee, R.; Fraser, D.A.S. Some aspects of matching priors. Lect. Notes-Monogr. Ser. 2003, 42, 31–43. [Google Scholar]
- Datta, G.S.; Mukerjee, R. Probability Matching Priors: Higher Order Asymptotics; Springer: New York, NY, USA, 2004; ISBN 978-0-387-20329-4. [Google Scholar]
- Peréz, J.M.; Berger, J.O. Expected-posterior prior distributions for model selection. Biometrika 2002, 89, 491–511. [Google Scholar] [CrossRef]
- Moreno, E.; Pericchi, L.R. Intrinsic priors for objective Bayesian model selection. Adv. Econom. 2014, 34, 279–300. [Google Scholar]
- Kim, S.W.; Sun, D. Intrinsic priors for model selection using an encompassing model with applications to censored failure time data. Lifetime Data Anal. 2000, 6, 251–269. [Google Scholar] [CrossRef]


| x = 13 | x = 14 | x = 15 | x = 16 | x = 17 | x = 18 | ||
|---|---|---|---|---|---|---|---|
| 0.2 | 3 | ||||||
| 4 | |||||||
| 0.8 | 3 | ||||||
| 4 | |||||||
| Propositions | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| p-Value | |||||||||||
| 0.2 | 0.2 | 3 | 30 | 0.436 (0.443) | 0.437 (0.444) | 0.420 (0.447) | 0.526 | 6.7 | 2.5 | 93.2 | 95.8 |
| 50 | 0.350 (0.378) | 0.351 (0.379) | 0.339 (0.380) | 0.521 | 5.0 | 3.2 | 95.0 | 98.2 | |||
| 100 | 0.263 (0.361) | 0.263 (0.362) | 0.259 (0.369) | 0.540 | 3.3 | 3.7 | 95.3 | 97.6 | |||
| 4 | 30 | 0.391 (0.431) | 0.392 (0.434) | 0.376 (0.461) | 0.501 | 5.9 | 4.0 | 94.1 | 98.1 | ||
| 50 | 0.306 (0.353) | 0.307 (0.354) | 0.296 (0.348) | 0.518 | 4.1 | 3.1 | 95.8 | 98.8 | |||
| 100 | 0.231 (0.298) | 0.231 (0.297) | 0.228 (0.331) | 0.519 | 3.1 | 4.1 | 95.9 | 99.0 | |||
| 0.4 | 3 | 30 | 92.405 (498.004) | 92.726 (500.266) | 113.300 (615.487) | 0.070 | 69.9 | 43.2 | 43.2 | 73.2 | |
| 50 | 0.016 | 82.7 | 66.6 | 66.6 | 83.9 | ||||||
| 100 | 97.3 | 95.0 | 95.0 | 97.6 | |||||||
| 4 | 30 | 332.806 | 334.251 | 429.511 | 0.022 | 73.9 | 61.8 | 61.8 | 87.9 | ||
| 50 | 0.003 | 87.0 | 85.0 | 85.0 | 97.9 | ||||||
| 100 | 99.1 | 99.1 | 99.1 | 100.0 | |||||||
| 0.6 | 3 | 30 | 0.004 | 99.7 | 80.2 | 80.2 | 80.5 | ||||
| 50 | 100.0 | 93.1 | 93.1 | 93.1 | |||||||
| 100 | 100.0 | 99.9 | 99.9 | 99.9 | |||||||
| 4 | 30 | 99.9 | 98.8 | 98.7 | 98.7 | ||||||
| 50 | 100.0 | 100.0 | 100.0 | 100.0 | |||||||
| 100 | 100.0 | 100.0 | 100.0 | 100.0 | |||||||
| 0.8 | 0.4 | 3 | 30 | 3.36 × (2.53 × ) | 3.37 × (2.56 × ) | 3.82 × (2.86 × ) | 99.5 | 99.3 | 99.3 | 99.8 | |
| 50 | 6.33 × (4.93 × ) | 6.33 × (4.93 × ) | 7.83 × (6.13 × ) | 100.0 | 100.0 | 100.0 | 100.0 | ||||
| 100 | 7.97 × (1.02 × ) | 7.95 × (1.02 × ) | 1.04 × (1.32 × ) | <2 | 100.0 | 100.0 | 100.0 | 100.0 | |||
| 4 | 30 | 1.10 × (1.12 × ) | 1.10 × (1.13 × ) | 1.11 × (1.10 × ) | 99.7 | 99.7 | 99.7 | 100.0 | |||
| 50 | 5.00 × (6.96 × ) | 4.99 × (6.95 × ) | 5.48 × (7.52 × ) | 100.0 | 100.0 | 100.0 | 100.0 | ||||
| 100 | 4.06 × (4.70 × ) | 4.05 × (4.69 × ) | 5.01 × (5.78 × ) | <2 | 100.0 | 100.0 | 100.0 | 100.0 | |||
| 0.6 | 3 | 30 | 362.850 (2.40 × ) | 363.706 (2.41 × ) | 527.645 (3.45 × ) | 0.014 | 70.6 | 67.0 | 67.0 | 96.5 | |
| 50 | 1.38 × (1.03 × ) | 1.38 × (1.03 × ) | 2.06 × (1.54 × ) | 0.002 | 85.9 | 86.9 | 85.9 | 99.0 | |||
| 100 | 2.87 × (2.80 × ) | 2.87 × (2.80 × ) | 4.33 × (4.23 × ) | 98.1 | 99.0 | 98.1 | 99.1 | ||||
| 4 | 30 | 444.102 (2.62 × ) | 444.267 (2.62 × ) | 618.955 (3.62 × ) | 0.011 | 69.3 | 68.0 | 68.0 | 98.8 | ||
| 50 | 1.98 × (2.26 × ) | 1.99 × (2.27 × ) | 2.83 × (3.21 × ) | 0.001 | 88.5 | 88.5 | 88.5 | 100.0 | |||
| 100 | 1.28 × (1.47 × ) | 1.29 × (1.48 × ) | 1.87 × (2.15 × ) | 98.7 | 99.1 | 98.7 | 99.6 | ||||
| 0.8 | 3 | 30 | 0.316 (0.350) | 0.313 (0.345) | 0.327 (0.439) | 0.497 | 6.6 | 4.3 | 93.4 | 97.7 | |
| 50 | 0.259 (0.361) | 0.258 (0.357) | 0.257 (0.438) | 0.422 | 5.6 | 6.0 | 94.0 | 99.6 | |||
| 100 | 0.224 (0.618) | 0.223 (0.610) | 0.201 (0.431) | 0.418 | 3.9 | 7.3 | 92.7 | 96.6 | |||
| 4 | 30 | 0.315 (0.354) | 0.311 (0.347) | 0.307 (0.398) | 0.596 | 4.4 | 4.1 | 95.6 | 99.6 | ||
| 50 | 0.273 (0.399) | 0.271 (0.393) | 0.259 (0.436) | 0.457 | 5.9 | 5.9 | 94.0 | 100.0 | |||
| 100 | 0.220 (0.487) | 0.219 (0.486) | 0.201 (0.413) | 0.526 | 4.0 | 5.9 | 94.1 | 98.1 | |||
| Posterior Probability; | ||||||
|---|---|---|---|---|---|---|
| 0.6 | 3 | 20 | 0.031 (0.048) | 0.085 (0.130) | 0.451 (0.331) | 0.711 (0.303) |
| 30 | 0.016 (0.052) | 0.053 (0.098) | 0.471 (0.343) | 0.794 (0.270) | ||
| 50 | 0.006 (0.025) | 0.025 (0.064) | 0.512 (0.355) | 0.896 (0.203) | ||
| 4 | 20 | 0.024 (0.041) | 0.073 (0.127) | 0.441 (0.337) | 0.724 (0.308) | |
| 30 | 0.012 (0.023) | 0.044 (0.091) | 0.468 (0.353) | 0.807 (0.275) | ||
| 50 | 0.004 (0.005) | 0.021 (0.063) | 0.514 (0.364) | 0.906 (0.199) | ||
| 0.6 | 3 | 20 | 0.058 (0.078) | 0.131 (0.162) | 0.548 (0.316) | 0.807 (0.236) |
| 30 | 0.030 (0.048) | 0.088 (0.131) | 0.566 (0.323) | 0.868 (0.204) | ||
| 50 | 0.011 (0.015) | 0.042 (0.072) | 0.604 (0.330) | 0.945 (0.137) | ||
| 4 | 20 | 0.049 (0.078) | 0.115 (0.158) | 0.544 (0.327) | 0.821 (0.238) | |
| 30 | 0.024 (0.045) | 0.077 (0.123) | 0.564 (0.334) | 0.884 (0.201) | ||
| 50 | 0.009 (0.012) | 0.034 (0.057) | 0.608 (0.339) | 0.952 (0.133) | ||
| 0.7 | 3 | 20 | 0.107 (0.108) | 0.221 (0.201) | 0.679 (0.265) | 0.898 (0.147) |
| 30 | 0.059 (0.064) | 0.148 (0.160) | 0.696 (0.276) | 0.949 (0.097) | ||
| 50 | 0.022 (0.023) | 0.080 (0.116) | 0.743 (0.278) | 0.985 (0.047) | ||
| 4 | 20 | 0.090 (0.097) | 0.198 (0.197) | 0.676 (0.275) | 0.910 (0.148) | |
| 30 | 0.047 (0.050) | 0.129 (0.152) | 0.697 (0.285) | 0.961 (0.091) | ||
| 50 | 0.018 (0.018) | 0.069 (0.108) | 0.744 (0.288) | 0.991 (0.035) | ||
| p-Value | ||||
|---|---|---|---|---|
| 0.2 | 0.234 | 0.223 | 0.250 | 0.496 |
| 0.25 | 0.205 | 0.200 | 0.193 | 0.942 |
| 0.3 | 0.295 | 0.292 | 0.252 | 0.444 |
| 0.35 | 0.631 | 0.633 | 0.504 | 0.156 |
| 0.4 | 1.931 | 1.958 | 1.474 | 0.041 |
| 0.45 | 8.332 | 8.517 | 6.207 | 0.008 |
| 0.5 | 51.140 | 52.627 | 37.895 | 0.001 |
| 0.55 | 459.887 | 475.909 | 345.409 | |
| 0.6 | ||||
| 0.65 | ||||
| 0.7 | ||||
| 0.75 | ||||
| 0.8 |
| p-Value | ||||
|---|---|---|---|---|
| 0.2 | <2 | |||
| 0.25 | <2 | |||
| 0.3 | <2 | |||
| 0.35 | ||||
| 0.4 | ||||
| 0.45 | 113.364 | 114.286 | 108.393 | |
| 0.5 | 0.588 | 0.595 | 0.557 | 0.057 |
| 0.55 | 0.094 | 0.095 | 0.090 | 0.959 |
| 0.6 | 0.477 | 0.484 | 0.470 | 0.067 |
| 0.65 | 101.381 | 102.990 | 105.429 | |
| 0.7 | ||||
| 0.75 | ||||
| 0.8 | <2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hong, J.; Kim, K.; Kim, S.W. Default Priors in a Zero-Inflated Poisson Distribution: Intrinsic Versus Integral Priors. Mathematics 2025, 13, 773. https://doi.org/10.3390/math13050773
Hong J, Kim K, Kim SW. Default Priors in a Zero-Inflated Poisson Distribution: Intrinsic Versus Integral Priors. Mathematics. 2025; 13(5):773. https://doi.org/10.3390/math13050773
Chicago/Turabian StyleHong, Junhyeok, Kipum Kim, and Seong W. Kim. 2025. "Default Priors in a Zero-Inflated Poisson Distribution: Intrinsic Versus Integral Priors" Mathematics 13, no. 5: 773. https://doi.org/10.3390/math13050773
APA StyleHong, J., Kim, K., & Kim, S. W. (2025). Default Priors in a Zero-Inflated Poisson Distribution: Intrinsic Versus Integral Priors. Mathematics, 13(5), 773. https://doi.org/10.3390/math13050773






