Inverse Acoustic Scattering from a Bounded Homogeneous Penetrable Obstacle
Abstract
:1. Introduction
2. Problem Formulation
3. From Time Domain to Laplace Domain
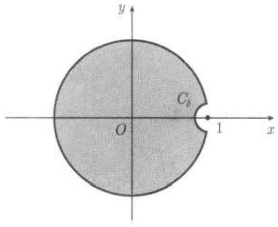
4. Parameterization of the Curve
- where is known as Bessel function of order zero.
- Correspondingly,
- Here, we define , , denote Euler’s constant.
- For ,
- are both absolutely convergent.
- For we consider the second part of :
5. Time Discretization
6. The Inverse Scheme
6.1. Nonlinear Integral Equations
- where
- For simplicity, we use symbols to represent the retarded single layer potential (3)
6.2. Fully Discretization
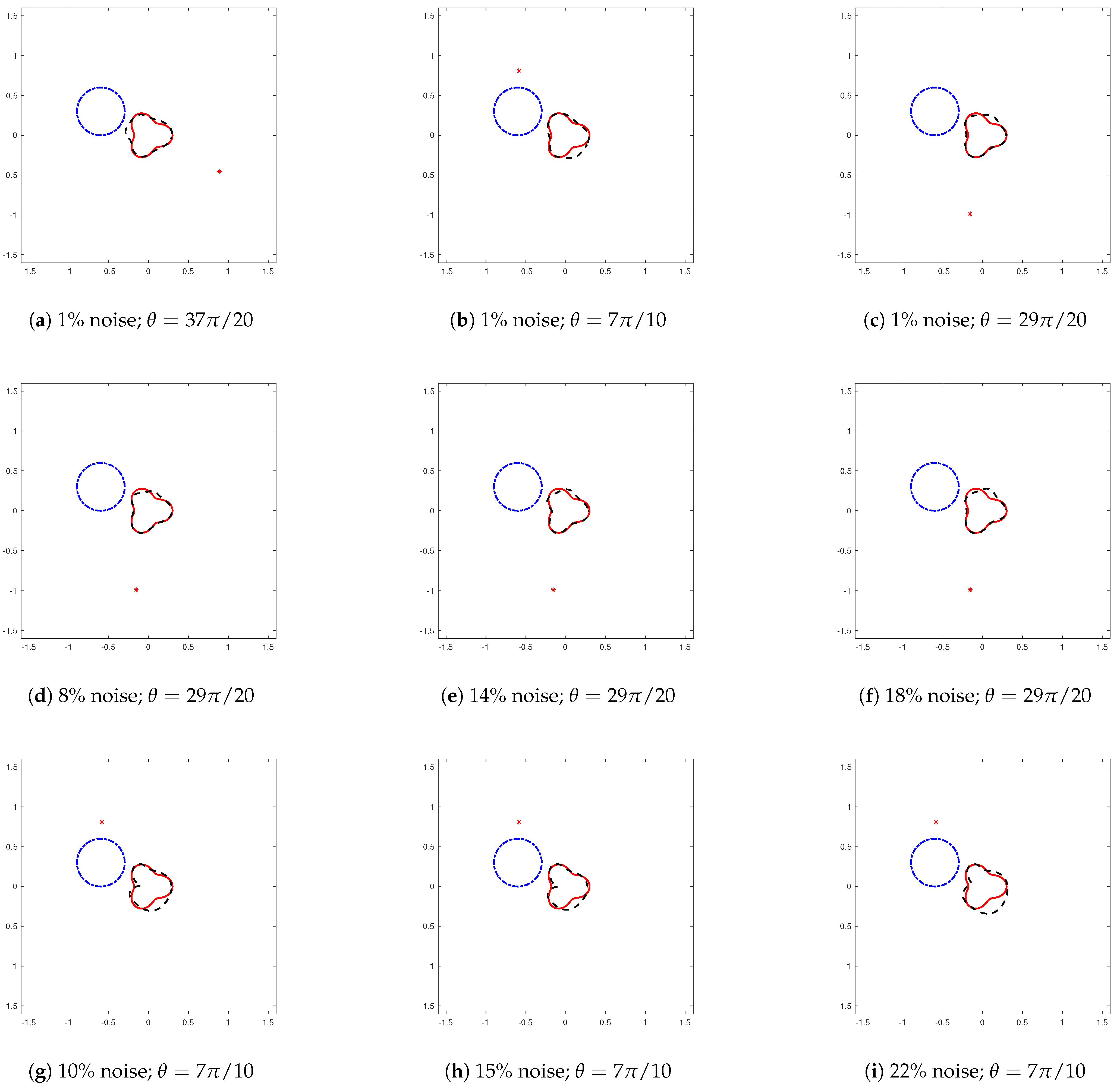
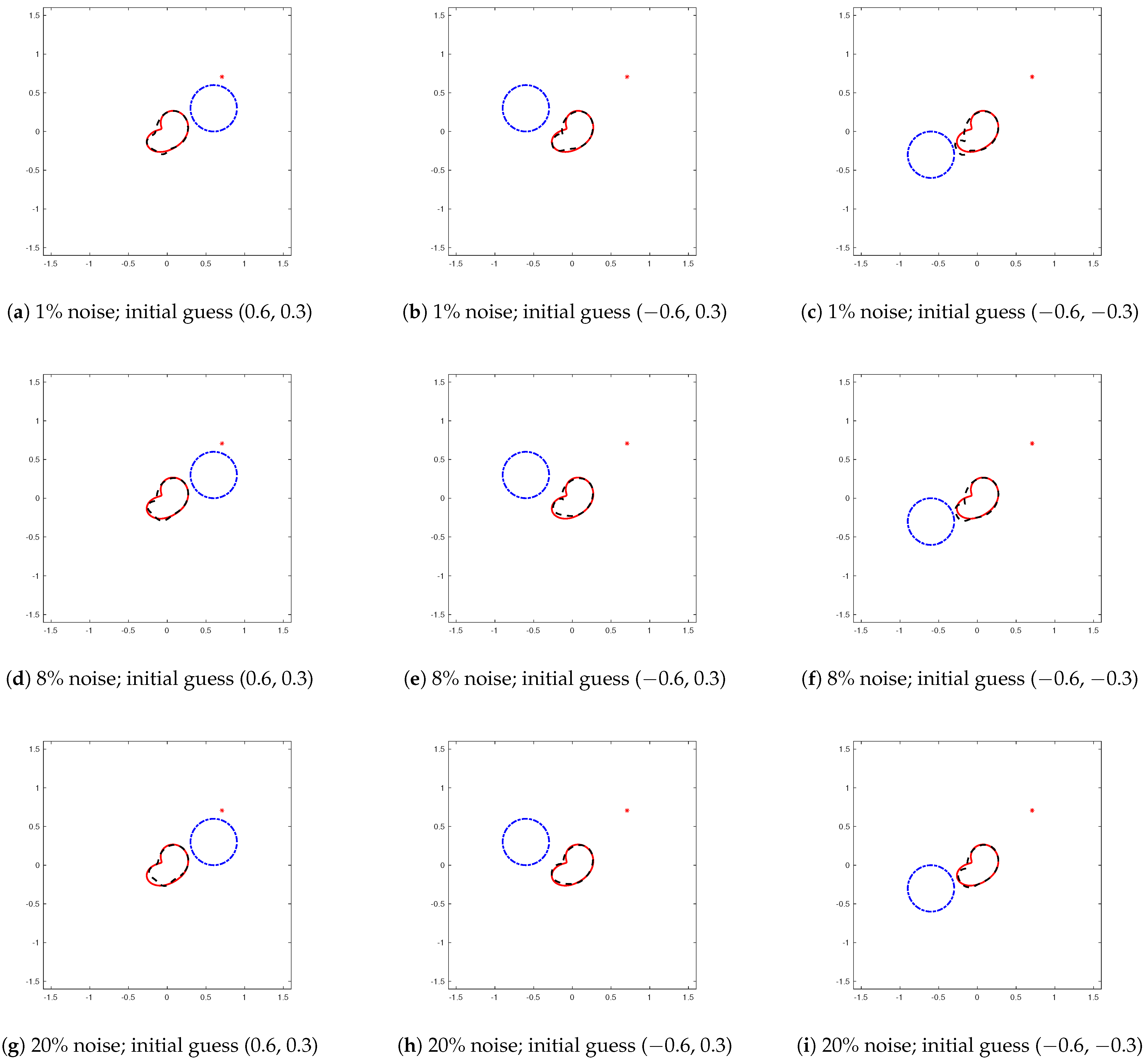
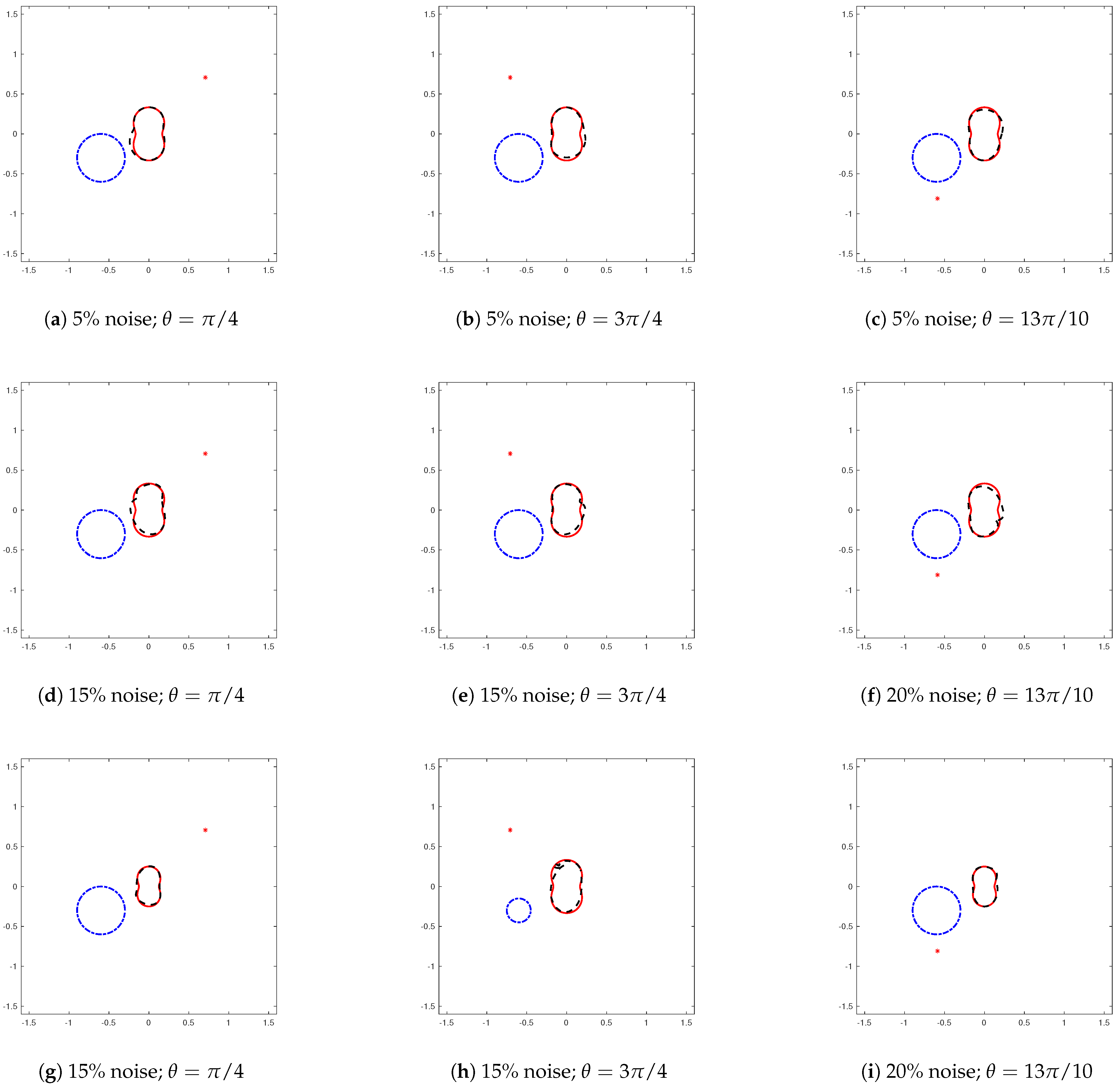
7. Numerical Experiments
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
- Let , for , we have
- with order . Let
- Then, we have
- (2) if and , is a pole of order .
- Let , then we havethus,(3) if and , the integral is equal to zero. and for , the integral is equal to . □
References
- Ikehata, M. The framework of the enclosure method with dynamical data and its applications. Inverse Probl. 2011, 27, 065005. [Google Scholar] [CrossRef]
- Ikehata, M.; Ohe, T. A numerical method for finding the convex hull of polygonal cavities using the enclosure method. Inverse Probl. 2002, 18, 111. [Google Scholar] [CrossRef]
- Ikehata, M. The enclosure method for inverse obstacle scattering using a single electromagnetic wave in time domain. arXiv 2013, arXiv:1401.0083. [Google Scholar] [CrossRef]
- Ikehata, M.; Kawashita, M. The enclosure method for the heat equation. Inverse Probl. 2009, 25, 075005. [Google Scholar] [CrossRef]
- Ikehata, M. The enclosure method for inverse obstacle scattering problems with dynamical data over a finite time interval. Inverse Probl. 2010, 26, 055010. [Google Scholar] [CrossRef]
- Ikehata, M. Revisiting the probe and enclosure methods. Inverse Probl. 2022, 38, 075009. [Google Scholar] [CrossRef]
- Haddar, H.; Lechleiter, A.; Marmorat, S. An improved time domain linear sampling method for Robin and Neumann obstacles. Appl. Anal. 2014, 93, 369–390. [Google Scholar] [CrossRef]
- Guo, Y.; Monk, P.; Colton, D. Toward a time domain approach to the linear sampling method. Inverse Probl. 2013, 29, 095016. [Google Scholar] [CrossRef]
- Guo, Y.; Monk, P.; Colton, D. The linear sampling method for sparse small aperture data. Appl. Anal. 2016, 95, 1599–1615. [Google Scholar] [CrossRef]
- Cakoni, F.; Colton, D. On the mathematical basis of the linear sampling method. Georgian Math. J. 2003, 411–425. [Google Scholar] [CrossRef]
- Arens, T.; Lechleiter, A. The linear sampling method revisited. J. Integral Equ. Appl. 2009, 21, 179–202. [Google Scholar] [CrossRef]
- Arens, T. Why linear sampling works. Inverse Probl. 2003, 20, 163. [Google Scholar] [CrossRef]
- Cakoni, F.; Colton, D. The linear sampling method for cracks. Inverse Probl. 2003, 19, 279. [Google Scholar] [CrossRef]
- Chen, Q.; Haddar, H.; Lechleiter, A.; Monk, P. A sampling method for inverse scattering in the time domain. Inverse Probl. 2010, 26, 085001. [Google Scholar] [CrossRef]
- Cakoni, F.; Monk, P.; Selgas, V. Analysis of the linear sampling method for imaging penetrable obstacles in the time domain. Anal. PDE 2021, 14, 667–688. [Google Scholar] [CrossRef]
- Cakoni, F.; Haddar, H.; Lechleiter, A. On the factorization method for a far field inverse scattering problem in the time domain. SIAM J. Math. Anal. 2019, 51, 854–872. [Google Scholar] [CrossRef]
- Haddar, H.; Liu, X. A time domain factorization method for obstacles with impedance boundary conditions. Inverse Probl. 2020, 36, 105011. [Google Scholar] [CrossRef]
- Litynskyy, S.; Muzychuk, Y.; Muzychuk, A. On the numerical solution of the initial-boundary value problem with neumann condition for the wave equation by the use of the laguerre transform and boundary elements method. Acta Mech. Autom. 2016, 10, 285–290. [Google Scholar] [CrossRef]
- Hlova, A.; Litynskyy, S.; Muzychuk Yu, A.; Muzychuk, A. Coupling of Laguerre transform and Fast BEM for solving Dirichlet initial-boundary value problems for the wave equation. J. Comput. Appl. Math 2018, 128, 42–60. [Google Scholar]
- Litynskyy, S.; Muzychuk, A. Solving of initial-boundary value problems for the wave equation using retarded surface potential and Laguerre transform. Mat. Stud. 2015, 44, 185–203. [Google Scholar] [CrossRef]
- Lubich, C. Convolution quadrature and discretized operational calculus. I. Numer. Math. 1988, 52, 129–145. [Google Scholar] [CrossRef]
- Lubich, C. Convolution quadrature and discretized operational calculus. II. Numer. Math. 1988, 52, 413–425. [Google Scholar] [CrossRef]
- Laliena, A.R.; Sayas, F.J. Theoretical aspects of the application of convolution quadrature to scattering of acoustic waves. Numer. Math. 2009, 112, 637–678. [Google Scholar] [CrossRef]
- Lubich, C. Convolution quadrature revisited. BIT Numer. Math. 2004, 44, 503–514. [Google Scholar] [CrossRef]
- Labarca, I.; Hiptmair, R. Acoustic scattering problems with convolution quadrature and the method of fundamental solutions. Commun. Comput. Phys. 2021, 30, 985–1008. [Google Scholar] [CrossRef]
- Aldahham, E.; Banjai, L. A modified convolution quadrature combined with the method of fundamental solutions and Galerkin BEM for acoustic scattering. arXiv 2022, arXiv:2203.00996. [Google Scholar]
- Ballani, J.; Banjai, L.; Sauter, S.A.; Veit, A. Numerical solution of exterior Maxwell problems by Galerkin BEM and Runge-Kutta convolution quadrature. Numer. Math. 2013, 123, 643–670. [Google Scholar] [CrossRef]
- Lubich, C. On the multistep time discretization of linear initial-boundary value problems and their boundary integral equations. Numer. Math. 1994, 67, 365–389. [Google Scholar] [CrossRef]
- Banjai, L.; Sauter, S. Rapid solution of the wave equation in unbounded domains. SIAM J. Numer. Anal. 2009, 47, 227–249. [Google Scholar] [CrossRef]
- Banjai, L.; Lubich, C. An error analysis of Runge–Kutta convolution quadrature. BIT Numer. Math. 2011, 51, 483–496. [Google Scholar] [CrossRef]
- Zhao, L.; Dong, H.; Ma, F. Inverse obstacle scattering for acoustic waves in the time domain. Inverse Probl. Imaging 2021, 15, 1269. [Google Scholar] [CrossRef]
- Ivanyshyn, O.; Johansson, T. Nonlinear integral equation methods for the reconstruction of an acoustically sound-soft obstacle. J. Integral Equ. Appl. 2007, 19, 289–308. [Google Scholar] [CrossRef]
- Johansson, T.; Sleeman, B.D. Reconstruction of an acoustically sound-soft obstacle from one incident field and the far-field pattern. IMA J. Appl. Math. 2007, 72, 96–112. [Google Scholar] [CrossRef]
- Sayas, F.J. Retarded Potentials and Time Domain Boundary Integral Equations: A Road Map; Springer: Berlin/Heidelberg, Germany, 2016; Volume 50. [Google Scholar]
- Dong, H.; Lai, J.; Li, P. A highly accurate boundary integral method for the elastic obstacle scattering problem. Math. Comput. 2021, 90, 2785–2814. [Google Scholar] [CrossRef]
- Kress, R. Linear Integral Equations; Springer: Berlin/Heidelberg, Germany, 1989. [Google Scholar]
- Kirsch, A. An Introduction to the Mathematical Theory of Inverse Problems; Springer: Berlin/Heidelberg, Germany, 2011; Volume 120. [Google Scholar]
- Dong, H.; Zhang, D.; Guo, Y. A reference ball based iterative algorithm for imaging acoustic obstacle from phaseless far-field data. arXiv 2018, arXiv:1804.05062. [Google Scholar] [CrossRef]
- Courant, R.; Hilbert, D. Methoden der Mathematischen Physik; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Dennery, P.; Krzywicki, A. Mathematics for Physicists; Courier Corporation: Chelmsford, MA, USA, 2012. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qu, Z.; Ma, F. Inverse Acoustic Scattering from a Bounded Homogeneous Penetrable Obstacle. Mathematics 2025, 13, 771. https://doi.org/10.3390/math13050771
Qu Z, Ma F. Inverse Acoustic Scattering from a Bounded Homogeneous Penetrable Obstacle. Mathematics. 2025; 13(5):771. https://doi.org/10.3390/math13050771
Chicago/Turabian StyleQu, Zhenpu, and Fuming Ma. 2025. "Inverse Acoustic Scattering from a Bounded Homogeneous Penetrable Obstacle" Mathematics 13, no. 5: 771. https://doi.org/10.3390/math13050771
APA StyleQu, Z., & Ma, F. (2025). Inverse Acoustic Scattering from a Bounded Homogeneous Penetrable Obstacle. Mathematics, 13(5), 771. https://doi.org/10.3390/math13050771