Euler–Riemann–Dirichlet Lattices: Applications of η(s) Function in Physics
Abstract
1. Introduction
2. The Function and Its Relation to
3. Euler–Riemann–Dirichlet Lattices
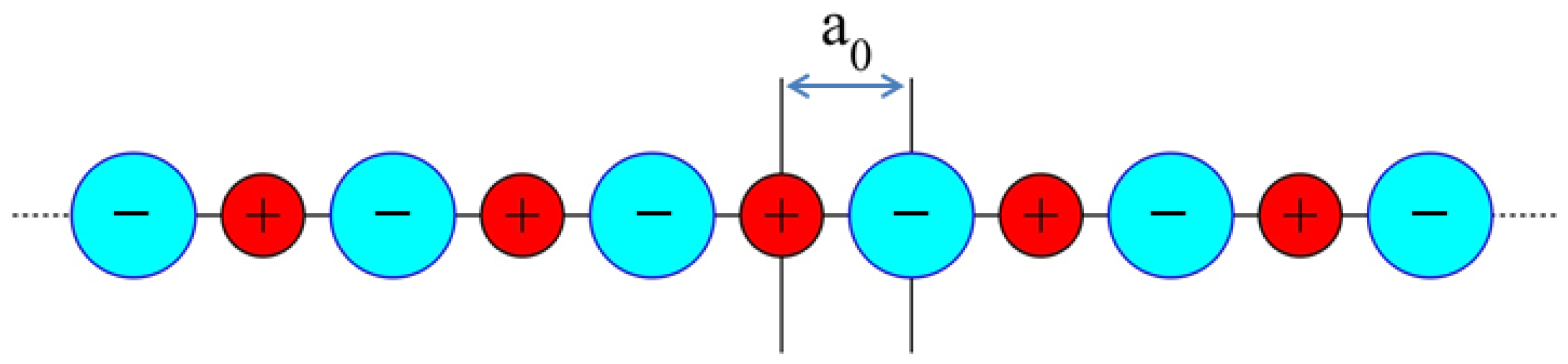
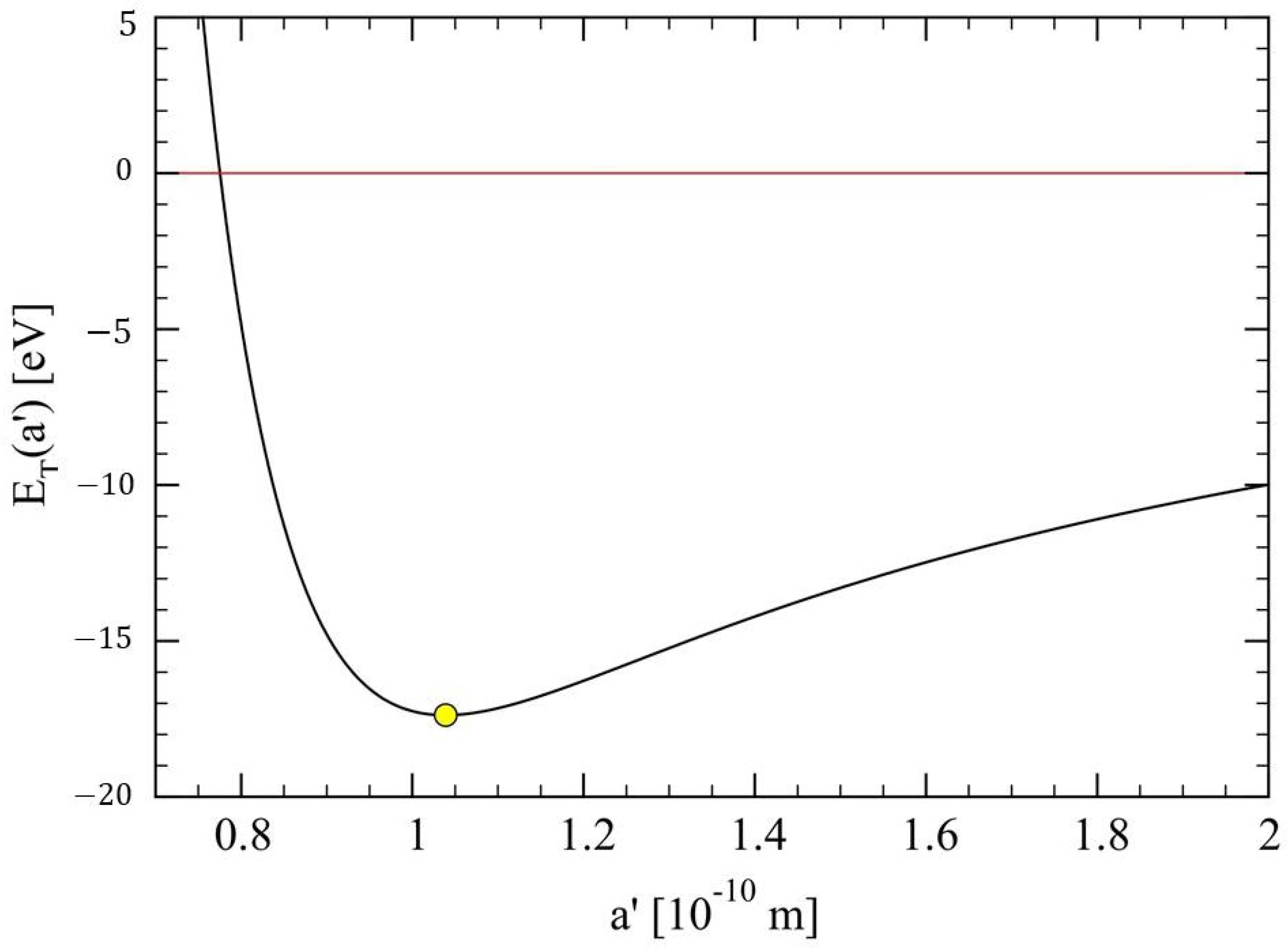
3.1. Standard Ionic Lattices
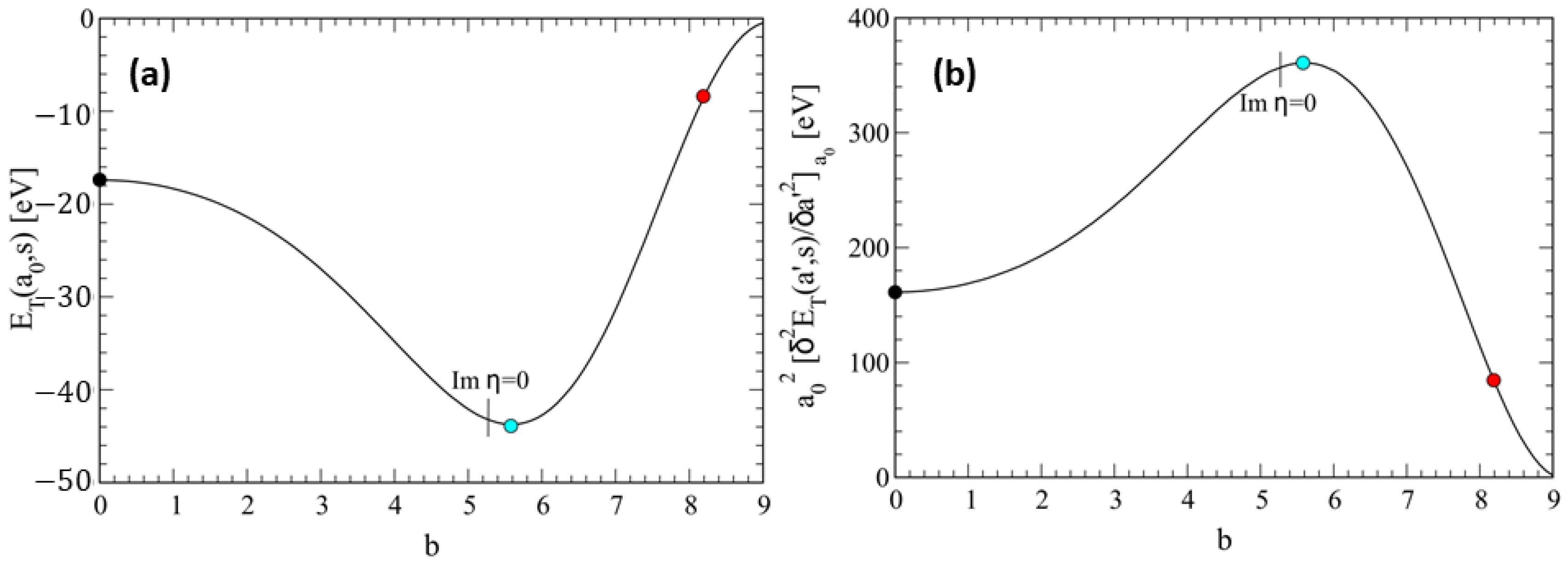
3.2. ERD Complex Lattices: Beyond the Standard Picture
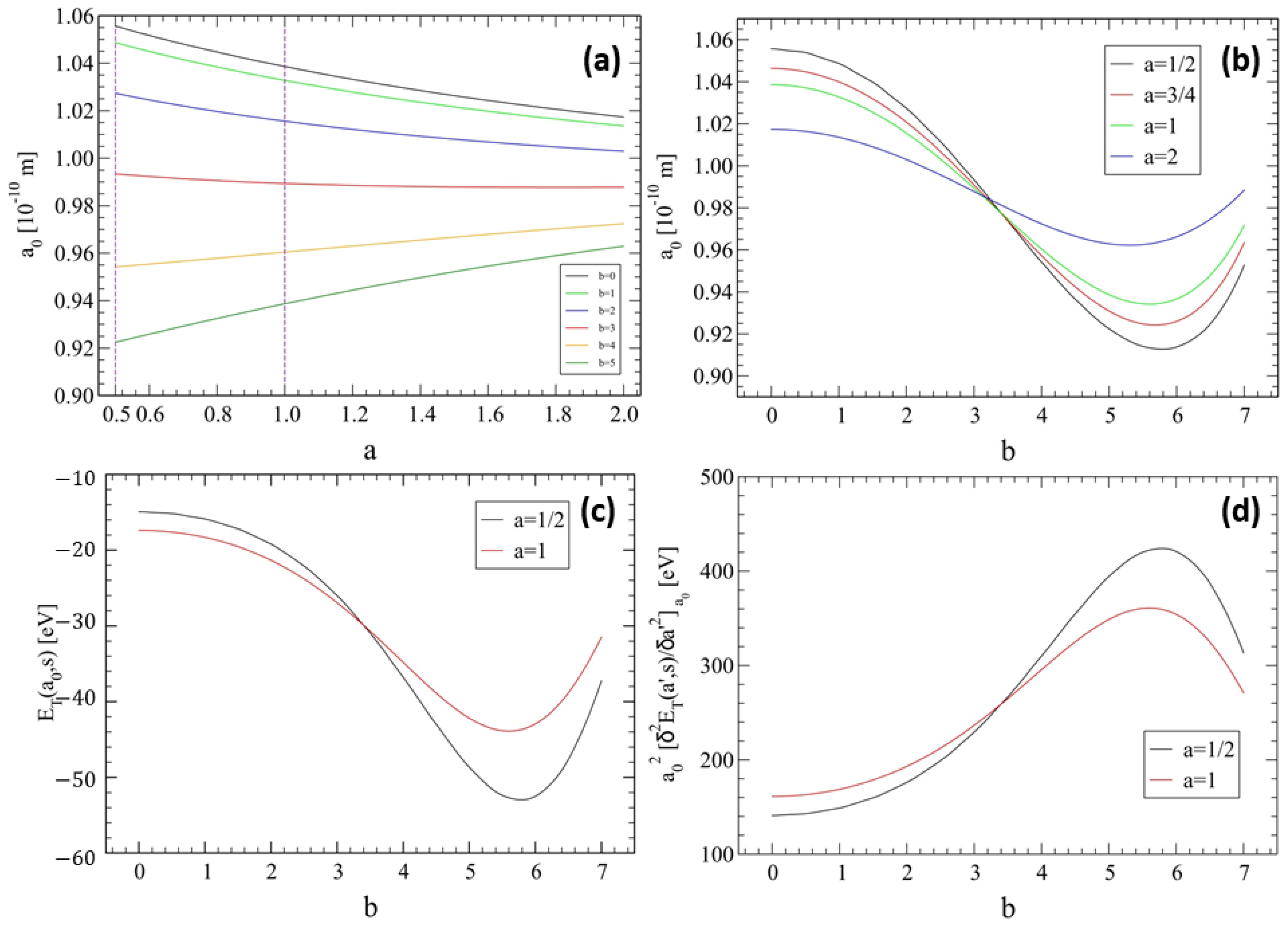
4. The ERD Complex Lattices Within the Range:
5. Concluding Remarks
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
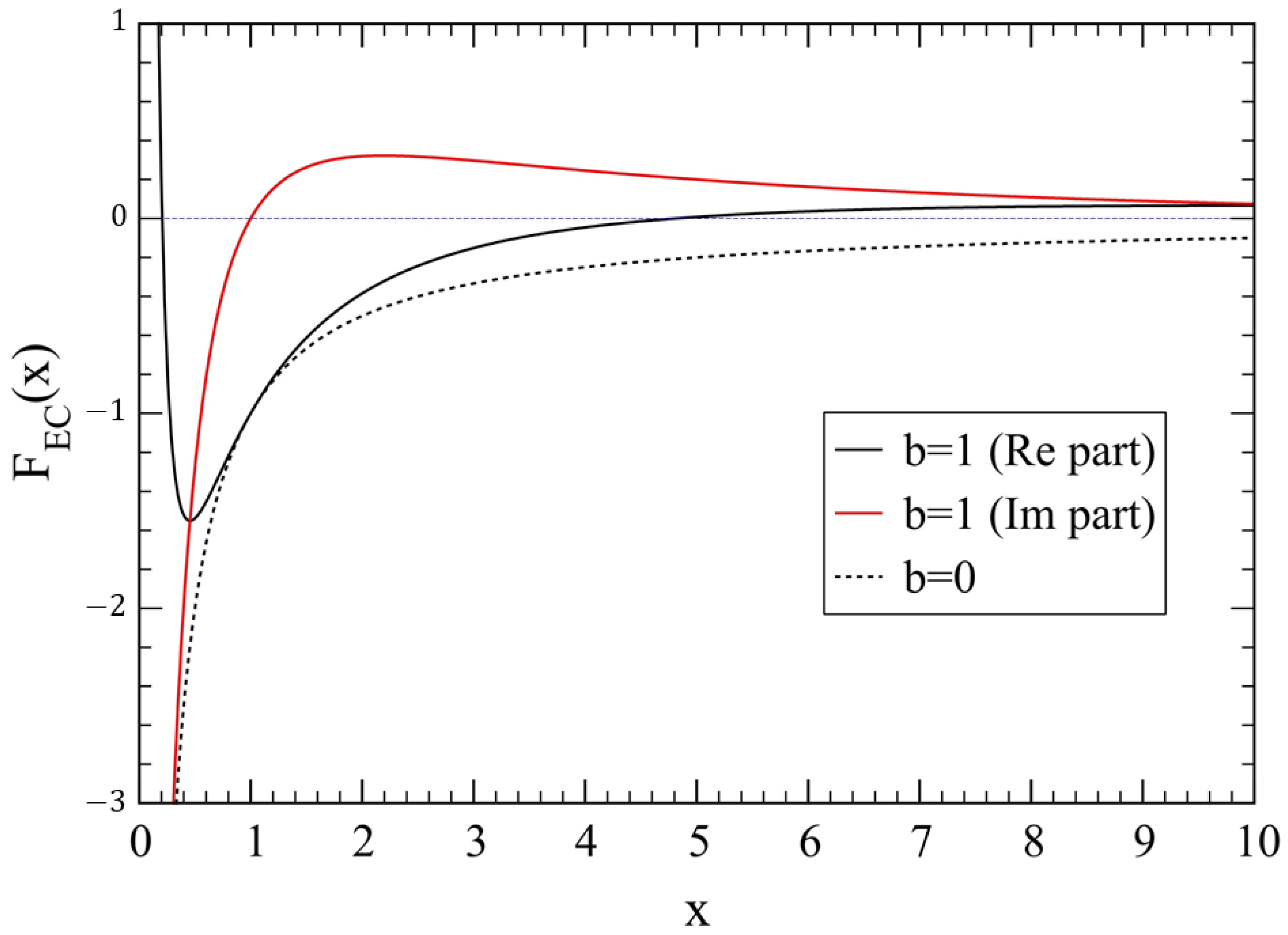
Appendix A. The Dirichlet Function η(s)
Appendix B. Some Properties of Prime Numbers and the Riemann Hypothesis
Appendix B.1. Numerical Results on Prime Numbers
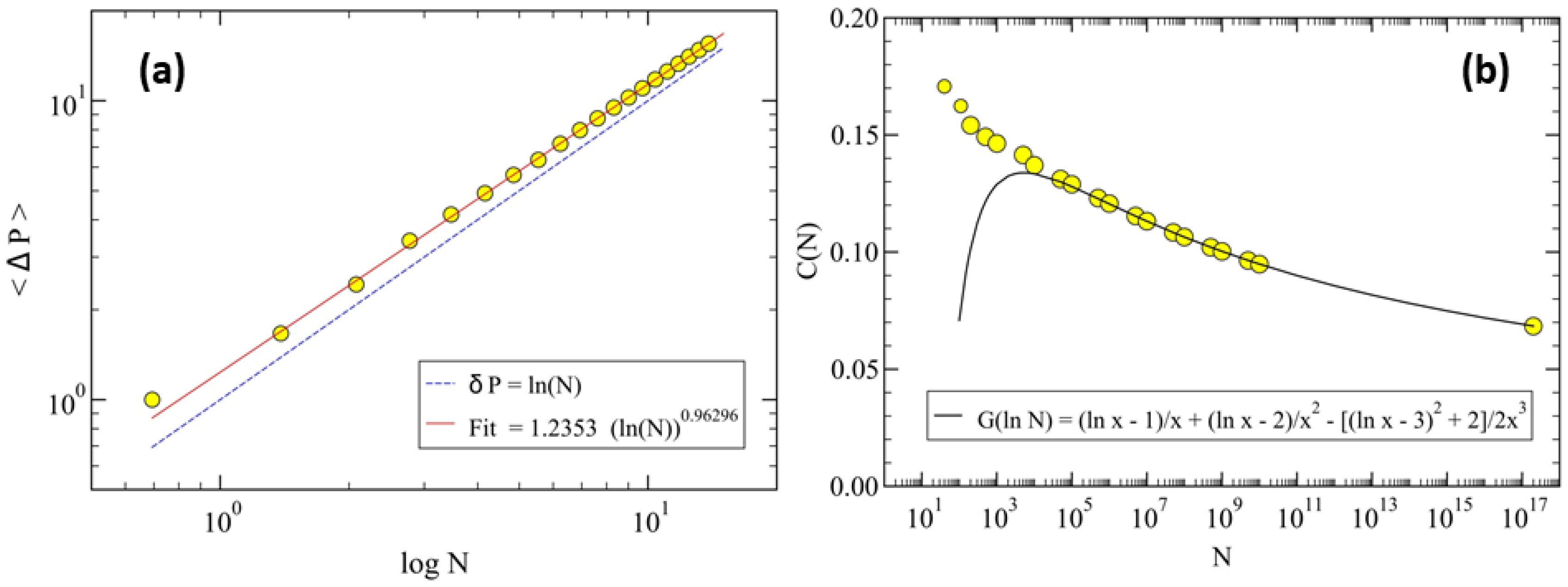
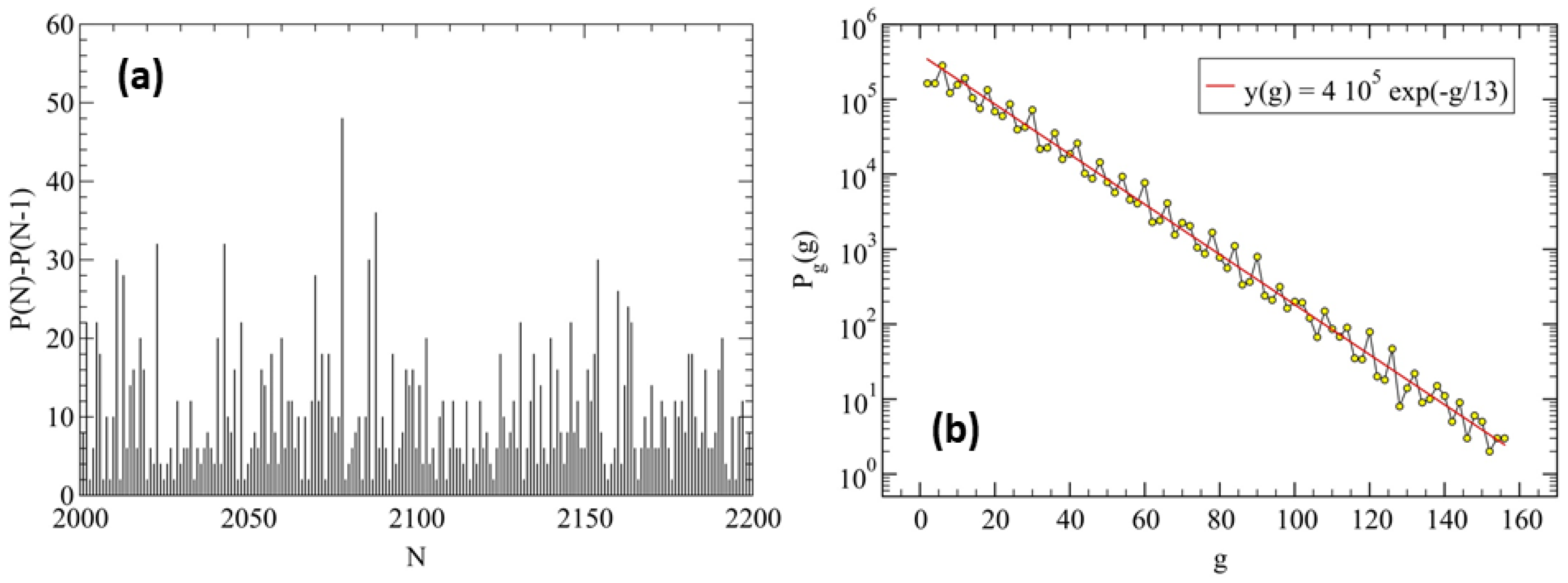
Appendix B.2. Fluctuation Analysis
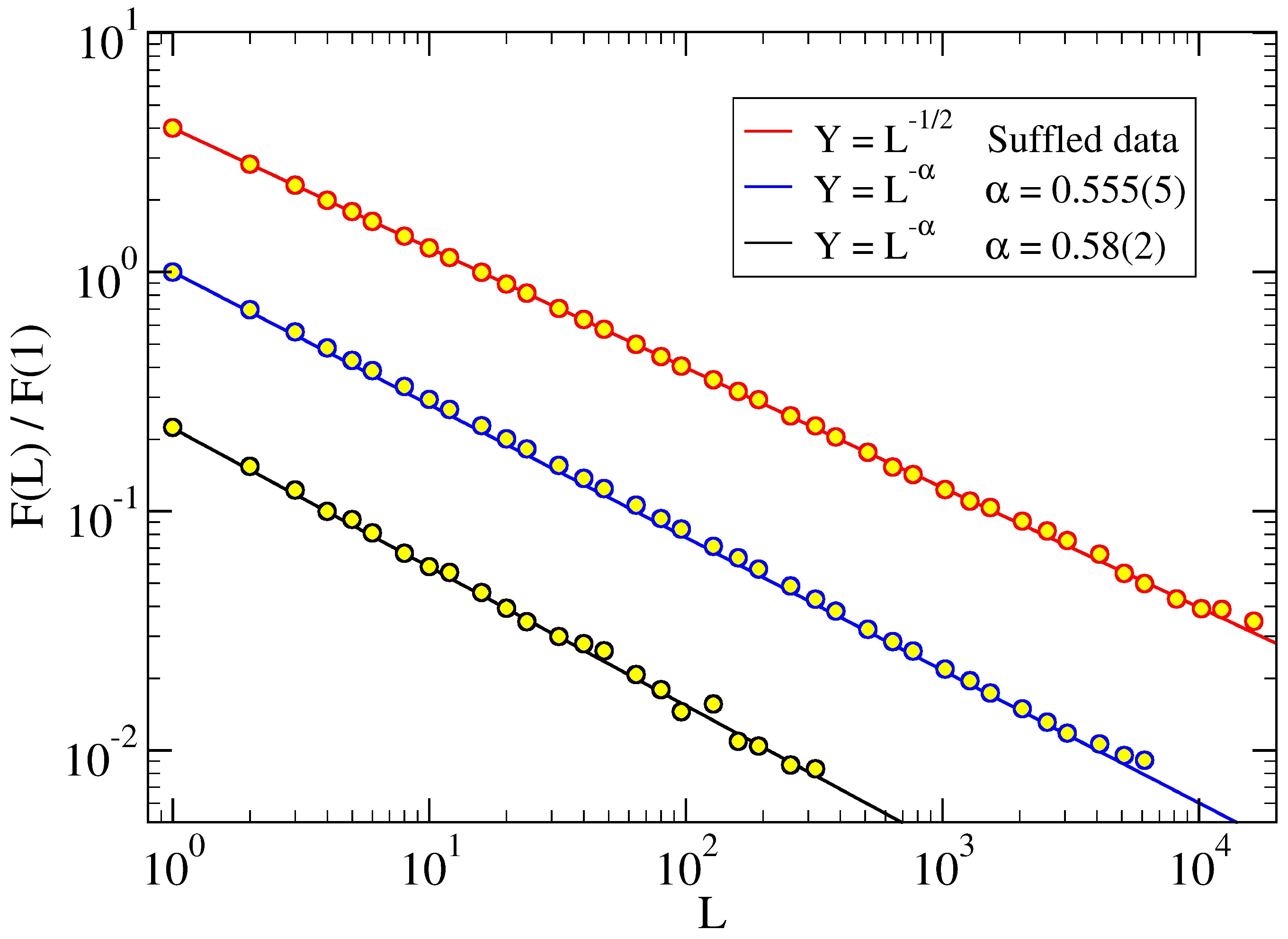
Appendix C. The Non-Trivial Zeros of ζ(s) Within: 0 < a < 1
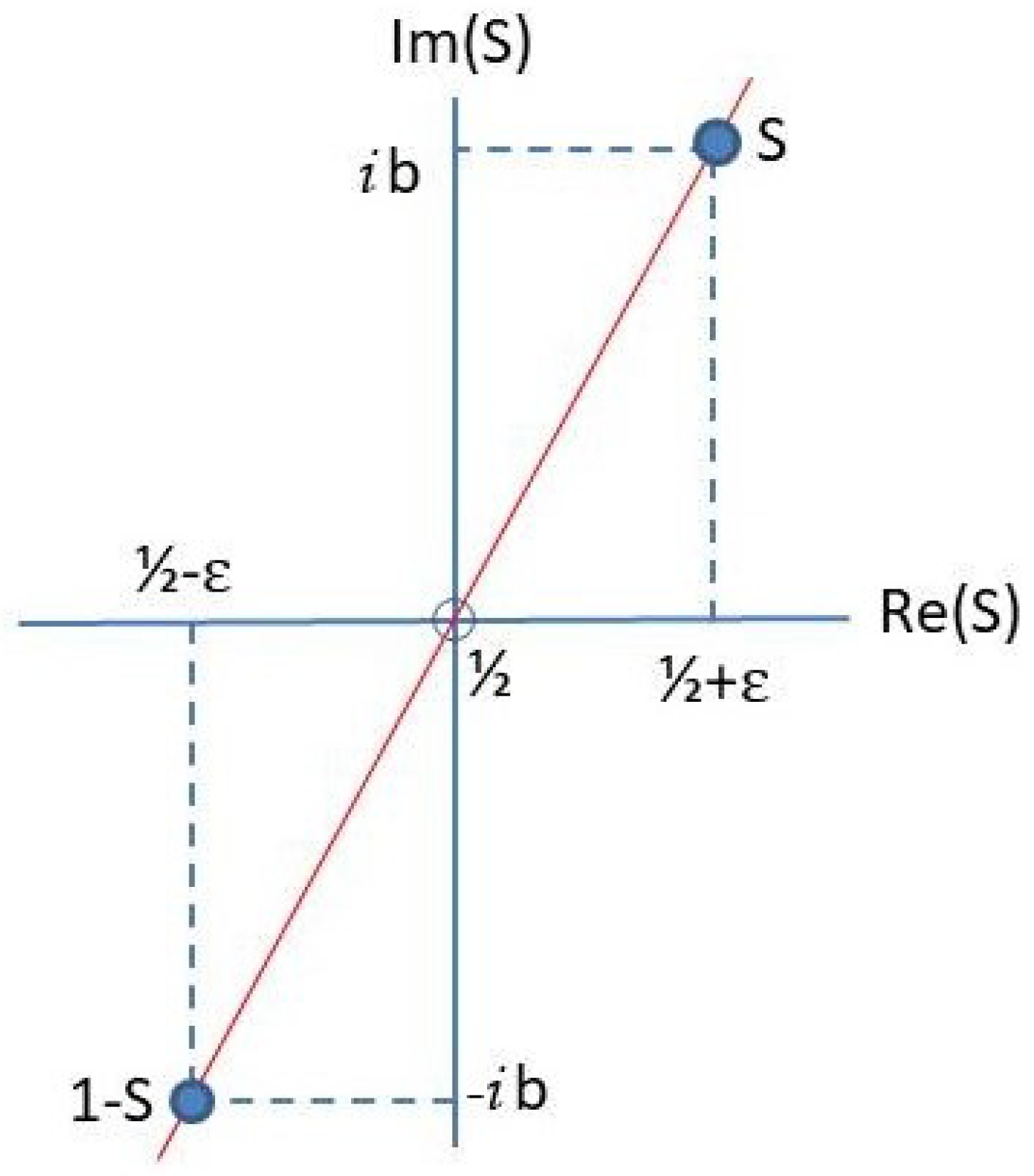
Appendix C.1. The Case a = 1/2
Appendix C.2. The General Case 0 < a < 1
References
- Schumayer, D.; Hutchinson, D.A. Colloquium: Physics of the Riemann Hypothesis. Rev. Mod. Phys. 2011, 83, 307–330. [Google Scholar] [CrossRef]
- Remmen, G.N. Amplitudes and the Riemann zeta function. Phys. Rev. Lett. 2021, 127, 241602. [Google Scholar] [CrossRef] [PubMed]
- Wolf, M. Will a physicist prove the Riemann hypothesis? Rep. Prog. Phys. 2020, 83, 036001. [Google Scholar] [CrossRef] [PubMed]
- Barbarani, V. A Quantum Model of the Distribution of Prime Numbers and the Riemann hypothesis. Int. J. Theor. Phys. 2020, 59, 2425–2470. [Google Scholar] [CrossRef]
- Tamburini, F.; Licata, I. Majorana quanta, string scattering, curved spacetimes and the Riemann Hypothesis. Phys. Scr. 2021, 96, 125276. [Google Scholar] [CrossRef]
- Abdelaziz, S.; Shaker, A.; Salah, M.M. Development of a New Zeta Formula and Its Role in Riemann Hypothesis and Quantum Physics. Mathematics 2023, 11, 3025. [Google Scholar] [CrossRef]
- Tschaffon, M.E.N.; Tkáčová, I.; Maier, H.; Schleich, W.P. A Primer on the Riemann Hypothesis. In Sketches of Physics; Citro, R., Lewenstein, M., Rubio, A., Schleich, W.P., Wells, J.D., Zank, G.P., Eds.; Lecture Notes in Physics 1000; Springer: Cham, Switzerland, 2023; Chapter 7. [Google Scholar] [CrossRef]
- Schuch, D.; Chung, K.M.; Hartmann, H. Nonlinear Schrödinger-type field equation for the description of dissipative systems. I. Derivation of the nonlinear field equation and one-dimensional example. J. Math. Phys. 1983, 24, 1652–1660. [Google Scholar] [CrossRef]
- Bulyzhenkov, I.E. Complex charge densities unify particles with fields and gravitation with electricity. Bull. Lebedev Phys. Inst. 2016, 43, 138–142. [Google Scholar] [CrossRef]
- Ashida, Y.; Gong, Z.; Ueda, M. Non-Hermitian Physics. Adv. Phys. 2020, 69, 249–435. [Google Scholar] [CrossRef]
- Bender, C.M. Making sense of non-Hermitian Hamiltonians. Rep. Prog. Phys. 2007, 70, 947. [Google Scholar] [CrossRef]
- Matsoukas-Roubeas, A.S.; Roccati, F.; Cornelius, J.; Xu, Z.; Chenu, A.; del Campo, A. Non-Hermitian Hamiltonian deformations in quantum mechanics. J. High Energy Phys. 2023, 2023, 1–31. [Google Scholar] [CrossRef]
- LeClair, A. Riemann hypothesis and random walks: The zeta case. Symmetry 2021, 13, 2014. [Google Scholar] [CrossRef]
- Orús-Lacort, M.; Orús, R.; Jouis, C. Analyzing Riemann’s hypothesis. Ann. Math. Phys. 2023, 6, 75–82. [Google Scholar] [CrossRef]
- Silva, S.D. The Riemann Hypothesis: A Fresh and Experimental Exploration. J. Adv. Math. Comput. Sci. 2024, 39, 100–112. [Google Scholar] [CrossRef]
- Tosi, M.P. Cohesion of Ionic Solids in the Born Model. Solid State Phys. 1964, 16, 1–120. [Google Scholar] [CrossRef]
- Damm, J.Z.; Chvoj, Z. Optimization of Tosi-Fumi Ionic Radii for F.C.C. Alkali Halide Crystals. Phys. Status Solidi B 1982, 114, 413–418. [Google Scholar] [CrossRef]
- Roman, H.E. A Study of Physical Properties of Ionic Systems. Ph.D. Thesis, International School for Advanced Studies (I.S.A.S-S.I.S.S.A), Trieste, Italy, 1983. [Google Scholar] [CrossRef]
- Kittel, C. Introduction to Solid State Physics, 8th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005; Available online: https://www.wiley.com/en-us/Introduction+to+Solid+State+Physics%2C+8th+Edition-p-9780471415268 (accessed on 1 January 2025).
- Osler, T.J. Euler and the Functional Equation for the Zeta Function. Math. Sci. 2009, 34, 62–73. Available online: https://www.appliedprobability.org/publications/the-mathematical-scientist (accessed on 1 January 2025).
- Riemann, B. Ueber die Anzahl der Primzahlen unter einer gegebenen Grösse. Monatsberichte Berl. Akad. VII 1859, 136. Translated by Wilkins, D.R., On the number of prime numbers less than a given quantity. Available online: https://www.claymath.org/wp-content/uploads/2023/04/Wilkins-translation.pdf (accessed on 1 January 2025).
- Titchmarsh, E.C. The Theory of the Riemann Zeta Function, 2nd ed.; The Clarendon Press Oxford University Press: Oxford, UK, 1986. [Google Scholar]
- Hardy, G.H. Notes on some points in the integral calculus LV: On the integration of Fourier series. Messenger Math. 1922, 51, 186–192, 392. Available online: https://ia800208.us.archive.org/32/items/messengerofmathe5051cambuoft/messengerofmathe5051cambuoft.pdf (accessed on 1 January 2025).
- Borwein, P. An Efficient Algorithm for the Riemann Zeta Function. 1995. Available online: https://citeseerx.ist.psu.edu/document?repid=rep1&type=pdf&doi=d9c5d06fadb170ad82f7697c225a610a321d8d0a (accessed on 1 January 2025).
- Car, R.; Parrinello, M. Unified Approach for Molecular Dynamics and Density-Functional Theory. Phys. Rev. Lett. 1985, 55, 2471. [Google Scholar] [CrossRef] [PubMed]
- Borwein, J.M.; Glasser, M.L.; McPhedran, R.C.; Wan, J.G.; Zucker, I.J. Lattice Sums Then and Now (Encyclopedia of Mathematics and Its Applications No. 150); Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Broglia, R.A.; Colò, G.; Onida, G.; Roman, H.E. Solid State Physics of Finite Systems: Metal Clusters, Fullerenes, Atomic Wires, Advanced Texts in Physics; Springer: Berlin, Germany, 2004. [Google Scholar] [CrossRef]
- Ziman, J.M. Principles of the Theory of Solids; Cambridge University Press: Cambridge, UK, 1979. [Google Scholar]
- Zagier, D. The First 50 Million Prime Numbers. Math. Intell. 1977, 1 (Suppl. S2), 7–19. [Google Scholar] [CrossRef]
- Meer, R.V.D. Zeros of the Zeta Function. Ph.D. Thesis, Faculty of Science and Engineering, Mathematics and Applied Mathematics, University of Groningen, Groningen, The Netherlands, 2020. Available online: https://fse.studenttheses.ub.rug.nl/21726/1/bMATH_2020_MeerRvander.pdf (accessed on 1 January 2025).
- Apostol, T.M. Introduction to Analytic Number Theory; Springer: New York, NY, USA, 1976. [Google Scholar]
- Cesàro, E. Sur une formule empirique de M. Pervouchine. C. R. Math. Acad. Sci. Paris 1894, 119, 848–849. [Google Scholar]
- Jakimczuk, R. An approximate formula for prime numbers. Int. J. Contemp. Math. Sci. 2008, 3, 1069–1086. Available online: https://www.m-hikari.com/ijcms-password2008/21-24-2008/jakimczukIJCMS21-24-2008.pdf (accessed on 1 January 2025).
- Arias de Reyna, J.; Toulisse, J. The n-th prime asymptotically. J. ThéOrie Nombres Bordx. 2013, 25, 521–555. [Google Scholar] [CrossRef]
- Shanks, D. On maximal gaps between successive primes. Math. Comput. 1964, 18, 646–651. [Google Scholar] [CrossRef]
- Nicely, T. New maximal prime gaps and first occurrences. Math. Comput. 1999, 68, 1311–1315. [Google Scholar] [CrossRef]
- Koscielny-Bunde, E.; Bunde, A.; Havlin, S.; Roman, H.E.; Goldreich, Y.; Schellnhuber, H.J. Indication of a universal persistence law governing atmospheric variability. Phys. Rev. Lett. 1998, 81, 729. [Google Scholar] [CrossRef]
- Tosi, M.P. In Memoriam; Department of Physics, University of Trieste: Trieste, Italy, 2015; Available online: https://df.units.it/en/news/14180 (accessed on 1 January 2025).


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roman, H.E. Euler–Riemann–Dirichlet Lattices: Applications of η(s) Function in Physics. Mathematics 2025, 13, 570. https://doi.org/10.3390/math13040570
Roman HE. Euler–Riemann–Dirichlet Lattices: Applications of η(s) Function in Physics. Mathematics. 2025; 13(4):570. https://doi.org/10.3390/math13040570
Chicago/Turabian StyleRoman, Hector Eduardo. 2025. "Euler–Riemann–Dirichlet Lattices: Applications of η(s) Function in Physics" Mathematics 13, no. 4: 570. https://doi.org/10.3390/math13040570
APA StyleRoman, H. E. (2025). Euler–Riemann–Dirichlet Lattices: Applications of η(s) Function in Physics. Mathematics, 13(4), 570. https://doi.org/10.3390/math13040570





