Effect of Rotation in Radial Microwave Irradiation: A Numerical Approach
Abstract
1. Introduction
2. Mathematical Model
2.1. Initial Conditions
2.2. Numerical Implementation
2.2.1. Temporal Discretization and Projection Scheme
- A predictor velocity field is obtained from Equation (22) by including the predictor pressure .
- In a final step, the systemis solved to obtain and .
2.2.2. Spatial Discretization
3. Results and Discussion
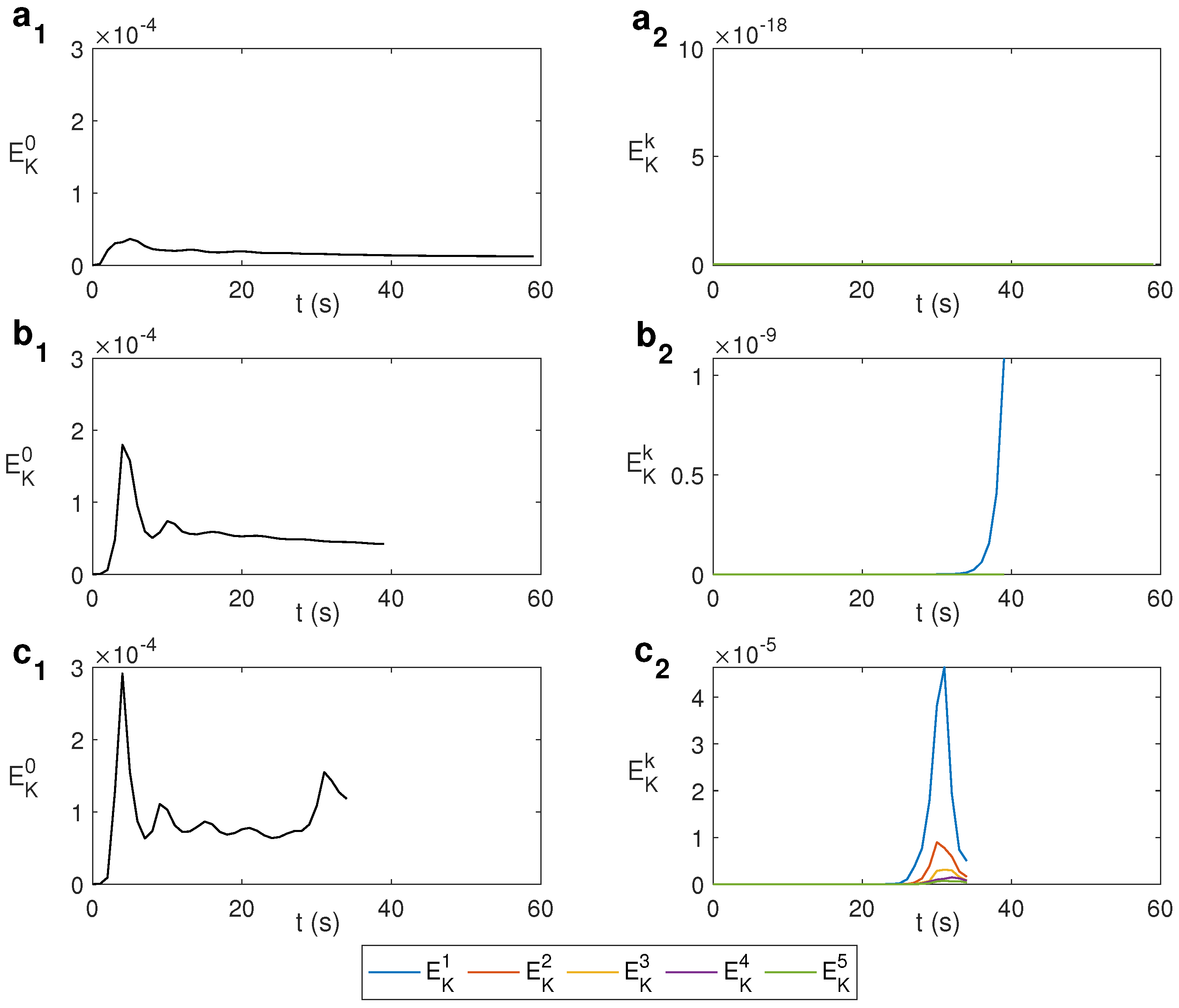
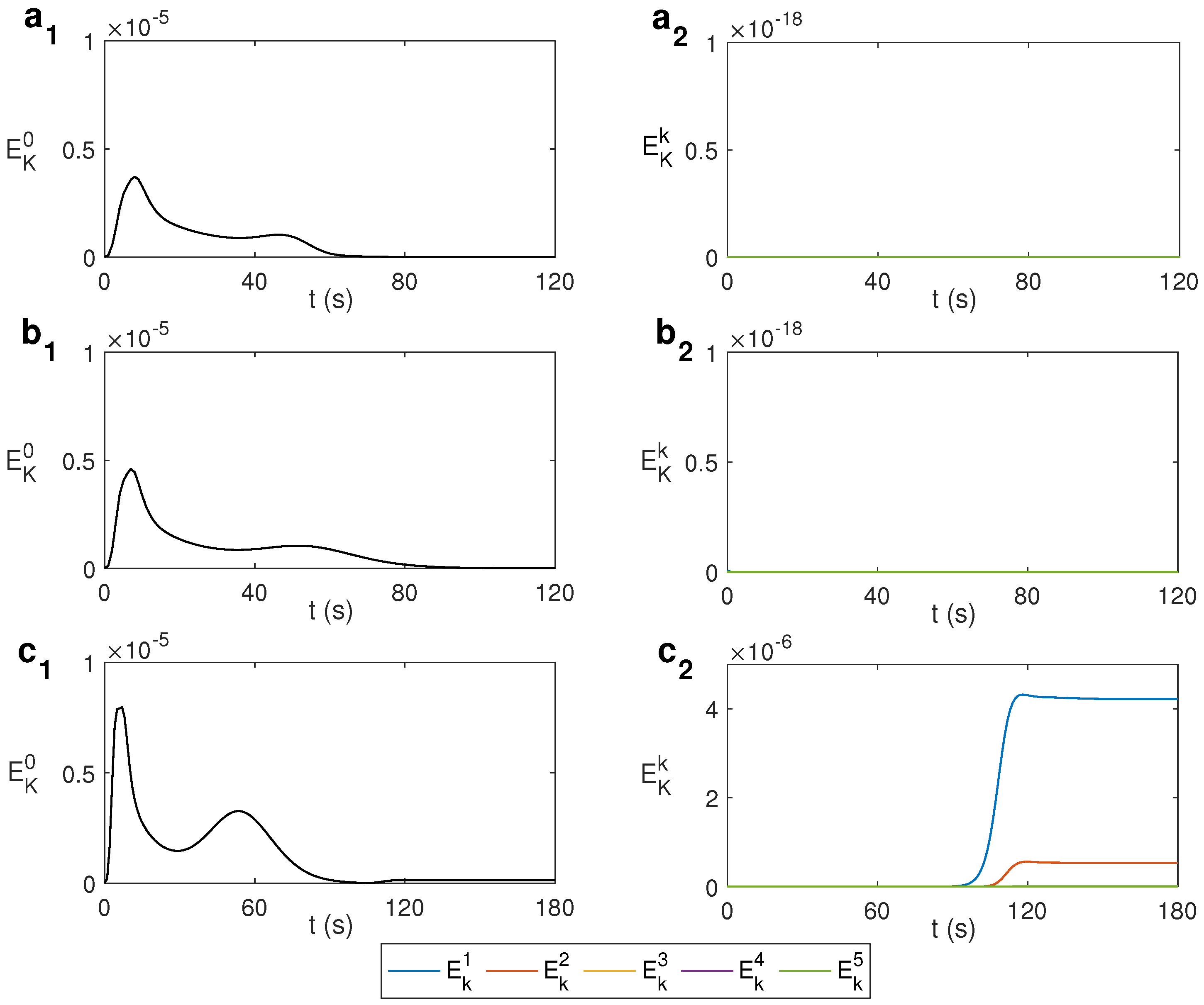
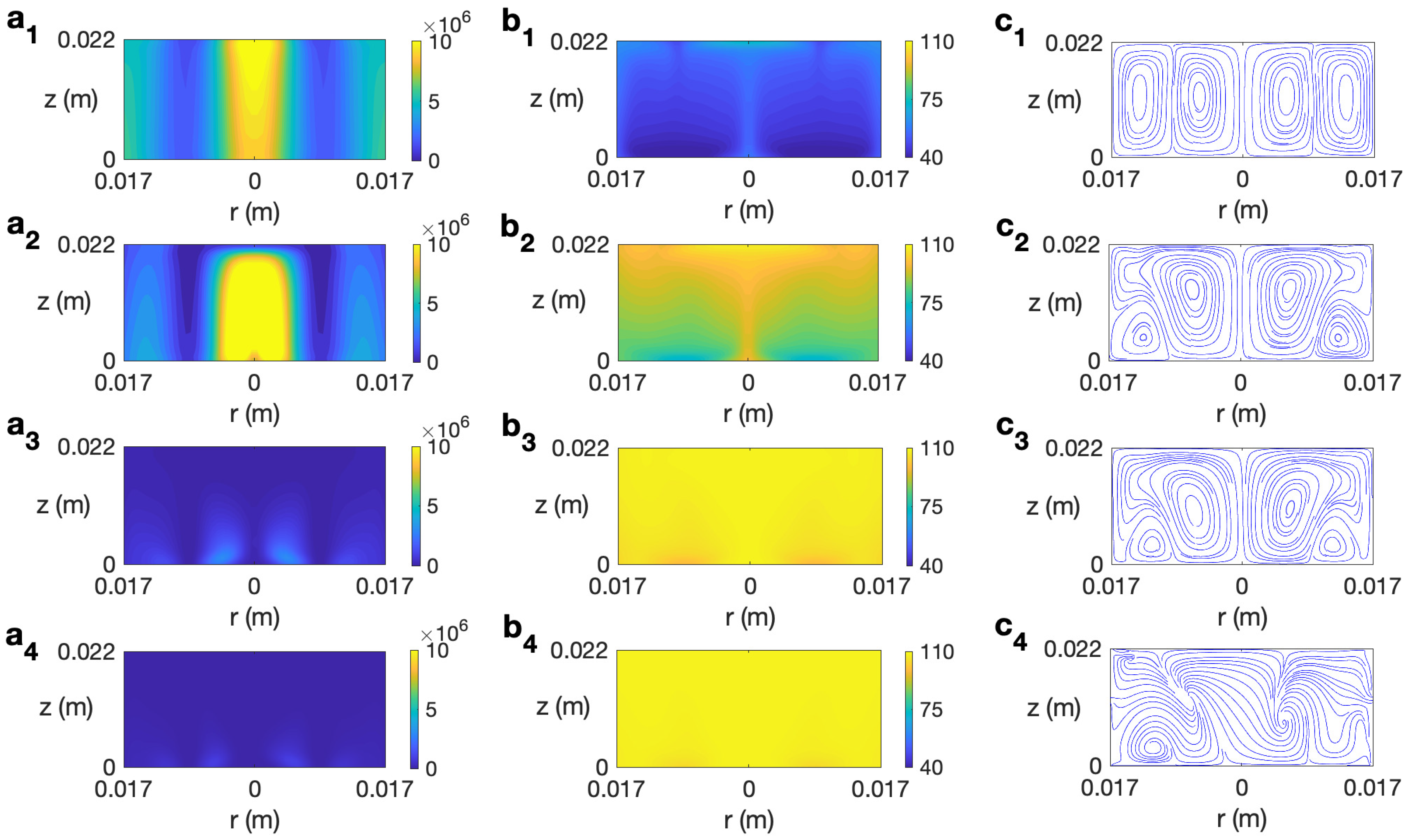
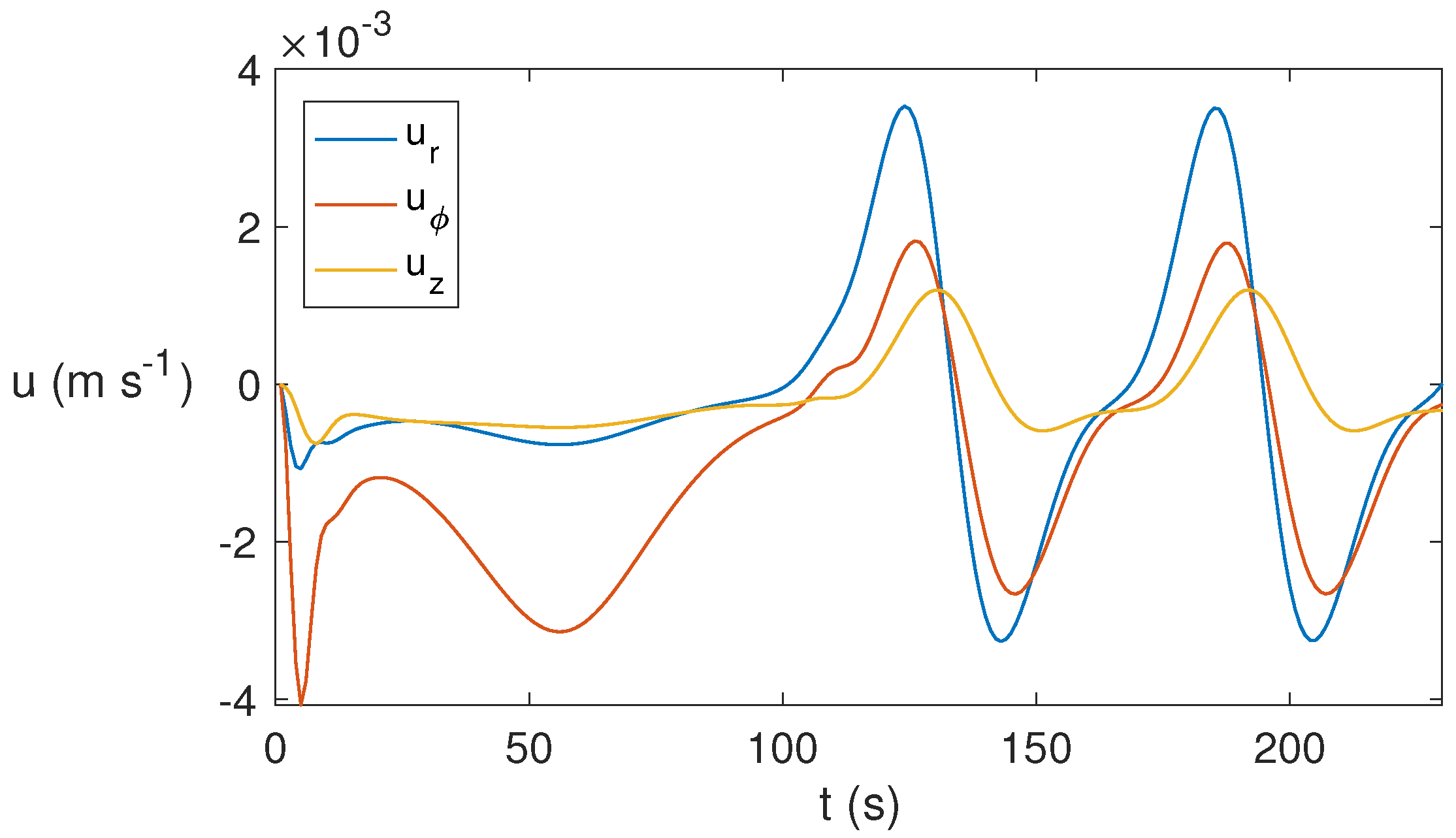
3.1. State Character Depending on
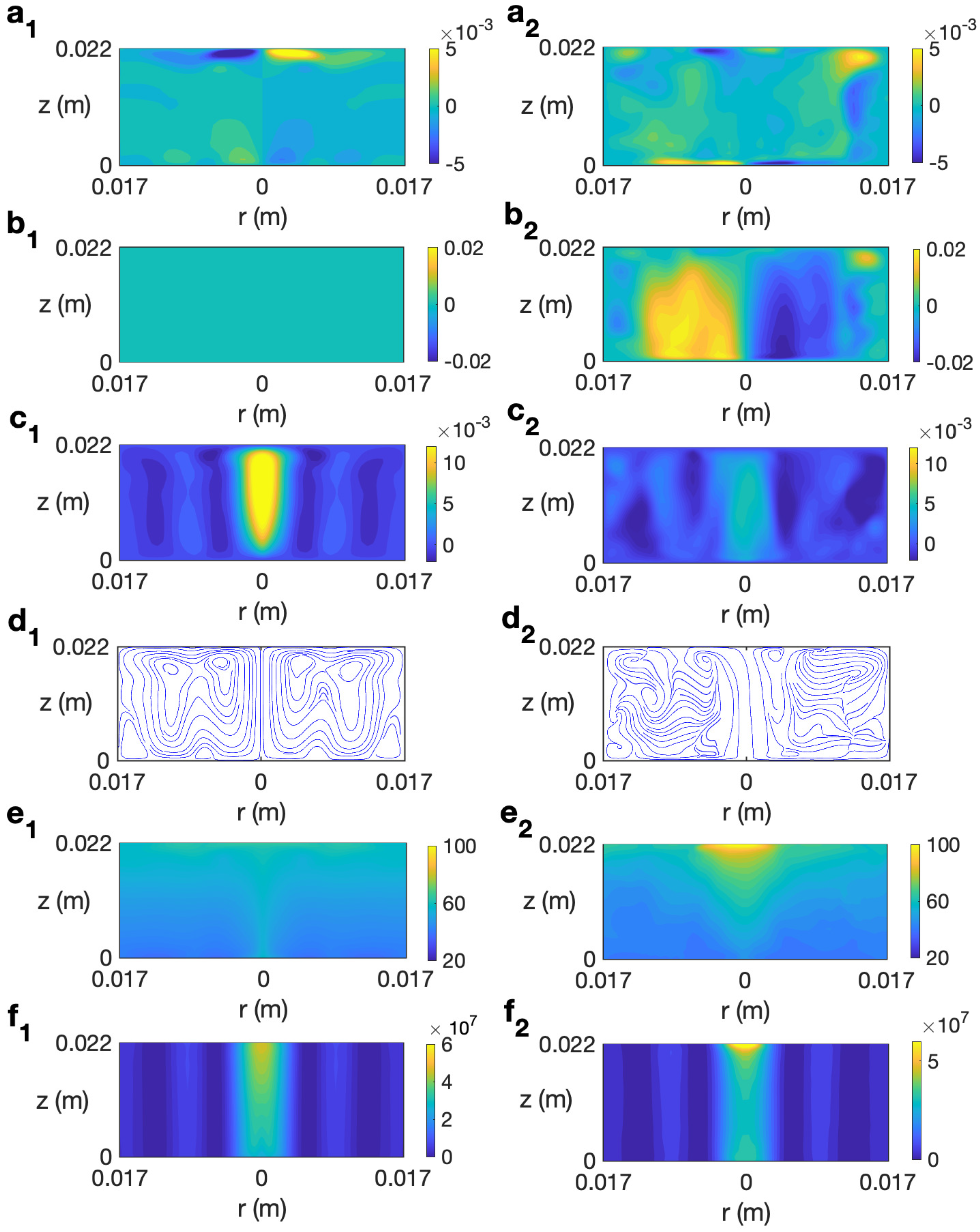
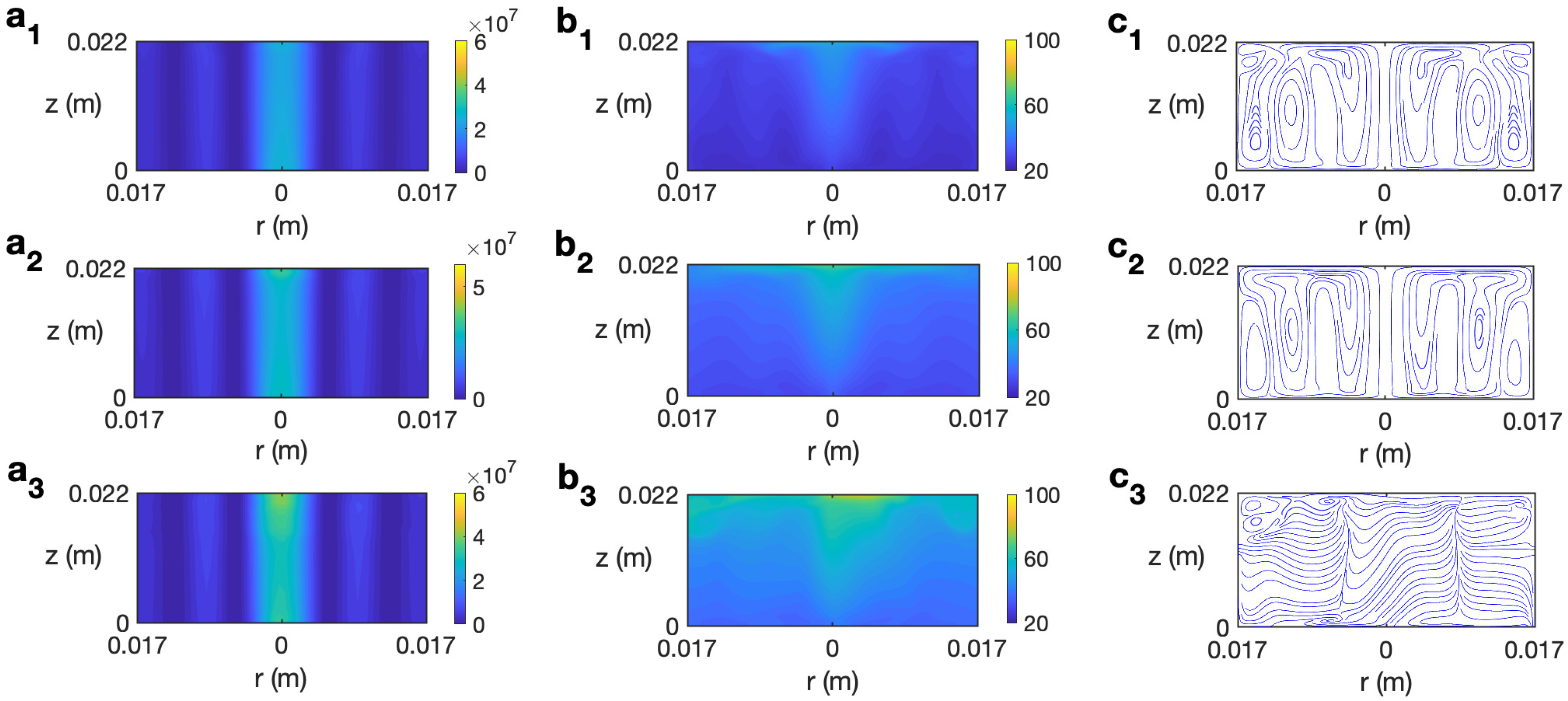
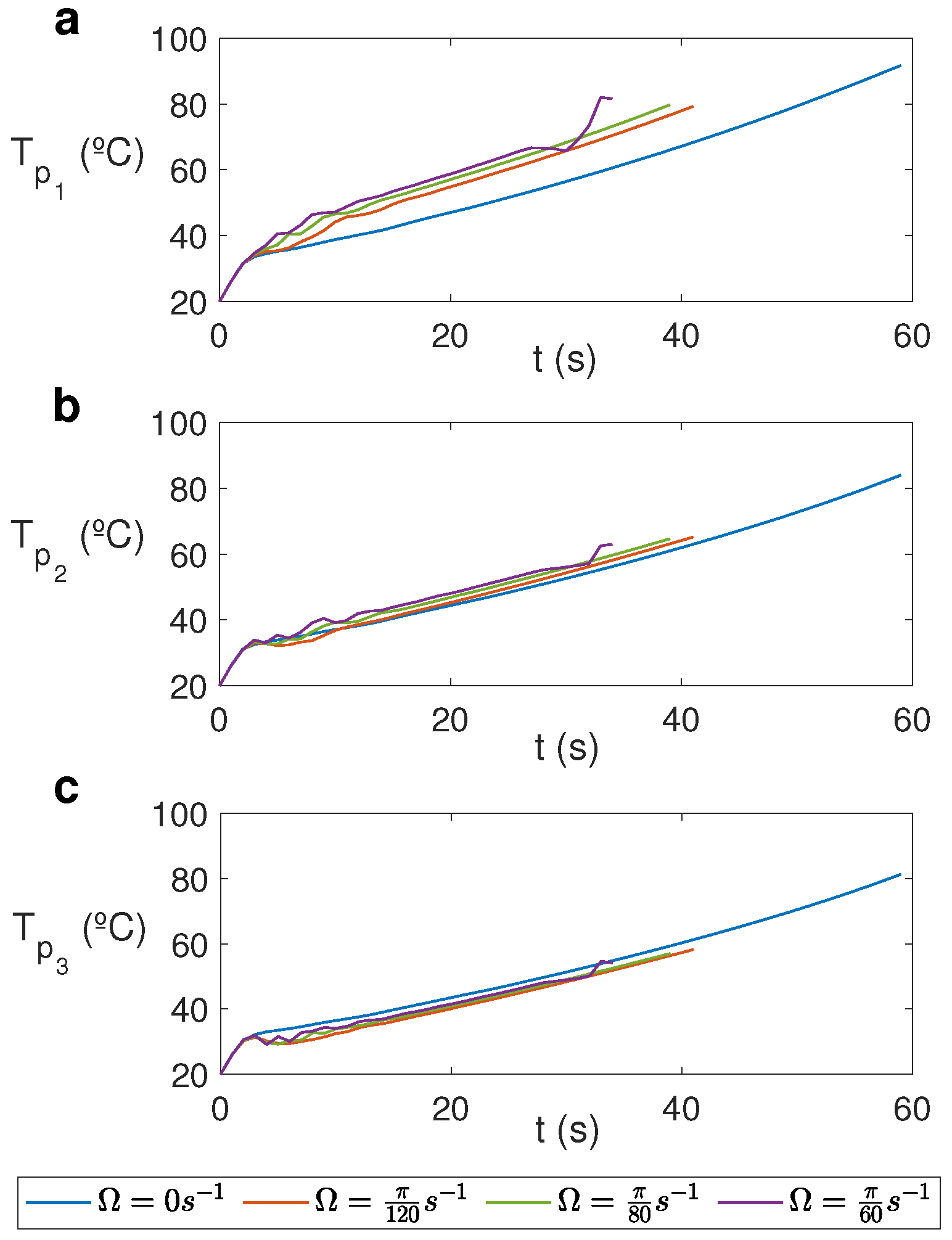
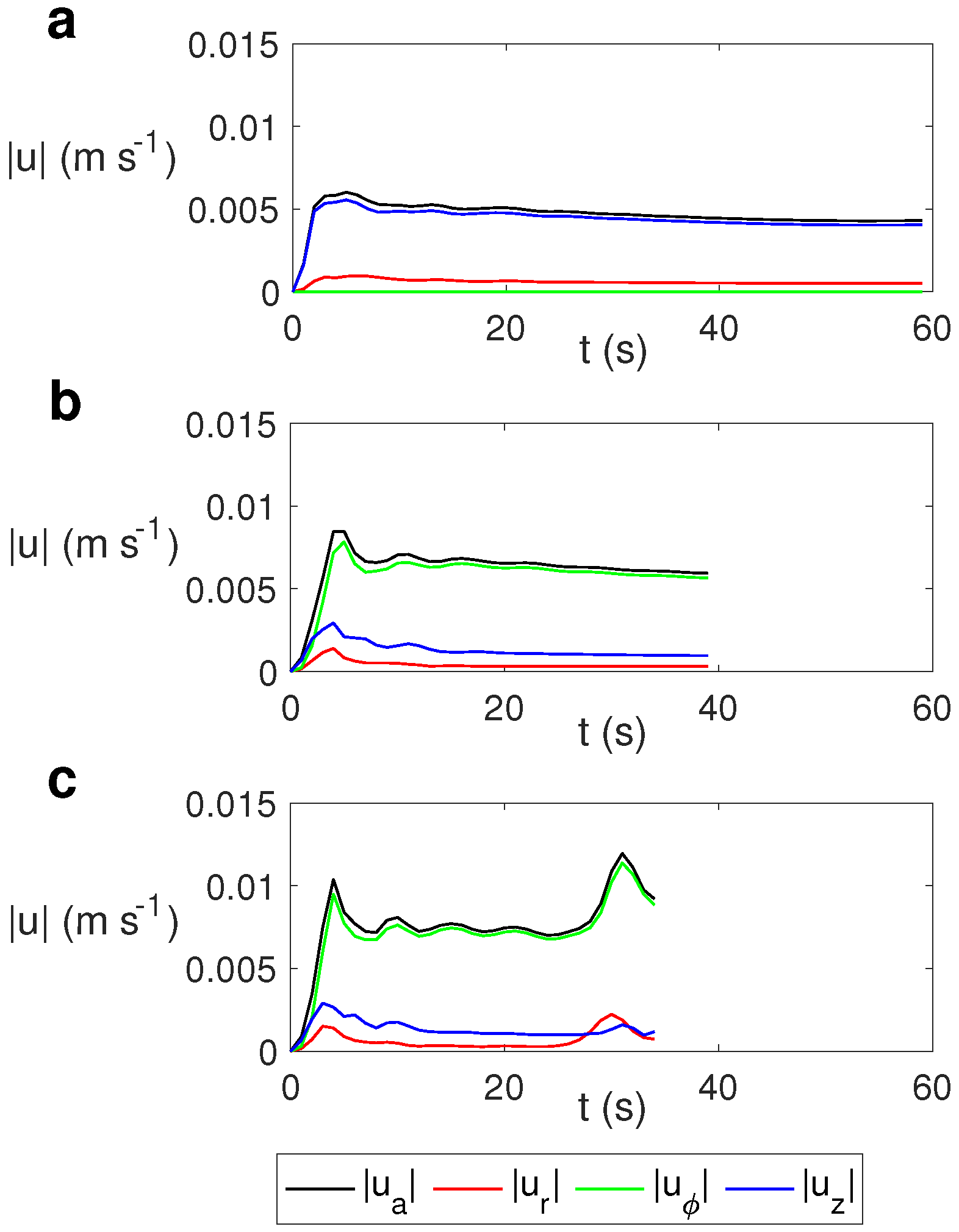
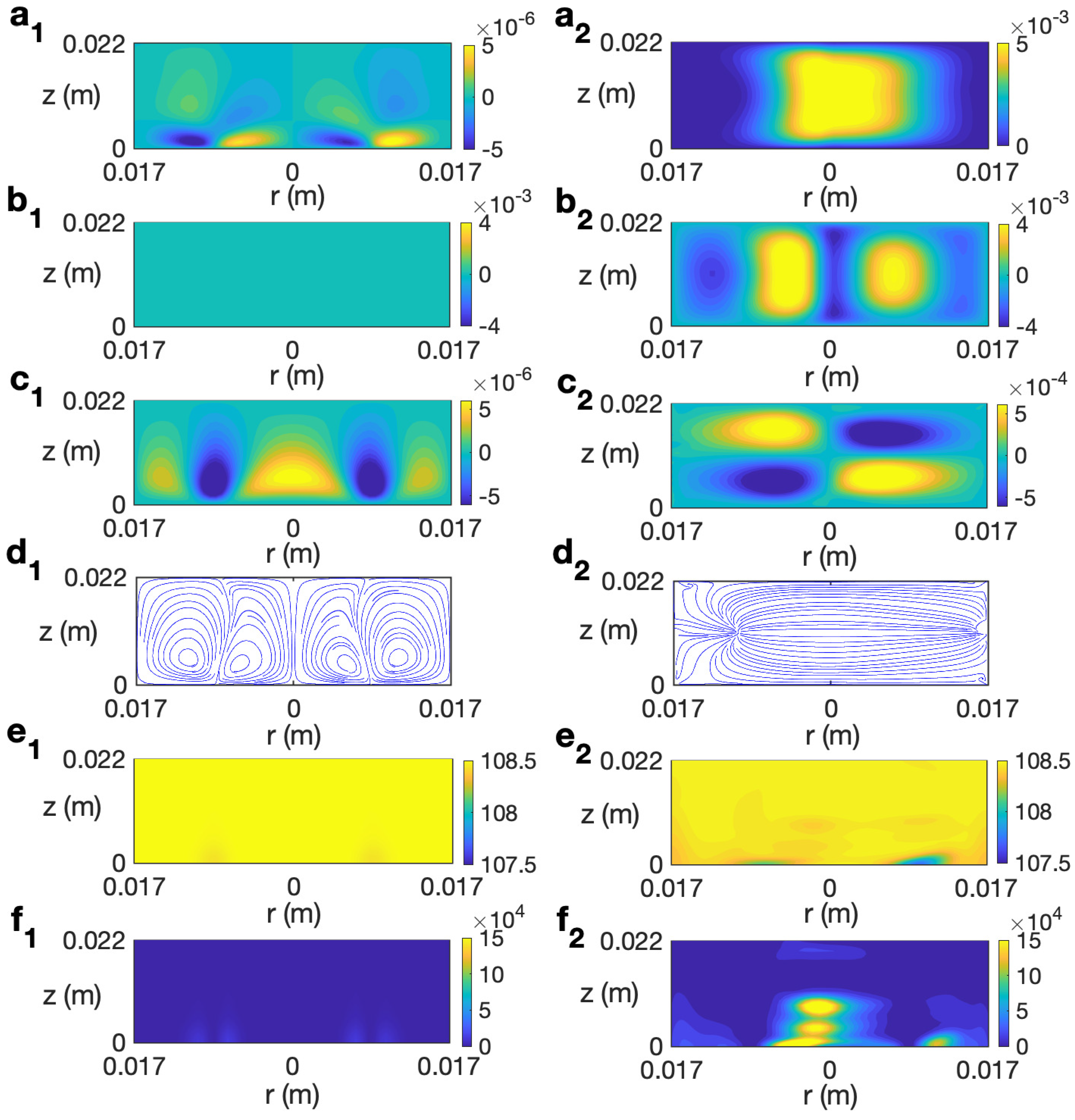
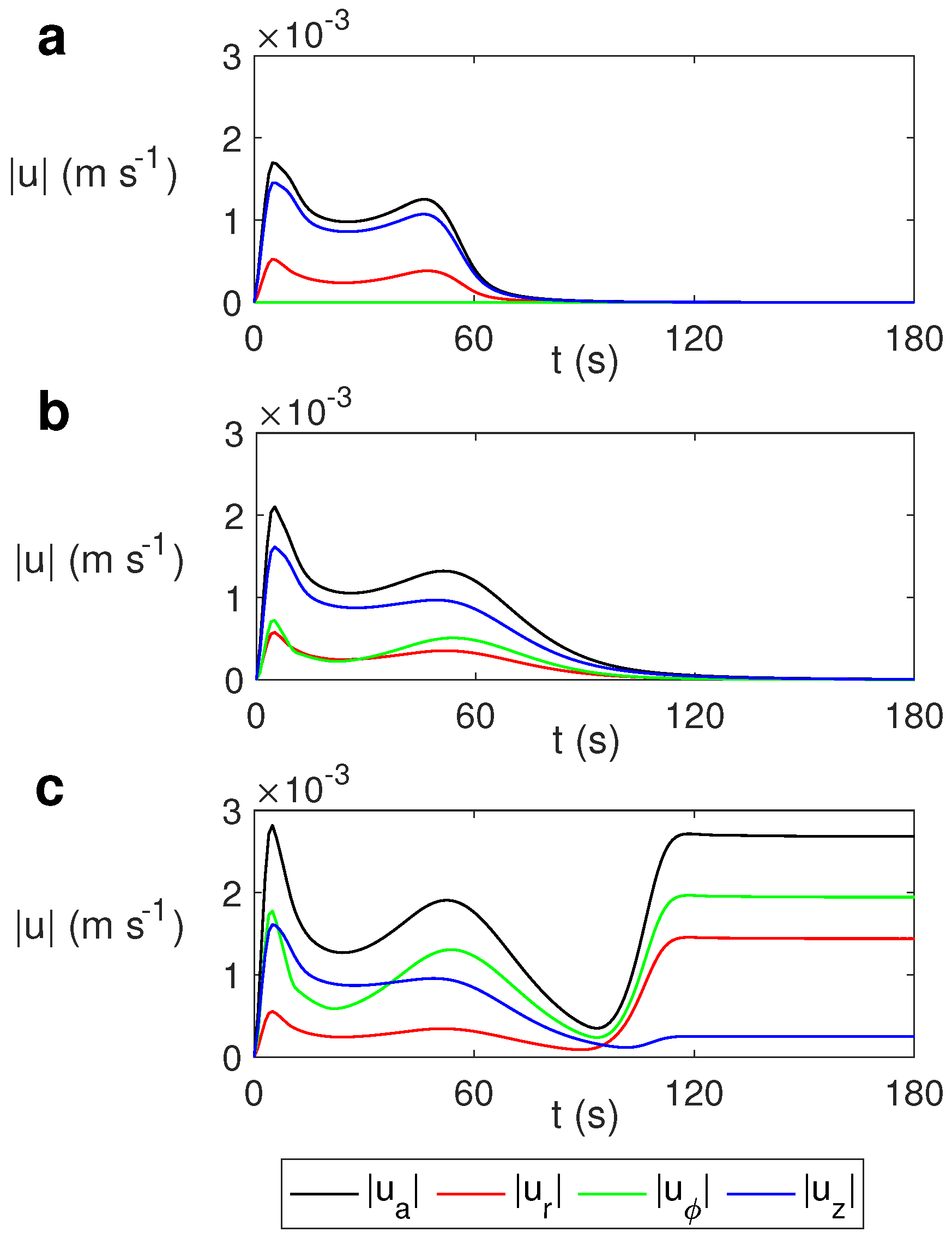
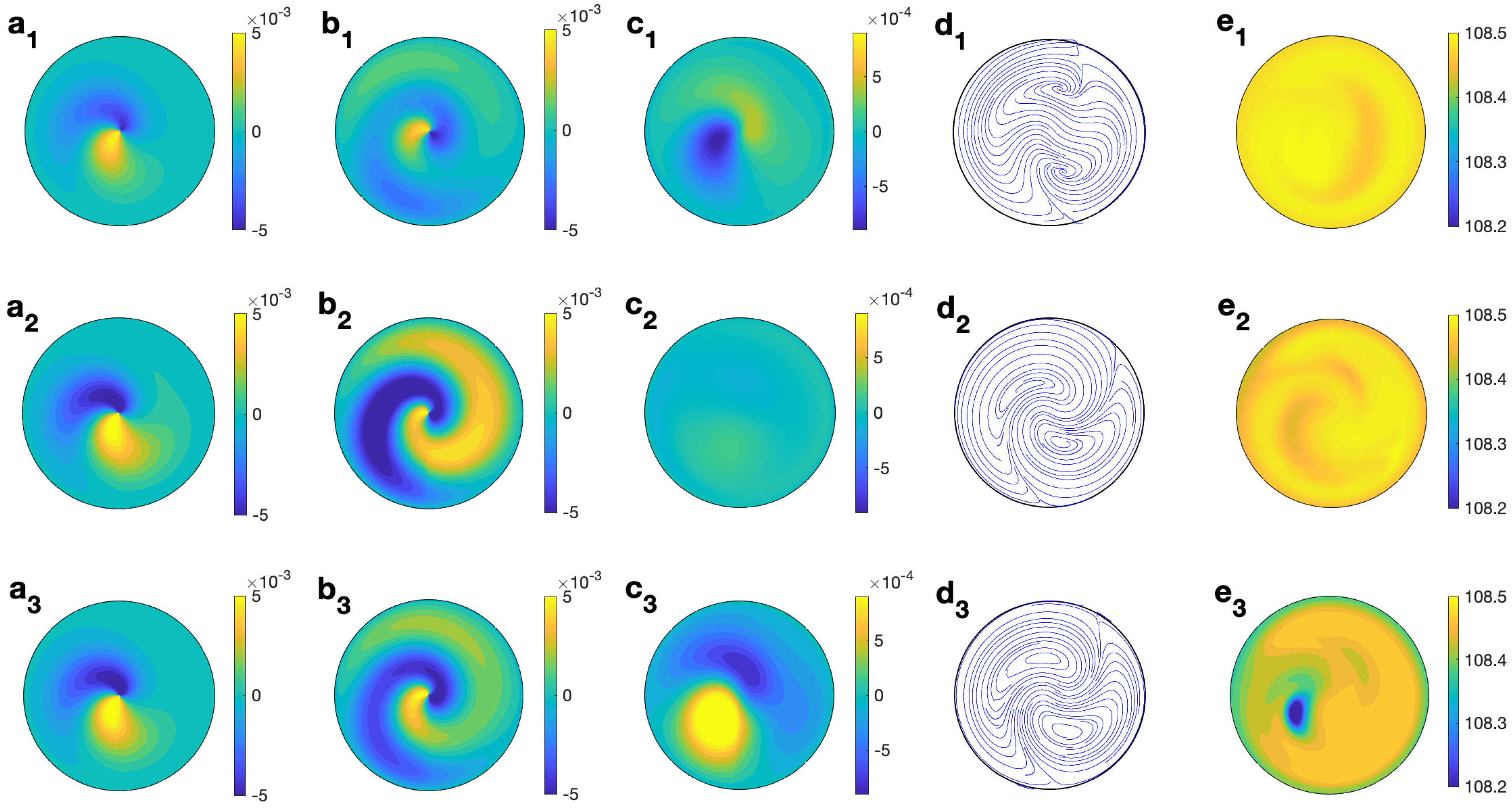
3.2. Effect of Rotation on the Velocity Flow and Temperature Profile
3.2.1. Solvent 1: Water
3.2.2. Solvent 2: Ethylene Glycol
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| E | |
| f | |
| g | |
| H | |
| P | |
| p | |
| Q | |
| R | |
| T | |
| t | |
| Free-space wave number, m−1 | |
| k | |
| Free-space magnetic permeability, H m−1 | |
References
- Gabriel, C.; Gabriel, S.; Grant, E.H.; Halstead, B.S.; Mingos, D.M.P. Dielectric Parameters Relevant to Microwave Dielectric Heating. Chem. Soc. Rev. 1998, 27, 213–224. [Google Scholar] [CrossRef]
- Oliveira, M.E.C.; Franca, A.S. Microwave Heating of Foodstuffs. J. Food Eng. 2002, 53, 347–359. [Google Scholar] [CrossRef]
- Campañone, L.A.; Zaritzky, N.E. Mathematical Modeling and Simulation of Microwave Thawing of Large Solid Foods Under Different Operating Conditions. Food Bioprocess. Technol. 2010, 3, 813–825. [Google Scholar] [CrossRef]
- Mao, W.; Watanabe, M.; Sakai, N. Analysis of Temperature Distributions in Kamaboko During Microwave Heating. J. Food Eng. 2005, 71, 187–192. [Google Scholar] [CrossRef]
- Campañone, L.A.; Paola, C.A.; Mascheroni, R.H. Modeling and Simulation of Microwave Heating of Foods Under Different Process Schedules. Food Bioprocess. Technol. 2012, 5, 738–749. [Google Scholar] [CrossRef]
- Fan, D.; Li, C.; Li, Y.; Chen, W.; Zhao, J.; Hu, M.; Zhang, H. Experimental Analysis and Numerical Modeling of Microwave Reheating of Cylindrically Shaped Instant Rice. Int. J. Food Eng. 2014, 10, 59–67. [Google Scholar] [CrossRef]
- Navarro, M.C.; Burgos, J. A Spectral Method for Numerical Modeling of Radial Microwave Heating in Cylindrical Samples with Temperature Dependent Dielectric Properties. Appl. Math. Model. 2017, 43, 268–278. [Google Scholar] [CrossRef]
- Yousefi, T.; Mousavi, S.A.; Saghir, M.Z.; Farahbakhsh, B. An Investigation on the Microwave Heating of Flowing Water: A Numerical Study. Int. J. Therm. Sci. 2013, 71, 118–127. [Google Scholar] [CrossRef]
- Salvi, D.; Boldor, D.; Aita, G.M.; Sabliov, C.M. COMSOL Multiphysics Model for Continuous Flow Microwave Heating Liquids. J. Food Eng. 2011, 104, 422–429. [Google Scholar] [CrossRef]
- Tuta, S.; Palazoglu, T.K. Finite Element Modeling of Continuous-Flow Microwave Heating of Fluid Foods and Experimental Validation. J. Food Eng. 2017, 192, 79–92. [Google Scholar] [CrossRef]
- Ratanadecho, P.; Aoki, K.; Akahori, M. A Numerical and Experimental Investigation of the Modeling of Microwave Heating for Liquid Layers Using a Rectangular Wave Guide (Effects of Natural Convection and Dielectric Properties). Appl. Math. Model. 2002, 26, 449–472. [Google Scholar] [CrossRef]
- Cha-Um, W.; Rattanadecho, P.; Pakdee, W. Experimental and Numerical Analysis of Microwave Heating of Water and Oil Using a Rectangular Wave Guide: Influence of Sample Sizes, Position, and Microwave Power. Food Bioprocess Technol. 2011, 4, 544–558. [Google Scholar] [CrossRef]
- Yakovlev, V.V. Examination of Contemporary Electromagnetic Software Capable of Modeling Problems of Microwave Heating, Advances in Microwave and Radio Frequency Processing; Willert-Porada, M., Ed.; Springer: Berlin/Heidelberg, Germany, 2005; pp. 178–190. [Google Scholar]
- Navarro, M.C.; Diaz-Ortiz, A.; Prieto, P.; de la Hoz, A. A Spectral Numerical Model and an Experimental Investigation on Radial Microwave Irradiation of Water an Ethanol in a Cylindrical Vessel. Appl. Math. Model. 2019, 66, 680–694. [Google Scholar] [CrossRef]
- Liu, S.; Fukuoka, M.; Sakai, N. A finite element model for simulating temperature distributions in rotating microwave heating. J. Food Eng. 2013, 115, 49–62. [Google Scholar] [CrossRef]
- Pitchai, K.; Chen, J.; Birla, S.; Gonzalez, R.; Jones, D.; Subbiah, J. A microwave heat transfer model for a rotating multi-component meal in a domestic oven: Development and validation. J. Food Eng. 2014, 128, 60–71. [Google Scholar] [CrossRef]
- Zhu, H.; He, J.; Hong, T.; Yang, W.; Wu, Y.; Yang, Y.; Huang, K. A rotary radiation structure for microwave heating uniformity improvement. Appl. Therm. Eng. 2018, 141, 648–658. [Google Scholar] [CrossRef]
- Topcam, H.; Karatas, O.; Erol, B.; Erdogdu, F. Effect of rotation on temperature uniformity of microwave processed low-high viscosity liquids: A computational study with experimental validation. Innov. Food Sci. Emerg. Technol. 2020, 60, 102306. [Google Scholar] [CrossRef]
- Khaghanikavkani, E.; Farid, M.M.; Holdem, J.; Williamson, A. Microwave pyrolysis of plastic. J. Chem. Eng. Process Technol. 2013, 4, 1000150. [Google Scholar] [CrossRef]
- Kouzaev, G.A. A Method and Apparatus for Separate Supply of Microwave and Mechanical Energies to Liquid Reagents in Coaxial Rotating Chemical Reactors. UK Patent Application GB1704095.7, 15 March 2017. [Google Scholar]
- Chatterjee, S.; Basak, T.; Das, S.K. Microwave driven convection in a rotating cylindrical cavity: A numerical study. J. Food Eng. 2007, 79, 1269–1279. [Google Scholar] [CrossRef]
- Balanis, C.A. Advanced Engineering Electromagnetics; Wiley: New York, NY, USA, 1989. [Google Scholar]
- Canuto, C.; Hussain, M.Y.; Quarteroni, A.; Zang, T.A. Spectral Methods in Fluid Dynamics; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Herrero, H.; Mancho, A.M. On pressure boundary conditions for thermoconvective problems. Int. J. Numer. Meth. Fluids 2002, 39, 391–402. [Google Scholar] [CrossRef]
- Mercader, I.; Batiste, O.; Alonso, A. An Efficient Spectral Code for Incompressible Flows in Cylindrical Geometries. Comput. Fluids 2010, 39, 215–224. [Google Scholar] [CrossRef]
- Horikoshi, S.; Matsuzaki, S.; Mitani, T.; Serpone, N. Microwave frequency effects on dielectric properties of some common solvents and on microwave-assisted syntheses: 2-Allyphenol and the C12–C2–C12 Gemini Surfactant. Radiat. Phys. Chem. 2012, 81, 1885–1895. [Google Scholar] [CrossRef]
- Faghri, A.; Zhang, Y. Transport Phenomena in Multiphase Systems; Elsevier: Burlington, MA, USA, 2006. [Google Scholar]
- Faghri, A.; Zhang, Y.; Howell, J. Advanced Heat and Mass Transfer; Global Digital Press: Columbia, OH, USA, 2010. [Google Scholar]
- Vargaftik, N.B. Handbook of Physical Properties of Liquids and Gases; Hemisphere: New York, NY, USA, 1975. [Google Scholar]
- Navarro, M.C.; Castaño, D. Microwave irradiation and conventional heating: A comparison using in silico experiments with water and ethylene glycol. Heat Transf. Res. 2022, 53, 73–91. [Google Scholar] [CrossRef]
- Navarro, M.C. Water, Toluene, Methanol and Ethanol Under Microwave Irradiation: Numerical Simulations of the Effect of the Vessels Size. J. Heat Transf. 2020, 142, 102104. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Navarro, M.C.; Castaño, D. Effect of Rotation in Radial Microwave Irradiation: A Numerical Approach. Mathematics 2025, 13, 357. https://doi.org/10.3390/math13030357
Navarro MC, Castaño D. Effect of Rotation in Radial Microwave Irradiation: A Numerical Approach. Mathematics. 2025; 13(3):357. https://doi.org/10.3390/math13030357
Chicago/Turabian StyleNavarro, María Cruz, and Damián Castaño. 2025. "Effect of Rotation in Radial Microwave Irradiation: A Numerical Approach" Mathematics 13, no. 3: 357. https://doi.org/10.3390/math13030357
APA StyleNavarro, M. C., & Castaño, D. (2025). Effect of Rotation in Radial Microwave Irradiation: A Numerical Approach. Mathematics, 13(3), 357. https://doi.org/10.3390/math13030357


_Constantinou_Generalis.png)



