Bifurcations and Exact Solutions of the Coupled Nonlinear Generalized Zakharov Equations with Anti-Cubic Nonlinearity: Dynamical System Approach
Abstract
1. Introduction
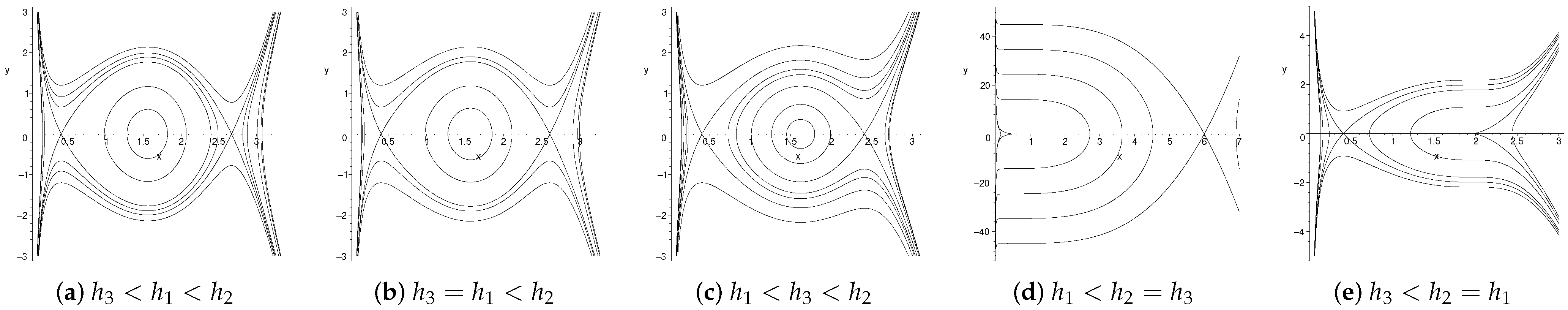

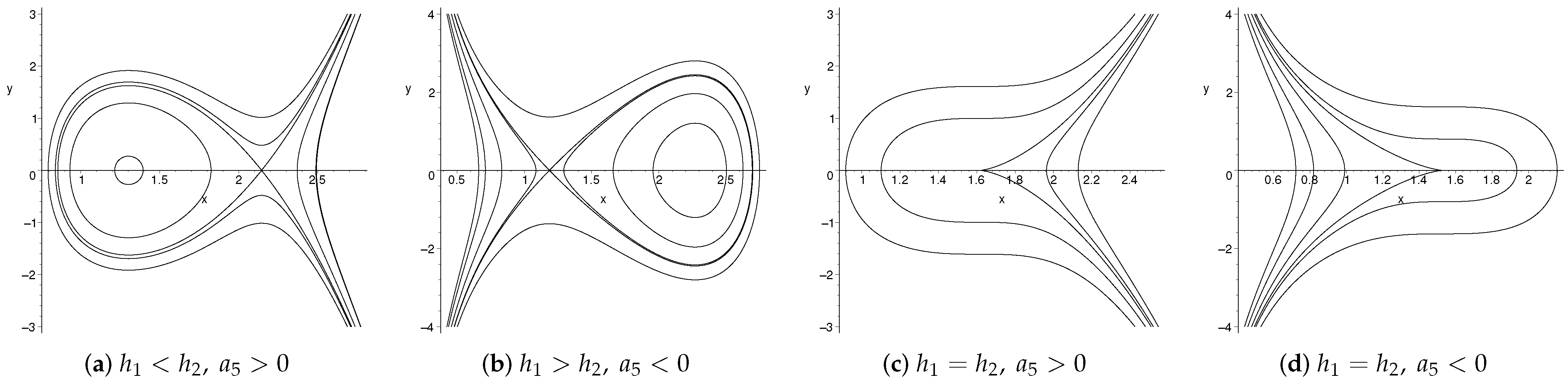
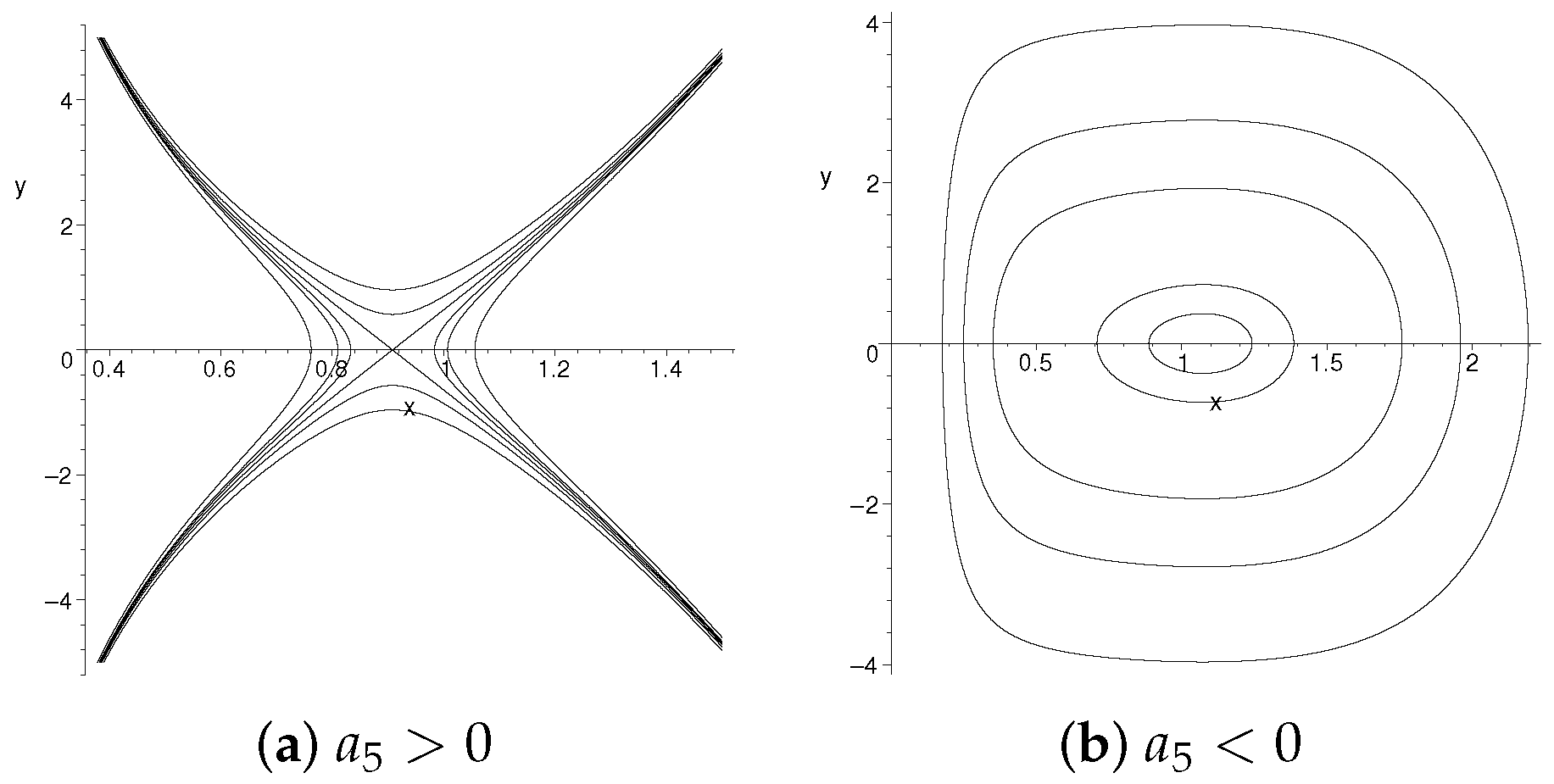
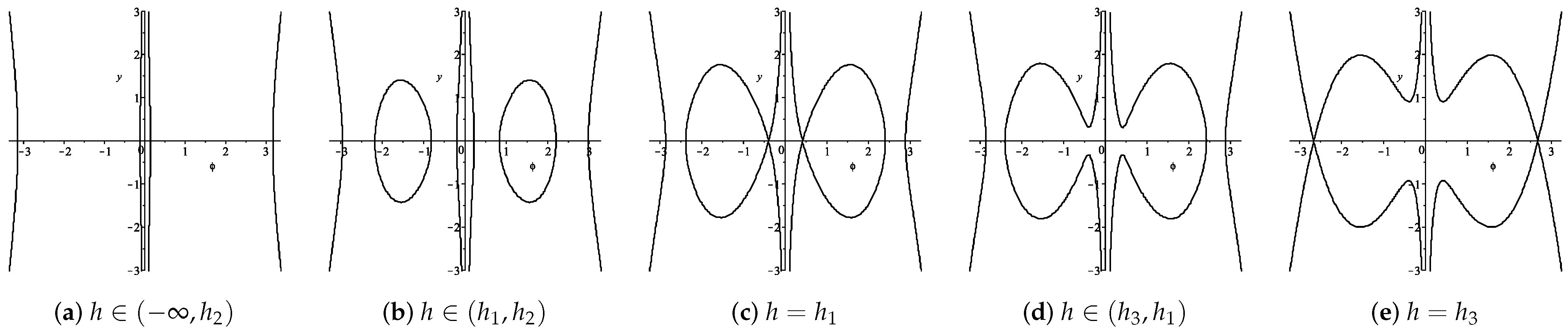
2. Bifurcations of Phase Portraits of the System (4)
- (i)
- If and then has two positive real zeros. Furthermore,
- (i1)
- If and then has three positive real zeros satisfying for and for .
- (i2)
- If and either or then has a double positive real zero.
- (i3)
- If and , then has two positive real zeros satisfying for and for .
- (ii)
- When if then has an positive real zero.
- (I)
- The case that there exist three equilibrium points (including double points) of the system (4) in the positive axis.
- (II)
- The case that there exist two equilibrium points (including double points) of the system (4) in the positive axis.
- (III)
- The case that there exists one equilibrium point of the system (4) in the positive axis.
3. Explicit Exact Parametric Representations of the Solutions of the System (4) with Three Equilibrium Points and a5 >0
3.1. The Parametric Representations of the Bounded Orbits Given by Figure 1a
- (i)
- Corresponding to the level curves defined by with , there exist two families of open orbits of the system (4), for which one family tends to the singular straight line , when (see Figure 5a). This open orbit family gives rise to a family of compacton solutions. Correspondingly, (13) can be written asfrom which we obtain the parametric representation of the family of compacton solutions given byHerewhere are the Jacobin elliptic functions (see [23]).
- (ii)
- Corresponding to the level curves defined by with there exists a family of periodic orbits and two families of open orbits of the system (4) (see Figure 5b). For the family of periodic orbits, (13) can be written asfrom which we obtain the parametric representation of the family of periodic solutions of the system (4) given bywhere
- (iii)
- The level curves defined by contain a homoclinic orbit to the equilibrium point and an open orbit (see Figure 5c). For the homoclinic orbit enclosing the equilibrium point , (13) can be written asfrom which one has the parametric representation of a solitary wave solution of the system (4)where For the given by (18), letone hasIt follows that
- (iv)
- The level curves defined by with are two open curve families, for which one curve family tends to the singular straight line as . It gives rise to a compacton solution family having the same parametric representation as that of (14).
- (v)
- The level curves defined by are two stable manifolds and two unstable manifolds of the saddle point , for which two manifolds tend to the singular straight line as (see Figure 5e). For the left stable manifold, (13) can be written asfrom which one has the following parametric representation of the stable manifoldwhere and
3.2. The Parametric Representations of the Heteroclinic Orbits Given by Figure 1b
3.3. The Parametric Representations of the Homoclinic Orbit Given by Figure 1c
3.4. The Parametric Representations of the Stable Manifold of the Cusp Point Given by Figure 1d
4. Explicit Exact Parametric Representations of the Solutions of the System (4) with Three Equilibrium Points and a5 < 0
4.1. The Parametric Representations of the Bounded Orbits Given by Figure 2a
- (i)
- (ii)
- Corresponding to the level curves defined by with there exist two families of periodic orbits of the system (4), enclosing the equilibrium point and , respectively. For the left family of periodic orbits enclosing the center , (13) can be written asfrom which we obtain the parametric representation of this family of periodic solutions of the system (4) given bywhereWe notice that when h approaches , the periodic orbit in the left family defined by tends to the left homoclinic loop which is close to the singular straight line . Therefore, the left homoclinic orbit gives rise to an envelope pseudo-peakon solution, and the periodic orbit family gives rise to a pseudo-periodic peakon family (see Figure 6a,b).
- (iii)
- Corresponding to the level curves defined by , there exist two homoclinic orbits of the system (4) to the saddle point , enclosing the equilibrium point and , respectively. For the right homoclinic orbit, (13) can be written asfrom which the parametric representation of the bright envelope soliton solution of the system (4) is given by (see Figure 6d):where .
- (iv)
- Corresponding to the level curves defined by with , there exists a global family of periodic orbits enclosing three equilibrium points of the system (4). In this case, this family has the same parametric representation as that of (27).Note that there exists a segment of every periodic orbit in this periodic family for which it is very close to the singular straight line . This global periodic orbit family gives rise to a family of pseudo-periodic peakon solution of the system (4) (see Figure 6c).Similarly, for the orbits shown in Figure 2b,c, one can calculate their parametric representations. We skip it here.
4.2. The Parametric Representations of the Homoclinic Orbit to the Cusp Point Given by Figure 2e
5. The Parametric Representations of the Homoclinic Orbit and Periodic Orbits Given by Figure 3b
- (i)
- Corresponding to the level curves defined by with there exist a family of periodic orbits enclosing the equilibrium point of the system (4) and an open curve family which tends to the singular straight line as For the periodic family, (13) can be written asfrom which one has the parametric representation of the family of periodic solutions of the system (4) given bywhere
- (ii)
- Corresponding to the level curves defined by there exist a homoclinic orbit of the system (4) to the saddle point enclosing the equilibrium point . Equation (13) can be written asfrom which we have the the parametric representation of the bright envelope soliton solution of the system (4) given bywhere
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Al Qahtani, S.A.; Alngar, M.E.M. Soliton Solutions for Coupled Nonlinear Generalized Zakharov Equations with Anti-cubic Nonlinearity Using Various Techniques. Int. J. Appl. Comput. Math. 2024, 10, 9. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Optical solitons of the model with generalized anti-cubic nonlinearity. Optik 2022, 257, 168746. [Google Scholar] [CrossRef]
- Olver, P.J.; Rosenau, P. Tri-Hamiltonian duality between solitons and solitary-wave solutions having compact support. Phys. Rev. E 1996, 53, 1900–1906. [Google Scholar] [CrossRef] [PubMed]
- Camassa, R.; Holm, D.D. An integrable shallow water equation with peak solutions. Phys. Rev. Lett. 1993, 71, 1161–1164. [Google Scholar] [CrossRef]
- Camassa, R.; Holm, D.D.; Hyman, J.M. A new integrable shallow water equation. Adv. Appl. Mech. 1994, 31, 1–33. [Google Scholar]
- Camassa, R.; Hyman, J.M.; Luce, B.P. Nonlinear waves and solitons in physical systems. Phys. D 1998, 123, 1–20. [Google Scholar] [CrossRef]
- Degasperis, A.; Holm, D.D.; Hone, A.N.W. A new integrable equation with peakon solutions. Theoret. Math. Phys. 2002, 133, 1463–1474. [Google Scholar] [CrossRef]
- Degasperis, A.; Procesi, M. Asymptotic integrability. In Symmetry and Perturbation Theory; Degasperis, A., Gaeta, G., Eds.; World Scientific: Singapore, 1999; pp. 23–27. [Google Scholar]
- Fokas, A.S. On class of physically important integrable equations. Phys. D 1995, 87, 145–150. [Google Scholar] [CrossRef]
- Li, J.B.; Qiao, Z.J. Peakon, pseudo-peakon, and cuspon solutions for two generalized Camassa-Holm equations. J. Math. Phys. 2013, 54, 123501. [Google Scholar] [CrossRef]
- Li, J.B.; Zhou, W.J.; Chen, G.R. Understanding peakons, periodic peakons and compactons via a shallow water wave equation. Int. J. Bifur. Chaos 2016, 26, 1650207. [Google Scholar] [CrossRef]
- Novikov, V. Generalizations of the Camassa-Holm equation. J. Phys. A Math. Theor. 2009, 42, 342002. [Google Scholar] [CrossRef]
- Qiao, Z. A new integrable equation with cuspons and W/M-shape-peaks solitons. J. Math. Phys. 2006, 47, 112701–112710. [Google Scholar] [CrossRef]
- Li, J.B. Singular Nonlinear Traveling Wave Equations: Bifurcation and Exact Solutions; Science Press: Beijing, China, 2013. [Google Scholar]
- Li, J.B.; Chen, G.R. On a class of singular nonlinear traveling wave equations. Int. J. Bifurc. Chaos 2017, 17, 4049–4065. [Google Scholar] [CrossRef]
- Li, J.B.; Chen, G.R.; Song, J. Completing the study of traveling wave solutions for three two-component shallow water wave models. Internat. J. Bifur. Chaos 2020, 30, 2050036. [Google Scholar] [CrossRef]
- Li, J.B.; Han, M.A.; Ke, A. Bifurcations and exact traveling wave solutions of the Khorbatly’s geophysical Boussinesq system. J. Math. Anal. Appl. 2024, 537, 128263. [Google Scholar] [CrossRef]
- Li, J.B.; Liu, Z.R. Smooth and non-smooth traveling waves in a nonlinear dispersive equation. Appl. Math. Model 2000, 25, 41–56. [Google Scholar] [CrossRef]
- Li, J.B.; Liu, Z.R. Traveling wave solutions for a class of nonlinear dispersive equations. Chin. Ann. Math. 2002, 23, 397–418. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, L.; Zhang, M. Studies on pull-in instability of all electrostatic MEMS actuator: Dynamical system approach. J. Appl. Anal. Comput. 2022, 12, 850–861. [Google Scholar]
- Zhang, L.; Han, M.; Zhang, M.; Khalique, C.M. A new type of solitary wave solution of the mKdV equation under singular perturbations. Int. J. Bifur. Chaos 2020, 30, 2050162. [Google Scholar] [CrossRef]
- Zhang, X.; Tian, Y.; Zhang, M.; Qi, Y. Mathematical studies on generalized Burgers Huxley equation and its singularly perturbed form: Existence of traveling wave solutions. Nonlinear Dyn. 2024, 3, 2625–2634. [Google Scholar] [CrossRef]
- Byrd, P.F.; Fridman, M.D. Handbook of Elliptic Integrals for Engineers and Scientists; Springer: Berlin/Heidelberg, Germany, 1971. [Google Scholar]
- Song, J. Bifurcations and exact traveling wave solutions of the (n+1) dimensional q-deformed double sinh-gordon equations. Discret. Cont. Dyn. Syst. S 2023, 16, 573–588. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhuang, J.S.; Li, J.B. Bifurcations and exact traveling wave solutions in two nonlinear wave systems. Int. J. Bifur. Chaos 2021, 31, 2150093. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, J.; Li, F.; Zhang, M. Bifurcations and Exact Solutions of the Coupled Nonlinear Generalized Zakharov Equations with Anti-Cubic Nonlinearity: Dynamical System Approach. Mathematics 2025, 13, 217. https://doi.org/10.3390/math13020217
Song J, Li F, Zhang M. Bifurcations and Exact Solutions of the Coupled Nonlinear Generalized Zakharov Equations with Anti-Cubic Nonlinearity: Dynamical System Approach. Mathematics. 2025; 13(2):217. https://doi.org/10.3390/math13020217
Chicago/Turabian StyleSong, Jie, Feng Li, and Mingji Zhang. 2025. "Bifurcations and Exact Solutions of the Coupled Nonlinear Generalized Zakharov Equations with Anti-Cubic Nonlinearity: Dynamical System Approach" Mathematics 13, no. 2: 217. https://doi.org/10.3390/math13020217
APA StyleSong, J., Li, F., & Zhang, M. (2025). Bifurcations and Exact Solutions of the Coupled Nonlinear Generalized Zakharov Equations with Anti-Cubic Nonlinearity: Dynamical System Approach. Mathematics, 13(2), 217. https://doi.org/10.3390/math13020217







