Convergence Speed of Bermudan, Randomized Bermudan, and Canadian Options
Abstract
1. Introduction
2. Setting, Notation and Assumptions
- Discounted underlying asset price. For simplicity, we assume a constant interest rate, . We also assume that the underlying asset is risk-neutral, meaning is a martingale. As is meant to be the price of some underlying asset, we also require that . Furthermore, we consider an associated process such that the pair forms a right process. A typical example arises in stochastic volatility models where represents the stochastic volatility, making a Markov process even though alone is not. If is already a right process, can be omitted. Recall that right processes form a very broad class of strong Markov processes characterized by càdlàg (right-continuous with left limits) trajectories. This class encompasses Hunt, Feller, Lévy, and diffusion processes, such as geometric Brownian motion, among others. As noted in [37], “the requirement of the strong Markov property is not onerous, as this includes solutions of stochastic differential equations and integrals of Lévy processes, so almost all common models used in quantitative finance”. The additional condition that the strong Markov process be a right process is similarly unrestrictive.
- Payoff functions. Recall that the value is called the intrinsic value of the option at time t. For simplicity, we consider payoff functions which are bounded, convex, and Lipschitz with compact support. For the American put, , for some .Probability space and counting process N. We consider a probability space, denoted by , which satisfies the usual conditions. We assume that the processes and a counting process are realized on this probability space, with being independent of .
- Conditional expectation notation. In this article, denotes the conditional expectation given that . Similarly, denotes the conditional expectation given that , and denotes the conditional expectation given that .
- Stopping time . Fix and an integer , we denote by the first time such that .
- Randomized options. Throughout this paper, we fix some maturity , a payoff function h, and some integer . In order to avoid undue generality, we focus on two cases: (a) the Poisson case, where is a Poisson process with intensity ; and (b) the deterministic case where the jumps of occur at times , for . We are interested in the following randomized American-style options, which at time can be described in the following manner:
- The Canadian option can be exercised at any time until .
- The randomized Bermudan option can be exercised at times , for .
- Randomized option prices. Fix , integer , and the payoff function h. Suppose that , , and . We denote by the value of the American option, by the value of the randomized Bermudan option, and by the value of the Canadian option. For , let be the class of stopping times such that for every , . Then
- Let be the class of stopping times such that for every , , for . Then and for ,
- Note that the price of a randomized Bermudan option might be less than its intrinsic value since, by definition, it is not exercisable at inception. An optimal stopping time exists: it is the first exercisable time at which the intrinsic value is no less than the option’s value. Note also that replacing by does not affect the value of the randomized Bermudan option because the increments of a Poisson process are independent and identically distributed. (In the deterministic case, where the jumps of occur at times , the value of is uniquely determined by t).Uniform -terms. Consider real valued functions , and , where for some arbitrary set . We say that uniformly if there exists a function such that for every and a constant c, that may depend only on and h, such that for every . In this paper, all O-terms are uniform.
3. Convergence Speed of Randomized Options
3.1. Maximal Immediate Expected Gain
3.2. Bermudan and Randomized Bermudan Options
3.3. Canadian Options
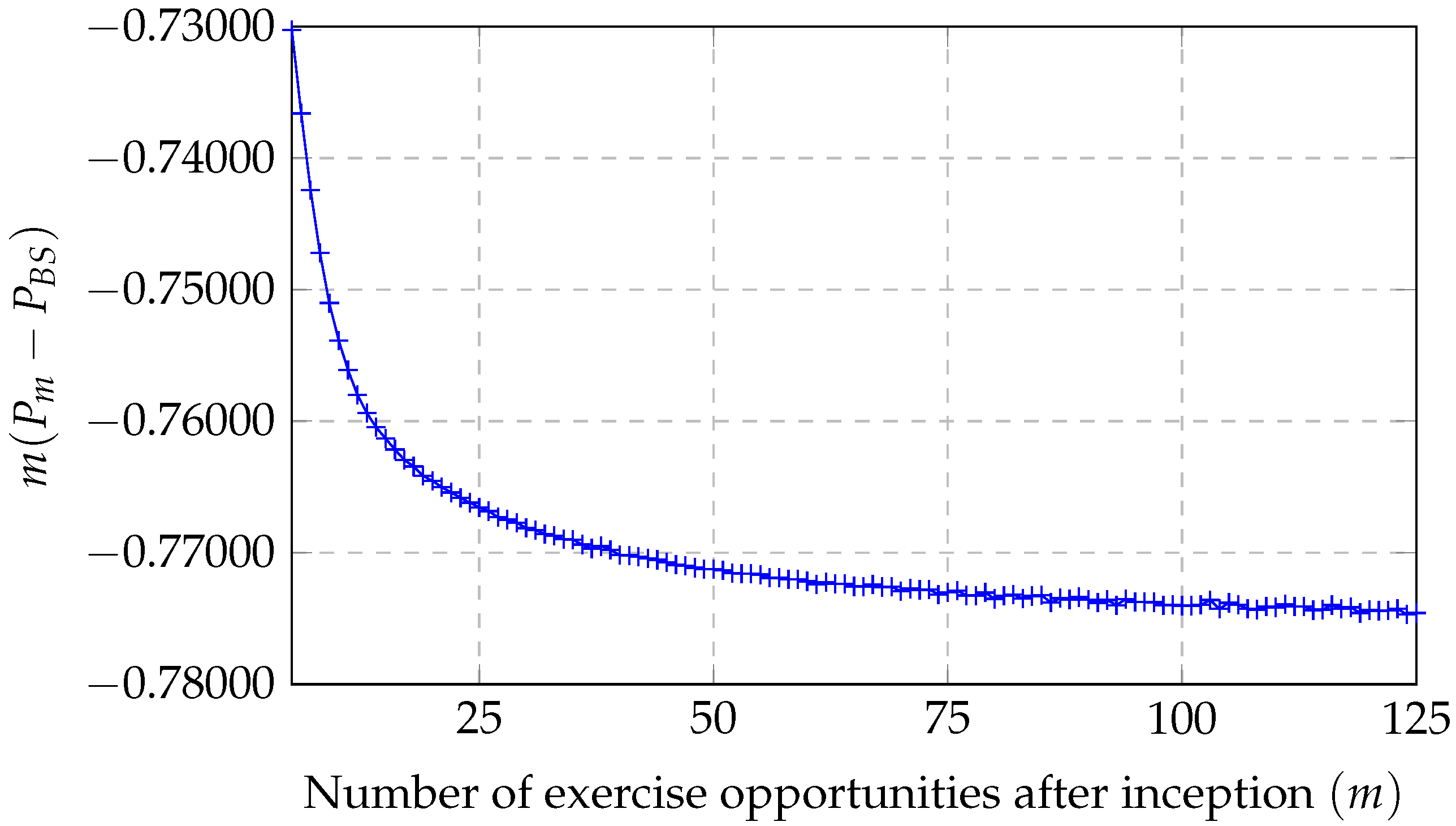
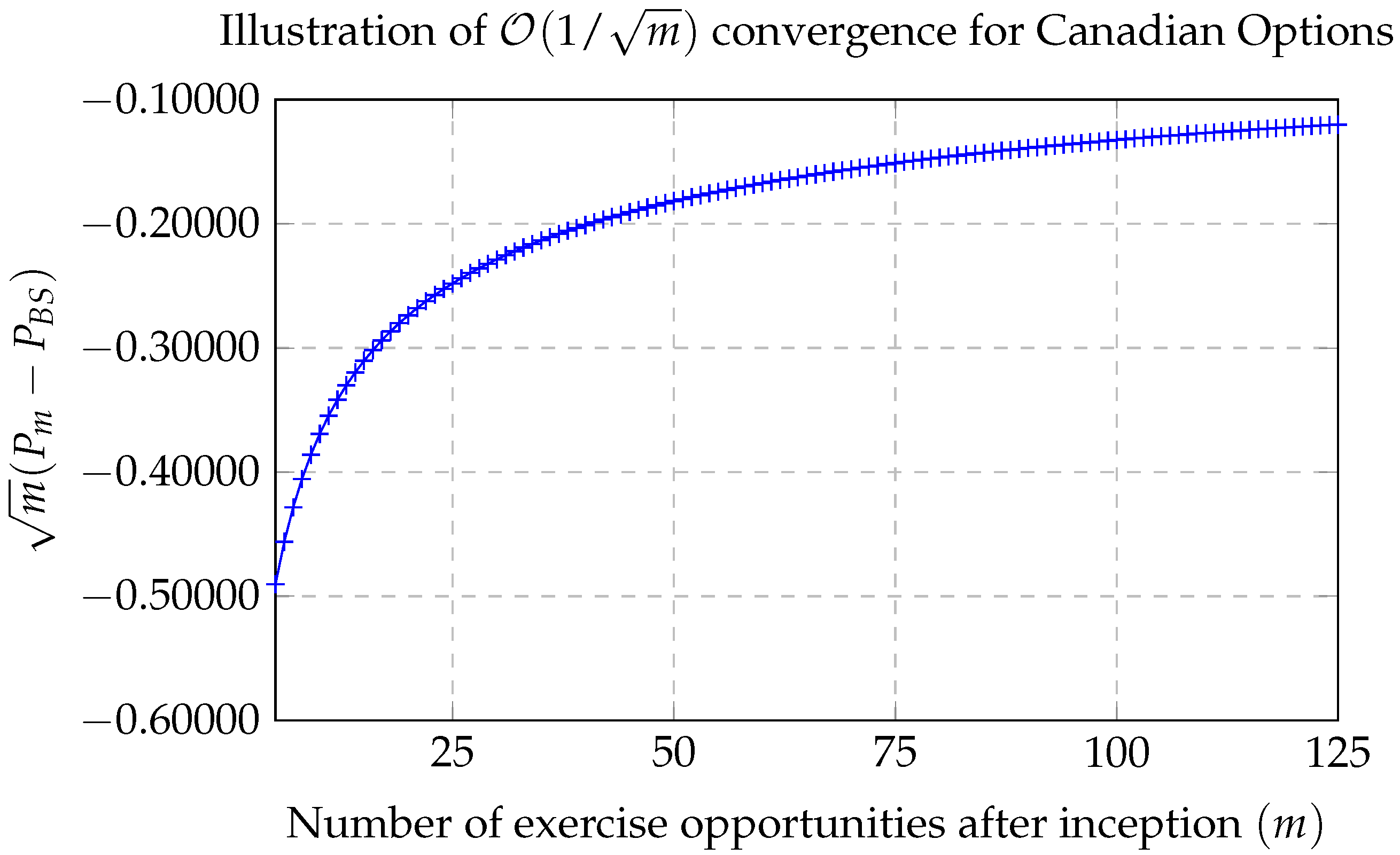
4. Numerical Illustration
Funding
Data Availability Statement
Conflicts of Interest
References
- Lord, R.; Fang, F.; Bervoets, F.; Oosterlee, C.W. A fast and accurate FFT-based method for pricing early-exercise options under Lévy processes. SIAM J. Sci. Comput. 2008, 30, 1678–1705. [Google Scholar] [CrossRef]
- Fang, F.; Oosterlee, C.W. Pricing early-exercise and discrete barrier options by Fourier-cosine series expansions. Numer. Math. 2009, 114, 27–62. [Google Scholar] [CrossRef]
- Chang, C.-C.; Chung, S.-L.; Stapleton, R.C. Richardson extrapolation techniques for the pricing of American-style options. J. Futur. Mark. 2007, 27, 791–817. [Google Scholar] [CrossRef]
- Chang, C.-C.; Lin, J.-B.; Tsai, W.-C.; Wang, Y.-H. Using Richardson extrapolation techniques to price American options with alternative stochastic processes. Rev. Quant. Financ. Account. 2012, 39, 383–406. [Google Scholar] [CrossRef]
- Dupuis, P.; Wang, H. Optimal stopping with random intervention times. Adv. Appl. Probab. 2002, 34, 141–157. [Google Scholar] [CrossRef]
- Dupuis, P.; Wang, H. On the convergence from discrete to continuous time in an optimal stopping problem. Ann. Appl. Probab. 2005, 15, 1339–1366. [Google Scholar] [CrossRef][Green Version]
- Eriksson, B.; Pistorius, M. American option valuation under continuous-time Markov chains. Adv. Appl. Probab. 2015, 47, 378–401. [Google Scholar] [CrossRef]
- Guo, X.; Liu, J. Stopping at the maximum of geometric Brownian motion when signals are received. J. Appl. Probab. 2005, 826–838. [Google Scholar] [CrossRef]
- Lange, R.-J.; Ralph, D.; Støre, K. Real-option valuation in multiple dimensions using Poisson optional stopping times. J. Financ. Quant. Anal. 2020, 55, 653–677. [Google Scholar] [CrossRef]
- Leduc, G. Exercisability randomization of the American option. Stoch. Anal. Appl. 2008, 26, 832–855. [Google Scholar] [CrossRef]
- Leduc, G. The randomized American option as a classical solution to the penalized problem. J. Funct. Spaces 2015, 2015, 245436. [Google Scholar] [CrossRef]
- Lempa, J. Optimal stopping with information constraint. Appl. Math. Optim. 2012, 66, 147–173. [Google Scholar] [CrossRef]
- Miclo, L.; Villeneuve, S. On the forward algorithm for stopping problems on continuous-time Markov chains. J. Appl. Probab. 2021, 58, 1043–1063. [Google Scholar] [CrossRef]
- Bayer, C.; Belomestny, D.; Hager, P.; Pigato, P.; Schoenmakers, J. Randomized optimal stopping algorithms and their convergence analysis. SIAM J. Financ. Math. 2021, 12, 1201–1225. [Google Scholar] [CrossRef]
- Forsyth, P.; Vetzal, K. Quadratic convergence for valuing American options using a penalty method. SIAM J. Sci. Comput. 2002, 23, 2095–2122. [Google Scholar] [CrossRef]
- Howison, S.; Reisinger, C.; Witte, J. The effect of nonsmooth payoffs on the penalty approximation of American options. SIAM J. Financ. Math. 2013, 4, 539–574. [Google Scholar] [CrossRef]
- Howison, S. A matched asymptotic expansions approach to continuity corrections for discretely sampled options. Part 2: Bermudan options. Appl. Math. Financ. 2007, 14, 91–104. [Google Scholar] [CrossRef]
- Abi Jaber, E.; El Euch, O. Markovian structure of the Volterra Heston model. Stat. Probab. Lett. 2019, 149, 63–72. [Google Scholar] [CrossRef]
- Abi Jaber, E.; Larsson, M.; Pulido, S. Affine Volterra processes. Ann. Appl. Probab. 2019, 29, 3155–3200. [Google Scholar] [CrossRef]
- Chevalier, E.; Pulido, S.; Zúñiga, E. American options in the Volterra Heston model. SIAM J. Financ. Math. 2022, 13, 426–458. [Google Scholar] [CrossRef]
- Lai, T.L.; Yao, Y.-C.; AitSahlia, F. Corrected random walk approximations to free boundary problems in optimal stopping. Adv. Appl. Probab. 2007, 39, 753–775. [Google Scholar] [CrossRef]
- Ballestra, L.V.; Cecere, L. A fast numerical method to price American options under the Bates model. Comput. Math. Appl. 2016, 72, 1305–1319. [Google Scholar] [CrossRef]
- Dilloo, M.J.; Tangman, D.Y. A high-order finite difference method for option valuation. Comput. Math. Appl. 2017, 74, 652–670. [Google Scholar] [CrossRef]
- Feng, L.; Lin, X. Pricing Bermudan options in Lévy process models. SIAM J. Financ. Math. 2013, 4, 474–493. [Google Scholar] [CrossRef]
- Gong, X.; Zhuang, X. American option valuation under time changed tempered stable Lévy processes. Phys. A Stat. Mech. Its Appl. 2017, 466, 57–68. [Google Scholar] [CrossRef]
- Shoude, H.; Guo, X. A Shannon wavelet method for pricing American options under two-factor stochastic volatilities and stochastic interest rate. Discret. Dyn. Nat. Soc. 2020, 2020, 8531959. [Google Scholar] [CrossRef]
- Bunch, D.S.; Johnson, H. A simple and numerically efficient valuation method for American puts using a modified Geske-Johnson approach. J. Financ. 1992, 47, 809–816. [Google Scholar]
- Chen, W.; Du, K.; Qiu, X. Analytic properties of American option prices under a modified Black–Scholes equation with spatial fractional derivatives. Phys. A Stat. Mech. Its Appl. 2018, 491, 37–44. [Google Scholar] [CrossRef]
- Huang, J.; Subrahmanyam, M.; Yu, G. Pricing and hedging American options: A recursive integration method. Rev. Financ. Stud. 1996, 9, 277–300. [Google Scholar] [CrossRef]
- Ibáñez, A. Robust pricing of the American put option: A note on Richardson extrapolation and the early exercise premium. Manag. Sci. 2003, 49, 1210–1228. [Google Scholar] [CrossRef]
- Jin, X.; Tan, H.H.; Sun, J. A state-space partitioning method for pricing high-dimensional American-style options. Math. Financ. 2007, 17, 399–426. [Google Scholar] [CrossRef]
- Lim, H.; Lee, S.; Kim, G. Efficient pricing of Bermudan options using recombining quadratures. J. Comput. Appl. Math. 2014, 271, 195–205. [Google Scholar] [CrossRef]
- Omberg, E. A note on the convergence of binomial-pricing and compound-option models. J. Financ. 1987, 42, 463–469. [Google Scholar]
- Prekopa, A.; Szántai, T. On the analytical–numerical valuation of the Bermudan and American options. Quant. Financ. 2010, 10, 59–74. [Google Scholar] [CrossRef]
- Shang, Q.; Byrne, B. American option pricing: Optimal lattice models and multidimensional efficiency tests. J. Futur. Mark. 2021, 41, 514–535. [Google Scholar] [CrossRef]
- Carr, P. Randomization and the American put. Rev. Financ. Stud. 1998, 11, 597. [Google Scholar] [CrossRef]
- Volk-Makarewicz, W.; Borovkova, S.; Heidergott, B. Assessing the impact of jumps in an option pricing model: A gradient estimation approach. Eur. J. Oper. Res. 2022, 298, 740–751. [Google Scholar] [CrossRef]
- Geske, R.; Johnson, H.E. The American put option valued analytically. J. Financ. 1984, 39, 1511–1524. [Google Scholar] [CrossRef]
- Chang, L.B.; Palmer, K. Smooth convergence in the binomial model. Financ. Stoch. 2007, 11, 91–105. [Google Scholar] [CrossRef]
- Joshi, M.S. The convergence of binomial trees for pricing the American put. J. Risk 2009, 11, 87–108. [Google Scholar] [CrossRef]
- Chan, J.H.; Joshi, M.; Tang, R.; Yang, C. Trinomial or binomial: Accelerating American put option price on trees. J. Futur. Mark. 2009, 29, 826–839. [Google Scholar] [CrossRef]
- Leduc, G.; Nurkanovic Hot, M. Joshi’s split tree for option pricing. Risks 2020, 8, 81. [Google Scholar] [CrossRef]
- Leduc, G. A European option general first-order error formula. ANZIAM J. 2013, 54, 248–272. [Google Scholar]
- Leduc, G.; Palmer, K. The convergence rate of option prices in trinomial trees. Risks 2023, 11, 52. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leduc, G. Convergence Speed of Bermudan, Randomized Bermudan, and Canadian Options. Mathematics 2025, 13, 213. https://doi.org/10.3390/math13020213
Leduc G. Convergence Speed of Bermudan, Randomized Bermudan, and Canadian Options. Mathematics. 2025; 13(2):213. https://doi.org/10.3390/math13020213
Chicago/Turabian StyleLeduc, Guillaume. 2025. "Convergence Speed of Bermudan, Randomized Bermudan, and Canadian Options" Mathematics 13, no. 2: 213. https://doi.org/10.3390/math13020213
APA StyleLeduc, G. (2025). Convergence Speed of Bermudan, Randomized Bermudan, and Canadian Options. Mathematics, 13(2), 213. https://doi.org/10.3390/math13020213





