Inequalities of a Class of Analytic Functions Involving Multiplicative Derivative
Abstract
1. Introduction
1.1. Motivation and Novelty
1.2. A Brief Introduction to Multiplicative Calculus
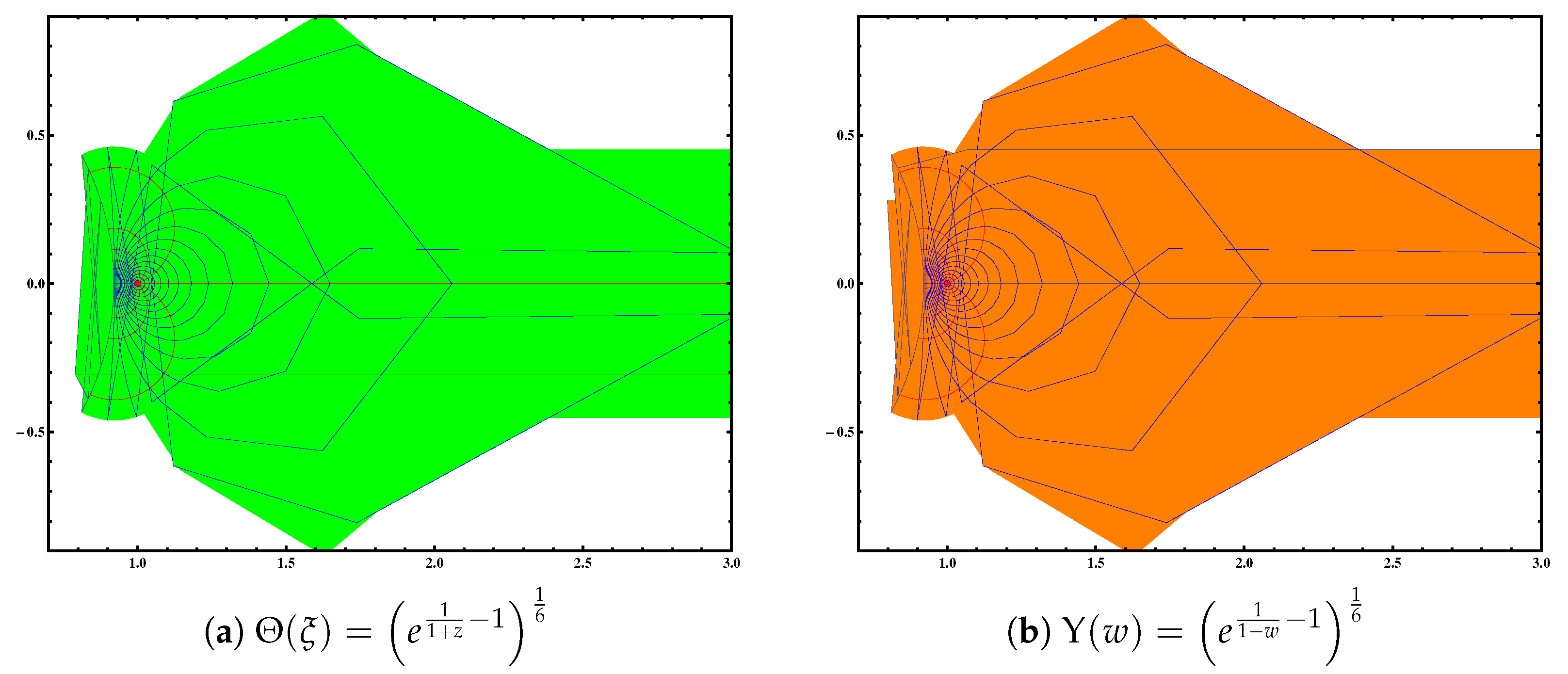
1.3. Definitions
2. Coefficient Estimates for the Class
3. Fekete-Szegő Inequalities
4. Coefficient Estimates for the Class
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Srivastava, H.M. A survey of some recent developments on higher transcendental functions of analytic number theory and applied mathematics. Symmetry 2021, 13, 2294. [Google Scholar] [CrossRef]
- Mittag-Leffler, G.M. Sur la nouvelle fonction Eα(x). Comptes Rendus Acad. Sci. Paris 1903, 137, 554–558. [Google Scholar]
- Wiman, A. Über den Fundamentalsatz in der Teorie der Funktionen Ea(x). Acta Math. 1905, 29, 191–201. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Bansal, M.; Harjule, P. A study of fractional integral operators involving a certain generalized multi-index Mittag-Leffler function. Math. Methods Appl. Sci. 2018, 41, 6108–6121. [Google Scholar] [CrossRef]
- Prabhakar, T.R. A singular integral equation with a generalized Mittag Leffler function in the kernel. Yokohama Math. J. 1971, 19, 7–15. [Google Scholar]
- Murat, C.; Karthikeyan, K.R.; Murugusundaramoorthy, G. Inequalities on a class of analytic functions defined by generalized Mittag-Leffler function. Filomat 2024, 37, 6277–6288. [Google Scholar]
- Ibrahim, R.W.; Darus, M. Subordination inequalities of a new Salagean-difference operator. Int. J. Math. Comput. Sci. 2019, 14, 573–582. [Google Scholar]
- Ibrahim, R.W. On a Janowski formula based on a generalized differential operator. Commun. Fac. Sci. Univ. Ank. Ser. A1 Math. Stat. 2020, 69, 1320–1328. [Google Scholar]
- Umadevi, E.; Karthikeyan, K.R. A subclass of close-to-convex function involving Srivastava-Tomovski operator. In Recent Developments in Algebra and Analysis, Proceedings of the International Conference on Recent Developments in Mathematics (ICRDM), Dubai, United Arab Emirates, 24–26 August 2022; Leung, H.H., Sivaraj, R., Kamalov, F., Eds.; ICRDM 2022. Trends in Mathematics; Birkhäuser: Cham, Switzerland, 2024. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Mishra, A.K.; Gochhayat, P. Certain subclasses of analytic and bi-univalent functions. Appl. Math. Lett. 2010, 23, 1188–1192. [Google Scholar] [CrossRef]
- Altinkaya, S.; Yalcin, S. Coefficient estimates for a certain subclass of analytic and bi-univalent functions. Acta Univ. Apulensis Math. Inform. 2014, 40, 347–354. [Google Scholar]
- Ali, R.M.; Lee, S.K.; Ravichandran, V.; Supramaniam, S. Coefficient estimates for bi-univalent Ma-Minda starlike and convex functions. Appl. Math. Lett. 2012, 25, 344–351. [Google Scholar] [CrossRef]
- Karthikeyan, K.R.; Murugusundaramoorthy, G. Properties of a class of analytic functions influenced by multiplicative calculus. Fractal Fract. 2024, 8, 131. [Google Scholar] [CrossRef]
- Breaz, D.; Karthikeyan, K.R.; Murugusundaramoorthy, G. Applications of Mittag–Leffler functions on a subclass of meromorphic functions influenced by the definition of a non-Newtonian derivative. Fractal Fract. 2024, 8, 509. [Google Scholar] [CrossRef]
- Bashirov, A.E.; Kurpinar, E.M.; Özyapıcı, A. Multiplicative calculus and its applications. J. Math. Anal. Appl. 2008, 337, 36–48. [Google Scholar] [CrossRef]
- Bashirov, A.E.; Mısırlı, E.; Tandoğdu, Y.; Özyapıcı, A. On modeling with multiplicative differential equations. Appl. Math. J. Chin. Univ. 2011, 26, 425–438. [Google Scholar] [CrossRef]
- Bashirov, A.E.; Riza, M. On complex multiplicative differentiation. TWMS J. Appl. Eng. Math. 2011, 1, 75–85. [Google Scholar]
- Riza, M.; Özyapici, A.; Mısırlı, E. Multiplicative finite difference methods. Quart. Appl. Math. 2009, 67, 745–754. [Google Scholar] [CrossRef]
- Karthikeyan, K.R.; Varadharajan, S. A class of analytic functions with respect to symmetric points involving multiplicative derivative. Commun. Appl. Nonlinear Anal. 2024, 31, 540–551. [Google Scholar] [CrossRef]
- Murugusundaramoorthy, G.; Vijaya, K.; Karthikeyan, K.R.; El-Deeb, S.M.; Ro, J.-S. Bi-univalent functions subordinated to a three leaf function induced by multiplicative calculus. AIMS Math. 2024, 9, 26983–26999. [Google Scholar] [CrossRef]
- Pommerenke, C. Univalent Functions; Vandenhoeck & Ruprecht: Göttingen, Germany, 1975. [Google Scholar]
- Zaprawa, P. On the Fekete-Szegő problem for classes of bi-univalent functions. Bull. Belg. Math. Soc. Simon Stevin 2014, 21, 169–178. [Google Scholar] [CrossRef]
- Zaprawa, P. Estimates of initial coefficients for bi-univalent functions. Abst. Appl. Anal. 2014, 6, 357480. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karthikeyan, K.R.; Breaz, D.; Murugusundaramoorthy, G.; Thirupathi, G. Inequalities of a Class of Analytic Functions Involving Multiplicative Derivative. Mathematics 2025, 13, 1606. https://doi.org/10.3390/math13101606
Karthikeyan KR, Breaz D, Murugusundaramoorthy G, Thirupathi G. Inequalities of a Class of Analytic Functions Involving Multiplicative Derivative. Mathematics. 2025; 13(10):1606. https://doi.org/10.3390/math13101606
Chicago/Turabian StyleKarthikeyan, Kadhavoor R., Daniel Breaz, Gangadharan Murugusundaramoorthy, and Ganapathi Thirupathi. 2025. "Inequalities of a Class of Analytic Functions Involving Multiplicative Derivative" Mathematics 13, no. 10: 1606. https://doi.org/10.3390/math13101606
APA StyleKarthikeyan, K. R., Breaz, D., Murugusundaramoorthy, G., & Thirupathi, G. (2025). Inequalities of a Class of Analytic Functions Involving Multiplicative Derivative. Mathematics, 13(10), 1606. https://doi.org/10.3390/math13101606








