Riccati-Type Pseudo-Potential Approach to Quasi-Integrability of Deformed Soliton Theories
Abstract
:1. Introduction
2. Riccati-Type Pseudo-Potentials and Modified Integrable Models
3. Deformation of the Sine-Gordon Model
3.1. Riccati-Type Pseudo-Potential and Conservation Laws
3.2. Riccati-Type Pseudo-Potential and Dual Conservation Laws
3.3. Pseudo-Potentials and a Linear System Associated to DSG
Non-Local Conservation Laws
4. Modified NLS Model as Reduction of the Deformed AKNS Model
Dual Riccati-Type Formulation and Novel Anomalous Charges
5. Riccati-Type Pseudo-Potentials and Deformations of KdV
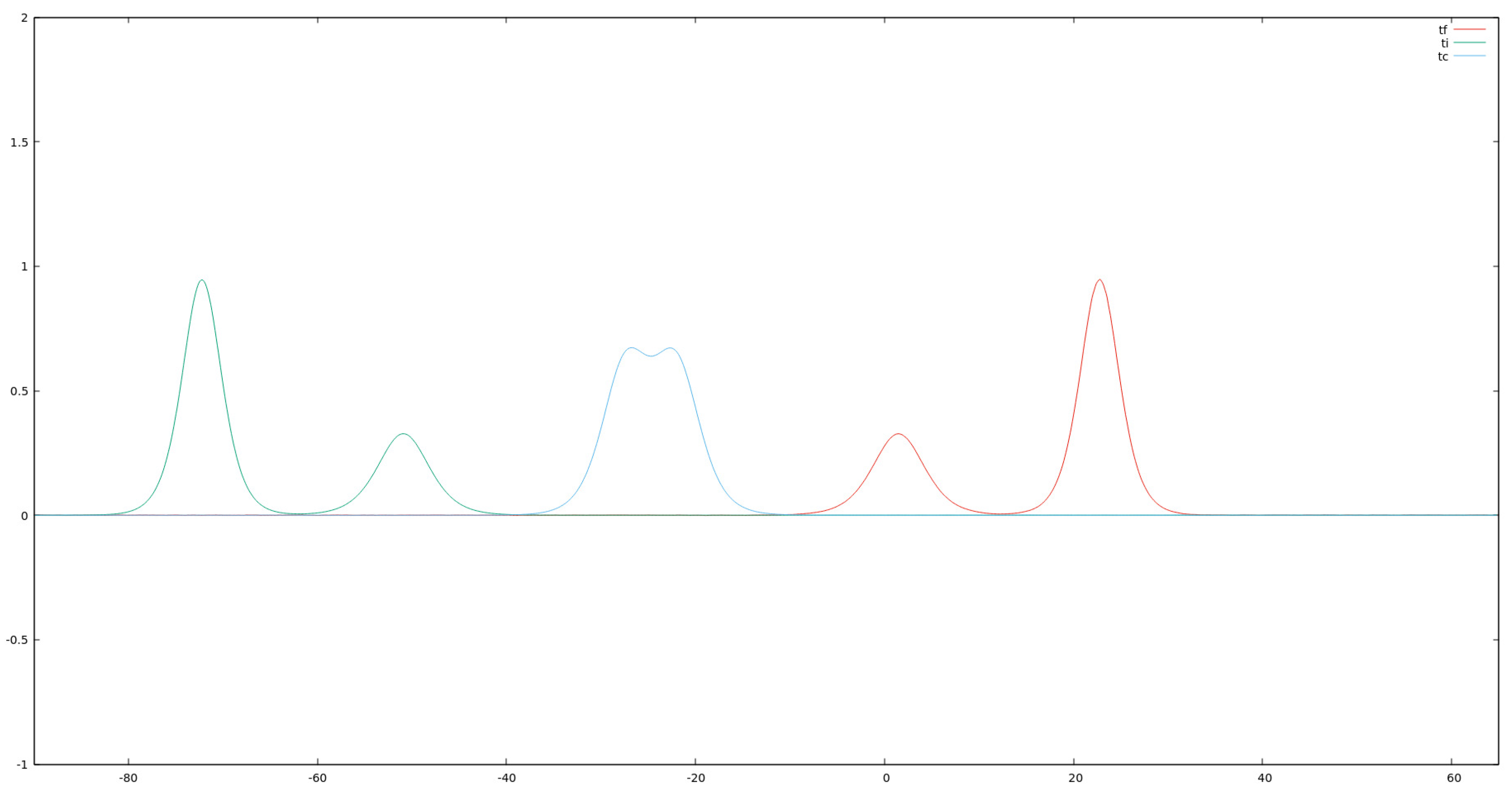
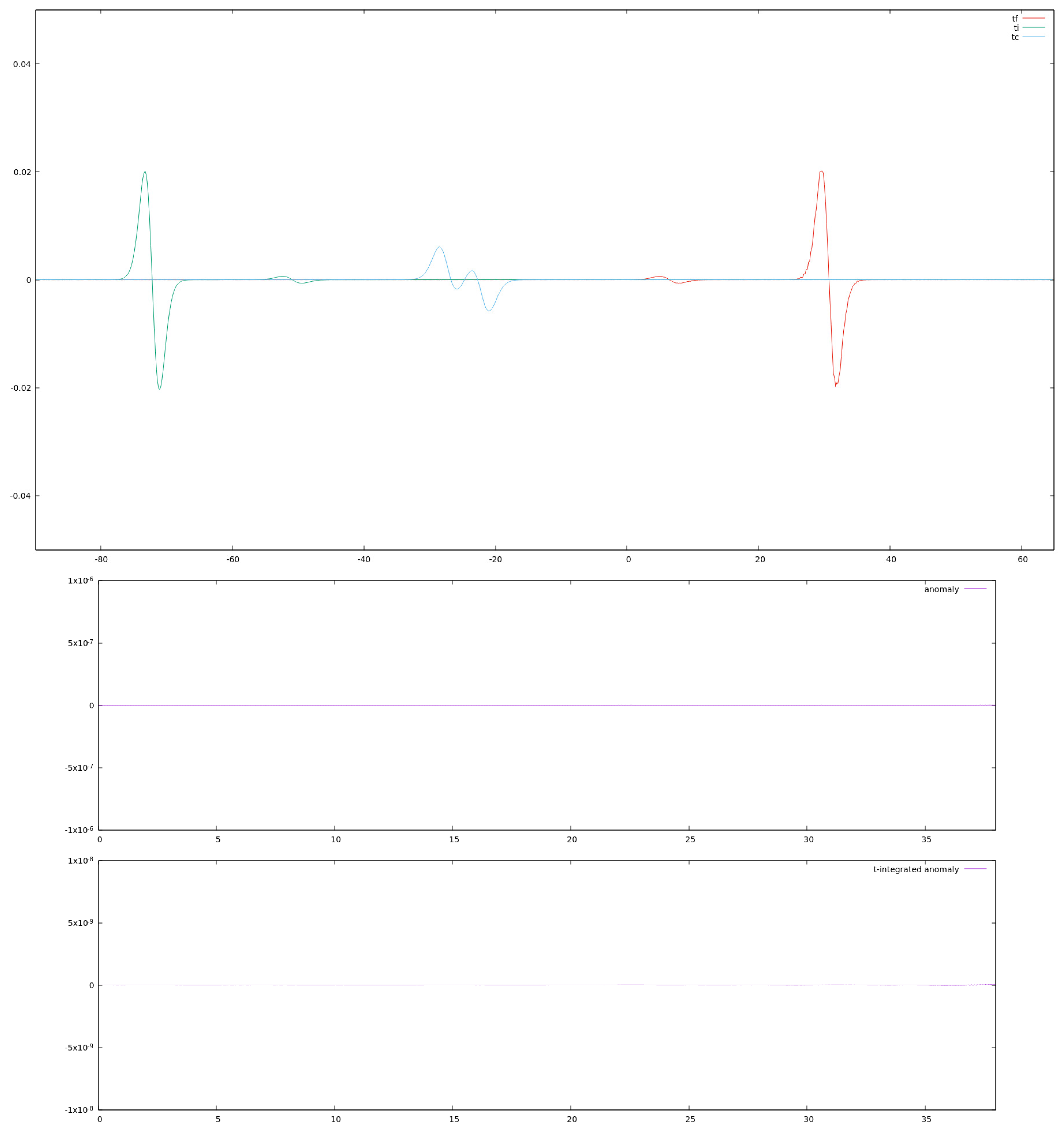
Particular Deformations of the KdV
6. Conclusions and Discussions
Funding
Conflicts of Interest
References
- Ferreira, L.A.; Zakrzewski, W.J. The concept of quasi-integrability: A concrete example. J. High Energy Phys. 2011, 5, 130. [Google Scholar] [CrossRef]
- Ferreira, L.A.; Zakrzewski, W.J. Numerical and analytical tests of quasi-integrability in modified sine-Gordon models. J. High Energy Phys. 2014, 1, 58. [Google Scholar] [CrossRef]
- Ferreira, L.A.; Zakrzewski, W.J. Breather-like structures in modified sine-Gordon models. Nonlinearity 2016, 29, 1622. [Google Scholar] [CrossRef]
- Aurichio, V.H.; Ferreira, L.A. Quasi-integrable deformations of the Bullough-Dodd model. J. High Energy Phys. 2015, 3, 152. [Google Scholar] [CrossRef]
- Ferreira, L.A.; Luchini, G.; Zakrzewski, W.J. The concept of quasi-integrability for modified non-linear Schrödinger models. J. High Energy Phys. 2012, 9, 103. [Google Scholar] [CrossRef]
- Ferreira, L.A.; Klimas, P.; Zakrzewskic, W.J. Quasi-integrable deformations of the SU(3) Affine Toda theory. J. High Energy Phys. 2016, 5, 65. [Google Scholar] [CrossRef]
- Blas, H.; Callisaya, H.F. Quasi-integrability in deformed sine-Gordon models and infinite towers of conserved charges. Commun. Nonlinear Sci. Num. Simulat. 2018, 55, 105. [Google Scholar] [CrossRef]
- Blas, H.; Zambrano, M. Quasi-integrability in the modified defocusing non-linear Schrödinger model and dark solitons. J. High Energy Phys. 2016, 3, 5. [Google Scholar] [CrossRef]
- Blas, H.; Zambrano, M. Modified non-linear Schrödinger models, infinite tower of conserved charges and dark solitons. J. Phys. Conf. Ser. 2018, 1143, 12004. [Google Scholar] [CrossRef]
- Blas, H.; do Bonfim, A.C.R.; Vilela, A.M. Quasi-integrable non-linear Schrödinger models, infinite towers of exactly conserved charges and bright solitons. J. High Energy Phys. 2017, 5, 106. [Google Scholar] [CrossRef]
- Abhinav, K.; Guha, P. Quasi-integrability in supersymmetric sine-Gordon models. Europhys. Lett. 2016, 116, 10004. [Google Scholar] [CrossRef]
- ter Braak, F.; Ferreira, L.A.; Zakrzewski, W.J. Quasi-integrability of deformations of the KdV equation. Nucl. Phys. 2019, 939B, 49. [Google Scholar] [CrossRef]
- Blas, H.; Callisaya, H.F.; Campos, J.P.R. Riccati-type pseudo-potentials, conservation laws and solitons of deformed sine-Gordon models. Nucl. Phys. 2020, 950B, 114852. [Google Scholar] [CrossRef]
- Blas, H.; Ochoa, R.; Suarez, D. Quasi-integrable KdV models, towers of infinite number of anomalous charges and soliton collisions. J. High Energy Phys. 2020, 3, 136. [Google Scholar] [CrossRef]
- Blas, H.; Ochoa, R.; Suarez, D. Deformations of KdV and soliton collisions. J. Phys. Conf. Ser. 2020, 1558, 12012. [Google Scholar] [CrossRef]
- Blas, H.; Cerna Maguiña, M.; dos Santos, L. Modified nonlinear Schrödinger models, CPsTd invariant N-bright solitons and infinite towers of anomalous charges. Int. J. Mod. Phys. 2021, 35B, 2150272. [Google Scholar] [CrossRef]
- Blas, H.; Cerna Maguiña, M.; dos Santos, L. Modified AKNS model, Riccati-type pseudo-potential approach and infinite towers of quasi-conservation laws. Int. J. Mod. Phys. 2022, 36B, 2250070. [Google Scholar] [CrossRef]
- Hietarinta, J. Hirota’s bilinear method and partial integrability. In Partially Integrable Evolution Equations in Physics; Conte, R., Boccara, N., Eds.; NATO ASI Series C310; Springer: Dordrecht, The Netherlands, 1990; pp. 459–478. [Google Scholar]
- Das, A. Integrable Models; World Scientific: Teaneck, NJ, USA, 1989. [Google Scholar]
- Faddeev, L.D.; Takhtajan, L.A. Hamiltonian Methods in the Theory of Solitons; Springer: Berlin, Germany, 2007. [Google Scholar]
- Abdalla, E.; Abadalla, M.B.; Rothe, D. Non-Perturbative Methods in Two-Dimensional Quantum Field Theory, 2nd ed.; World Scientific: Singapore, 2001. [Google Scholar]
- Babelon, O.; Bernard, D. The sine-Gordon solitons as an N-body problem. Phys. Lett. 1993, 317B, 363. [Google Scholar] [CrossRef]
- Babelon, O.; Bernard, D.; Smirnov, F.A. Quantization of solitons and the restricted sine-Gordon model. Commun. Math. Phys. 1996, 182, 319. [Google Scholar] [CrossRef]
- Blas, H.; Callisaya, H.F.; Campos, J.P.R.; Cerna, M.; Reyes, C. Deformed Sine-Gordon Models, Solitons and Anomalous Charges. In Advances in the Solution of Nonlinear Differential Equations; Carpentieri, B., Ed.; IntechOpen: London, UK, 2021; Volume 1, pp. 1–21. [Google Scholar]
- Nucci, M.C. Pseudopotentials, Lax equations and Backlund transformations for nonlinear evolution equations. J. Phys. A Math. Gen. 1988, 21A, 73. [Google Scholar] [CrossRef]
- Nucci, M.C. Riccati-type pseudopotentials and their applications. In Nonlinear Equations in the Applied Sciences; Ames, W.F., Rogers, C., Eds.; Academic Press, Inc.: London, UK, 1992. [Google Scholar]
- Chen, H.-H. General Derivation of Bäcklund Transformations from Inverse Scattering Problems. Phys. Rev. Lett. 1974, 33, 925. [Google Scholar] [CrossRef]
- Wadati, M.; Sanuki, H.; Konno, K. Relationships among Inverse Method, Bäcklund Transformation and an Infinite Number of Conservation Laws. Prog. Theor. Phys. 1975, 53, 419. [Google Scholar] [CrossRef]
- Blas, H. Asymptotically Conserved Charges and 2-Kink Collision in Quasi-integrable Potential KdV Models. Braz. J. Phys. 2024, 54, 146. [Google Scholar] [CrossRef]
- Abhinav, K.; Guha, P. On quasi-integrable deformation scheme of the KdV system. Sci. Rep. 2025, 15, 2402. [Google Scholar] [CrossRef] [PubMed]
- Blas, H.; Cerna, M.; dos Santos, L. Modified Non-linear Schrödinger Models, Symmetry, Dark Solitons, and Infinite Towers of Anomalous Charges. In Advances in Nonlinear Dynamics; Lacarbonara, W., Balachandran, B., Leamy, M.J., Ma, J., Tenreiro Machado, J.A., Stepan, G., Eds.; NODYCON Conference Proceedings Series; Springer: Cham, Switzerland, 2022. [Google Scholar] [CrossRef]
- Hille, E. Ordinary Differential Equations in the Complex Domain; Wiley & Sons: New York, NY, USA, 1976. [Google Scholar]
- Nucci, M.C. Painleve property and pseudopotentials for nonlinear evolution equations. J. Phys. A Math. Gen. 1989, 22A, 2897. [Google Scholar] [CrossRef]
- Ikhdair, S.M.; Sever, R. Exact quantization rule to the Kratzer-type potentials: An application to the diatomic molecules. J. Math. Chem. 2009, 45, 1137–1152. [Google Scholar] [CrossRef]
- Ma, Z.-Q.; Xu, B.-W. Quantum correction in exact quantization rules. Europhys. Lett. 2005, 69, 685. [Google Scholar] [CrossRef]
- Qiang, W.-C.; Dong, S.-H. Proper quantization rule. Europhys. Lett. 2010, 89, 10003. [Google Scholar] [CrossRef]
- Sanuki, H.; Konno, K. Conservation laws of sine-Gordon equation. Phys. Lett. 1974, 48A, 221. [Google Scholar] [CrossRef]
- Blas, H.; Callisaya, H.F. Research Highlight: “An Exploration of Kinks/Anti-Kinks and Breathers in Deformed Sine-Gordon Models” in Advances in Engineering. Available online: https://advanceseng.com/kinks-anti-kinks-breathers-deformed-sine-gordon-models (accessed on 1 March 2025).
- Brezin, E.; Itzykson, C.; Zinn-Justin, J.; Zuber, J.-B. Remarks about the existence of non-local charges in two-dimensional models. Phys. Lett. 1979, 82B, 442. [Google Scholar] [CrossRef]
- Ablowitz, M.J.; Musslimani, Z.H. Integrable Nonlocal Nonlinear Equations. Stud. Appl. Math. 2017, 139, 7. [Google Scholar] [CrossRef]
- Ablowitz, M.J.; Musslimani, Z.H. Integrable Nonlocal Nonlinear Schrödinger Equation. Phys. Rev. Lett. 2013, 110, 64105. [Google Scholar] [CrossRef]
- Nian, J. Gravity dual of two-dimensional N=(2,2)★ supersymmetric Yang-Mills theory and integrable models. J. High Energy Phys. 2018, 3, 32. [Google Scholar] [CrossRef]
- Lou, S.Y.; Huang, F. Alice-Bob Physics: Coherent Solutions of Nonlocal KdV Systems. Sci. Rep. 2017, 7, 869. [Google Scholar] [CrossRef] [PubMed]
- Jia, M.; Lou, S.Y. Exact PsTd invariant and PsTd symmetric breaking solutions, symmetry reductions and Bäcklund transformations for an AB–KdV system. Phys. Lett. 2018, 382A, 1157. [Google Scholar] [CrossRef]
- Ojeda, E.; Perez, A. Boundary conditions for General Relativity in three-dimensional spacetimes, integrable systems and the KdV/mKdV hierarchies. J. High Energy Phys. 2019, 8, 79. [Google Scholar] [CrossRef]
- Krasilshchik, I.S.; Vinogradov, A.M. Nonlocal trends in the geometry of differential equations: Symmetries, conservation laws, and Bäcklund transformations. Acta Appl. Math. 1989, 15, 161. [Google Scholar] [CrossRef]
- Krasilshchik, I.S.; Vinogradov, A.M. (Eds.) Symmetries and Conservation Laws for Differential Equations of Mathematical Physics; AMS: Providence, RI, USA, 1999. [Google Scholar]
- Igonin, S. Coverings and fundamental algebras for partial differential equations. J. Geom. Phys. 2006, 56, 939. [Google Scholar] [CrossRef]
- Morozov, O.I. The four-dimensional Martínez Alonso–Shabat equation: Differential coverings and recursion operators. J. Geom. Phys. 2014, 85, 75. [Google Scholar] [CrossRef]
- Blas, H.; Monsalve, J.J.; Quicaño, R.; Pereira, J.R.V. Majorana zero mode-soliton duality and in-gap and BIC bound states in modified Toda model coupled to fermion. J. High Energy Phys. 2022, 9, 82. [Google Scholar] [CrossRef]
- Eneias, R.; Ferraz, A. BCS coupling in a 1D Luttinger liquid. New J. Phys. 2015, 17, 123006. [Google Scholar] [CrossRef]
- Wieckowski, A.; Maska, M.M.; Mierzejewski, M. Identification of Majorana Modes in Interacting Systems by Local Integrals of Motion. Phys. Rev. Lett. 2018, 120, 040504. [Google Scholar] [CrossRef]
- Blas, H. Biorthogonal Majorana zero modes, ELC waves and soliton-fermion duality in non-Hermitian sl(2) affine Toda coupled to fermions. J. High Energy Phys. 2024, 6, 7. [Google Scholar] [CrossRef]
- Frantzeskakis, D.J. Small-amplitude solitary structures for an extended nonlinear Schrödinger equation. J. Phys. 1996, 29, 3631. [Google Scholar] [CrossRef]
- Kevrekidis, P.G.; Frantzeskakis, D.J. Multiple dark solitons in Bose-Einstein condensates at finite temperature. Discret. Contin. Dyn. Syst. 2011, 4, 1199. [Google Scholar] [CrossRef]
- Gurevich, A.; Vinokur, V.M. Interband Phase Modes and Nonequilibrium Soliton Structures in Two-Gap Superconductors. Phys. Rev. Lett. 2003, 90, 47004. [Google Scholar] [CrossRef]
- Tanaka, Y. Soliton in Two-Band Superconductor. Phys. Rev. Lett. 2002, 88, 17002. [Google Scholar] [CrossRef]
- Agafontsev, D.S.; Zakharov, V.E. Integrable turbulence generated from modulational instability of cnoidal waves. Nonlinearity 2016, 29, 3551. [Google Scholar] [CrossRef]
- Agafontsev, D.S.; Randoux, S.; Suret, P. Integrable turbulence developing from strongly nonlinear partially coherent waves. Phys. Rev. 2021, 103E, 032209. [Google Scholar] [CrossRef]
- Pelinovsky, E.N.; Shurgalina, E.G.; Sergeeva, A.V.; Talipova, T.G.; El, G.A.; Grimshaw, R.H.J. Two-soliton interaction as an elementary act of soliton turbulence in integrable systems. Phys. Lett. 2013, 377A, 272. [Google Scholar] [CrossRef]
- Pelinovsky, E.N.; Shurgalina, E.G. Two-Soliton Interaction Within the Framework of the Modified Korteweg–de Vries Equation. Radiophys. Quantum Electron. 2015, 57, 737. [Google Scholar] [CrossRef]
- Roberti, G.; El, G.; Randoux, S.; Suret, P. Early stage of integrable turbulence in the one-dimensional nonlinear Schrödinger equation: A semiclassical approach to statistics. Phys. Rev. 2019, 100E, 32212. [Google Scholar] [CrossRef] [PubMed]
- Gelash, A.A.; Agafontsev, D.S. Strongly interacting soliton gas and formation of rogue waves. Phys. Rev. 2018, 98E, 42210. [Google Scholar] [CrossRef]
- Redor, I.; Barthelemy, E.; Michallet, H.; Onorato, M.; Mordant, N. Experimental Evidence of a Hydrodynamic Soliton Gas. Phys. Rev. Lett. 2019, 122, 214502. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Blas, H. Riccati-Type Pseudo-Potential Approach to Quasi-Integrability of Deformed Soliton Theories. Mathematics 2025, 13, 1564. https://doi.org/10.3390/math13101564
Blas H. Riccati-Type Pseudo-Potential Approach to Quasi-Integrability of Deformed Soliton Theories. Mathematics. 2025; 13(10):1564. https://doi.org/10.3390/math13101564
Chicago/Turabian StyleBlas, Harold. 2025. "Riccati-Type Pseudo-Potential Approach to Quasi-Integrability of Deformed Soliton Theories" Mathematics 13, no. 10: 1564. https://doi.org/10.3390/math13101564
APA StyleBlas, H. (2025). Riccati-Type Pseudo-Potential Approach to Quasi-Integrability of Deformed Soliton Theories. Mathematics, 13(10), 1564. https://doi.org/10.3390/math13101564





