Exploring Dynamic Spalling Behavior in Rock–Shotcrete Combinations: A Theoretical and Numerical Investigation
Abstract
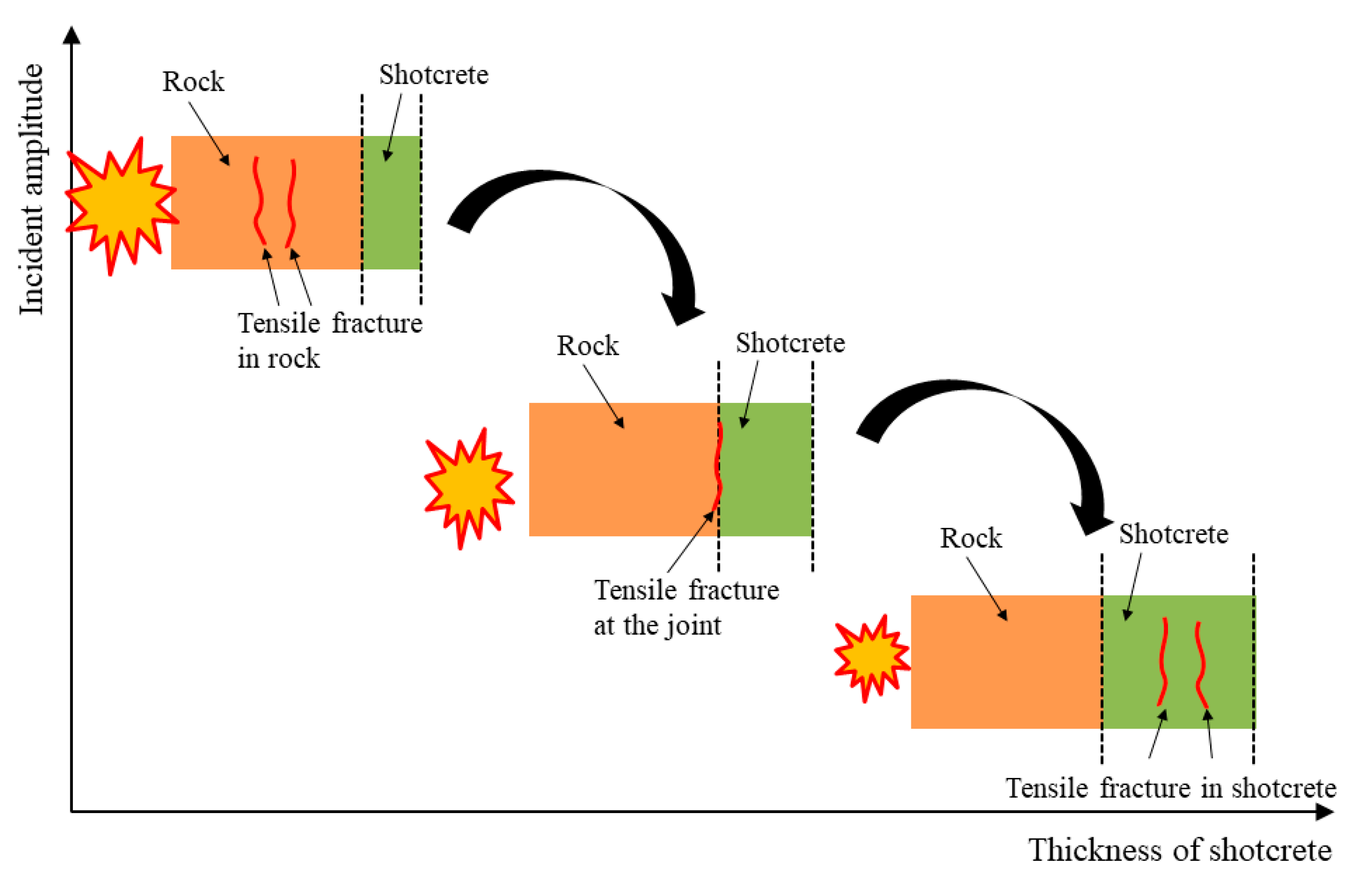
1. Introduction
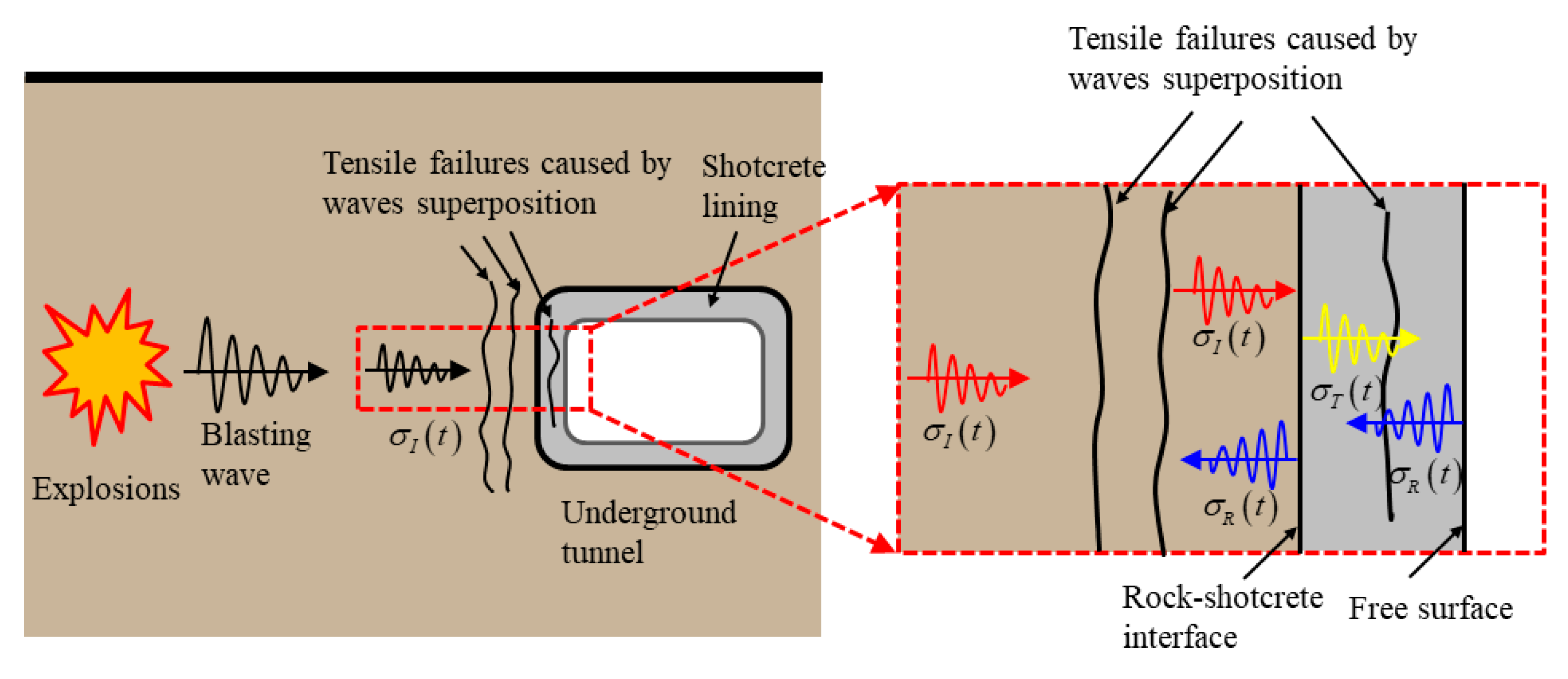
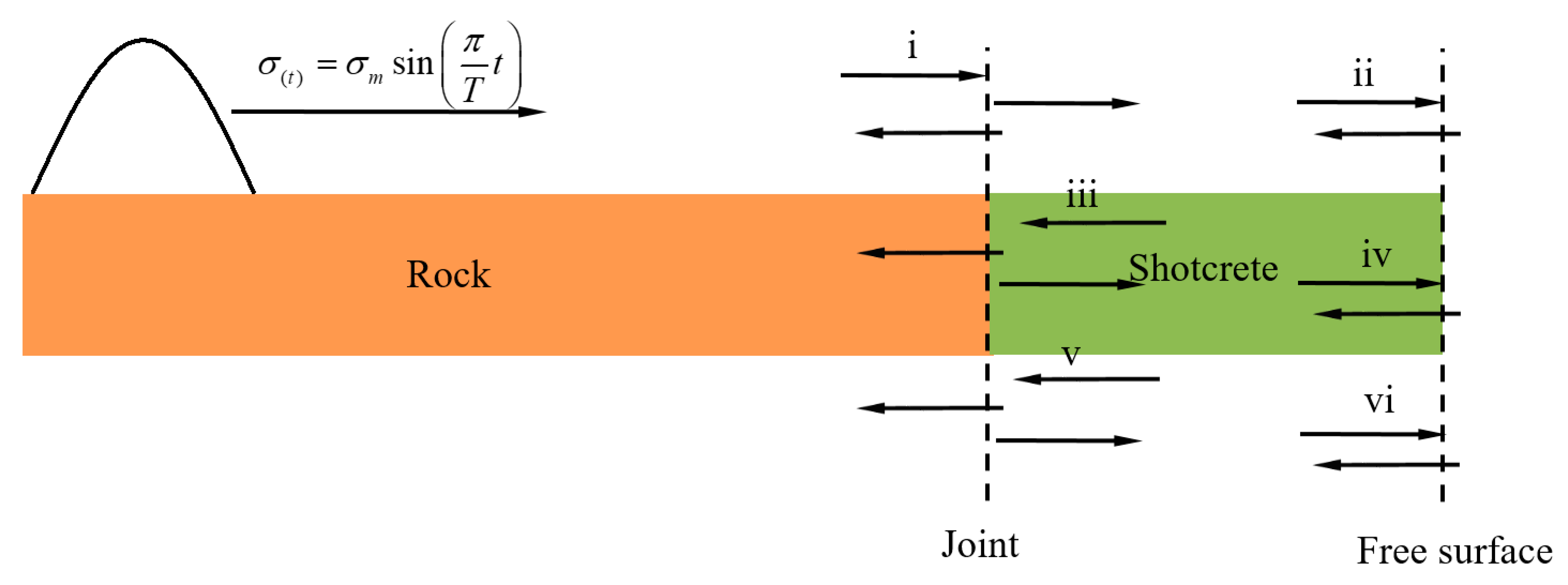
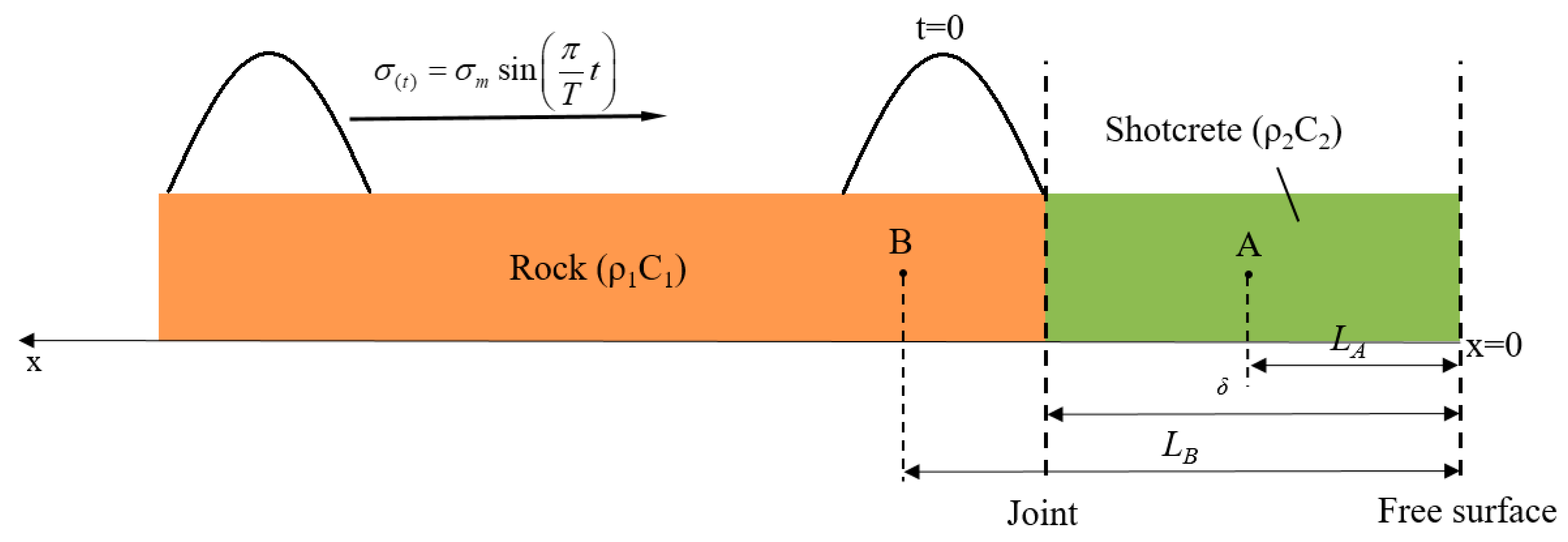
2. Mathematical Representation of Stress Wave Propagation in Rock–Shotcrete Interface Bar
2.1. Propagation Process of Stress Wave in Rock–Shotcrete Combination
2.2. Overview of Reflection and Transmission Coefficients
2.3. Stress Wave Representation in Rock–Shotcrete Combination
2.4. Modifications of Stress Wave Due to the Nonlinear Elastic Behavior of Joints
3. Theoretical Prediction for Initial Spalling of Rock–Shotcrete Combination
4. Comparison between Numerical Investigation and Theoretical Investigation
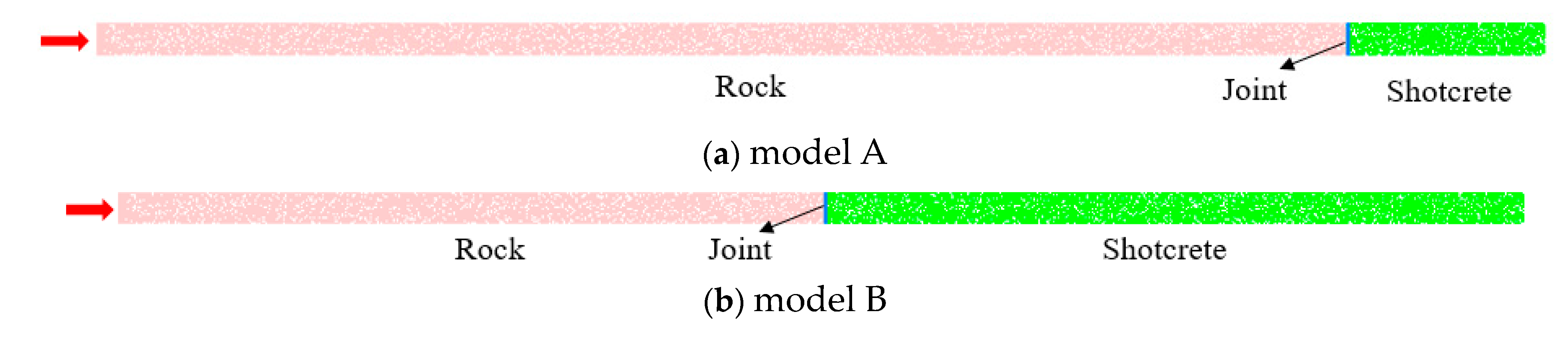
4.1. Numerical Modeling
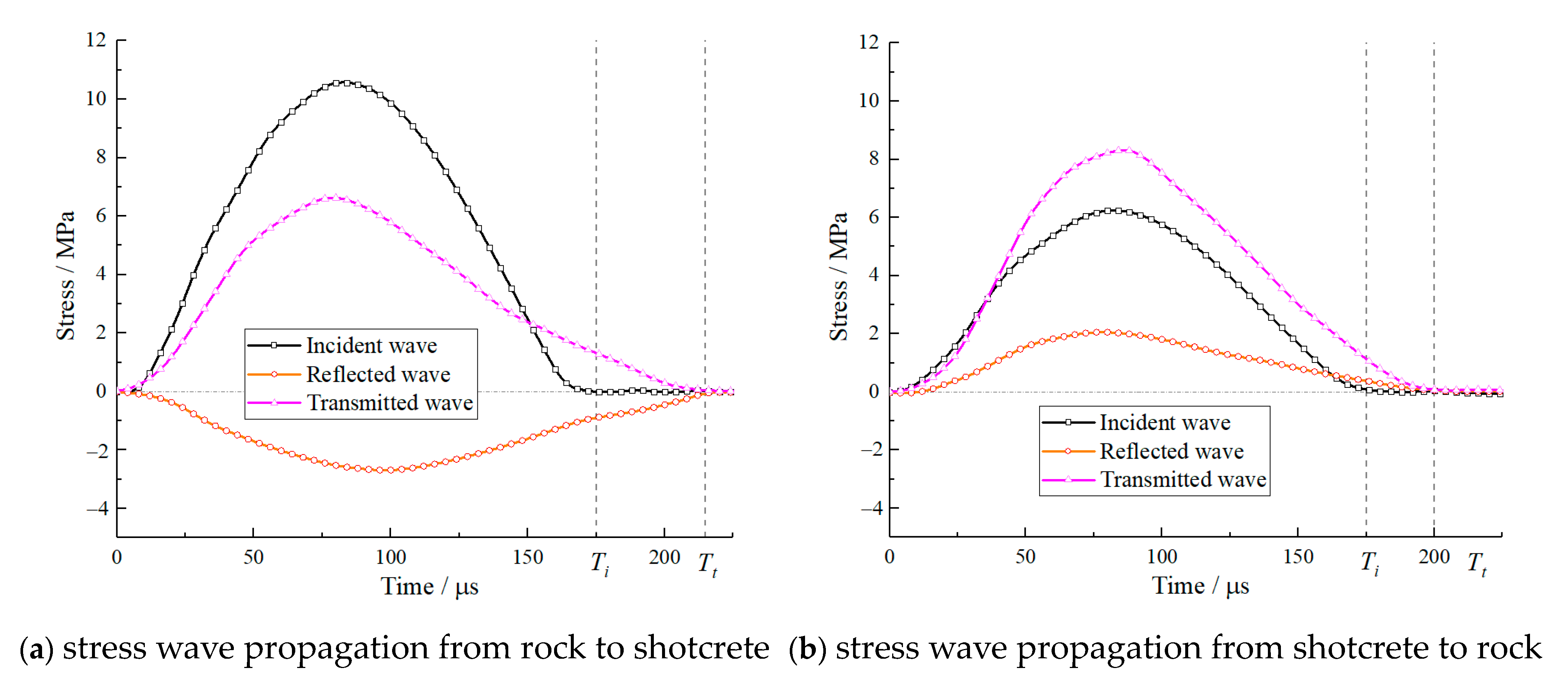
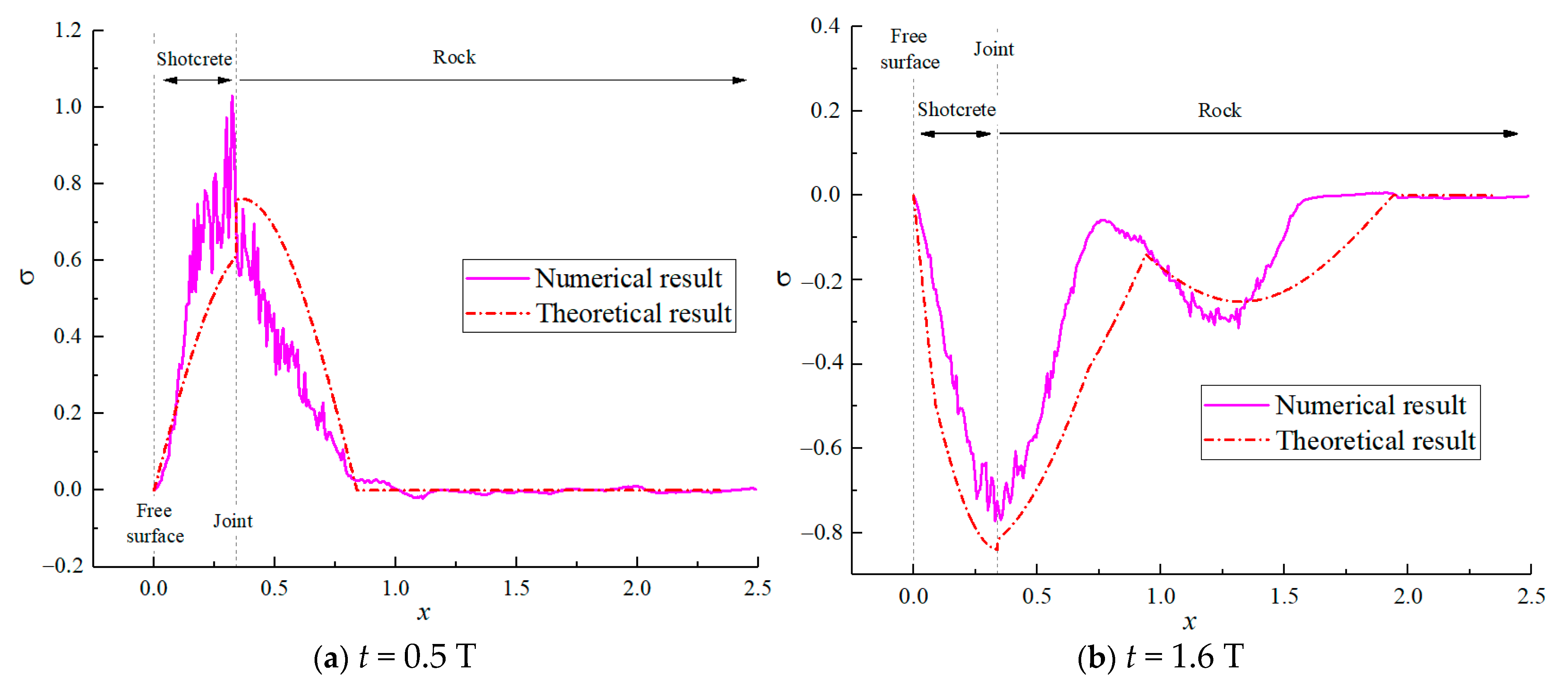
4.2. Stress Evolution Characteristics
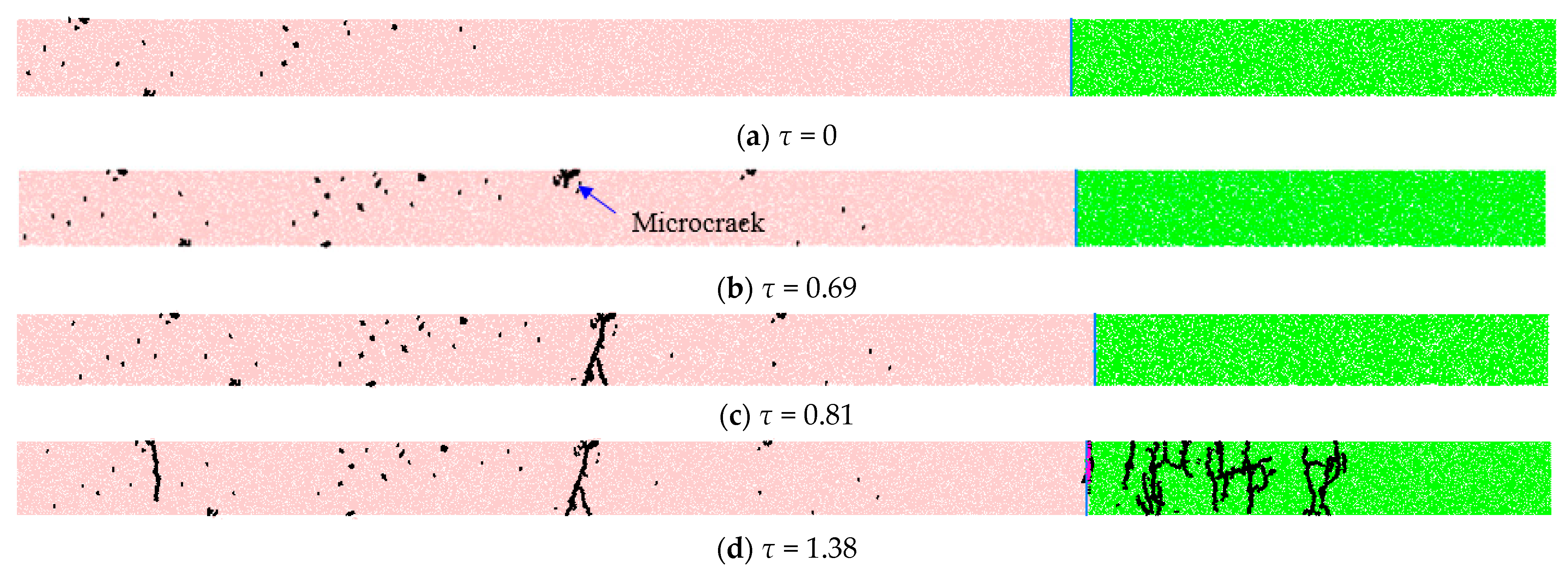
4.3. Spalling Characteristics
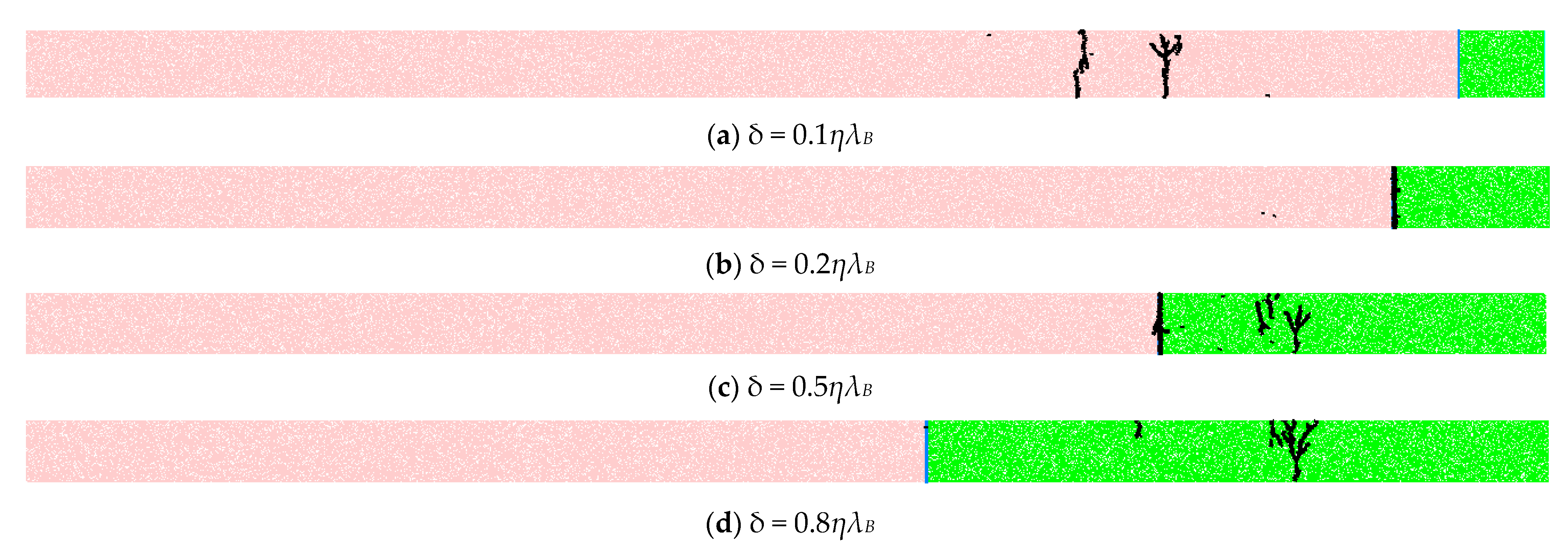
5. Influence of the Thickness of Shotcrete
5.1. Influence of the Thickness of Shotcrete on Stress Evolution
- (a)
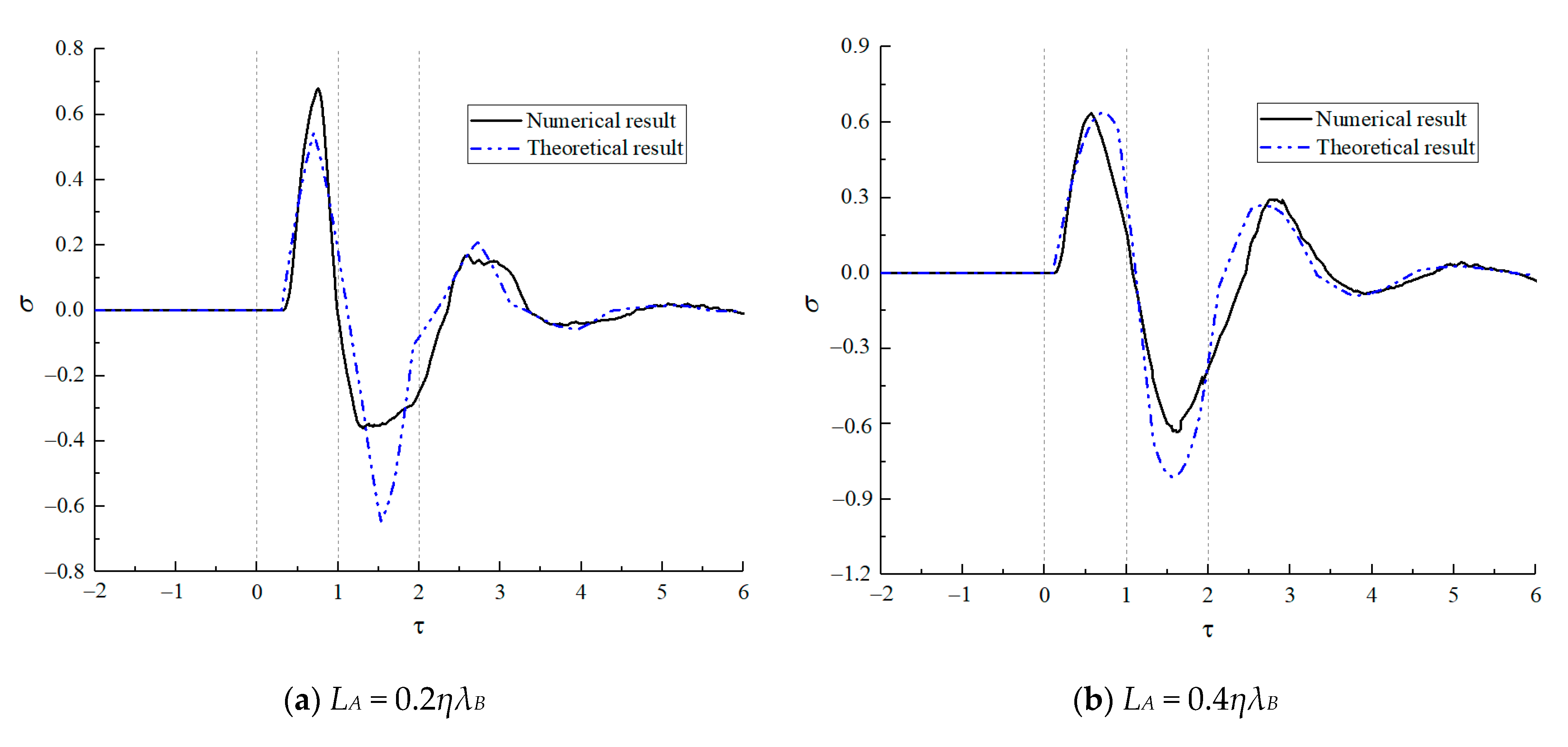
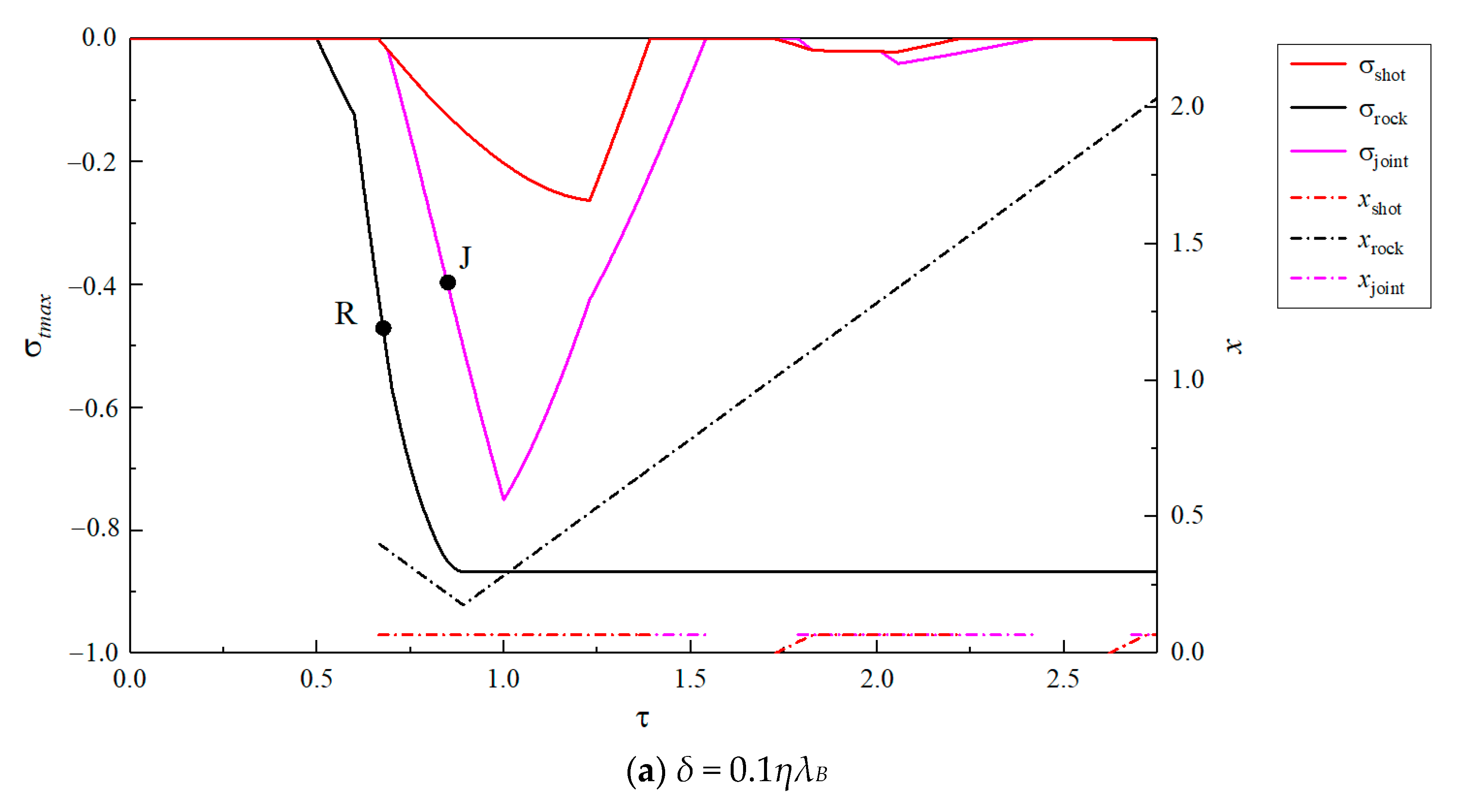
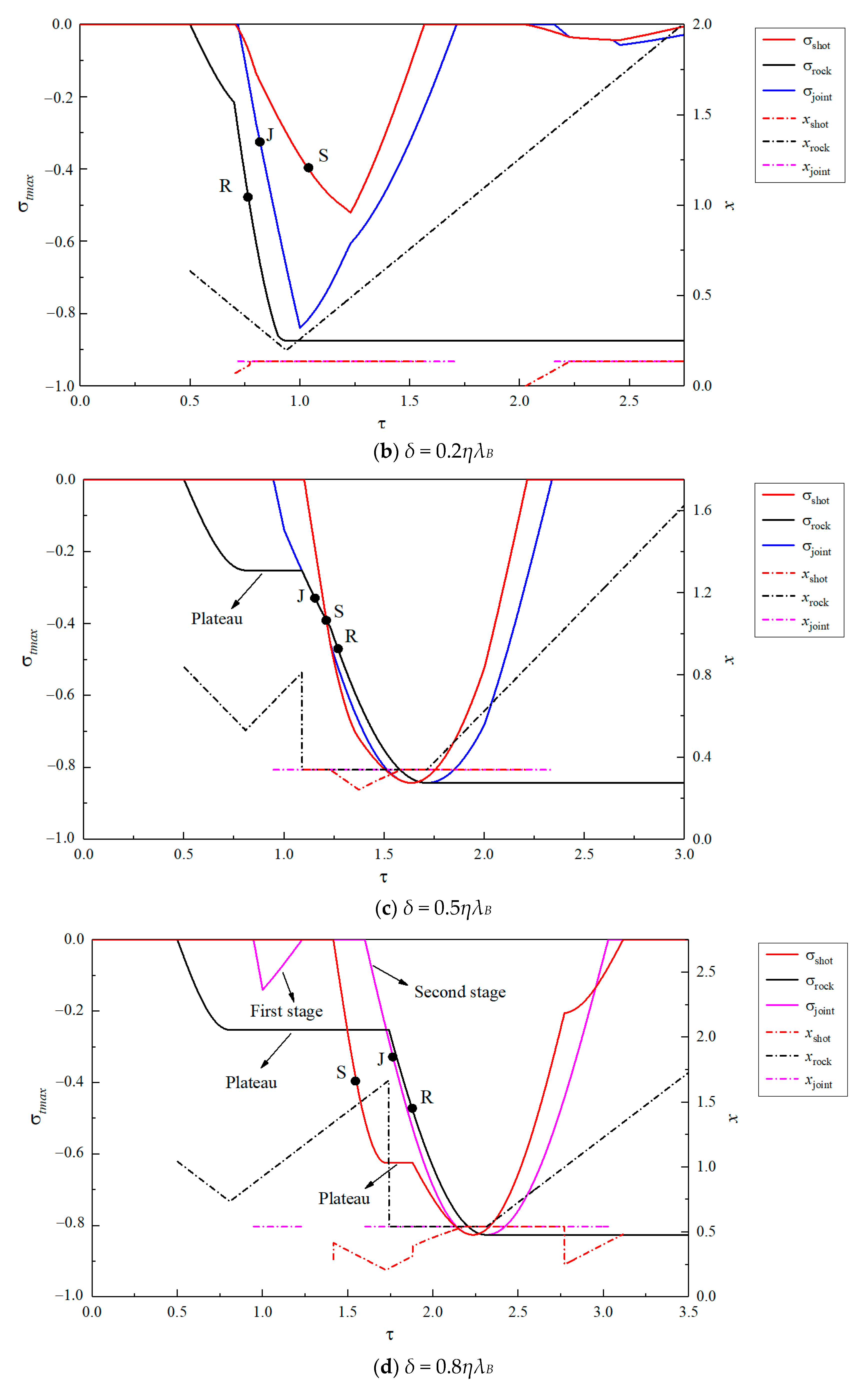
- From Figure 14, we observed that the tensile stress always occurred first in the rock, while after a certain time interval, the tensile stress appeared in the shotcrete and at the joint. This result was obtained because the reflected wave in the rock was the tensile stress wave after the incident wave reached the joint for the first time, while in the shotcrete, the transmitted wave was the compressive stress wave. Thus, for generating the tensile stress in the shotcrete, one reflection is required to occur on the free surface at least, and the actual time of the net tensile stress lags behind the reflection time on the free surface due to the superposition effect with the original compression wave. Moreover, if the shotcrete was not long enough, it might take a considerable time for the total stress to change to tensile stress. At the joint, the tensile stress primarily depends on the stress difference between the two sides. In conclusion, for the thin shotcrete (e.g., δ = 0.1ηλB and δ = 0.2ηλB), the tensile stress at the joint and in the shotcrete appeared practically simultaneously. However, for the thick shotcrete (e.g., δ = 0.5ηλB and δ = 0.8ηλB), the tensile stress of the joint preceded the shotcrete, as shown in Figure 14.
- (b)
- For the thick shotcrete (e.g., δ = 0.5ηλB and δ = 0.8ηλB), there was a plateau in the stress–time curves of rock and shotcrete. However, this phenomenon did not occur in the thin shotcrete (e.g., δ = 0.1ηλB and δ = 0.2ηλB) because when the shotcrete was thick, it took a long time to generate new stress waves from the joint. Therefore, the original stress waves in the rock and shotcrete propagated stably for a period until the subsequent waves were sufficiently superimposed with them.
- (c)
- When the thickness was small (e.g., δ = 0.1ηλB and δ = 0.2ηλB), the tensile stress of the shotcrete was lower than that of the joint, and the tensile stress of the rock was the largest. However, when the thickness was moderate (e.g., δ = 0.5ηλB), the peaks of maximum tensile stress of rock, shotcrete, and joint were nearly equal. We noted that when the thickness was large enough (e.g., δ = 0.8ηλB), the tensile stress of the joint exhibited two stages: in the first stage, the peak of this maximum tensile stress was small; while in the second stage, the peak of the joint was equal to that of rock and shotcrete.
5.2. Influence of the Thickness of Shotcrete on Spalling Characteristics
6. Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
The Detailed Propagation of Stress Wave in Rock–Shotcrete Combination
| Time | Sketch | Stress Expression | Parameters |
|---|---|---|---|
| N = 0 | 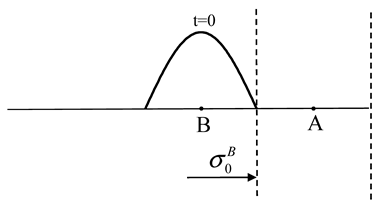 | ||
| N = 1 | 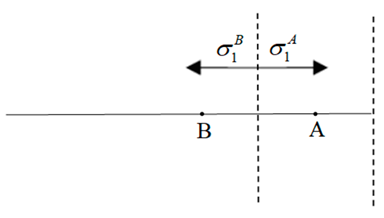 | ||
| N = 2 |  | ||
| N = 3 | 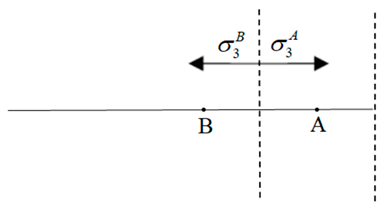 | ||
| N = 4 | 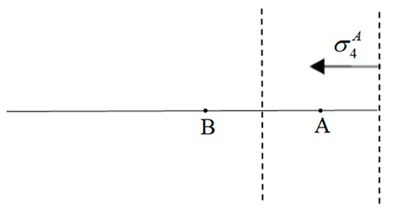 | ||
| N = 5 | 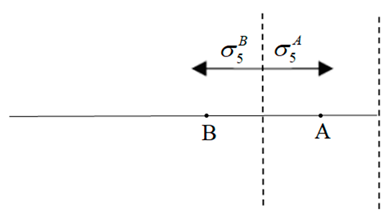 | ||
| N = 6 |  | ||
| N = 7 |  | ||
| … | … | … | … |
References
- Min, G.; Fukuda, D.; Oh, S.; Cho, S. Investigation of the dynamic tensile fracture process of rocks associated with spalling using 3-D FDEM. Comput. Geotech. 2023, 164, 105825. [Google Scholar] [CrossRef]
- Wang, S.M.; Wang, J.Q.; Xong, X.R.; Chen, Z.H.; Gui, Y.L.; Zhou, J. Effect of oblique incident wave perturbation on rock spalling: An insight from DEM modelling. J. Cent. South Univ. 2023, 30, 1981–1992. [Google Scholar] [CrossRef]
- Chen, B.P.; Li, Y.C.; Tang, C.A. Numerical Study on the Zonal Disintegration of a Deeply Buried High-Temperature Roadway during Cooling. Comput. Geotech. 2024, 165, 105848. [Google Scholar] [CrossRef]
- Qiu, J.D.; Xie, H.P.; Zhu, J.B.; Wang, J.; Deng, J.H. Dynamic response and rockburst characteristics of underground cavern with unexposed joint. Int. J. Rock Mech. Min. Sci. 2023, 169, 105442. [Google Scholar] [CrossRef]
- Xiao, P.; Liu, H.X.; Zhao, G.Y. Characteristics of ground pressure disaster and rockburst proneness in deep gold mine. Lithosphere 2023, 2022, 9329667. [Google Scholar] [CrossRef]
- Zhao, R.; Tao, M.; Wu, C.Q.; Wang, S.F.; Zhu, J.B. Spallation damage of underground openings caused by excavation disturbance of adjacent tunnels. Tunn. Undergr. Sp. Technol. 2023, 132, 104892. [Google Scholar] [CrossRef]
- Jiang, Q.; Feng, X.T.; Chen, J.; Huang, K.; Jiang, Y.L. Estimating in-situ rock stress from spalling veins: A case study. Eng. Geol. 2013, 152, 38–47. [Google Scholar] [CrossRef]
- Weerheijm, J.; Van Doormaal, J.C.A.M. Tensile failure of concrete at high loading rates: New test data on strength and fracture energy from instrumented spalling tests. Int. J. Impact Eng. 2007, 34, 609–626. [Google Scholar] [CrossRef]
- Li, X.B.; Tao, M.; Gong, F.Q.; Yin, Z.Q.; Du, K. Theoretical and experimental study of hard rock spalling fracture under impact dynamic loading. Chin. J. Rock Mech. Eng. 2011, 30, 1081–1088. [Google Scholar]
- Zhu, J.B.; Li, Y.S.; Peng, Q.; Deng, X.F.; Gao, M.Z.; Zhang, J.G. Stress wave propagation across jointed rock mass under dynamic extension and its effect on dynamic response and supporting of underground opening. Tunn. Undergr. Sp. Technol. 2021, 108, 103648. [Google Scholar] [CrossRef]
- Qiu, J.D.; Li, D.Y.; Li, X.B.; Zhu, Q.Q. Numerical investigation on the stress evolution and failure behavior for deep roadway under blasting disturbance. Soil Dyn. Earthquake Eng. 2020, 137, 106278. [Google Scholar] [CrossRef]
- Li, X.B.; Tao, M.; Wu, C.Q.; Du, K.; Wu, Q.H. Spalling strength of rock under different static pre-confining pressures. Int. J. Impact Eng. 2017, 99, 69–74. [Google Scholar] [CrossRef]
- Xie, H.P.; Zhu, J.; Zhou, T.; Zhang, K.; Zhou, C.T. Conceptualization and preliminary study of engineering disturbed rock dynamics. Geomech. Geophys. Geo-Energy Geo-Resour. 2020, 6, 1–14. [Google Scholar] [CrossRef]
- Si, X.F.; Luo, Y.; Luo, S. Influence of lithology and bedding orientation on failure behavior of “D” shaped tunnel. Theor. Appl. Fract. Mech. 2024, 129, 104219. [Google Scholar] [CrossRef]
- Li, C.J.; Li, X.B. Influence of wavelength-to-tunnel-diameter ratio on dynamic response of underground tunnels subjected to blasting loads. Int. J. Rock Mech. Min. Sci. 2018, 112, 323–338. [Google Scholar] [CrossRef]
- Xie, H.P.; Zhu, J.B.; Zhou, T.; Zhao, J. Novel three-dimensional rock dynamic tests using the true triaxial electromagnetic Hopkinson bar system. Rock Mech. Rock Eng. 2021, 54, 2079–2086. [Google Scholar] [CrossRef]
- Qiu, J.D.; Li, D.Y.; Li, X.B. Dynamic failure of a phyllite with a low degree of metamorphism under impact Brazilian test. Int. J. Rock Mech. Min. Sci. 2017, 94, 10–17. [Google Scholar] [CrossRef]
- Fan, L.F.; Yang, Q.H.; Du, X.L. Spalling characteristics of high-temperature treated granitic rock at different strain rates. J. Rock Mech. Geotech. 2023, 16, 1280–1288. [Google Scholar] [CrossRef]
- Martin, C.D.; Christiansson, R. Estimating the potential for spalling around a deep nuclear waste repository in crystalline rock. Int. J. Rock Mech. Min. Sci. 2009, 46, 219–228. [Google Scholar] [CrossRef]
- Kubota, S.; Ogata, Y.; Wada, Y.; Simangunsong, G.; Shimada, H.; Matsui, K. Estimation of dynamic tensile strength of sandstone. Int. J. Rock Mech. Min. Sci. 2008, 45, 397–406. [Google Scholar] [CrossRef]
- Zhao, H.T.; Tao, M.; Li, X.B.; Cao, W.Z.; Wu, C.Q. Estimation of spalling strength of sandstone under different pre-confining pressure by experiment and numerical simulation. Int. J. Impact Eng. 2019, 133, 103359. [Google Scholar] [CrossRef]
- Cho, S.H.; Ogata, Y.; Kaneko, K. Strain-rate dependency of the dynamic tensile strength of rock. Int. J. Rock Mech. Min. Sci. 2003, 40, 763–777. [Google Scholar] [CrossRef]
- Salem, S.; Eissa, E.; Zarif, E.; Sherif, S.; Shazly, M. Influence of moisture content on the concrete response under dynamic loading. Ain Shams Eng. J. 2023, 14, 101976. [Google Scholar] [CrossRef]
- Peng, S.; Wu, B.; Du, X.Q.; Zhao, Y.F.; Yu, Z.P. Study on dynamic splitting tensile mechanical properties and microscopic mechanism analysis of steel fiber reinforced concrete. Structures 2023, 58, 105502. [Google Scholar] [CrossRef]
- Erzar, B.; Forquin, P. An experimental method to determine the tensile strength of concrete at high rates of strain. Exp. Mech. 2010, 50, 941–955. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Ren, H.Q.; Han, F.; Sun, G.J.; Wang, X.; Zhao, Q.; Zhang, L. Spall strength of steel-fiber-reinforced concrete under one-dimensional stress state. Mech. Mater. 2020, 141, 103273. [Google Scholar] [CrossRef]
- Ahmed, L.; Ansell, A. Laboratory investigation of stress waves in young shotcrete on rock. Mag. Concr. Res. 2012, 64, 899–908. [Google Scholar] [CrossRef]
- Ahmed, L. Models for Analysis of Shotcrete on Rock Exposed to Blasting. Ph.D. Thesis, KTH Royal Institute of Technology, Stockholm, Sweden, 2012. [Google Scholar]
- Luo, L.; Li, X.B.; Tao, M.; Dong, L.J. Mechanical behavior of rock-shotcrete interface under static and dynamic tensile loads. Tunn. Undergr. Sp. Technol. 2017, 65, 215–224. [Google Scholar] [CrossRef]
- Shen, Y.J.; Wang, Y.Z.; Yang, Y.; Sun, Q.; Luo, T.; Zhang, H. Influence of surface roughness and hydrophilicity on bonding strength of concrete-rock interface. Constr. Build Mater. 2019, 213, 156–166. [Google Scholar] [CrossRef]
- Zhou, Z.L.; Lu, J.Y.; Cai, X. Static and dynamic tensile behavior of rock-concrete bi-material disc with different interface inclinations. Constr. Build. Mater. 2020, 256, 119424. [Google Scholar] [CrossRef]
- Qiu, J.D.; Luo, L.; Li, X.B.; Li, D.Y.; Chen, Y.; Luo, Y. Numerical investigation on the tensile fracturing behavior of rock-shotcrete interface based on discrete element method. Int. J. Min. Sci. Technol. 2020, 30, 293–301. [Google Scholar] [CrossRef]
- Li, X.B. Rock Dynamics Fundamentals and Applications; Science Press: Changsha, China, 2014. [Google Scholar]
- Wang, L.L. Foundations of Stress Waves; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Qiu, J.D.; Zhou, C.T.; Wang, Z.H.; Feng, F. Dynamic responses and failure behavior of jointed rock masses considering pre-existing joints using a hybrid BPM-DFN approach. Comput. Geotech. 2023, 155, 105237. [Google Scholar] [CrossRef]
- Goodman, R.E. Methods of Geological Engineering in Discontinuous Rocks; West Publishing Company: St. Paul, MN, USA, 1976. [Google Scholar]
- Kulhawy, F.H. Stress-deformation properties of rock and rock discontinuities. Eng. Geol. 1975, 9, 327–350. [Google Scholar] [CrossRef]
- Gao, K.; Rougier, E.; Guyer, R.A. Simulation of crack induced nonlinear elasticity using the combined finitediscrete element method. Ultrasonics 2019, 98, 51–61. [Google Scholar] [CrossRef]
- Liu, Y.G.; Xu, S.L.; Xi, D.Y. Dispersion effect of elastic wave in jointed basalts. Chin. J. Rock Mech. Eng. 2010, 29, 3314–3320. [Google Scholar]
- Langford, J.C.; Diederichs, M.S. Quantifying uncertainty in Hoek–Brown intact strength envelopes. Int. J. Rock Mech. Min. Sci. 2015, 74, 91–102. [Google Scholar] [CrossRef]
- Long, X.T.; Wang, G.L.; Lin, W.J.; Wang, J.; He, Z.Q.; Ma, J.C.; Yin, X.F. Locating geothermal resources using seismic exploration in Xian County, China. Geothermics 2023, 112, 102747. [Google Scholar] [CrossRef]
- Ma, Y.C.; Chen, J.X.; Qiu, H.; Zhuang, J.P.; Zhou, L.; Wang, M. Investigation of stress-wave-induced crack penetration behavior at the rock-Ultra-High-Performance Concrete (UHPC) interface. Theor. Appl. Fract. Mech. 2024, 104399. [Google Scholar] [CrossRef]
- Mitelman, A.; Elmo, D. Analysis of tunnel support design to withstand spalling induced by blasting. Tunn. Undergr. Sp. Technol. 2016, 51, 354–361. [Google Scholar] [CrossRef]
- Malmgren, L.; Nordlund, E.; Rolund, S. Adhesion strength and shrinkage of shotcrete. Tunn. Undergr. Sp. Technol. 2005, 20, 33–48. [Google Scholar] [CrossRef]
- Malmgren, L.; Nordlund, E. Interaction of shotcrete with rock and rock bolts—A numerical study. Int. J. Rock Mech. Min. Sci. 2008, 45, 538–553. [Google Scholar] [CrossRef]
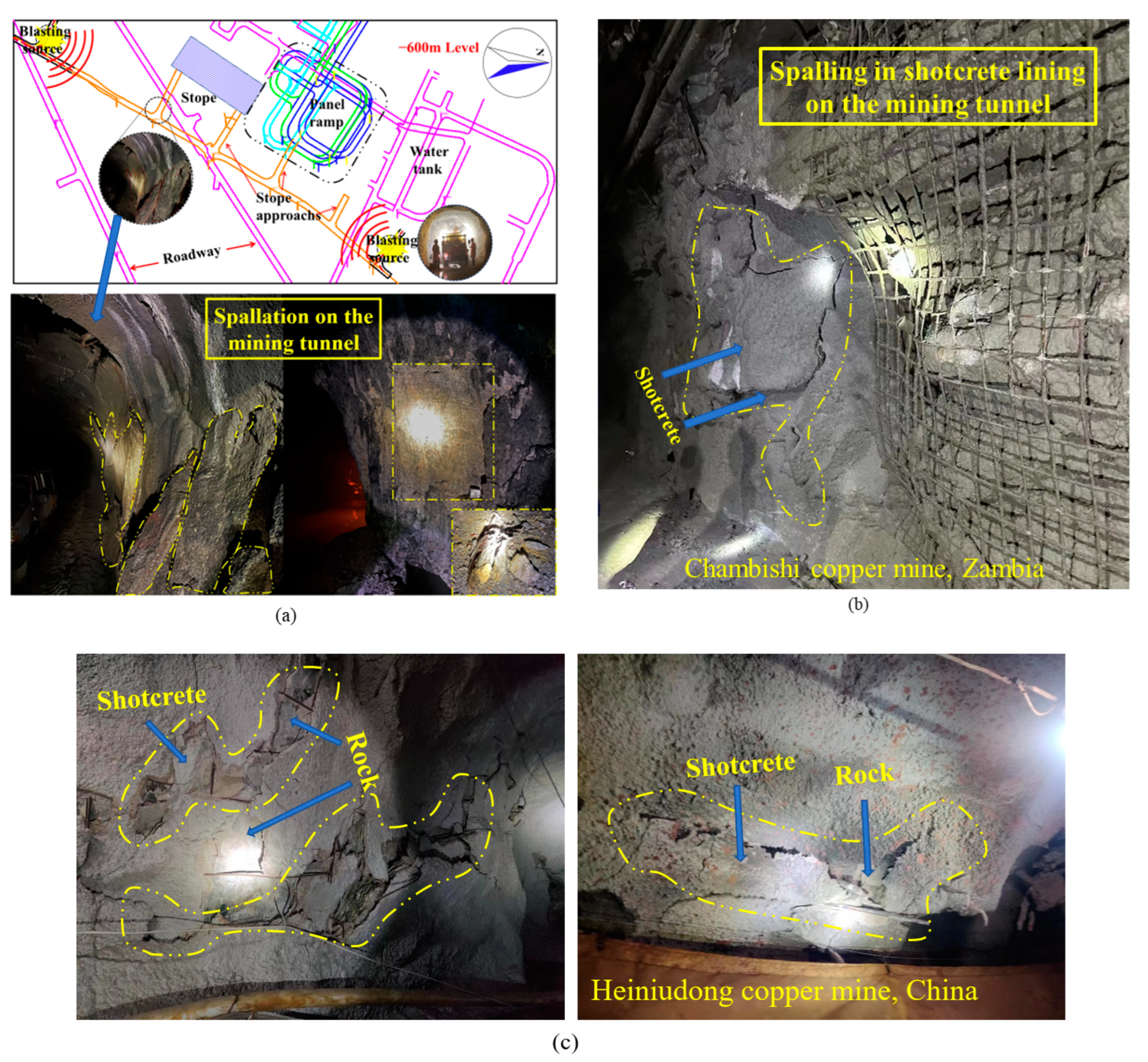





| Year | Researchers | Works |
|---|---|---|
| 2012 | Ahmed and Ansell [27] | conducted a laboratory experiment and verified the previous recommendations |
| 2012 | Ahmed [28] | conducted numerical simulation and recommended maximum allowed peak particle vibration velocities |
| 2017 | Luo et al. [29] | investigated the effect of rock–shotcrete interface geometry on the dynamic tensile behavior |
| 2019 | Shen et al. [30] | investigated the influence of the hydrophilicity and roughness of the rock interface on interfacial bond strength |
| 2020 | Zhou et al. [31] | conducted splitting tests to investigate the effect of interface inclination on the mechanical properties of rock–concrete bi-material discs |
| 2020 | Qiu et al. [32] | investigated the effects of elastic modulus and tensile strength of shotcrete on the dynamic tensile behavior of rock–shotcrete interfaces |
| 2021 | Zhu et al. [10] | conducted numerical simulation to study dynamic response of underground openings with shotcrete |
| Group | Component | Density ρ, kg·m−3 | Tensile Strength σt, MPa | UCS, MPa | Young’s Modulus E, GPa | Poisson Ratio υ | P-Wave Velocity λ |
|---|---|---|---|---|---|---|---|
| Group 1 | Rock | 2628 | 14.13 | 146.6 | 31.6 | 0.15 | 3700 |
| Shotcrete | 1866 | 11.84 | 57.4 | 10.5 | 0.23 | 2510 | |
| Joint 1 | - | 6.95 | - | - | - | ||
| Group 2 | Rock | 2628 | 14.13 | 146.6 | 31.6 | 0.15 | 3700 |
| Shotcrete | 1866 | 11.84 | 57.4 | 10.5 | 0.23 | 2510 | |
| Joint 2 | - | 9.80 | - | - | - |
| Parameters | Analog Component | |||
|---|---|---|---|---|
| Rock | Shotcrete | Joint 1 | Joint 2 | |
| Particle density (kg/m3) | 2628 | 1866 | - | - |
| Particle radius (mm) | 0.25–0.5 | 0.25–0.5 | - | - |
| Damping | 0.0 | 0.0 | - | - |
| Friction angle | 45° | 45° | - | - |
| Linear contact modulus Ec (GPa) | 19 | 7 | - | - |
| Linear contact stiffness ratio (kn/ks) | 1.08 | 1.65 | - | - |
| Parallel bond modulus (GPa) | 19 | 7 | - | - |
| Parallel bond stiffness ratio () | 1.08 | 1.65 | - | - |
| Joint normal stiffness () | - | - | 1 × 1014 | 1 × 1014 |
| Joint shear stiffness () | - | - | 1 × 1014 | 1 × 1014 |
| Joint friction angle | - | - | 0° | 0° |
| Tensile strength (MPa) | 93 9 | 45 4 | 5 | 25 |
| Cohesion (MPa) | 93 9 | 45 4 | 5 | 25 |
| Case | Model | Material | Incident Amplitude σm, MPa | Spalling Time, τ | Spalling Location, x | ||
|---|---|---|---|---|---|---|---|
| Theoretical | Numerical | Theoretical | Numerical | ||||
| Case 1 | A | Group 1 | 15 | 1.24 | 1.19–1.23 | 0.339 | 0.339 |
| Case 2 | A | Group 2 | 30 | 1.15 | 1.29–1.40 | 0.339 | 0.339, 0.22 |
| Case 3 | A | Group 2 | 60 | 0.74 | 0.69–0.81 | 0.605 | 0.69 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, L.; Rui, Y.; Qiu, J.; Li, C.; Liu, X.; Chen, C. Exploring Dynamic Spalling Behavior in Rock–Shotcrete Combinations: A Theoretical and Numerical Investigation. Mathematics 2024, 12, 1346. https://doi.org/10.3390/math12091346
Luo L, Rui Y, Qiu J, Li C, Liu X, Chen C. Exploring Dynamic Spalling Behavior in Rock–Shotcrete Combinations: A Theoretical and Numerical Investigation. Mathematics. 2024; 12(9):1346. https://doi.org/10.3390/math12091346
Chicago/Turabian StyleLuo, Lin, Yichao Rui, Jiadong Qiu, Chongjin Li, Xiong Liu, and Cong Chen. 2024. "Exploring Dynamic Spalling Behavior in Rock–Shotcrete Combinations: A Theoretical and Numerical Investigation" Mathematics 12, no. 9: 1346. https://doi.org/10.3390/math12091346
APA StyleLuo, L., Rui, Y., Qiu, J., Li, C., Liu, X., & Chen, C. (2024). Exploring Dynamic Spalling Behavior in Rock–Shotcrete Combinations: A Theoretical and Numerical Investigation. Mathematics, 12(9), 1346. https://doi.org/10.3390/math12091346








