Efficient Methods for the Chebyshev-Type Prolate Spheroidal Wave Functions and Corresponding Eigenvalues
Abstract
1. Introduction
- The CPSWFs are real and smooth on . They constitute a complete orthonormal system in withSince the normalized Chebyshev polynomials also constitute an orthonormal basis in , can be expanded by
- The eigenvalues of the Sturm–Liouville problem CPSWFs are distinct, real and positive, and can be ordered asFor any and , the following uniform bounds for were established by Wang and Zhang ([25], Lem. 3.1)
- is an even function when n is even, and an odd function when n is odd. There holds the parity
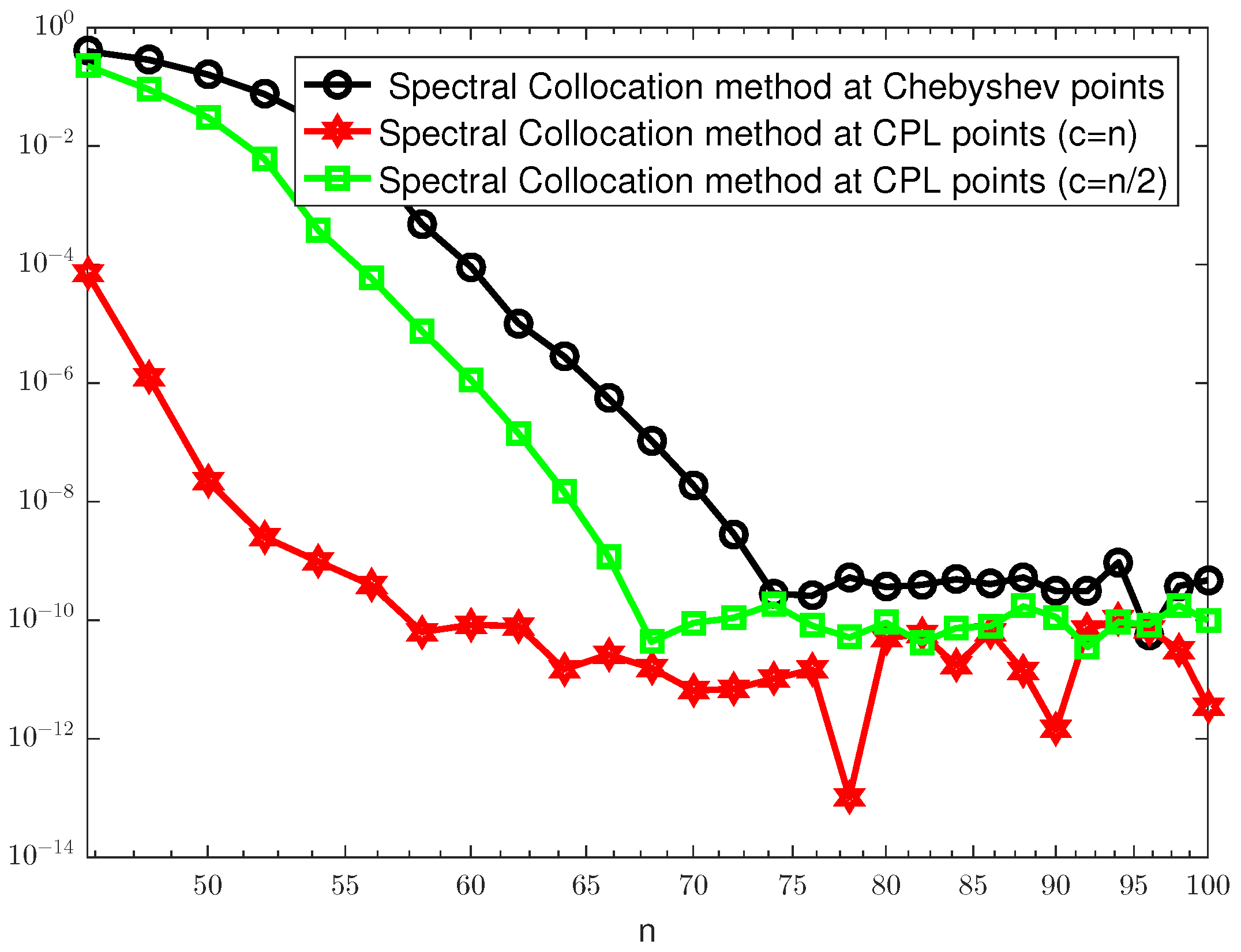
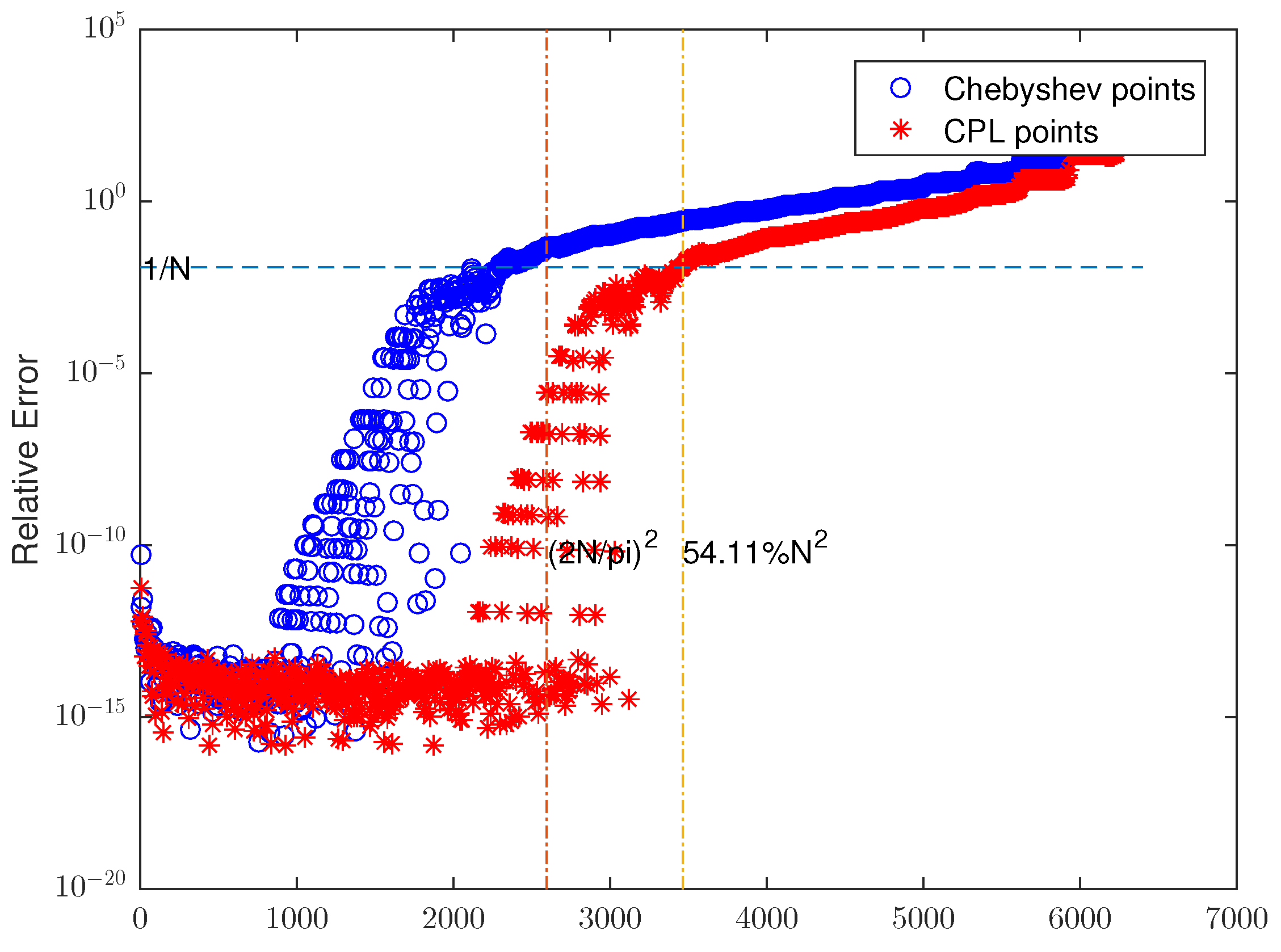
- has exactly n real distinct zeros in the interval . When , has real zeros in that interlace with the n zeros of . We define the roots of as the Chebyshev–Prolate–Lobatto points (CPL points). In computation, we use Newton’s iteration method with zeros of Chebyshev polynomial as the initial points [16].
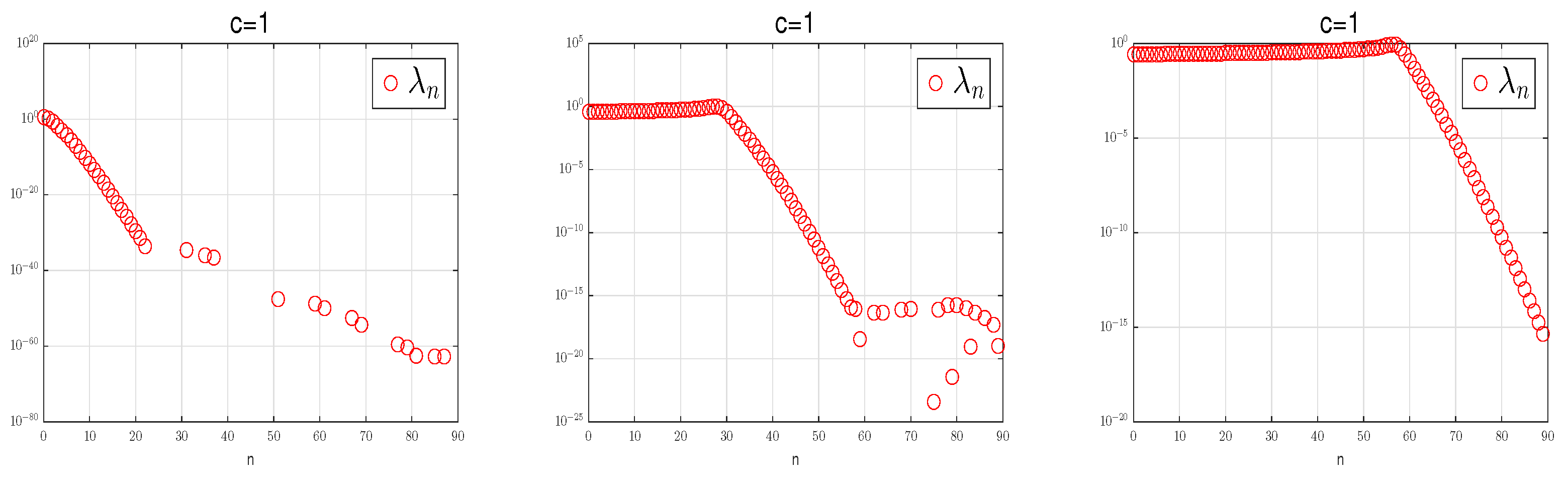
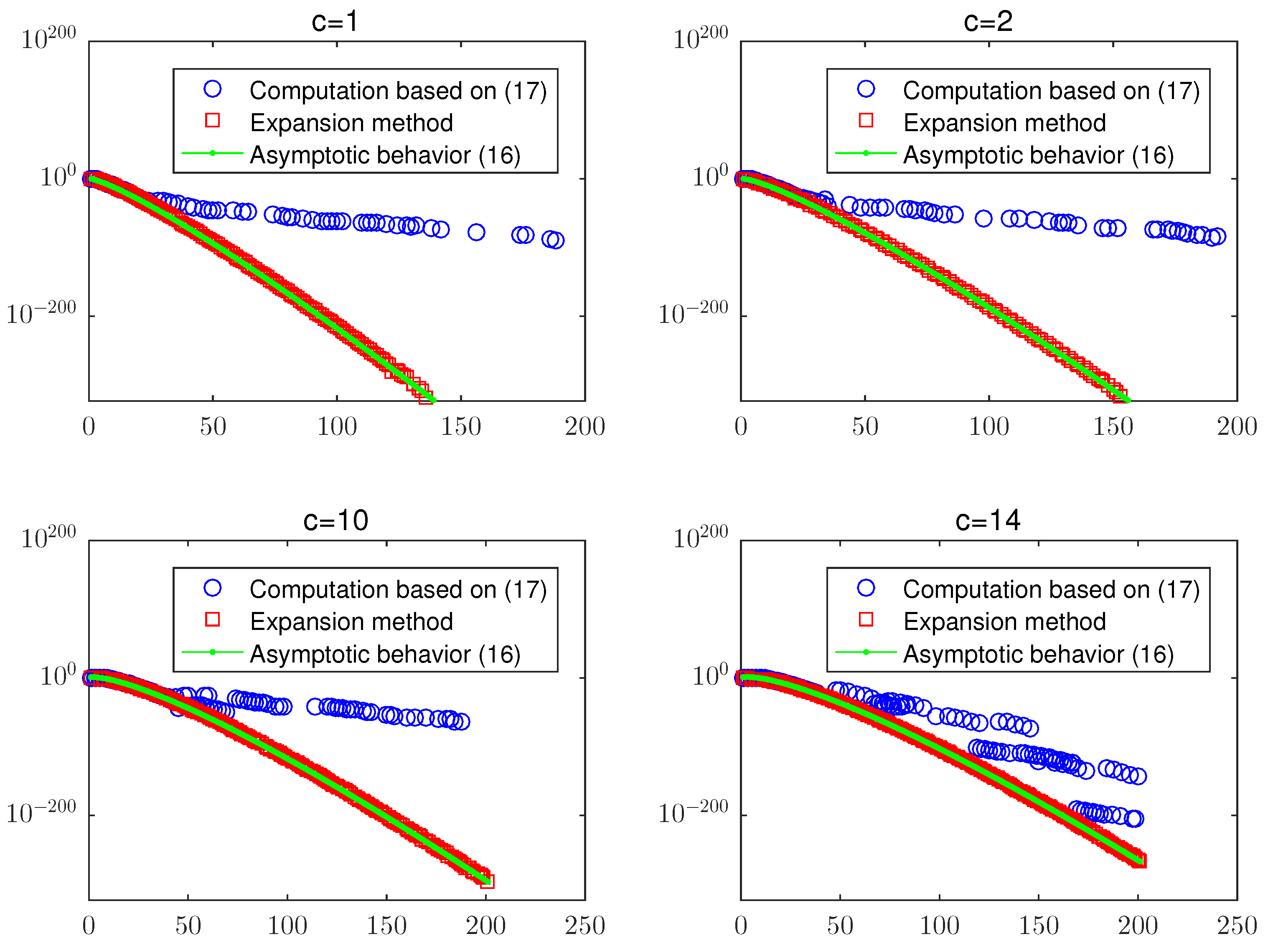
2. The Eigenvalues of the Integral Operator
3. Evaluation of CPSWF and Related Quantities
4. Numerical Results
| n | CPU Time (s) | Absolute Errors | |
|---|---|---|---|
| Fast Interpolation Method | Direct Method | ||
| 200 | 0.002 | 0.015 | |
| 400 | 0.004 | 0.036 | |
| 800 | 0.008 | 0.181 | |
| 1600 | 0.030 | 1.310 | |
| n | CPU Time (s) | Absolute Errors | |
|---|---|---|---|
| Fast Interpolation Method | Direct Method | ||
| 200 | 0.02 | 0.02 | |
| 400 | 0.03 | 0.06 | |
| 800 | 0.11 | 0.20 | |
| 1600 | 0.44 | 1.26 | |
| n | CPU Time (s) | Absolute Errors | |
|---|---|---|---|
| Fast Interpolation Method | Direct Method | ||
| 160 | 0.007 | 0.008 | |
| 320 | 0.017 | 0.021 | |
| 640 | 0.043 | 0.110 | |
| 1280 | 0.159 | 0.740 | |
| 2560 | 0.651 | 4.913 | |
| 5120 | 2.719 | 53.400 | |
5. Applications
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Slepian, D.; Pollak, H. Prolate spheroidal wave functions, Fourier analysis and uncertainty—I. Bell Syst. Tech. J. 1961, 40, 43–63. [Google Scholar] [CrossRef]
- Slepian, D. Prolate spheroidal wave functions, Fourier analysis and uncertainty—IV. Extensions to many dimensions generalized prolate spheroidal functions. Bell Syst. Tech. J. 1964, 43, 3009–3057. [Google Scholar] [CrossRef]
- Wang, L. A review of prolate spheroidal wave functions from the perspective of spectral methods. J. Math. Study 2017, 50, 101–143. [Google Scholar] [CrossRef]
- Wang, L. Analysis of spectral approximations using prolate spheroidal wave functions. Math. Comp. 2009, 79, 807–827. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, J. An improved estimate of PSWF approximation and approximation by Mathieu functions. J. Math. Anal. Appl. 2011, 379, 35–47. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, J.; Zhang, Z. On hp-convergence of prolate spheroidal wave functions and a new well-conditioned prolate-collocation scheme. J. Comput. Phys. 2014, 268, 377–398. [Google Scholar] [CrossRef]
- Xiao, H.; Rokhlin, V.; Yarvin, N. Prolate spheroidal wave functions, quadrature and interpolation. Inverse Probl. 2011, 17, 805–838. [Google Scholar] [CrossRef]
- Beylkin, G.; Sandberg, K. Wave propagation using bases for bandlimited functions. Wave Motion 2005, 41, 263–291. [Google Scholar] [CrossRef]
- Boyd, J. Prolate spheroidal wavefunctions as an alternative to chebyshev and Legendre polynomials for spectral element and pseudospectral algorithms. J. Comput. Phys. 2004, 199, 688–716. [Google Scholar] [CrossRef]
- Chen, Q.; Gottlieb, D.; Hesthaven, J. Spectral methods based on prolate spheroidal wave functions for hyperbolic PDEs. SIAM J. Numer. Anal. 2005, 43, 1912–1933. [Google Scholar] [CrossRef]
- Lin, W. Theory and Applications of Biorthorgonal Ridgelets and Prolate Spheroidal Wave Functions. Ph.D. Thesis, Duke University, Durham, NC, USA, 2005. [Google Scholar]
- Lin, W.; Kovvali, N.; Carin, L. Pseudospectral method based on prolate spheroidal wave functions for semiconductor nanodevice simulation. Comput. Phys. Commun. 2006, 175, 78–85. [Google Scholar] [CrossRef]
- Slepian, D. Some asymptotic expansions for prolate spheroidal wave functions. J. Math. Phys. 1965, 44, 99–140. [Google Scholar] [CrossRef]
- Rokhlin, V.; Xiao, H. Approxiamte formulae for certain prolate spheroidal wave functions valid for large values of both order and band-limit. Appl. Comput. Harmon. Anal. 2007, 22, 105–123. [Google Scholar] [CrossRef]
- Osipov, A.; Rokhlin, V.; Xiao, H. Prolate Spheroidal Wave Functions of Order Zero; Springer: Berlin, Germany, 2013. [Google Scholar]
- Boyd, J. Algorithm 840: Computation of grid points, quadrature weights and derivatives for spectral element methods using prolate spheroidal wave functions–prolate elements. ACM Trans. Math. Softw. 2005, 31, 149–165. [Google Scholar] [CrossRef]
- Karoui, A.; Mehrzi, I. Asymptotic behaviors and numerical computations of the eigenfunctions and eigenvalues associated with the classical and circular prolate spheroidal wave functions. Appl. Math. Comput. 2012, 218, 10871–10888. [Google Scholar] [CrossRef]
- Karoui, A.; Moumni, T. New efficient methods of computing the prolate spheroidal wave functions and their corresponding eigenvalues. Appl. Comput. Harmon. Anal. 2008, 24, 269–289. [Google Scholar] [CrossRef]
- Karoui, A.; Moumni, T. Spectral analysis of the finite Hankel transform and circular prolate spheroidal wave functions. J. Comput. Appl. Math. 2009, 233, 315–333. [Google Scholar] [CrossRef]
- Schmutzhard, S.; Hrycak, T.; Feichtinger, H. A numerical study of the Legendre-Galerkin method for the evaluation of the prolate spheroidal wave functions. Numer. Algorithms 2015, 68, 691–710. [Google Scholar] [CrossRef]
- Beylkin, G.; Kurcz, C.; Monzón, L. Grids and transforms for band-limited functions in a disk. Inverse Probl. 2007, 23, 2059–2088. [Google Scholar] [CrossRef]
- Bates, A.; Khalid, Z.; Kennedy, R. Efficient computation of slepian functions on the sphere. IEEE Trans. Signal Process. 2016, 65, 4379–4393. [Google Scholar] [CrossRef]
- Miranian, A. Slepian functions on the sphere, generalized Gaussian quadrature rule. Inverse Probl. 2004, 20, 877–892. [Google Scholar] [CrossRef]
- Simons, F.; Dahlen, F.; Wieczorek, M. Spatiospectral concentration on a sphere. SIAM Rev. 2006, 48, 504–536. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, J. A new generalization of the PSWFs with applications to spectral approximations on quasi-uniform grids. Appl. Comput. Harmon. Anal. 2010, 29, 303–329. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, L.; Li, H.; Zhang, Z. Optimal spectral schemes based on generalized prolate spheroidal wave functions of order -1. J. Sci. Comput. 2017, 70, 451–477. [Google Scholar] [CrossRef]
- Shen, J.; Tang, T.; Wang, L. Spectral Methods: Algorithms, Analysis and Applications; Springer: Berlin, Germany, 2011. [Google Scholar]
- Bouwkamp, C. On the theory of spheroidal wave functions of order zero. Nederl. Akad. Wetensch. Proc. 1950, 53, 931–944. [Google Scholar]
- Piessens, R.; Poleunis, F. A numerical method for the integration of oscillatory functions. BIT 1971, 11, 317–327. [Google Scholar] [CrossRef]
- Gradshteyn, I.; Ryzhik, I. Tables of Integrals, Series, and Products, 6th ed.; Academic Press: Cambridge, MA, USA, 2007. [Google Scholar]
- Abramowitz, M.; Stegun, I. Handbook of Mathematical Functions; National Bureau of Standards: Washington, DC, USA, 1964.
- Mason, J.; Handscomb, D. Chebyshev Polynomials; Chapman and Hall/CRC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Cooley, J.; Tukey, J. An algorithm for the machine calculation of complex Fourier series. Math. Comput. 1965, 19, 297–301. [Google Scholar] [CrossRef]
- Berrut, J.; Trefethen, L. Barycentric Lagrange interpolation. SIAM Rev. 2004, 46, 501–517. [Google Scholar] [CrossRef]
- Trefethen, L. Approximation Theory and Approximation Practice; SIAM: Philadelphia, PA, USA, 2013. [Google Scholar]
- Wang, H.; Huybrechs, D.; Vandewalle, S. Explicit barycentric weights for polynomial interpolation in the roots or extrema of classical orthogonal polynomials. Math. Comput. 2014, 83, 2893–2914. [Google Scholar] [CrossRef]
- Wang, H.; Xiang, S. On the convergence rates of Legendre approximation. Math. Comput. 2012, 81, 861–877. [Google Scholar] [CrossRef]
- Tian, Y. Barycentric prolate interpolation and pseudospectral differentiation. Numer. Algorithms 2021, 88, 793–811. [Google Scholar] [CrossRef]
- Liu, G.; Xiang, S. Fast multipole methods for approximating a function from sampling values. Numer. Algorithms 2007, 76, 727–743. [Google Scholar] [CrossRef]
- Tian, Y.; Xiang, S.; Liu, G. Fast computation of the spectral differentiation by the fast multipole method. Comput. Math. Appl. 2019, 78, 240–253. [Google Scholar] [CrossRef]
- Wang, L.; Samson, M.; Zhao, X. A well-conditioned collocation method using a pseudospectral integration matrix. SIAM J. Sci. Comput. 2014, 36, A907–A929. [Google Scholar] [CrossRef]
- Trefethen, L. Spectral Methods in Matlab; SIAM: Philadelphia, PA, USA, 2000. [Google Scholar]
- Zhang, Z. How many numerical eigenvalues can we trust? J. Sci. Comput. 2015, 65, 455–466. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Y.; Liu, G. Efficient Methods for the Chebyshev-Type Prolate Spheroidal Wave Functions and Corresponding Eigenvalues. Mathematics 2024, 12, 807. https://doi.org/10.3390/math12060807
Tian Y, Liu G. Efficient Methods for the Chebyshev-Type Prolate Spheroidal Wave Functions and Corresponding Eigenvalues. Mathematics. 2024; 12(6):807. https://doi.org/10.3390/math12060807
Chicago/Turabian StyleTian, Yan, and Guidong Liu. 2024. "Efficient Methods for the Chebyshev-Type Prolate Spheroidal Wave Functions and Corresponding Eigenvalues" Mathematics 12, no. 6: 807. https://doi.org/10.3390/math12060807
APA StyleTian, Y., & Liu, G. (2024). Efficient Methods for the Chebyshev-Type Prolate Spheroidal Wave Functions and Corresponding Eigenvalues. Mathematics, 12(6), 807. https://doi.org/10.3390/math12060807






