Eigenvalue Problem Describing Magnetorotational Instability in Outer Regions of Galaxies
Abstract
1. Introduction
2. Basic Equations
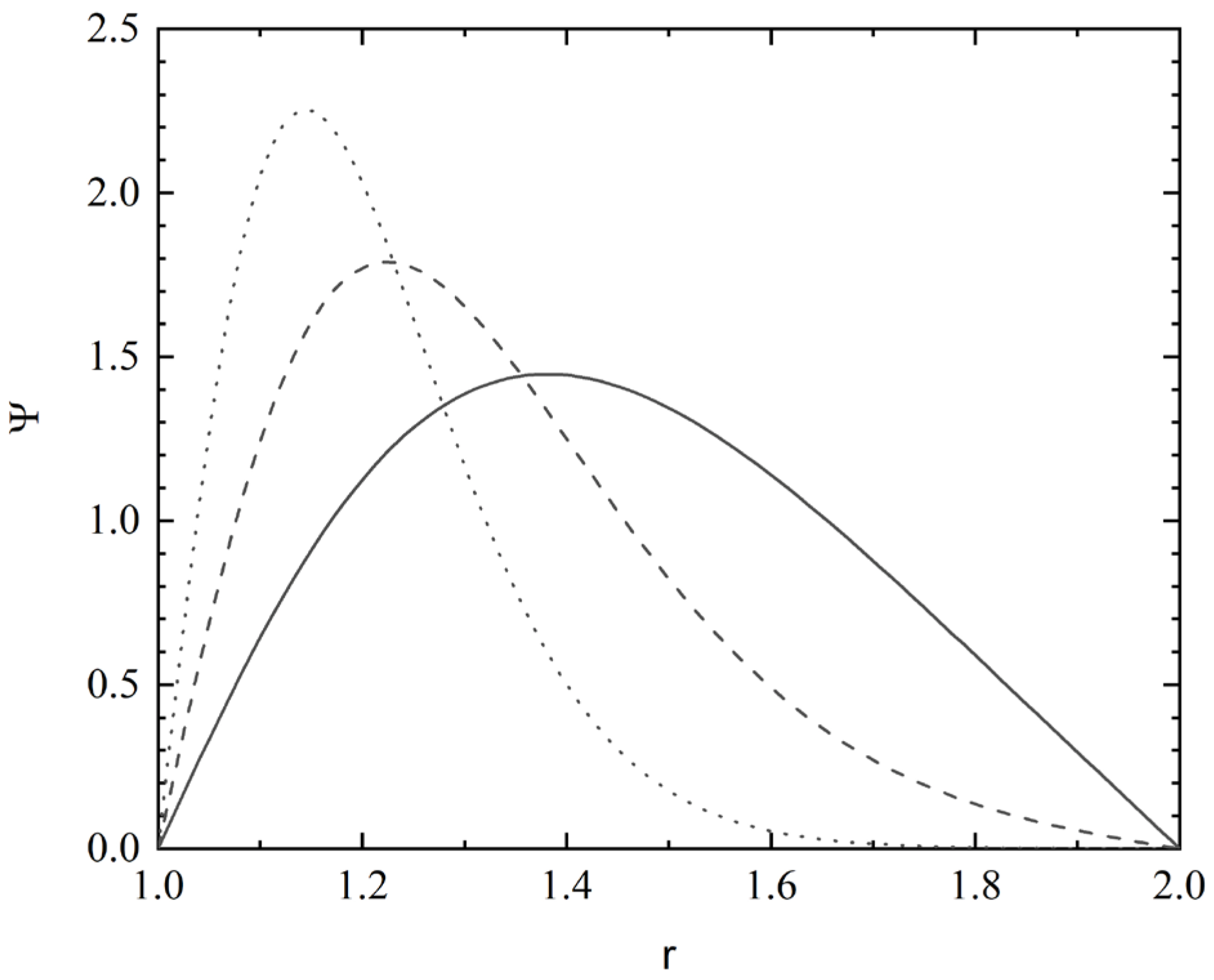
3. Eigenvalue Problem for Flat Rotation Curve
4. Eigenvalue Problem for the Brandt Rotation Curve
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Obridko, V.N.; Pipin, V.V.; Sokoloff, D.; Shibalova, A.S. Solar large-scale magnetic field and cycle patterns in solar dynamo. Mon. Not. R. Astron. Soc. 2021, 504, 4990–5000. [Google Scholar] [CrossRef]
- Parker, E.N. Cosmical magnetic fields. Their origin and their activity. In The International Series of Monographs on Physics; Clarendon Press: Oxford, UK, 1979. [Google Scholar]
- Glatzmaier, G.A.; Roberts, H. Rotation and Magnetism of Earth’s Inner Core. Science 1996, 274, 1887–1891. [Google Scholar] [CrossRef]
- Christensen, U.R. A deep dynamo generating Mercury’s magnetic field. Nature 2006, 444, 1056–1058. [Google Scholar] [CrossRef]
- Smith, E.J.; Davis, L., Jr.; Jones, D.E.; Coleman, J., Jr.; Colburn, D.S.; Dyal, P.; Sonett, C.; Frandsen, A.M.A. The planetary magnetic field and magnetosphere of Jupiter: Pioneer 10. J. Geophys. Res. 1974, 79, 3501. [Google Scholar] [CrossRef]
- Borra, E.F.; Landstreet, J.D. The magnetic fields of the AP stars. Astrophys. J. Suppl. Ser. 1980, 42, 421–445. [Google Scholar] [CrossRef]
- Vidotto, A.A.; Gregory, S.G.; Jardine, M.; Donati, J.F.; Petit, P.; Morin, J.; Folsom, C.P.; Bouvier, J.; Cameron, A.C.; Hussain, G.; et al. Stellar magnetism: Empirical trends with age and rotation. Mon. Not. R. Astron. Soc. 2014, 441, 2361–2374. [Google Scholar] [CrossRef]
- Girart, J.M.; Rao, R.; Marrone, D. Magnetic Fields in the Formation of Sun-Like Stars. Science 2006, 313, 812–814. [Google Scholar] [CrossRef]
- Moss, D.; Sokoloff, D.; Suleimanov, V. Dynamo generated magnetic configurations in accretion discs and the nature of quasi-periodic oscillations in accreting binary systems. Astron. Astrophys. 2016, 588, A18. [Google Scholar] [CrossRef]
- Boneva, D.V.; Mikhailov, E.A.; Pashentseva, M.V.; Sokoloff, D.D. Magnetic fields in the accretion disks for various inner boundary conditions. Astron. Astrophys. 2021, 652, A38. [Google Scholar] [CrossRef]
- Thompson, C.; Duncan, R.C. Neutron Star Dynamos and the Origins of Pulsar Magnetism. Astrophys. J. 1993, 408, 194. [Google Scholar] [CrossRef]
- Moss, D. On the generation of bisymmetric magnetic field structures in spiral galaxies by tidal interactions. Mothly Not. R. Astron. Soc. 1995, 275, 191–194. [Google Scholar] [CrossRef]
- Beck, R.; Brandenburg, A.; Moss, D.; Shukurov, A.; Sokoloff, D. Galactic Magnetism: Recent Developments and Perspectives. Annu. Rev. Astron. Astrophys. 1996, 34, 155. [Google Scholar] [CrossRef]
- Berdyugina, S.V.; Solanki, S.K. The molecular Zeeman effect and diagnostics of solar and stellar magnetic fields. I. Theoretical spectral patterns in the Zeeman regime. Astron. Astrophys. 2002, 385, 701–715. [Google Scholar] [CrossRef]
- Ginzburg, V.L. Radio astronomy and the origin of cosmic rays. Paris Simp. Radio Astron. IAU Simp. 1959, 9, 589. [Google Scholar] [CrossRef][Green Version]
- Han, J.L.; Manchester, R.N.; van Straten, W. Demorest, Pulsar Rotation Measures and Large-scale Magnetic Field Reversals in the Galactic Disk. Astrophys. J. Suppl. Ser. 2018, 234, 16. [Google Scholar] [CrossRef]
- Manchester, R.N. Pulsar Rotation and Dispersion Measures and the Galactic Magnetic Field. Astrophys. J. 1972, 172, 43. [Google Scholar] [CrossRef]
- Andreasyan, R.R.; Mikhailov, E.A.; Andreasyan, H.R. Structure and Features of the Galactic Magnetic-Field Reversals Formation. Astron. Rep. 2020, 97, 261. [Google Scholar] [CrossRef]
- Kravchenko, E.; Giroletti, M.; Hada, K.; Meier, D.L.; Nakamura, M.; Park, J.; Walker, R.C. Linear polarization in the nucleus of M87 at 7 mm and 1.3 cm. Astron. Astrophys. 2002, 637, 9. [Google Scholar] [CrossRef]
- Ro, H.; Kino, M.; Sohn, B.W.; Hada, K.; Park, J.; Nakamura, M.; Cui, Y. Spectral analysis of a parsec-scale jet in M 87: Observational constraint on the magnetic field strengths in the jet. Astron. Astrophys. 2023, 673, 16. [Google Scholar] [CrossRef]
- Dormy, E.; Soward, A.M. Mathematical Aspects of Natural Dynamos; Chapman and Hall/CRC: New York, NY, USA, 2007. [Google Scholar]
- Ruzmaikin, A.A.; Sokoloff, D.D.; Shukurov, A.M. Magnetic Fields of Galaxies; Springer: Berlin/Heidelberg, Germany, 1988; ISBN 90-277-2450-4. [Google Scholar]
- Mikhailov, E.; Pashentseva, M. Eigenvalue Problem for a Reduced Dynamo Model in Thick Astrophysical Discs. Mathematics 2023, 11, 3106. [Google Scholar] [CrossRef]
- Mikhailov, E.A. Symmetry of the magnetic fields in galactic dynamo and the material arms. Magnetohydrodynamics 2020, 56, 403. [Google Scholar]
- Mikhailov, E.; Kasparova, A.; Moss, D.; Beck, R.; Sokoloff, D.; Zasov, A. Magnetic fields near the peripheries of galactic discs. Astron. Astrophys. 2014, 568, A66. [Google Scholar] [CrossRef][Green Version]
- Velikhov, E. Stability of an Ideally Conducting Liquid Flowing between Cylinders Rotating in a Magnetic Field. Sov. J. Exp. Theor. Phys. 1959, 9, 995–998. [Google Scholar]
- Chandrasekhar, S. The Stability of Non-Dissipative Couette Flow in Hydromagnetics. Proc. Natl. Acad. Sci. USA 1960, 46, 253–257. [Google Scholar] [CrossRef] [PubMed]
- Winters, W.F.; Balbus, S.A.; Hawley, J.F. Chaos in turbulence driven by the magnetorotational instability. Mon. Not. R. Astron. Soc. 2003, 340, 519–524. [Google Scholar] [CrossRef]
- Balbus, S.A.; Hawley, J.F. Instability, turbulence, and enhanced transport in accretion disks. Rev. Mod. Phys. 1998, 70, 1–53. [Google Scholar] [CrossRef]
- Korpi, M.J.; Mac Low, M.-M. A Numerical Investigation on the Role of the Magneto-rotational Instability in Galactic Disks. Astron. Nachrichten 2003, 324, 67. [Google Scholar]
- Kitchatinov, L.L.; Rüdiger, G. Seed fields for galactic dynamos by the magnetorotational instability. Astron. Astrophys. 2004, 424, 565–570. [Google Scholar] [CrossRef][Green Version]
- Machida, M.; Nakamura, K.E.; Kudoh, T.; Akahori, T.; Sofue, Y.; Matsumoto, R. Dynamo Activities Driven by Magnetorotational Instability and the Parker Instability in Galactic Gaseous Disks. Astrophys. J. 2013, 764, 9. [Google Scholar] [CrossRef]
- Shakura, N.I.; Postnov, K.A.; Kolesnikov, D.A.; Lipunova, G.V. Magnetorotational instability in Keplerian disks: A nonlocal approach. Phys. Uspekhi 2023, 66, 1262–1276. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 24, 337–355. [Google Scholar]
- Brandt, J.C. On the Distribution of mass in Galaxies. I. The Large-Scale Structure of Ordinary Spirals with Applications to M 31. Astrophys. J. 1960, 131, 293. [Google Scholar] [CrossRef]
- Yoshiaki, S. The Most Completely Sampled Rotation Curves for Galaxies. Astrophys. J. 1996, 458, 120. [Google Scholar]
- De Block, W.J.G.; Walter, F.; Brinks, E.; Trachternach, C.; Oh, S.H.; Kennicutt, R.C., Jr. Hight-resolution Rotation Curves and Galaxy Mass Models from Things. Astron. J. 2008, 136, 2648. [Google Scholar] [CrossRef]
- Roberts, M.S.; Whitehurst, R.N. The rotation curve and geometry of M31 at large galactocentric distances. Astrophys. J. 1975, 201, 327–346. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Theoretical physics. In Quantum Mechanics (Non-Relativistic Theory); Pergamon Press: Oxford, UK, 1977. [Google Scholar]
- Khasaeva, T.T.; Mikhailov, E.A. Mehanismy formirovaniya magnitnyh poley na bolshom rasstoyaniy ot centra galaktiki. Zhurnal Teh. Fiz. 2023, 93, 1771. [Google Scholar]
- Andreasyan, R.R. Mechanism of formation of a dipole magnetic field in the central regions of active galaxies. Astrophysics 1996, 39, 58–62. [Google Scholar] [CrossRef]

| 1.1131 × C | 0.4650 × C | |
| 1.2551 × C | 0.4896 × C | |
| 1.2946 × C | 0.4953 × C |
| 2955.56 | 431.456 |
| C | Numerical | ||
|---|---|---|---|
| 600 | 20.772 | 19.458 | 1.314 |
| 400 | 15.756 | 15.338 | 0.418 |
| 200 | 10.058 | 10.059 | 0.001 |
| 100 | 6.414 | 6.415 | 0.001 |
| 50 | 3.809 | 3.795 | 0.014 |
| 1.2408 × D − 0.8349 | 0.4840 × D − 0.3487 | |
| 1.4417 × D − 0.9414 | 0.5121 × D − 0.3673 | |
| 1.5051 × D − 0.9710 | 0.5187 × D − 0.3714 |
| 123.246 | 48.054 | |
| 98.150 | 5.225 | |
| 211.526 | 43.410 |
| D | Numerical | Analytical | |
|---|---|---|---|
| 600 | 20.145 | 21.691 | 0.546 |
| 400 | 15.863 | 16.367 | 0.504 |
| 200 | 10.379 | 10.375 | 0.004 |
| 100 | 6.595 | 6.589 | 0.006 |
| 50 | 3.895 | 3.907 | 0.012 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mikhailov, E.; Khasaeva, T. Eigenvalue Problem Describing Magnetorotational Instability in Outer Regions of Galaxies. Mathematics 2024, 12, 760. https://doi.org/10.3390/math12050760
Mikhailov E, Khasaeva T. Eigenvalue Problem Describing Magnetorotational Instability in Outer Regions of Galaxies. Mathematics. 2024; 12(5):760. https://doi.org/10.3390/math12050760
Chicago/Turabian StyleMikhailov, Evgeny, and Tatiana Khasaeva. 2024. "Eigenvalue Problem Describing Magnetorotational Instability in Outer Regions of Galaxies" Mathematics 12, no. 5: 760. https://doi.org/10.3390/math12050760
APA StyleMikhailov, E., & Khasaeva, T. (2024). Eigenvalue Problem Describing Magnetorotational Instability in Outer Regions of Galaxies. Mathematics, 12(5), 760. https://doi.org/10.3390/math12050760






