A Model of the Control Problem of the Thermal Effect of a Laser Beam on a Two-Layer Biomaterial
Abstract
1. Introduction
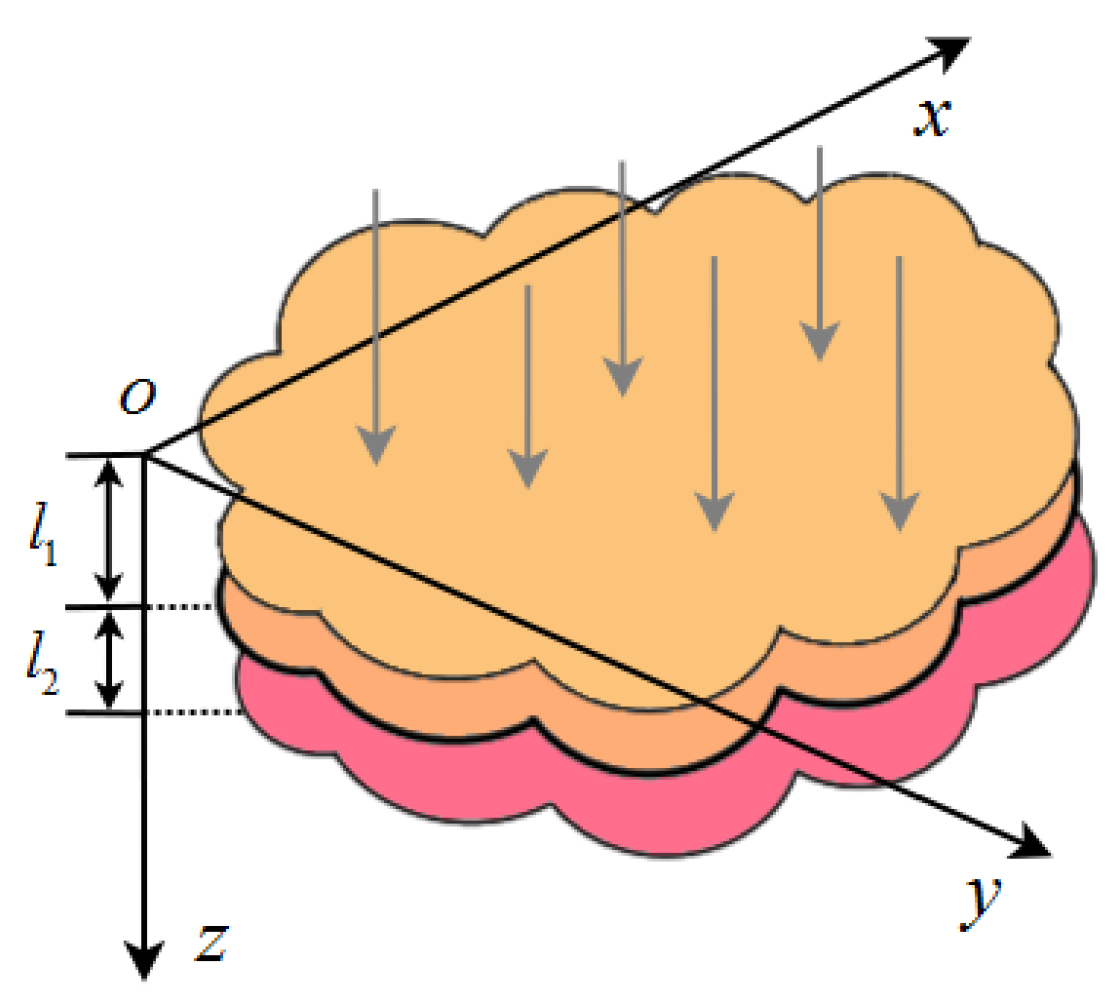
2. Mathematical Model of a Two-Layer Biomaterial and Problem Statement
3. Reduction of the Problem to a Problem with Zero Boundary Conditions
4. Solving the Problem
5. Problem Solving for n = 2
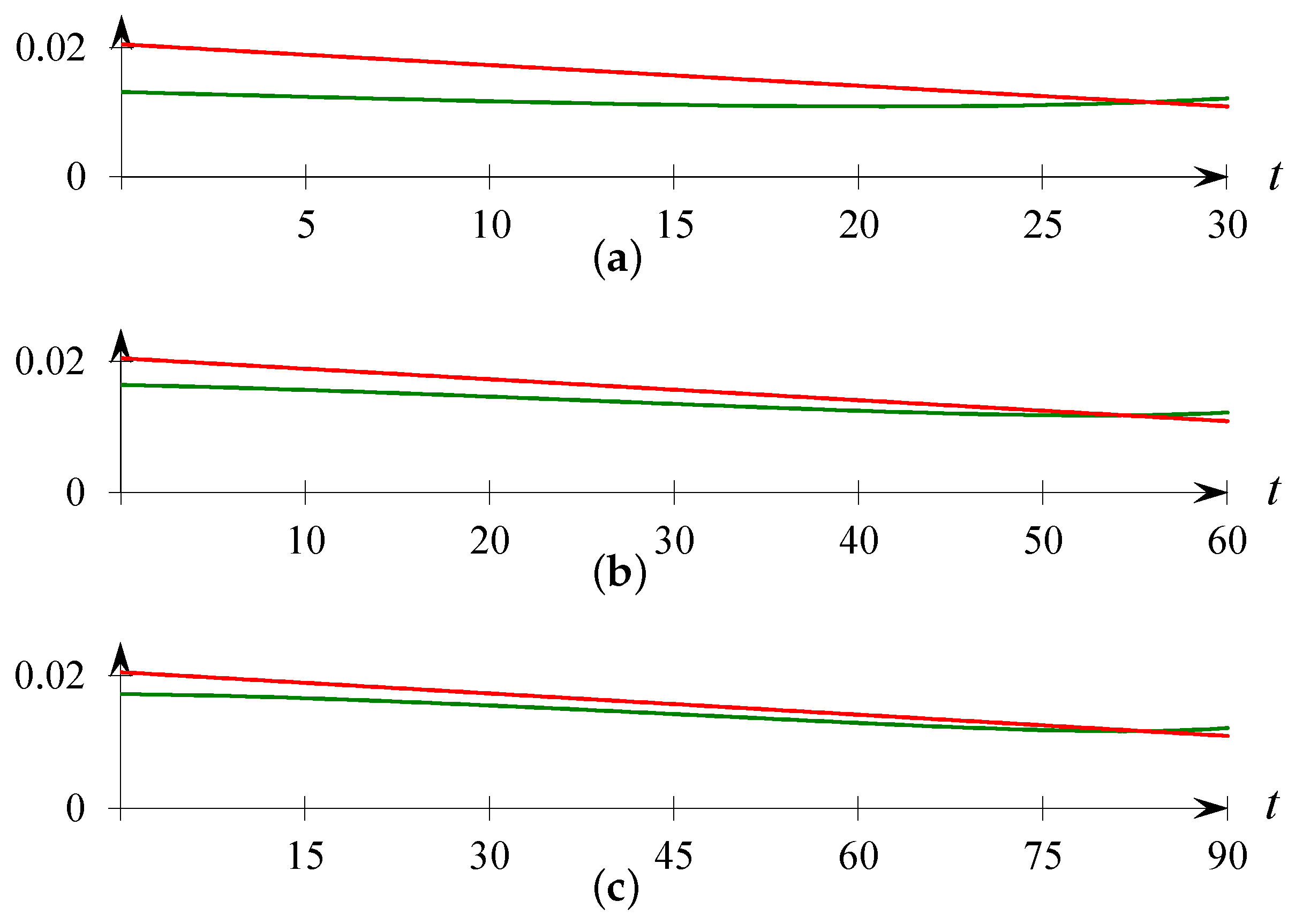
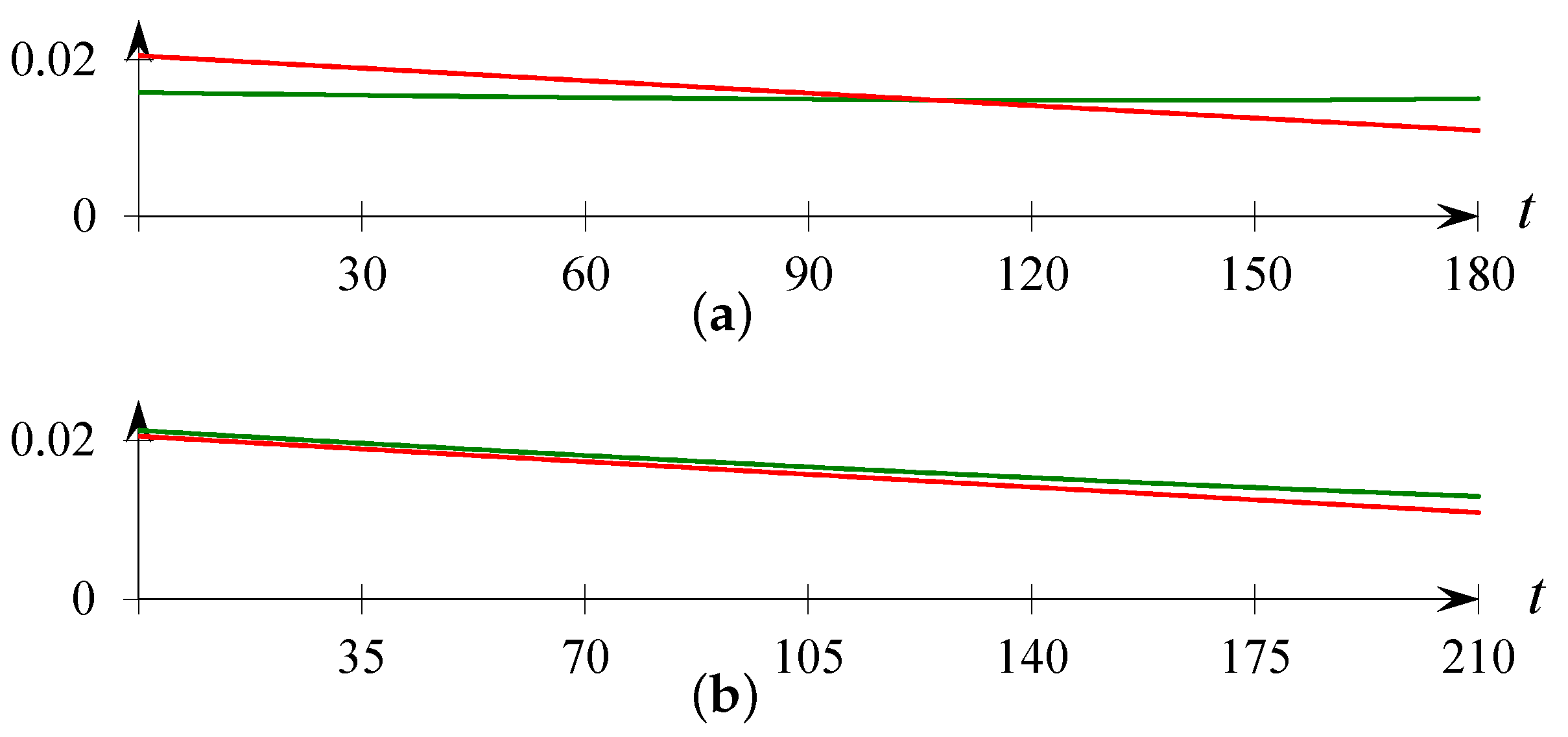
6. Computational Experiment
6.1. Case 1
6.2. Case 2
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Shangina, O.R.; Gaynutdinova, R.D. Interaction of laser radiation with biological tissues. Pract. Med. 2019, 17, 24–27. (In Russian) [Google Scholar] [CrossRef]
- Elagin, V.V.; Shakhova, M.A.; Karabut, M.M.; Kuznetsova, D.S.; Bredikhin, V.I.; Prodanets, N.N.; Snopova, L.B.; Baskina, O.S.; Shakhov, A.V.; Kamensky, V.A. Evaluation of Cutting Properties of a Laser Scalpel with Heavily Absorbing Coatings of an Optical Fiber. Sovrem. Tehnol. Med. 2015, 7, 55–60. [Google Scholar] [CrossRef][Green Version]
- Skobelkin, O.K. Lasers in Surgery; Meditsina: Moscow, Russia, 1989; 256p. (In Russian) [Google Scholar]
- Privalov, V.E.; Turkin, V.A.; Shemanin, V.G. Lasers and green energy. Lasers Meas. Inf. 2022, 2, 5–11. (In Russian) [Google Scholar] [CrossRef]
- Kumar, R.; Pérez del Pino, A.; Sahoo, S.; Kumar Singh, R.; Tan, W.K.; Kar, K.K.; Matsuda, A.; Joanni, E. Laser processing of graphene and related materials for energy storage: State of the art and future prospects. Prog. Energy Combust. Sci. 2022, 91, 100981. [Google Scholar] [CrossRef]
- Gupta, M.C.; Carlson, D.E. Laser processing of materials for renewable energy applications. MRS Energy Sustain. 2015, 2, 2. [Google Scholar] [CrossRef]
- Son, E.E.; Sheindlin, M.A. Lasers in high-temperature energy materials. Proc. Russ. Acad. Sci. Energy 2011, 5, 88–103. (In Russian) [Google Scholar]
- Wongchadakul, P.; Rattanadecho, P. Mathematical modeling of multilayered skin with embedded tumor through combining laser ablation and nanoparticles: Effects of laser beam area, wavelength, intensity, tumor absorption coefficient and its position. Int. J. Heat Technol. 2021, 39, 89–100. [Google Scholar] [CrossRef]
- Cummins, L.; Nauenberg, M. Thermal effects of laser radiation in biological tissue. Biophys. J. 1983, 42, 99–102. [Google Scholar] [CrossRef]
- Zhou, J.; Chen, J.K.; Zhang, Y. Theoretical analysis of thermal damage in biological tissues caused by laser irradiation. Mol. Cell. Biomech. 2007, 4, 27–39. [Google Scholar] [PubMed]
- Belozerov, L.G.; Kireev, V.A. Composite Shells under Force and Thermal Effects; Fizmatlit: Moscow, Russia, 2003; 388p. (In Russian) [Google Scholar]
- Gubin, A.I.; Malaya, Y.A. Mathematical modeling of thermal processes during laser processing of materials based on the nonlinear hyperbolic equation of heat conduction. In Tekhnichna Teplofizika i Promislova Teploenergetika; New Ideology: Dnipropetrovsk, Ukraine, 2011; Volume 3, pp. 72–85. (In Russian) [Google Scholar]
- Svet, E.V. The nonstationary problem of heat conduction in three-dimensional formulation for laminated plates with complex shape. Bull. NTU KhPI 2013, 63, 122–131. (In Russian) [Google Scholar]
- Yamaoka, N.; Sugie, J. Multilayer structures of second-order linear differential equations of Euler type and their application to nonlinear oscillations Ukr. Math. J. 2006, 58, 1935–1949. [Google Scholar]
- Megel, Y.E.; Levkin, D.A. Mathematical model of thermal heating of a multilayer microbiological object. East Eur. J. Adv. Technol. 2012, 3/4, 4–7. (In Russian) [Google Scholar]
- Shupikov, A.N.; Buzko, Y.P.; Smetankina, N.V.; Ugrimov, S.V. Non-Stationary Oscillations of Multilayer Plates and Shells and Their Optimization; Scientific Publication; Publishing House of KhNEU: Kharkov, Ukraine, 2004; 252p. (In Russian) [Google Scholar]
- Pyatkov, S.G. Certain inverse problems for parabolic equations. J. Math. Sci. 2008, 150, 2422–2433. [Google Scholar] [CrossRef]
- Kantor, B.Y.; Smetankina, N.V.; Shupikov, A.N. Analysis of non-stationary temperature fields in laminated strips and plates. Int. J. Solids Struct. 2001, 38, 8673–8684. [Google Scholar] [CrossRef]
- Barseghyan, V.R. Control of Stage by Stage Changing Linear Dynamic Systems. Yugosl. J. Oper. Res. 2012, 22, 31–39. [Google Scholar] [CrossRef]
- Barseghyan, V.R. On the controllability and observability of linear dynamic systems with variable structure. In Proceedings of the 2016 International Conference “Stability and Oscillations of Nonlinear Control Systems” (Pyatnitskiy’s Conference), STAB 2016, Moscow, Russia, 1–3 June 2016. [Google Scholar]
- Barseghyan, V.R. The Problem of Boundary Control of Displacement at Two Ends by the Process of Oscillation of a Rod Consisting of Two Sections of Different Density and Elasticity. Mech. Solids 2023, 58, 483–491. [Google Scholar] [CrossRef]
- Barseghyan, V.; Solodusha, S. On the Optimal Control Problem for Vibrations of the Rod/String Consisting of Two Non-Homogeneous Sections with the Condition at an Intermediate Time. Mathematics 2022, 10, 4444. [Google Scholar] [CrossRef]
- Barseghyan, V.R.; Barseghyan, T.V. On an Approach to the Problems of Control of Dynamic System with Nonseparated Multipoint Conditions. Autom. Remote Control 2015, 76, 549–559. [Google Scholar] [CrossRef]
- Zubov, V.I. Lectures on Control Theory; Nauka: Moscow, Russia, 1975. (In Russian) [Google Scholar]
- Mispahov, I.S.; Magomedov, D.A. Mathematical model of the thermoelectric system for short-term storage and transportation of biological material. Bull. Dagestan State Tech. Univ. Tech. Sci. 2012, 26, 7–15. (In Russian) [Google Scholar]
| (s) | 30 | 60 | 90 |
|---|---|---|---|
| (s) | 30 | 60 | 90 | 180 | 210 |
|---|---|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barseghyan, V.; Solodusha, S. A Model of the Control Problem of the Thermal Effect of a Laser Beam on a Two-Layer Biomaterial. Mathematics 2024, 12, 374. https://doi.org/10.3390/math12030374
Barseghyan V, Solodusha S. A Model of the Control Problem of the Thermal Effect of a Laser Beam on a Two-Layer Biomaterial. Mathematics. 2024; 12(3):374. https://doi.org/10.3390/math12030374
Chicago/Turabian StyleBarseghyan, Vanya, and Svetlana Solodusha. 2024. "A Model of the Control Problem of the Thermal Effect of a Laser Beam on a Two-Layer Biomaterial" Mathematics 12, no. 3: 374. https://doi.org/10.3390/math12030374
APA StyleBarseghyan, V., & Solodusha, S. (2024). A Model of the Control Problem of the Thermal Effect of a Laser Beam on a Two-Layer Biomaterial. Mathematics, 12(3), 374. https://doi.org/10.3390/math12030374







