Convergence of Graph-Based Fixed Point Results with Application to Fredholm Integral Equation

Abstract

In this manuscript, we present a novel concept termed graphical ${\Theta }_c$-Kannan contraction within the context of graphically controlled metric-type spaces. Unlike traditional Kannan contraction, this novel concept presents a modified method of contraction mapping. We discuss the significance and the existence of fixed point results within the framework of this novel contraction. To strengthen the credibility of our theoretical remarks, we provide a comparison example demonstrating the efficiency of our suggested framework. Our study not only broadens the theoretical foundations inside graphically controlled metric-type spaces by introducing and examining visual ${\Theta }_c$-Kannan contraction, but it also demonstrates the practical significance of our innovations through significant examples. Furthermore, applying our findings to second-order differential equations by constructing integral equations into the domain of Fredholm sheds light on the broader implications of our research in the field of mathematical analysis and contributes to the advancement of this field.

1. Introduction

Fixed point theory is a fundamentally important subject in mathematics, with applications in a variety of disciplines, including functional analysis, nonlinear analysis, and optimization. The idea is based on the investigation of mappings that contain points that remain unchanged under the mapping, known as fixed points. Fixed point theory appeared in the late nineteenth and early twentieth century when famous mathematicians had significant breakthroughs in this field. The inception of fixed point theory can be attributed to the pioneering work of Henri Poincare. In 1890, Poincare introduced the concept of a fixed point while studying the properties of continuous mappings in Euclidean spaces [1]. His investigations laid the groundwork for subsequent developments in this field. Another crucial advancement occurred in the early 20th century with the famous Brouwer fixed point theorem. In 1912, Luitzen Egbertus Jan Brouwer formulated and proved this theorem, which asserts that any continuous function from a closed ball to itself must possess at least one fixed point [2]. Brouwer’s theorem had far-reaching consequences in topology and nonlinear analysis. Building upon these foundational results, the mid-20th century witnessed a surge of interest in fixed point theory. Banach’s fixed point theorem, proven by Stefan Banach in 1922, holds a significant position in this landscape. This theorem guarantees the existence of a unique fixed point for contraction mapping in a complete metric space [3]. Fixed point theory continues to evolve with contemporary research exploring advanced topics such as variational inequalities, generalized contractions, and fixed points in metric and partial metric spaces. The theory’s versatility and profound implications make it an indispensable tool in the arsenal of mathematicians and scientists alike. P. S. Kumari et al. [4] explored the concept of cyclic compatible contractions, extending fixed point theorems by introducing new types of mappings. The authors conducted a rigorous investigation into the conditions under which such mappings guarantee fixed points in metric spaces. Subsequently, P. S. Kumari et al. [5] connected different types of cyclic contractions with contractive self-mappings and examined their relations with Hardy–Rogers self-mappings, offering novel insights into the convergence properties of these mappings in the context of fixed point theory. Afterwards, P.S. Kumari [6] presented a straightforward and effective method of solving nonlinear integral equations and nonlinear fractional differential equations of the Caputo type with the application of the fixed point approach in 2018.

The study of graph-based metric spaces and their significant applications in fixed point theory has resulted in a number of outstanding achievements over the years. This chronological narrative focuses on major articles that have enhanced our understanding of such mathematical ideas and their applications.

S. Shukla, in [7], introduced a pioneering perspective in 2017 by formulating the concept of a graphical metric space. Their work extended the horizons of fixed point theory by introducing a novel setting, laying the groundwork for subsequent research in this area. In 2019, the field saw significant development with two pivotal articles. N. Chuensupantharat et al. [8] delved into graphical b-metric spaces via graphic contraction mappings, establishing foundational results and showcasing their applications. This work illustrated the practicality of graph-based metric spaces in modeling real-world systems. Also, it expanded the theoretical understanding of these spaces and highlighted their potential applications across diverse areas. On the other hand, in developing extensive research of fixed point theory for various abstract spaces, due to the very numerous articles published in the last ten years in this field, and with the aim of not having too extensive a bibliography, we will limit ourselves to the reference [9].

In 2023, M. Younis and D. Bahuguna [10] offered a distinctive perspective by applying graph-based metric spaces to the realm of rocket ascension. This unique approach showcased the versatility of these mathematical structures in capturing and analyzing dynamic phenomena. A recent investigation expanded the scope of graph-based metric spaces and highlighted further connections to fixed point theory. This chronological overview highlights the progressive evolution of fixed point theory via metric spaces connected with graphs. From foundational concepts to diverse applications, the contributions outlined above collectively illustrate the ongoing exploration and significance of this emerging mathematical field. Graphical fixed point theory is an intersection of fixed point theory and graph theory.

In recent years, the field of fixed point theory has witnessed significant developments and applications. In 2014, N. Hussain [11] introduced the concept of $\alpha -\psi -$contractions in metric spaces endowed with a graph. Building upon this, N. Hussain [12] extended the theory by introducing generalized Mizoguchi–Takahashi graphic contractions in 2016. In 2021, M. Younis [13] delved into the graphical structure of extended b-metric spaces and applied it to study the transverse oscillations of a homogeneous bar. Moreover, it has been used in constructing mathematical models in some fields of fractional calculus and in obtaining (potential) applications to physics. These works collectively contribute to the ongoing development and application of fixed point theory in various mathematical contexts.

Recent articles published in 2024 have made notable contributions to the field of graphical metric spaces, particularly in the development of fixed point theorems and their diverse applications. Baradol et al. [14] introduced a novel fixed point result in graphical $bv\left(s\right)$-metric spaces, extending the scope of fixed point theory and applying it to solve differential equations. This is complemented by Fallahi [15], who explored graphical cyclic $\mathcal{K}$-quasi-contractive mappings and their best proximity points, contributing to the understanding of proximity in graphical spaces. Jiddah et al. [16] further extended these results by formulating hybrid fixed point theorems involving graphically contractions, which have wide applications in diverse mathematical models. Similarly, Jabeen et al. [17] focused on convergence results based on graph-Reich contractions in fuzzy metric spaces, offering solutions to control and optimization problems. Lastly, Dubey et al. [18] addressed graphical symmetric spaces, proposing fixed point theorems and positive solutions for fractional periodic boundary value problems, further bridging the gap between graphical and fractional-order systems.

Motivated by the work conducted in the literature, we dive into a similar exciting concept, calling it graphical ${\Theta }_c$-Kannan-type contraction in the setting of graphically controlled metric-type spaces, and show that every graphical ${\Theta }_c$-Kannan-type contraction is not Kannan contraction, and subsequently establish some fixed point results for this new contraction. Then, we provide an example to check the validity of our theoretical result. Subsequently, we use the results gained to demonstrate the validity of the solution to a particular second-order boundary value problem via the Fredholm integral equation.

2. Preliminaries

Following Jachymski [19], let $\Omega$ be a nonempty set and $\Delta$ be the diagonal of $\Omega \times \Omega$. Further, let $\Theta =\left(V\right(\Theta ),E(\Theta \left)\right)$ be a directed graph without parallel edges, where $V(\Theta )$ is the vertex set and $E(\Theta )$ is the edge set that contains all the loops of $\Theta$. Also, we denote a graph obtained by reversing the direction of $E(\Theta )$ as ${\Theta }^{-1}$. The graph $\Theta$ is labeled as $\breve{\Theta }$, especially when it contains symmetrical edges such that

$$E\left(\breve{\Theta }\right)=E\left({\Theta }^{-1}\right)\cup E(\Theta ).$$

Let ℑ and $\nu$ be vertices of a graph $\Theta$. A path in $\Theta$ is defined as a sequence ${\left\{{\Im }_j\right\}}_{j=0}^m$ containing $(m+1)$ vertices in such a way that ${\Im }_0=\Im$, ${\Im }_m=\nu$ with $\left({\Im }_{j-1},{\Im }_j\right)\in E(\Theta )$, where $j=1,2,\dots ,m$. A graph $\Theta$ is said to be connected if there is a path between any pair of vertices. If the graph $\Theta$ is undirected and contains a path that connects each pair of vertices, then we say that it is weakly connected. Furthermore, a graph $\theta =\left(V\left(\theta \right),E\left(\theta \right)\right)$ is frequently referred to as a subgraph if $V\left(\theta \right)\subseteq V(\Theta )$ and $E\left(\theta \right)\subseteq E(\Theta )$.

The following are the notations of Shukla [7]:

• ${[\Im ]}_{\Theta }^l=\{\nu \in \Omega :$ There exists a directed path from ℑ to $\nu$ in graph $\Theta$ with a length of $l\}$.
  Further, a relation exists between $\mathrm{P}$ and $\Omega$ in such a way that the following is true:
• ${\left(\Im \mathrm{P}\nu \right)}_{\Theta }$: if there exists a directing path from ℑ to $\nu$ in $\Theta$ and $\xi {\in (\Im \mathrm{P}\nu )}_{\Theta }$, then $\xi$ lies within the path $\xi {\in (\Im \mathrm{P}\nu )}_{\Theta }$.

A sequence $\left\{{\Im }_n\right\}\subset \Omega$ is considered $\Theta$-termwise-connected $(\Theta -\Psi WC)$ if ${\left({\Im }_n\mathrm{P}{\Im }_{n+1}\right)}_{\Theta }$ for all $n\in \mathbb{N}$. We will treat all graphs as directed unless mentioned otherwise. From now on, we will represent a controlled metric-type space as $(\Omega ,\Gamma )$ and a graphically controlled metric-type space as $(\Omega ,{\Gamma }_{\Theta })$.

In 2018, N. Mlaiki [20] gave the concept of a controlled metric-type space as follows:

Definition 1.

Let $\alpha :\Omega \times \Omega \to [1,\infty )$ and $\Gamma :\Omega \times \Omega \to [0,\infty )$ be a pair of mappings on a nonempty set Ω satisfying the following for all $\Im ,\nu ,\xi \in \Omega$:

1.

If $\Gamma (\Im ,\nu )=0,$ then $\Im =\nu .$

2.

If $\Im =\nu ,$ then $\Gamma (\Im ,\nu )=0.$

3.

$\Gamma (\Im ,\nu )=\Gamma (\nu ,\Im ),$ for all $\Im \in \Omega .$

4.

${(\Im \mathrm{P}\nu )}_{\Theta },\xi \in {(\Im \mathrm{P}\nu )}_{\Theta }\Rightarrow \Gamma (\Im ,\nu )\le \alpha (\Im ,\xi )\Gamma (\Im ,\xi )+\alpha (\xi ,\nu )\Gamma (\xi ,\nu ).$

Then, the pair $(\Omega ,\Gamma )$, is referred to as a controlled metric-type space.

In 2023, the proposal of graphically controlled metric-type space was established by M. Younis as follows:

Definition 2

([10]). Let Ω be a nonempty set associated with graph Θ, and $\alpha :\Omega \times \Omega \to [1,\infty )$ be a function. Assume that $\Gamma :\Omega \times \Omega \to [0,\infty )$ satisfies the following:

1.

If $\Gamma (\Im ,\nu )=0,$ then $\Im =\nu .$

2.

If $\Im =\nu ,$ then $\Gamma (\Im ,\nu )=0.$

3.

$\Gamma (\Im ,\nu )=\Gamma (\nu ,\Im ),$ for all $\Im \in \Omega .$

4.

${(\Im \mathrm{P}\nu )}_{\Theta },\xi \in {(\Im \mathrm{P}\nu )}_{\Theta }\Rightarrow \Gamma (\Im ,\nu )\le \alpha (\Im ,\xi )\Gamma (\Im ,\xi )+\alpha (\xi ,\nu )\Gamma (\xi ,\nu ),$

for all $\Im ,\nu ,\xi \in \Omega$. Then, the pair $(\Omega ,{\Gamma }_{\Theta })$ is referred to as a graphically controlled metric-type space.

Remark 1.

Note that every controlled metric-type space $(\Omega ,\Gamma )$ is a graphically controlled metric-type space $(\Omega ,{\Gamma }_{\Theta })$. The preceding example endorses our claim.

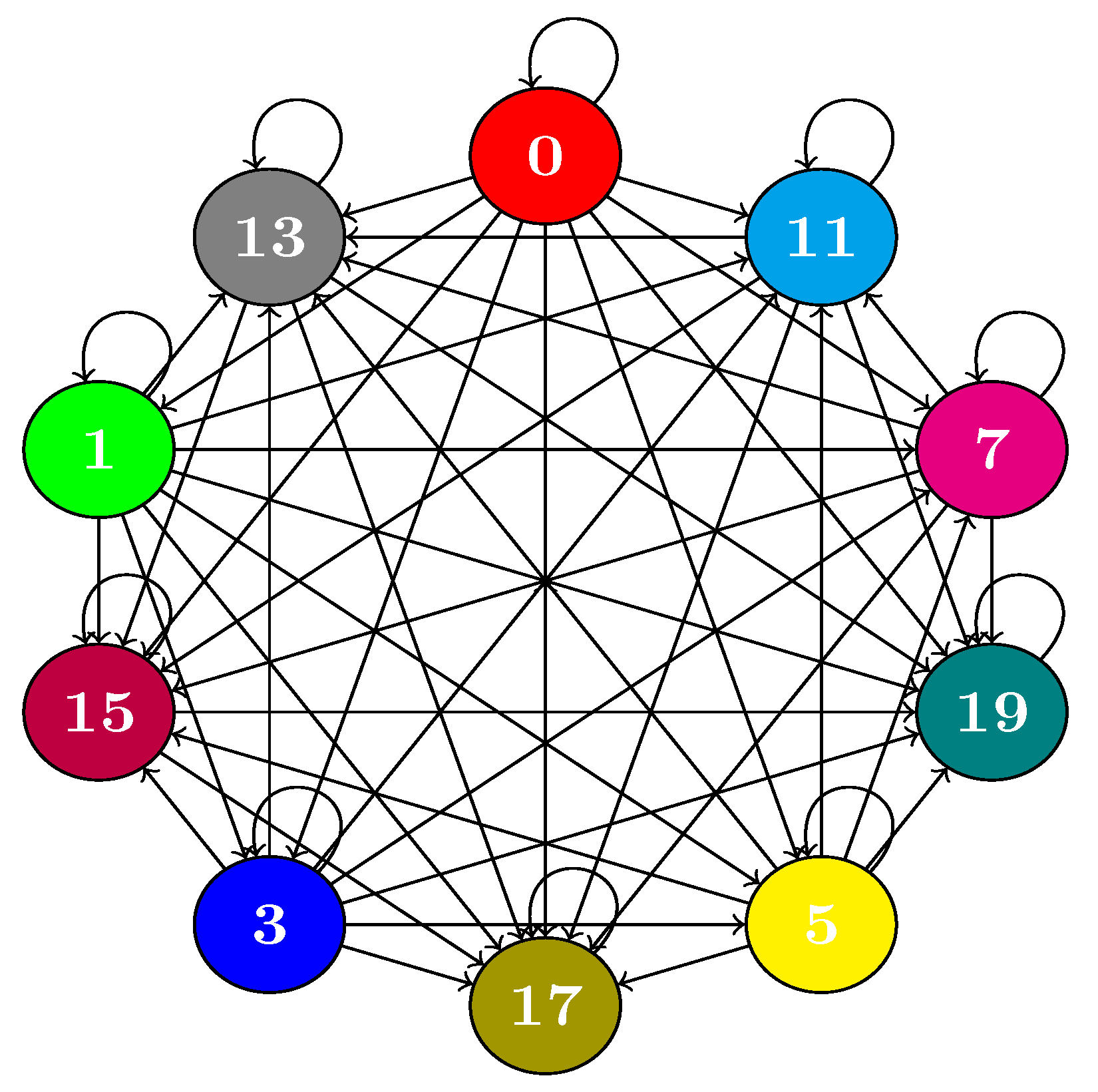
Example 1.

Let $\Omega =\{0,1,3,5,7,11,13,15,17,19\}$ with $\alpha (\Im ,\nu )={\displaystyle \frac{1}{\Im \nu +2}}+{\displaystyle \frac{1}{\Im \nu +3}}+2$ and define $\Gamma :\Omega \times \Omega \to [0,\infty )$ such that

$$\Gamma (\Im ,\nu )=\left\{\begin{array}{cc}{\left|\Im -\nu \right|}^2\hfill & \mathit{if}\Im \ne \nu \hfill \\ 0,\hfill & \mathit{if}\Im =\nu \hfill \end{array}\right..$$

Consequently, $(\Omega ,\Gamma )$ is a controlled metric-type space. Next, we will examine it with the graph Θ, where $V(\Theta )=\Omega$, and $E(\Theta )$ is classified as

$$E(\Theta )=\Delta \cup \left\{\begin{array}{c}(0,1),(0,3),(0,5),(0,7),(0,11),(0,13),(0,15),(0,17),\\ (0,19),(1,3),(1,5),(1,7),(1,11),(1,13),(1,15),(1,17),\\ (1,19),(3,5),(3,7),(3,11),(3,13),(3,15),(3,17),(3,19),\\ (5,7),(5,11),(5,13),(5,15),(5,17),(5,19),(7,11),(7,13),\\ (7,15),(7,17),(7,19),(11,13),(11,15),(11,17),(11,19),\\ (13,15),(13,17),(13,19),(15,17),(15,19),(17,19).\end{array}\right.$$

Obviously, a controlled metric-type space $(\Omega ,\Gamma )$ is a graphically controlled metric-type space $(\Omega ,{\Gamma }_{\Theta })$ spanning the graph Θ, as shown in Figure 1.

Remark 2.

Note that a graphically controlled metric-type space $(\Omega ,{\Gamma }_{\Theta })$ is not always a controlled metric-type space $(\Omega ,\Gamma )$. The preceding Example 2 validates our argument.

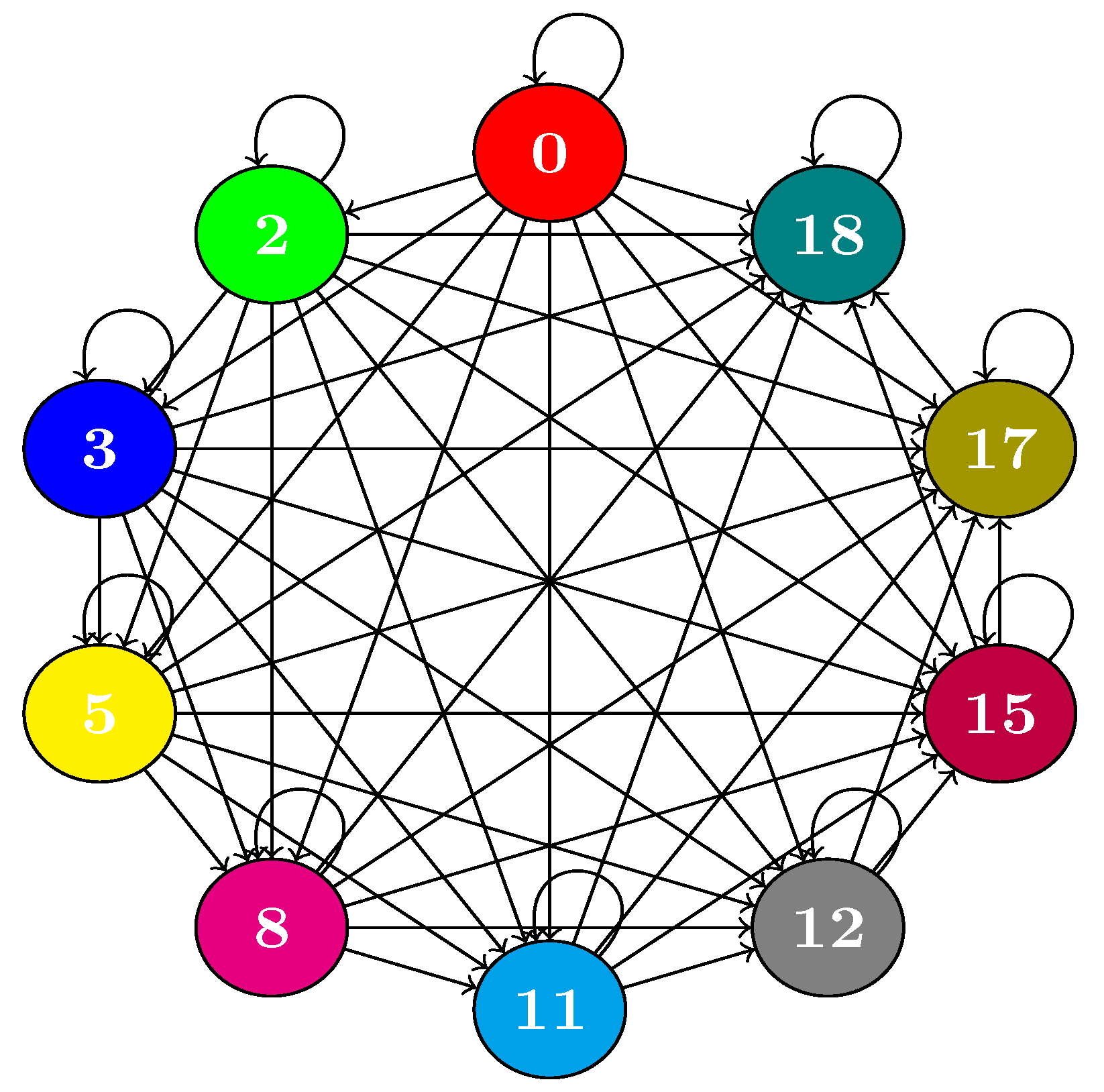
Example 2.

Let $\Omega =\{0,2,3,5,8,11,12,15,17,18\}$, $\Gamma :\Omega \times \Omega \to [0,\infty )$ be a function defined as

$$\Gamma (\Im ,\nu )=\left\{\begin{array}{cc}{\left|\Im -\nu \right|}^2\hfill & \mathit{if}\Im \ne \nu \hfill \\ 0,\hfill & \mathit{if}\Im =\nu \hfill \end{array}\right.$$

and $\alpha :\Omega \times \Omega \to [1,\infty )$ be a function such that $\alpha (\Im ,\nu )={\displaystyle \frac{1}{\Im \nu +2}}+{\displaystyle \frac{1}{\Im \nu +3}}+1.9803.$ Clearly $(\Omega ,{\Gamma }_{\Theta })$ is a graphically controlled metric-type space, where $\Omega =V(\Theta )$ and $E(\Theta )$, as shown in Figure 2.

Now, we observe that

$$\begin{array}{ccc}\hfill \Gamma (12,18)& \le & \alpha (12,15)\Gamma (12,15)+\alpha (15,18)\Gamma (15,18)]\hfill \\ \hfill 36& \nleqq & 35.806.\hfill \end{array}$$

Hence, a graphically controlled metric-type space $(\Omega ,{\Gamma }_{\Theta })$ is not necessarily a controlled metric-type space $(\Omega ,\Gamma )$.

Remark 3.

In light of Examples 1 and 2, we can see that the results propounded in this article are real extensions of the results proven in the existing state of the art. More specifically, Γ does not satisfy the contraction conditions within the context of b-metric spaces, controlled metric spaces, extended b-metric spaces, and graphical metric spaces. Hence, the results in the aforementioned spaces can not be applied to Γ induced in this article.

Definition 3

([10]). Let $(\Omega ,{\Gamma }_{\Theta })$ be a graphically controlled metric-type space.

(i) 

A sequence $\left\{{\Im }_n\right\}$ is called convergent to ℑ if for each $ϵ\ge 0$, there is some positive integer $N_{ϵ}$ such that $\Gamma \left({\Im }_n,\Im \right)<ϵ$ for each $n\ge N_{ϵ}$. This can be written as

$$\underset{n\to \infty }{lim}{\Im }_n=\Im .$$

(ii) 

A sequence $\left\{{\Im }_n\right\}$ is called a Cauchy sequence if for each $ϵ>0$, $\Gamma \left({\Im }_n,{\Im }_m\right)<ϵ$ for all $m,n\ge N_{ϵ}$, as $N_{ϵ}\in \mathbb{N}.$

(iii) 

The $(\Omega ,{\Gamma }_{\Theta })$ is referred to as $\Theta -$complete provided each Cauchy sequence becomes converged in Ω.

3. Main Results

In the following section, we examine fixed points linked with a through visually controlled metric space $(\Omega ,{\Gamma }_{\Theta }).$ In addition, we also consider the graph $\Theta$ to be a graph with weights. Let ${\Im }_0\in \Omega$ be the initial value of the sequence $\left\{{\Im }_n\right\}$; we say $\left\{{\Im }_n\right\}$ is $\Psi -$Picard’s sequence $(\Psi -PS)$ if ${\Im }_n=\Psi {\Im }_{n-1}$∀$n\in \mathbb{N}.$

Notion: We denote as ${(\Im \mathrm{P}\nu )}_{\Theta },$ and consider a relation to exist between $\mathrm{P}$ and $\Omega$ if there exists a path from ℑ to $\nu$ in $\Theta$. If $\xi \in {(\Im \mathrm{P}\nu )}_{\Theta }$, we say $\xi$ lies on the path $\mathrm{P}$. Furthermore, a sequence $\left\{{\Im }_n\right\}\in \Omega$ is considered $\Theta$-termwise-connected $(\Theta -\Psi WC)$ if $\left({\Im }_n\mathrm{P}{\Im }_{n+1}\right)$ for all $\Omega \in \Omega$, and $n\in \mathbb{N}.$ Also, a graph $\Theta =\left(V\right(\Theta ),E(\Theta \left)\right)$ meets the criteria $\left(\mathrm{P}\right),$ if $\Theta$ is connected termwise to $\Psi$-Picard’s sequence and converges in $\Omega ,$ which guarantees that there is a limit ${\Im }^{\prime }$, such as $\left({\Im }_n,{\Im }^{\prime }\right)\in E(\Theta )$ or $\left({\Im }^{\prime },{\Im }_n\right)\in E(\Theta )$, for all $n>n_0.$

Definition 4.

Let $(\Omega ,{\Gamma }_{\Theta })$ be a graphically controlled metric-type space. A mapping $\Psi :\Omega \to \Omega$ is said to be a Kannan contraction if it satisfies

$$\Gamma (\Psi \Im ,\Psi \nu )\le \beta [\Gamma (\Im ,\Psi \Im )+\Gamma (\nu ,\Psi \nu \left)\right]$$

for all $\Im ,\nu \in \Omega$ and $\beta \in (0,{\displaystyle \frac{1}{2}})$.

Definition 5.

Let $(\Omega ,{\Gamma }_{\Theta })$ be linked with a graph Θ containing all of the loops. A mapping $\Psi :\Omega \to \Omega$ is said to be a graphical ${\Theta }_c-$Kannan-type contraction with following properties:

1.

For all $\Im ,\nu \in \Omega$

$$\mathit{if}(\Im ,\nu )\in E(\Theta )\mathit{then}(\Psi \Im ,\Psi \nu )\in E(\Theta ),$$

(1)

so that Ψ retains the edges of $E(\Theta ).$

2.

Then, there exists $\beta \in (0,{\displaystyle \frac{1}{2}})$ and $(\Im ,\nu )\in E(\Theta )$, ∀$\Im ,\nu \in \Omega$, in such a way that

$$\Gamma (\Psi \Im ,\Psi \nu )\le \beta [\Gamma (\Im ,\Psi \Im )+\Gamma (\nu ,\Psi \nu \left)\right].$$

(2)

Remark 4.

Note that graphical ${\Theta }_c-$Kannan is not always Kannan mapping. The following example supports our argument.

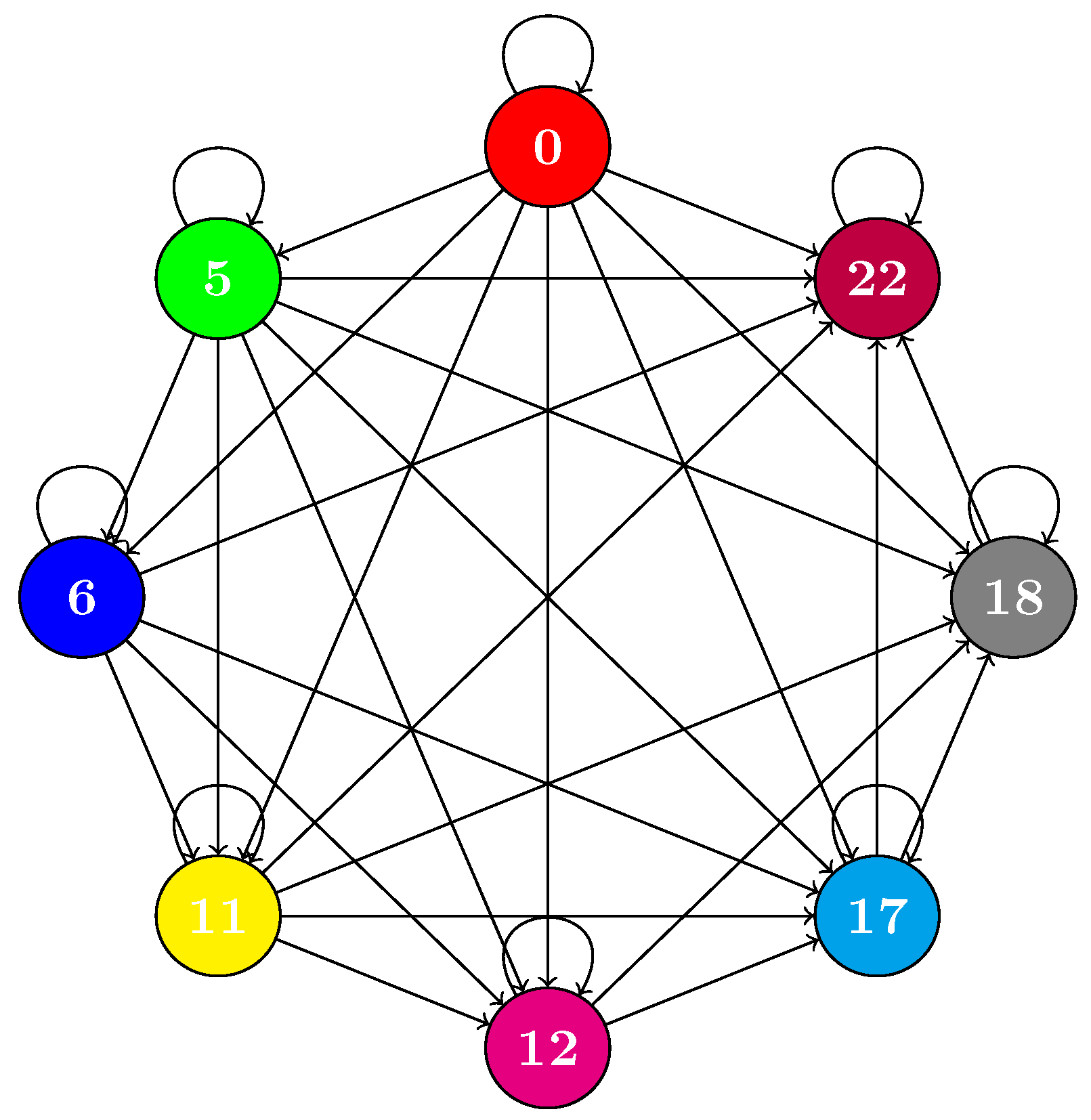
Example 3.

Let $\Omega =\{0,5,6,11,12,17,18,22\}$ and $\alpha :\Omega \times \Omega \to [1,\infty )$ be a function such that

$$\alpha (\Im ,\nu )={\displaystyle \frac{1}{\Im \nu +2}}+{\displaystyle \frac{1}{\Im \nu +3}}+1.9803.$$

The function $\Gamma :\Omega \times \Omega \to [0,\infty )$ is defined as

$$\Gamma =\left\{\begin{array}{cc}{\left|\Im -\nu \right|}^2\hfill & \mathit{if}\Im \ne \nu \hfill \\ 0,\hfill & \mathit{if}\Im =\nu \hfill \end{array}\right.$$

and is associated with a graph where $V(\Theta )=\Omega$ is the vertex set and $E(\Theta )$ is the edge set mentioned, as demonstrated in Figure 3.

Certainly, $(\Omega ,{\Gamma }_{\Theta })$ is a graphically controlled metric-type space, as demonstrated in Figure 3, but not a traditionally controlled metric-type space for

$$\begin{array}{ccc}\hfill \Gamma (6,18)& \le & \alpha (6,12)\Gamma (6,12)+\alpha (12,18)\Gamma (12,18)]\hfill \\ \hfill 144& \nleqq & 143.88.\hfill \end{array}$$

Similarly, for

$$\begin{array}{ccc}\hfill \Gamma (12,22)& \le & \alpha (12,17)\Gamma (12,17)+\alpha (17,22)\Gamma (17,22)]\hfill \\ \hfill 100& \nleqq & 99.39.\hfill \end{array}$$

Define a mapping as

$$\Psi \Im =\left\{\begin{array}{cc}6,\hfill & \mathit{if}\Im =\left\{22\right\}\hfill \\ 11,\hfill & \mathit{for}\Im =\{12,0\}.\hfill \end{array}\right.$$

It is easy to see that Ψ is a graphical ${\Theta }_c-$Kannan mapping for $\beta ={\displaystyle \frac{1}{11}}.$

If we take $\Im =22$ and $\nu =0$, then

$$\begin{array}{ccc}\hfill \Gamma (T22,T0)& \le & \beta [\Gamma (22,\Psi 22)+\Gamma (0,\Psi 0\left)\right]\hfill \\ \hfill 25& \le & 377\beta .\hfill \end{array}$$

But if we do not use a traditional Kannan mapping for $\Im =22$ and $\nu =12$, then

$$\begin{array}{ccc}\hfill \Gamma (T22,T12)& \le & \beta [\Gamma (22,\Psi 22)+\Gamma (12,\Psi 12\left)\right]\hfill \\ \hfill 25& \nleqq & 257\beta .\hfill \end{array}$$

Hence, the above inequality demonstrates that Ψ is a graphical ${\Theta }_c-$Kannan mapping rather than a Kannan mapping.

Theorem 1.

Assume that ${\Theta }_c-$ is a graphical Kannan-type contraction on a Θ-complete graph $(\Omega ,{\Gamma }_{\Theta }).$ If the graph Θ fulfills the $\left(\mathrm{P}\right)$ property, then there is ${\Im }_0\in \Omega$ with $\Psi {\Im }_0\in {\left[{\Im }_0\right]}_{\Theta }^l$, with $l\in \mathbb{N}$ in particular, where $\left\{{\Im }_n\right\}$ is $\Psi -PS$ with the initial value ${\Im }_0\in \Omega$. Then, there exists ${\Im }^{\prime }\in \Omega$ such that the $\Psi -PS\left\{{\Im }_n\right\}$ is $\Theta -\Psi WC$ and converges to ${\Im }^{\prime }.$

Proof. 

Let ${\Im }_0\in \Omega$, such that $\Psi {\Im }_0\in {\left[{\Im }_0\right]}_{\Theta }^l$, with $l\in \mathbb{N}$ in particular. By choosing an arbitrary ${\Im }_0$ from the $\Psi$ Picard sequence $\left\{{\Im }_n\right\},$ the path ${\left\{{\nu }_j\right\}}_{j=0}^l,$ can be derived by calculating ${\Im }_0={\nu }_0,$$\Psi {\Im }_0={\nu }_1$ and $({\nu }_{j-1},{\nu }_j)\in E(\Theta )$, particularly for $j=1,2,\dots ,l.$ Utilizing (1), we are now equipped with $(\Psi {\nu }_{j-1},\Psi {\nu }_j)\in E(\Theta )$, specifically for $j=1,2,\dots ,l.$ This indicates that ${\{\Psi {\nu }_j\}}_{j=0}^l$ is a path from $\Psi {\nu }_0=\Psi {\Im }_0={\Im }_1$ to $\Psi {\nu }_1={\Psi }^2{\Im }_0$$={\Im }_2$ with l as its length such that ${\Im }_2\in {\left[{\Im }_1\right]}_{\Theta }^l.$ Using this approach, we arrive at the premise that ${\left\{{\Psi }^n{\nu }_j\right\}}_{j=0}^l$ is a path from ${\Psi }^n{\Im }_0={\Psi }^n{\nu }_0={\Im }_n$ to ${\Psi }^n{\Im }_1={\Psi }^n\Psi {\nu }_0={\Im }_{n+1}$ with l as its length, and consequently, ${\Im }_{n+1}\in {\left[{\Im }_n\right]}_{\Theta }^l$ for all $n\in \mathbb{N}$. This highlights that $\left\{{\Im }_n\right\}$ is a $\Theta -\Psi WC$ sequence, so

$$({\Psi }^n{\Im }_{j-1},{\Psi }^n{\Im }_j)\in E(\Theta )\mathrm{for}n\in \mathbb{N}\mathrm{and}j=1,2,\dots ,l.$$

Following (2), we obtain

$$\begin{array}{ccc}\hfill \Gamma ({\Psi }^n{\Im }_{j-1},{\Psi }^n{\Im }_j)& =& \Gamma (\Psi \left({\Psi }^{n-1}{\Im }_{j-1}\right),\Psi \left({\Psi }^{n-1}{\Im }_j\right))\hfill \\ & \le & \beta [\Gamma ({\Psi }^{n-1}{\Im }_{j-1},\Psi \left({\Psi }^{n-1}{\Im }_{j-1}\right)+\Gamma ({\Psi }^{n-1}{\Im }_j,\Psi \left({\Psi }^{n-1}{\Im }_j\right)]\hfill \\ & \le & \beta [\Gamma ({\Psi }^{n-1}{\Im }_{j-1},{\Psi }^{n-1}{\Im }_j)+\Gamma ({\Psi }^n{\Im }_{j-1},{\Psi }^n{\Im }_j)],\hfill \\ \hfill \Gamma ({\Psi }^n{\Im }_{j-1},{\Psi }^n{\Im }_j)& \le & {\displaystyle \frac{\beta }{1-\beta }}\Gamma ({\Psi }^{n-1}{\Im }_{j-1},{\Psi }^{n-1}{\Im }_j).\hfill \end{array}$$

Set ${\displaystyle \frac{\beta }{1-\beta }}=\eta \in [0,1)$; then,

$$\Gamma ({\Psi }^n{\Im }_{j-1},{\Psi }^n{\Im }_j)\le \eta d_{\mathfrak{e}}({\Psi }^{n-1}{\Im }_{j-1},{\Psi }^{n-1}{\Im }_j).$$

Continuing the same procedure, we arrive at

$$\Gamma ({\Psi }^n{\Im }_{j-1},{\Psi }^n{\Im }_j)\le {\eta }^n\Gamma ({\Im }_{j-1},{\Im }_j).$$

We need to subsequently establish that $\left\{{\Im }_n\right\}$ is a Cauchy sequence and a $\Theta -$termwise-connected sequence. Since $(\Omega ,{\Gamma }_{\Theta })$ is complete, ∀$n<m(n,m\in \mathbb{N})$, we have

$$\begin{array}{ccc}\hfill \Gamma ({\Im }_n,{\Im }_m)& \le & \alpha ({\Im }_n,{\Im }_{n+1})\Gamma ({\Im }_n,{\Im }_{n+1})+\alpha ({\Im }_{n+1},{\Im }_m)\Gamma ({\Im }_{n+1},{\Im }_m)\hfill \\ & \le & \alpha ({\Im }_n,{\Im }_{n+1})\Gamma ({\Im }_n,{\Im }_{n+1})+\alpha ({\Im }_{n+1},{\Im }_m)\alpha ({\Im }_{n+1},{\Im }_{n+2})\Gamma ({\Im }_{n+1},{\Im }_{n+2})\hfill \\ & +& \alpha ({\Im }_{n+1},{\Im }_m)\alpha ({\Im }_{n+2},{\Im }_m)\Gamma ({\Im }_{n+2},{\Im }_m)\hfill \\ & \le & \alpha ({\Im }_n,{\Im }_{n+1})\Gamma ({\Im }_n,{\Im }_{n+1})+\alpha ({\Im }_{n+1},{\Im }_m)\alpha ({\Im }_{n+1},{\Im }_{n+2})\Gamma ({\Im }_{n+1},{\Im }_{n+2})\hfill \\ & +& \alpha ({\Im }_{n+1},{\Im }_m)\alpha ({\Im }_{n+2},{\Im }_m)\alpha ({\Im }_{n+2},{\Im }_{n+3})\Gamma ({\Im }_{n+2},{\Im }_{n+3})+\alpha ({\Im }_{n+1},{\Im }_m)\hfill \\ & & \alpha ({\Im }_{n+2},{\Im }_m)\alpha ({\Im }_{n+3},{\Im }_m)\Gamma ({\Im }_{n+3},{\Im }_m)\hfill \\ & \le & \alpha ({\Im }_n,{\Im }_{n+1})\Gamma ({\Im }_n,{\Im }_{n+1})+\sum _{i=n+1}^{m-2}(\prod _{j=n+1}^i\alpha ({\Im }_j,{\Im }_m))\alpha ({\Im }_i,{\Im }_{i+1})\Gamma ({\Im }_i,{\Im }_{i+1})\hfill \\ & +& \prod _{k=n+1}^{m-1}\alpha ({\Im }_k,{\Im }_m)\Gamma ({\Im }_{m-1},{\Im }_m)\hfill \\ & \le & \alpha ({\Im }_n,{\Im }_{n+1}){\eta }^n\Gamma ({\Im }_0,{\Im }_1)+\sum _{i=n+1}^{m-2}(\prod _{j=n+1}^i\alpha ({\Im }_j,{\Im }_m))\alpha ({\Im }_i,{\Im }_{i+1}){\eta }^i\Gamma ({\Im }_0,{\Im }_1)\hfill \\ & +& \prod _{k=n+1}^{m-1}\alpha ({\Im }_k,{\Im }_m){\eta }^{m-1}\Gamma ({\Im }_0,{\Im }_1)\hfill \\ & \le & \alpha ({\Im }_n,{\Im }_{n+1}){\eta }^n\Gamma ({\Im }_0,{\Im }_1)+\sum _{i=n+1}^{m-2}(\prod _{j=n+1}^i\alpha ({\Im }_j,{\Im }_m))\alpha ({\Im }_i,{\Im }_{i+1}){\eta }^i\Gamma ({\Im }_0,{\Im }_1)\hfill \\ & +& \prod _{k=n+1}^{m-1}\alpha ({\Im }_k,{\Im }_m)\alpha ({\Im }_{m-1},{\Im }_m){\eta }^{m-1}\Gamma ({\Im }_0,{\Im }_1)\hfill \\ & =& \alpha ({\Im }_n,{\Im }_{n+1}){\eta }^n\Gamma ({\Im }_0,{\Im }_1)+\sum _{i=n+1}^{m-1}(\prod _{j=n+1}^i\alpha ({\Im }_j,{\Im }_m))\alpha ({\Im }_i,{\Im }_{i+1}){\eta }^i\Gamma ({\Im }_0,{\Im }_1)\hfill \\ & \le & \alpha ({\Im }_n,{\Im }_{n+1}){\eta }^n\Gamma ({\Im }_0,{\Im }_1)+\sum _{i=n+1}^{m-1}(\prod _{j=0}^i\alpha ({\Im }_j,{\Im }_m))\alpha ({\Im }_i,{\Im }_{i+1}){\eta }^i\Gamma ({\Im }_0,{\Im }_1).\hfill \end{array}$$

Assume that

$$S_l=\sum _{i=0}^l(\prod _{j=0}^i\alpha ({\Im }_j,{\Im }_m))\alpha ({\Im }_i,{\Im }_{i+1}){\eta }^i.$$

Therefore, we acquire

$$\Gamma ({\Im }_n,{\Im }_m)\le \Gamma ({\Im }_0,{\Im }_1)[{\eta }^n\alpha ({\Im }_n,{\Im }_{n+1})+(S_{m-1}-S_n)].$$

(3)

Making use of the ratio test, our result is

$$a_i=\prod _{j=0}^i\alpha ({\Im }_j,{\Im }_m)\alpha ({\Im }_i,{\Im }_{i+1}){\eta }^i,\mathrm{where}{\displaystyle \frac{a_{i+1}}{a_i}}<{\displaystyle \frac{1}{k}}.$$

As a result of taking a limit of $n,m\to \infty$, (3) becomes

$$\underset{n\to \infty }{lim}\Gamma ({\Im }_n,{\Im }_m)=0,$$

which demonstrates that $\left\{{\Im }_n\right\}$ is a Cauchy sequence in a $\Theta$-complete space $(\Omega ,{\Gamma }_{\Theta })$. Subsequently, $\left\{{\Im }_n\right\}$ converges in $\Omega$ and, as an assumption, there exists ${\Im }^{\prime }\in \Omega ,n_0\in \mathbb{N}$ in such a way that $({\Im }_n,{\Im }^{\prime })\in E(\Theta )$ or $({\Im }^{\prime },{\Im }_n)\in E(\Theta )$, ∀$n>n_0$

$$\underset{n\to \infty }{lim}\Gamma ({\Im }_n,{\Im }^{\prime })=0,$$

which shows that ${\Im }_n$ converges to ${\Im }^{\prime }.$ □

Definition 6.

Let $\Psi :\Omega \to \Omega$ be a selfmap on $(\Omega ,{\Gamma }_{\Theta })$. We say that the triplet $(\Omega ,\Gamma ,\Psi )$ meets the $\left(\mathrm{P}\right)$ property whenever it aligns to two limits, ${\Im }^{\prime }\in \Omega$ and ${\nu }^{\prime }\in \Psi (\Omega )$, where Picard’s sequence $\left\{{\Im }_n\right\}$ is termwise-connected; then, ${\Im }^{\prime }={\nu }^{\prime }.$

Theorem 2.

If the premises in Theorem (1) meet the criteria, and if the triplet $(\Omega ,\Gamma ,\Psi )$ is equipped with the property $\left(\mathrm{P}\right)$, then Ψ concedes a fixed point.

Proof. 

Theorem (1) ensures that with the initial point ${\Im }_0$ in Picard’s sequence, $\Psi -$ converges simultaneously to ${\Im }^{\prime }$ and $\Psi {\Im }^{\prime }.$ Given that $\Theta$ is connected, which causes ${({\Im }^{\prime }\mathrm{P}\Psi {\Im }^{\prime })}_{\Theta }\in E(\Theta )$ or ${(\Psi {\Im }^{\prime }\mathrm{P}{\Im }^{\prime })}_{\Theta }\in E(\Theta ),$ we have

$$\begin{array}{ccc}\hfill \Gamma ({\Im }^{\prime },\Psi {\Im }^{\prime })& \le & \alpha ({\Im }^{\prime },{\Im }_n)\Gamma ({\Im }^{\prime },{\Im }_n)+\alpha ({\Im }_n,\Psi {\Im }^{\prime })\Gamma ({\Im }_n,\Psi {\Im }^{\prime })\hfill \\ & =& \alpha ({\Im }^{\prime },{\Im }_n)\Gamma ({\Im }^{\prime },{\Im }_n)+\alpha ({\Im }_n,\Psi {\Im }^{\prime })\Gamma (\Psi {\Im }_{n-1},\Psi {\Im }^{\prime }),\hfill \end{array}$$

Using Equation (2), we have

$$\begin{array}{ccc}\hfill \Gamma ({\Im }^{\prime },\Psi {\Im }^{\prime })& \le & \alpha ({\Im }^{\prime },{\Im }_n)\Gamma ({\Im }^{\prime },{\Im }_n)+\alpha ({\Im }_n,\Psi {\Im }^{\prime })\beta [\Gamma ({\Im }_{n-1},\Psi {\Im }_{n-1})\hfill \\ & +& \Gamma ({\Im }^{\prime },\Psi {\Im }^{\prime })]\hfill \\ & =& \alpha ({\Im }^{\prime },{\Im }_n)\Gamma ({\Im }^{\prime },{\Im }_n)+\beta \alpha ({\Im }_n,\Psi {\Im }^{\prime })\Gamma ({\Im }_{n-1},\Psi {\Im }_{n-1})\hfill \\ & +& \beta \alpha ({\Im }_n,\Psi {\Im }^{\prime })\Gamma ({\Im }^{\prime },\Psi {\Im }^{\prime }).\hfill \end{array}$$

After a simple calculation, we obtain

$$\Gamma ({\Im }^{\prime },\Psi {\Im }^{\prime })\le \left({\displaystyle \frac{\alpha ({\Im }^{\prime },{\Im }_n)\Gamma ({\Im }^{\prime },{\Im }_n)+\beta \alpha ({\Im }_n,\Psi {\Im }^{\prime })\Gamma ({\Im }_{n-1},\Psi {\Im }_{n-1})}{1-\beta \alpha ({\Im }_n,\Psi {\Im }^{\prime })}}\right).$$

Taking $\underset{n\to \infty }{lim},$ we have

$$\Gamma ({\Im }^{\prime },\Psi {\Im }^{\prime })=0.$$

Hence, ${\Im }^{\prime }=\Psi {\Im }^{\prime }$ is a fixed point of $\Psi .$ □

Example 4.

Suppose $\Omega =[0,1]$ and define a mapping $\alpha :\Omega \times \Omega \to [1,\infty )$ using the following:

$$\alpha (\Im ,\nu )=5\Im +7\nu +9.$$

Assume that $\Gamma :\Omega \times \Omega \to [0,\infty )$ is defined as

$$\Gamma =\left\{\begin{array}{cc}{\left|\Im -\nu \right|}^2\hfill & \mathit{if}\Im \ne \nu \hfill \\ 0,\hfill & \mathit{if}\Im =\nu .\hfill \end{array}\right.$$

Clearly, $(\Omega ,{\Gamma }_{\Theta })$ is a graphically controlled metric-type space with the edge set $E(\Theta )=\Delta \cup \left\{\right(\Im ,\nu )\in [0,1]\times [0,1]:\Im \le \nu \}$ and the vertex set $\Omega =V(\Theta )$, as shown in Figure 3.

Define a mapping $\Psi :\Omega \to \Omega$ as

$$\Psi \Im =\left({\displaystyle \frac{\Im }{2\Im +3}}\right).$$

Case (i):

Taking $\Im =0$ and $\nu =1$, we conclude that

$$\begin{array}{ccc}\hfill \Gamma (\Psi 0,\Psi 1)& \le & \beta [\Gamma (0,\Psi 0)+\Gamma (1,\Psi 1\left)\right]\hfill \\ \hfill {\displaystyle \frac{1}{25}}& \le & \beta \left({\displaystyle \frac{16}{25}}\right).\hfill \end{array}$$

Case (ii):

When $\Im =0$ and $\nu ={\displaystyle \frac{1}{2}}$, we obtain

$$\begin{array}{ccc}\hfill \Gamma (\Psi 0,\Psi {\displaystyle \frac{1}{2}})& \le & \beta [\Gamma (0,\Psi 0)+\Gamma ({\displaystyle \frac{1}{2}},\Psi {\displaystyle \frac{1}{2}})]\hfill \\ \hfill {\displaystyle \frac{1}{64}}& \le & \beta \left({\displaystyle \frac{9}{64}}\right).\hfill \end{array}$$

Case (iii):

If $\Im ={\displaystyle \frac{1}{3}}$ and $\nu ={\displaystyle \frac{1}{4}}$, then

$$\begin{array}{ccc}\hfill \Gamma (\Psi {\displaystyle \frac{1}{3}},\Psi {\displaystyle \frac{1}{4}})& \le & \beta [\Gamma ({\displaystyle \frac{1}{3}},\Psi {\displaystyle \frac{1}{3}})+\Gamma ({\displaystyle \frac{1}{4}},\Psi {\displaystyle \frac{1}{4}})]\hfill \\ \hfill {\displaystyle \frac{9}{23716}}& \le & \beta \left({\displaystyle \frac{77401}{853776}}\right).\hfill \end{array}$$

Case (iv):

If $\Im ={\displaystyle \frac{1}{2}}$ and $\nu =1$, then

$$\begin{array}{ccc}\hfill \Gamma (\Psi {\displaystyle \frac{1}{2}},\Psi 1)& \le & \beta [\Gamma ({\displaystyle \frac{1}{2}},\Psi {\displaystyle \frac{1}{2}})+\Gamma (1,\Psi 1)]\hfill \\ \hfill {\displaystyle \frac{9}{1600}}& \le & \beta \left({\displaystyle \frac{1249}{1600}}\right).\hfill \end{array}$$

Consequently, all of the remaining cases are accomplished for $\beta ={\displaystyle \frac{21}{43}}\in (0,{\displaystyle \frac{1}{2}})$, also endorsed by Figure 4. By exhaustively testing the initial guesses within the interval $[0,1]$ under the Ψ mapping, we observe that all iterations converge to 0. This indicates that 0 is the sole fixed point of the Ψ mapping, as shown in Figure 5. All of the objectives for Theorem 1 are fulfilled, and Ψ concedes 0 as a fixed point.

4. Application of Second-Order Differential Equation to Fredholm Integral Equation

Let $\Omega =C\left[\right[0,1],\mathbb{R}]$ be a set of all real valued continuous functions on $[0,1]$ and $\Gamma :\Omega \times \Omega \to \mathbb{R}$ be defined as

$$\Gamma (\Im ,\nu )=\underset{\mathsf{t}\in [0,1]}{sup}{\left|\Im \left(\mathsf{t}\right)-\nu \left(\mathsf{t}\right)\right|}^2.$$

Let $\alpha :\Omega \times \Omega \to$$[1,\infty )$ be defined as

$$\alpha (\Im ,\nu )=5\Im \left(\mathsf{t}\right)+8\nu \left(\mathsf{t}\right)+5,$$

for all $\Im ,\nu \in \Omega$ and $\mathsf{t}\in [a,b].$

Moreover, let the graph $\Theta =\left(V\right(\Theta ),E(\Theta ))$ be such that $V(\Theta )=\Omega \mathrm{and}E(\Theta )=\left\{\right(\Im ,\nu )\in \Omega \times \Omega :\Im \le \nu \}.$ Clearly, $(\Omega ,{\Gamma }_{\Theta })$ is a complete graphically controlled metric-type space. Now, we will consider the following second-order differential equation to be

$$\left\{\begin{array}{c}{\Im }^{\prime \prime }\left(\mathsf{t}\right)=f(\mathsf{t},\Im \left(\mathsf{t}\right)),\hfill \\ \Im \left(0\right)={\Im }_0,\Im \left(1\right)=\Im ,\hfill \end{array}\right.$$

(4)

for all $\mathsf{t}\in [0,1]$, where $f:[0,1]\times \mathbb{R}$ is a continuous function. The problem defined in (4) is equivalent to the second-kind Fredholm integral equation

$$\Im \left(\mathsf{t}\right)=L\left(\mathsf{t}\right)+\gamma {\int }_0^1\dot{{\rm Y}}(\mathsf{t},s)\Im \left(s\right)ds,$$

(5)

where $\mathsf{t}\in [0,1]$ and $L\left(\mathsf{t}\right)=u_0+\mathsf{t}(u_1-u_0).$ In (5), $\dot{{\rm Y}}(\mathsf{t},s)$ is Green’s function, that is,

$$\dot{{\rm Y}}(\mathsf{t},s)=\left\{\begin{array}{cc}s(1-s)\hfill & 0\le s\le \mathsf{t}\hfill \\ \mathsf{t}(1-s)\hfill & \mathsf{t}\le s\le 1\hfill \end{array}\right.$$

and if $\Im \in \Omega$ is a fixed point of $\Psi$, then ℑ is a solution of (4).

Theorem 3.

Let $\Psi :\Omega \to \Omega$ be a continuous nonlinear integral operator defined by

$$\Im \left(\mathsf{t}\right)=L\left(\mathsf{t}\right)+\gamma {\int }_0^1\dot{{\rm Y}}(\mathsf{t},s)\Im \left(s\right)ds,$$

for all $\mathsf{t}\in [0,1].$ Suppose that the following conditions holds:

1.

$\alpha \in C\left[\right[0,1],\mathbb{R}]$ is a lower solution for the Problem 5, i.e.,

$$\alpha \left(\mathsf{t}\right)\le L\left(\mathsf{t}\right)+{\int }_0^1\dot{\mathsf{{\rm Y}}}(\mathsf{t},s)\alpha \left(s\right)ds,\alpha \in [0,1].$$

2.

For any $k>0,$ we have

$$\begin{array}{ccc}\hfill \underset{0\le \mathsf{t}\le 1}{inf}\dot{{\rm Y}}(\mathsf{t},s)& >& 0,\hfill \\ \hfill 0<\underset{0\le \mathsf{t}\le 1}{sup}{(\Lambda (k,s))}^2& <& {\displaystyle \frac{1}{2}},\hfill \end{array}$$

where

$$\Lambda (k,s)=({\displaystyle \frac{{\mathsf{t}}^3}{6}}-{\displaystyle \frac{{\mathsf{t}}^2}{2}}+{\displaystyle \frac{\mathsf{t}}{2}}).$$

3.

For each $\mathsf{t}\in [0,1]$ and $\Im ,\nu \in \Omega ,$ we have

$$\left|\Psi \Im \left(\mathsf{t}\right)-\Psi \nu \left(\mathsf{t}\right)\right|\le {\displaystyle \frac{1}{\gamma }}\sqrt{{\left|\Im \left(s\right)-\Psi \Im \left(s\right)\right|}^2+{\left|\nu \left(s\right)-\Psi \nu \left(s\right)\right|}^2}.$$

Then the second-order differential Equation (4) has a solution in Ω.

Proof. 

For any ${\Im }_0\in \Omega ,$ we define a sequence $\left\{{\Im }_n\right\}$ in $\Omega$ as ${\Im }_{n+1}=\Psi {\Im }_n={\Psi }^{n+1}{\Im }_0,$$n\ge 1$; then, we obtain

$${\Im }_{n+1}\left(\mathsf{t}\right)=\Psi {\Im }_n\left(\mathsf{t}\right)=L\left(\mathsf{t}\right)+\gamma {\int }_0^1\dot{{\rm Y}}(\mathsf{t},s){\Im }_n\left(s\right)ds,$$

for $\Im ,\nu \in \Omega ,$ we derive

$$\begin{array}{ccc}\hfill \left|\Psi \Im \left(\mathsf{t}\right)-\Psi \nu \left(\mathsf{t}\right)\right|& =& \left|L\left(\mathsf{t}\right)+\gamma {\int }_0^1\dot{{\rm Y}}(\mathsf{t},s)\Psi \Im \left(s\right)ds-L(\Im )+\gamma {\int }_0^1\dot{{\rm Y}}(\mathsf{t},s)\Psi \nu \left(s\right)ds\right|\hfill \\ & \le & \gamma {\int }_0^1\dot{{\rm Y}}(\mathsf{t},s)\left|\Psi \Im \left(s\right)-\Psi \nu \left(s\right)\right|ds\hfill \\ & \le & \gamma \underset{\mathsf{t}\in [0,1]}{sup}\left|\Psi \Im \left(\mathsf{t}\right)-\Psi \nu \left(\mathsf{t}\right)\right|{\int }_0^1\dot{{\rm Y}}(\mathsf{t},s)ds\hfill \\ & \le & \gamma \underset{\Im \in [0,1]}{sup}({\displaystyle \frac{{\mathsf{t}}^3}{6}}-{\displaystyle \frac{{\mathsf{t}}^2}{2}}+{\displaystyle \frac{\mathsf{t}}{2}}){\displaystyle \frac{1}{\gamma }}\sqrt{{\left|\Im \left(s\right)-\Psi \Im \left(s\right)\right|}^2+{\left|\nu \left(s\right)-\Psi \nu \left(s\right)\right|}^2},\hfill \end{array}$$

which indicates that

$$\underset{\mathsf{t}\in [0,1]}{sup}{\left|\Psi \Im \left(\mathsf{t}\right)-\Psi \nu \left(\mathsf{t}\right)\right|}^2\le \left\{\underset{0\le \mathsf{t}\le 1}{sup}{(\Lambda (k,s))}^2\right\}\underset{\mathsf{t}\in [0,1]}{sup}\sqrt{{\left|\Im \left(\mathsf{t}\right)-\Psi \Im \left(\mathsf{t}\right)\right|}^2+{\left|\nu \left(\mathsf{t}\right)-\Psi \nu \left(\mathsf{t}\right)\right|}^2},$$

and we know that

$$\left\{\underset{0\le \mathsf{t}\le 1}{sup}{(\Lambda (k,s))}^2\right\}<{\displaystyle \frac{1}{2}},$$

Thus, we infer the following:

$$\Gamma (\Psi \Im ,\Psi \nu )\le \beta [\Gamma (\Im ,\Psi \Im )+\Gamma (\nu ,\Psi \nu \left)\right],$$

where $\beta \in (0,{\displaystyle \frac{1}{2}})$. As a result, each of the prerequisites of Theorem (1) are encountered. Hence, $\Psi$ has a fixed point and Fredholm integral Equation (5) has a solution. □

5. Conclusions

In this manuscript, we established convergence results for the ${\Theta }_c-$Kannan contraction in the context of graphically controlled metric-type spaces $(\Omega ,{\Gamma }_{\Theta })$. Through the use of a detailed, nontrivial example, we demonstrated the convergence of fixed points under this contraction mapping, providing graphical evidence of its effectiveness in this extended setting. To further highlight the significance of our findings, we showed that every Kannan contraction is a graphical ${\Theta }_c-$Kannan contraction, but the converse does not hold. This distinction illustrates the broader class of mappings that emerge when extending from a Kannan contraction to a graphical ${\Theta }_c-$Kannan contraction. Similarly, we established that every controlled metric-type space $(\Omega ,\Gamma )$ is a special case of a graphically controlled metric-type space $(\Omega ,{\Gamma }_{\Theta })$, but the converse is not true, emphasizing the uniqueness and generality of graphically controlled metric-type spaces.

To demonstrate the practical utility of these theoretical results, we applied them to a nonlinear second-order boundary value problem, which was transformed into a Fredholm integral equation. This application not only highlights the power of the ${\Theta }_c-$Kannan contraction in solving complex problems, but also illustrates its role in extending fixed point theory to more general settings. Both of these results are supported by illustrative examples, which underscore the diversity and richness of our findings. These examples effectively show how these results extend existing theorems and provide new insights into fixed point theory in graphical spaces. Through these examples, we demonstrate the potential of our approach to offer new tools and solutions in this area of mathematics.

Future Direction: In this inquiry, we explore the potential applicability of Theorem 1 beyond its initial scope. Specifically, we question whether its principles and conclusions can be extended to encompass graphical bipolar metric spaces, graphical double controlled metric-like spaces, and graphical suprametric spaces. This exploration is aimed at broadening the theorem’s applicability and understanding its implications within a wider mathematical framework.