Mathematical Modeling of the Optimal Cost for the Design of Strap Combined Footings
Abstract
1. Introduction
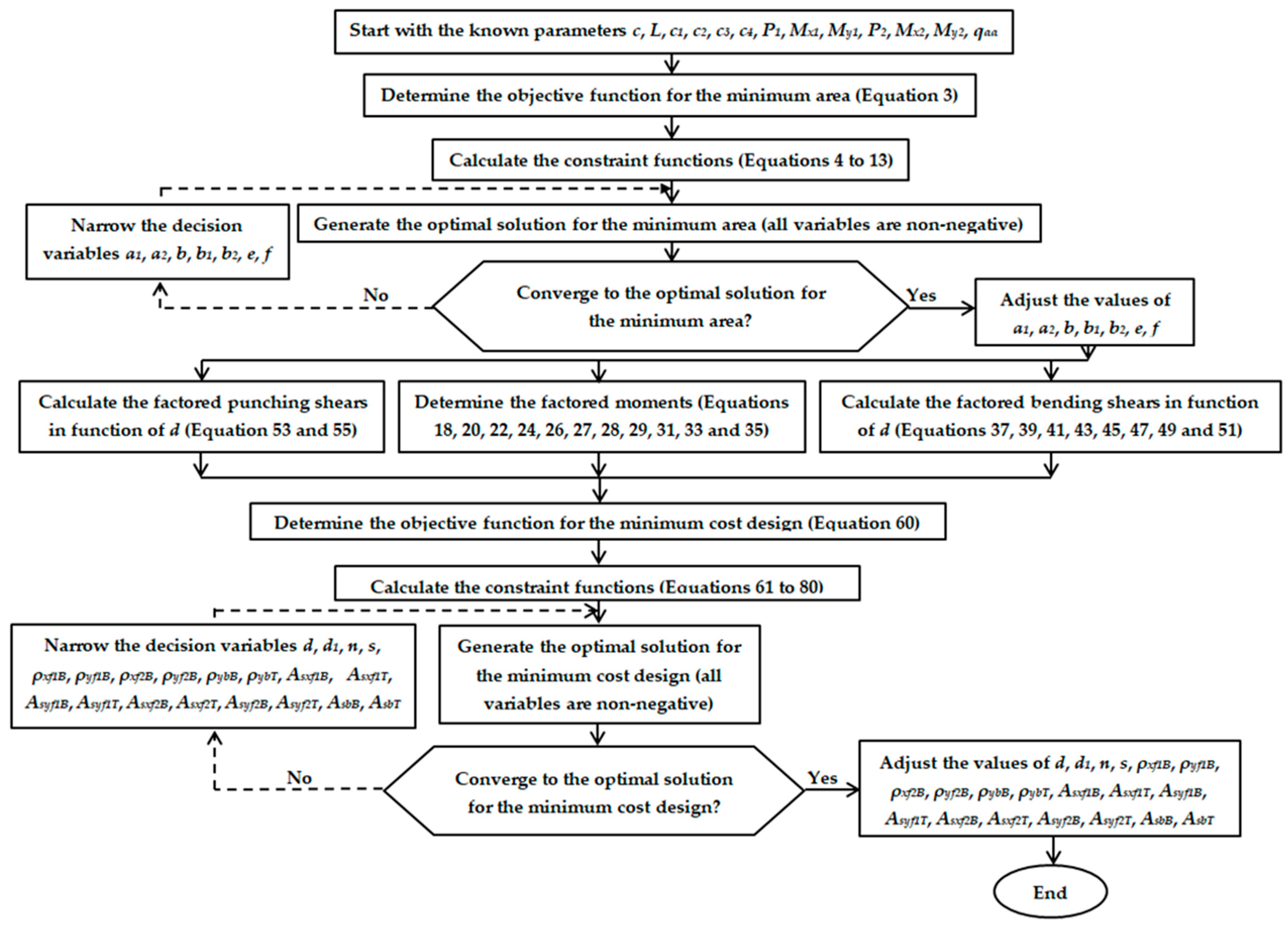
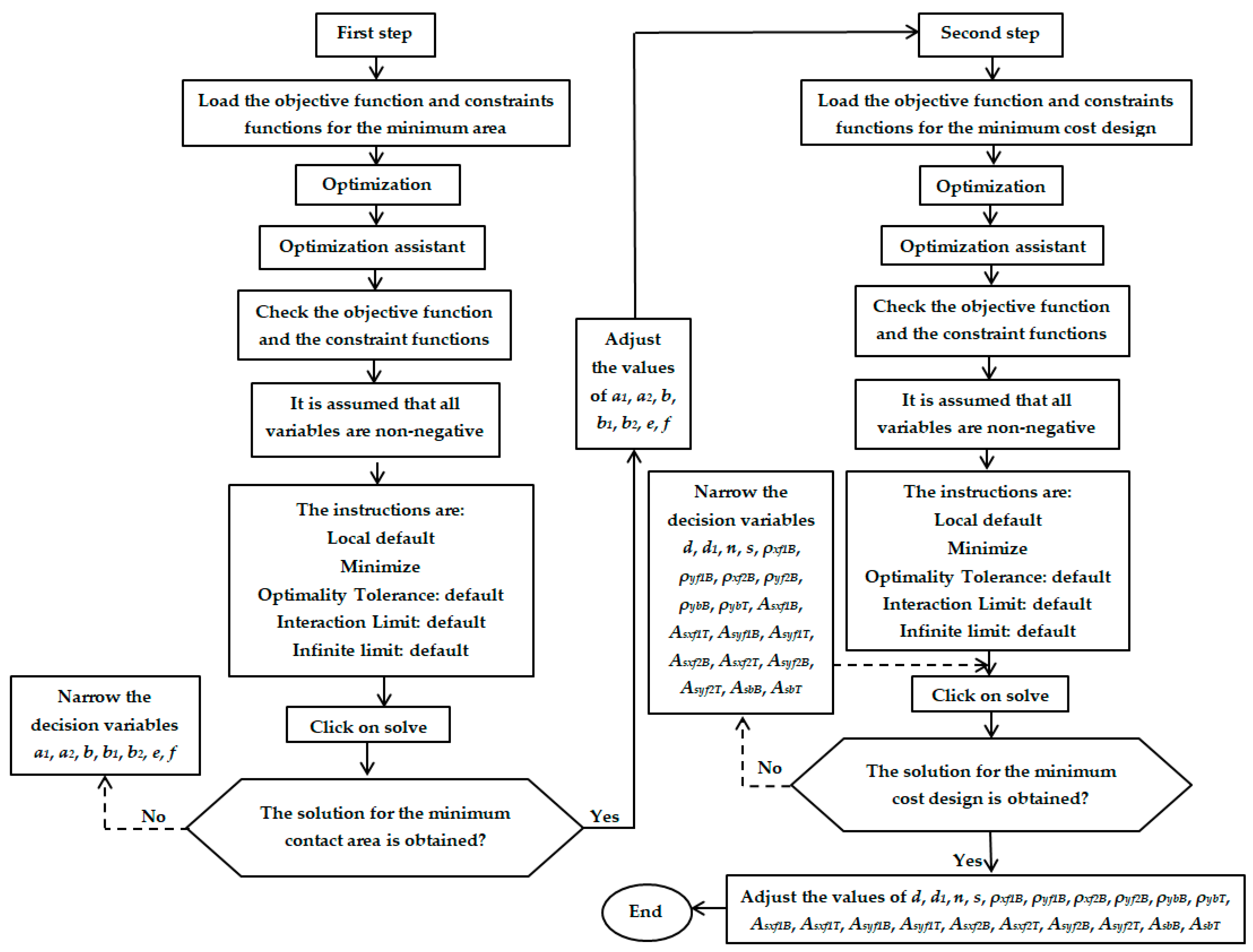
2. Materials and Methods
2.1. Minimum Contact Area on the Soil for Strap Combined Footings
2.2. Minimum-Cost Design for Strap Combined Footings
2.2.1. Moments
2.2.2. Bending Shears
2.2.3. Punching Shears
2.2.4. Objective Function for Lower Cost
2.2.5. Constraint Functions
3. Numerical Examples
4. Results
- For moments:
- If c1/2 is replaced by a1/2 in Equation (17) to find the moment about the axis formed by q1 and q3, the moment Mq1q3 = 0.
- If c1/2 is replaced by −a1/2 and the addition of Pu1 a1/2 + Muy1 into Equation (17) to obtain the moment about the axis formed by q2 and q6, the moment Mq2q6 = 0.
- If c3/2 is replaced by b1/2 in Equation (19) to find the moment about the axis formed by q7 and q11, the moment Mq7q11 = 0.
- If c3/2 is replaced by −b1/2 and the addition of Pu2b1/2 + Muy2 into Equation (19) to obtain the moment about the axis formed by q10 and q12, the moment Mq10q12 = 0.
- If c2/2 and e are eliminated from Equation (21) to obtain the moment about the axis formed by q1 and q2, the moment Mq1q2 = 0.
- If c4/2 and f are eliminated in the integrals and replaced by Pu2f and Pu2c4/2 in Equation (34) to find the moment about the axis formed by q11 and q12, the moment Mq11q12 = 0.
- For bending shears:
- If c1/2 + d is replaced by a1/2 in Equation (36) to find the bending shear about the axis formed by q1 and q3, the bending shear Vq1q3 = 0.
- If c1/2 + d is replaced by −a1/2 and the addition of −Pu1 into Equation (36) to find the bending shear about the axis formed by q2 and q6, the bending shear Vq2q6 = 0.
- If c3/2 + d is replaced by b1/2 in Equation (38) to find the bending shear about the axis formed by q7 and q11, the bending shear Vq7q11 = 0.
- If c3/2 + d is replaced by −b1/2 and the addition of −Pu2 into Equation (38) to find the bending shear about the axis formed by q10 and q12, the bending shear Vq10q12 = 0.
- If e, c2/2 and d are eliminated from Equation (40) to obtain the bending shear about the axis formed by q1 and q2, the bending shear Vq1q2 = 0.
- If c4/2, d and f are eliminated from Equation (50) to obtain the bending shear about the axis formed by q11 and q12, the bending shear Vq11q12 = 0.
- For moments and bending shears:
- If Equations (27)–(29) are developed and differentiated with respect to ym and set equal to zero, the position of the maximum moment is obtained. Now, if yt − e − c2/2 − d is replaced by ym in Equation (42), yt − b − b2 is replaced by ym in Equation (46) and yt − b + f + c4/2 + d is replaced by ym in Equation (48) and the equations are developed and set equal to zero, the position of the maximum moment is obtained. The positions of the maximum moment ym through the moment and bending shear equations are the same.
- For punching shears:
- If s1 = 0 and e = c2/2 are substituted into Equation (53), the punching shear Vup1 is obtained with footing 1 limited in the Y direction. This equation is presented in equation (63) by Yañez-Palafox [24].
- If b = c2/2 + L + b2/2, s2 = d/2 and f = b2/2 are substituted into Equation (55), the punching shear Vup2 is obtained with footing 1 limited in the Y direction and footing 2 is square and column 2 is located in the center of the footing. This equation is presented in equation (65) by Yañez-Palafox [24].
5. Conclusions
- Some engineers use trial and error to find the dimensions of strap combined footings under biaxial bending, and the design is obtained by assuming maximum and uniform pressure along the bottom of the footing.
- The equations for the moments, bending shears and punching shears are verified by equilibrium (see Section 4).
- The minimum area does not guarantee that it is the lowest cost, since the smallest area is presented in example 1 and the lowest cost appears in example 2.
- The proposed model in this paper can be used for any other building code, taking into account the equations that resist the moments, the bending shears and the punching shears, and the equations to obtain the steel areas of the footings and beams.
- This model can be used for T-shaped combined footings by substituting c with b1 and b2 with b − a2 in all equations.
- This model can be used for rectangular combined footings by substituting c with a1, b1 with a1, b2 with b and a2 with b in all equations.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Girgin, K. Simplified formulations for the determination of rotational spring constants in rigid spread footings resting on tensionless soil. JCEM 2017, 23, 464–474. [Google Scholar] [CrossRef]
- Basudhar, P.K.; Das, A.; Das, S.K.; Dey, A.; Deb, K.; De, S. Optimal cost design of rigid raft foundation. In Proceedings of the 10th East Asia-Pacific Conference on Structural Engineering and Construction (EASEC-10), Bangkok, Thailand, 3–5 August 2006; pp. 39–44. [Google Scholar]
- Algin, H.M. Stresses from linearly distributed pressures over rectangular areas. Int. J. Numer. Anal. Methods Geomech. 2000, 24, 681–692. [Google Scholar] [CrossRef]
- Algin, H.M. Practical formula for dimensioning a rectangular footing. Eng. Struct. 2007, 29, 1128–1134. [Google Scholar] [CrossRef]
- Aydogdu, I. New Iterative method to Calculate Base Stress of Footings under Biaxial Bending. Int. J. Eng. Appl. Sci. 2016, 8, 40–48. [Google Scholar] [CrossRef][Green Version]
- Highter, W.H.; Anders, J.C. Dimensioning Footings Subjected to Eccentric Loads. J. Geotech. Eng. 1985, 111, 659–665. [Google Scholar] [CrossRef]
- Vitone, D.M.A.; Valsangkar, A.J. Stresses from Loads over Rectangular Areas. J. Geotech. Eng. 1986, 112, 961–964. [Google Scholar] [CrossRef]
- Özmen, G. Determination of Base Stresses in Rectangular Footings under Biaxial Bending. Tek. Dergi Digest. 2011, 22, 1519–1535. [Google Scholar]
- Dagdeviren, U. Shear stresses below the rectangular foundations subjected to biaxial bending. Geomech. Eng. 2016, 10, 189–205. [Google Scholar] [CrossRef]
- Keskin, M.S.; Bildik, S.; Laman, M. Experimental and Numerical Studies of Vertical Stresses Beneath the Circular Footings on Sand. Appl. Sci. 2023, 13, 1635. [Google Scholar] [CrossRef]
- Shaaban, M.Q.; Al-kuaity, S.A.; Aliakbar, M.R.M. Simple function to find base pressure under triangular and trapezoidal footing with two eccentric loads. Open Eng. 2023, 13, 20220458. [Google Scholar] [CrossRef]
- Naik, B.; Sandeep Nighojkar, S.; Pendharkar, D.U. Effectiveness of Skirt in Rectangular Combined Footing for Two Symmetric Columns. IOSR-JMCE 2020, 17, 11–23. [Google Scholar]
- Aguilera-Mancilla, G.; Luévanos-Rojas, A.; López-Chavarría, S.; Medina-Elizondo, M. Modeling for the strap combined footings Part I: Optimal dimensioning. Steel Compos. Struct. 2019, 30, 97–108. [Google Scholar] [CrossRef]
- Luévanos-Rojas, A.; López-Chavarría, S.; Medina-Elizondo, M. A new model for T-shaped combined footings Part I: Optimal dimensioning. Geomech. Eng. 2018, 14, 51–60. [Google Scholar] [CrossRef]
- Babu, T.S.; Ibrahim, S.K.N. Design of circular footings considering soil-structure interaction. In Proceedings of the International Conference on Smart Materials and Structures, ICSMS-2022, Andhra Pradesh, India, 15–16 February 2022. [Google Scholar] [CrossRef]
- Fazeli-Dehkordi, P.; Karim, U.F.A. Behaviour of circular footings confined by rigid base and geocell reinforcement. Arab. J. Geosci. 2020, 13, 1100. [Google Scholar] [CrossRef]
- Magade, S.B.; Ingle, R.K. Numerical method for analysis and design of isolated square footing under concentric loading. Int. J. Adv. Struct. Eng. 2019, 11, 9–20. [Google Scholar] [CrossRef]
- Galvis, F.A.; Smith-Pardo, P.J. Axial load biaxial moment interaction (PMM) diagrams for shallow foundations: Design aids, experimental verification, and examples. Eng. Struct. 2020, 213, 110582. [Google Scholar] [CrossRef]
- Rawat, S.; Mittal, R.K.; Muthukumar, G. Isolated Rectangular Footings under Biaxial Bending: A Critical Appraisal and Simplified Analysis Methodology. Pract. Period. Struct. Des. Const. 2020, 25, 04020011. [Google Scholar] [CrossRef]
- Al-Ansari, M.S.; Afzal, M.S. Structural analysis and design of irregular shaped footings subjected to eccentric loading. Eng. Rep. 2021, 3, e12283. [Google Scholar] [CrossRef]
- Luévanos-Rojas, A. Design of boundary combined footings of trapezoidal form using a new model. Struct. Eng. Mech. 2015, 56, 745–765. [Google Scholar] [CrossRef]
- Naik, B.; Sandeep Nighojkar, S.; Pendharkar, D.U. Design of Skirted Rectangular Combined Footing With Vertical Skirt All Around The Four Edges. IJCRT 2020, 8, 674–680. [Google Scholar]
- Aishwarya, K.M.; Balaji, N.C. Analysis and design of eccentrically loaded corner combined footing for rectangular columns. In Proceedings of the International Conference on Advances in Sustainable Construction Materials, Guntur, India, 18–19 March 2022. [Google Scholar] [CrossRef]
- Yáñez-Palafox, J.A.; Luévanos-Rojas, A.; López-Chavarría, S.; Medina-Elizondo, M. Modeling for the strap combined footings Part II: Mathematical model for design. Steel Compos. Struct. 2019, 30, 109–121. [Google Scholar] [CrossRef]
- Luévanos-Rojas, A.; López-Chavarría, S.; Medina-Elizondo, M. A new model for T-shaped combined footings Part II: Mathematical model for design. Geomech. Eng. 2018, 14, 61–69. [Google Scholar] [CrossRef]
- Camp, C.V.; Assadollahi, A. CO2 and cost optimization of reinforced concrete footings using a hybrid big bang-big crunch algorithm. Struct. Multidiscip. Optim. 2013, 48, 411–426. [Google Scholar] [CrossRef]
- Juang, C.H.; Wang, L. Reliability-Based Robust Geotechnical Design of Spread Foundations Using Multi-Objective Genetic Algorithm. Comput. Geotech. 2013, 48, 96–106. [Google Scholar] [CrossRef]
- Khajehzadeh, M.; Taha, M.R.; Eslami, M. Multi-objective optimization of foundation using global-local gravitational search algorithm. Struct. Eng. Mech. 2014, 50, 257–273. [Google Scholar] [CrossRef]
- Jelušič, P.; Žlender, B. Optimal design of pad footing based on MINLP optimization. Soils Found. 2018, 58, 277–289. [Google Scholar] [CrossRef]
- Chaudhuri, P.; Maity, D. Cost optimization of rectangular RC footing using GA and UPSO. Soft Comput. 2020, 24, 709–721. [Google Scholar] [CrossRef]
- Solorzano, G.; Plevris, V. Optimum design of RC footings with genetic algorithms according to ACI 318-19. Buildings 2020, 10, 110. [Google Scholar] [CrossRef]
- Nawaz, M.N.; Ali, A.S.; Jaffar, S.T.A.; Jafri, T.H.; Oh, T.-M.; Abdallah, M.; Karam, S.; Azab, M. Cost-Based Optimization of Isolated Footing in Cohesive Soils Using Generalized Reduced Gradient Method. Buildings 2022, 12, 1646. [Google Scholar] [CrossRef]
- Al-Gahtani, H.J.; Adekunle, S.K. A boundary-type approach for the computation of vertical stresses in soil due to arbitrarily shaped foundations. World J. Eng. 2019, 16, 419–426. [Google Scholar] [CrossRef]
- Nigdeli, S.M.; Bekdaş, G.; Yang, X.-S. Metaheuristic Optimization of Reinforced Concrete Footings. KSCE J. Civ. Eng. 2018, 22, 4555–4563. [Google Scholar] [CrossRef]
- Waheed, J.; Azam, R.; Riaz, M.R.; Shakeel, M.; Mohamed, A.; Ali, E. Metaheuristic-Based Practical Tool for Optimal Design of Reinforced Concrete Isolated Footings: Development and Application for Parametric Investigation. Buildings 2022, 12, 471. [Google Scholar] [CrossRef]
- Rawat, S.; Mittal, R.K. Optimization of eccentrically loaded reinforced-concrete isolated footings. Pract. Period. Struct. Des. Constr. 2018, 23, 06018002. [Google Scholar] [CrossRef]
- Nigdeli, S.M.; Bekdas, G. The Investigation of Optimization of Eccentricity in Reinforced Concrete Footings. In Proceedings of 7th International Conference on Harmony Search. Soft Comput. Appl. 2022, 140, 207–215. [Google Scholar]
- Luévanos-Rojas, A. Minimum Cost Design for Rectangular Isolated Footings Taking into Account That the Column Is Located in Any Part of the Footing. Buildings 2023, 13, 2269. [Google Scholar] [CrossRef]
- Basudhar, P.K.; Dey, A.; Mondal, A.S. Optimal Cost-Analysis and Design of Circular Footings. Int. J. Eng. Technol. Innov. 2012, 2, 243–264. [Google Scholar]
- Rizwan, M.; Alam, B.; Rehman, F.U.; Masud, N.; Shahzada, K.; Masud, T. Cost Optimization of Combined Footings Using Modified Complex Method of Box. Int. J. Adv. Struct. Geotech. Eng. 2012, 1, 24–28. [Google Scholar]
- Kashani, A.R.; Camp, C.V.; Akhani, M.; Ebrahimi, S. Optimum design of combined footings using swarm intelligence-based algorithms. Adv. Eng. Soft. 2022, 169, 103140. [Google Scholar] [CrossRef]
- Al-Douri, E.M.F. Optimum design of trapezoidal combined footings. Tikrit J. Eng. Sci. 2007, 14, 85–115. [Google Scholar] [CrossRef]
- ACI 318-14; Building Code Requirements for Structural Concrete and Commentary. American Concrete Institute: Farmington Hills, MI, USA, 2014.
- López-Chavarría, S.; Luévanos-Rojas, A.; Medina-Elizondo, M.; Sandoval-Rivas, R.; Velázquez-Santillán, F. Optimal design for the reinforced concrete circular isolated footings. Adv. Comput. Des. 2019, 4, 273–294. [Google Scholar] [CrossRef]
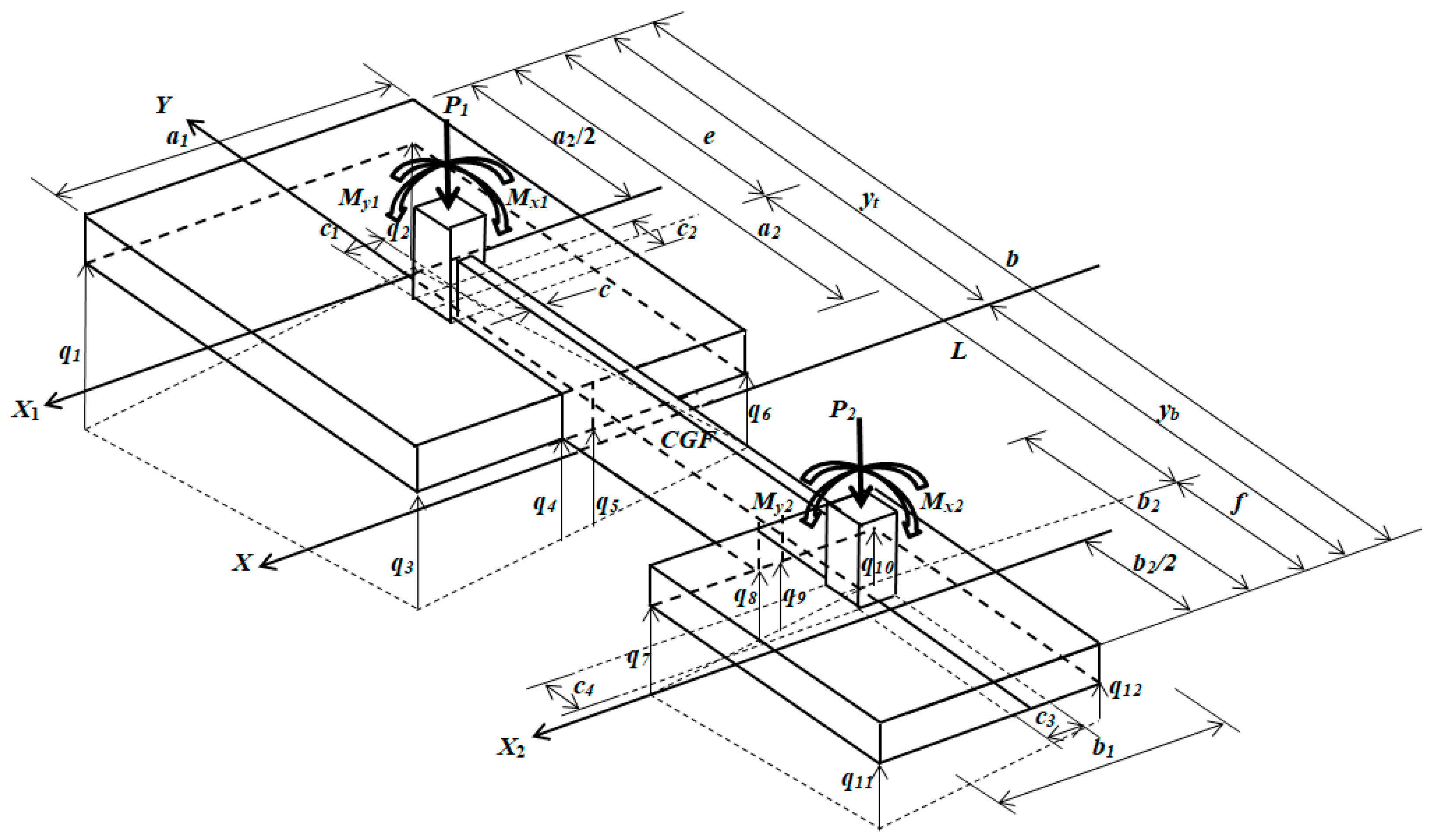
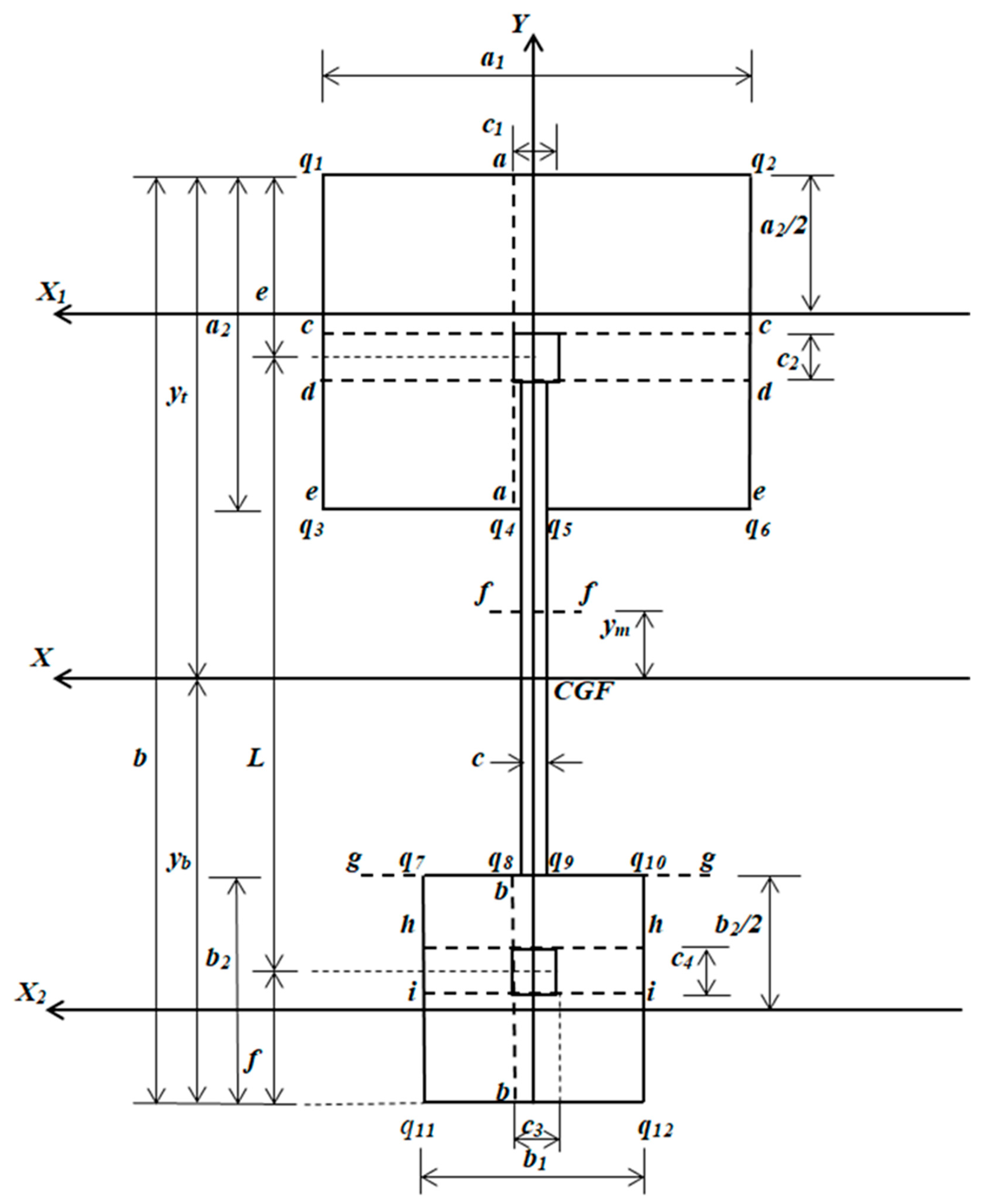

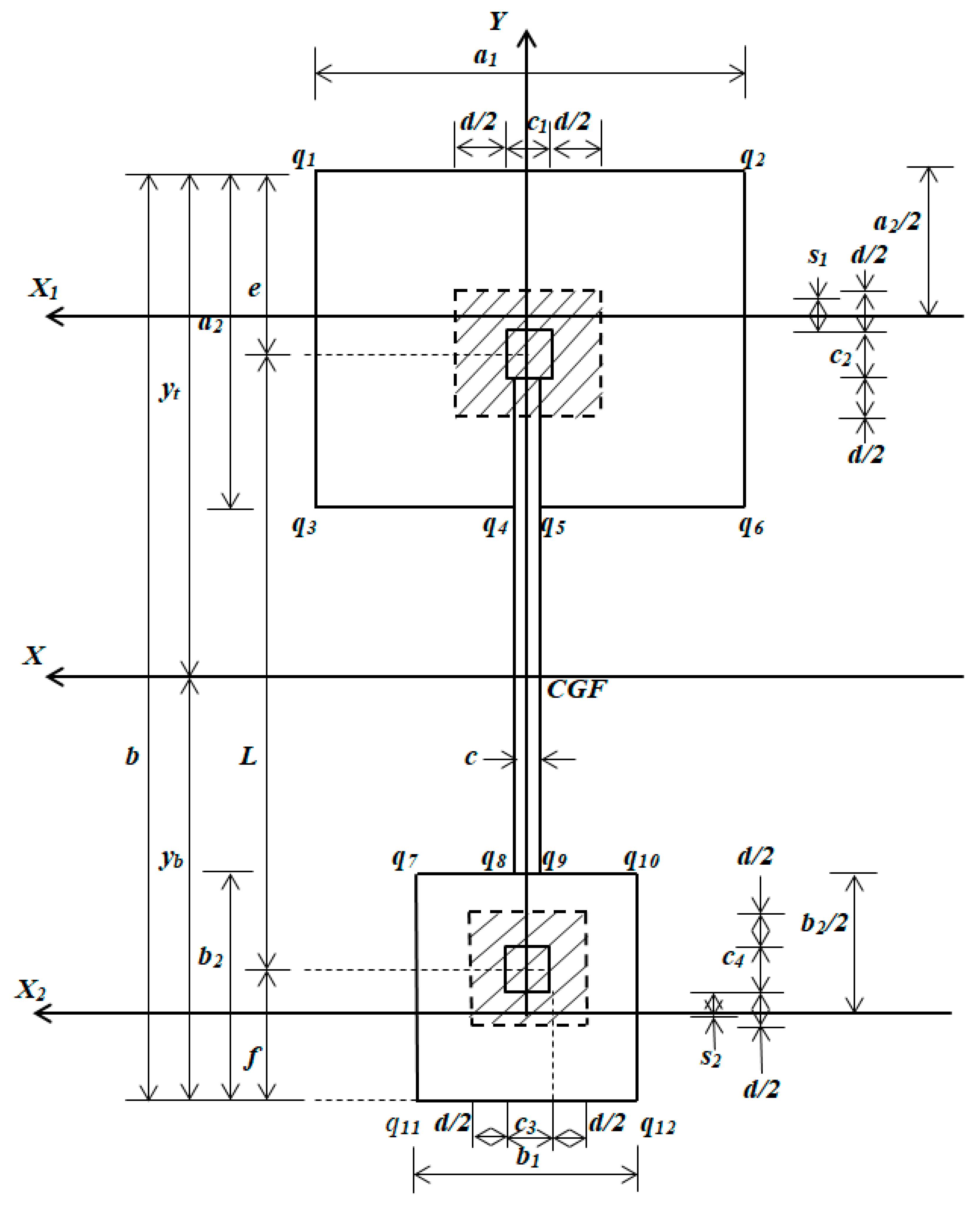
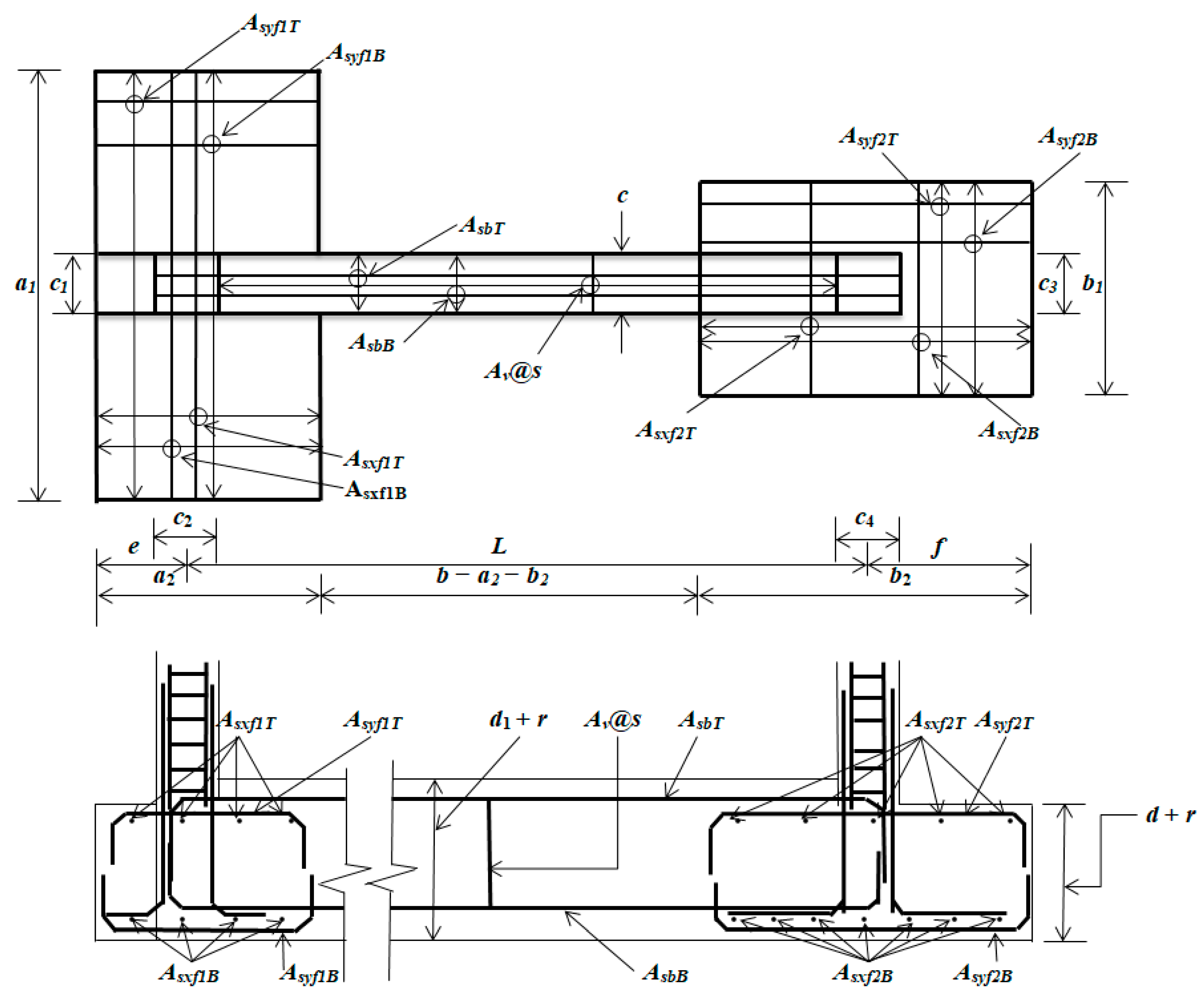
| Pressures below Footing | q1 | q2 | q3 | q4 | q5 | q6 | q7 | q8 | q9 | q10 | q11 | q12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Coordinates | x1 | x2 | x3 | x4 | x5 | x6 | x7 | x8 | x9 | x10 | x11 | x12 |
| a1/2 | −a1/2 | a1/2 | c/2 | −c/2 | −a1/2 | b1/2 | c/2 | −c/2 | −b1/2 | b1/2 | −b1/2 | |
| y1 | y2 | y3 | y4 | y5 | y6 | y7 | y8 | y9 | y10 | y11 | y12 | |
| yt | yt | yt − a2 | yt − a2 | yt − a2 | yt − a2 | b2− yb | b2− yb | b2− yb | b2− yb | −yb | −yb |
| Pressures below Footing 1 | q1 | q2 | q3 | q6 |
|---|---|---|---|---|
| Coordinates | x1 | x2 | x3 | x6 |
| a1/2 | −a1/2 | a1/2 | −a1/2 | |
| y1 | y2 | y3 | y6 | |
| a2/2 | a2/2 | −a2/2 | −a2/2 |
| Pressures below Footing 2 | q7 | q10 | q11 | q12 |
|---|---|---|---|---|
| Coordinates | x7 | x10 | x11 | x12 |
| b1/2 | −b1/2 | b1/2 | −b1/2 | |
| y7 | y10 | y11 | y12 | |
| b2/2 | b2/2 | −b2/2 | −b2/2 |
| Concept | Free Ends | Equal Sides for the Footings 1 and 2 | Equal Sides for the Footing 1 | Equal Sides for the Footing 2 | ||||
|---|---|---|---|---|---|---|---|---|
| Theoretical | Practical | Theoretical | Practical | Theoretical | Practical | Theoretical | Practical | |
| Ix (m4) | 139.75 | 143.19 | 179.49 | 189.20 | 150.79 | 154.13 | 116.58 | 120.65 |
| Iy (m4) | 44.03 | 44.54 | 9.94 | 11.09 | 19.49 | 19.89 | 39.41 | 40.66 |
| MxT (kN-m) | −623.79 | −553.30 | −572.81 | −611.62 | 753.88 | 611.46 | −585.50 | −475.07 |
| MyT (kN-m) | 480 | 480 | 480 | 480 | 480 | 480 | 480 | 480 |
| R (kN) | 2300 | 2300 | 2300 | 2300 | 2300 | 2300 | 2300 | 2300 |
| a1 (m) | 8.07 | 8.10 | 3.11 | 3.20 | 2.64 | 2.70 | 7.72 | 7.80 |
| a2 (m) | 1.00 | 1.00 | 3.11 | 3.20 | 2.64 | 2.70 | 1.00 | 1.00 |
| b (m) | 9.63 | 9.70 | 9.68 | 9.75 | 8.52 | 8.55 | 8.12 | 8.15 |
| b1 (m) | 1.00 | 1.00 | 2.24 | 2.30 | 5.70 | 5.70 | 1.85 | 1.90 |
| b2 (m) | 2.63 | 2.70 | 2.24 | 2.30 | 1.00 | 1.00 | 1.85 | 1.90 |
| c (m) | 0.40 | 0.40 | 0.40 | 0.40 | 0.40 | 0.40 | 0.40 | 0.40 |
| e (m) | 0.20 | 0.20 | 1.56 | 1.60 | 1.32 | 1.35 | 0.20 | 0.20 |
| f (m) | 2.43 | 2.50 | 1.12 | 1.15 | 0.20 | 0.20 | 0.92 | 0.95 |
| yt (m) | 2.71 | 2.74 | 4.09 | 4.12 | 4.43 | 4.40 | 2.73 | 2.78 |
| σ1 (kN/m2) | 207.44 | 207.29 | 201.97 | 189.41 | 211.90 | 204.09 | 206.88 | 205.35 |
| σ2 (kN/m2) | 119.48 | 120.00 | 51.93 | 50.95 | 146.83 | 138.92 | 112.79 | 113.27 |
| σ3 (kN/m2) | 211.90 | 211.15 | 211.90 | 199.76 | 198.69 | 193.37 | 211.90 | 209.29 |
| σ4 (kN/m2) | 170.10 | 169.67 | 146.44 | 139.18 | 171.08 | 165.62 | 167.29 | 165.61 |
| σ5 (kN/m2) | 165.74 | 165.36 | 127.12 | 121.87 | 161.23 | 155.96 | 162.42 | 160.89 |
| σ6 (kN/m2) | 123.94 | 123.87 | 61.66 | 61.29 | 133.62 | 128.21 | 117.82 | 117.21 |
| σ7 (kN/m2) | 200.15 | 196.08 | 204.74 | 194.03 | 211.90 | 210.34 | 202.61 | 195.14 |
| σ8 (kN/m2) | 196.88 | 192.85 | 160.24 | 152.92 | 146.69 | 146.38 | 193.79 | 186.28 |
| σ9 (kN/m2) | 192.52 | 188.54 | 140.92 | 135.61 | 136.84 | 136.72 | 188.92 | 181.56 |
| σ10 (kN/m2) | 189.25 | 185.31 | 96.41 | 94.50 | 71.63 | 72.76 | 180.09 | 211.90 |
| σ11 (kN/m2) | 211.90 | 206.52 | 211.90 | 201.46 | 206.90 | 206.37 | 211.90 | 202.62 |
| σ12 (kN/m2) | 201.00 | 195.74 | 103.57 | 101.94 | 66.93 | 68.79 | 189.38 | 180.19 |
| Amin (m2) | 13.10 | 13.20 | 16.44 | 17.23 | 14.63 | 14.93 | 13.25 | 13.51 |
| Concept | Free ends | Equal Sides for the Footings 1 and 2 | Equal Sides for the Footing 1 | Equal Sides for the Footing 2 | ||||
|---|---|---|---|---|---|---|---|---|
| Theoretical | Practical | Theoretical | Practical | Theoretical | Practical | Theoretical | Practical | |
| d (cm) | 90.92 | 92.00 | 39.57 | 42.00 | 41.86 | 42.00 | 90.17 | 92.00 |
| d1 (cm) | 141.90 | 142.00 | 63.56 | 67.00 | 67.62 | 72.00 | 97.31 | 102.00 |
| n | 21.24 | 22 | 23.28 | 24 | 21.89 | 21 | 21.15 | 22 |
| s (cm) | 34.83 | 34 | 31.78 | 31 | 33.81 | 36.00 | 34.99 | 35 |
| AsbB (cm2) | 20.63 | 25.35 (5Ø1”) | 8.47 | 10.14 (2Ø1”) | 9.01 | 10.14 (2Ø1”) | 12.96 | 15.21 (3Ø1”) |
| AsbT (cm2) | 18.90 | 20.28 (4Ø1”) | 25.40 | 30.42 (6Ø1”) | 30.47 | 35.49 (7Ø1”) | 30.92 | 35.49 (7Ø1”) |
| Asxf1B (cm2) | 54.13 | 55.77 (11Ø1”) | 46.46 | 50.70 (10Ø1”) | 37.64 | 40.56 (8Ø1”) | 52.46 | 55.77 (11Ø1”) |
| Asxf1T (cm2) | 17.81 | 20.28 (4Ø1”) | 27.40 | 30.42 (6Ø1”) | 24.23 | 25.35 (5Ø1”) | 17.67 | 20.28 (4Ø1”) |
| Asxf2B (cm2) | 81.74 | 86.19 (17Ø1”) | 30.31 | 35.49 (7Ø1”) | 73.70 | 76.05 (15Ø1”) | 57.05 | 60.84 (12Ø1”) |
| Asxf2T (cm2) | 48.07 | 50.70 (10Ø1”) | 19.70 | 25.35 (5Ø1”) | 8.98 | 10.14 (2Ø1”) | 33.57 | 35.49 (7Ø1”) |
| Asyf1B (cm2) | 245.23 | 248.43 (49Ø1”) | 42.17 | 45.63 (9Ø1”) | 37.64 | 40.56 (8Ø1”) | 243.21 | 243.36 (48Ø1”) |
| Asyf1T (cm2) | 144.22 | 147.03 (29Ø1”) | 27.40 | 30.42 (6Ø1”) | 24.23 | 25.35 (5Ø1”) | 137.83 | 141.96 (28Ø1”) |
| Asyf2B (cm2) | 32.17 | 35.49 (13Ø1”) | 34.93 | 35.49 (7Ø1”) | 79.46 | 81.12 (16Ø1”) | 57.05 | 60.84 (12Ø1”) |
| Asyf2T (cm2) | 17.81 | 20.28 (4Ø1”) | 19.70 | 25.35 (5Ø1”) | 51.16 | 55.77 (11Ø1”) | 33.57 | 35.49 (7Ø1”) |
| Asv (cm2) | 1.42 | 1.42 (2Ø3/8”) | 1.42 | 1.42 (2Ø3/8”) | 1.42 | 1.42 (2Ø3/8”) | 1.42 | 1.42 (2Ø3/8”) |
| ρxf1B | 0.00595 | 0.00606 | 0.00367 | 0.00377 | 0.00333 | 0.00358 | 0.00582 | 0.00606 |
| ρxf2B | 0.00333 | 0.00347 | 0.00333 | 0.00367 | 0.01761 | 0.01811 | 0.00333 | 0.00348 |
| ρybB | 0.00363 | 0.00446 | 0.00333 | 0.00378 | 0.00333 | 0.00352 | 0.00333 | 0.00373 |
| ρybT | 0.00333 | 0.00357 | 0.00999 | 0.01135 | 0.01126 | 0.01232 | 0.00794 | 0.00870 |
| ρyf1B | 0.00333 | 0.00333 | 0.00333 | 0.00340 | 0.00333 | 0.00358 | 0.00333 | 0.00339 |
| ρyf2B | 0.00354 | 0.00386 | 0.00384 | 0.00367 | 0.00333 | 0.00339 | 0.00333 | 0.00348 |
| Cmin | 28.79Cc | 29.89Cc | 17.60Cc | 19.26Cc | 19.36Cc | 20.35Cc | 27.98Cc | 29.53Cc |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luévanos-Rojas, A.; Santiago-Hurtado, G.; Moreno-Landeros, V.M.; Olguin-Coca, F.J.; López-León, L.D.; Diaz-Gurrola, E.R. Mathematical Modeling of the Optimal Cost for the Design of Strap Combined Footings. Mathematics 2024, 12, 294. https://doi.org/10.3390/math12020294
Luévanos-Rojas A, Santiago-Hurtado G, Moreno-Landeros VM, Olguin-Coca FJ, López-León LD, Diaz-Gurrola ER. Mathematical Modeling of the Optimal Cost for the Design of Strap Combined Footings. Mathematics. 2024; 12(2):294. https://doi.org/10.3390/math12020294
Chicago/Turabian StyleLuévanos-Rojas, Arnulfo, Griselda Santiago-Hurtado, Victor Manuel Moreno-Landeros, Francisco Javier Olguin-Coca, Luis Daimir López-León, and Eyran Roberto Diaz-Gurrola. 2024. "Mathematical Modeling of the Optimal Cost for the Design of Strap Combined Footings" Mathematics 12, no. 2: 294. https://doi.org/10.3390/math12020294
APA StyleLuévanos-Rojas, A., Santiago-Hurtado, G., Moreno-Landeros, V. M., Olguin-Coca, F. J., López-León, L. D., & Diaz-Gurrola, E. R. (2024). Mathematical Modeling of the Optimal Cost for the Design of Strap Combined Footings. Mathematics, 12(2), 294. https://doi.org/10.3390/math12020294






