A Non-Traditional Finite Element Method for Scattering by Partly Covered Grooves with Multiple Media
Abstract
1. Introduction
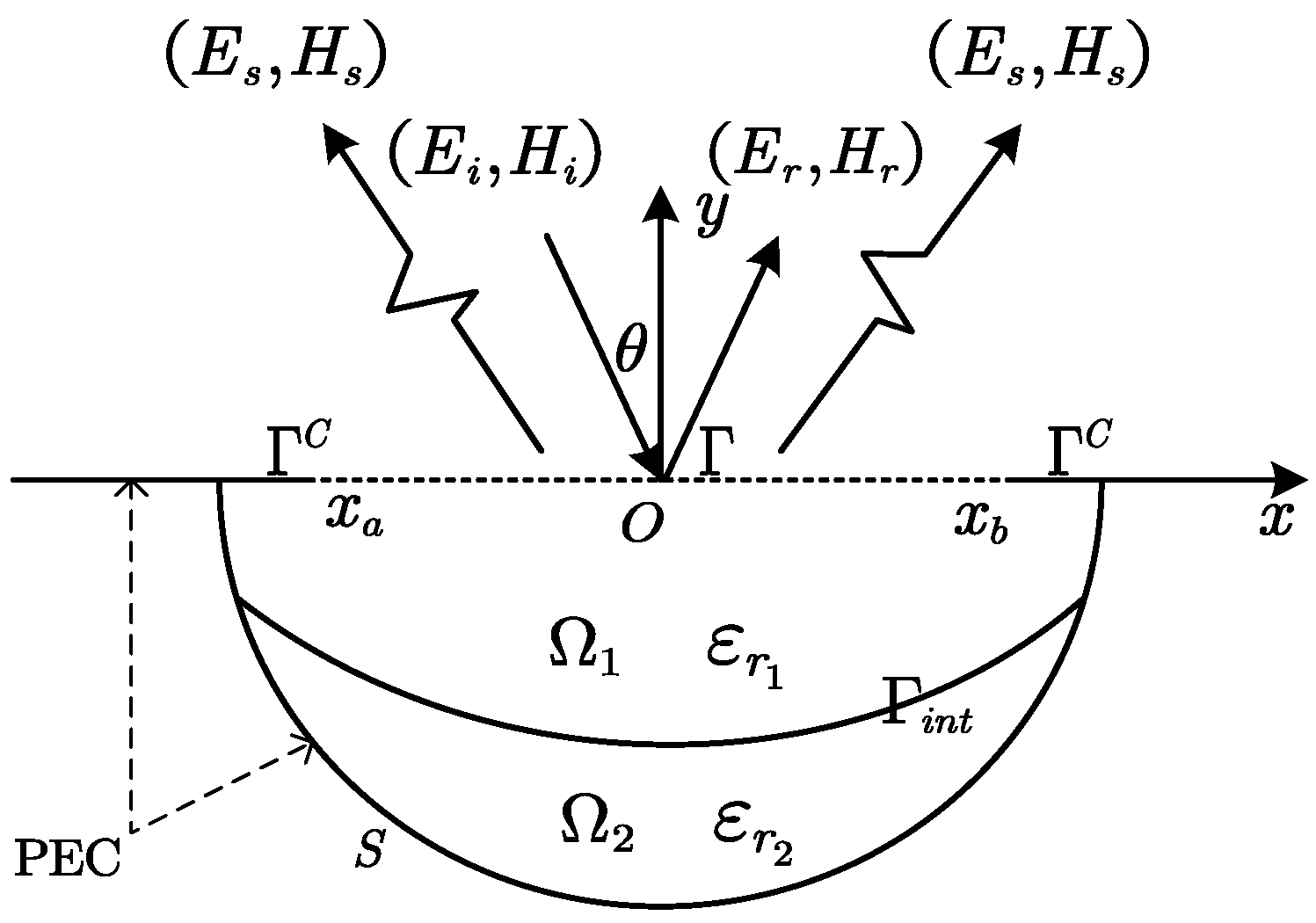
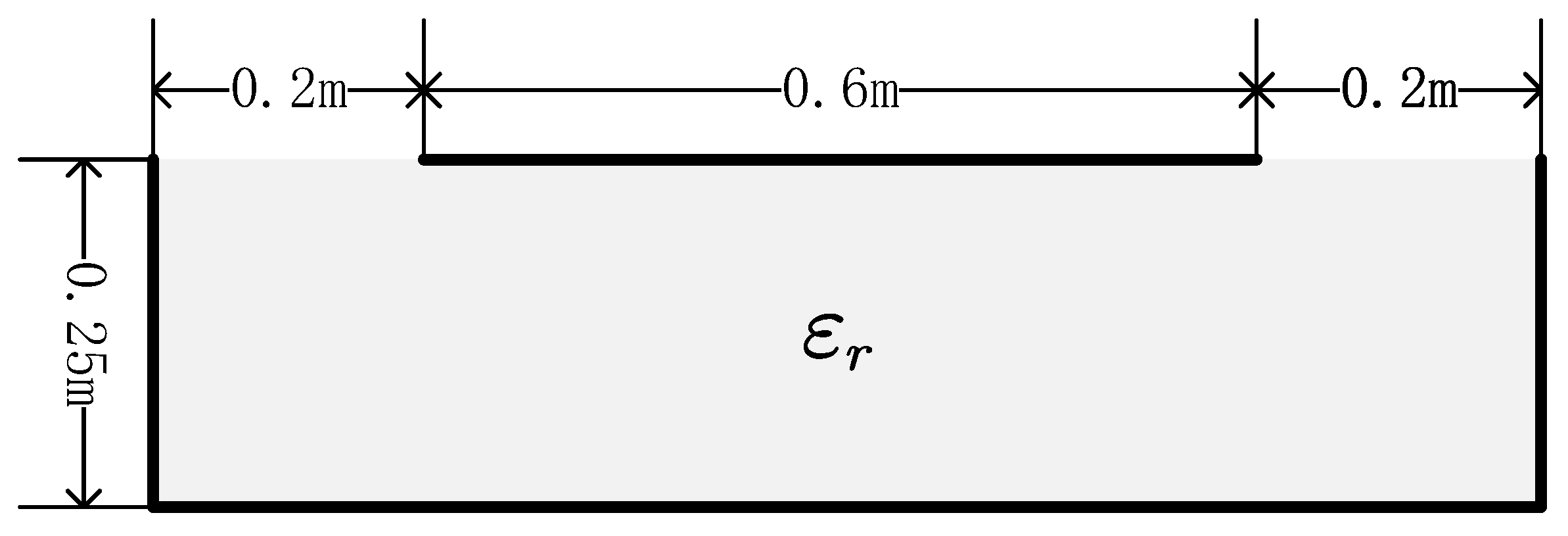
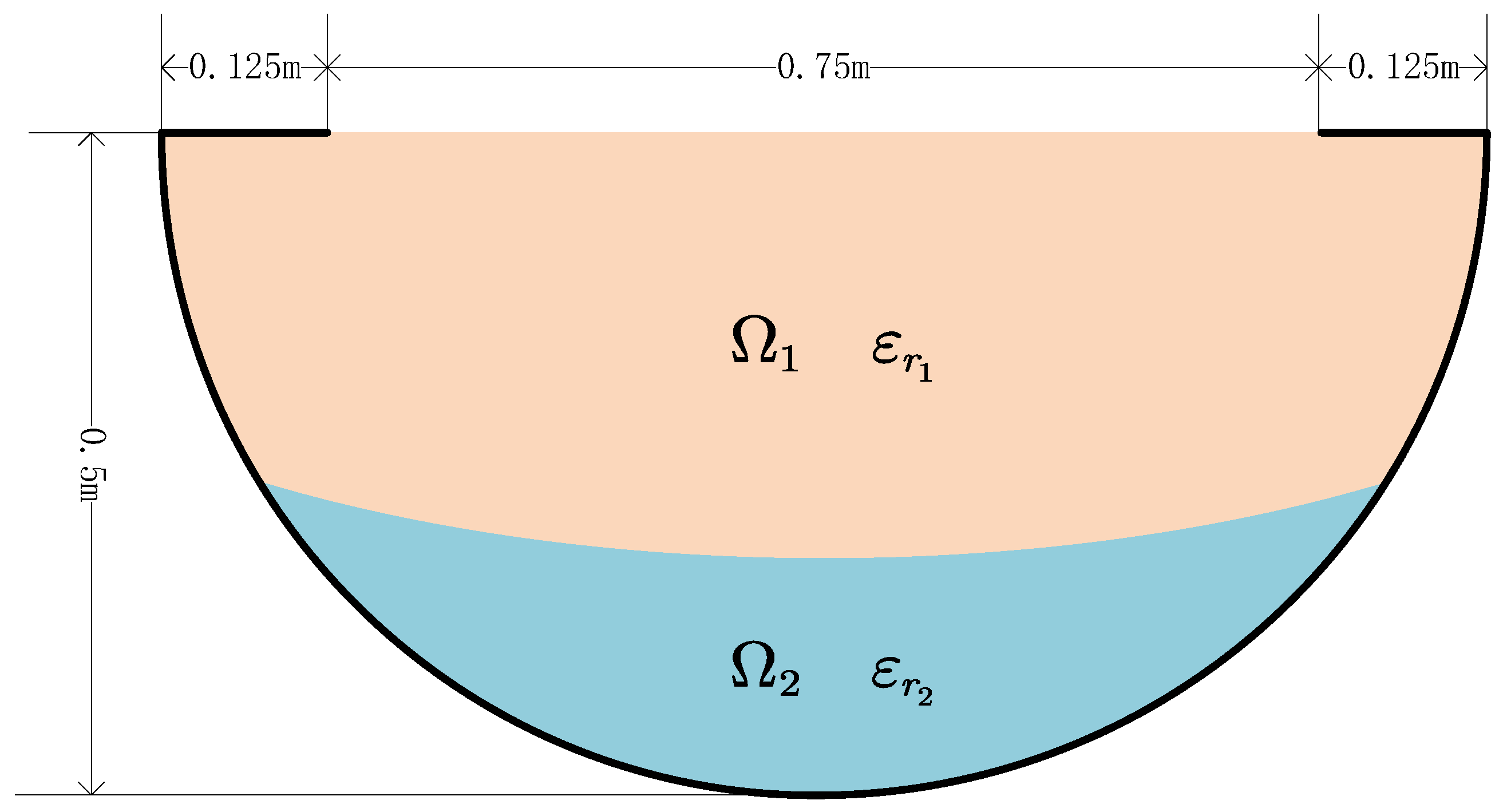
2. The Mathematical Model of a Partly Covered Groove
3. Weak Form of the Scattering Problem
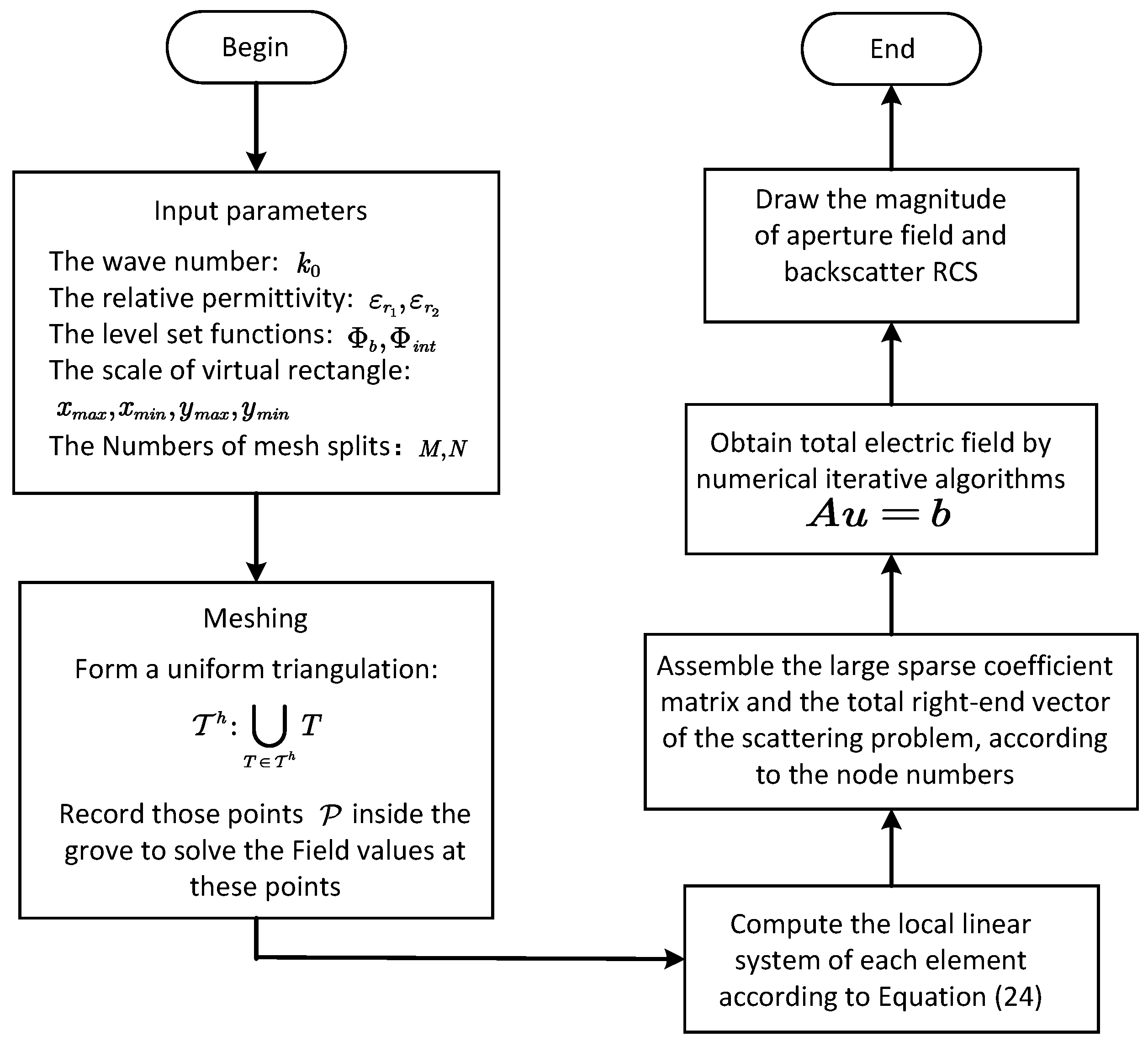
4. Non-Traditional Finite Element Method
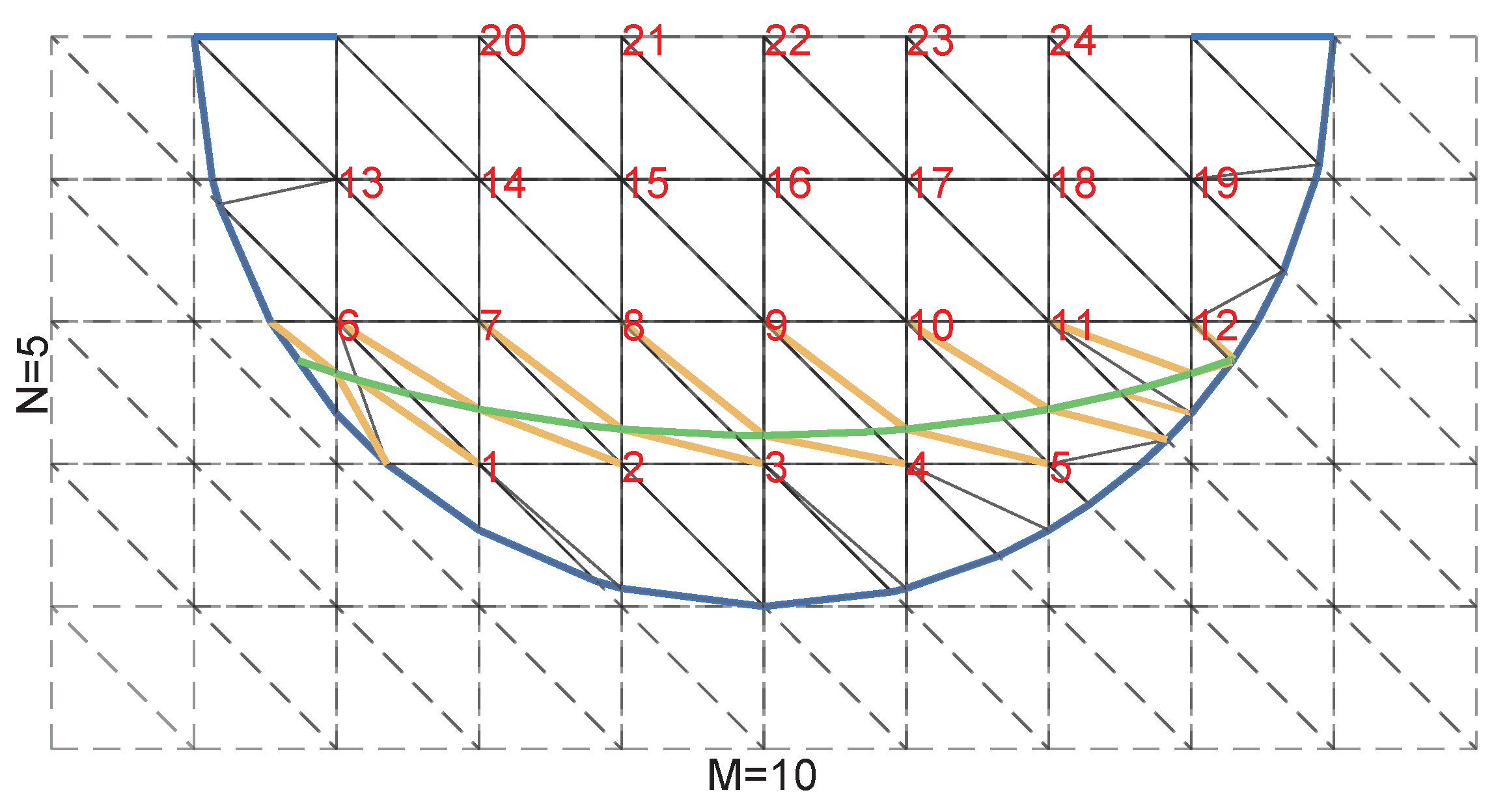
4.1. Non-Body-Fitted Mesh Generation
4.2. The Processing of Intersecting Elements with Interface
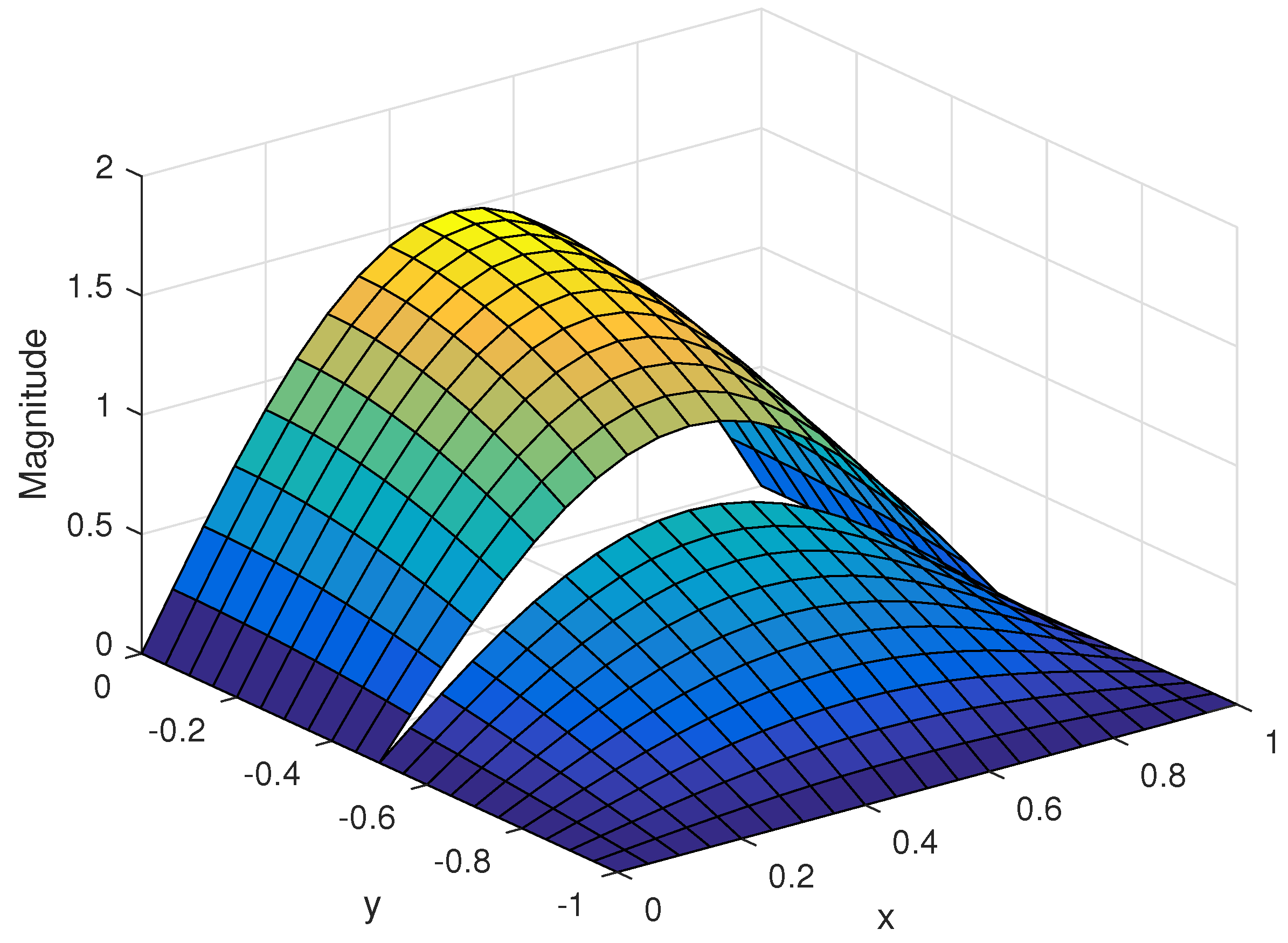
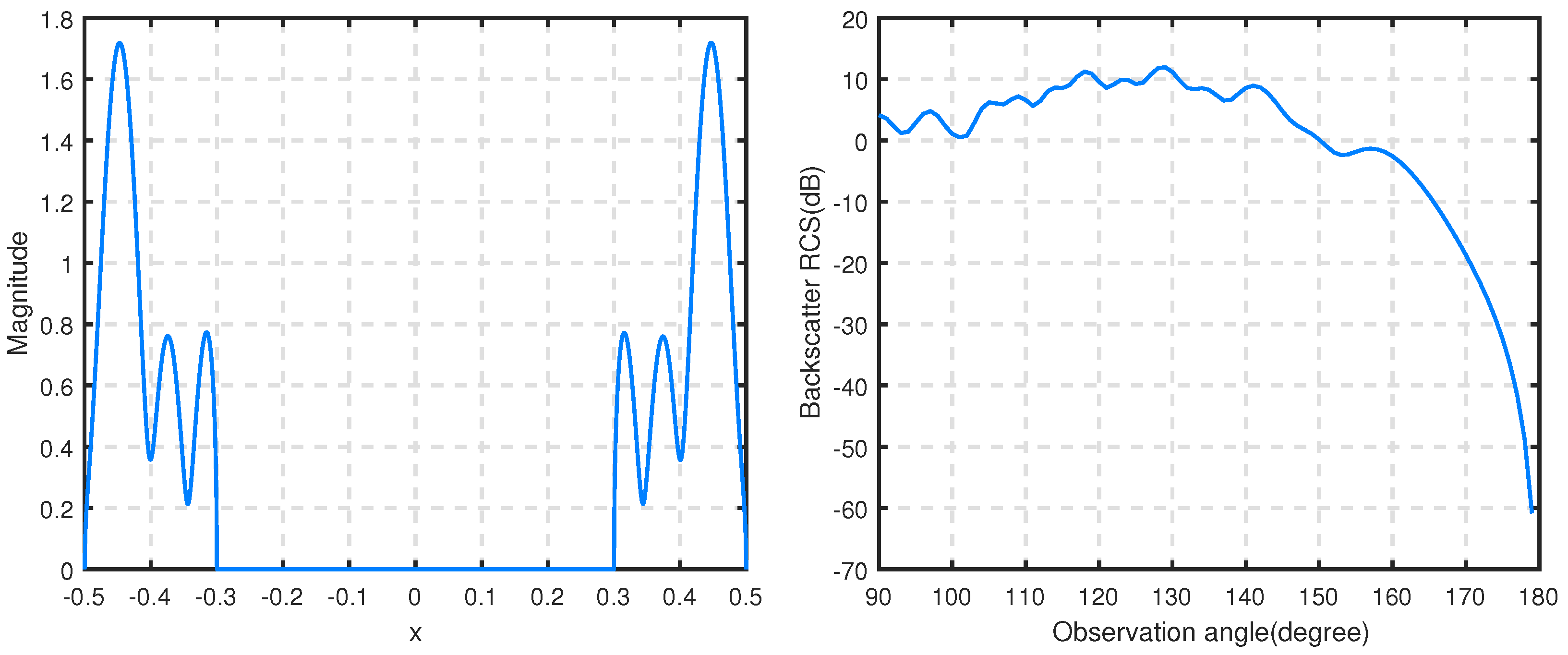
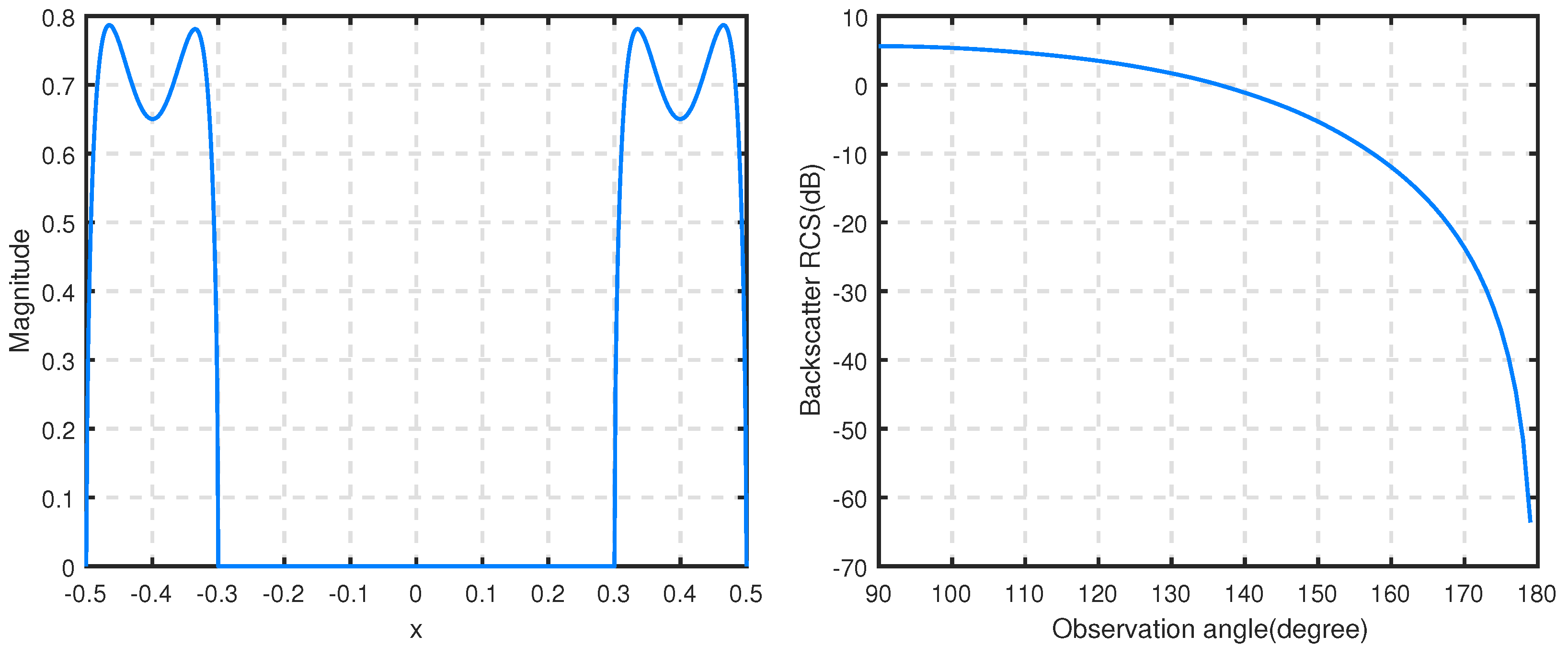
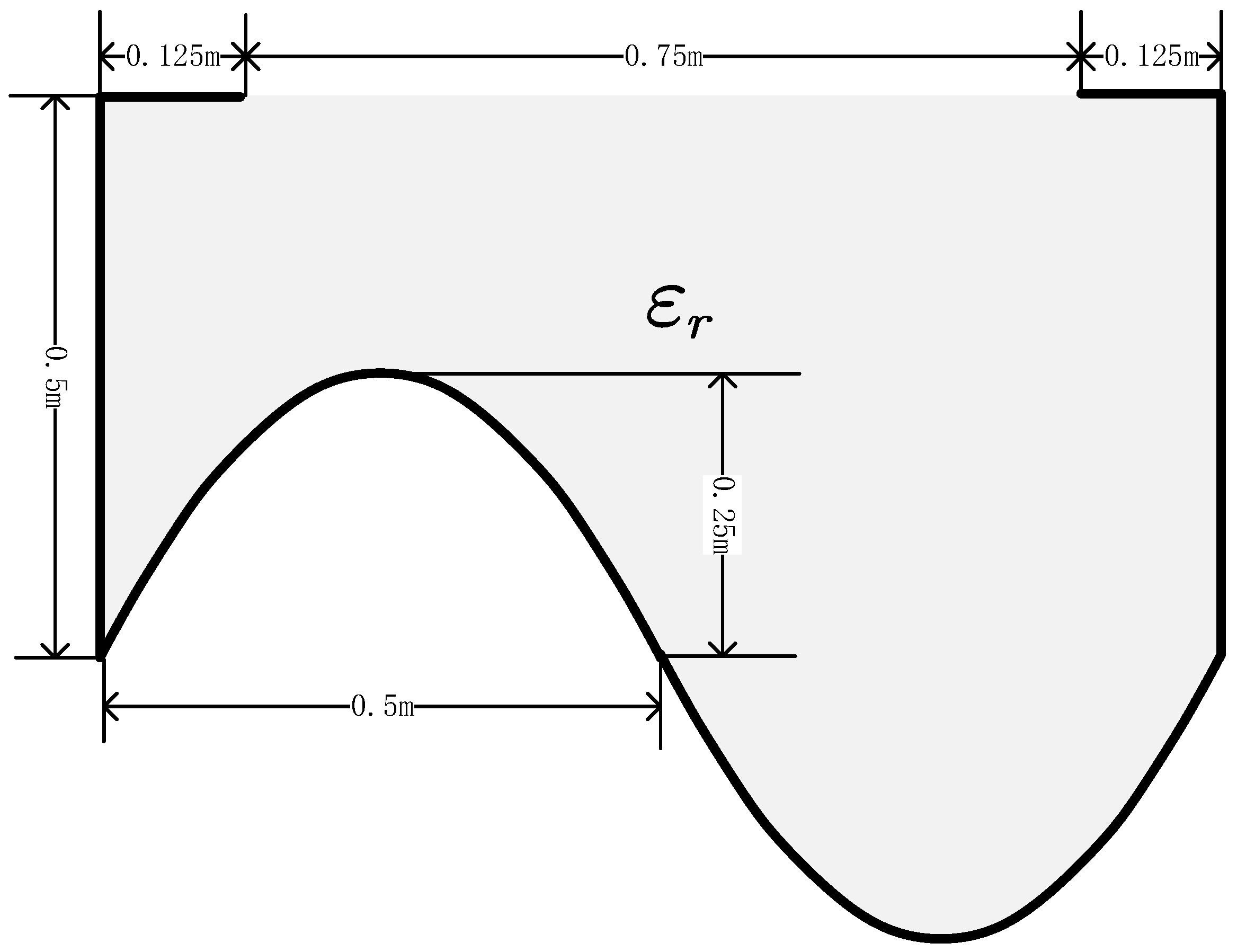
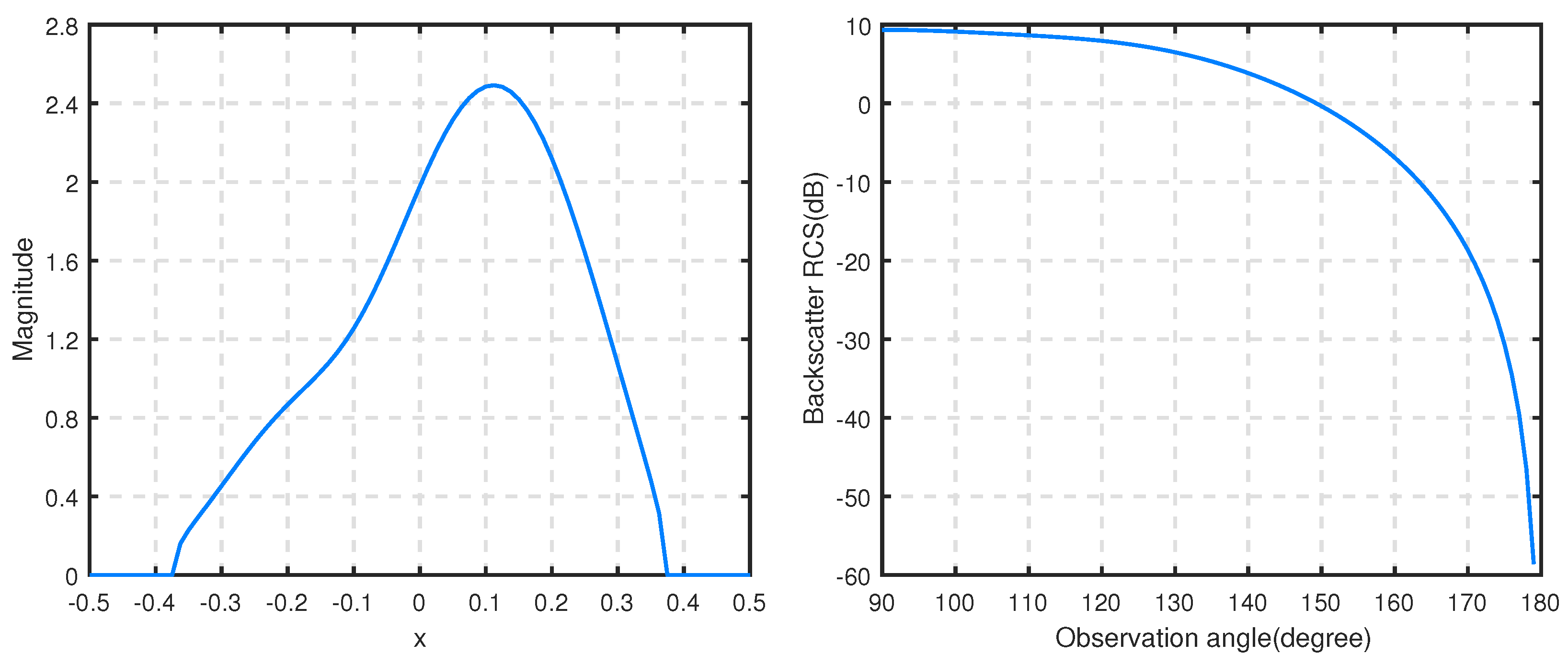
5. Numerical Examples
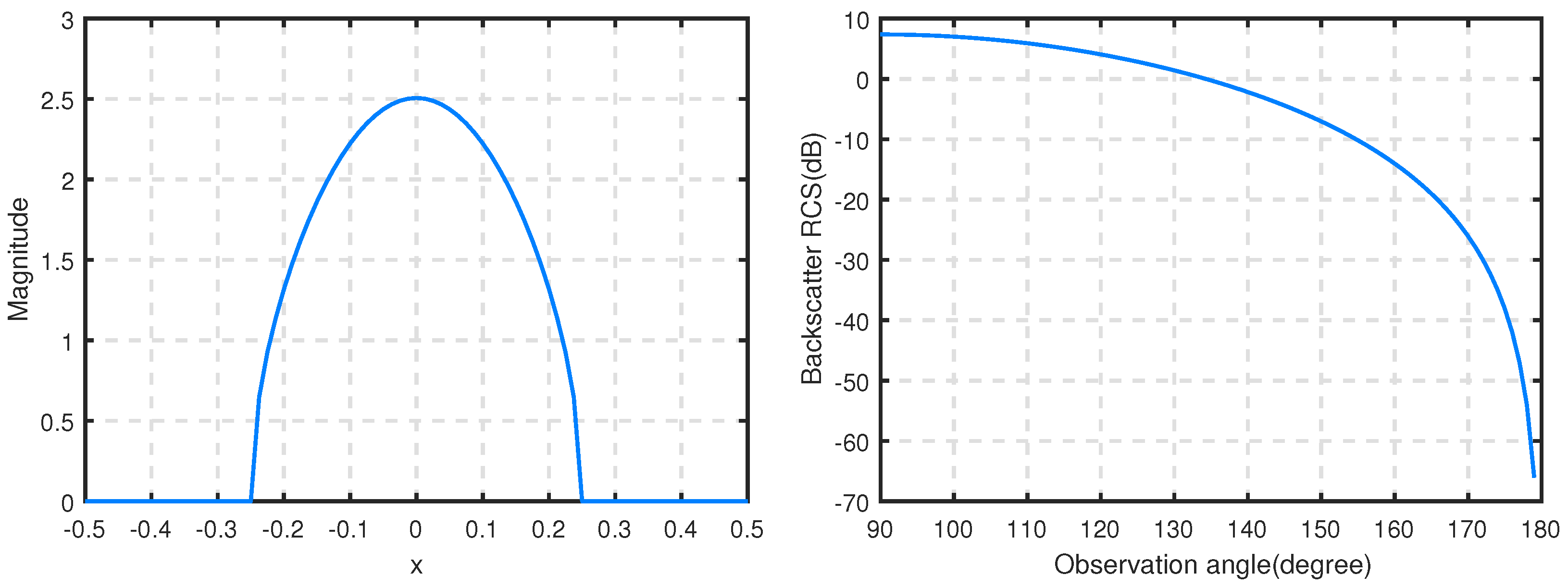
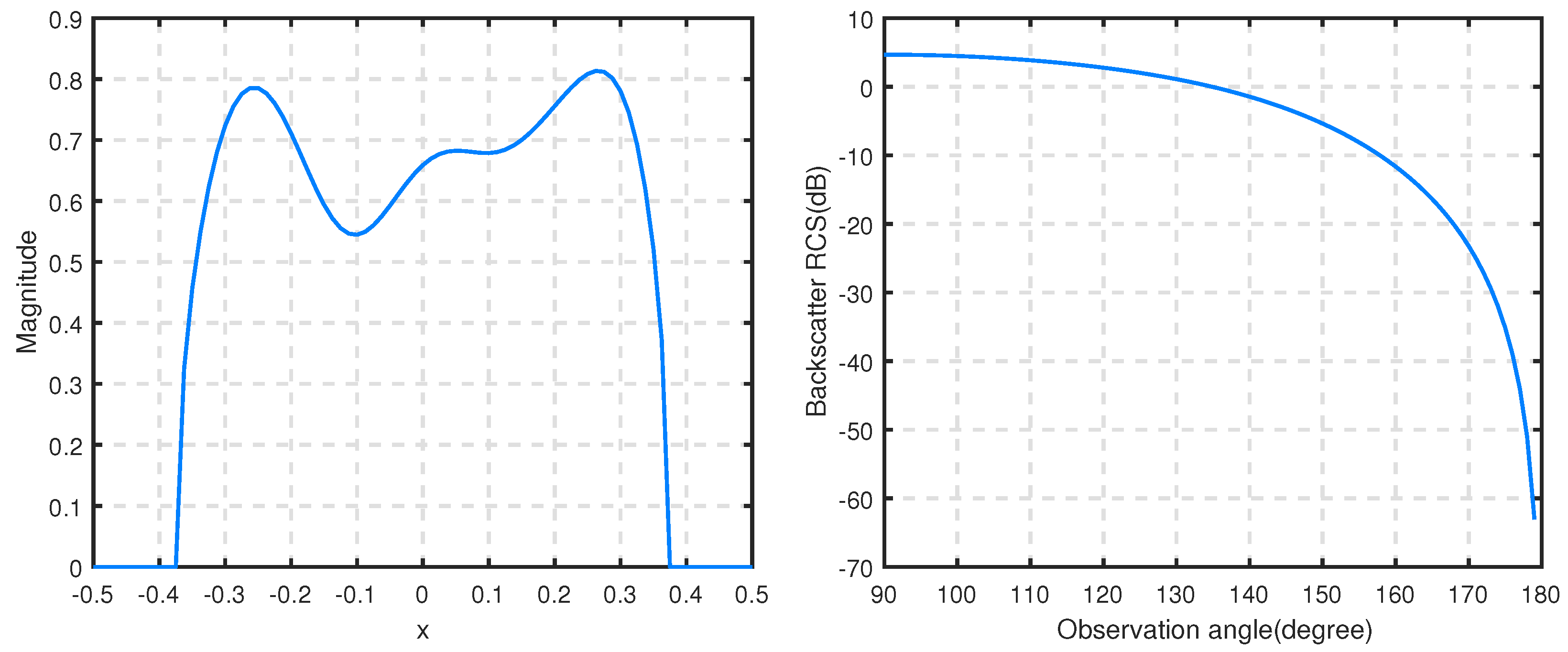
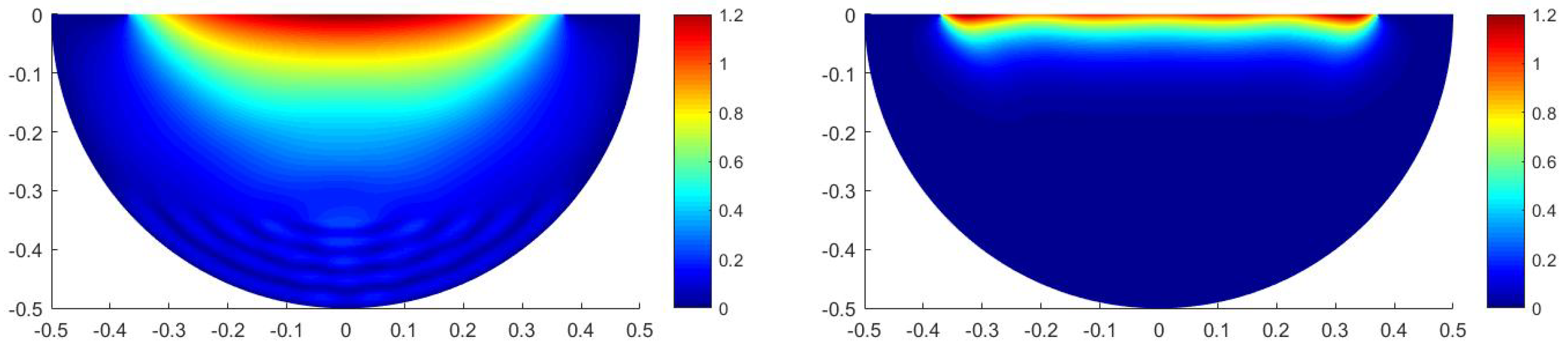
5.1. Example 1
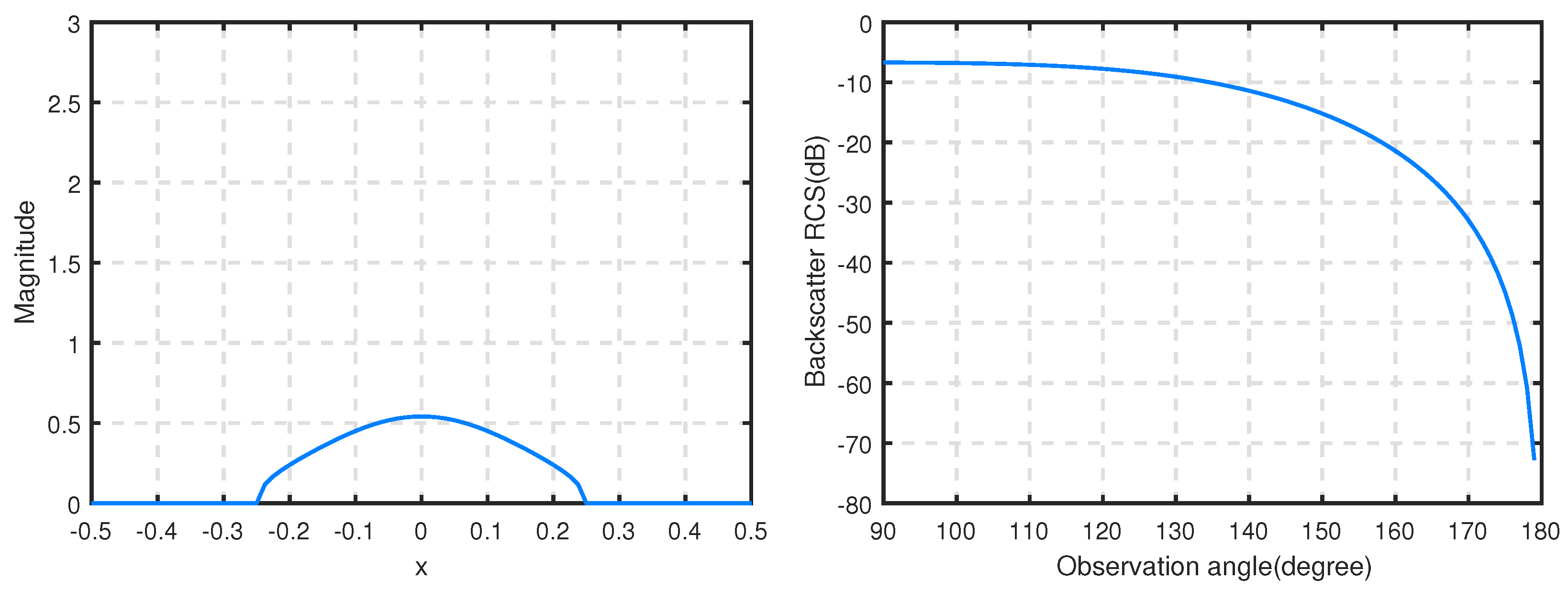
5.2. Example 2
5.3. Example 3
5.4. Example 4
5.5. Example 5
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bao, G.; Sun, W.W. A fast algorithm for the electromagnetic scattering from a large cavity. SIAM J. Sci. Comput. 2005, 27, 553–574. [Google Scholar] [CrossRef]
- Wang, Y.X.; Du, K.; Sun, W.W. A second-order method for the electromagnetic scattering from a large cavity. Numer. Math. Theory Methods Appl. 2008, 1, 357–382. [Google Scholar]
- Zhao, M.L.; Zhu, N.; Wang, L.Q. Fast algorithms for the electromagnetic scattering by partly covered cavities. Appl. Math. Comput. 2021, 40, 7. [Google Scholar] [CrossRef]
- Zhao, M.L.; Zhu, N. A fast preconditioned iterative method for the electromagnetic scattering by multiple cavities with high wave numbers. J. Comput. Phys. 2019, 398, 108826. [Google Scholar] [CrossRef]
- Singer, I.; Turkel, E. High-order finite difference methods for the Helmholtz equation. Comput. Methods Appl. Mech. Eng. 1998, 163, 343–358. [Google Scholar] [CrossRef]
- Du, K.; Sun, W.W. Numerical solution of electromagnetic scattering from a large partly covered cavity. J. Comput. Appl. Math. 2011, 235, 3791–3806. [Google Scholar] [CrossRef]
- Zhao, M.L.; Qiao, Z.H.; Tang, T. A fast high order method for electromagnetic scattering by large open cavities. J. Comput. Math. 2011, 29, 287–304. [Google Scholar] [CrossRef]
- Jin, J.M.; Volakis, J.L. A finite-element-boundary integral formulation for scattering by three-dimensional cavity-backed apertures. IEEE Trans. Antennas Propag. 1991, 39, 97–104. [Google Scholar] [CrossRef]
- Jin, J.M. The Finite Element Method in Electromagnetics, 2nd ed.; John Wiley Sons: New York, NY, USA, 2002; pp. 192–206. [Google Scholar]
- Van, T.; Wood, A.H. A time-domain finite element method for Helmholtz Equations. J. Comput. Phys. 2002, 183, 486–507. [Google Scholar] [CrossRef]
- Du, K. Two transparent boundary conditions for the electromagnetic scattering from two-dimensional overfilled cavities. J. Comput. Phys. 2011, 230, 5822–5835. [Google Scholar] [CrossRef]
- Huang, J.Q.; Wood, A.H.; Havrilla, M.J. A hybrid finite element-Laplace transform method for the analysis of transient electromagnetic scattering by an over-filled cavity in the ground plane. Commun. Comput. Phys. 2009, 5, 126–141. [Google Scholar]
- Wang, J.X.; Zhang, Z.M. A hybridizable weak Galerkin method for the Helmholtz equation with large wave number: hp Analysis. Int. J. Numer. Anal. Model. 2017, 14, 744–761. [Google Scholar]
- Xiang, Z.G.; Chia, T. A hybrid BEM/WTM approach for analysis of the EM scattering from large open-ended cavities. IEEE Trans. Antennas Propag. 2001, 49, 165–173. [Google Scholar] [CrossRef]
- Lee, J.W.; Liu, L.W.; Hong, H.K.; Chen, J.T. Applications of the Clifford algebra valued boundary element method to electromagnetic scattering problems. Eng. Anal. Bound. Elem. 2016, 71, 140–150. [Google Scholar] [CrossRef]
- Simpson, R.N.; Liu, Z.; Vázquez, R.; Evans, J.A. An isogeometric boundary element method for electromagnetic scattering with compatible B-spline discretizations. J. Comput. Phys. 2018, 362, 264–289. [Google Scholar] [CrossRef]
- Takahashi, T. A fast time-domain boundary element method for three-dimensional electromagnetic scattering problems. J. Comput. Phys. 2023, 482, 112053. [Google Scholar] [CrossRef]
- Liu, J.; Ma, F.M. A PML method for electromagnetic scattering from two-dimensional overfilled cavities. Commun. Math. Res. 2009, 25, 53–68. [Google Scholar]
- Tang, J.W.; Paulsen, K.D.; Haider, S.A. Perfectly matched layer mesh terminations for nodal-based finite-element methods in electromagnetic scattering. IEEE Trans. Antennas Propag. 1998, 46, 507–516. [Google Scholar] [CrossRef]
- Banks, H.T.; Browning, B.L. Time domain electromagnetic scattering using finite elements and perfectly matched layers. Comput. Methods Appl. Mech. Eng. 2005, 194, 149–168. [Google Scholar] [CrossRef]
- Wu, X.M.; Zheng, W.Y. An adaptive perfectly matched layer method for multiple cavity scattering problems. Commun. Comput. Phys. 2016, 19, 534–558. [Google Scholar] [CrossRef]
- Wu, J.M.; Wang, Y.X.; Li, W.; Sun, W.W. Toeplitz-type approximations to the Hadamard integral operator and their applications to electromagnetic cavity problems. Appl. Numer. Math. 2008, 58, 101–121. [Google Scholar] [CrossRef]
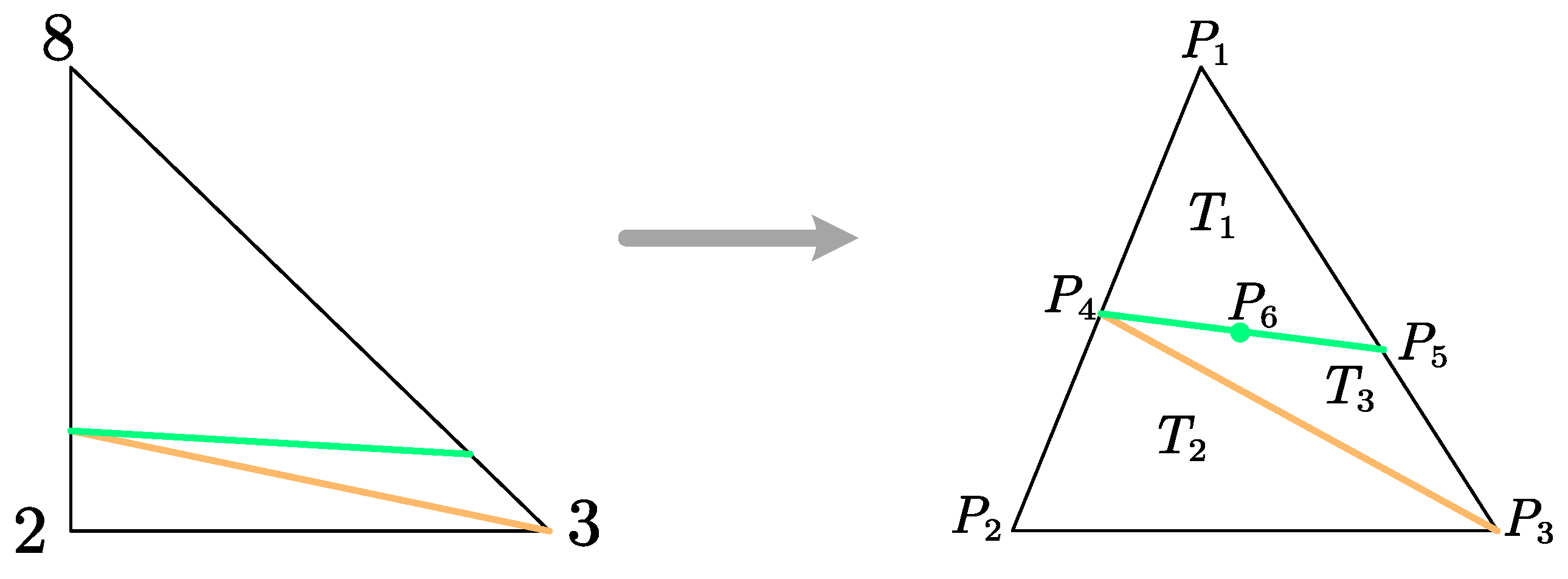




| Method | Meshes | Error | Order |
|---|---|---|---|
| 20 × 20 | 0.0536 | – | |
| Non-traditional | 40 × 40 | 0.0136 | 1.9796 |
| finite element | 80 × 80 | 0.0034 | 1.9823 |
| method | 160 × 160 | 0.0009 | 1.9820 |
| 20 × 20 | 1.7569 | – | |
| Traditional finite | 40 × 40 | 1.6787 | 0.0657 |
| element method | 80 × 80 | 1.6398 | 0.0339 |
| 160 × 160 | 1.6204 | 0.0172 |
| Meshes | Error | Order | Error | Order |
|---|---|---|---|---|
| 192 × 48 | 0.0359 | – | 0.1971 | – |
| 384 × 96 | 0.0094 | 1.9308 | 0.0543 | 1.8597 |
| 768 × 192 | 0.0022 | 2.1025 | 0.0144 | 1.9176 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, X.; Zhang, W.; Zhao, M. A Non-Traditional Finite Element Method for Scattering by Partly Covered Grooves with Multiple Media. Mathematics 2024, 12, 254. https://doi.org/10.3390/math12020254
Fang X, Zhang W, Zhao M. A Non-Traditional Finite Element Method for Scattering by Partly Covered Grooves with Multiple Media. Mathematics. 2024; 12(2):254. https://doi.org/10.3390/math12020254
Chicago/Turabian StyleFang, Xianqi, Wenbin Zhang, and Meiling Zhao. 2024. "A Non-Traditional Finite Element Method for Scattering by Partly Covered Grooves with Multiple Media" Mathematics 12, no. 2: 254. https://doi.org/10.3390/math12020254
APA StyleFang, X., Zhang, W., & Zhao, M. (2024). A Non-Traditional Finite Element Method for Scattering by Partly Covered Grooves with Multiple Media. Mathematics, 12(2), 254. https://doi.org/10.3390/math12020254






