Finite Element Method for a Fractional-Order Filtration Equation with a Transient Filtration Law
Abstract
1. Introduction
2. Materials and Methods
2.1. Formulation of the Problem
2.2. The Uniqueness of the Solution and Its Continuous Dependence on the Input Data
2.2.1. Weak Variational Formulation of the Problem
2.2.2. Uniqueness of the Solution and Its Continuous Dependence on the Input Data
2.3. A Numerical Method
2.3.1. Construction of a Semi-Discrete Numerical Scheme
2.3.2. Construction of the Fully Discrete Scheme
2.4. Stability of the Numerical Scheme
2.5. Convergence of the Numerical Method
2.5.1. Convergence of a Semi-Discrete Scheme
2.5.2. Convergence of a Fully Discrete Scheme
2.5.3. Convergence of the Iterative Process
3. Results
Verification of the Order of Convergence Based on Computational Experiments
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Podlubny, I. Fractional Differential Equations. Math. Sci. Eng. 1999, 198, 1–340. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon and Breach Science Publishers: Basel, Switzerland; Philadelphia, PA, USA, 1993. [Google Scholar]
- Mainardi, F. (Ed.) Fractional Calculus: Theory and Applications; MDPI: Basel, Switzerland, 2018; ISBN 9783038972068. [Google Scholar]
- Oliveira, E.; Machado, J. A review of definitions for fractional derivatives and integral. Math. Probl. Eng. 2014, 2014, 238459. [Google Scholar] [CrossRef]
- Teodoro, G.; Machado, J.; Oliveira, E. A review of definitions of fractional derivatives and other operators. J. Comput. Phys. 2019, 388, 195–208. [Google Scholar] [CrossRef]
- Troparevsky, M.I.; Seminara, S.A.; Fabio, M.A. A Review on Fractional Differential Equations and a Numerical Method to Solve Some Boundary Value Problems; IntechOpen: London, UK, 2020. [Google Scholar]
- Caputo, M. Models of Flux in Porous Media with Memory. Water Resour. Res. 2000, 36, 693–705. [Google Scholar] [CrossRef]
- Darcy, H. Les Fontaines Publiques de la Ville de Dijon: Exposition et Application des Principes à Suivre et des Formules à Employer dans les Questions; Dalmont: Paris, France, 1856. [Google Scholar]
- Ansari, S.U.; Hussain, M.; Rashid, A.; Mazhar, S.; Ahmad, S.M. Numerical solution and analysis of three-dimensional transient Darcy flow. Transp. Porous Media 2018, 123, 289–305. [Google Scholar] [CrossRef]
- Lewis, R.W.; Nithiarasu, P.; Seetharamu, K.N. Fundamentals of the Finite Element Method for Heat and Fluid Flow; Wiley: New York, NY, USA, 2004. [Google Scholar]
- Istok, J. Groundwater Modeling by the Finite Element Method; Wiley: New York, NY, USA, 2013. [Google Scholar]
- Alcántara-López, F.; Fuentes, C.; Brambila-Paz, F.; López-Estrada, J. Quasi-analytical model of the transient behavior pressure in an oil reservoir made up of three porous media considering the fractional time derivative. Math. Comput. Appl. 2020, 25, 74. [Google Scholar] [CrossRef]
- Chueh, C. Integrated Adaptive Numerical Methods for Transient Two-Phase Flow in Heterogeneous Porous Media. Ph.D. Thesis, University of Victoria, Greater Victoria, BC, Canada, 2010. [Google Scholar]
- Heymans, N.; Podlubny, I. Physical interpretation of initial conditions for fractional differential equations with Riemann-Liouville fractional derivatives. Rheol. Acta 2006, 45, 765–771. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A New Definition of Fractional Derivative without Singular Kernel. Prog. Fract. Differ. Appl. 2015, 2, 73–85. [Google Scholar]
- Tarasov, V.E. Caputo-Fabrizio operator in terms of integer derivatives: Memory or distributed lag? Comput. Appl. Math. 2019, 38, 113. [Google Scholar] [CrossRef]
- El Amin, M.F.; Radwan, A.G.; Sun, S. Analytical solution for fractional derivative gas-flow equation in porous media. Results Phys. 2017, 7, 2432–2438. [Google Scholar] [CrossRef]
- Ray, S.S. Exact solutions for time-fractional diffusion-wave equations by decomposition method. Phys. Scr. 2007, 75, 53–61. [Google Scholar] [CrossRef]
- Zhang, Y.N.; Sun, Z.Z.; Liao, H.L. Finite difference methods for the time fractional diffusion equation on non-uniform meshes. J. Comput. Phys. 2014, 265, 195–210. [Google Scholar] [CrossRef]
- Alikhanov, A.A. A new difference scheme for the time fractional diffusion equation. J. Comput. Phys. 2015, 280, 424–438. [Google Scholar] [CrossRef]
- Qiao, H.L.; Liu, Z.G.; Cheng, A.J. Two unconditionally stable difference schemes for time distributed-order differential equation based on Caputo–Fabrizio fractional derivative. Adv. Differ. Equ. 2020, 2020, 36. [Google Scholar] [CrossRef]
- Du, R.; Cao, W.R.; Sun, Z.Z. A compact difference scheme for the fractional diffusion-wave equation. Appl. Math. Model. 2010, 34, 2998–3007. [Google Scholar] [CrossRef]
- Huang, J.; Tang, Y.; Wang, W.; Yang, J. A compact difference scheme for time fractional diffusion equation with Neumann boundary conditions. Commun. Comput. Inf. Sci. 2012, 323, 273–284. [Google Scholar]
- Bliyeva, D.; Baigereyev, D.; Imomnazarov, K. Computer Simulation of the Seismic Wave Propagation in Poroelastic Medium. Symmetry 2022, 14, 1516. [Google Scholar] [CrossRef]
- Xu, T.; Lu, S.; Chen, W.; Chen, H. Finite difference scheme for multi-term variable-order fractional diffusion equation. Adv. Differ. Equ. 2018, 1, 103. [Google Scholar] [CrossRef]
- Liu, J.; Zhou, Z. Finite element approximation of time fractional optimal control problem with integral state constraint. AIMS Math. 2020, 6, 979–997. [Google Scholar] [CrossRef]
- Berdyshev, A.; Baigereyev, D.; Boranbek, K. Numerical method for fractional-order generalization of the stochastic Stokes-Darcy model. Mathematics 2023, 11, 3763. [Google Scholar] [CrossRef]
- Baigereyev, D.; Omariyeva, D.; Temirbekov, N.; Yergaliyev, Y.; Boranbek, K. Numerical method for a filtration model involving a nonlinear partial integro-differential equation. Mathematics 2022, 10, 1319. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, H.; Zhou, Z.J. A priori error analysis for time-stepping discontinuous Galerkin finite element approximation of time fractional optimal control problem. J. Sci. Comput. 2019, 80, 993–1018. [Google Scholar] [CrossRef]
- Liu, K.; Feckan, M.; O’Regan, D.; Wang, J.R. Hyers–Ulam stability and existence of solutions for differential equations with Caputo–Fabrizio fractional derivative. Mathematics 2019, 7, 333. [Google Scholar] [CrossRef]
- Liu, Y.; Du, Y.; Li, H.; Li, J.; He, S. A two-grid mixed finite element method for a nonlinear fourth-order reaction-diffusion problem with time-fractional derivative. Comput. Math. Appl. 2015, 70, 2474–2492. [Google Scholar] [CrossRef]
- Baigereyev, D.; Alimbekova, N.; Berdyshev, A.; Madiyarov, M. Convergence analysis of a numerical method for a fractional model of fluid flow in fractured porous media. Mathematics 2021, 9, 2179. [Google Scholar] [CrossRef]
- Liu, F.; Zhuang, P.; Turner, I.; Burrage, K.; Anh, V. A new fractional finite volume method for solving the fractional diffusion equation. Appl. Math. Model. 2013, 38, 3871–3878. [Google Scholar] [CrossRef]
- Wang, S.; Zhao, M. Analytical solution of the transient electro-osmotic flow of a generalized fractional Maxwell fluid in a straight pipe with a circular cross-section. Eur. J. Mech. B Fluid 2015, 54, 82–86. [Google Scholar] [CrossRef]
- Coronel-Escamilla, A.; Gomez-Aguilar, J.F.; Torres, L.; Escobar-Jimenez, R.F. A numerical solution for a variable-order reaction-diffusion model by using fractional derivatives with non-local and non-singular kernel. Phys. A Stat. Mech. Appl. 2017, 491, 406–424. [Google Scholar] [CrossRef]
- Mallawi, F.; Alzaidy, J.F.; Hafez, R.M. Application of a Legendre collocation method to the space–time variable fractional-order advection–dispersion equation. J. Taibah Univ. Sci. 2019, 13, 324–330. [Google Scholar] [CrossRef]
- Oldham, K.B.; Spanier, J. The Fractional Calculus; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Lin, Y.; Xu, C. Finite difference/spectral approximations for the time-fractional diffusion equation. J. Comput. Phys. 2007, 225, 1533–1552. [Google Scholar] [CrossRef]
- Jin, B.; Lazarov, R.; Zhou, Z. An analysis of the L1 scheme for the subdiffusion equation with nonsmooth data. IMA J. Numer. Anal. 2016, 36, 197–221. [Google Scholar] [CrossRef]
- Yan, Y.; Khan, M.; Ford, N. An analysis of the modified L1 scheme for time-fractional partial differential equations with nonsmooth data. SIAM J. Numer. Anal. 2018, 56, 210–227. [Google Scholar] [CrossRef]
- Siddiqi, S.; Arshed, S. Numerical solution of time-fractional fourth-order partial differential equations. Int. J. Comput. Math. 2014, 92, 1496–1518. [Google Scholar] [CrossRef]
- Gao, G.H.; Sun, Z.Z.; Zhang, H.W. A new fractional numerical differentiation formula to approximate the Caputo fractional derivative and its applications. J. Comput. Phys. 2014, 259, 33–50. [Google Scholar] [CrossRef]
- Luo, W.-H.; Li, C.; Huang, T.-Z.; Gu, X.-M.; Wu, G.-C. A High-Order Accurate Numerical Scheme for the Caputo Derivative with Applications to Fractional Diffusion Problems. Numer. Funct. Anal. Optim. 2018, 39, 600–622. [Google Scholar] [CrossRef]
- Alikhanov, A.; Huang, C. A high-order L2 type difference scheme for the time-fractional diffusion equation. Appl. Math. Comput. 2021, 411, 126545. [Google Scholar] [CrossRef]
- Cao, J.; Li, C.; Chen, Y. High-order approximation to Caputo derivatives and Caputo-type advection-diffusion equations (II). Fract. Calc. Appl. Anal. 2015, 18, 735–761. [Google Scholar] [CrossRef]
- Xuhao, L. Numerical Methods for Fractional Differential Equations. Ph.D. Thesis, Nanyang Technological University, Nanyang, Singapore, 2018. [Google Scholar]
- Yu, F.; Chen, M. Finite difference/spectral approximations for the two-dimensional time Xaputo–Fabrizio fractional diffusion equation. arXiv 2019, arXiv:1906.00328v1. [Google Scholar]
- Caputo, M. Lineal model of dissipation whose q is almost frequancy independent—II. Geophys. J. Astronom. Soc. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Alikhanov, A.A. A priori estimates for solutions of boundary value problems for fractional-order equations. Differ. Equ. 2010, 46, 660–666. [Google Scholar] [CrossRef]
- Atangana, A. Extension of rate of change concept: From local to nonlocal operators with applications. Results Phys. 2020, 19, 103515. [Google Scholar] [CrossRef]
- Ouyang, Y.; Wang, W. Comparison of definition of several fractional derivatives. In Proceedings of the 2016 6th International Conference on Education, Management and Computer Science (ICEMC 2016), Shenyang, China, 27–29 May 2016; pp. 553–557. [Google Scholar]
- Zhumagulov, B.; Temirbekov, N.; Baigereyev, D. Efficient difference schemes for the three-phase non-isothermal flow problem. AIP Conf. Proc. 2017, 1880, 060001. [Google Scholar]
- Radu, F.A.; Pop, I.S. Simulation of reactive contaminant transport with non-equilibrium sorption by mixed finite elements and newton method. Comput. Geosci. 2011, 15, 431–450. [Google Scholar] [CrossRef]
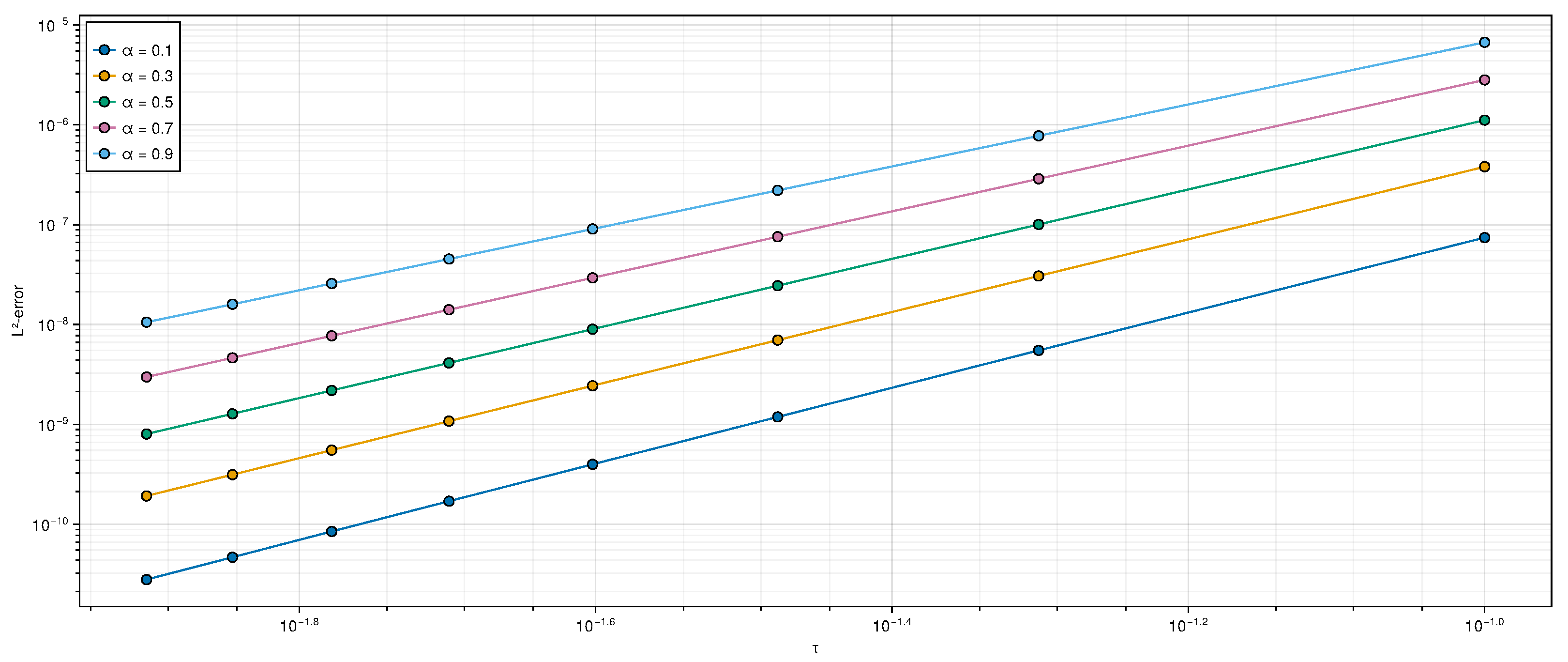
| -Error | Order | -Error | Order | -Error | Order | |
|---|---|---|---|---|---|---|
| 1/10 | - | - | - | |||
| 1/20 | 3.75 | 3.69 | 3.63 | |||
| 1/30 | 3.78 | 3.72 | 3.65 | |||
| 1/40 | 3.80 | 3.73 | 3.66 | |||
| 1/50 | 3.81 | 3.74 | 3.66 | |||
| 1/60 | 3.83 | 3.75 | 3.67 | |||
| 1/70 | 3.85 | 3.76 | 3.67 | |||
| 1/80 | 3.86 | 3.77 | 3.68 | |||
| Predicted | 3.9 | 3.8 | 3.7 | |||
| -Error | Order | -Error | Order | -Error | Order | |
| 1/10 | - | - | - | |||
| 1/20 | 3.55 | 3.47 | 3.38 | |||
| 1/30 | 3.57 | 3.48 | 3.39 | |||
| 1/40 | 3.57 | 3.48 | 3.39 | |||
| 1/50 | 3.58 | 3.49 | 3.39 | |||
| 1/60 | 3.58 | 3.49 | 3.39 | |||
| 1/70 | 3.58 | 3.49 | 3.39 | |||
| 1/80 | 3.58 | 3.49 | 3.40 | |||
| Predicted | 3.6 | 3.5 | 3.4 | |||
| -Error | Order | -Error | Order | -Error | Order | |
| 1/10 | - | - | - | |||
| 1/20 | 3.29 | 3.19 | 3.11 | |||
| 1/30 | 3.29 | 3.20 | 3.10 | |||
| 1/40 | 3.29 | 3.20 | 3.10 | |||
| 1/50 | 3.30 | 3.20 | 3.10 | |||
| 1/60 | 3.30 | 3.20 | 3.10 | |||
| 1/70 | 3.30 | 3.20 | 3.10 | |||
| 1/80 | 3.30 | 3.20 | 3.10 | |||
| Predicted | 3.3 | 3.2 | 3.1 | |||
| Mesh Parameters | |||||
|---|---|---|---|---|---|
| Nodes | 441 | 1681 | 10,201 | 40,401 | 160,801 |
| Cells | 100 | 400 | 2500 | 10,000 | 40,000 |
| CPU Time (s) | |||||
| 1/10 | 0.0322 | 0.1113 | 1.0653 | 3.6686 | 16.0136 |
| 1/20 | 0.0828 | 0.2362 | 1.2875 | 6.2702 | 30.8062 |
| 1/30 | 0.1233 | 0.3537 | 1.9482 | 9.3582 | 46.3597 |
| 1/40 | 0.1564 | 0.4630 | 2.5717 | 12.3262 | 61.7358 |
| 1/50 | 0.1866 | 0.5783 | 3.1718 | 15.0545 | 76.6795 |
| 1/60 | 0.2306 | 0.6953 | 3.8219 | 18.0586 | 91.8569 |
| 1/70 | 0.2624 | 0.8053 | 4.5044 | 21.4017 | 106.8736 |
| 1/80 | 0.2985 | 0.9102 | 5.1263 | 24.2711 | 122.1779 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alimbekova, N.; Berdyshev, A.; Madiyarov, M.; Yergaliyev, Y. Finite Element Method for a Fractional-Order Filtration Equation with a Transient Filtration Law. Mathematics 2024, 12, 2519. https://doi.org/10.3390/math12162519
Alimbekova N, Berdyshev A, Madiyarov M, Yergaliyev Y. Finite Element Method for a Fractional-Order Filtration Equation with a Transient Filtration Law. Mathematics. 2024; 12(16):2519. https://doi.org/10.3390/math12162519
Chicago/Turabian StyleAlimbekova, Nurlana, Abdumauvlen Berdyshev, Muratkan Madiyarov, and Yerlan Yergaliyev. 2024. "Finite Element Method for a Fractional-Order Filtration Equation with a Transient Filtration Law" Mathematics 12, no. 16: 2519. https://doi.org/10.3390/math12162519
APA StyleAlimbekova, N., Berdyshev, A., Madiyarov, M., & Yergaliyev, Y. (2024). Finite Element Method for a Fractional-Order Filtration Equation with a Transient Filtration Law. Mathematics, 12(16), 2519. https://doi.org/10.3390/math12162519







