Reservoir Slope Stability Analysis under Dynamic Fluctuating Water Level Using Improved Radial Movement Optimisation (IRMO) Algorithm
Abstract
1. Introduction
2. Rigorous Limit Equilibrium Analysis Considering Seepage Force
2.1. Seepage Analysis
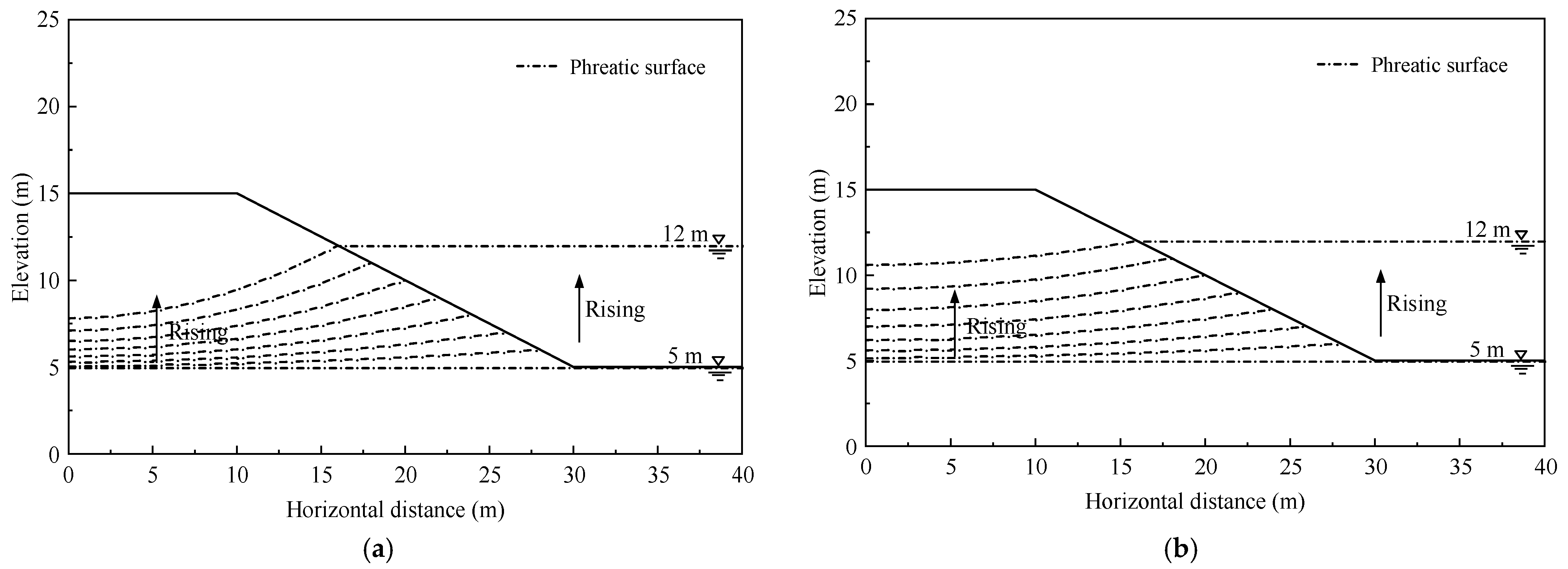
2.1.1. Relationship of Phreatic Surface and Fluctuating External Water Level
- The reservoir slope is considered a vertical slope. The reservoir slope within the declining amplitude is much smaller than the ground, so it is considered a vertical slope for simplification.
- The change in phreatic flow parallel to the slope surface is caused by the reservoir water-level fluctuation. (The change in phreatic flow perpendicular to the slope surface caused by the rainfall infiltration is not considered herein.)
- The external reservoir water level is decreasing or increasing at a constant speed of V0.
- The aquifer within the slope is homogeneous and isotropic with an infinite lateral extension.
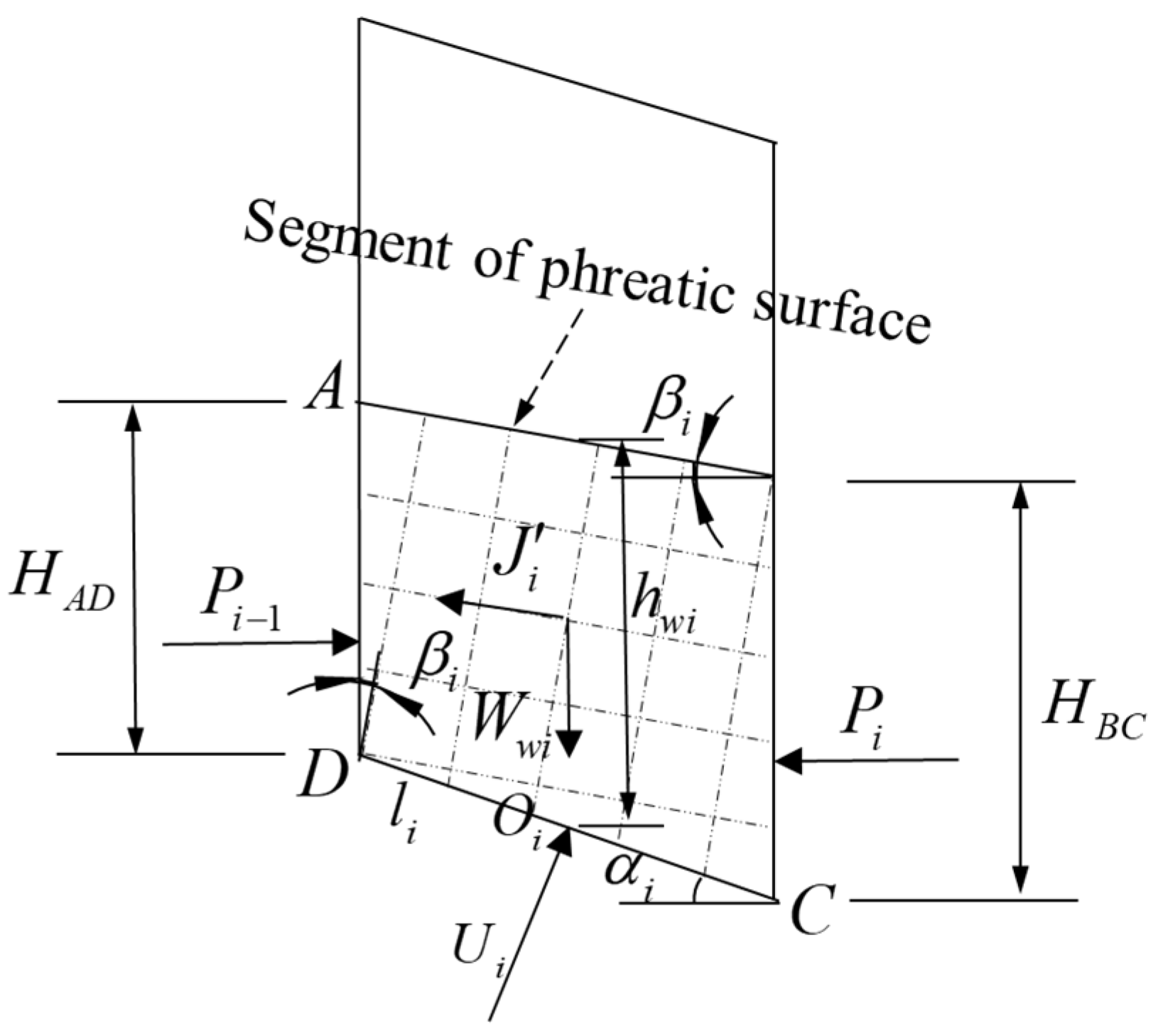
2.1.2. Simplification of Seepage Field
2.1.3. Seepage Force Analysis
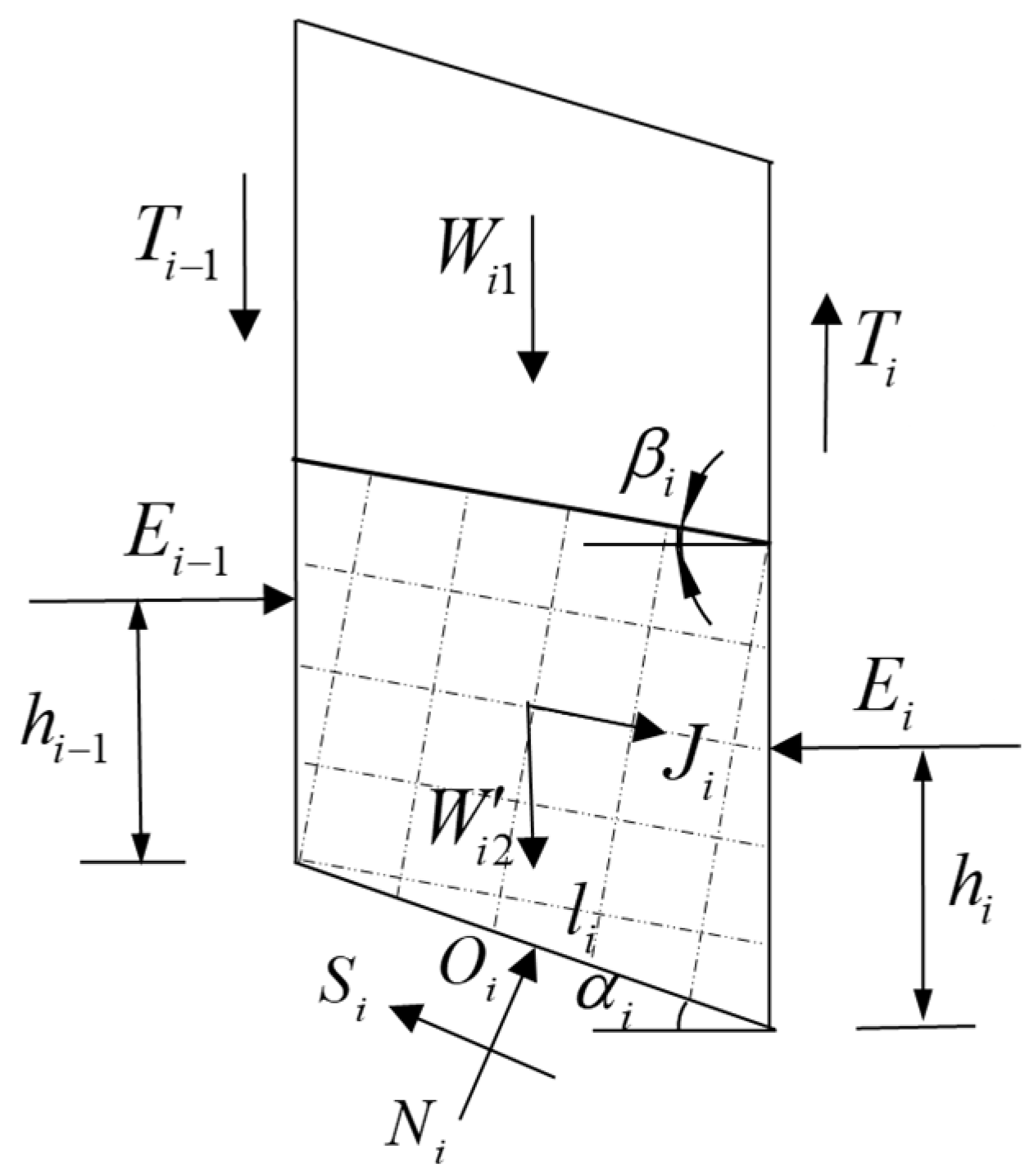
2.2. Rigorous Janbu Method Combined with Seepage Force
- Assuming ΔTi = 0 at the beginning, there is only FS unknown in Equation (18).
- Using the Newton iteration method, the initial FS1 can be obtained by Equation (18).
- Substitute the initial FS1 and assumed ΔTi into Equation (17) to obtain the value of ΔEi and Ei.
- According to Equation (14), the ΔTi and Ti can be updated iteratively with known ΔEi and Ei.
- Repeat steps (1)–(2) above, the FSk can also be updated with the Newton iteration method.
- If FSk − FSk−1 < δ, δ is the requirement of calculation accuracy set in advance, the FSk will be output as the final result. Otherwise, repeat steps (3)–(5) above until the calculation accuracy is satisfied.
2.3. Non-Circular Critical Failure Surface Model
3. Improved Radial Movement Optimisation (IRMO) Algorithm
3.1. Introduction of IRMO Algorithm
3.2. Computation Process of the IRMO Algorithm
3.2.1. Initialisation
3.2.2. Evolution: New Particles Updated
3.2.3. Evolution: Radial Movement of the Central Particle
3.3. Implementation of IRMO Algorithm for Reservoir Slope Stability Analysis
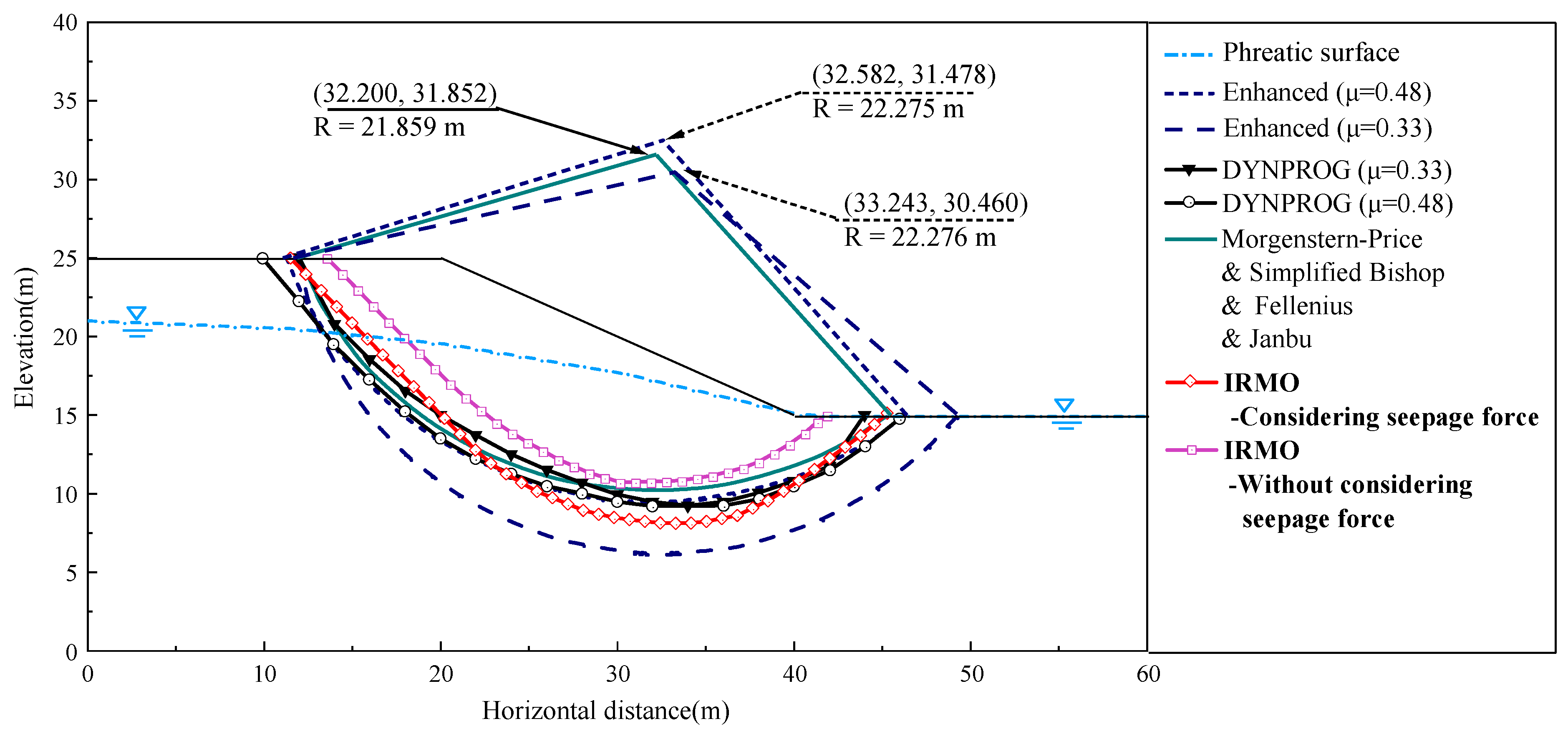
4. Case Studies
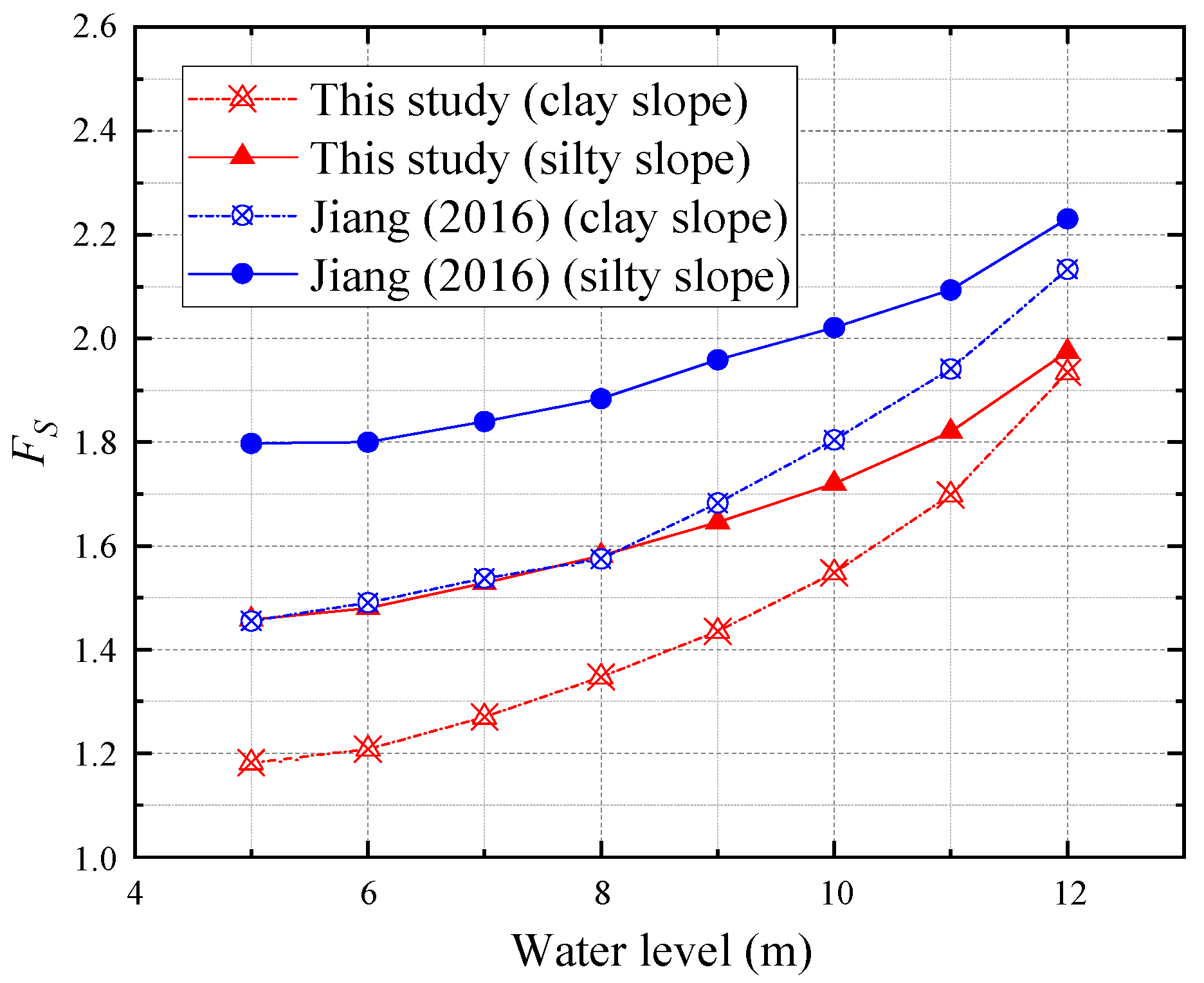
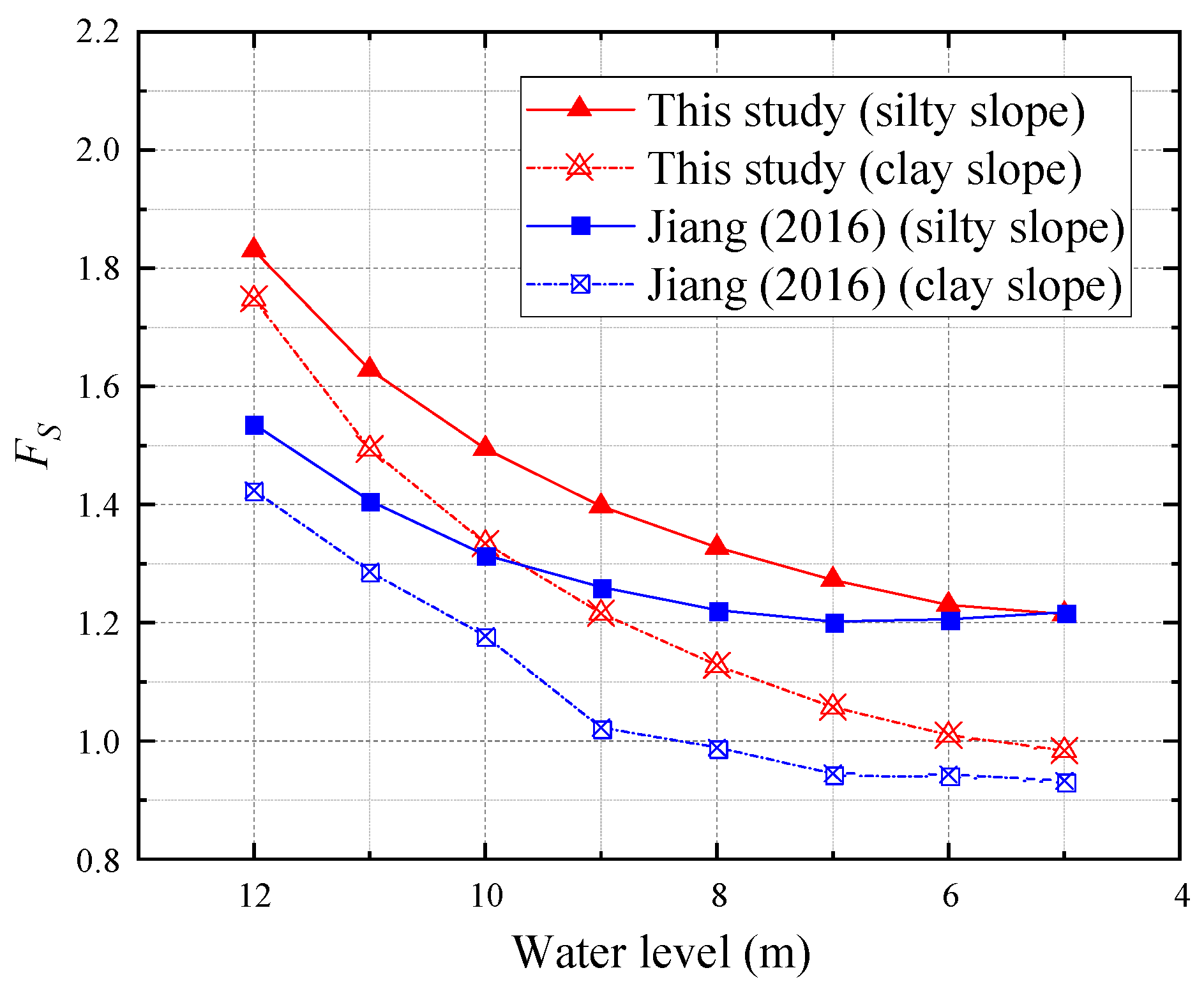
4.1. Comparative Analysis of the Impact of Seepage Force
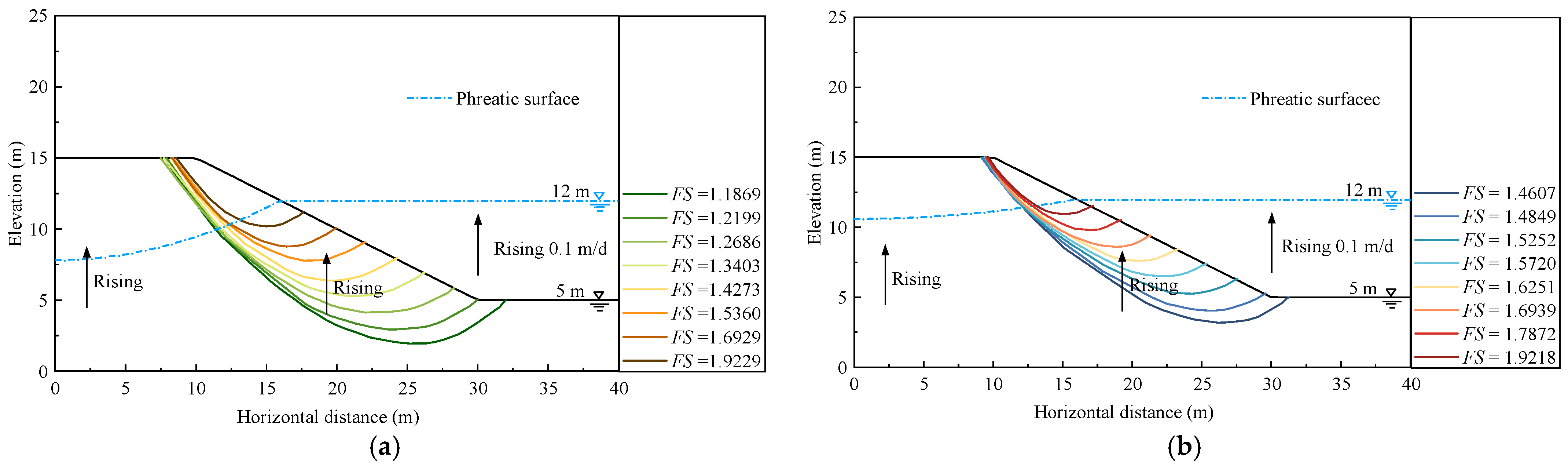
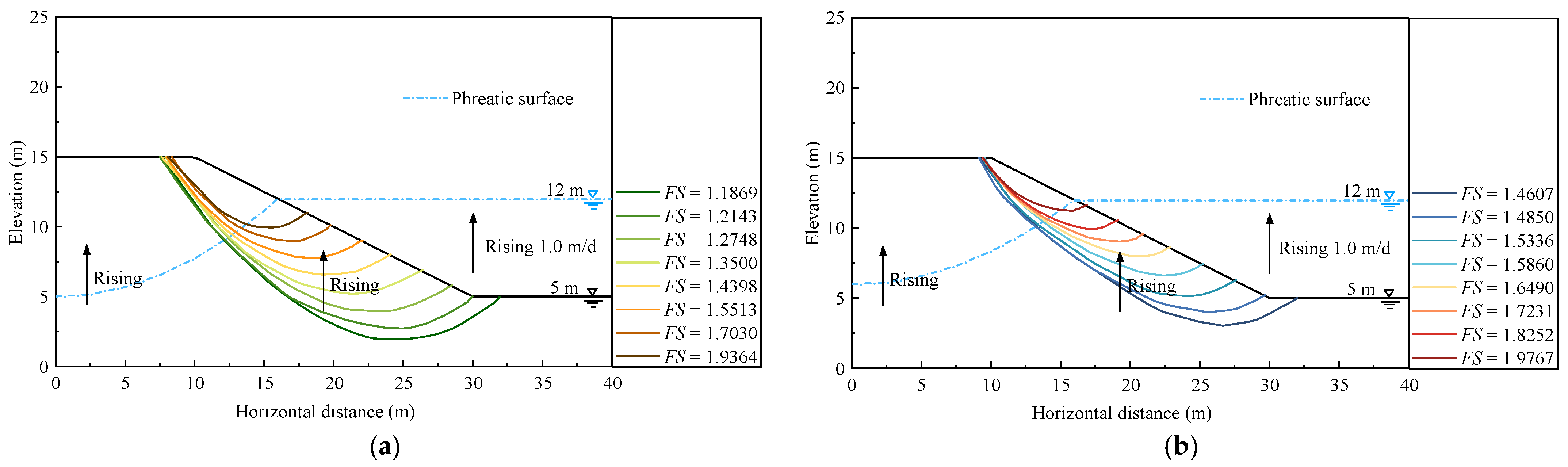
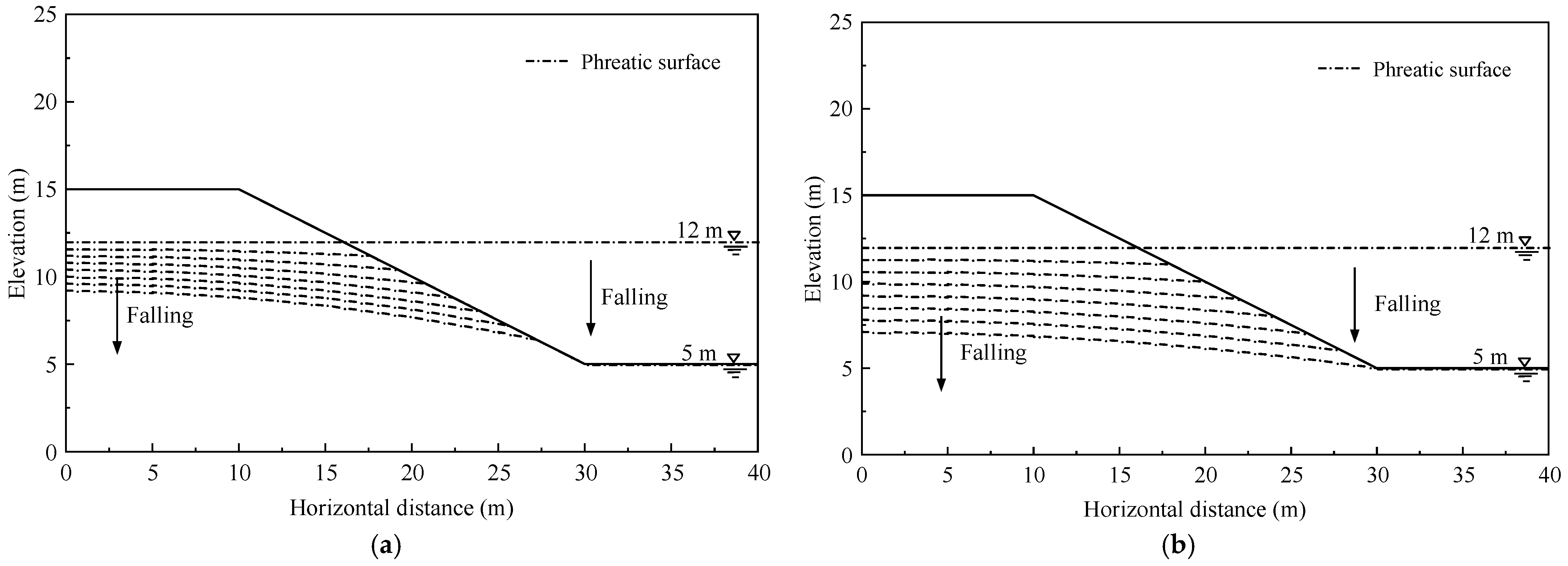
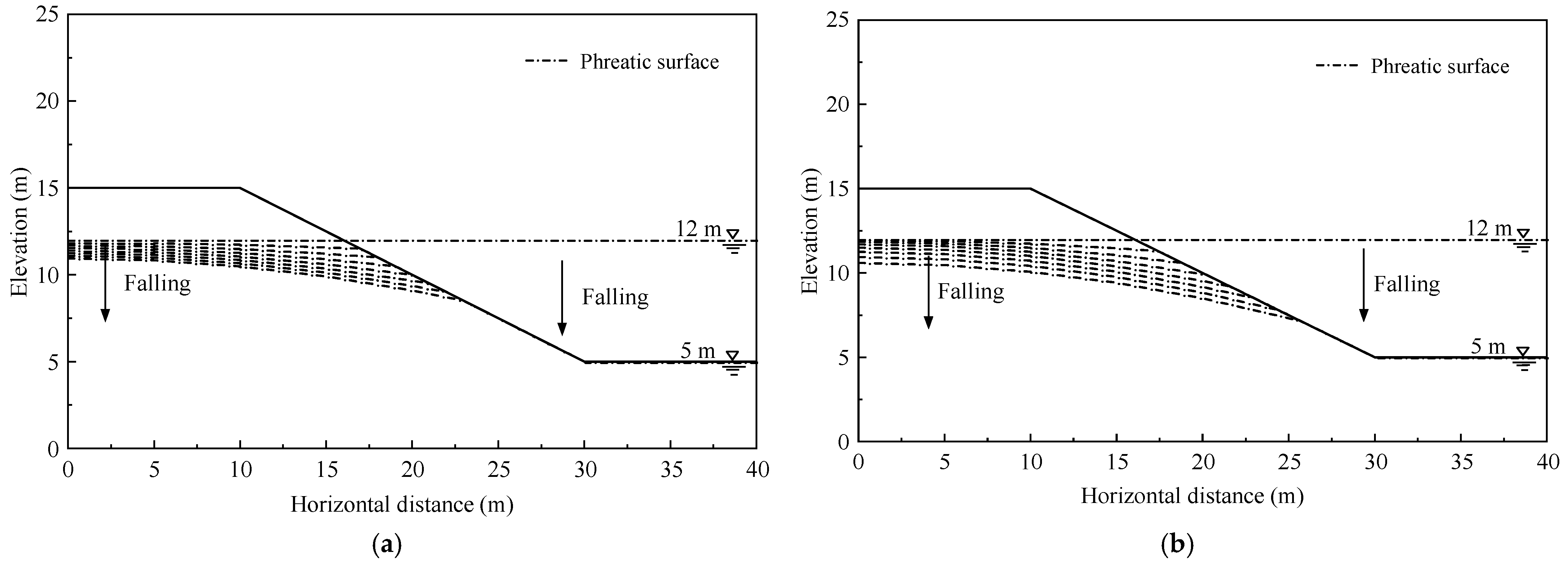
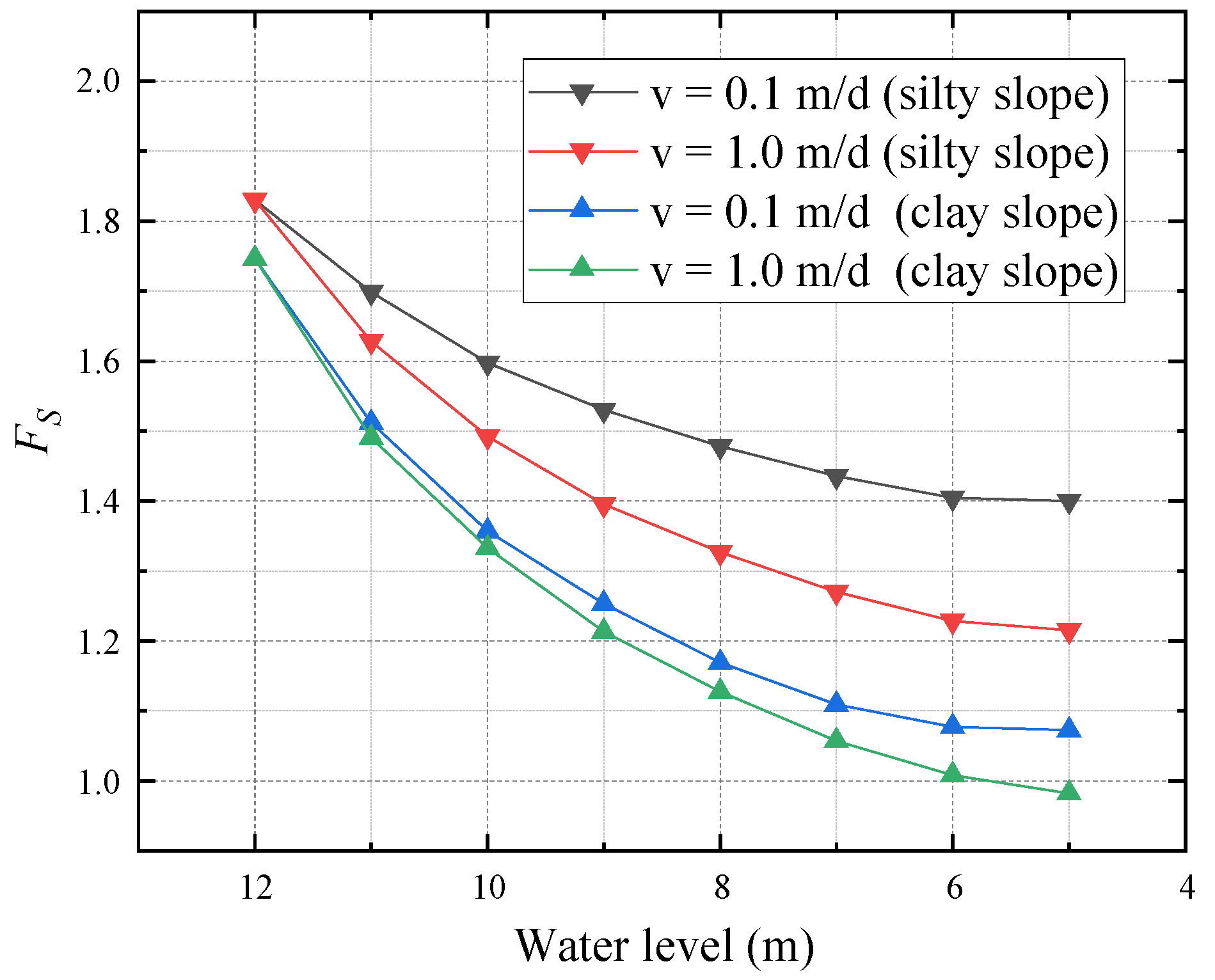
4.2. Effect of Varying Fluctuation Direction and Rate
4.2.1. Effect of Reservoir Water Level Rising with Different Rates
4.2.2. Effect of Reservoir Water Level Dropping
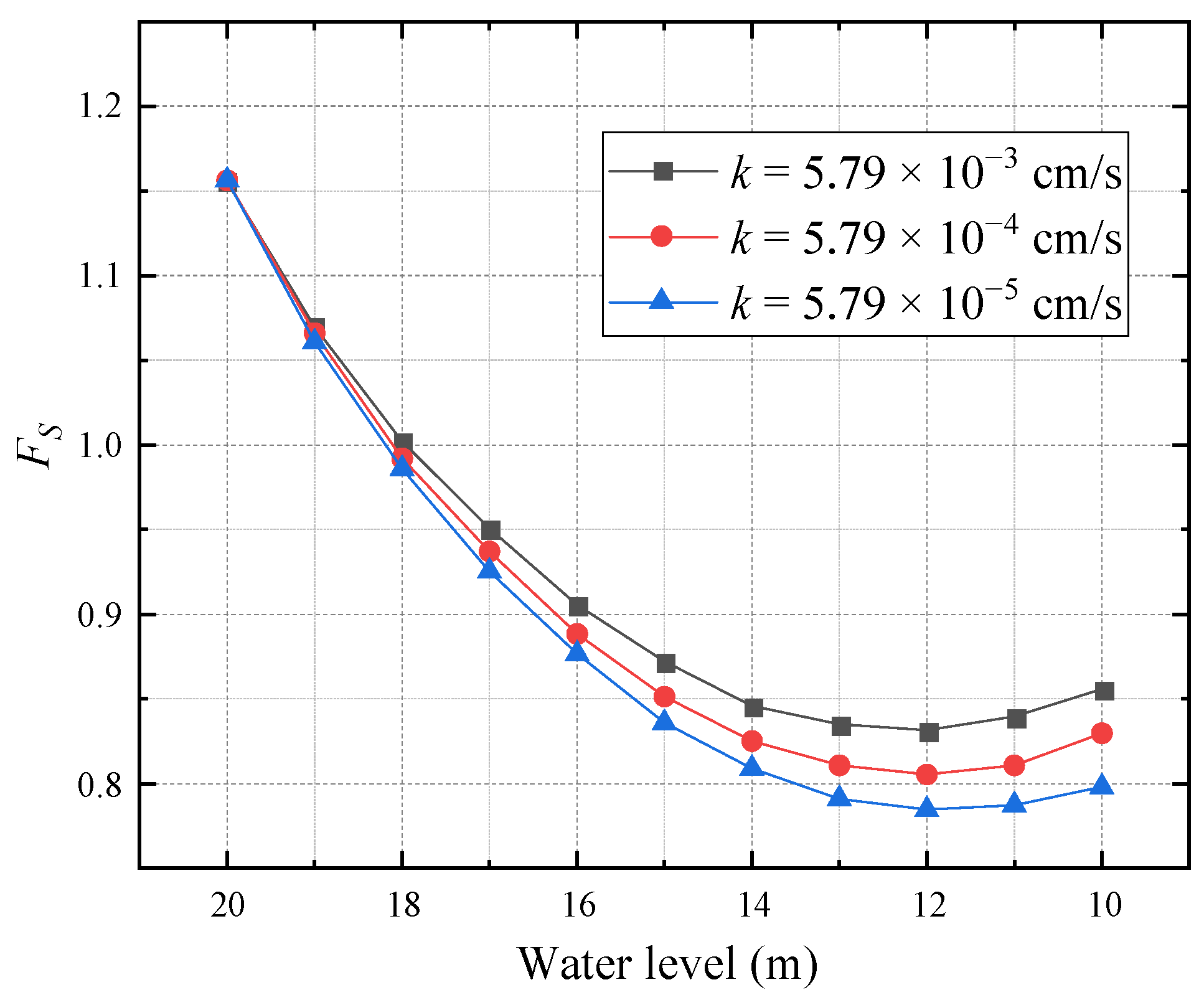
4.3. Effect of Permeability Coefficient
5. Conclusions
- The studies herein demonstrate that both the increase and decrease in the reservoir water level will significantly impact the stability of the reservoir slope. With the rising reservoir water level, the minimum FS of silt slope and clay slope will increase, and the possible slide body will be also be larger. It can be argued that the rising reservoir water level significantly increases the hydrostatic pressure, which is beneficial for stability, compared to the unfavourable seepage force which increases less relatively due to the hysteresis of the seepage field. When the reservoir water level drops, the minimum Fs will decrease significantly. But, with the lower water level, the minimum Fs will decrease slowly and then increase slightly after reaching the most dangerous water level. Due to the relative hysteresis of the seepage changes, the phreatic surface will continue to decrease after reaching the lowest reservoir water level, which leads to a slight increase in the minimum FS of the reservoir slope.
- In the process of the reservoir water level rising and falling, the fluctuation rate of water level and soil permeability coefficient could influence the reservoir slope stability. With a higher change rate of water level rising or falling and a smaller permeability coefficient, the hysteresis effect of seepage will be more serious, and the corresponding minimum Fs will increase or decrease more rapidly.
- However, the proposed method in this study did not consider the influence of the matric suction of the saturated–unsaturated area. Compared to the results that consider the matric suction effect in the same slope model, the minimum FS results are higher during the water level rising and lower during the water level falling. This illustrates that the matric suction has a great influence on the stability of the slope. Therefore, it is necessary to figure out the change and effect of matric suction inside the slope in further studies for stability analysis.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nakamura, H.; Wang, G. Study on landslide in reservior area. Bull. Soil Water Conserv. 1990, 10, 53–64. [Google Scholar] [CrossRef]
- Li, X. Stability Analysis of Colluvial Landslide in Relation to Variation of Water Level in Wanzhou District, Three Gorges Reservoir Doctor; China University of Geosciences: Wuhan, China, 2021. [Google Scholar]
- Michalowski, R.L.; Nadukuru, S.S. Three-Dimensional Limit Analysis of Slopes with Pore Pressure. J. Geotech. Geoenviron. Eng. 2013, 139, 1604–1610. [Google Scholar] [CrossRef]
- Rao, P.; Zhao, L.; Chen, Q.; Nimbalkar, S. Three-dimensional slope stability analysis incorporating coupled effects of pile reinforcement and reservoir drawdown. Int. J. Geomech. 2019, 19, 6019002. [Google Scholar] [CrossRef]
- Viratjandr, C.; Michalowski, R.L. Limit analysis of submerged slopes subjected to water drawdown. Can. Geotech. J. 2006, 43, 802–814. [Google Scholar] [CrossRef]
- Meng, Q.; Qian, K.; Zhong, L.; Gu, J.; Li, Y.; Fan, K.; Yan, L. Numerical analysis of slope stability under reservoir water level fluctuations using a FEM-LEM-combined method. Geofluids 2020, 2020, 6683311. [Google Scholar] [CrossRef]
- Zhou, X.-P.; Wei, X.; Liu, C.; Cheng, H. Three-dimensional stability analysis of bank slopes with reservoir drawdown based on rigorous limit equilibrium method. Int. J. Geomech. 2020, 20, 4020229. [Google Scholar] [CrossRef]
- Kafle, L.; Xu, W.-J.; Zeng, S.-Y.; Nagel, T. A numerical investigation of slope stability influenced by the combined effects of reservoir water level fluctuations and precipitation: A case study of the Bianjiazhai landslide in China. Eng. Geol. 2022, 297, 106508. [Google Scholar] [CrossRef]
- Sun, L.; Tang, X.; Abdelaziz, A.; Liu, Q.; Grasselli, G. Stability analysis of reservoir slopes under fluctuating water levels using the combined finite-discrete element method. Acta Geotech. 2023, 18, 5403–5426. [Google Scholar] [CrossRef]
- Zhang, G.; Lu, G.; Xia, C.; Bai, D.; Liu, T. A Time-Scale Varying Finite Difference Method for Analyzing the Influence of Rainfall and Water Level on the Stability of a Bank Slope. Appl. Sci. Basel 2023, 13, 5268. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, W.; Zhang, L.; Zhu, Z.; Hu, J.; Wei, H. Probabilistic stability analyses of undrained slopes by 3D random fields and finite element methods. Geosci. Front. 2018, 9, 1657–1664. [Google Scholar] [CrossRef]
- Wang, M.-Y.; Liu, Y.; Ding, Y.-N.; Yi, B.-L. Probabilistic stability analyses of multi-stage soil slopes by bivariate random fields and finite element methods. Comput. Geotech. 2020, 122, 103529. [Google Scholar] [CrossRef]
- Griffiths, D.V. Slope stability analysis by finite elements. Géotechnique 1999, 49, 387–403. [Google Scholar] [CrossRef]
- Mandal, A.K.; Li, X.; Shrestha, R. Influence of Water Level Rise on the Bank of Reservoir on Slope Stability: A Case Study of Dagangshan Hydropower Project. Geotech. Geol. Eng. 2019, 37, 5187–5198. [Google Scholar] [CrossRef]
- Zhang, W.J.; Zhan, L.T.; Ling, D.S.; Chen, Y.M. Influence of reservoir water level fluctuations on stability of unsaturated soil banks. J. Zhejiang Univ. Eng. Sci. 2006, 40, 1365–1370. [Google Scholar] [CrossRef]
- Zhang, X.; Dai, Z. Analysis of slope stability under seepage by using ABAQUS program. Chin. J. Rock Mech. Eng. 2010, 29, 2927–2934. [Google Scholar]
- Mao, J.-Z.; Guo, J.; Fu, Y.; Zhang, W.-P.; Ding, Y.-N. Effects of Rapid Water-Level Fluctuations on the Stability of an Unsaturated Reservoir Bank Slope. Adv. Civ. Eng. 2020, 2020, 2360947. [Google Scholar] [CrossRef]
- Zheng, Y.; Shi, W.; Kong, W. Calculation of seepage forces and phreatic surface under drawdown conditions. Chin. J. Rock Mech. Eng. 2004, 23, 3203. [Google Scholar] [CrossRef]
- Zheng, Y.; Tang, X. Stability analysis of slopes under drawdown condition of reservoirs. Chin. J. Geotech. Eng. 2007, 29, 1115–1121. [Google Scholar] [CrossRef]
- Sun, G.; Yang, Y.; Cheng, S.; Zheng, H. Phreatic line calculation and stability analysis of slopes under the combined effect of reservoir water level fluctuations and rainfall. Can. Geotech. J. 2017, 54, 631–645. [Google Scholar] [CrossRef]
- Deng, D.; Li, L.; Zhao, L. A new method for analysis of slope stability under steady seepage. Chin. J. Eng. Geol. 2011, 19, 29–36. [Google Scholar] [CrossRef]
- Lu, Y.; Chen, X. Solution for stability of soil slopes subjected to seepage of groundwater via simplified Bishop Method. Chin. J. Appl. Mech. 2018, 35, 524–529+687. [Google Scholar] [CrossRef]
- Chen, Z.-Y. Random trials used in determining global minimum factors of safety of slopes. Can. Geotech. J. 1992, 29, 225–233. [Google Scholar] [CrossRef]
- Li, S.; Shangguan, Z.; Duan, H.; Liu, Y.; Luan, M. Searching for critical failure surface in slope stability analysis by using hybrid genetic algorithm. Geomech. Eng. 2009, 1, 85–96. [Google Scholar] [CrossRef]
- Zolfaghari, A.R.; Heath, A.C.; McCombie, P.F. Simple genetic algorithm search for critical non-circular failure surface in slope stability analysis. Comput. Geotech. 2005, 32, 139–152. [Google Scholar] [CrossRef]
- Liu, Q.Q.; Li, J.C. Effects of Water Seepage on the Stability of Soil-slopes. Procedia IUTAM 2015, 17, 29–39. [Google Scholar] [CrossRef]
- Gao, W. Determination of the Noncircular Critical Slip Surface in Slope Stability Analysis by Meeting Ant Colony Optimization. J. Comput. Civ. Eng. 2016, 30, 06015001. [Google Scholar] [CrossRef]
- Kahatadeniya, K.S.; Nanakorn, P.; Neaupane, K.M. Determination of the critical failure surface for slope stability analysis using ant colony optimization. Eng. Geol. 2009, 108, 133–141. [Google Scholar] [CrossRef]
- Khajehzadeh, M.; Taha, M.R.; El-Shafie, A.; Eslami, M. Stability assessment of earth slope using modified particle swarm optimization. Chin. J. Inst. Eng. 2014, 37, 79–87. [Google Scholar] [CrossRef]
- Shinoda, M.; Miyata, Y. PSO-based stability analysis of unreinforced and reinforced soil slopes using non-circular slip surface. Acta Geotech. 2018, 14, 907–919. [Google Scholar] [CrossRef]
- Himanshu, N.; Burman, A. Determination of Critical Failure Surface of Slopes Using Particle Swarm Optimization Technique Considering Seepage and Seismic Loading. Geotech. Geol. Eng. 2018, 37, 1261–1281. [Google Scholar] [CrossRef]
- Li, S.J.; Liu, Y.X.; He, X.; Liu, Y.J. Global search algorithm of minimum safety factor for slope stability analysis based on annealing simulation. Chin. J. Rock Mech. Eng. 2003, 22, 236. [Google Scholar] [CrossRef]
- Zhang, K.; Xu, Q.; Wang, Y.; A, H. Application of self-adaptive differnetial evolution algorithm in searching for critical slip surface of slope. Rock Soil Mech. 2017, 38, 1503–1509. [Google Scholar] [CrossRef]
- Cen, W.J.; Luo, J.R.; Yu, J.S.; Shamin Rahman, M. Slope Stability Analysis Using Genetic Simulated Annealing Algorithm in Conjunction with Finite Element Method. KSCE J. Civ. Eng. 2019, 24, 30–37. [Google Scholar] [CrossRef]
- Biniyaz, A.; Azmoon, B.; Liu, Z. Coupled transient saturated–unsaturated seepage and limit equilibrium analysis for slopes: Influence of rapid water level changes. Acta Geotech. 2021, 17, 2139–2156. [Google Scholar] [CrossRef]
- Jin, L.X.; Wei, J.J.; Luo, C.W. Slope stability analysis based on improved radial movement optimization considering seepage effect. Alex. Eng. J. 2023, 79, 591–607. [Google Scholar] [CrossRef]
- Rahmani, R.; Yusof, R. A new simple, fast and efficient algorithm for global optimization over continuous search-space problems: Radial Movement Optimization. Appl. Mech. Mater. 2014, 248, 287–300. [Google Scholar] [CrossRef]
- Pan, Z.F.; Jin, L.X.; Chen, W.S. Improved radial movement optimization algorithm for slope stability analysis. Rock Soil Mech. 2016, 37, 2080–2084. [Google Scholar] [CrossRef]
- Jin, L.; Feng, Q.; Pan, Z. Slope Stability Analysis Based on Morgenstern-Price Method and Improved Radial Movement Optimization Algorithm. China J. Highw. Transp. 2018, 31, 39–47. [Google Scholar] [CrossRef]
- Jin, L.; Feng, Q. Improved radial movement optimization to determine the critical failure surface for slope stability analysis. Environ. Earth Sci. 2018, 77, 564. [Google Scholar] [CrossRef]
- Pham, H.T.V.; Fredlund, D.G. The application of dynamic programming to slope stability analysis. Can. Geotech. J. 2003, 40, 830–847. [Google Scholar] [CrossRef]
- Qin, J. Analysis and Improved of Classical Slope Stability Methods in Seepage Soil Mass. Master’s Thesis, Tianjin University, Tianjin, Chiana, 2014. [Google Scholar]
- Chen, Y.; Wei, X.; Yuchao, K. Location non-circular critical slip surfaces by particle swarm optimization algorithm. Chin. J. Rock Mech. Eng. 2006, 25, 1443. [Google Scholar] [CrossRef]
- Cheng, Y.M.; Li, L.; Chi, S.C. Performance studies on six heuristic global optimization methods in the location of critical slip surface. Comput. Geotech. 2007, 34, 462–484. [Google Scholar] [CrossRef]
- Singh, J.; Banka, H.; Verma, A.K. A BBO-based algorithm for slope stability analysis by locating critical failure surface. Neural Comput. Appl. 2018, 31, 6401–6418. [Google Scholar] [CrossRef]
- Jiang, Z. Improvement of Critical Slip Field Method of Slope and Development of Its Computing System. Ph.D. Thesis, Hefei University of Technology, Hefei, China, 2016. [Google Scholar]
- Shi, D. Numerical Analysis of Binary Structure Slope Stability in Condition of Reservoir Water Level Fluctuation. Master’s Thesis, Kunming University of Science and Technology, Kunming, China, 2020. [Google Scholar]

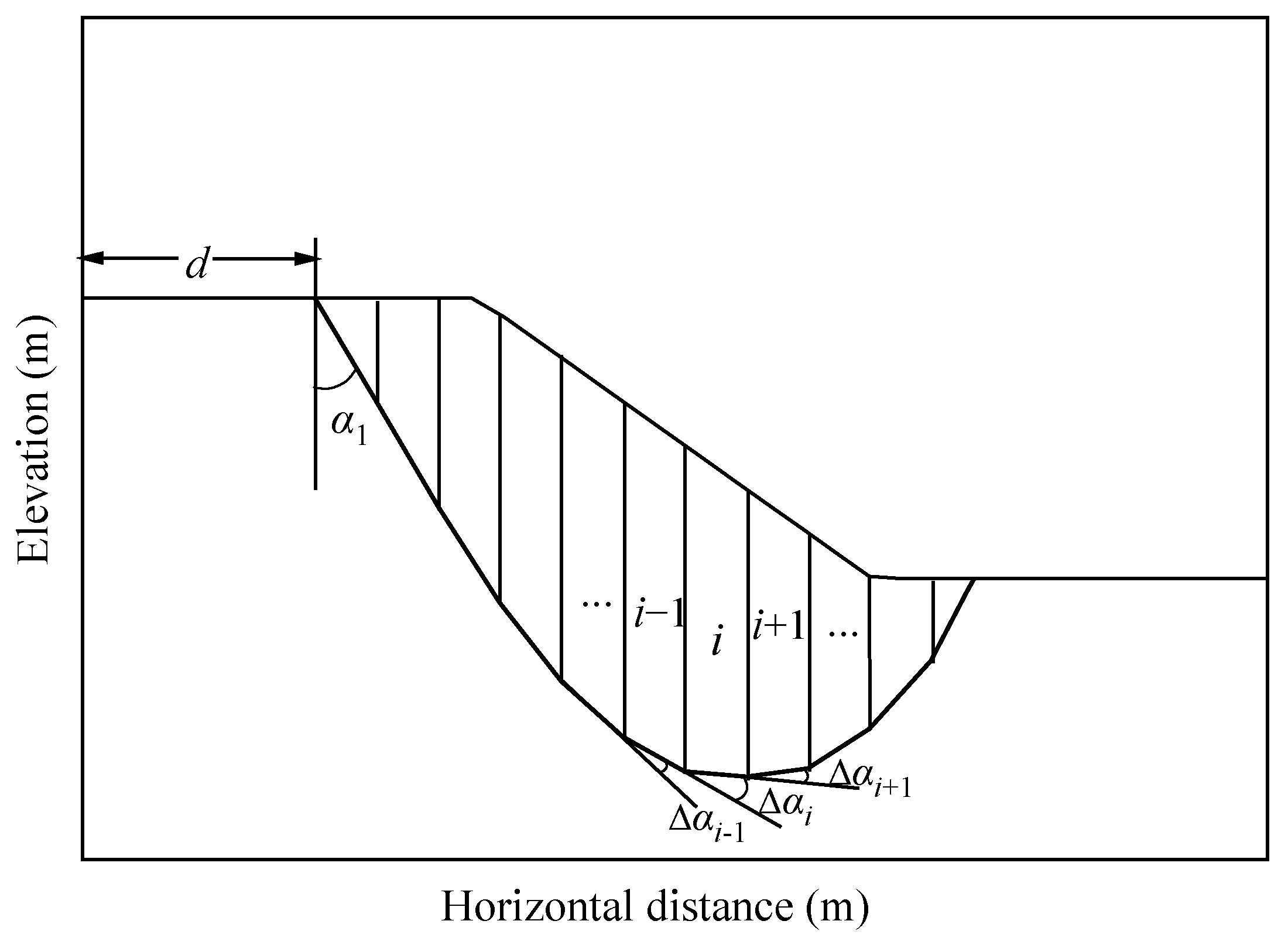
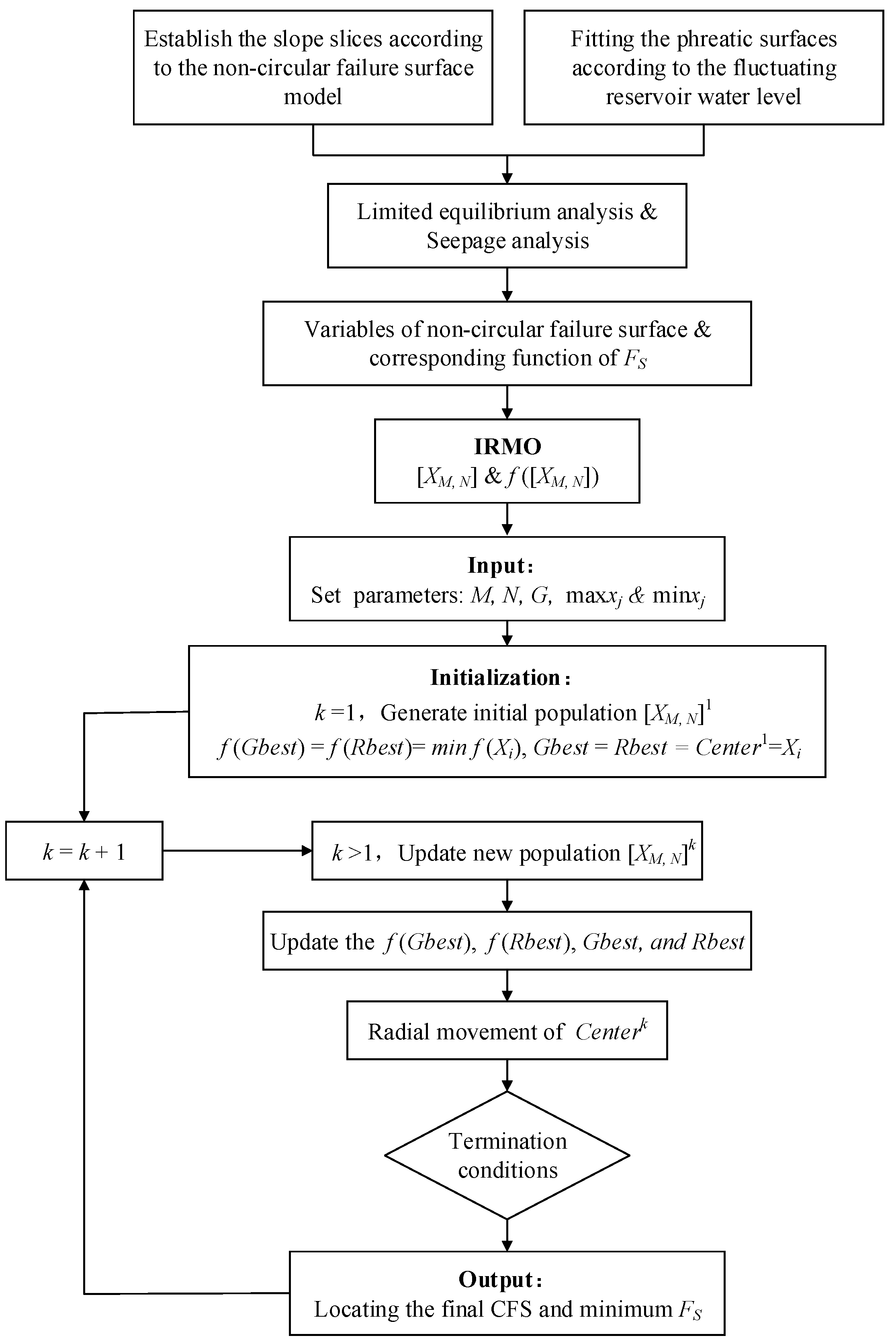






| Reference | LEMs | Seepage Analysis Methods | CFSs | Optimisation Techniques |
|---|---|---|---|---|
| Zolfaghari et al. [25] | Morgenstern–Price | Pore–water pressure from distribution steady seepage | Non-circular | GA |
| Himanshu et al. [31] | Bishop | Pore–water pressure from distribution steady seepage | Circular | PSO |
| Biniyaz et al. [35] | Simplified Bishop | Transient pore–water pressure distribution from saturated–unsaturated flow | Circular | FEM (FEniCS) |
| Liu et al. [26] | Spencer | One-dimensional groundwater flow in the unconfined aquifer | Non-circular (spline curve) | GA |
| Jin et al. [36] | Rigorous Janbu | Infiltration analysis from two-dimensional steady seepage | Non-circular | IRMO |
| Cases | Reference | Optimisation Methods | LEMs | Minimum FS | Error |
|---|---|---|---|---|---|
| Homogeneous slope case | Pham and Fredlund [41] | SLOPE/W | Morgenstern–Price | 1.168 | −13.8% |
| SLOPE/W | Simplified Bishop | 1.167 | −13.7% | ||
| Qin [42] | Fortran | Fellenius | 1.070 | −12.9% | |
| Fortran | Simplified Bishop | 1.185 | −33.5% | ||
| Fortran | Rigorous Janbu | 1.178 | −32.3% | ||
| Jin et al. [36] | IRMO | Rigorous Janbu | 1.007 | Average in −21.2% | |
| Inhomogeneous slope case with two-layered soil | Pham and Fredlund [41] | SLOPE/W | Morgenstern–Price | 1.485 | −8.9% |
| SLOPE/W | Simplified Bishop | 1.483 | −8.8% | ||
| Qin [42] | Fortran | Fellenius | 1.376 1.489 | −1.7% | |
| Fortran | Bishop | −9.1% | |||
| Jin et al. [36] | IRMO | Rigorous Janbu | 1.353 | Average in −7.1% | |
| Inhomogeneous slope case with a weak inter-layer | Pham and Fredlund [41] | SLOPE/W | Morgenstern–Price | 1.140 | −7.7% |
| SLOPE/W | Simplified Bishop | 1.125 | −10.9% | ||
| Chen et al. [43] | PSO & FEM | - | 1.053 | −0.1% | |
| Jin et al. [36] | IRMO | Rigorous Janbu | 1.052 | Average in −6.2% | |
| Inhomogeneous slope case in four soil layered | Zolfaghari et al. [25] | GA | Morgenstern–Price | 1.360 | −10.6% |
| Cheng et al. [44] | SA | Spencer | 1.284 | −5.3% | |
| GA | Spencer | 1.232 | −1.3% | ||
| PSO | Spencer | 1.210 | +0.5% | ||
| Tabu search | Spencer | 1.343 | −10.4% | ||
| ACO | Spencer | 1.449 | −16.1% | ||
| Kahatadeniya et al. [28] | ACO | Morgenstern–Price | 1.377 | −11.7% | |
| Khajehzadeh et al. [29] | PSO | Morgenstern–Price | 1.203 | +1.1% | |
| MPSO | Morgenstern–Price | 1.171 | +3.8% | ||
| Singh et al. [45] | BBO | Bishop | 1.348 | −9.8% | |
| BBO | Fellenius | 1.226 | −0.8% | ||
| BBO | Janbu | 2.103 | −42.2% | ||
| BBO | Janbu corrected | 2.104 | −42.2% | ||
| Jin et al. [36] | IRMO | Rigorous Janbu | 1.216 | Average in −11.2% |
| Optimisation Algorithms | Minimum Fs | Standard Deviation | Average CPU Time (ms) | |||
|---|---|---|---|---|---|---|
| Maximum | Minimum | Average | ||||
| Homogeneous slope case [36,41] | IRMO | 1.0092 | 1.0042 | 1.0066 | 0.0013 | 755.85 |
| RMO | 1.0302 | 1.0116 | 1.0173 | 0.0048 | 713.90 | |
| DE | 1.0715 | 1.0342 | 1.0571 | 0.0092 | 431.30 | |
| PSO | 1.0890 | 1.0293 | 1.0594 | 0.0196 | 2104.05 | |
| Inhomogeneous slope case with a weak inter-layer [36,43] | IRMO | 1.0775 | 1.0412 | 1.0638 | 0.0093 | 665.50 |
| RMO | 1.1497 | 1.0341 | 1.0932 | 0.0249 | 643.20 | |
| DE | 1.1965 | 1.1048 | 1.1395 | 0.0216 | 395.90 | |
| PSO | 1.2978 | 1.1138 | 1.1962 | 0.0505 | 2087.60 | |
| IRMO Algorithm: |
|---|
Input:
|
Initialisation:
|
Evolution:
|
Output:
|
| Population Size (M) | Number of Variables (N) | Generations (G) | Coefficients | ||
|---|---|---|---|---|---|
| C1 | C2 | ||||
| IRMO | 250 | 50 | 250 | 0.4 | 0.5 |
| Reference | Computation Techniques | Seepage Analysis | Slope Stability Analysis | Critical Failure Surface | Minimum FS |
|---|---|---|---|---|---|
| Pham and Fredlund [41] | FlexPDS | Distribution of pore–water pressures | DNYPROG (μ = 0.48) | Non-circular | 1.187 |
| FlexPDS | DNYPROG (μ = 0.33) | Non-circular | 1.041 | ||
| SIGMA/W and SEEP/W | Enhanced (μ = 0.48) | Circular | 1.171 | ||
| SIGMA/W and SEEP/W | Enhanced (μ = 0.33) | Circular | 1.132 | ||
| SLOPE/W | Morgenstern–Price | Circular | 1.168 | ||
| SLOPE/W | Simplified Bishop | Circular | 1.167 | ||
| Qin [42] | Fortran | Considering seepage force | Fellenius | Circular | 1.070 |
| Simplified Bishop | Circular | 1.185 | |||
| Rigorous Janbu | Circular | 1.178 | |||
| This study | IRMO | Without considering seepage force | Rigorous Janbu | Non-circular | 1.181 |
| Considering seepage force | Rigorous Janbu | Non-circular | 1.007 |
| Soil | γ (kN/m3) | c (kPa) | φ (°) | Permeability Coefficient k (m/s) |
|---|---|---|---|---|
| clay | 20.2 | 10 | 20 | 5.4 × 10−7 |
| silty | 20.2 | 5 | 30 | 5.4 × 10−6 |
| Soil | γ (kN/m3) | c (kPa) | φ (°) |
|---|---|---|---|
| Upper silt layer | 20 | 10 | 25 |
| Limestone bedrock | 25 | 67 | 42 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, L.; Luo, C.; Wei, J.; Liu, P. Reservoir Slope Stability Analysis under Dynamic Fluctuating Water Level Using Improved Radial Movement Optimisation (IRMO) Algorithm. Mathematics 2024, 12, 2055. https://doi.org/10.3390/math12132055
Jin L, Luo C, Wei J, Liu P. Reservoir Slope Stability Analysis under Dynamic Fluctuating Water Level Using Improved Radial Movement Optimisation (IRMO) Algorithm. Mathematics. 2024; 12(13):2055. https://doi.org/10.3390/math12132055
Chicago/Turabian StyleJin, Liangxing, Chunwa Luo, Junjie Wei, and Pingting Liu. 2024. "Reservoir Slope Stability Analysis under Dynamic Fluctuating Water Level Using Improved Radial Movement Optimisation (IRMO) Algorithm" Mathematics 12, no. 13: 2055. https://doi.org/10.3390/math12132055
APA StyleJin, L., Luo, C., Wei, J., & Liu, P. (2024). Reservoir Slope Stability Analysis under Dynamic Fluctuating Water Level Using Improved Radial Movement Optimisation (IRMO) Algorithm. Mathematics, 12(13), 2055. https://doi.org/10.3390/math12132055