A Novel Solution Approach for Time-Fractional Hyperbolic Telegraph Differential Equation with Caputo Time Differentiation
Abstract
1. Introduction
2. Preliminaries Results
- ;
- ;
- , for any ;
- ;
- (1)
- (2)
3. Construction of MFS-ASs via Laplace FPSM
- ▪
- , and for , and .
- ▪
- , and for , and .
- ▪
- , and , for , , and .
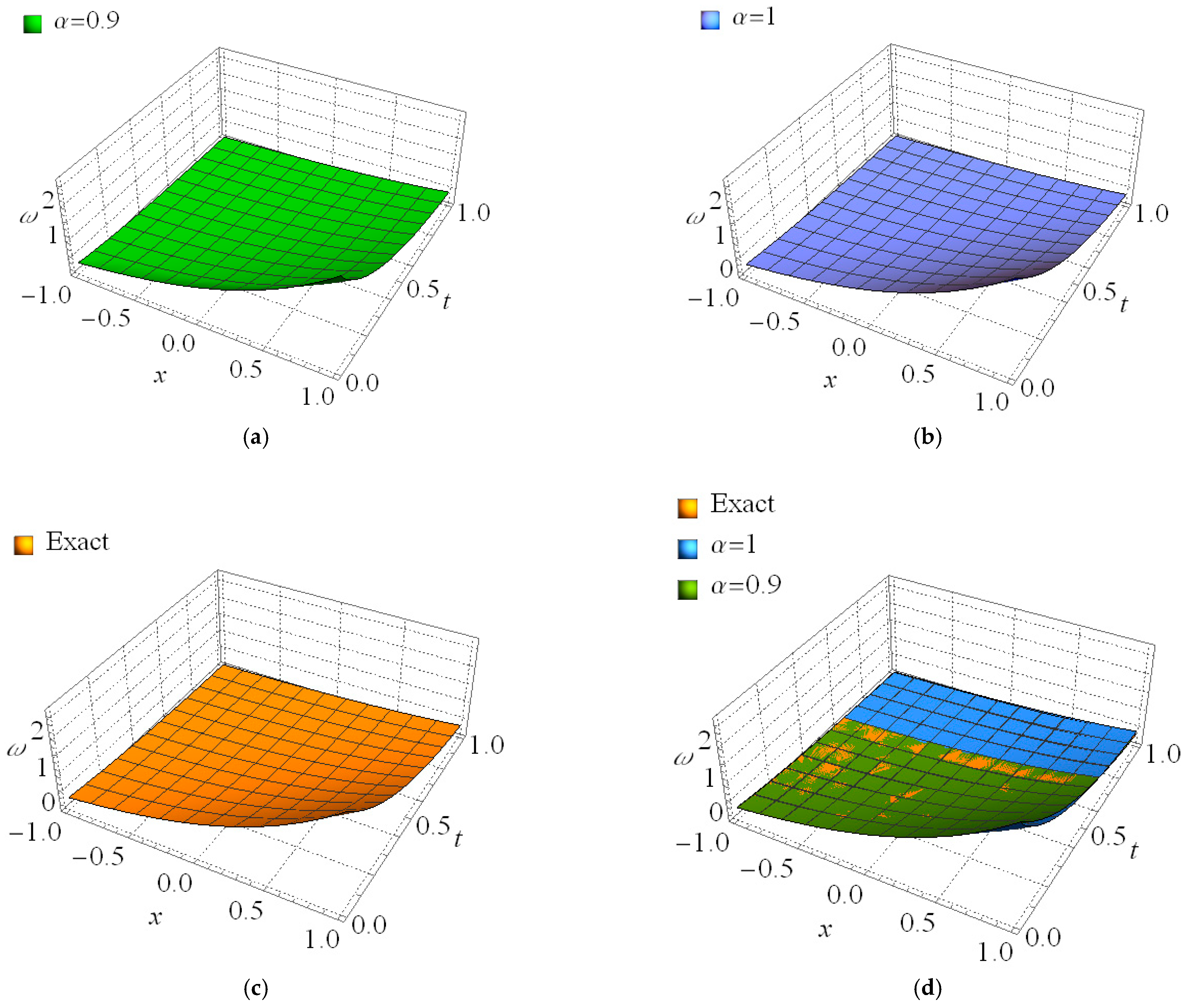
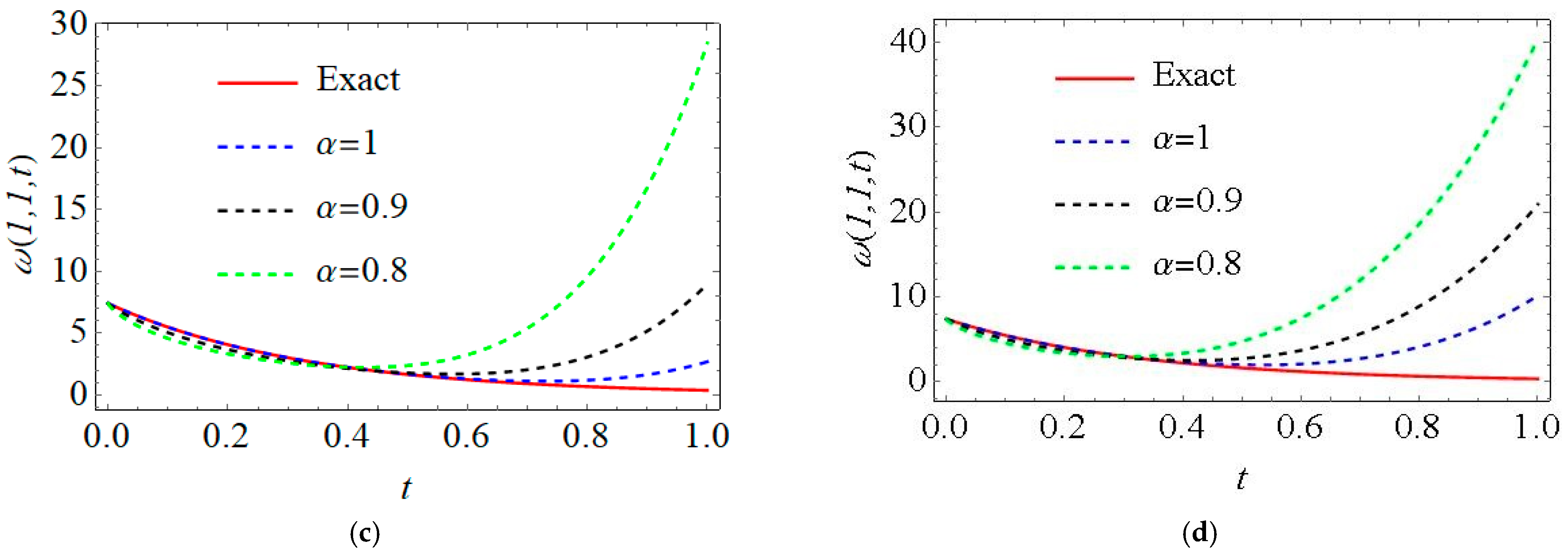
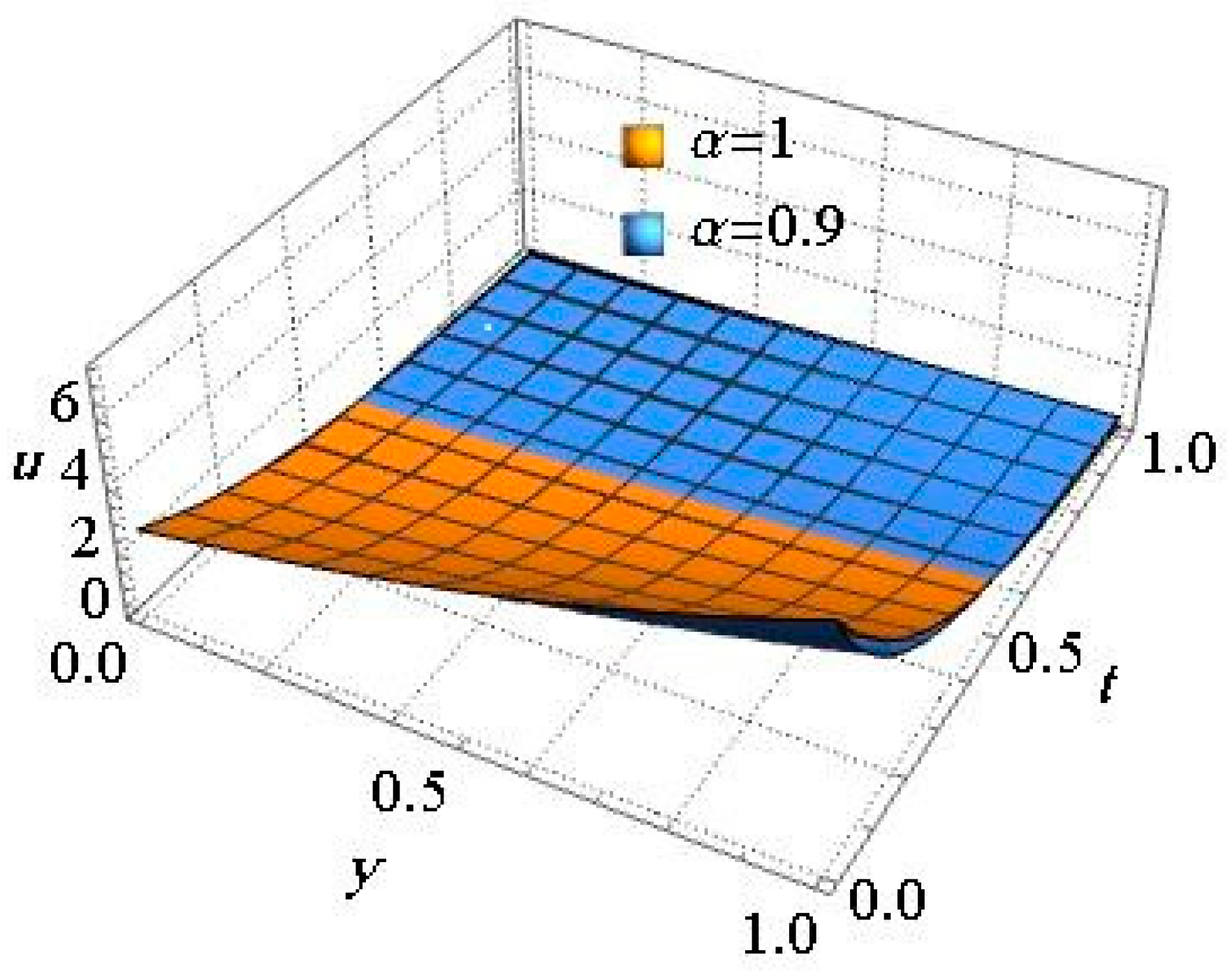
4. Numerical Experiments
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Oldham, K.; Spanier, J. The Fractional Calculus: Theory and Applications of Differentiation and Integration to Arbitrary Order; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Baleanu, D.; Machado, J.A.T.; Luo, A.C. Fractional Dynamics and Control; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Bendouma, B. Monotone Iterative Technique for a Coupled System of Nonlinear Conformable Fractional Dynamic Equations on Time Scales. Jordan J. Math. Stat. JJMS 2023, 16, 41–55. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D. New fractional derivatives with non-local and non-singular kernel: Theory and application to heat transfer model. Therm. Sci. 2016, 20, 763–769. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Progr. Fract. Differ. Appl. 2015, 1, 73–85. [Google Scholar]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity; Imperial College Press: London, UK, 2010. [Google Scholar]
- Podlubny, I. Fractional Differential Equation; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Singh, J.; Kumar, D.; Baleanu, D. On the analysis of fractional diabetes model with exponential law. Adv. Differ. Equ. 2018, 231, 231. [Google Scholar] [CrossRef]
- Khan, H.; Shah, R.; Kumam, P.; Arif, M. Analytical solutions of fractional-order heat and wave equations by the natural transform decomposition method. Entropy 2019, 21, 597. [Google Scholar] [CrossRef]
- Shokhanda, R.; Goswami, P.; He, J.-H.; Althobaiti, A. An Approximate Solution of the Time-Fractional Two-Mode Coupled Burgers Equation. Fractal Fract. 2021, 5, 196. [Google Scholar] [CrossRef]
- Amin, A.Z.; Lopes, A.M.; Hashim, I. A space-time spectral collocation method for solving the variable-order fractional Fokker-Planck equation. J. Appl. Anal. Comput. 2023, 13, 969–985. [Google Scholar] [CrossRef]
- Yavuz, M.; Ozdemir, N. Numerical inverse Laplace homotopy technique for fractional heat equations. Therm. Sci. 2018, 22, 185–194. [Google Scholar] [CrossRef]
- Safari, M.; Ganji, D.D.; Moslemi, M. Application of He’s variational iteration method and Adomian’s decomposition method to the fractional KdV–Burgers–Kuramoto equation. Comput. Math. Appl. 2009, 58, 2091–2097. [Google Scholar] [CrossRef]
- Singh, J.; Kumar, D.; Baleanu, D.; Rathore, S. An efficient numerical algorithm for the fractional Drinfeld–Sokolov–Wilson equation. Appl. Math. Comput. 2018, 335, 12–24. [Google Scholar] [CrossRef]
- Shah, R.; Khan, H.; Arif, M.; Kumam, P. Application of Laplace–Adomian decomposition method for the analytical solution of third-order dispersive fractional partial differential equations. Entropy 2019, 21, 335. [Google Scholar] [CrossRef]
- Dahmani, Z.; Anber, A.; Jebril, I. Solving Conformable Evolution Equations by an Extended Numerical Method. Jordan, J. Math. Stat. JJMS 2022, 15, 363–380. [Google Scholar] [CrossRef]
- Srivastava, V.K.; Awasthi, M.K.; Tamsir, M. RDTM solution of Caputo time fractional-order hyperbolic telegraph equation. AIP Adv. 2013, 3, 032142. [Google Scholar] [CrossRef]
- Khan, H.; Shah, R.; Baleanu, D.; Kumam, P.; Arif, M. Analytical solution of fractional-order hyperbolic telegraph equation, using natural transform decomposition method. Electronics 2019, 8, 1015. [Google Scholar] [CrossRef]
- Azhar, O.F.; Naeem, M.; Mofarreh, F.; Kafle, J. Numerical Analysis of the Fractional-Order Telegraph Equations. J. Funct. Spaces 2021, 2021, 2295804. [Google Scholar] [CrossRef]
- Ali, I.; Khan, H.; Farooq, U.; Baleanu, D.; Kumam, P.; Arif, M. An approximate-analytical solution to analyze fractional view of telegraph equations. IEEE Access 2020, 8, 25638–25649. [Google Scholar] [CrossRef]
- Das, S.; Gupta, P.K. Homotopy analysis method for solving fractional hyperbolic partial differential equations. Int. J. Comput. Math. 2011, 88, 578–588. [Google Scholar] [CrossRef]
- Harrouche, N.; Momani, S.; Hasan, S.; Al-Smadi, M. Computational algorithm for solving drug pharmacokinetic model under uncertainty with nonsingular kernel type Caputo-Fabrizio fractional derivative. Alex. Eng. J. 2021, 60, 4347–4362. [Google Scholar] [CrossRef]
- Abassy, T.A.; El-Tawil, M.A.; El-Zoheiry, H. Toward a modified variational iteration method. J. Comput. Appl. Math. 2007, 207, 137–147. [Google Scholar] [CrossRef]
- Momani, S.; Freihat, A.; Al-Smadi, M. Analytical study of fractional-order multiple chaotic Fitzhugh-Nagumo neurons model using multistep generalized differential transform method. Abstr. Appl. Anal. 2014, 2014, 276279. [Google Scholar] [CrossRef]
- Aljarrah, H.; Alaroud, M.; Ishak, A.; Darus, M. Adaptation of Residual-Error Series Algorithm to Handle Fractional System of Partial Differential Equations. Mathematics 2021, 9, 2868. [Google Scholar] [CrossRef]
- Freihet, A.; Hasan, S.; Alaroud, M.; Al-Smadi, M.; Ahmad, R.R.; Salma Din, U.K. Toward computational algorithm for time-fractional Fokker–Planck models. Adv. Mech. Eng. 2019, 11, 1–11. [Google Scholar] [CrossRef]
- Bataineh, M.; Alaroud, M.; Al-Omari, S.; Agarwal, P. Series Representations for Uncertain Fractional IVPs in the Fuzzy Conformable Fractional Sense. Entropy 2021, 23, 1646. [Google Scholar] [CrossRef] [PubMed]
- Khirsariya, S.; Rao, S.; Chauhan, J. Semi-analytic solution of time-fractional Korteweg-de Vries equation using fractional residual power series method. Results Nonlinear Anal. 2022, 5, 222–234. [Google Scholar] [CrossRef]
- Arafa, A.; Elmahdy, G. Application of residual power series method to fractional coupled physical equations arising in fluids flow. Int. J. Differ. Equ. 2018, 2018, 7692849. [Google Scholar] [CrossRef]
- Shqair, M.; Al-Smadi, M.; Momani, S.; El-Zahar, E. Adaptation of Conformable Residual Power Series Scheme in Solving Nonlinear Fractional Quantum Mechanics Problems. Appl. Sci. 2020, 10, 890. [Google Scholar] [CrossRef]
- Modanli, M.; Abdulazeez, S.T.; Husien, A.M. A residual power series method for solving pseudo hyperbolic partial differential equations with nonlocal conditions. Numer. Methods Partial. Differ. Equ. 2021, 37, 2235–2243, . [Google Scholar] [CrossRef]
- El-Ajou, A. Adapting the Laplace transform to create solitary solutions for the nonlinear time-fractional dispersive PDEs via a new approach. Eur. Phys. J. Plus 2021, 136, 229. [Google Scholar] [CrossRef]
- Alaroud, M. Application of Laplace residual power series method for ASs of fractional IVP’s. Alex. Eng. J. 2022, 61, 1585–1595. [Google Scholar] [CrossRef]
- Alaroud, M.; Alomari, A.-K.; Tahat, N.; Ishak, A. Analytical Computational Scheme for Multivariate Nonlinear Time-Fractional Generalized Biological Population Model. Fractal Fract. 2023, 7, 176. [Google Scholar] [CrossRef]
- Alaroud, M.; Ababneh, O.; Tahat, N.; Al-Omari, S. Analytic technique for solving temporal time-fractional gas dynamics equations with Caputo fractional derivative. AIMS Math 2022, 7, 17647–17669. [Google Scholar] [CrossRef]
- Aljarrah, H.; Alaroud, M.; Ishak, A.; Darus, M. AS of Nonlinear Time-Fractional PDEs by Laplace Residual Power Series Method. Mathematics 2022, 10, 1980. [Google Scholar] [CrossRef]
- Oqielat, M.A.N.; Eriqat, T.; Al-Zhour, Z.; Ogilat, O.; El-Ajou, A.; Hashim, I. Construction of fractional series solutions to nonlinear fractional reaction–diffusion for bacteria growth model via Laplace residual power series method. Int. J. Dyn. Control 2023, 11, 520–527. [Google Scholar] [CrossRef]
- Prakash, A.; Veeresha, P.; Prakasha, D.G.; Goyal, M.A. Homotopy technique for a fractional order multi-dimensional telegraph equation via the Laplace transform. Eur. Phys. J. Plus 2019, 134, 134. [Google Scholar] [CrossRef]




| 1.105171 | 1.105171 | |||
| 0.818731 | 0.818731 | |||
| 0.606531 | 0.606531 | |||
| 0.449329 | 0.449329 | |||
| 0.332871 | 0.332874 | |||
| 0.246597 | 0.246618 | |||
| 0.496585 | 0.496585 | |||
| 0.367879 | 0.367879 | |||
| 0.272532 | 0.272532 | |||
| 0.201897 | 0.201897 | |||
| 0.149569 | 0.14957 | |||
| 0.110803 | 0.110813 |
| 6th-MFS Solutions | |||||||
|---|---|---|---|---|---|---|---|
| 0.1 | 0.904837 | 0.904837 | 0.880178 | 0.825680 | 0.765968 | ||
| 0.3 | 0.606536 | 0.606531 | 0.583764 | 0.543398 | 0.515907 | ||
| 0.5 | 0.406764 | 0.406570 | 0.398782 | 0.398511 | 0.466931 | ||
| 0.7 | 0.274494 | 0.272532 | 0.286770 | 0.371405 | 0.745565 | ||
| 0.9 | 0.193599 | 0.182684 | 0.245210 | 0.556451 | 1.740233 | ||
| 0.1 | 0.740818 | 0.740818 | 0.720628 | 0.676004 | 0.627092 | ||
| 0.3 | 0.496590 | 0.496585 | 0.477923 | 0.444708 | 0.421253 | ||
| 0.5 | 0.333030 | 0.332871 | 0.326319 | 0.324557 | 0.371555 | ||
| 0.7 | 0.224737 | 0.223130 | 0.233829 | 0.294632 | 0.558375 | ||
| 0.9 | 0.158505 | 0.149569 | 0.196769 | 0.419641 | 1.253198 | ||
| Laplace FPSM | NDM [20] | ||||
|---|---|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alaroud, M.; Alomari, A.-K.; Tahat, N.; Al-Omari, S.; Ishak, A. A Novel Solution Approach for Time-Fractional Hyperbolic Telegraph Differential Equation with Caputo Time Differentiation. Mathematics 2023, 11, 2181. https://doi.org/10.3390/math11092181
Alaroud M, Alomari A-K, Tahat N, Al-Omari S, Ishak A. A Novel Solution Approach for Time-Fractional Hyperbolic Telegraph Differential Equation with Caputo Time Differentiation. Mathematics. 2023; 11(9):2181. https://doi.org/10.3390/math11092181
Chicago/Turabian StyleAlaroud, Mohammad, Abedel-Karrem Alomari, Nedal Tahat, Shrideh Al-Omari, and Anuar Ishak. 2023. "A Novel Solution Approach for Time-Fractional Hyperbolic Telegraph Differential Equation with Caputo Time Differentiation" Mathematics 11, no. 9: 2181. https://doi.org/10.3390/math11092181
APA StyleAlaroud, M., Alomari, A.-K., Tahat, N., Al-Omari, S., & Ishak, A. (2023). A Novel Solution Approach for Time-Fractional Hyperbolic Telegraph Differential Equation with Caputo Time Differentiation. Mathematics, 11(9), 2181. https://doi.org/10.3390/math11092181







