Spectral Collocation Technique for Solving Two-Dimensional Multi-Term Time Fractional Viscoelastic Non-Newtonian Fluid Model
Abstract
1. Introduction
2. Adopted Notation and Preliminary Concepts
2.1. Caputo Fractional Derivative
2.2. Brief Introduction to Shifted Legendre Polynomials
3. Solving the Time FNNFM
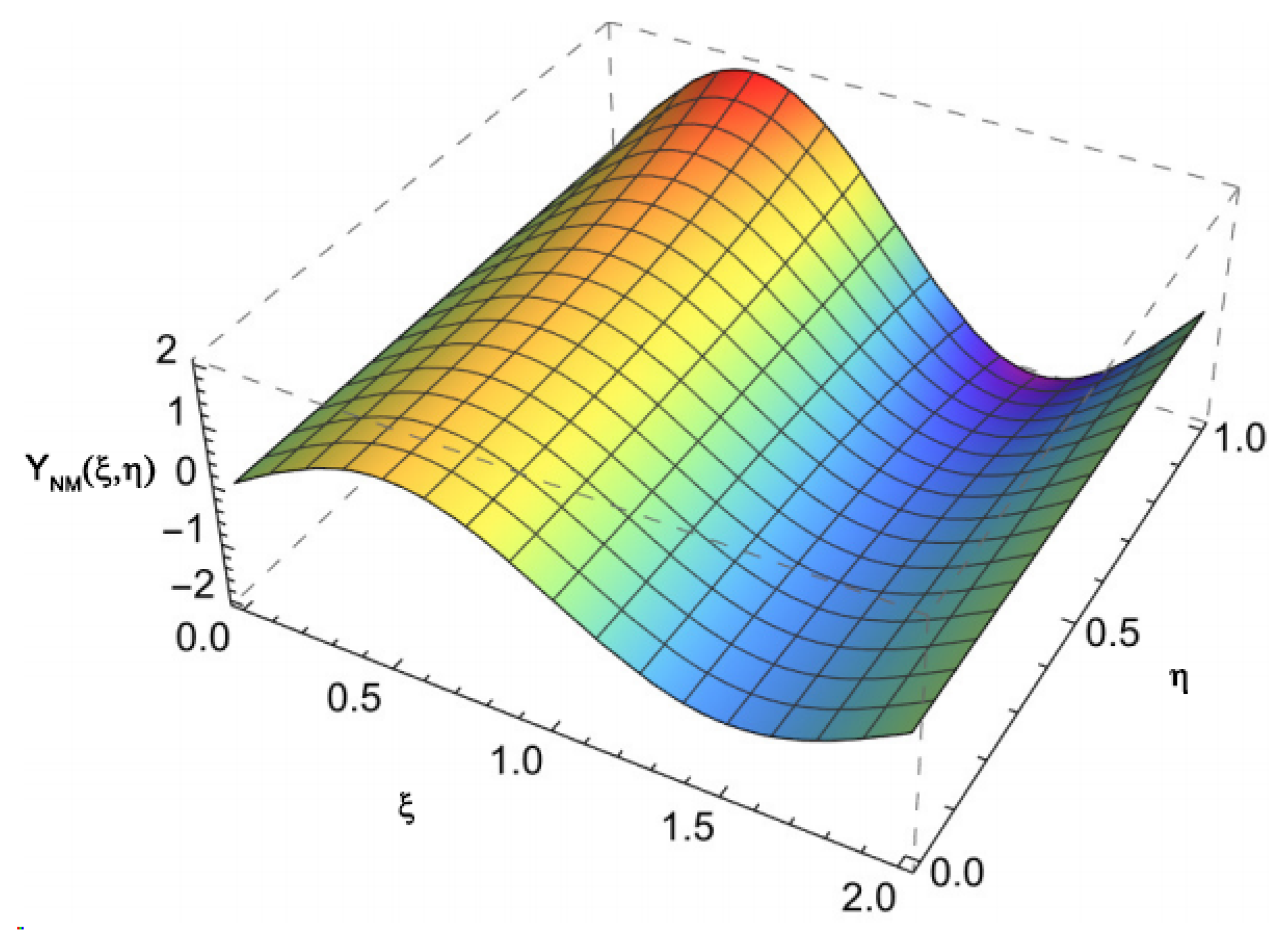
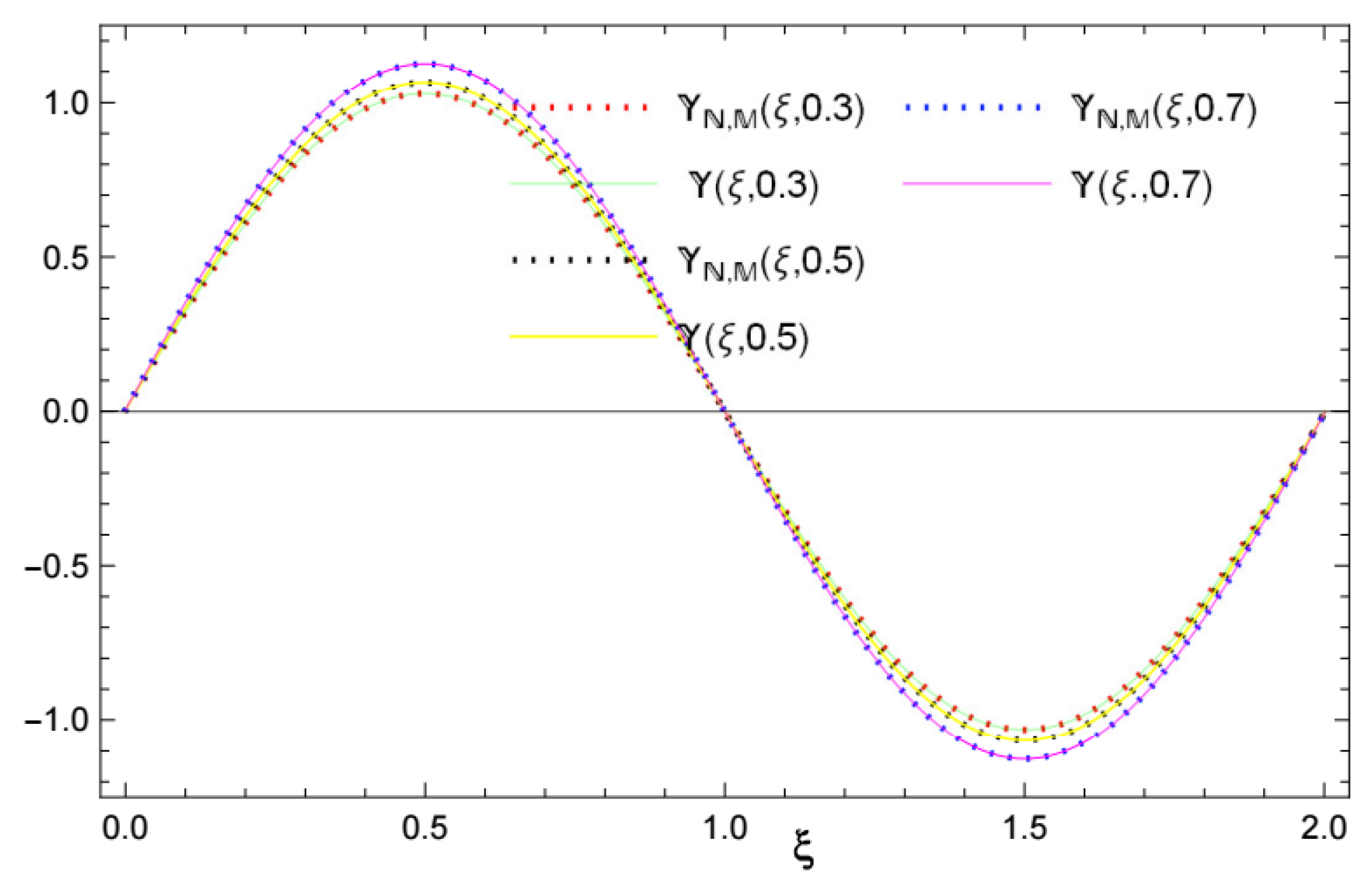
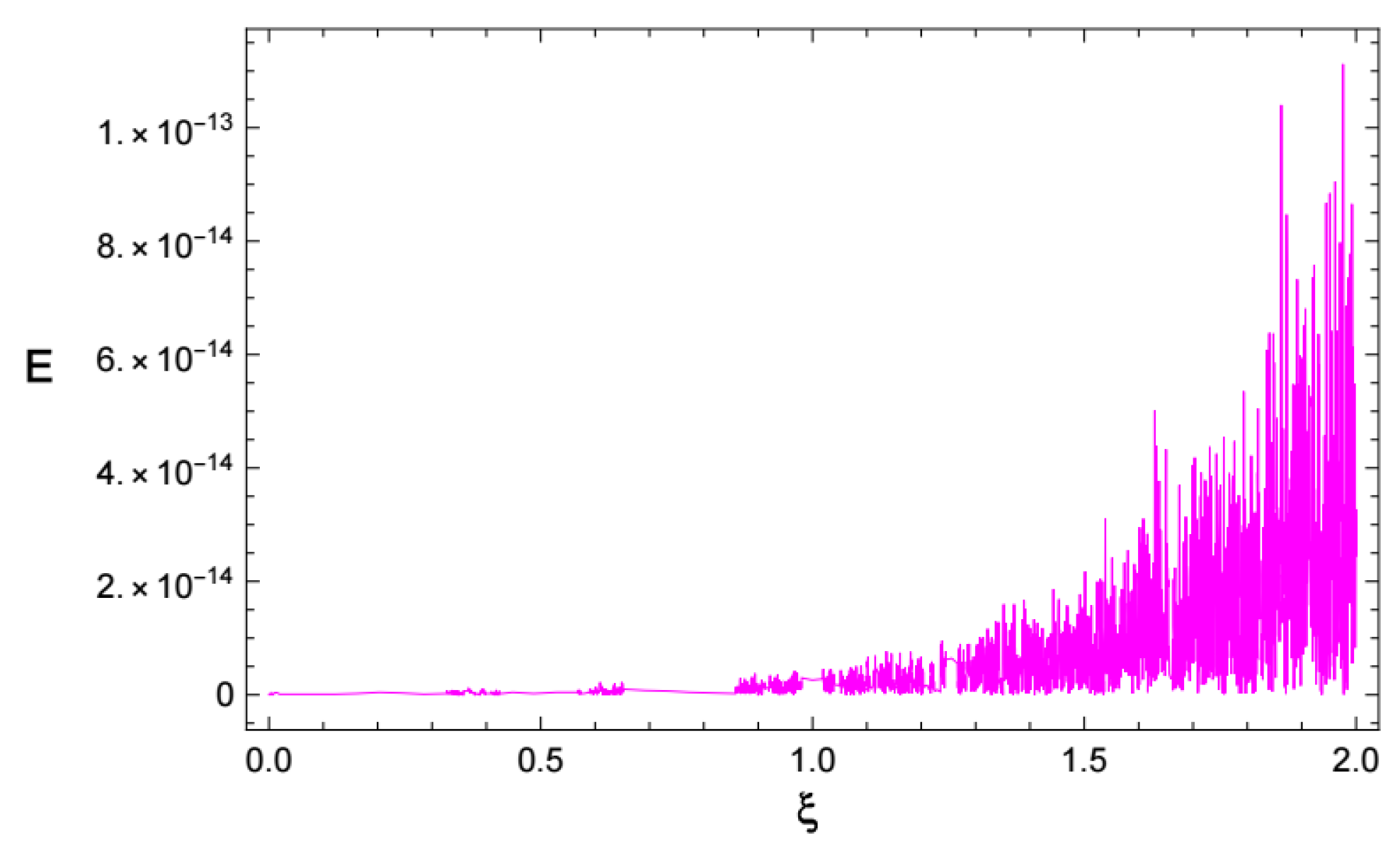
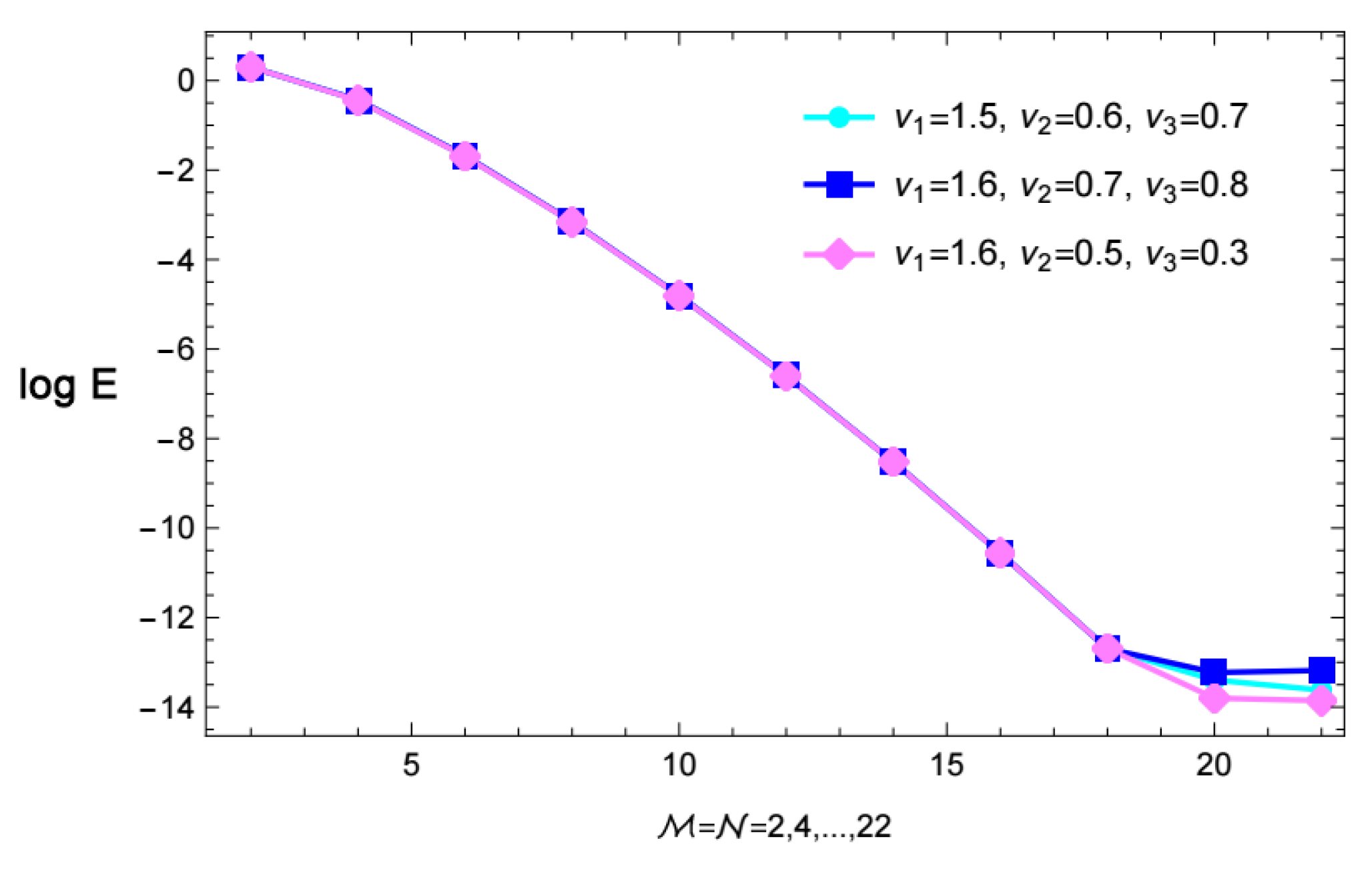
4. Some Numerical Examples
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations and Symbols
| Velocity field (meter/second) | |
| Relaxation time (second) | |
| Retardation time (second) | |
| Dynamic viscosity (Pascal-second) | |
| Constant density of the fluid | |
| Legendre polynomials | |
| Weight function of Legendre polynomials | |
| Orthogonality constant of Legendre polynomials | |
| Christoffel numbers of the Legendre-quadrature formula | |
| Orthogonality constant of shifted Legendre polynomials | |
| Space (meter) and time (second) | |
| Shifted Legendre polynomials | |
| FNNFM | Fractional non-Newtonian fluid model |
References
- Singh, S.; Devi, V.; Tohidi, E.; Singh, V.K. An efficient matrix approach for two-dimensional diffusion and telegraph equations with Dirichlet boundary conditions. Phys. A Stat. Mech. Appl. 2020, 545, 123784. [Google Scholar] [CrossRef]
- West, B.J. Nature’s Patterns and the Fractional Calculus; Walter de Gruyter GmbH & Co. KG: Berlin, Germany, 2017; Volume 2. [Google Scholar]
- Liu, Y. A new method for converting boundary value problems for impulsive fractional differential equations to integral equations and its applications. Adv. Nonlinear Anal. 2019, 8, 386–454. [Google Scholar] [CrossRef]
- Abdelkawy, M.; Babatin, M.M.; Alnahdi, A.S.; Taha, T. Legendre spectral collocation technique for fractional inverse heat conduction problem. Int. J. Mod. Phys. C 2022, 33, 2250065. [Google Scholar] [CrossRef]
- Abdelkawy, M.; Lopes, A.M. Spectral Solutions for Fractional Black–Scholes Equations. Math. Probl. Eng. 2022, 2022. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific: London, UK, 2010. [Google Scholar]
- Leonenko, N.N.; Meerschaert, M.M.; Sikorskii, A. Fractional pearson diffusions. J. Math. Anal. Appl. 2013, 403, 532–546. [Google Scholar] [CrossRef] [PubMed]
- Bagley, R.L.; Torvik, P. A theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 1983, 27, 201–210. [Google Scholar] [CrossRef]
- Makris, N.; Constantinou, M. Fractional-derivative Maxwell model for viscous dampers. J. Struct. Eng. 1991, 117, 2708–2724. [Google Scholar] [CrossRef]
- Animasaun, I.L.; Shah, N.A.; Wakif, A.; Mahanthesh, B.; Sivaraj, R.; Koríko, O.K. Ratio of Momentum Diffusivity to Thermal Diffusivity: Introduction, Meta-Analysis, and Scrutinization; CRC Press: Boca Raton, FL, USA, 2022. [Google Scholar]
- Jiang, Y.; Qi, H.; Xu, H.; Jiang, X. Transient electroosmotic slip flow of fractional Oldroyd-B fluids. Microfluid. Nanofluid. 2017, 21, 7. [Google Scholar] [CrossRef]
- Sutton, G.W.; Sherman, A. Engineering Magnetohydrodynamics; Courier Dover Publications: Oxford, UK, 2006. [Google Scholar]
- Khan, M.; Maqbool, K.; Hayat, T. Influence of Hall current on the flows of a generalized Oldroyd-B fluid in a porous space. Acta Mech. 2006, 184, 1–13. [Google Scholar] [CrossRef]
- Zheng, L.; Liu, Y.; Zhang, X. Slip effects on MHD flow of a generalized Oldroyd-B fluid with fractional derivative. Nonlinear Anal. Real World Appl. 2012, 13, 513–523. [Google Scholar] [CrossRef]
- Jiang, X.; Qi, H. Thermal wave model of bioheat transfer with modified Riemann–Liouville fractional derivative. J. Phys. A Math. Theor. 2012, 45, 485101. [Google Scholar] [CrossRef]
- Fetecau, C.; Fetecau, C.; Kamran, M.; Vieru, D. Exact solutions for the flow of a generalized Oldroyd-B fluid induced by a constantly accelerating plate between two side walls perpendicular to the plate. J. Non-Newton. Fluid Mech. 2009, 156, 189–201. [Google Scholar] [CrossRef]
- Zaky, M.A.; Doha, E.H.; Taha, T.M.; Baleanu, D. New recursive approximations for variable-order fractional operators with applications. arXiv 2018, arXiv:1804.01198. [Google Scholar] [CrossRef]
- Bhrawy, A.; Doha, E.; Baleanu, D.; Hafez, R. A highly accurate Jacobi collocation algorithm for systems of high-order linear differential–difference equations with mixed initial conditions. Math. Methods Appl. Sci. 2015, 38, 3022–3032. [Google Scholar] [CrossRef]
- Ding, H.; Li, C. Fractional-compact numerical algorithms for Riesz spatial fractional reaction-dispersion equations. Fract. Calc. Appl. Anal. 2017, 20, 722–764. [Google Scholar] [CrossRef]
- Alikhanov, A.A. A new difference scheme for the time fractional diffusion equation. J. Comput. Phys. 2015, 280, 424–438. [Google Scholar] [CrossRef]
- Liu, Y.; Du, Y.; Li, H.; Li, J.; He, S. A two-grid mixed finite element method for a nonlinear fourth-order reaction–diffusion problem with time-fractional derivative. Comput. Math. Appl. 2015, 70, 2474–2492. [Google Scholar] [CrossRef]
- Zhao, Y.; Bu, W.; Huang, J.; Liu, D.Y.; Tang, Y. Finite element method for two-dimensional space-fractional advection–dispersion equations. Appl. Math. Comput. 2015, 257, 553–565. [Google Scholar] [CrossRef]
- Simmons, A.; Yang, Q.; Moroney, T. A finite volume method for two-sided fractional diffusion equations on non-uniform meshes. J. Comput. Phys. 2017, 335, 747–759. [Google Scholar] [CrossRef]
- Jia, J.; Wang, H. A fast finite volume method for conservative space-fractional diffusion equations in convex domains. J. Comput. Phys. 2016, 310, 63–84. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Abdelkawy, M.A.; Baleanu, D.; Amin, A.Z. A spectral technique for solving two-dimensional fractional integral equations with weakly singular kernel. Hacet. J. Math. Stat. 2018, 47, 553–566. [Google Scholar]
- Zayernouri, M.; Karniadakis, G.E. Fractional spectral collocation method. SIAM J. Sci. Comput. 2014, 36, A40–A62. [Google Scholar] [CrossRef]
- Doha, E.H.; Abdelkawy, M.A.; Amin, A.Z.M.; Lopes, A.M. On spectral methods for solving variable-order fractional integro-differential equations. Comput. Appl. Math. 2018, 37, 3937–3950. [Google Scholar] [CrossRef]
- Abdelkawy, M.; Amin, A.; Lopes, A.M. Fractional-order shifted Legendre collocation method for solving non-linear variable-order fractional Fredholm integro-differential equations. Comput. Appl. Math. 2022, 41, 2. [Google Scholar] [CrossRef]
- Doha, E.H.; Abdelkawy, M.A.; Amin, A.Z.M.; Baleanu, D. Spectral technique for solving variable-order fractional Volterra integro-differential equations. Numer. Methods Partial Differ. Equ. 2018, 34, 1659–1677. [Google Scholar]
- Abdelkawy, M.A.; Amin, A.Z.M.; Babatin, M.M.; Alnahdi, A.S.; Zaky, M.A.; Hafez, R.M. Jacobi spectral collocation technique for time-fractional inverse heat equations. Fractal Fract. 2021, 5, 115. [Google Scholar] [CrossRef]
- Abdelkawy, M.A.; Amin, A.Z.; Bhrawy, A.H.; Machado, J.A.T.; Lopes, A.M. Jacobi collocation approximation for solving multi-dimensional Volterra integral equations. Int. J. Nonlinear Sci. Numer. Simul. 2017, 18, 411–425. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Zaky, M.A. A fractional-order Jacobi tau method for a class of time-fractional PDEs with variable coefficients. Math. Methods Appl. Sci. 2016, 39, 1765–1779. [Google Scholar] [CrossRef]
- Hafez, R.M.; Zaky, M.A. High-order continuous Galerkin methods for multi-dimensional advection–reaction–diffusion problems. Eng. Comput. 2020, 36, 1813–1829. [Google Scholar] [CrossRef]
- Hafez, R.M.; Zaky, M.A.; Hendy, A.S. A novel spectral Galerkin/Petrov–Galerkin algorithm for the multi-dimensional space–time fractional advection–diffusion–reaction equations with nonsmooth solutions. Math. Comput. Simul. 2021, 190, 678–690. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Zaky, M.A. Highly accurate numerical schemes for multi-dimensional space variable-order fractional Schrödinger equations. Comput. Math. Appl. 2017, 73, 1100–1117. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Zaky, M.A. An improved collocation method for multi-dimensional space–time variable-order fractional Schrödinger equations. Appl. Numer. Math. 2017, 111, 197–218. [Google Scholar] [CrossRef]
- Eslahchi, M.R.; Dehghan, M.; Parvizi, M. Application of the collocation method for solving nonlinear fractional integro-differential equations. J. Comput. Appl. Math. 2014, 257, 105–128. [Google Scholar] [CrossRef]
- Abdelkawy, M.A.; Doha, E.H.; Bhrawy, A.H.; Amin, A.Z. Efficient pseudospectral scheme for 3D integral equations. Proc. Rom. Acad. Ser. A Math. Phys. Tech. Sci. Inf. Sci. 2017, 18, 199–206. [Google Scholar]
- Ezz-Eldien, S.; Wang, Y.; Abdelkawy, M.; Zaky, M.; Aldraiweesh, A.; Machado, J.T. Chebyshev spectral methods for multi-order fractional neutral pantograph equations. Nonlinear Dyn. 2020, 100, 3785–3797. [Google Scholar] [CrossRef]
- Abdelkawy, M. Numerical solutions for fractional initial value problems of distributed-order. Int. J. Mod. Phys. C (IJMPC) 2021, 32, 2150096. [Google Scholar] [CrossRef]
- Abdelkawy, M.A. Shifted Legendre spectral collocation technique for solving stochastic Volterra–Fredholm integral equations. Int. J. Nonlinear Sci. Numer. Simul. 2021, 24, 123–136. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Ezz-Eldien, S.S.; Doha, E.H.; Abdelkawy, M.A.; Baleanu, D. Solving fractional optimal control problems within a Chebyshev–Legendre operational technique. Int. J. Control 2017, 90, 1230–1244. [Google Scholar] [CrossRef]
- Canuto, C.; Hussaini, M.Y.; Quarteroni, A.; Zang, T.A. Spectral Methods: Fundamentals in Single Domains; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Bhrawy, A.; Zaky, M.; Tenreiro Machado, J. Efficient Legendre spectral tau algorithm for solving the two-sided space–time Caputo fractional advection–dispersion equation. J. Vib. Control 2016, 22, 2053–2068. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Zaky, M.A.; Baleanu, D. New numerical approximations for space-time fractional Burgers’ equations via a Legendre spectral-collocation method. Rom. Rep. Phys. 2015, 67, 340–349. [Google Scholar]
- Liu, Y.; Yin, X.; Feng, L.; Sun, H. Finite difference scheme for simulating a generalized two-dimensional multi-term time fractional non-Newtonian fluid model. Adv. Differ. Equ. 2018, 2018, 442. [Google Scholar] [CrossRef]
- Feng, L.; Liu, F.; Turner, I.; Zhuang, P. Numerical methods and analysis for simulating the flow of a generalized Oldroyd-B fluid between two infinite parallel rigid plates. Int. J. Heat Mass Transf. 2017, 115, 1309–1320. [Google Scholar] [CrossRef]
- Sun, Z.Z.; Wu, X. A fully discrete difference scheme for a diffusion-wave system. Appl. Numer. Math. 2006, 56, 193–209. [Google Scholar] [CrossRef]
- Zheng, L.; Zhao, F.; Zhang, X. Exact solutions for generalized Maxwell fluid flow due to oscillatory and constantly accelerating plate. Nonlinear Anal. Real World Appl. 2010, 11, 3744–3751. [Google Scholar] [CrossRef]
- Zaky, M.A.; Abdelkawy, M.A.; Ezz-Eldiene, S.S.; Dohaf, E.H. Pseudospectral methods for the Riesz space-fractional Schrödinger equation. Fract.-Order Model. Dyn. Syst. Appl. Optim. Signal Process. Control 2021, 2, 323–353. [Google Scholar]
- Abdelkawy, M.; Alyami, S. Legendre-Chebyshev spectral collocation method for two-dimensional nonlinear reaction-diffusion equation with Riesz space-fractional. Chaos Solitons Fractals 2021, 151, 111279. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Abdelkawy, M.A. A fully spectral collocation approximation for multi-dimensional fractional Schrödinger equations. J. Comput. Phys. 2015, 294, 462–483. [Google Scholar] [CrossRef]
- Feng, L.; Liu, F.; Turner, I.; Zheng, L. Novel numerical analysis of multi-term time fractional viscoelastic non-Newtonian fluid models for simulating unsteady MHD Couette flow of a generalized Oldroyd-B fluid. Fract. Calc. Appl. Anal. 2018, 21, 1073–1103. [Google Scholar] [CrossRef]


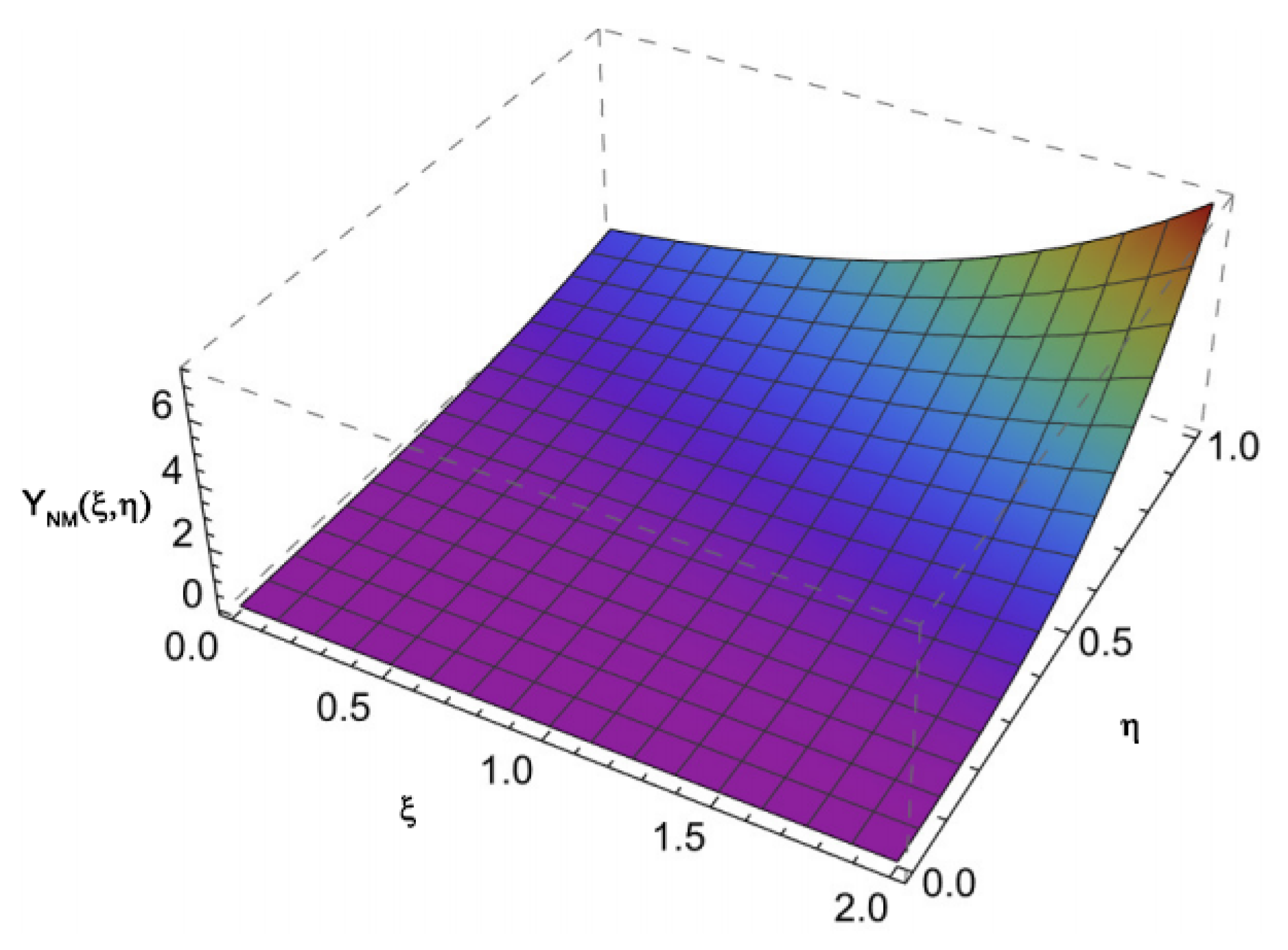
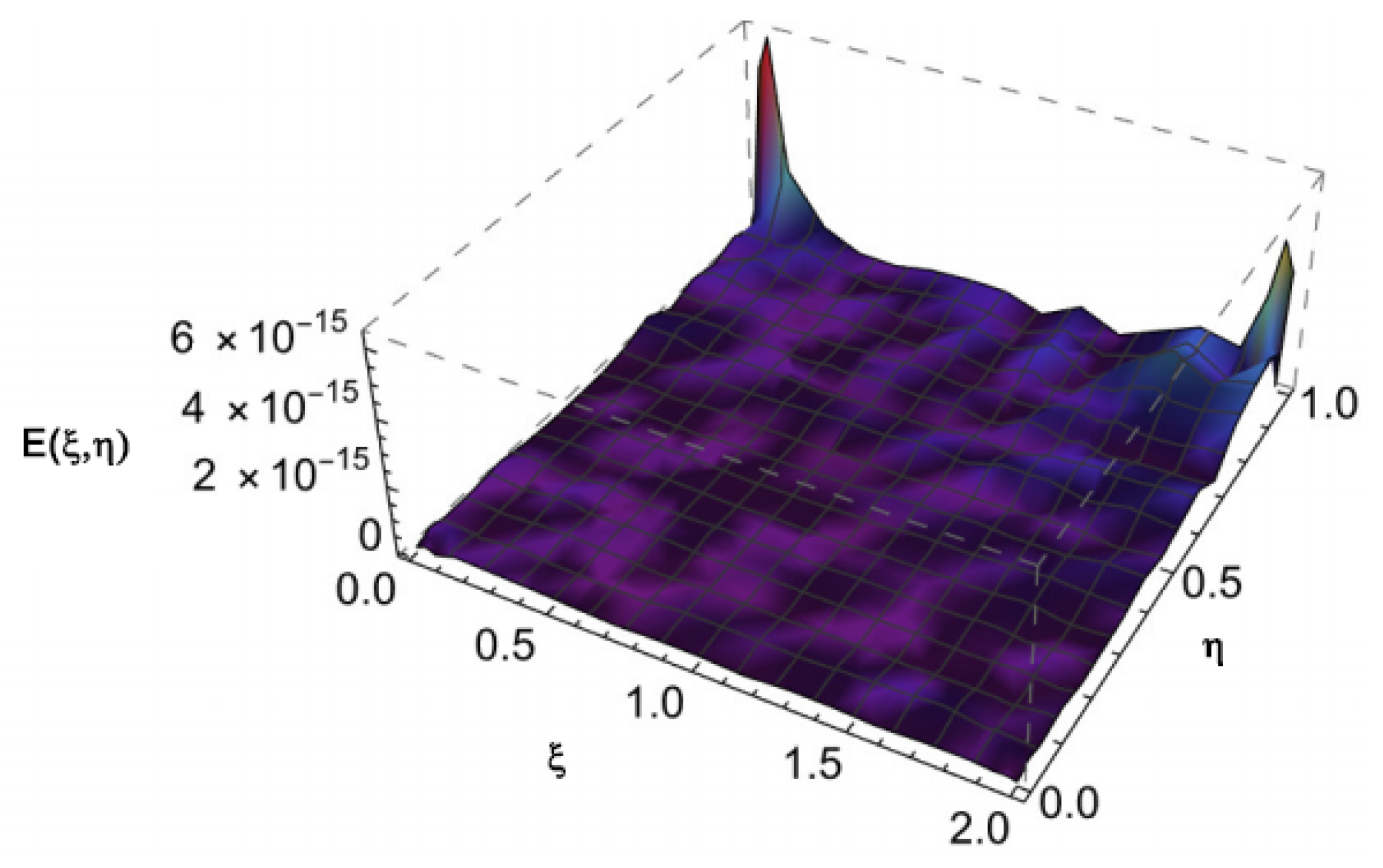
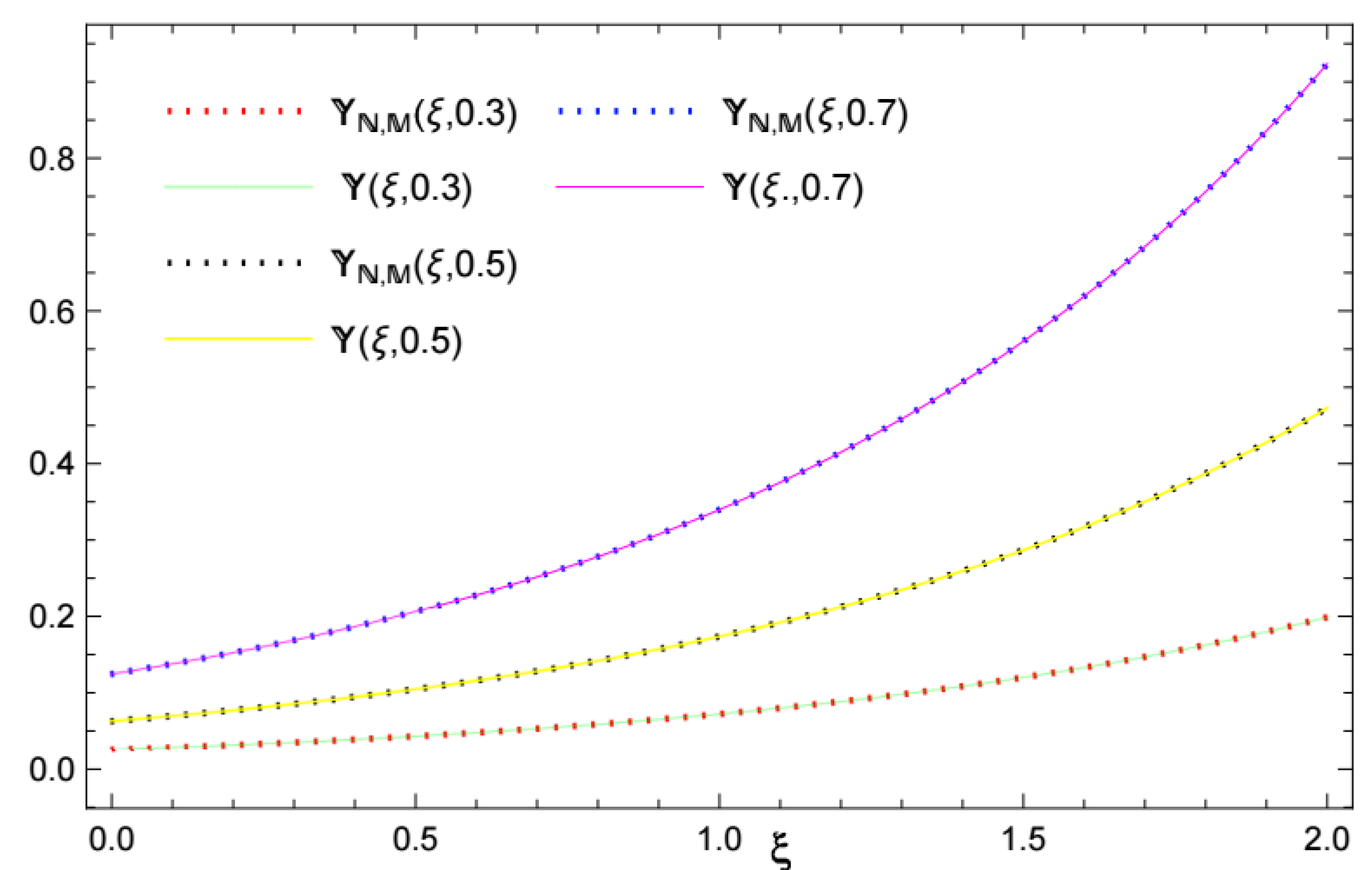
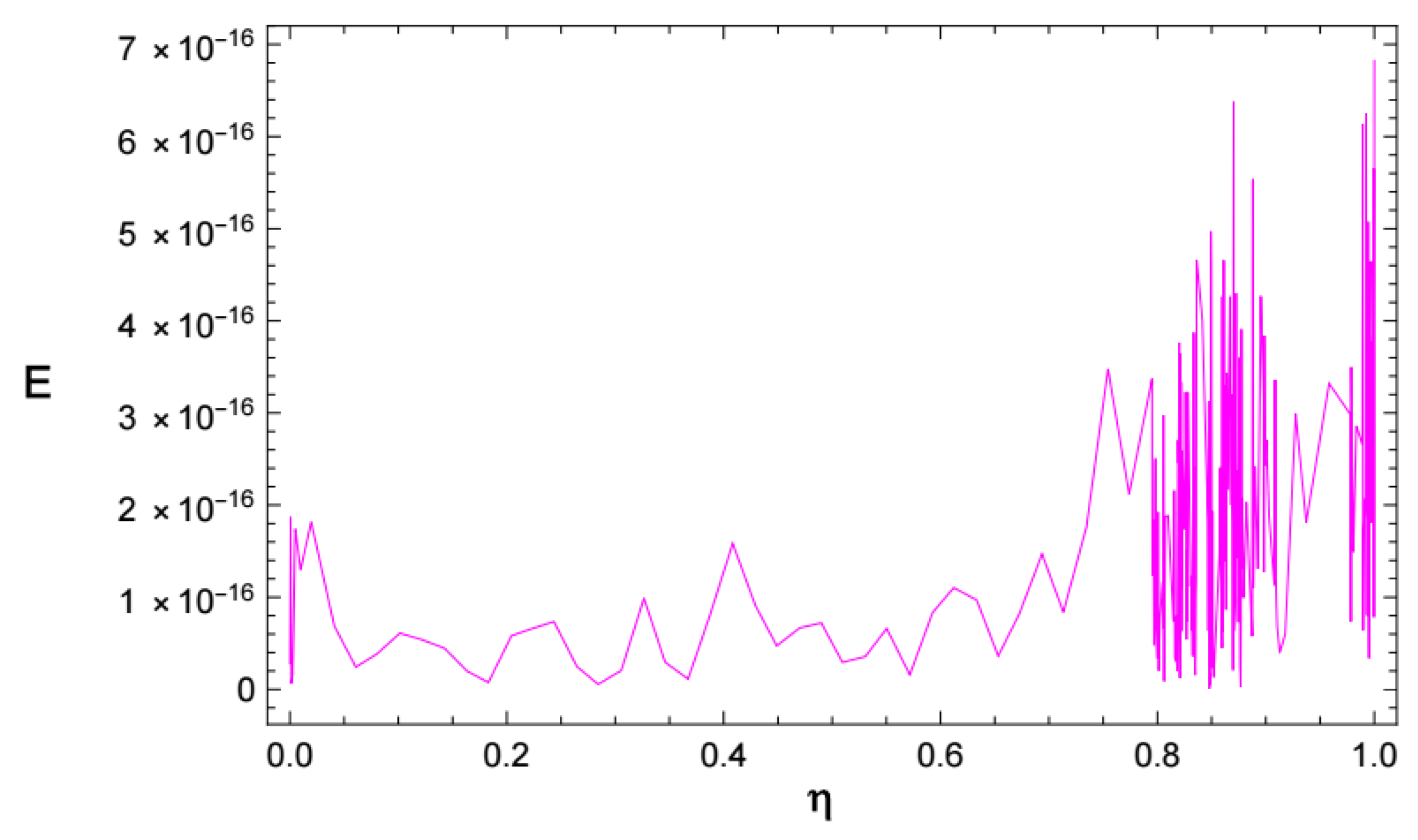
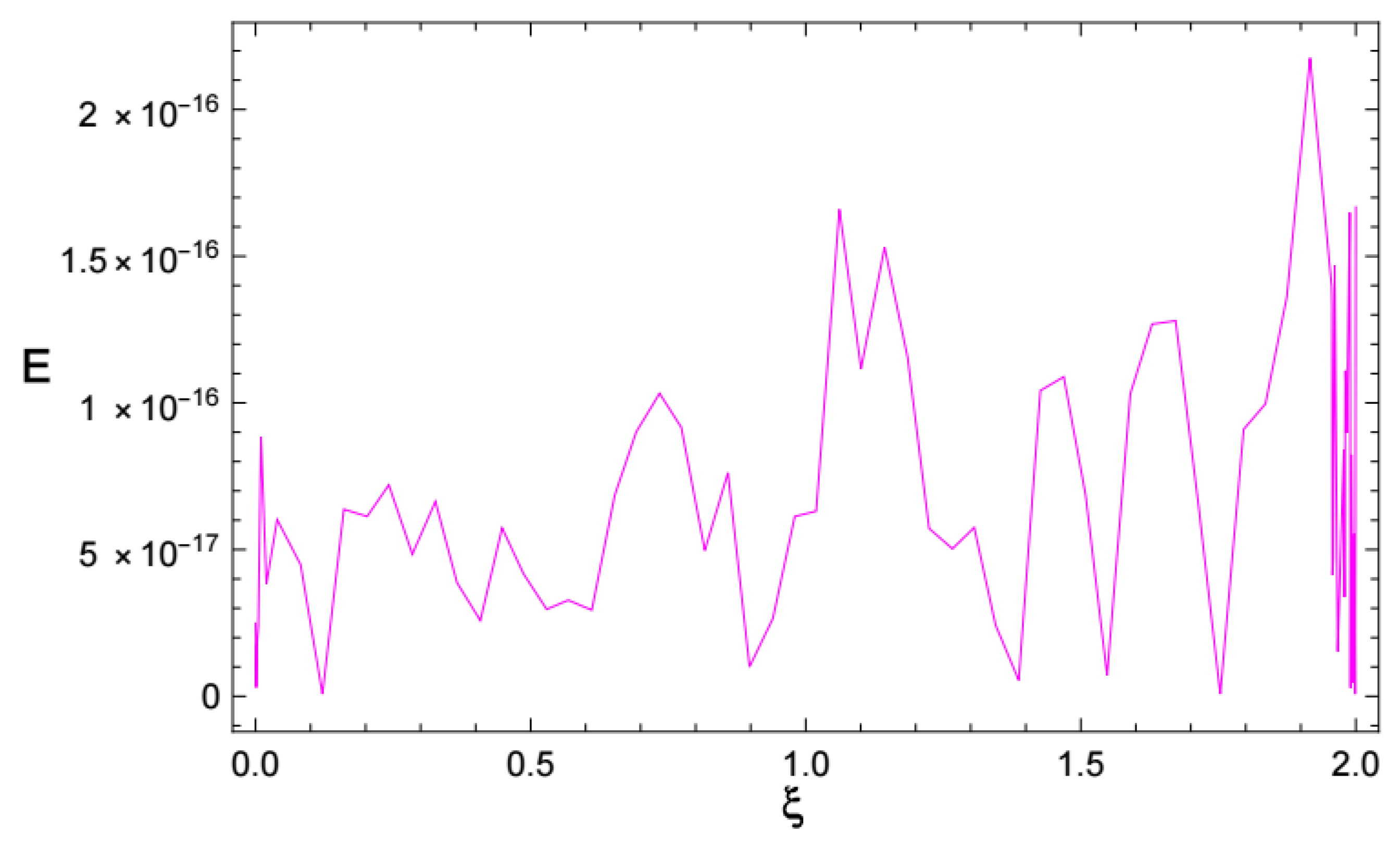
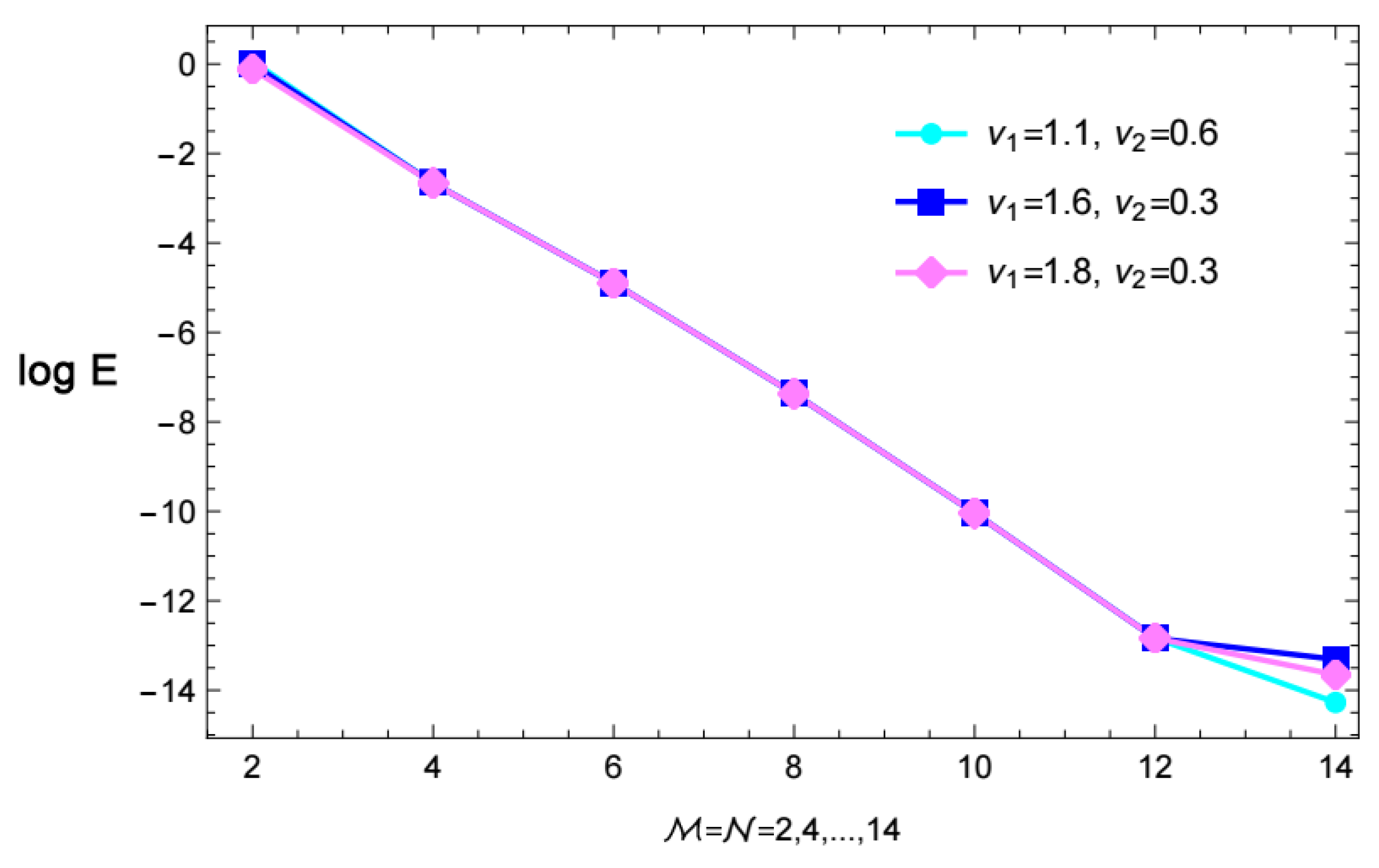
| Finite difference method [53] | |||
| h | |||
| New method | |||
| Finite difference method [47] | |||
| h | |||
| New method | |||
| (4,4) | |||
| (8,8) | |||
| (12,12) | |||
| (14,14) | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Shomrani, M.M.; Abdelkawy, M.A.; Lopes, A.M. Spectral Collocation Technique for Solving Two-Dimensional Multi-Term Time Fractional Viscoelastic Non-Newtonian Fluid Model. Mathematics 2023, 11, 2078. https://doi.org/10.3390/math11092078
Al-Shomrani MM, Abdelkawy MA, Lopes AM. Spectral Collocation Technique for Solving Two-Dimensional Multi-Term Time Fractional Viscoelastic Non-Newtonian Fluid Model. Mathematics. 2023; 11(9):2078. https://doi.org/10.3390/math11092078
Chicago/Turabian StyleAl-Shomrani, Mohammed M., Mohamed A. Abdelkawy, and António M. Lopes. 2023. "Spectral Collocation Technique for Solving Two-Dimensional Multi-Term Time Fractional Viscoelastic Non-Newtonian Fluid Model" Mathematics 11, no. 9: 2078. https://doi.org/10.3390/math11092078
APA StyleAl-Shomrani, M. M., Abdelkawy, M. A., & Lopes, A. M. (2023). Spectral Collocation Technique for Solving Two-Dimensional Multi-Term Time Fractional Viscoelastic Non-Newtonian Fluid Model. Mathematics, 11(9), 2078. https://doi.org/10.3390/math11092078







