Analysis of Generalized Nonlinear Quadrature for Novel Fractional-Order Chaotic Systems Using Sinc Shape Function
Abstract
1. Introduction
2. Formulation of the Problem
2.1. Fractional-Order Lorenz System [34]
2.2. Fractional-Order Chen System [5,35,36]
2.3. Fractional-Order Lu System [37]
2.4. Fractional-Order Yang System [38]
2.5. Fractional-Order Liu System [39]
2.6. Fractional-Order Shimizu–Morioka System [40]
2.7. Fractional-Order Burke-Shaw System [41]
3. Method of Solution
3.1. Generalized Caputo-Kind Fractional Derivative [27,28,29,30,31]
3.2. Differential Quadrature Method Based on Cardinal Sine Shape Function (SINC-DQM)
4. Numerical Results
4.1. Problem 1
4.2. Problem 2
4.3. Problem 3
4.4. Problem 4
4.5. Problem 5
4.6. Problem 6
4.7. Problem 7
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rahman, Z.-A.S.A.; Jasim, B.H.; Al-Yasir, Y.I.A.; Hu, Y.-F.; Abd-Alhameed, R.A.; Alhasnawi, B.N. A New Fractional-Order Chaotic System with Its Analysis, Synchronization, and Circuit Realization for Secure Communication Applications. Mathematics 2021, 9, 2593. [Google Scholar] [CrossRef]
- Cafagna, D.; Grassi, G. Bifurcation and Chaos in the Fractional-Order Chen System via a Time-Domain Approach. Int. J. Bifurc. Chaos 2008, 18, 1845–1863. [Google Scholar] [CrossRef]
- Grigorenko, I.; Grigorenko, E. Chaotic Dynamics of the Fractional Lorenz System. Phys. Rev. Lett. 2003, 91, 034101. [Google Scholar] [CrossRef] [PubMed]
- Li, C.; Deng, W. Chaos synchronization of fractional-order differential Systems. Int. J. Mod. Phys. B 2006, 20, 791–803. [Google Scholar] [CrossRef]
- Lü, J.; Chen, G. A New Chaotic Attractor Coined. Int. J. Bifurc. Chaos 2002, 12, 659–661. [Google Scholar] [CrossRef]
- Petráš, I. The fractional-order Lorenz-type systems: A review. Fract. Calc. Appl. Anal. 2022, 25, 362–377. [Google Scholar] [CrossRef]
- Tan, Y.; Abbasbandy, S. Homotopy analysis method for quadratic Riccati differential equation. Commun. Nonlinear Sci. Numer. Simul. 2008, 13, 539–546. [Google Scholar] [CrossRef]
- Li, Y.; Hu, L. Solving Fractional Riccati Differential Equations Using Haar Wavelet. In Proceedings of the 2010 Third International Conference on Information and Computing, Wuxi, China, 4–6 June 2010; IEEE: New Jersey, NJ, USA, 2010; Volume 1, pp. 314–317. [Google Scholar]
- Cang, J.; Tan, Y.; Xu, H.; Liao, S.-J. Series solutions of non-linear Riccati differential equations with fractional order. Chaos Solitons Fractals 2009, 40, 1–9. [Google Scholar] [CrossRef]
- Gülsu, M.; Sezer, M. On the solution of the Riccati equation by the Taylor matrix method. Appl. Math. Comput. 2006, 176, 414–421. [Google Scholar] [CrossRef]
- Aminikhah, H.; Hemmatnezhad, M. An Efficient Method for Quadratic Riccati Differential Equation. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 835–839. [Google Scholar] [CrossRef]
- Alam Khan, N.; Ara, A.; Jamil, M. An efficient approach for solving the Riccati equation with fractional orders. Comput. Math. Appl. 2011, 61, 2683–2689. [Google Scholar] [CrossRef]
- Odibat, Z.; Momani, S. Modified homotopy perturbation method: Application to quadratic Riccati differential equation of fractional order. Chaos Solitons Fractals 2008, 36, 167–174. [Google Scholar] [CrossRef]
- Bi, Y.; Jiang, Z. The finite volume element method for the two-dimensional space-fractional convection–diffusion equation. Adv. Differ. Equ. 2021, 2021, 1–22. [Google Scholar] [CrossRef]
- Aboelenen, T. A direct discontinuous Galerkin method for fractional convection-diffusion and Schrödinger-type equations. Eur. Phys. J. Plus 2018, 133, 316. [Google Scholar] [CrossRef]
- Parvizi, M.; Eslahchi, M.R.; Dehghan, M. Numerical solution of fractional advection-diffusion equation with a nonlinear source term. Numer. Algorithms 2014, 68, 601–629. [Google Scholar] [CrossRef]
- Gu, X.-M.; Huang, T.-Z.; Ji, C.-C.; Carpentieri, B.; Alikhanov, A.A. Fast Iterative Method with a Second-Order Implicit Difference Scheme for Time-Space Fractional Convection–Diffusion Equation. J. Sci. Comput. 2017, 72, 957–985. [Google Scholar] [CrossRef]
- Sheu, L.-J.; Chen, H.-K.; Chen, J.-H.; Tam, L.-M.; Chen, W.-C.; Lin, K.-T.; Kang, Y. Chaos in the Newton–Leipnik system with fractional order. Chaos Solitons Fractals 2008, 36, 98–103. [Google Scholar] [CrossRef]
- Li, C.; Peng, G. Chaos in Chen’s system with a fractional order. Chaos Solitons Fractals 2004, 22, 443–450. [Google Scholar] [CrossRef]
- Katugampola, U.N. New approach to a generalized fractional integral. Appl. Math. Comput. 2011, 218, 860–865. [Google Scholar] [CrossRef]
- Almeida, R.; Malinowska, A.B.; Odzijewicz, T. Fractional Differential Equations with Dependence on the Caputo–Katugampola Derivative. J. Comput. Nonlinear Dyn. 2016, 11, 061017. [Google Scholar] [CrossRef]
- Wang, Z.; Huang, X.; Li, N.; Song, X.-N. Image encryption based on a delayed fractional-order chaotic logistic system. Chin. Phys. B 2012, 21, 111–116. [Google Scholar] [CrossRef]
- Wu, G.-C.; Baleanu, D.; Lin, Z.-X. Image encryption technique based on fractional chaotic time series. J. Vib. Control 2015, 22, 2092–2099. [Google Scholar] [CrossRef]
- Anderson, D.R.; Ulness, D.J. Properties of the Katugampola fractional derivative with potential application in quantum mechanics. J. Math. Phys. 2015, 56, 063502. [Google Scholar] [CrossRef]
- Korkmaz, A.; Dağ, İ. Shock Wave Simulations Using Sinc Differential Quadrature Method. Eng. Comput. 2011, 28, 654–674. [Google Scholar] [CrossRef]
- Qiu, W.; Xu, D.; Guo, J. Numerical solution of the fourth-order partial integro-differential equation with multi-term kernels by the Sinc-collocation method based on the double exponential transformation. Appl. Math. Comput. 2020, 392, 125693. [Google Scholar] [CrossRef]
- Daftardar-Gejji, V.; Bhalekar, S.; Gade, P. Dynamics of fractional-ordered Chen system with delay. Pramana 2012, 79, 61–69. [Google Scholar] [CrossRef]
- Katugampola, U.N. Existence and Uniqueness Results for a Class of Generalized Fractional Differential Equations. arXiv 2014, arXiv:1411.5229. [Google Scholar]
- Khan, N.A.; Ara, A.; Alam Khan, N. Fractional-Order Riccati Differential Equation: Analytical Approximation and Numerical Results. Adv. Differ. Equ. 2013, 2013, 1–16. [Google Scholar] [CrossRef]
- Odibat, Z.; Baleanu, D. Numerical simulation of initial value problems with generalized Caputo-type fractional derivatives. Appl. Numer. Math. 2020, 156, 94–105. [Google Scholar] [CrossRef]
- Baleanu, D.; Wu, G.; Zeng, S. Chaos analysis and asymptotic stability of generalized Caputo fractional differential equations. Chaos Solitons Fractals 2017, 102, 99–105. [Google Scholar] [CrossRef]
- Sprott, J.C. Some simple chaotic flows. Phys. Rev. E 1994, 50, R647–R650. [Google Scholar] [CrossRef] [PubMed]
- Pan, I.; Das, S. Evolving chaos: Identifying new attractors of the generalised Lorenz family. Appl. Math. Model. 2018, 57, 391–405. [Google Scholar] [CrossRef]
- Li, C.; Yan, J. The synchronization of three fractional differential systems. Chaos Solitons Fractals 2007, 32, 751–757. [Google Scholar] [CrossRef]
- Lu, J.G.; Chen, G. A note on the fractional-order Chen system. Chaos Solitons Fractals 2006, 27, 685–688. [Google Scholar] [CrossRef]
- Zhou, T.; Tang, Y.; Chen, G. Chen’s Attractor Exists. Int. J. Bifurc. Chaos 2004, 14, 3167–3177. [Google Scholar] [CrossRef]
- Deng, W.H.; Li, C.P. Chaos Synchronization of the Fractional Lü System. Phys. A Stat. Mech. Appl. 2005, 353, 61–72. [Google Scholar] [CrossRef]
- Yang, Q.; Chen, G. A Chaotic System with One Saddle and Two Stable Node-Foci. Int. J. Bifurc. Chaos 2008, 18, 1393–1414. [Google Scholar] [CrossRef]
- Liu, C.; Liu, T.; Liu, L.; Liu, K. A New Chaotic Attractor. Chaos Solitons Fractals 2004, 22, 1031–1038. [Google Scholar] [CrossRef]
- Wei, Z.; Zhang, X. Chaos in the Shimizu-Morioka Model with Fractional Order. Front. Phys. 2021, 9, 636173. [Google Scholar] [CrossRef]
- Mahmoud, G.M.; Arafa, A.A.; Abed-Elhameed, T.M.; Mahmoud, E.E. Chaos control of integer and fractional orders of chaotic Burke–Shaw system using time delayed feedback control. Chaos Solitons Fractals 2017, 104, 680–692. [Google Scholar] [CrossRef]
- Shaw, R. Strange Attractors, Chaotic Behavior, and Information Flow. Z. Naturforschung A 1981, 36, 80–112. [Google Scholar] [CrossRef]
- Sunthrayuth, P.; Ullah, R.; Khan, A.; Shah, R.; Kafle, J.; Mahariq, I.; Jarad, F. Numerical Analysis of the Fractional-Order Nonlinear System of Volterra Integro-Differential Equations. J. Funct. Spaces 2021, 2021, 1–10. [Google Scholar] [CrossRef]
- Alaoui, M.K.; Fayyaz, R.; Khan, A.; Shah, R.; Abdo, M.S. Analytical Investigation of Noyes–Field Model for Time-Fractional Belousov–Zhabotinsky Reaction. Complexity 2021, 2021, 1–21. [Google Scholar] [CrossRef]
- Fathima, D.; Alahmadi, R.A.; Khan, A.; Akhter, A.; Ganie, A.H. An Efficient Analytical Approach to Investigate Fractional Caudrey–Dodd–Gibbon Equations with Non-Singular Kernel Derivatives. Symmetry 2023, 15, 850. [Google Scholar] [CrossRef]
- Mishra, N.K.; AlBaidani, M.M.; Khan, A.; Ganie, A.H. Numerical Investigation of Time-Fractional Phi-Four Equation via Novel Transform. Symmetry 2023, 15, 687. [Google Scholar] [CrossRef]
- Caputo, M. Linear Models of Dissipation whose Q is almost Frequency Independent--II. Geophys. J. Int. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Weilbeer, M. Efficient Numerical Methods for Fractional Differential Equations and Their Analytical Background; Papierflieger: Clausthal-Zellerfeld, Germany, 2006. [Google Scholar]
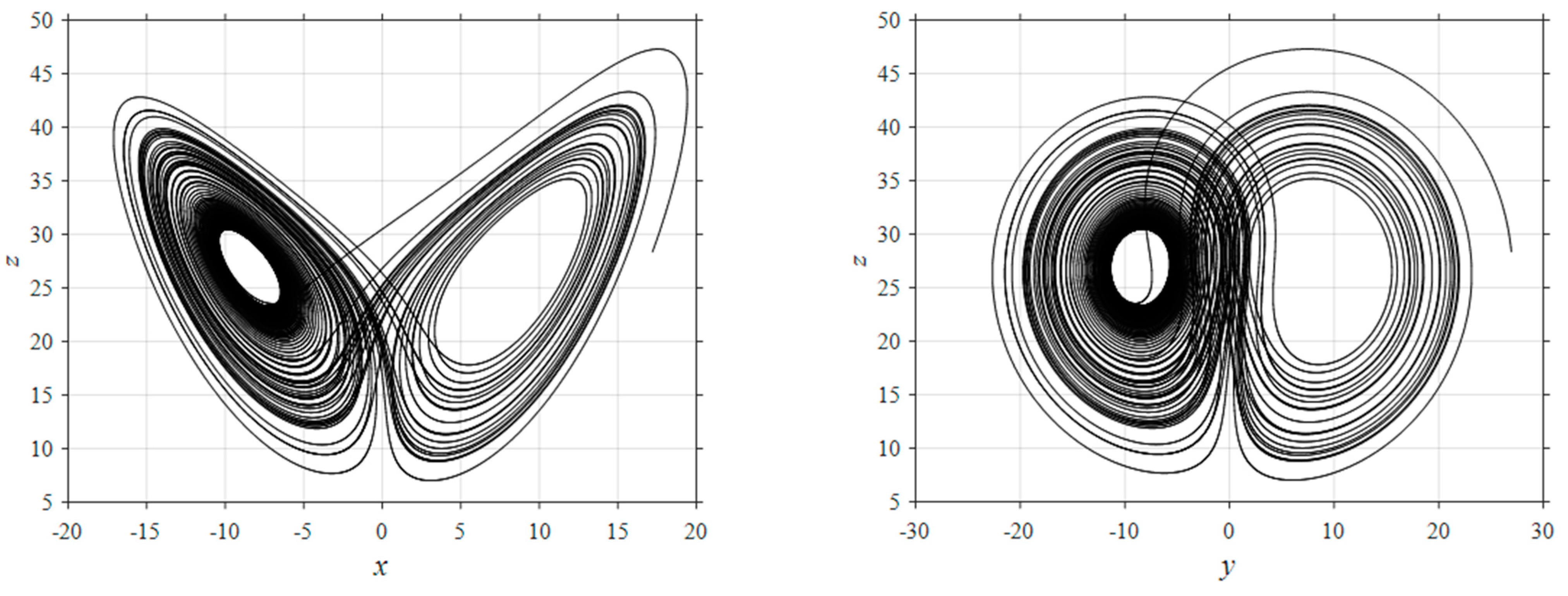
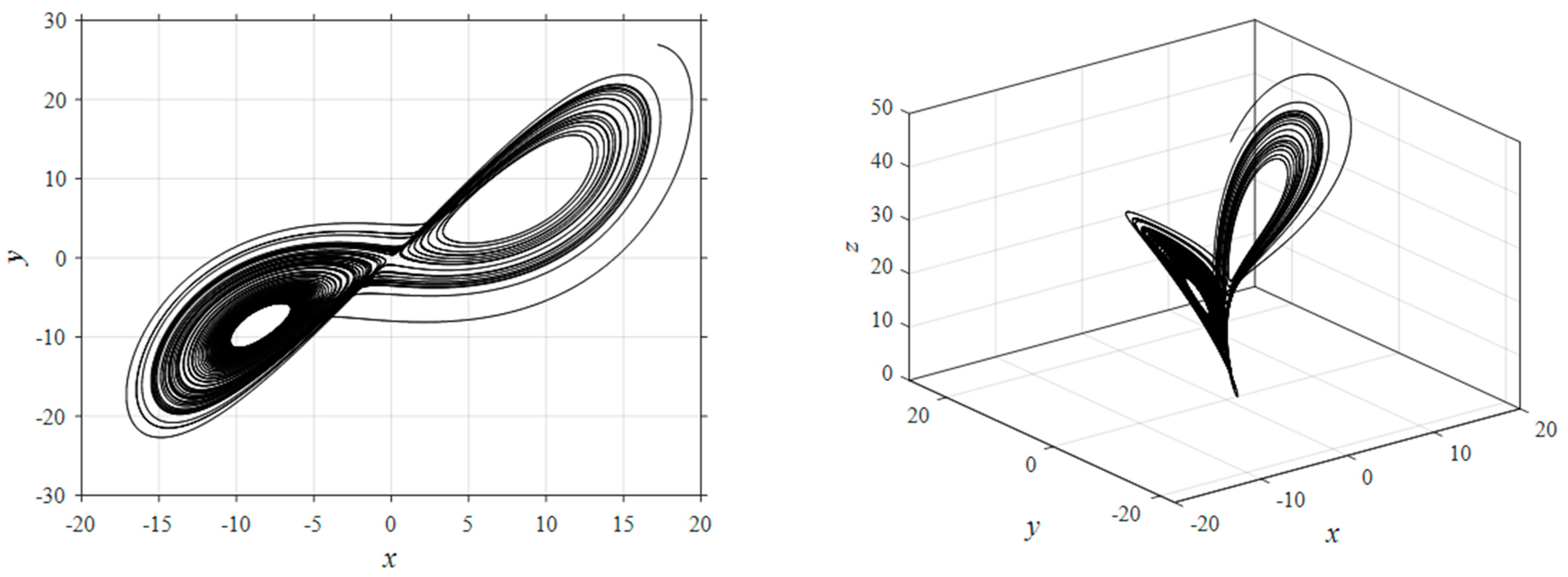


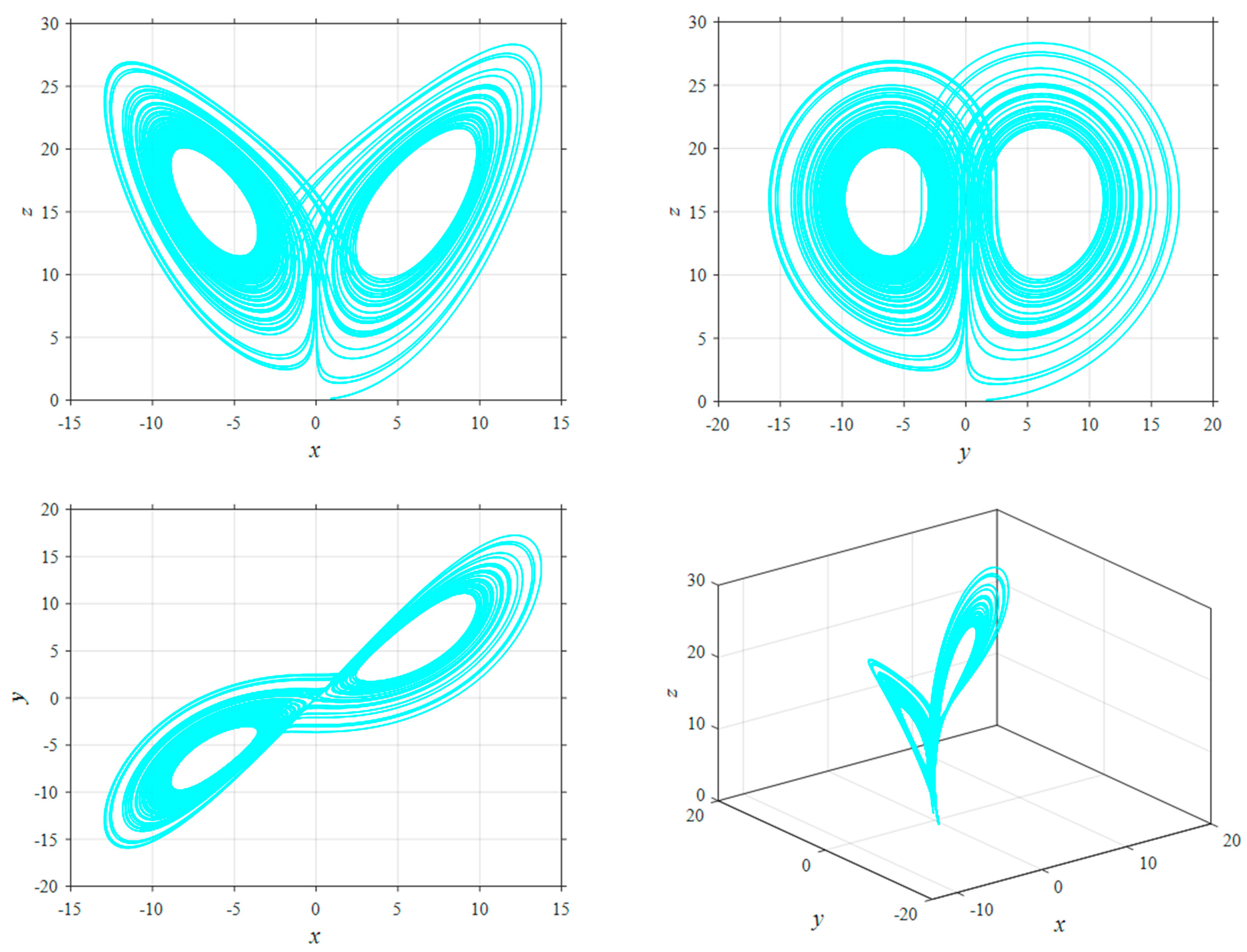


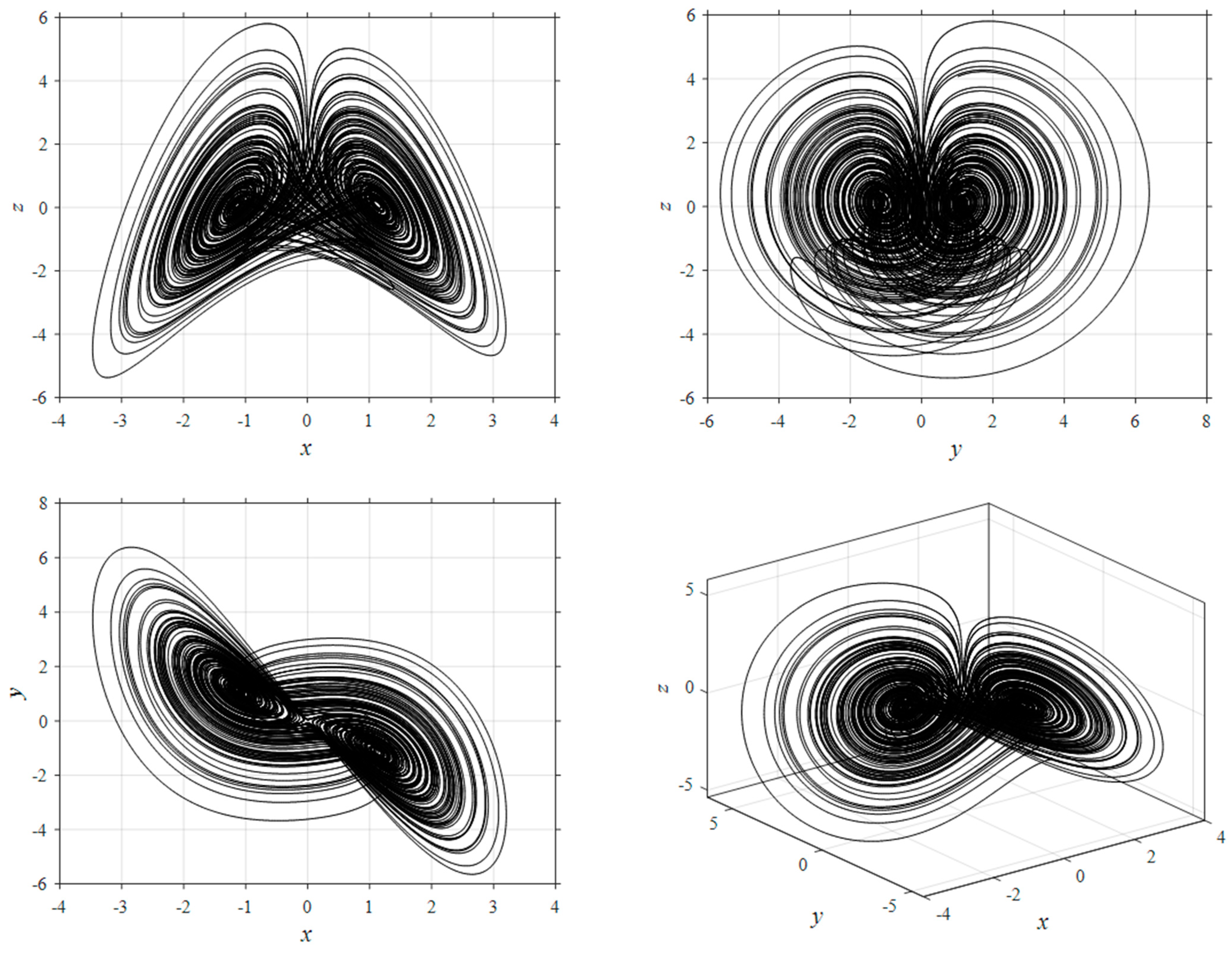
| N | SINC-DQM | CPU (s) | N | Earlier Numerical Solutions [27,28,29,30,31] | ||||
|---|---|---|---|---|---|---|---|---|
| 4 | 0.837919933 | 0.623902547 | 0.302407988 | 0.02 | 5 | 0.76932157 | 0.51235478 | 0.24658714 |
| 5 | 0.771720200 | 0.527485798 | 0.248194100 | 0.021 | 10 | 0.76547321 | 0.50805837 | 0.24436125 |
| 6 | 0.761378603 | 0.49832669 | 0.2415685802 | 0.022 | 20 | 0.76301723 | 0.50206750 | 0.24290222 |
| 7 | 0.760223666 | 0.497601296 | 0.2409074397 | 0.023 | 40 | 0.76241447 | 0.50063345 | 0.24253181 |
| 9 | 0.762203989 | 0.500122597 | 0.242400811 | 0.024 | 80 | 0.76226520 | 0.50028279 | 0.24243886 |
| 10 | 0.762216222 | 0.500169299 | 0.2424081878 | 0.025 | 160 | 0.76222806 | 0.50019611 | 0.24241560 |
| 11 | 0.76221575 | 0.500167396 | 0.242407838 | 0.025 | 320 | 0.76221880 | 0.50017456 | 0.24240979 |
| 12 | 0.76221573 | 0.500167394 | 0.2424078434 | 0.026 | 640 | 0.76221649 | 0.50016919 | 0.24240833 |
| 13 | 0.76221572 | 0.500167392 | 0.2424078432 | 0.028 | 1280 | 0.76221572 | 0.50016739 | 0.24240783 |
| N | SINC-DQM | CPU (s) | N | Earlier Numerical Solutions [27,28,29,30,31] | ||||
|---|---|---|---|---|---|---|---|---|
| x | y | z | x | y | z | |||
| 4 | 1.98744231 | 1.95521788 | 1.77785472 | 0.08 | 5 | 1.87823591 | 1.91235487 | 1.77214577 |
| 5 | 1.87775412 | 1.94621547 | 1.76812478 | 0.082 | 10 | 1.87691249 | 1.90833765 | 1.76232887 |
| 6 | 1.87702358 | 1.93987952 | 1.76214785 | 0.083 | 20 | 1.87411505 | 1.93108732 | 1.75629505 |
| 7 | 1.87412004 | 1.93952410 | 1.75874532 | 0.084 | 40 | 1.87336221 | 1.93662856 | 1.75468966 |
| 9 | 1.87311223 | 1.9392005 | 1.75623587 | 0.086 | 80 | 1.87317222 | 1.93800680 | 1.75428450 |
| 10 | 1.87310922 | 1.93894152 | 1.75469874 | 0.087 | 160 | 1.87312481 | 1.93835118 | 1.75418327 |
| 11 | 1.87310905 | 1.93847456 | 1.75418742 | 0.088 | 320 | 1.87311299 | 1.93843729 | 1.75415800 |
| 12 | 1.87310903 | 1.93846621 | 1.75415001 | 0.089 | 640 | 1.87311004 | 1.93845883 | 1.75415169 |
| 13 | 1.87310902 | 1.93846581 | 1.75414950 | 0.09 | 1280 | 1.87310902 | 1.93846581 | 1.75414950 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mustafa, A.; Salama, R.S.; Mohamed, M. Analysis of Generalized Nonlinear Quadrature for Novel Fractional-Order Chaotic Systems Using Sinc Shape Function. Mathematics 2023, 11, 1932. https://doi.org/10.3390/math11081932
Mustafa A, Salama RS, Mohamed M. Analysis of Generalized Nonlinear Quadrature for Novel Fractional-Order Chaotic Systems Using Sinc Shape Function. Mathematics. 2023; 11(8):1932. https://doi.org/10.3390/math11081932
Chicago/Turabian StyleMustafa, Abdelfattah, Reda S. Salama, and Mokhtar Mohamed. 2023. "Analysis of Generalized Nonlinear Quadrature for Novel Fractional-Order Chaotic Systems Using Sinc Shape Function" Mathematics 11, no. 8: 1932. https://doi.org/10.3390/math11081932
APA StyleMustafa, A., Salama, R. S., & Mohamed, M. (2023). Analysis of Generalized Nonlinear Quadrature for Novel Fractional-Order Chaotic Systems Using Sinc Shape Function. Mathematics, 11(8), 1932. https://doi.org/10.3390/math11081932








