On the Soliton Solutions for the Stochastic Konno–Oono System in Magnetic Field with the Presence of Noise
Abstract
1. Introduction
- is taken for the Stratonovich sense;
- is taken for the It sense.
2. Wiener Process
- is a continuous functionif .
- = 0.
- For , is independent.
- has a Gaussian distribution .
3. Soliton Solutions for K–O System with Stratonovich Sense
- Family-I: When , and , then . So, we obtain the soliton solutions of Equation (5) as follows:
- Family-II: When , and , then . So, we obtained the Soliton solutions of Equation (5) as follow,
- Family-III: When , and , then or . So, we obtain the soliton solutions of Equation (5) as follows:
- Family-IV: When , and , then or . So, we obtain the soliton solutions of Equation (5) as follows:
- Family-V: When , and , then or . So, we obtain the trigonometric solutions of Equation (5) as follows:
- Family-VI: When , and , then or . So, we obtain the trigonometric solutions of Equation (5) as follows:
- Family-VII: When , and , then . So, we obtain the trigonometric solutions of Equation (5) as follows:
- Family-VIII: When , and , then . So, we obtain the trigonometric solutions of Equation (5) as follows:
- Family-IX: When , and , then . So, we obtain the rational solutions of Equation (5) as follows:
4. Soliton Solutions for K–O System with It Sense
- Case 1.
- When we take , we obtain
- Case 2.
- When we take , we obtain
- Case 3.
- When we take , but , then we obtain
- Family-I: When , and , then . So, we obtain the soliton solutions of Equation (45) as follows:
- Family-II: When , and , then . So, we obtain the soliton solutions of Equation (45) as follows:
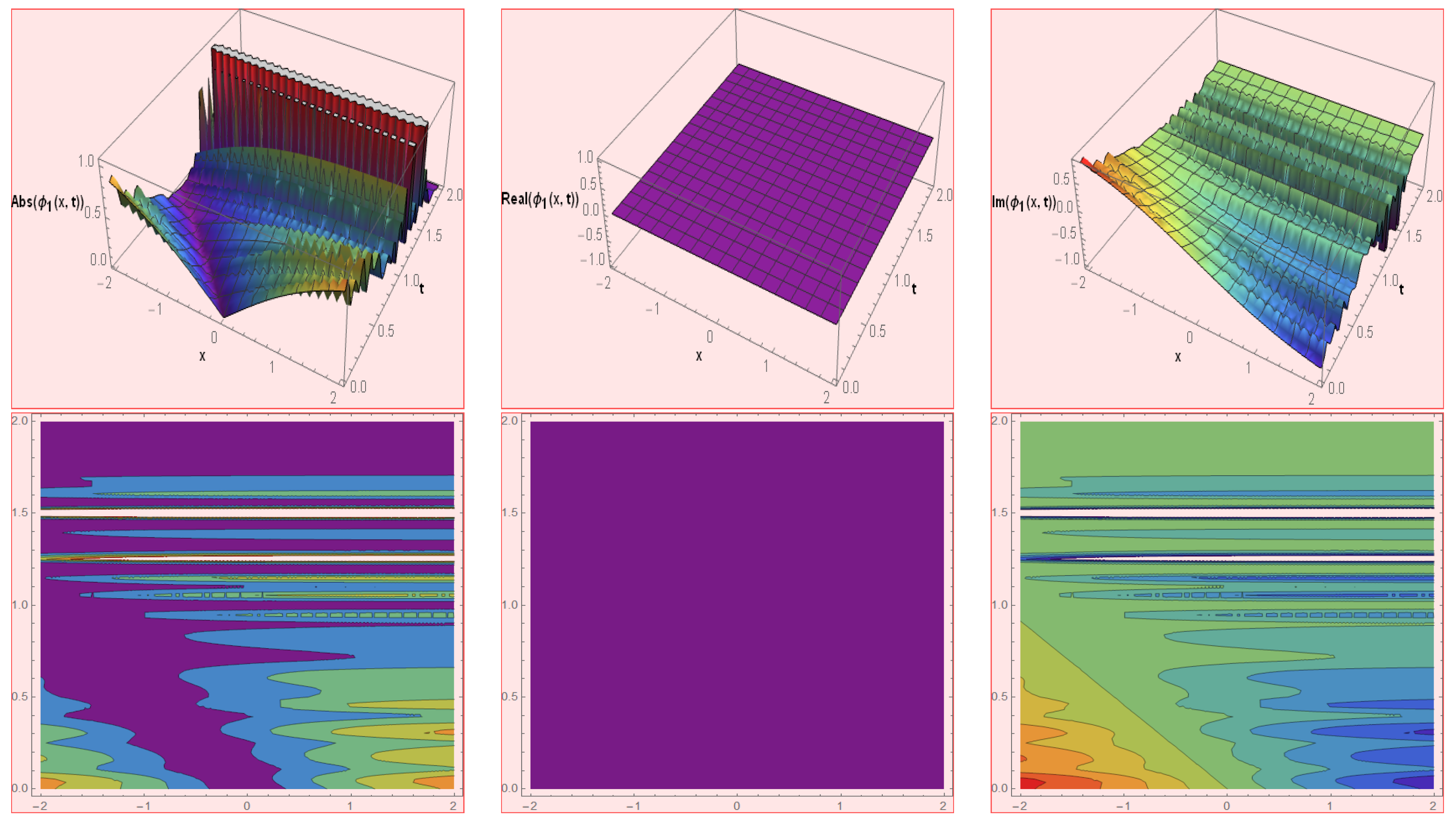
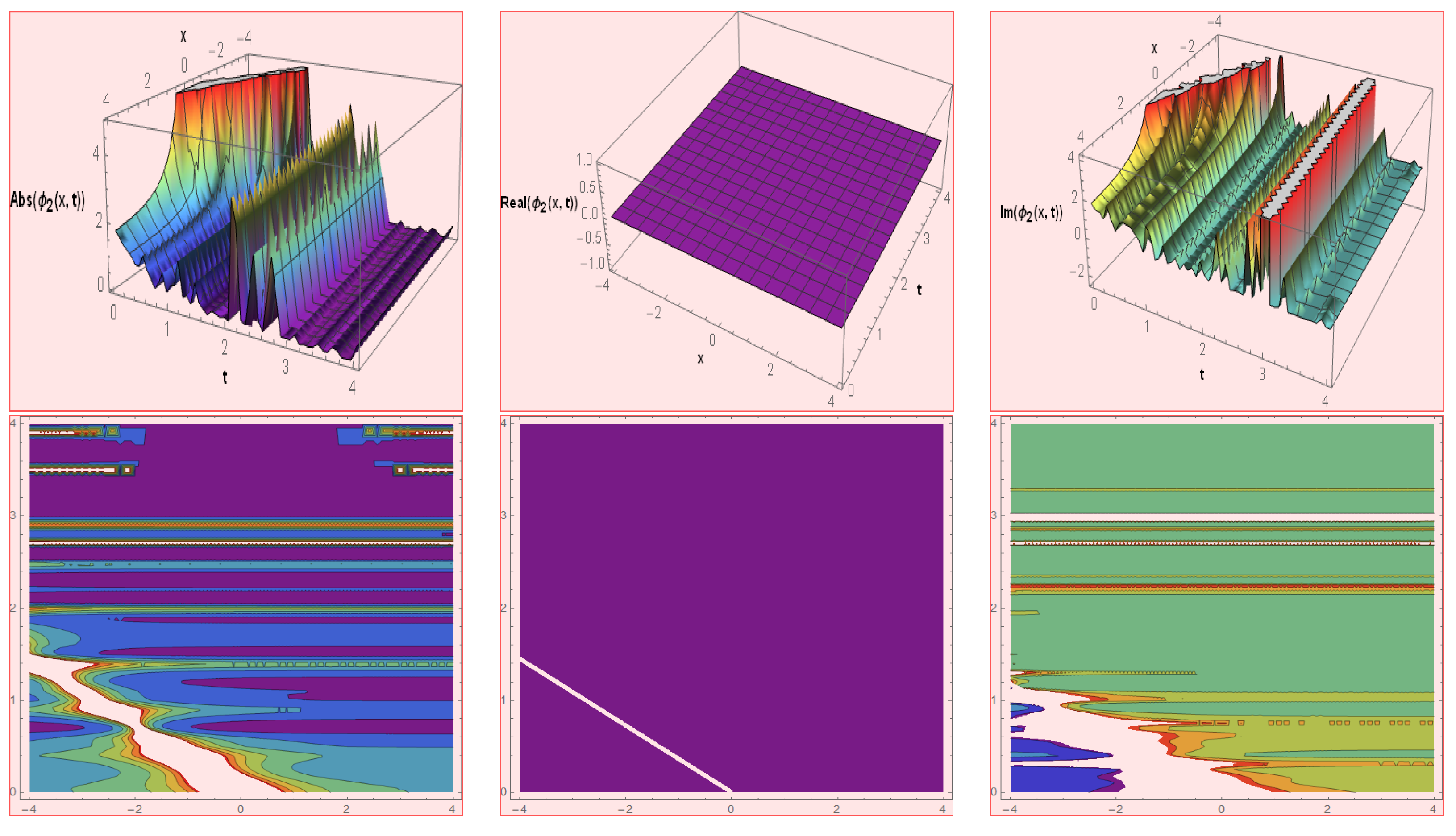
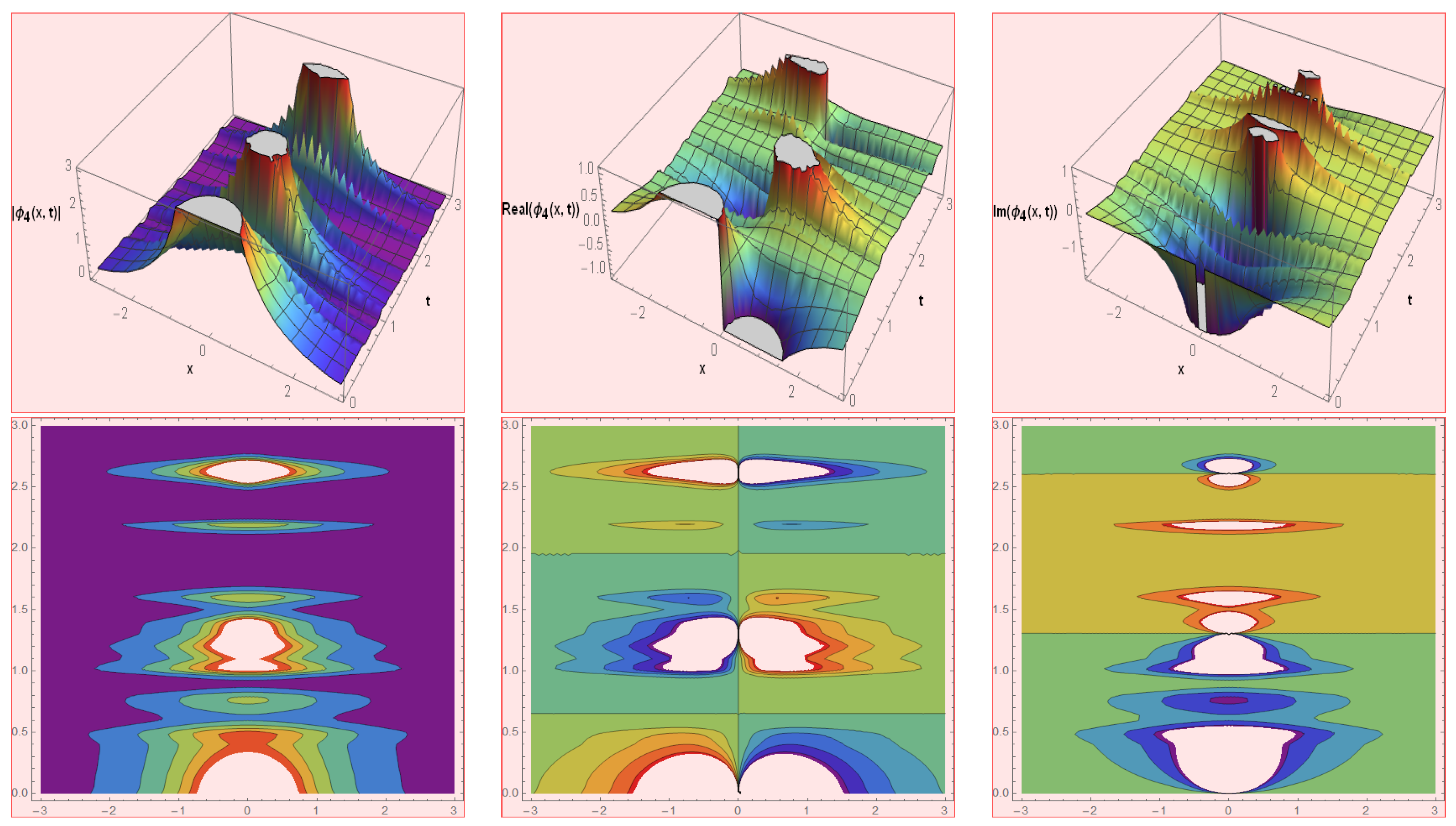
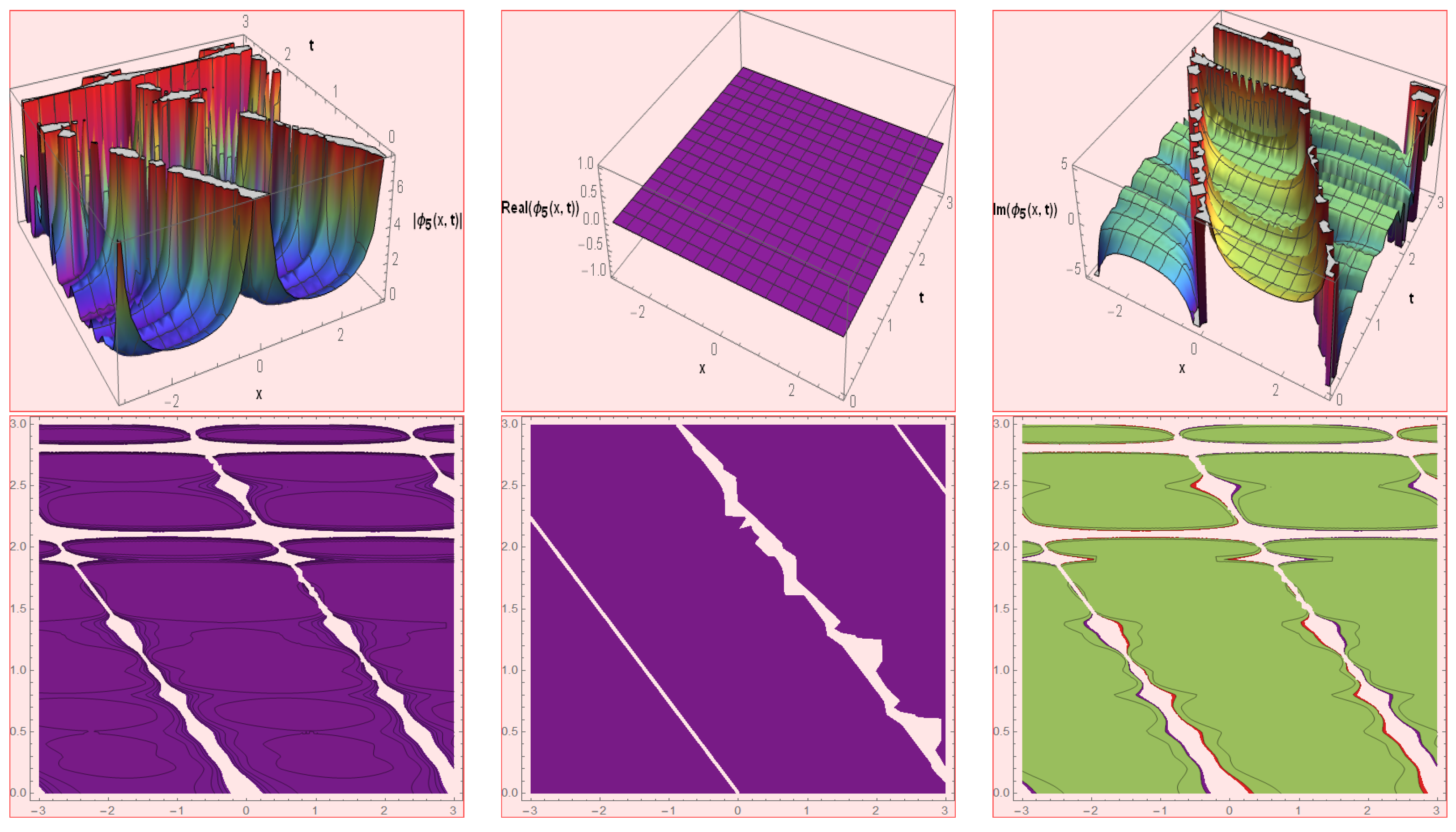
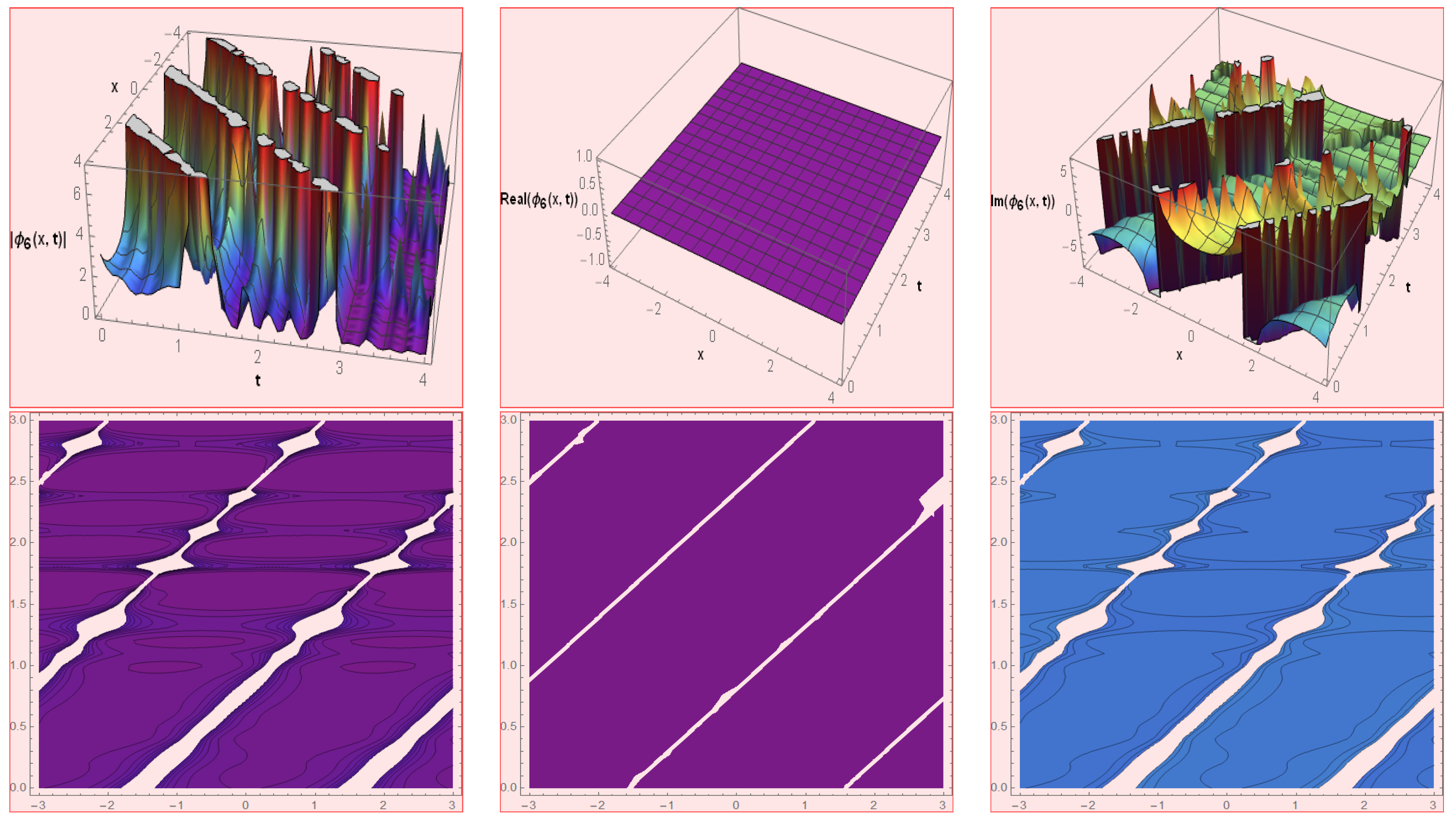
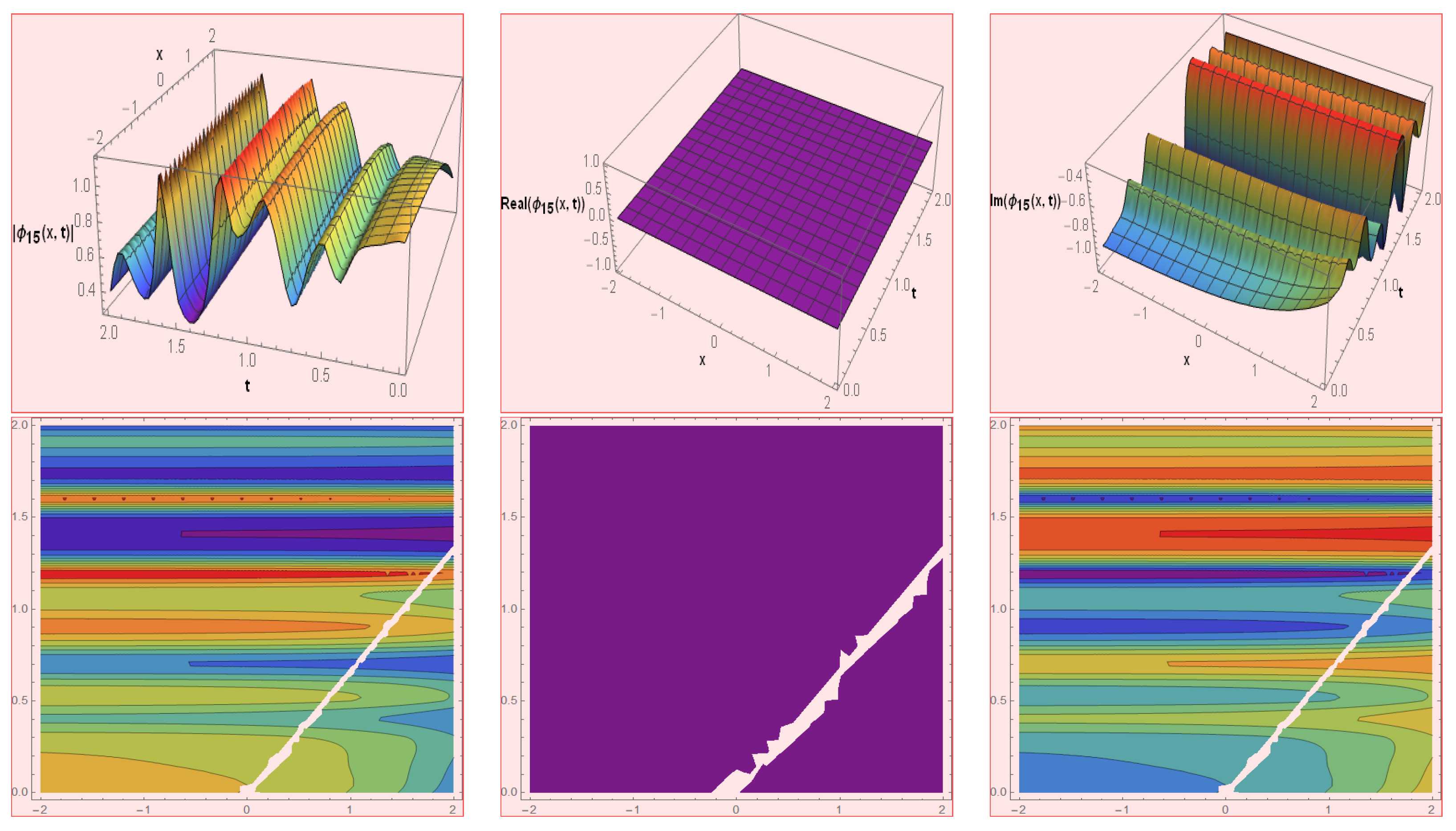
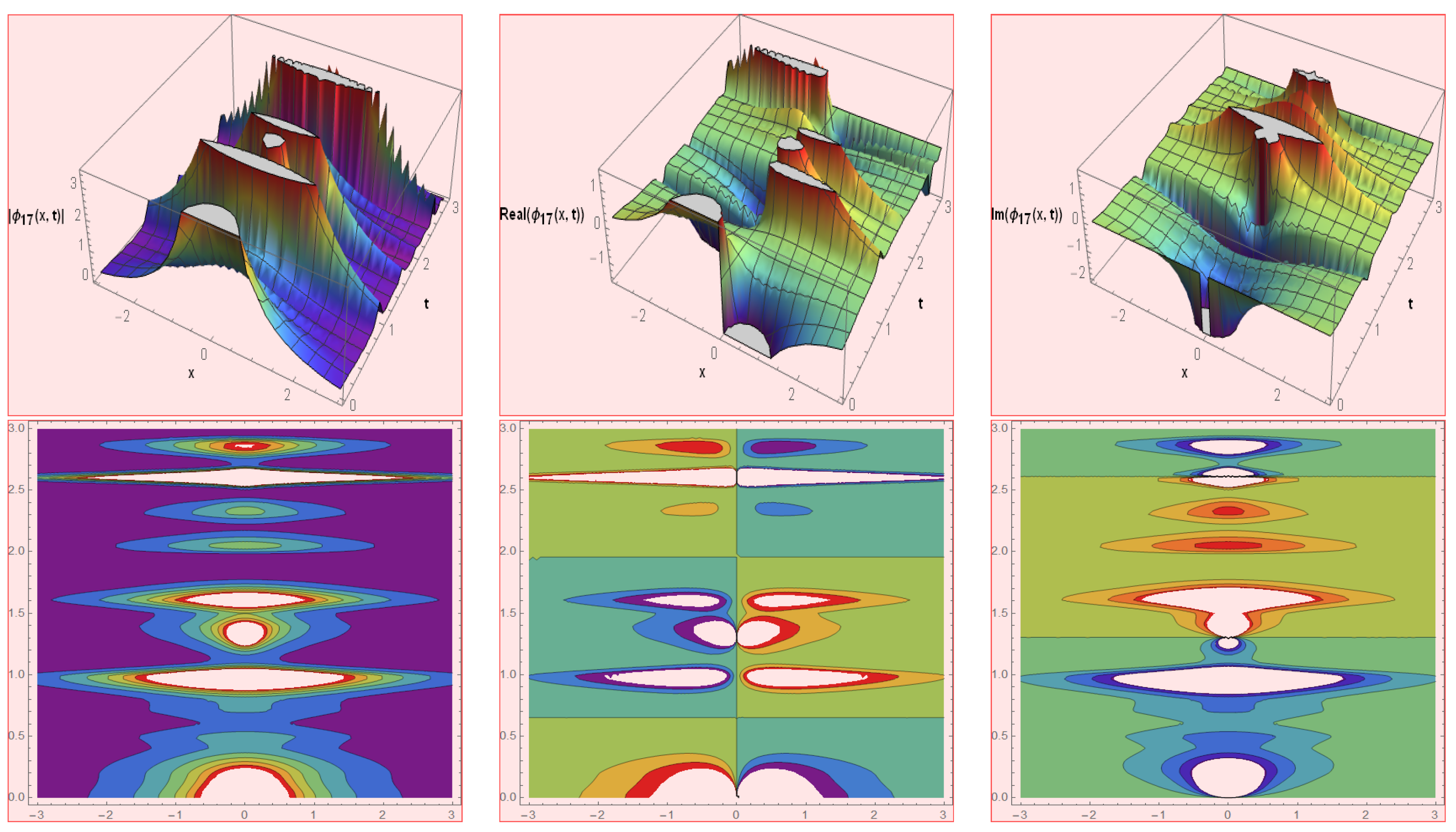
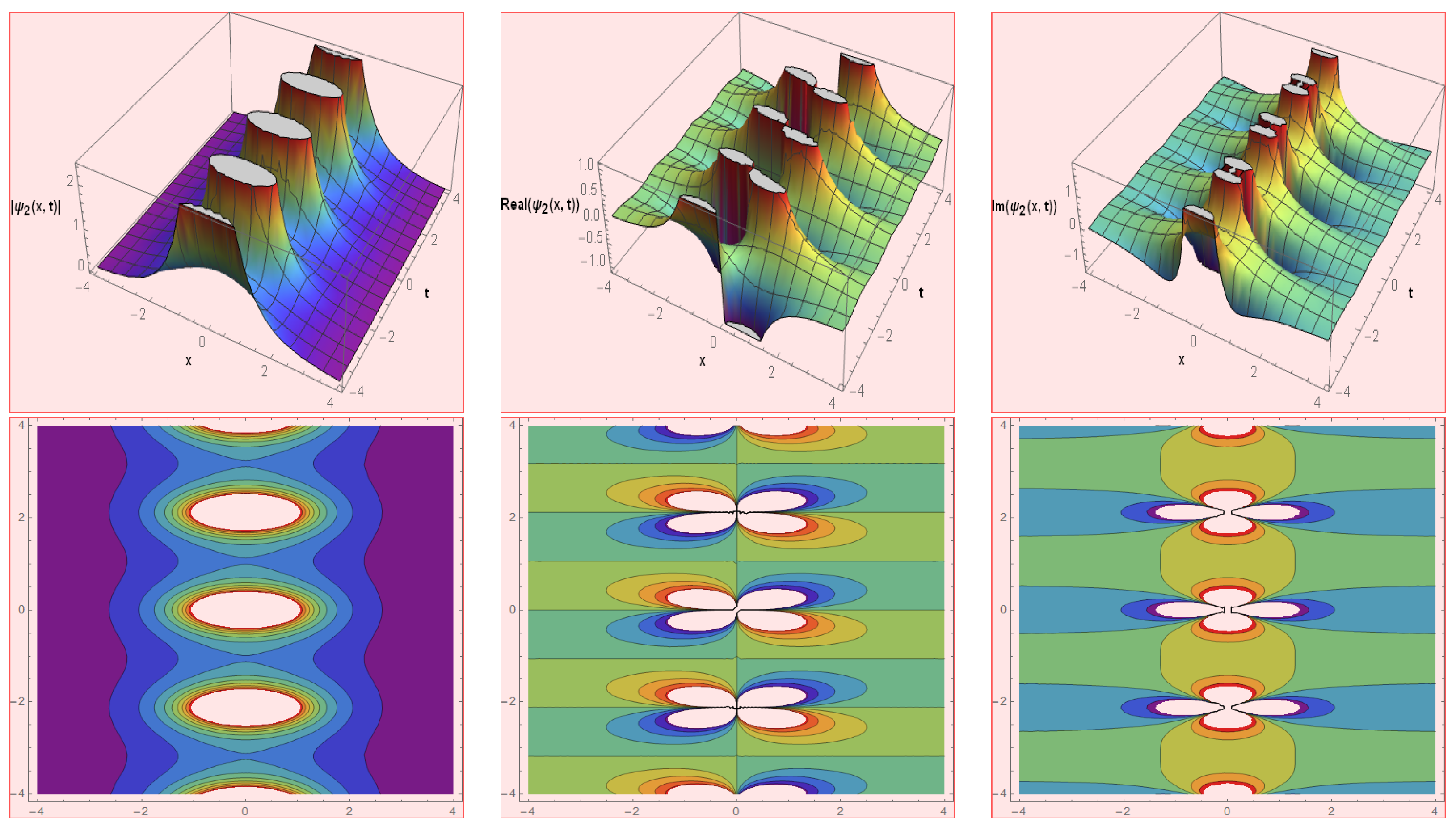
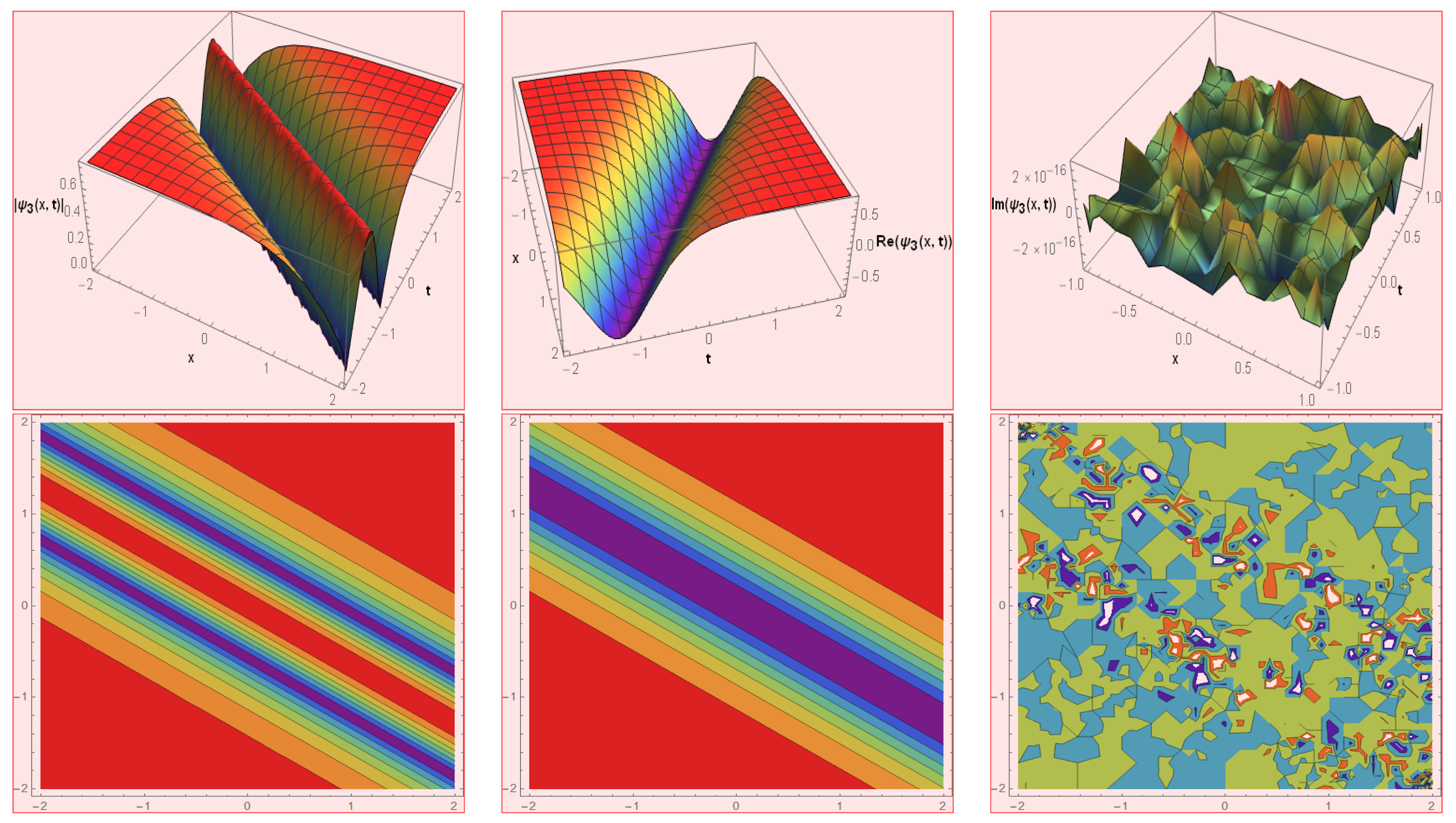
5. Graphical Discussion
| Algorithm 1 Pseudocode for WhiteNoise |
|
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sarwar, A.; Gang, T.; Arshad, M.; Ahmed, I.; Ahmad, M.O. Abundant solitary wave solutions for space-time fractional unstable nonlinear Schrödinger equations and their applications. Ain Shams Eng. J. 2023, 14, 101839. [Google Scholar] [CrossRef]
- Li, P.; Gao, R.; Xu, C.; Lu, Y.; Shang, Y. Dynamics in a Fractional Order Predator-Prey Model Involving Michaelis-Menten type Functional Response and both Unequal Delays. Fractals 2023. [Google Scholar] [CrossRef]
- Li, P.; Gao, R.; Xu, C.; Li, Y.; Akgül, A.; Baleanu, D. Dynamics exploration for a fractional-order delayed zooplankton–phytoplankton system. Chaos Solitons Fractals 2023, 166, 112975. [Google Scholar] [CrossRef]
- Iqbal, M.S.; Seadawy, A.R.; Baber, M.Z.; Qasim, M. Application of modified exponential rational function method to Jaulent–Miodek system leading to exact classical solutions. Chaos Solitons Fractals 2022, 164, 112600. [Google Scholar] [CrossRef]
- Taha, T.R.; Ablowitz, M.I. Analytical and numerical aspects of certain nonlinear evolution equations. II. Numerical, nonlinear Schrödinger equation. J. Comput. Phys. 1984, 55, 203–230. [Google Scholar] [CrossRef]
- Younis, M.; Seadawy, A.R.; Baber, M.Z.; Husain, S.; Iqbal, M.S.; Rizvi, S.T.R.; Baleanu, D. Analytical optical soliton solutions of the Schrödinger-Poisson dynamical system. Results Phys. 2021, 27, 104369. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Qahiti, R.; Ahmad, H.; Baili, J.; Mansour, F.E.; El-Morshedy, M. Exact solutions for the system of stochastic equations for the ion sound and Langmuir waves. Results Phys. 2021, 30, 104841. [Google Scholar] [CrossRef]
- Al-Askar, F.M.; Cesarano, C.; Mohammed, W.W. The analytical solutions of stochastic-fractional Drinfel’d-Sokolov-Wilson equations via (G′/G)-expansion method. Symmetry 2022, 14, 2105. [Google Scholar] [CrossRef]
- Burkardt, J.; Gunzburger, M.; Webster, C. Reduced order modeling of some nonlinear stochastic partial differential equations. Int. J. Numer. Anal. Model. 2007, 4, 368–391. [Google Scholar]
- Kamrani, M.; Hosseini, S.M.; Hausenblas, E. Implicit Euler method for numerical solution of nonlinear stochastic partial differential equations with multiplicative trace class noise. Math. Methods Appl. Sci. 2018, 41, 4986–5002. [Google Scholar] [CrossRef]
- Venturi, D.; Wan, X.; Mikulevicius, R.; Rozovskii, B.L.; Karniadakis, G.E. Wick–Malliavin approximation to nonlinear stochastic partial differential equations: Analysis and simulations. Proc. R. Soc. A Math. Phys. Eng. Sci. 2013, 469, 20130001. [Google Scholar] [CrossRef]
- Wang, X.; Yasin, M.W.; Ahmed, N.; Rafiq, M.; Abbas, M. Numerical approximations of stochastic Gray–Scott model with two novel schemes. Aims Math. 2023, 8, 5124–5147. [Google Scholar] [CrossRef]
- Luo, W. Wiener Chaos Expansion and Numerical Solutions of Stochastic Partial Differential Equations; California Institute of Technology: Pasadena, CA, USA, 2006. [Google Scholar]
- Yasin, M.W.; Ahmed, N.; Iqbal, M.S.; Raza, A.; Rafiq, M.; Eldin, E.M.T.; Khan, I. Spatio-temporal numerical modeling of stochastic predator-prey model. Sci. Rep. 2023, 13, 1990. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Iqbal, N.; Ali, A.; El-Morshedy, M. Exact solutions of the stochastic new coupled Konno-Oono equation. Results Phys. 2021, 21, 103830. [Google Scholar] [CrossRef]
- Mohammed, W.W.; El-Morshedy, M. The influence of multiplicative noise on the stochastic exact solutions of the Nizhnik–Novikov–Veselov system. Math. Comput. Simul. 2021, 190, 192–202. [Google Scholar] [CrossRef]
- Khater, M.M.; Seadawy, A.R.; Lu, D. Dispersive solitary wave solutions of new coupled Konno-Oono, Higgs field and Maccari equations and their applications. J. King Saud Univ.-Sci. 2018, 30, 417–423. [Google Scholar] [CrossRef]
- Elbrolosy, M.E.; Elmandouh, A.A. Dynamical behaviour of conformable time-fractional coupled Konno-Oono equation in magnetic field. Math. Probl. Eng. 2022, 2022, 3157217. [Google Scholar] [CrossRef]
- Manafian, J.; Zamanpour, I.; Ranjbaran, A. On some new analytical solutions for new coupled Konno-Oono equation by the external trial equation method. J. Phys. Commun. 2018, 2, 015023. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.; Alshreef, G. Closed-form solutions to the new coupled Konno-Oono equation and the Kaup–Newell model equation in magnetic field with novel statistic application. Eur. Phys. J. Plus 2021, 136, 455. [Google Scholar] [CrossRef]
- Alam, M.N.; Belgacem, F.B.M. New Generalized (G′/G)-Expansion Method Applications to Coupled Konno-Oono Equation. Adv. Pure Math. 2016, 6, 168. [Google Scholar] [CrossRef]
- Mirhosseini-Alizamini, S.M.; Rezazadeh, H.; Srinivasa, K.; Bekir, A. New closed form solutions of the new coupled Konno-Oono equation using the new extended direct algebraic method. Pramana 2020, 94, 52. [Google Scholar] [CrossRef]
- Wang, K.J. Abundant analytical solutions to the new coupled Konno-Oono equation arising in magnetic field. Results Phys. 2021, 31, 104931. [Google Scholar] [CrossRef]
- Abdou, M.A. Further improved F-expansion and new exact solutions for nonlinear evolution equations. Nonlinear Dyn. 2008, 52, 277–288. [Google Scholar] [CrossRef]
- Wang, D.; Zhang, H.Q. Further improved F-expansion method and new exact solutions of Konopelchenko–Dubrovsky equation. Chaos Solitons Fractals 2005, 25, 601–610. [Google Scholar] [CrossRef]
- Ali Akbar, M.; Ali, N.H.M. The improved F-expansion method with Riccati equation and its applications in mathematical physics. Cogent Math. 2017, 4, 1282577. [Google Scholar] [CrossRef]
- Navickas, Z.; Timofejeva, I.; Telksnys, T.; Marcinkevicius, R.; Ragulskis, M. Construction of special soliton solutions to the stochastic Riccati equation. Open Math. 2022, 20, 829–844. [Google Scholar] [CrossRef]
- Mohammed, W.W.; El-Morshedy, M.; Moumen, A.; Ali, E.E.; Benaissa, M.; Abouelregal, A.E. Effects of M-Truncated Derivative and Multiplicative Noise on the Exact Solutions of the Breaking Soliton Equation. Symmetry 2023, 15, 288. [Google Scholar] [CrossRef]
- Al-Askar, F.M.; Mohammed, W.W.; El-Morshedy, M. The analytical solutions for stochastic fractional–space burgers’ equation. J. Math. 2022, 2022, 9878885. [Google Scholar] [CrossRef]
- Al-Askar, F.M.; Mohammed, W.W. The analytical solutions of the stochastic fractional RKL equation via Jacobi elliptic function method. Adv. Math. Phys. 2022, 2022, 1534067. [Google Scholar] [CrossRef]
- Lou, Y.; Hu, G.; Christofides, P.D. Model predictive control of nonlinear stochastic partial differential equations with application to a sputtering process. AIChE J. 2008, 54, 2065–2081. [Google Scholar] [CrossRef]
- Calin, O. An Informal Introduction to Stochastic Calculus with Applications; World Scientific Publishing: Singapore, 2015; Chapter 7; pp. 149–164. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shaikh, T.S.; Baber, M.Z.; Ahmed, N.; Shahid, N.; Akgül, A.; De la Sen, M. On the Soliton Solutions for the Stochastic Konno–Oono System in Magnetic Field with the Presence of Noise. Mathematics 2023, 11, 1472. https://doi.org/10.3390/math11061472
Shaikh TS, Baber MZ, Ahmed N, Shahid N, Akgül A, De la Sen M. On the Soliton Solutions for the Stochastic Konno–Oono System in Magnetic Field with the Presence of Noise. Mathematics. 2023; 11(6):1472. https://doi.org/10.3390/math11061472
Chicago/Turabian StyleShaikh, Tahira Sumbal, Muhammad Zafarullah Baber, Nauman Ahmed, Naveed Shahid, Ali Akgül, and Manuel De la Sen. 2023. "On the Soliton Solutions for the Stochastic Konno–Oono System in Magnetic Field with the Presence of Noise" Mathematics 11, no. 6: 1472. https://doi.org/10.3390/math11061472
APA StyleShaikh, T. S., Baber, M. Z., Ahmed, N., Shahid, N., Akgül, A., & De la Sen, M. (2023). On the Soliton Solutions for the Stochastic Konno–Oono System in Magnetic Field with the Presence of Noise. Mathematics, 11(6), 1472. https://doi.org/10.3390/math11061472







