Hybrid Fixed Point Theorems of Fuzzy Soft Set-Valued Maps with Applications in Integral Inclusions and Decision Making
Abstract
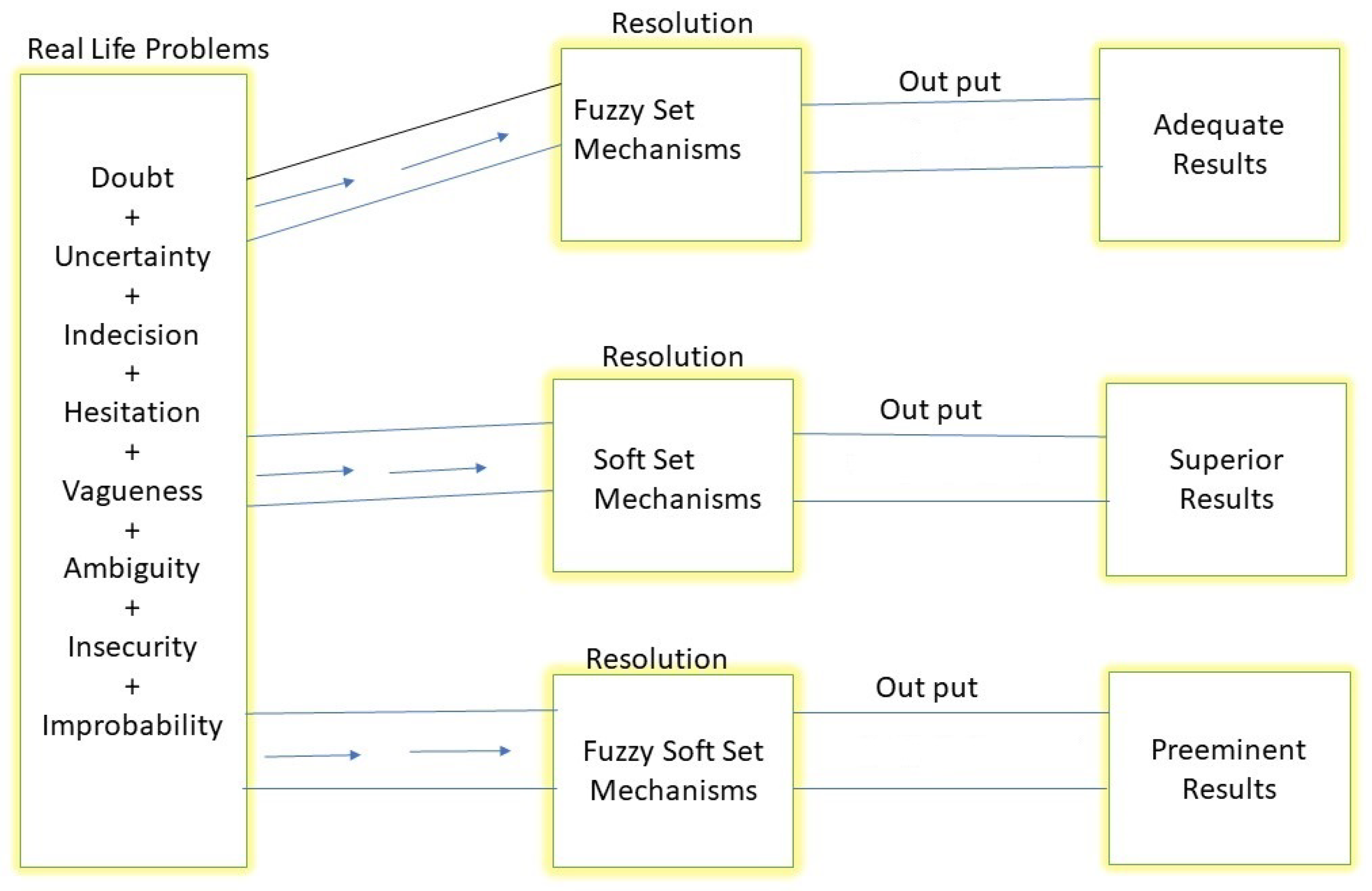
1. Introduction
2. Preliminaries
- (i)
- if and only if ;
- (ii)
- ;
- (iii)
- .
- (i)
- Convergent if, and only if, we can find for which as , and we write this as .
- (ii)
- Cauchy if, and only if, as .
- (iii)
- The b-metric Υ is said to be complete if every Cauchy sequence in Υ is convergent.
- (i)
- Compact if, and only if, for every sequence of elements of A, we can find a subsequence that converges to an element of A.
- (ii)
- Closed if, and only if, for every sequence of elements of A that converges to an element γ, we have .
- (i)
- A c-comparison function if as for every ;
- (ii)
- A b-comparison function if we can find , and a convergent non-negative series for which , for and any , where denotes the iterate of Δ.
- (i)
- is a b-comparison function;
- (ii)
- if, and only if, ;
- (iii)
- is continuous.
- (i)
- Each iterate is also a b-comparison function;
- (ii)
- for all .
3. Decision Making Using Fuzzy Soft Set-Valued Maps
4. Hybrid Invariant Point Theorems of Fuzzy Soft Set-Valued Maps
- Case 1:
- . Suppose that , then from (5), we have
- Case 2:
- . Employing (1) on account of b-comparison of , we have
5. Solvability of Fredholm-Type Integral Inclusions via Fuzzy Soft Set-Valued Maps
- For every , the map is lower semi-continuous;
- ;
- we can find a b-comparison function for which for all ,for each and , where and .Then, the integral inclusion (17) possesses solutions in .
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Czerwik, S. Contraction mappings in b-metric spaces. Acta Math. Inform. Univ. Ostrav. 1993, 1, 5–11. [Google Scholar]
- Hussain, N.; Mitrović, Z.D. On multi-valued weak quasi-contractions in b-metric spaces. J. Nonlinear Sci. Appl. 2017, 10, 3815–3823. [Google Scholar] [CrossRef]
- Kutbi, M.A.; Karapınar, E.; Ahmad, J.; Azam, A. Some fixed point results for multi-valued mappings in b-metric spaces. J. Inequal. Appl. 2014, 2014, 126. [Google Scholar] [CrossRef]
- Monairah, A.; Shehu, S.M.; Akbar, A.; Nawab, H. On multivalued hybrid contractions with applications. J. Funct. Spaces 2020, 2020, 8401403. [Google Scholar] [CrossRef]
- Rao, K.P.R.; Swamy, P.R.; Prasad, J.R. A common fixed point theorem in complex valued b-metric spaces. Bull. Math. Stat. Res. 2013, 1, 1–8. [Google Scholar]
- Tomonari, S. Basic inequality on a b-metric space and its applications. J. Inequal. Appl. 2017, 2017, 256. [Google Scholar]
- Karapinar, E.; Fulga, A. New hybrid contractions on b-metric spaces. Mathematics 2019, 7, 578. [Google Scholar] [CrossRef]
- Molodtsov, D. Soft set theory—First results. Comput. Math. Appl. 1999, 37, 19. [Google Scholar] [CrossRef]
- Yang, X.; Yu, D.; Yang, J.; Wu, C. Generalization of soft set theory: From crisp to fuzzy case. Fuzzy Inf. Eng. 2007, 345–354. [Google Scholar] [CrossRef]
- Maji, P.K.; Biswas, P.; Roy, A.R. Fuzzy soft sets. J. Fuzzy Math. 2001, 589–602. [Google Scholar] [CrossRef]
- Mohammed, S.S.; Azam, A. An algorithm for fuzzy soft set based decision making approach. Yugosl. J. Oper. Res. 2020, 30, 59–70. [Google Scholar] [CrossRef]
- Mohammed, S.S.; Azam, A. Fixed points of soft-set valued and fuzzy set-valued maps with applications. J. Intell. Fuzzy Syst. 2019, 37, 3865–3877. [Google Scholar] [CrossRef]
- Mohammed, S.S.; Azam, A. Integral type contractions of soft set-valued maps with application to neutral differential equation. AIMS Math. 2019, 5, 342–358. [Google Scholar]
- Frigon, M.; O’Regan, D. Fuzzy contractive maps and fuzzy fixed points. Fuzzy Sets Syst. 2002, 129, 39–45. [Google Scholar] [CrossRef]
- Boriceanu, M. Fixed point theory for multivalued generalized contraction on a set with two b-metrics. Mathematica 2009, 4, 126–132. [Google Scholar]
- Kannan, R.; O’Regan, D. A note on the solutions of integral inclusions. J. Integr. Equ. Appl. 2000, 12, 85–94. [Google Scholar] [CrossRef]
- Rus, I.A. Generalized Contractions and Applications; Cluj University Press: Cluj-Napoca, Romania, 2001; Volume 2, pp. 60–71. [Google Scholar]
- Mohammed, S.S. On fuzzy soft set-valued maps with application. J. Niger. Soc. Phys. Sci. 2020, 2, 26–35. [Google Scholar] [CrossRef]
- Michael, E. A selection theorem. Proc. Am. Math. Soc. 1966, 17, 1404–1406. [Google Scholar] [CrossRef]
- Aleksić, S.; Mitrovic, Z.; Radenovic, S. Picard sequences in b-metric spaces. Fixed Point Theory 2020, 21, 35–46. [Google Scholar] [CrossRef]
- Berinde, V. Generalized contractions in quasimetric spaces. In Seminar on Fixed Point Theory; Babes-Bolyai University: Cluj-Napoca, Romania, 1993; pp. 3–9. [Google Scholar]
- Debnath, P.; Konwar, N.; Radenovic, S. Metric Fixed Point Theory: Applications in Science, Engineering and Behavioural Sciences; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
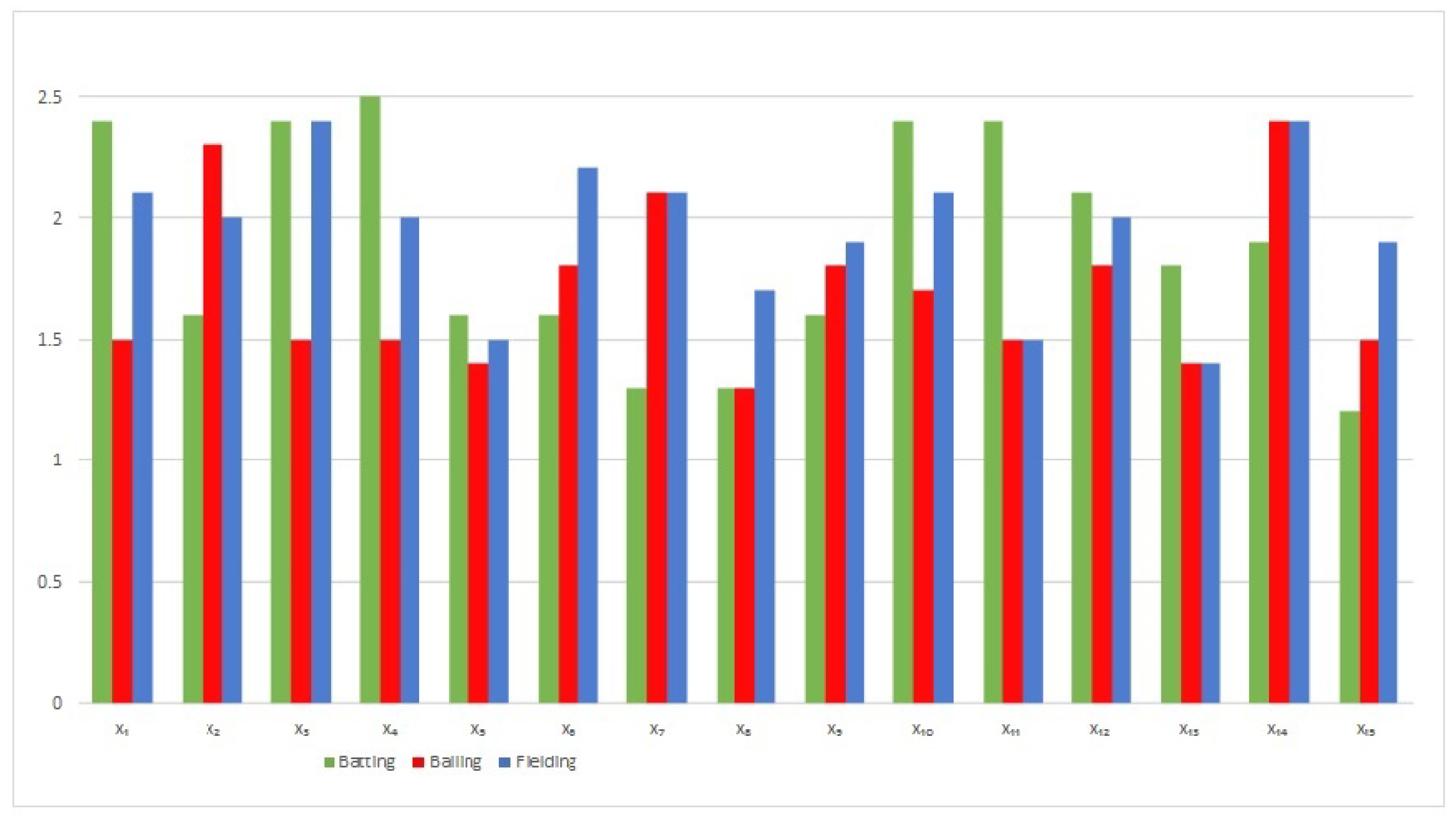

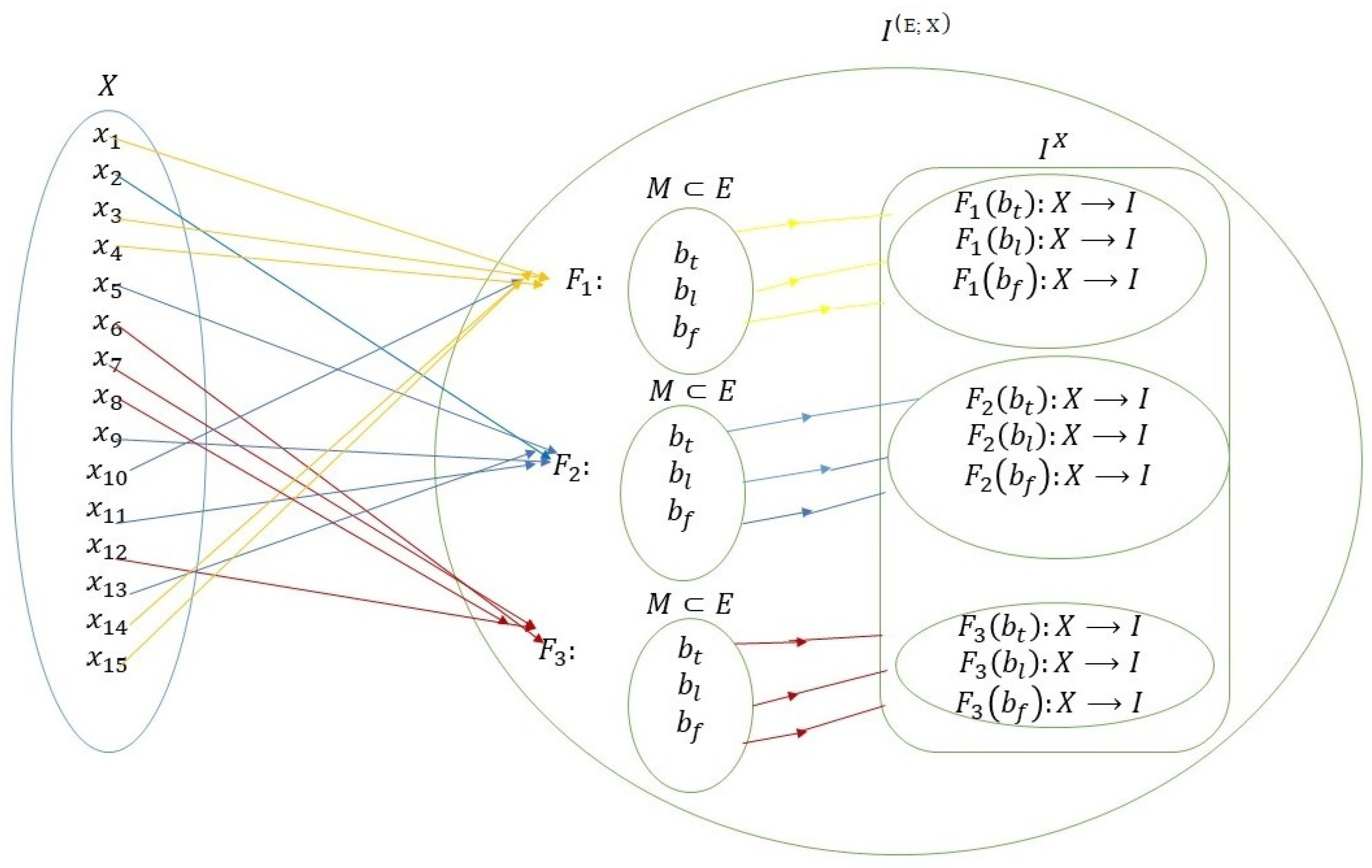
| Players | S | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.8 | 0.7 | 0.9 | 2.4 | 0.4 | 0.5 | 0.6 | 1.5 | 0.7 | 0.8 | 0.6 | 2.1 | 24.3 | |
| 0.5 | 0.5 | 0.6 | 1.6 | 0.8 | 0.7 | 0.8 | 2.3 | 0.6 | 0.7 | 0.7 | 2.0 | 23.2 | |
| 0.7 | 0.8 | 0.9 | 2.4 | 0.4 | 0.5 | 0.6 | 1.5 | 0.9 | 0.7 | 0.8 | 2.4 | 25.2 | |
| 0.9 | 0.8 | 0.8 | 2.5 | 0.4 | 0.6 | 0.5 | 1.5 | 0.8 | 0.6 | 0.6 | 2.0 | 24.5 | |
| 0.5 | 0.5 | 0.6 | 1.6 | 0.5 | 0.4 | 0.5 | 1.4 | 0.6 | 0.4 | 0.5 | 1.5 | 18.1 | |
| 0.5 | 0.5 | 0.6 | 1.6 | 0.5 | 0.6 | 0.7 | 1.8 | 0.7 | 0.7 | 0.8 | 2.2 | 21.8 | |
| 0.4 | 0.4 | 0.5 | 1.3 | 0.7 | 0.8 | 0.6 | 2.1 | 0.6 | 0.7 | 0.8 | 2.1 | 21.2 | |
| 0.4 | 0.4 | 0.5 | 1.3 | 0.4 | 0.5 | 0.4 | 1.3 | 0.6 | 0.6 | 0.5 | 1.7 | 16.8 | |
| 0.5 | 0.5 | 0.6 | 1.6 | 0.5 | 0.6 | 0.7 | 1.8 | 0.6 | 0.6 | 0.7 | 1.9 | 20.9 | |
| 0.9 | 0.8 | 0.7 | 2.4 | 0.6 | 0.5 | 0.6 | 1.7 | 0.7 | 0.8 | 0.6 | 2.1 | 25.1 | |
| 0.7 | 0.8 | 0.9 | 2.4 | 0.5 | 0.4 | 0.6 | 1.5 | 0.5 | 0.4 | 0.6 | 1.5 | 22.5 | |
| 0.6 | 0.7 | 0.8 | 2.1 | 0.5 | 0.6 | 0.7 | 1.8 | 0.7 | 0.6 | 0.7 | 2.0 | 23.7 | |
| 0.5 | 0.6 | 0.7 | 1.8 | 0.5 | 0.4 | 0.5 | 1.4 | 0.4 | 0.4 | 0.6 | 1.4 | 18.8 | |
| 0.6 | 0.7 | 0.6 | 1.9 | 0.7 | 0.8 | 0.9 | 2.4 | 0.8 | 0.9 | 0.7 | 2.4 | 26.3 | |
| 0.4 | 0.4 | 0.4 | 1.2 | 0.5 | 0.6 | 0.4 | 1.5 | 0.6 | 0.7 | 0.6 | 1.9 | 17.3 |
| Coaches∖Players | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ✓ | ✓ | ✓ | ✓ | ✓ | ✓ | ||||||||||
| ✓ | ✓ | ✓ | ✓ | ✓ | |||||||||||
| ✓ | ✓ | ✓ | ✓ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shagari, M.S.; Noorwali, M.; Azam, A. Hybrid Fixed Point Theorems of Fuzzy Soft Set-Valued Maps with Applications in Integral Inclusions and Decision Making. Mathematics 2023, 11, 1393. https://doi.org/10.3390/math11061393
Shagari MS, Noorwali M, Azam A. Hybrid Fixed Point Theorems of Fuzzy Soft Set-Valued Maps with Applications in Integral Inclusions and Decision Making. Mathematics. 2023; 11(6):1393. https://doi.org/10.3390/math11061393
Chicago/Turabian StyleShagari, Mohammed Shehu, Maha Noorwali, and Akbar Azam. 2023. "Hybrid Fixed Point Theorems of Fuzzy Soft Set-Valued Maps with Applications in Integral Inclusions and Decision Making" Mathematics 11, no. 6: 1393. https://doi.org/10.3390/math11061393
APA StyleShagari, M. S., Noorwali, M., & Azam, A. (2023). Hybrid Fixed Point Theorems of Fuzzy Soft Set-Valued Maps with Applications in Integral Inclusions and Decision Making. Mathematics, 11(6), 1393. https://doi.org/10.3390/math11061393







