Properties of Coordinated h₁,h₂-Convex Functions of Two Variables Related to the Hermite–Hadamard–Fejér Type Inequalities

Abstract

In this paper, we prove the Hermite–Hadamard–Fejér type inequalities for coordinated $\left(h_1,h_2\right)$-convex functions on the rectangle from the plane ${\mathbb{R}}^2$. Some generalizations of the Hermite–Hadamard-type inequalities of two variables are also obtained as a consequence. Some properties of two functionals which are connected with the coordinated $\left(h_1,h_2\right)$-convex functions are provided as well. Finally, we give applications of the acquired results to special means of positive real numbers.

1. Introduction

Definition 1.

Let I be an interval of real numbers. The function $\phi :I\to \mathbb{R}$ is said to be convex on I if for all $\theta ,\lambda \in I$ and $\alpha \in [0,1]$, one has the inequality:

$$\phi (\alpha \theta +(1-\alpha \left)\lambda \right)\le \alpha f\left(\theta \right)+(1-\alpha )\phi \left(\lambda \right)$$

Let $\phi :I\subseteq \mathbb{R}\to \mathbb{R}$ be a convex function and ${\sigma }_1,{\sigma }_2\in I$ with ${\sigma }_1<{\sigma }_2$. Then, the following double inequality:

$$\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2}\right)\le \frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta \right)d\theta \le \frac{\phi \left({\sigma }_1\right)+\phi \left({\sigma }_2\right)}{2}$$

(1)

is known as the Hermite–Hadamard inequality for convex mapping. The inequalities in (1) hold in reverse if $\phi$ is a concave function.

In [1], Hudzik and Maligranda introduced the definition of s-convexity of real valued functions.

Definition 2.

Let $s\in \left(0,1\right]$. A function $\phi :\left[0,\infty \right)\to \mathbb{R}$ is s-convex in the second sense provided

$$\phi (\alpha \theta +(1-\alpha )\lambda )\le {\alpha }^{s}f\left(\theta \right)+{(1-\alpha )}^s\phi \left(\lambda \right)$$

for all θ, $\lambda \in \left[0,\infty \right)$ and $\alpha \in \left[0,1\right]$. The class of s-convex functions is denoted by $K_s^2$.

Remark 1.

It is clear that s-convexity means just the convexity when $s=1$.

In [2], Dragomir and Fitzpatrick proved the following variant of Hermite–Hadamard’s inequality which holds for s-convex function in the second sense:

Theorem 1

([2]). Suppose that $\phi :[0,\infty )\to [0,\infty )$ is an s-convex function in the second sense, where $s\in (0,1)$ and let ${\sigma }_1,{\sigma }_2\in [0,\infty )$, ${\sigma }_1<{\sigma }_2$. If $\phi \in L_1\left([{\sigma }_1,{\sigma }_2]\right)$, then the following inequalities hold:

$$2^{s-1}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2}\right)\le \frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta \right)d\theta \le \frac{\phi \left({\sigma }_1\right)+\phi \left({\sigma }_2\right)}{s+1}$$

(2)

The constant $\frac{1}{s+1}$ is the best possible in the second inequality in (2).

In the paper [3], Varošanec considered a larger class of non-negative functions, which is known as the class of h-convex functions. This class contains several well-known classes of functions such as non-negative convex functions, s-convex functions in the second sense, Godunova–Levin functions and P-functions.

Definition 3

([3]). Let $h:J\subseteq \mathbb{R}\to \mathbb{R}$, where $(0,1)\subseteq J$, be a positive function. A function $\phi :I\subseteq \mathbb{R}\to \mathbb{R}$ is said to be h-convex or φ is said to belong to the class $SX(h,I)$, if φ is non-negative for all $\theta ,\lambda \in I$, $\alpha \in (0,1)$, we have

$$\phi (\alpha \theta +(1-\alpha \left)\lambda \right)\le h\left(\alpha \right)\phi \left(\theta \right)+h(1-\alpha )\phi \left(\lambda \right)$$

if the inequality is reversed then φ is said to be h-concave, and we say that φ belongs to the class $SV(h,I)$.

Fejér [4] established the following double inequality as a weighted generalization of (1):

$$\begin{array}{cc}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2}\right){\int }_{{\sigma }_1}^{{\sigma }_2}\mu \left(\theta \right)d\theta \le \frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta \right)\mu \left(\theta \right)d\theta \hfill & \\ & \hfill \le \frac{\phi \left({\sigma }_1\right)+\phi \left({\sigma }_2\right)}{2}{\int }_{{\sigma }_1}^{{\sigma }_2}\mu \left(\theta \right)d\theta ,\end{array}$$

(3)

where $\phi :I⟶\mathbb{R}$, $\varnothing \ne I\subseteq \mathbb{R}$, ${\sigma }_1,{\sigma }_2\in I$ with ${\sigma }_1<{\sigma }_2$ is any convex function, and $\mu :\left[{\sigma }_1,{\sigma }_2\right]\to \mathbb{R}$ is non-negative integrable symmetric function about $\theta =\frac{{\sigma }_1+{\sigma }_2}{2}$.

Bombardelli and Varošanec [5] discussed that there no change in the properties associated with the integral mean of the function $\phi$ if the class of convex functions is extended to the class of h-convex functions. Bombardelli and Varošanec also proved Hermite–Hadamard–Fejér inequalities for an h-convex function and discussed particular cases for other classes of functions such as convex functions and s-convex functions. It has also been observed in this research that the left-hand side inequality of their result is stronger than the right-hand side inequality in that result. This study also contains several characteristics of the functions $H,F:[0,1]\to \mathbb{R}$ defined by

$$H\left(\tau \right)=\frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\tau \theta +\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2}\right)d\theta$$

and

$$F\left(\tau ,s\right)=\frac{1}{{\left({\sigma }_2-{\sigma }_1\right)}^2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda \right)d\lambda d\theta$$

that arise when the function $\phi$ is an h-convex function.

Let us now discuss some defintions and results related to coordinated convex, coordinated s-convex functions and $\left(h_1,h_2\right)$-convex function on a rectangle $\Delta =:[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ in ${\mathbb{R}}^2$.

Let us now consider a bidimensional interval $\Delta =:[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ in ${\mathbb{R}}^2$ with ${\sigma }_1<{\sigma }_2$ and ${\nu }_1<{\nu }_2$. A mapping $\phi :\Delta \to \mathbb{R}$ is said to be convex on $\Delta$ if the following inequality:

$$\phi (\alpha \theta +(1-\alpha )z,\alpha \lambda +(1-\alpha \left)w\right)\le \alpha f(\theta ,\lambda )+(1-\alpha )\phi (z,w)$$

holds for all $(\theta ,\lambda ),(z,w)\in \Delta$ and $\alpha \in$$[0,1]$ .

A modification for convex functions also known as coordinated convex functions was introduced by Dragomir in [6].

Definition 4

([6]). A function $\phi :\Delta \to \mathbb{R}$ is said to be convex on the coordinates on Δ if the partial mappings ${\phi }_{\lambda }:[{\sigma }_1,{\sigma }_2]\to \mathbb{R},{\phi }_{\lambda }\left(u\right)=\phi (u,\lambda )$ and ${\phi }_{\theta }:[{\nu }_1,{\nu }_2]\to \mathbb{R},{\phi }_{\theta }\left(v\right)=\phi (\theta ,v)$ are convex where defined for all $\theta \in [{\sigma }_1,{\sigma }_2]\lambda \in [{\nu }_1,{\nu }_2]$.

A formal definition for coordinated convex functions may be stated as follows:

Definition 5

([7]). A function $\phi :\Delta \to \mathbb{R}$ is said to be convex on the coordinates on Δ if the following inequality:

$$\begin{array}{cc}\phi (\tau \theta +(1-\tau )\lambda ,su+(1-s\left)w\right)\hfill & \\ \hfill \le \tau sf(\theta ,u)+\tau (1-s)\phi (\theta ,w)+s(1-\tau )\phi (\lambda ,u)+(1-\tau )(1-s)\phi (\lambda ,w)\end{array}$$

holds for all $\tau ,s\in [0,1]$ and $(\theta ,\lambda ),(u,w)\in \Delta$.

Clearly, every convex mapping $\phi :\Delta \to \mathbb{R}$ is convex on the coordinates. Furthermore, there exists a coordinated convex function which is not convex, see for instance the reference [6].

The concept of s-convex functions and s-convex functions on the coordinates on $\Delta =:[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ from the plane $\mathbb{R}$ in the second sense was introduced by Almoari and Darus in [8].

Definition 6

([8]). Let $\Delta =:[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ in ${[0,\infty )}^2$ with ${\sigma }_1<{\sigma }_2$ and ${\nu }_1<{\nu }_2$. The mapping $\phi :\Delta \to \mathbb{R}$ is s-convex in the in the second sense on Δ if

$$\phi (\alpha \theta +\beta z,\alpha \lambda +\beta w)\le {\alpha }^s\phi (\theta ,\lambda )+{\beta }^s\phi (z,w),$$

holds for all $(\theta ,\lambda ),(z,w)\in \Delta$ with $\alpha ,\beta \ge 0$ with ${\alpha }^s+{\beta }^s=1$ and for some fixed $s\in (0,1]$. We write φ∈${\mathsf{\Xi }}_s^2$ when φ is s-convex in the second sense.

Definition 7

([8]). A function $\phi :\Delta =:[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]\subseteq {[0,\infty )}^2\to \mathbb{R}$ is called s-convex in the second sense on the coordinates on Δ if the partial mappings ${\phi }_{\lambda }:[{\sigma }_1,{\sigma }_2]\to \mathbb{R},{\phi }_{\lambda }\left(u\right)=\phi (u,\lambda )$ and ${\phi }_{\theta }:[{\nu }_1,{\nu }_2]\to \mathbb{R},{\phi }_{\theta }\left(v\right)=\phi (\theta ,v)$, are s-convex in the in the second sense for all $\lambda \in [{\nu }_1,{\nu }_2]$, $\theta \in [{\sigma }_1,{\sigma }_2]$ and $s\in (0,1]$, i.e, the partial mappings ${\phi }_{\lambda }$ and ${\phi }_{\theta }$ are s-convex in the second sense with the same fixed $s\in (0,1]$.

Lemma 1

([9]). Every s-convex mapping $\phi :\Delta =[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]\subseteq {[0,\infty )}^2\to [0,\infty )$ in the second sense is s-convex on the coordinates on Δ in the second sense, but the converse is not true in general.

Remark 2.

The s-convexity on the coordinates on $[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ in the second sense does not imply the s-convexity in the second sense, that is there exist functions which are s-convex on the coordinates in the second sense but are not s-convex in the second sense on $[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ (see [9]).

We refer the interested reader to [8] (see also [10]) for further modifications on s-convex functions and s-convex functions on the coordinates on $[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ from ${[0,\infty )}^2$ and related Hermite–Hadamard-type inequalities.

In [6], Dragomir established the following similar inequality of Hermite–Hadamard-type for convex functions on the coordinates on a rectangle $[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]$ from the plane ${\mathbb{R}}^2$.

Theorem 2

([6]). Suppose $\phi :\Delta =[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]\subseteq {\mathbb{R}}^2\to \mathbb{R}$ is a convex function on the coordinates on Δ. Then, one has the inequalities:

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \le \frac{1}{2}\left[\frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,\frac{{\nu }_1+{\nu }_2}{2}\right)d\theta +\frac{1}{{\nu }_2-{\nu }_1}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\lambda \right)d\lambda \right]\\ \le \frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\theta ,\lambda \right)d\lambda d\theta \\ \le \frac{1}{4}\left[\frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,{\nu }_1\right)d\theta +\frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,{\nu }_2\right)d\theta \right.\\ \left.+\frac{1}{{\nu }_2-{\nu }_1}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left({\sigma }_1,\lambda \right)d\lambda +\frac{1}{{\nu }_2-{\nu }_1}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left({\sigma }_2,\lambda \right)d\lambda \right]\\ \hfill \le \frac{\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)}{4}.\end{array}$$

(4)

In [8,9], Alomari and Darus proved the following variant of Hadamard-type inequalities for s-convex functions on the coordinates in the second sense on a rectangle from the plane ${\mathbb{R}}^2$.

Theorem 3

([8,9]). Suppose $\phi :\Delta =[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]\subseteq {[0,\infty )}^2\to \mathbb{R}$ is an s-convex function in the second sense on the coordinates on Δ. Then, one has the inequalities:

$$\begin{array}{c}{4}^{s-1}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \le 2^{s-2}\left[\frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,\frac{{\nu }_1+{\nu }_2}{2}\right)d\theta +\frac{1}{{\nu }_2-{\nu }_1}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\lambda \right)d\lambda \right]\\ \le \frac{1}{({\sigma }_2-{\sigma }_1)({\nu }_2-{\nu }_1)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi (\theta ,\lambda )d\lambda d\theta \\ \le \frac{1}{2\left(s+1\right)}\left[\frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,{\nu }_1\right)d\theta +\frac{1}{{\sigma }_2-{\sigma }_1}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,{\nu }_2\right)d\theta \right.\\ \left.+\frac{1}{{\nu }_2-{\nu }_1}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left({\sigma }_1,\lambda \right)d\lambda +\frac{1}{{\nu }_2-{\nu }_1}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left({\sigma }_2,\lambda \right)d\lambda \right]\\ \hfill \le \frac{\phi ({\sigma }_1,{\nu }_1)+\phi ({\sigma }_2,{\nu }_1)+\phi ({\sigma }_1,{\nu }_2)+\phi ({\sigma }_2,{\nu }_2)}{{(s+1)}^2}.\end{array}$$

(5)

The above inequalities are sharp.

Yang [11] considered a larger class known as coordinated (${\mu }_1,h_1$)-(${\mu }_2,h_2$)-convex functions that contains the coordinated convex functions and coordinated $\left(s_1,s_2\right)$-convex functions coordinated ($h_1,h_2$)-convex functions, coordinated (${\mu }_1,{\mu }_2$)-convex functions and obtained some Hermite–Hadamard-type inequalities of this class of functions.

Definition 8

([11]). Let Let $h:J\to \mathbb{R}$ be a nonnegative and non-zero function. A mapping $\phi :\Delta :=\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\subseteq {\left[0,\infty \right)}^2\to \left[0,\infty \right)$ is said to be ($\mu ,h$)-convex on the coordinates on Δ if the inequality

$$\begin{array}{c}\hfill \phi \left({\left[\alpha {\theta }^p+\left(1-\alpha \right){\lambda }^p\right]}^{\frac{1}{p}},{\left[\alpha z^p+\left(1-\alpha \right)w^p\right]}^{\frac{1}{p}}\right)\le h\left(\alpha \right)\phi \left(\theta ,z\right)+h\left(1-\alpha \right)\phi \left(\lambda ,w\right)\end{array}$$

holds for all $\alpha \in \left[0,1\right]$ and $\left(\theta ,z\right),\left(\lambda ,w\right)\in \Delta$.

Definition 9

([11]). Let $h_1$, $h_2:J\to \mathbb{R}$ be two non-negative and non-zero functions. A mapping $\phi :\Delta :=\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\subseteq {\left[0,\infty \right)}^2\to \left[0,\infty \right)$ is said to be ($p_1,h_1$)-($p_2,h_2$)-convex function on the coordinates on Δ, if the partial mappings ${\phi }_{\lambda }:\left[{\sigma }_1,{\sigma }_2\right]\to \left[0,\infty \right)$, ${\phi }_{\lambda }\left(u\right)=\phi \left(u,\lambda \right)$ and ${\phi }_{\theta }:\left[{\nu }_1,{\nu }_2\right]\to \left[0,\infty \right)$, ${\phi }_{\theta }\left(v\right)=\phi \left(\theta ,v\right)$ are ($p_1,h_1$)-convex with respect to u on $\left[{\sigma }_1,{\sigma }_2\right]$ and ($p_2,h_2$)-convex with respect to v on $\left[{\nu }_1,{\nu }_2\right]$ respectively for all $\lambda \in \left[{\nu }_1,{\nu }_2\right]$ and $\theta \in \left[{\sigma }_1,{\sigma }_2\right]$.

Remark 3.

From the above definition, we can say that if φ is a coordinated ($p_1,h_1$)-($p_2,h_2$)-convex function, then the following inequality holds:

$$\begin{array}{c}\phi \left({\left[\tau {\theta }^{p_1}+\left(1-\tau \right){\lambda }^{p_1}\right]}^{\frac{1}{p_1}},{\left[s{z}^{p_2}+\left(1-s\right)w^{p_2}\right]}^{\frac{1}{p_2}}\right)\hfill \\ \le h_1\left(\tau \right)h_2\left(s\right)\phi \left(\theta ,z\right)+h_1\left(\tau \right)h_2\left(1-s\right)\phi \left(\theta ,w\right)\\ \hfill +h_1\left(1-\tau \right)h_2\left(s\right)\phi \left(\lambda ,z\right)+h_1\left(1-\tau \right)h_2\left(1-s\right)\phi \left(\lambda ,w\right)\end{array}$$

for all $\tau ,s\in [0,1]$ and $(\theta ,\lambda ),(u,w)\in \Delta$.

An important result from [11] is presented below:

Theorem 4

([11]). Suppose $\phi :\Delta =[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]\subseteq {[0,\infty )}^2\to [0,\infty )$ is ($p_1,h_1$)-($p_2,h_2$)-convex function on the coordinates on Δ. Then, one has the inequalities:

$$\begin{array}{c}\frac{1}{4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\phi \left({\left[\frac{{\sigma }_1^{p_1}+{\sigma }_2^{p_1}}{2}\right]}^{\frac{1}{p_1}},{\left[\frac{{\nu }_1^{p_2}+{\nu }_2^{p_2}}{2}\right]}^{\frac{1}{p_2}}\right)\hfill \\ \le \frac{p_1}{4h_2\left(\frac{1}{2}\right)\left({\sigma }_2^{p_1}-{\sigma }_1^{p_1}\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\theta }^{p_1-1}\phi \left(\theta ,{\left[\frac{{\nu }_1^{p_2}+{\nu }_2^{p_2}}{2}\right]}^{\frac{1}{p_2}}\right)d\theta \\ +\frac{p_2}{4h_1\left(\frac{1}{2}\right)\left({\nu }_2^{p_2}-{\nu }_1^{p_2}\right)}{\int }_{{\nu }_1}^{{\nu }_2}{\lambda }^{p_2-1}\phi \left({\left[\frac{{\sigma }_1^{p_1}+{\sigma }_2^{p_1}}{2}\right]}^{\frac{1}{p_1}},\lambda \right)d\lambda \\ \le \frac{p_1{p}_2}{\left({\sigma }_2^{p_1}-{\sigma }_1^{p_1}\right)\left({\nu }_2^{p_2}-{\nu }_1^{p_2}\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\theta }^{p_1-1}{\lambda }^{p_2-1}\phi \left(\theta ,\lambda \right)d\lambda d\theta \\ \le \frac{p_1}{2\left({\sigma }_2^{p_1}-{\sigma }_1^{p_1}\right)}\left[{\int }_{{\sigma }_1}^{{\sigma }_2}{\theta }^{p_1-1}\phi \left(\theta ,{\nu }_1\right)d\theta +{\int }_{{\sigma }_1}^{{\sigma }_2}{\theta }^{p_1-1}\phi \left(\theta ,{\nu }_2\right)d\theta \right]{\int }_0^1{h}_2\left(\tau \right)d\tau \\ +\frac{p_2}{2\left({\nu }_2^{p_2}-{\nu }_1^{p_2}\right)}\left[{\int }_{{\nu }_1}^{{\nu }_2}{\lambda }^{p_2-1}\phi \left({\sigma }_1,\lambda \right)d\lambda +{\int }_{{\nu }_1}^{{\nu }_2}{\lambda }^{p_2-1}\phi \left({\sigma }_2,\lambda \right)d\lambda \right]{\int }_0^1{h}_1\left(\tau \right)d\tau \\ \hfill \le \left[\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau .\end{array}$$

(6)

Letting $p_1=p_2=1$ in Theorem 4, we obtain the result for the coordinated $\left(h_1,h_2\right)$-convex function on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\subseteq {\left[0,\infty \right)}^2$.

Theorem 5.

Suppose $\phi :\Delta =[{\sigma }_1,{\sigma }_2]\times [{\nu }_1,{\nu }_2]\subseteq {[0,\infty )}^2\to [0,\infty )$ is the ($h_1,h_2$)-convex function on the coordinates on Δ. Then, one has the inequalities:

$$\begin{array}{c}\frac{1}{4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \le \frac{1}{4h_2\left(\frac{1}{2}\right)\left({\sigma }_2-{\sigma }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,\frac{{\nu }_1+{\nu }_2}{2}\right)d\theta +\frac{1}{4h_1\left(\frac{1}{2}\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\lambda \right)d\lambda \\ \le \frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\theta ,\lambda \right)d\lambda d\theta \\ \le \frac{1}{2\left({\sigma }_2-{\sigma }_1\right)}\left[{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,{\nu }_1\right)d\theta +{\int }_{{\sigma }_1}^{{\sigma }_2}\phi \left(\theta ,{\nu }_2\right)d\theta \right]{\int }_0^1{h}_2\left(\tau \right)d\tau \\ +\frac{1}{2\left({\nu }_2-{\nu }_1\right)}\left[{\int }_{{\nu }_1}^{{\nu }_2}\phi \left({\sigma }_1,\lambda \right)d\lambda +{\int }_{{\nu }_1}^{{\nu }_2}\phi \left({\sigma }_2,\lambda \right)d\lambda \right]{\int }_0^1{h}_1\left(\tau \right)d\tau \\ \hfill \le \left[\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau .\end{array}$$

(7)

The readers are also invited to see [6,7,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25] the research conducted on coordinated convex and different types of coordinated convex functions on rectangle from the plane.

Motivated by the research of Bombardelli and Varošanec [5], the aim of this paper is to extend this research for functions of two variables that are coordinated ($h_1,h_2$)-convex functions on a rectangle from the plane ${\mathbb{R}}^2$. We will prove that there is no change in the properties associated with the integral mean of the function $\phi$ of two variables when the class of coordinated convex functions is extended to the class of coordinated $(h_1,h_2)$-convex functions. In this research, we also prove Hermite–Hadamard–Fejér-type inequalities for coordinated ($h_1,h_2$)-convex functions on a rectangle from the plane ${\mathbb{R}}^2$ and discuss particular cases for other classes of coordinated convex functions such as coordinated ($s_1,s_2$)-convex functions and on coordinated convex functions a rectangle from the plane ${\mathbb{R}}^2$. We observe in our findings that the left-hand side inequality of our result is stronger than the right-hand side the inequality in that result. In this research, we also prove several characteristics of the mappings on the rectangle $\left[0,1\right]\times \left[0,1\right]$ defined by

$$\begin{array}{cc}\hfill H\left(\tau ,s\right)& =\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}\hfill \\ \hfill & \times {\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2},s\lambda +\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}\right)d\lambda d\theta \hfill \end{array}$$

and

$$\begin{array}{cc}\hfill F\left(\tau ,s\right)& =\frac{1}{{\left({\sigma }_2-{\sigma }_1\right)}^2{\left({\nu }_2-{\nu }_1\right)}^2}\hfill \\ \hfill & \times {\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,sz+\left(1-s\right)w\right)d\lambda d\theta dzdw.\hfill \end{array}$$

that emerge when the function $\phi$ is a coordinated ($h_1,h_2$)-convex function on a rectangle from the plane ${\mathbb{R}}^2$.

2. Main Results

We begin with the result on the second Hermite–Hadamard–Fejér inequality for a coordinated $\left(h_1,h_2\right)$-convex function on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\subseteq {\left[0,\infty \right)}^2$:

Theorem 6.

Let $\phi :\Delta =\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\to \mathbb{R}$ be coordinated $\left(h_1,h_2\right)$-convex function and $w:\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\to \mathbb{R}$ be no-negative integrable and symmetric with respect to $\frac{{\sigma }_1+{\sigma }_2}{2}$ and $\frac{{\nu }_1+{\nu }_2}{2}$. Then,

$$\begin{array}{c}\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(u,v\right)w\left(u,v\right)dudv\hfill \\ \le \left[\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)\right]\\ \hfill \times {\int }_0^1{\int }_0^1{h}_1\left(u\right)h_2\left(v\right)\mu \left(u{\sigma }_1+\left(1-u\right){\sigma }_2,v{\nu }_1+\left(1-v\right){\nu }_2\right)dudv.\end{array}$$

(8)

If φ is coordinated $\left(h_1,h_2\right)$-concave, the the inequality in (8) is reversed.

Proof. 

For any $\left(\theta ,\lambda \right)\in \left({\sigma }_1,{\sigma }_2\right)\times \left({\nu }_1,{\nu }_2\right)$, there exist $\alpha$, $\beta \in \left(0,1\right)$ such that $\theta =\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2$ and $\lambda =\alpha {\nu }_1+\overline{\alpha }{\nu }_2$, $\overline{\alpha }=1-\alpha$, $\overline{\beta }=1-\beta$.

From the definition of coordinated $\left(h_1,h_2\right)$-convex function, we have

$$\begin{array}{c}\phi \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)\hfill \\ \le \left[h_1\left(\alpha \right)h_2\left(\beta \right)\phi \left({\sigma }_1,{\nu }_1\right)+h_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_1,{\nu }_2\right)+h_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\phi \left({\sigma }_2,{\nu }_1\right)\right.\\ \hfill \left.+h_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_2,{\nu }_2\right)\right]\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right),\end{array}$$

(9)

$$\begin{array}{c}\phi \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)\hfill \\ \le \left[h_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_1,{\nu }_1\right)+h_1\left(\alpha \right)h_2\left(\beta \right)\phi \left({\sigma }_1,{\nu }_2\right)+h_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_2,{\nu }_1\right)\right.\\ \hfill \left.+h_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\phi \left({\sigma }_2,{\nu }_2\right)\right]\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right),\end{array}$$

(10)

$$\begin{array}{c}\phi \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)\hfill \\ \le \left[h_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\phi \left({\sigma }_1,{\nu }_1\right)+h_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_1,{\nu }_2\right)+h_1\left(\alpha \right)h_2\left(\beta \right)\phi \left({\sigma }_2,{\nu }_1\right)\right.\\ \hfill \left.+h_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_2,{\nu }_2\right)\right]\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)\end{array}$$

(11)

and

$$\begin{array}{c}\phi \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)\hfill \\ \le \left[h_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_1,{\nu }_1\right)+h_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\phi \left({\sigma }_1,{\nu }_2\right)+h_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\phi \left({\sigma }_2,{\nu }_1\right)\right.\\ \hfill \left.+h_1\left(\alpha \right)h_2\left(\beta \right)\phi \left({\sigma }_2,{\nu }_2\right)\right]\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right).\end{array}$$

(12)

Adding (9)–(12), integrating over ${\left[0,1\right]}^2$ and using the symmetricity of the weight w, we obtain

$$\begin{array}{c}{\int }_0^1{\int }_0^1\phi \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \hfill \\ +{\int }_0^1{\int }_0^1\phi \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \\ \hfill +{\int }_0^1{\int }_0^1\phi \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \end{array}$$

$$\begin{array}{c}+{\int }_0^1{\int }_0^1\phi \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \hfill \\ \le \phi \left({\sigma }_1,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\beta \right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \\ +\phi \left({\sigma }_1,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \\ +\phi \left({\nu }_1,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \\ \hfill +\phi \left({\sigma }_1,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \end{array}$$

$$\begin{array}{c}+\phi \left({\sigma }_1,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \hfill \\ +\phi \left({\sigma }_1,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\beta \right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \\ +\phi \left({\sigma }_1,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \\ \hfill +\phi \left({\sigma }_1,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \end{array}$$

$$\begin{array}{c}+\phi \left({\sigma }_2,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \hfill \\ +\phi \left({\sigma }_2,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \\ +\phi \left({\sigma }_2,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\beta \right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \\ \hfill +\phi \left({\sigma }_2,{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \end{array}$$

$$\begin{array}{c}+\phi \left({\sigma }_2,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \hfill \\ +\phi \left({\sigma }_2,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\mu \left(\alpha {\sigma }_1+\overline{\alpha }{\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \\ +\phi \left({\sigma }_2,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\alpha {\nu }_1+\overline{\alpha }{\nu }_2\right)d\beta d\alpha \\ +\phi \left({\sigma }_2,{\nu }_2\right){\int }_0^1{\int }_0^1{h}_1\left(\alpha \right)h_2\left(\beta \right)\mu \left(\overline{\alpha }{\sigma }_1+\alpha {\sigma }_2,\overline{\beta }{\nu }_1+\beta {\nu }_2\right)d\beta d\alpha \\ =4\left[\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)\right]\\ \hfill \times {\int }_0^1{\int }_0^1{h}_1\left(u\right)h_2\left(v\right)\mu \left(u{\sigma }_1+\left(1-u\right){\sigma }_2,vc+\left(1-v\right){\nu }_2\right)dudv.\end{array}$$

After making suitable substitution, we observe that all the integrals in the first line of the above inequality are equal to $\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(u,v\right)\mu \left(u,v\right)dudv$.

This completes the proof of the theorem. □

Remark 4.

If $\mu \left(\tau ,r\right)=1$, for all $\left(\tau ,r\right)\in \left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ and $h_1\left(\tau \right)=\tau$, $h_2\left(s\right)=s$ in Theorem 6, i.e., if φ is a coordinated convex function, we have the right-hand side of the Hermite–Hadamard-type inequality (4). If $\mu \left(\tau ,r\right)=1$, for all $\left(\tau ,r\right)\in \left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ and $h_1\left(\tau \right)={\tau }^{s_1}$, $h_2\left(s\right)=r^{s_2}$, $s_1$, $s_2\in \left(0,1\right)$ in Theorem 6, i.e., if φ is a coordinated s-convex function in the second sense, then we have the following Hermite–Hadamard-type inequality:

$$\begin{array}{c}\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau ,r\right)drd\tau \hfill \\ \hfill \le \frac{\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)}{\left(s_1+1\right)\left(s_2+1\right)}.\end{array}$$

(13)

Definition 10

([3]). A function $h:J\to \mathbb{R}$ is said to be a supermultiplicative function if

$$h\left(xy\right)\ge h\left(x\right)h\left(y\right)$$

(14)

for all $x,y\in J$. If inequality (14) is reversed, then h is said to be a submultiplicative function. If the equality holds in (14), then h is said to be a multiplicative function.

Theorem 7.

Let $h_1$ be defined on $\left[0,max\left\{1,{\sigma }_2-{\sigma }_1\right\}\right]$ and $h_2$ on $\left[0,max\left\{1,{\nu }_2-{\nu }_1\right\}\right]$, respectively. Suppose that $\phi :\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\to \mathbb{R}$ is an $\left(h_1,h_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$. If $\mu :\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\to \mathbb{R}$ be non-negative, integrable and symmetric with respect to $\theta =\frac{{\sigma }_1+{\sigma }_2}{2}$, $\lambda =\frac{{\nu }_1+{\nu }_2}{2}$ and ${\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\mu \left(\tau ,r\right)d\tau dr>0$, then

$$\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\le \mathcal{C}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau ,r\right)\mu \left(\tau ,r\right)drd\tau ,$$

(15)

where

$$\mathcal{C}=\frac{4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}{{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\mu \left(\tau ,r\right)drd\tau }.$$

Furthermore, if

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right){\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{h}_1\left(\lambda -\theta \right)h_2\left(w-z\right)\hfill \\ \hfill \times \mu \left(\theta ,z\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,z\right)\mu \left(\lambda ,w\right)dzdwd\theta d\lambda \ne 0,\end{array}$$

$h_1\left(\theta \right),h_2\left(\lambda \right)\ne 0$ for $\theta \ne 0,\lambda \ne 0$ and if

 (i)

$h_1$, $h_2$ are multiplicative or

 (ii)

$h_1$, $h_2$ are supermultiplicative, and φ is non-negative. If φ is an $\left(h_1,h_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, then the inequality (15) holds for

$$\begin{array}{c}\mathcal{C}=min\left\{\frac{4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}{{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\mu \left(\tau ,r\right)d\tau dr},\right.\hfill \\ \hfill \left.\frac{{\int }_0^{\frac{{\sigma }_2-{\sigma }_1}{2}}{\int }_0^{\frac{{\nu }_2-{\nu }_1}{2}}{h}_1\left(\theta \right)h_2\left(\lambda \right)\mu \left(\theta +\frac{{\sigma }_1+{\sigma }_2}{2},\lambda +\frac{{\nu }_1+{\nu }_2}{2}\right)d\lambda d\theta }{\begin{array}{c}{\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{h}_1\left(\lambda -\theta \right)h_2\left(w-z\right)\\ \times \mu \left(\theta ,z\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,z\right)\mu \left(\lambda ,w\right)dzdwd\theta d\lambda \end{array}}\right\}.\end{array}$$

(16)

Proof. 

Let $\phi$ be an h-convex function. If $\alpha =\frac{1}{2}$, $\theta =\tau {\sigma }_1+\left(1-\tau \right){\sigma }_2$, $\lambda =\left(1-\tau \right){\sigma }_1+\tau {\sigma }_2$, $\beta =\frac{1}{2}$, $w=rc+\left(1-r\right){\nu }_2$ and $z=\left(1-r\right){\nu }_1+r{\nu }_2$, from the definition of coordinated $\left(h_1,h_2\right)$-convex function, we have the following inequality:

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ =\phi \left(\frac{\tau {\sigma }_1+\left(1-\tau \right){\sigma }_2+\left(1-\tau \right){\sigma }_1+\tau {\sigma }_2}{2},\frac{rc+\left(1-r\right){\nu }_2+\left(1-r\right){\nu }_1+r{\nu }_2}{2}\right)\\ \le h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)\left[\phi \left(\tau {\sigma }_1+\left(1-\tau \right){\sigma }_2,rc+\left(1-r\right){\nu }_2\right)\right.\\ +\phi \left(\tau {\sigma }_1+\left(1-\tau \right){\sigma }_2,\left(1-r\right){\nu }_1+r{\nu }_2\right)+\phi \left(\left(1-\tau \right){\sigma }_1+\tau {\sigma }_2,rc+\left(1-r\right){\nu }_2\right)\\ \hfill \left.+\phi \left(\left(1-\tau \right){\sigma }_1+\tau {\sigma }_2,\left(1-r\right){\nu }_1+r{\nu }_2\right)\right].\end{array}$$

(17)

Now, multiplying both sides of (17) with

$$\begin{array}{c}\mu \left(\tau {\sigma }_1+\left(1-\tau \right){\sigma }_2,rc+\left(1-r\right){\nu }_2\right)=\mu \left(\tau {\sigma }_1+\left(1-\tau \right){\sigma }_2,\left(1-r\right){\nu }_1+r{\nu }_2\right)\hfill \\ \hfill =\mu \left(\left(1-\tau \right){\sigma }_1+\tau {\sigma }_2,rc+\left(1-r\right){\nu }_2\right)=\mu \left(\left(1-\tau \right){\sigma }_1+\tau {\sigma }_2,\left(1-r\right){\nu }_1+r{\nu }_2\right),\end{array}$$

and integrating over u on $\left[0,1\right]$, over v on $\left[0,1\right]$, we obtain (15), which holds in the general case.

Let $h_1$ and $h_2$ be supermultiplicative $h_1\left(\theta \right)\ne 0$, for $\theta \ne 0$ and hence $h_1\left(\theta \right)>0$, for $\theta >0$. Similarly, $h_2\left(z\right)>0$, for $z>0$. For any $\theta$, $\lambda \in \left[{\sigma }_1,{\sigma }_2\right]$ and z, $w\in \left[{\nu }_1,{\nu }_2\right]$ such that ${\sigma }_1\le \theta <\frac{{\sigma }_1+{\sigma }_2}{2}<\lambda \le {\sigma }_2$ and ${\nu }_1\le z<\frac{{\nu }_1+{\nu }_2}{2}<w\le {\nu }_2$, we have

$$\frac{{\sigma }_1+{\sigma }_2}{2}=\left(\frac{\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}}{\lambda -\theta }\right)\theta +\left(\frac{\frac{{\sigma }_1+{\sigma }_2}{2}-\theta }{\lambda -\theta }\right)\lambda$$

and

$$\frac{{\nu }_1+{\nu }_2}{2}=\left(\frac{w-\frac{{\nu }_1+{\nu }_2}{2}}{w-z}\right)z+\left(\frac{\frac{{\nu }_1+{\nu }_2}{2}-z}{w-z}\right)w.$$

Denote $\alpha =\frac{\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}}{\lambda -\theta }$, $\beta =\frac{w-\frac{{\nu }_1+{\nu }_2}{2}}{w-z}$, then $\overline{\alpha }:=1-\alpha =\frac{\frac{{\sigma }_1+{\sigma }_2}{2}-\theta }{\lambda -\theta }$, $\overline{\beta }:=1-\beta =\frac{\frac{{\nu }_1+{\nu }_2}{2}-z}{w-z}$, we therefore have the following inequality:

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)=\phi \left(\alpha \theta +\overline{\alpha }\lambda ,\beta z+\overline{\beta }w\right)\hfill \\ \le h_1\left(\alpha \right)h_2\left(\beta \right)\phi \left(\theta ,z\right)+h_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\phi \left(\theta ,w\right)\\ \hfill +h_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\phi \left(\lambda ,z\right)+h_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\phi \left(\lambda ,w\right).\end{array}$$

Since $h_1$ and $h_2$ are supermultiplicative, we have

$$\begin{array}{cc}\hfill h_1\left(\alpha \right)& =h_1\left(\frac{\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}}{\lambda -\theta }\right)\le \frac{h_1\left(\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}\right)}{h_1\left(\lambda -\theta \right)},\hfill \\ \hfill h_1\left(\overline{\alpha }\right)& \le \frac{h_1\left(\frac{{\sigma }_1+{\sigma }_2}{2}-\theta \right)}{h_1\left(\lambda -\theta \right)},h_2\left(\beta \right)\le \frac{h_2\left(w-\frac{{\nu }_1+{\nu }_2}{2}\right)}{h_2\left(w-z\right)}\mathrm{and}{h}_2\left(\overline{\beta }\right)\le \frac{h_2\left(\frac{{\nu }_1+{\nu }_2}{2}-z\right)}{h_2\left(w-z\right)}.\hfill \end{array}$$

Hence, if $\phi >0$, we have

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)=\phi \left(\alpha \theta +\overline{\alpha }\lambda ,\beta z+\overline{\beta }w\right)\hfill \\ \le h_1\left(\alpha \right)h_2\left(\beta \right)\phi \left(\theta ,z\right)+h_1\left(\alpha \right)h_2\left(\overline{\beta }\right)\phi \left(\theta ,w\right)\\ \hfill +h_1\left(\overline{\alpha }\right)h_2\left(\beta \right)\phi \left(\lambda ,z\right)+h_1\left(\overline{\alpha }\right)h_2\left(\overline{\beta }\right)\phi \left(\lambda ,w\right).\end{array}$$

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \le \frac{h_1\left(\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}\right)h_2\left(w-\frac{{\nu }_1+{\nu }_2}{2}\right)}{h_1\left(\lambda -\theta \right)h_2\left(w-z\right)}\phi \left(\theta ,z\right)+\frac{h_1\left(\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}\right)h_2\left(\frac{{\nu }_1+{\nu }_2}{2}-z\right)}{h_1\left(\lambda -\theta \right)h_2\left(w-z\right)}\phi \left(\theta ,w\right)\\ \hfill +\frac{h_1\left(\frac{{\sigma }_1+{\sigma }_2}{2}-\theta \right)h_2\left(w-\frac{{\nu }_1+{\nu }_2}{2}\right)}{h_1\left(\lambda -\theta \right)h_2\left(w-z\right)}\phi \left(\lambda ,z\right)+\frac{h_1\left(\frac{{\sigma }_1+{\sigma }_2}{2}-\theta \right)h_2\left(\frac{{\nu }_1+{\nu }_2}{2}-z\right)}{h_1\left(\lambda -\theta \right)h_2\left(w-z\right)}\phi \left(\lambda ,w\right)\end{array}$$

that is

$$\begin{array}{c}{h}_1\left(\lambda -\theta \right)h_2\left(w-z\right)\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \le h_1\left(\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}\right)h_2\left(w-\frac{{\nu }_1+{\nu }_2}{2}\right)\phi \left(\theta ,z\right)\\ +h_1\left(\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}\right)h_2\left(\frac{{\nu }_1+{\nu }_2}{2}-z\right)\phi \left(\theta ,w\right)\\ +h_1\left(\frac{{\sigma }_1+{\sigma }_2}{2}-\theta \right)h_2\left(w-\frac{{\nu }_1+{\nu }_2}{2}\right)\phi \left(\lambda ,z\right)\\ \hfill +h_1\left(\frac{{\sigma }_1+{\sigma }_2}{2}-\theta \right)h_2\left(\frac{{\nu }_1+{\nu }_2}{2}-z\right)\phi \left(\lambda ,w\right).\end{array}$$

(18)

The inequality (18) holds if $h_1$ and $h_2$ are multiplicative regardless of the positivity of $\phi$.

From (18), we have that the following inequality holds:

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right){\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{h}_1\left(\lambda -\theta \right)h_2\left(w-z\right)\hfill \\ \times \mu \left(\theta ,z\right)\mu \left(\lambda ,w\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,z\right)dzdwd\theta d\lambda \\ \le {\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{h}_1\left(\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}\right)h_2\left(w-\frac{{\nu }_1+{\nu }_2}{2}\right)\\ \times \mu \left(\lambda ,w\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,z\right)dwd\lambda {\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}\phi \left(\theta ,z\right)\mu \left(\theta ,z\right)dzd\theta \\ +{\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{h}_1\left(\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}\right)h_2\left(\frac{{\nu }_1+{\nu }_2}{2}-z\right)\\ \times \mu \left(\theta ,z\right)\mu \left(\lambda ,w\right)\mu \left(\lambda ,z\right)dzd\lambda {\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}\phi \left(\theta ,w\right)\mu \left(\theta ,w\right)dwd\theta \\ +{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{h}_1\left(\frac{{\sigma }_1+{\sigma }_2}{2}-\theta \right)h_2\left(w-\frac{{\nu }_1+{\nu }_2}{2}\right)\\ \hfill \times \mu \left(\theta ,z\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,w\right)dwd\theta {\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}\phi \left(\lambda ,z\right)\mu (\lambda ,z)dzd\lambda \end{array}$$

$$\begin{array}{c}+{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{h}_1\left(\frac{{\sigma }_1+{\sigma }_2}{2}-\theta \right)h_2\left(\frac{{\nu }_1+{\nu }_2}{2}-z\right)\hfill \\ \hfill \times \mu \left(\theta ,z\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,z\right)dzd\theta {\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}\phi \left(\lambda ,w\right)\mu (\lambda ,w)dwd\lambda .\end{array}$$

(19)

After the substitutions $\lambda -\frac{{\sigma }_1+{\sigma }_2}{2}=\tau$, $\frac{{\sigma }_1+{\sigma }_2}{2}-\theta =\tau$ in the first integral on the right-hand side and the substitution $w-\frac{{\nu }_1+{\nu }_2}{2}=r$, $\frac{{\nu }_1+{\nu }_2}{2}-z=r$ in the integral in the second term of the sum, we obtain

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right){\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{h}_1\left(\lambda -\theta \right)h_2\left(w-z\right)\hfill \\ \times \mu \left(\theta ,z\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,z\right)\mu \left(\lambda ,w\right)dzdwd\theta d\lambda \\ \le {\int }_0^{\frac{{\sigma }_2-{\sigma }_1}{2}}{\int }_0^{\frac{{\nu }_2-{\nu }_1}{2}}{h}_1\left(\tau \right)h_2\left(r\right)\mu \left(\tau +\frac{{\sigma }_1+{\sigma }_2}{2},r+\frac{{\nu }_1+{\nu }_2}{2}\right)drd\tau \\ {\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}\phi \left(\theta ,z\right)\mu \left(\theta ,z\right)dzd\theta +{\int }_0^{\frac{{\sigma }_2-{\sigma }_1}{2}}{\int }_0^{\frac{{\nu }_2-{\nu }_1}{2}}{h}_1\left(\tau \right)h_2\left(r\right)\\ \times \mu \left(\tau +\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}-r\right)drd\tau {\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}\phi \left(\theta ,w\right)\mu \left(\theta ,w\right)dwd\theta \\ +{\int }_0^{\frac{{\sigma }_2-{\sigma }_1}{2}}{\int }_0^{\frac{{\nu }_2-{\nu }_1}{2}}{h}_1\left(\tau \right)h_2\left(r\right)\mu \left(\frac{{\sigma }_1+{\sigma }_2}{2}-\tau ,r+\frac{{\nu }_1+{\nu }_2}{2}\right)drd\tau \\ \times {\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}\phi \left(\lambda ,z\right)\mu (\theta ,z)dzd\lambda ={\int }_0^{\frac{{\sigma }_2-{\sigma }_1}{2}}{\int }_0^{\frac{{\sigma }_2-{\nu }_1}{2}}{h}_1\left(\tau \right)h_2\left(r\right)\\ \hfill \times \mu \left(\frac{{\sigma }_1+{\sigma }_2}{2}-\tau ,\frac{{\nu }_1+{\nu }_2}{2}-r\right)drd\tau {\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}\phi \left(\lambda ,w\right)\mu \left(\lambda ,w\right)d\lambda dw,\end{array}$$

where we have used the fact that the function $\mu$ is symmetric on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, i.e.,

$$\begin{array}{c}\mu \left(\frac{{\sigma }_1+{\sigma }_2}{2}-\tau ,\frac{{\nu }_1+{\nu }_2}{2}-r\right)=\mu \left(\tau +\frac{{\sigma }_1+{\sigma }_2}{2},r+\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \hfill =\mu \left(\frac{{\sigma }_1+{\sigma }_2}{2}-\tau ,r+\frac{{\nu }_1+{\nu }_2}{2}\right)=\mu \left(\tau +\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}-r\right)\end{array}$$

for all $\left(\tau ,r\right)\in \left[0,\frac{{\sigma }_2-{\sigma }_1}{2}\right]\times \left[0,\frac{{\nu }_2-{\nu }_1}{2}\right]$. □

Remark 5.

Suppose the assumptions of Theorem 7

 (a)

If φ is an $\left(h_1,h_2\right)$-concave function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, then the inequality (15) holds in reversed direction.

 (b)

If $h_1$ and $h_2$ are submultiplicative,

$$\begin{array}{c}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right){\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{h}_1\left(\lambda -\theta \right)h_2\left(w-z\right)\hfill \\ \hfill \times \mu \left(\theta ,z\right)\mu \left(\theta ,w\right)\mu \left(\lambda ,z\right)\mu \left(\lambda ,w\right)dzdwd\theta d\lambda \ne 0,\end{array}$$

$h_1$, $h_2>0$ and if φ is an $\left(h_1,h_2\right)$-concave function, then the inequality in (15) is reversed, and the constant $\mathcal{C}$ can be obtained from (16) by changing min to max.

Remark 6.

In Theorem 7

 (a)

If φ is convex on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, i.e., $h_1\left(\tau \right)=\tau$ and $h_2\left(r\right)=r$, then inequality (15) becomes the left-hand side of inequality (4).

 (b)

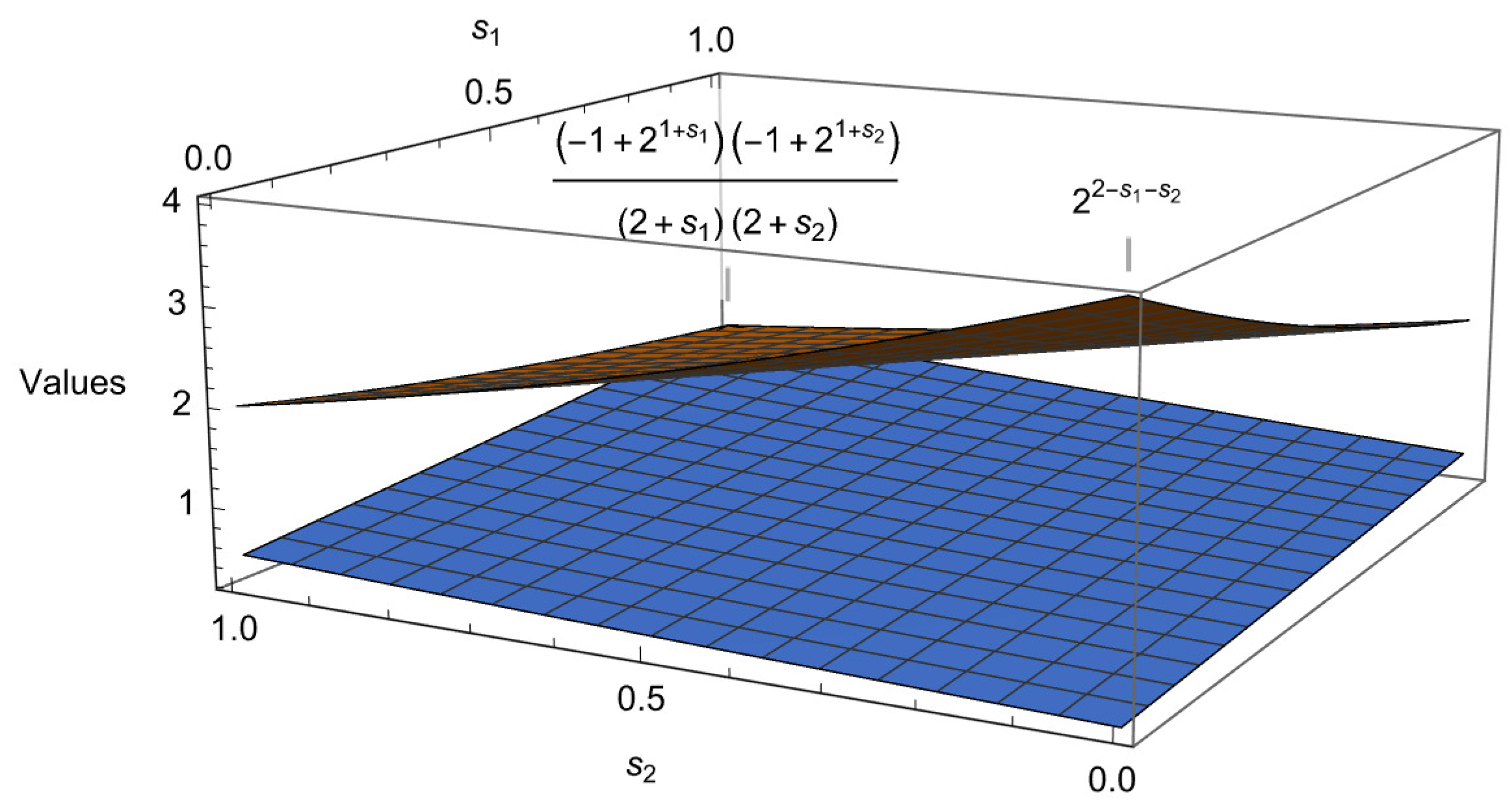
Let $\mu \left(\tau ,r\right)=1$ for all $\left(\tau ,r\right)\in \left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ and let φ be an $\left(s_1,s_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ in the second sense, i.e., φ be an $\left(h_1,h_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ with multiplicative $h_1\left(\tau \right)={\tau }^{s_1}$ and $h_2\left(r\right)=r^{s_2}$, $\left(s_1,s_2\right)\in \left(0,1\right)\times \left(0,1\right)$. Then, the constant $\mathcal{C}$ in Theorem 7 has a form

$$\begin{array}{c}\mathcal{C}=min\left\{\frac{2^{2-s_1-s_2}}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)},\frac{{\int }_0^{\frac{{\sigma }_2-{\sigma }_1}{2}}{\int }_0^{\frac{{\nu }_2-{\nu }_1}{2}}{\theta }^{s_1}{\lambda }^{s_2}d\lambda d\theta }{{\int }_{\frac{{\sigma }_1+{\sigma }_2}{2}}^{{\sigma }_2}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{\frac{{\nu }_1+{\nu }_2}{2}}^{{\nu }_2}{\int }_{{\nu }_1}^{\frac{{\nu }_1+{\nu }_2}{2}}{\left(\lambda -\theta \right)}^{s_1}{\left(w-z\right)}^{s_2}dzdwd\theta d\lambda }\right\}.\hfill \\ \hfill =min\left\{\frac{2^{2-s_1-s_2}}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)},\frac{\left(s_1+2\right)\left(s_2+2\right)}{\left(2^{s_1+1}-1\right)\left(2^{s_2+1}-1\right)\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}\right\}.\end{array}$$

(20)

From the graph below (Figure 1), we can observe that

$$\frac{\left(2^{s_1+1}-1\right)\left(2^{s_2+1}-1\right)}{\left(s_1+2\right)\left(s_2+2\right)}<2^{2-s_1-s_2}$$

for all $\left(s_1,s_2\right)\in \left(0,1\right)\times \left(0,1\right)$.

Thus, the inequality (15) for the s-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ in the second sense takes the form

$$\begin{array}{c}\frac{\left(2^{s_1+1}-1\right)\left(2^{s_2+1}-1\right)}{\left(s_1+2\right)\left(s_2+2\right)}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \hfill \le \frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau ,r\right)\mu \left(\tau ,r\right)drd\tau .\end{array}$$

(21)

The inequality (21) provides a refinement for the first inequality already proved in [9] for the $\left(s_1,s_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ in the second sense.

Let us now consider the non-weighted Hermite–Hadamard-type inequalities for the $\left(h_1,h_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ which can easily be obtained from Theorem 4 for ${\mu }_1={\mu }_2=1$:

$$\begin{array}{c}\frac{1}{4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\hfill \\ \le \frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\theta ,\lambda \right)d\lambda d\theta \le \left[\phi \left({\sigma }_1,{\nu }_1\right)\right.\\ \hfill \left.+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau ,\end{array}$$

(22)

where $h_1\left(\frac{1}{2}\right),h_2\left(\frac{1}{2}\right)>0$.

Let $F:\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\to \mathbb{R}$ and $G:\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\to \mathbb{R}$ be defined as

$$\begin{array}{c}F\left(\theta ,\lambda \right)=\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)\left[\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,\lambda \right)+\phi \left(\theta ,{\nu }_1\right)+\phi \left(\theta ,\lambda \right)\right]\hfill \\ \hfill \times {\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau -2{\int }_{{\sigma }_1}^{\theta }{\int }_{{\nu }_1}^{\lambda }\phi \left(\tau ,r\right)drd\tau \end{array}$$

and

$$\begin{array}{c}G\left(\theta ,\lambda \right)={\int }_{{\sigma }_1}^{\theta }{\int }_{{\nu }_1}^{\lambda }\phi \left(\tau ,r\right)drd\tau \hfill \\ -\frac{\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)}{8h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\left[\phi \left(\frac{{\sigma }_1+\theta }{2},{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\lambda \right)\right]\\ -\frac{\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)}{8h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\left[\phi \left(\theta ,\frac{{\nu }_1+\lambda }{2}\right)+\phi \left({\sigma }_1,\frac{{\nu }_1+\lambda }{2}\right)\right]\\ \hfill -\frac{\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)}{4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\phi \left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right).\end{array}$$

We now prove the following important result regarding the above mappings.

Theorem 8.

Suppose that the mapping $\phi :\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\to \mathbb{R}$ is an $\left(h_1,h_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, $\phi \left(\theta ,\lambda \right)\ge 0$ for all $\left(\theta ,\lambda \right)\in \left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, $h_1\left(\frac{1}{2}\right),h_2\left(\frac{1}{2}\right)>0$, $\frac{1}{16h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\ge {\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau$, then

$$F\left(\theta ,\lambda \right)\ge G\left(\theta ,\lambda \right)\ge 0$$

(23)

for all $\left(\theta ,\lambda \right)\in \left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$.

Proof. 

The second non-weighted Hermite–Hadamard inequality (22) on rectangles $\left[{\sigma }_1,\frac{{\sigma }_1+\theta }{2}\right]\times \left[{\nu }_1,\frac{{\nu }_1+\lambda }{2}\right]$, $\left[\frac{{\sigma }_1+\theta }{2},\theta \right]\times \left[\frac{{\nu }_1+\lambda }{2},\lambda \right]$, $\left[{\sigma }_1,\frac{{\sigma }_1+\theta }{2}\right]\times \left[\frac{{\nu }_1+\lambda }{2},\lambda \right]$ and $\left[\frac{{\sigma }_1+\theta }{2},\theta \right]\times \left[{\nu }_1,\frac{{\nu }_1+\lambda }{2}\right]$ gives us the following inequalities:

$$\begin{array}{c}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+\theta }{2}}{\int }_{{\nu }_1}^{\frac{{\nu }_1+\lambda }{2}}\phi \left(\tau ,r\right)drd\tau \le \left(\frac{\theta -{\sigma }_1}{2}\right)\left(\frac{\lambda -{\nu }_1}{2}\right)\hfill \\ \left[\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,\frac{{\nu }_1+\lambda }{2}\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},{\nu }_1\right)\right.\\ \hfill \left.+\phi \left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau ,\end{array}$$

(24)

$$\begin{array}{c}{\int }_{\frac{{\sigma }_1+\theta }{2}}^{\theta }{\int }_{\frac{{\nu }_1+\lambda }{2}}^{\lambda }\phi \left(\tau ,r\right)drd\tau \le \left(\frac{\theta -{\sigma }_1}{2}\right)\left(\frac{\lambda -{\nu }_1}{2}\right)\hfill \\ \times \left[\phi \left(\theta ,\lambda \right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\lambda \right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)\right.\\ \hfill \left.+\phi \left(\theta ,\frac{{\nu }_1+\lambda }{2}\right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau ,\end{array}$$

(25)

$$\begin{array}{c}{\int }_{{\sigma }_1}^{\frac{{\sigma }_1+\theta }{2}}{\int }_{\frac{{\nu }_1+\lambda }{2}}^{\lambda }\phi \left(\tau ,r\right)drd\tau \le \left(\frac{\theta -{\sigma }_1}{2}\right)\left(\frac{\lambda -{\nu }_1}{2}\right)\hfill \\ \left[\phi \left({\sigma }_1,\lambda \right)+\phi \left({\sigma }_1,\frac{{\nu }_1+\lambda }{2}\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)\right.\\ \hfill \left.+\phi \left(\frac{{\sigma }_1+\theta }{2},\lambda \right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau \end{array}$$

(26)

and

$$\begin{array}{c}{\int }_{\frac{{\sigma }_1+\theta }{2}}^{\theta }{\int }_{{\nu }_1}^{\frac{{\nu }_1+\lambda }{2}}\phi \left(\tau ,r\right)drd\tau \le \left(\frac{\theta -{\sigma }_1}{2}\right)\left(\frac{\lambda -{\nu }_1}{2}\right)\hfill \\ \times \left[\phi \left(\theta ,{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)\right.\\ \hfill \left.+\phi \left(\theta ,\frac{{\nu }_1+\lambda }{2}\right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau .\end{array}$$

(27)

Adding (24)–(27) and applying the coordinated $\left(h_1,h_2\right)$-convexity, we obtain

$$\begin{array}{c}{\int }_{{\sigma }_1}^{\theta }{\int }_{{\nu }_1}^{\lambda }\phi \left(\tau ,r\right)drd\tau \le \left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)\hfill \\ \times \left\{\frac{\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left(\theta ,\lambda \right)+\phi \left({\sigma }_1,\lambda \right)+\phi \left(\theta ,{\nu }_1\right)}{4}\right.\\ +\phi \left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)+\frac{1}{2}\left[\phi \left(\frac{{\sigma }_1+\theta }{2},{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\lambda \right)\right]\\ \hfill \left.+\frac{1}{2}\left[\phi \left(\theta ,\frac{{\nu }_1+\lambda }{2}\right)+\phi \left({\sigma }_1,\frac{{\nu }_1+\lambda }{2}\right)\right]\right\}{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau .\end{array}$$

(28)

Multiplying both sides of (28) by 4, we obtain

$$\begin{array}{c}2{\int }_{{\sigma }_1}^{\theta }{\int }_{{\nu }_1}^{\lambda }\phi \left(\tau ,r\right)drd\tau -\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)\hfill \\ \times \left[\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left(\theta ,\lambda \right)+\phi \left({\sigma }_1,\lambda \right)+\phi \left(\theta ,{\nu }_1\right)\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau \\ \le 4f\left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right){\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau \\ +\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)\left\{2\left[\phi \left(\frac{{\sigma }_1+\theta }{2},{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\lambda \right)\right]\right.\\ \left.+2\left[\phi \left(\theta ,\frac{{\nu }_1+\lambda }{2}\right)+\phi \left({\sigma }_1,\frac{{\nu }_1+\lambda }{2}\right)\right]\right\}\\ \hfill \times {\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau -2{\int }_{{\sigma }_1}^{\theta }{\int }_{{\nu }_1}^{\lambda }\phi \left(\tau ,r\right)drd\tau .\end{array}$$

(29)

We observe that

$$\begin{array}{c}G\left(\theta ,\lambda \right)=2{\int }_{{\sigma }_1}^{\theta }{\int }_{{\nu }_1}^{\lambda }\phi \left(\tau ,r\right)drd\tau -\frac{\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)}{8h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\hfill \\ \times \left[\phi \left(\frac{{\sigma }_1+\theta }{2},{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\lambda \right)\right]-\frac{\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)}{8h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\\ \times \left[\phi \left(\theta ,\frac{{\nu }_1+\lambda }{2}\right)+\phi \left({\sigma }_1,\frac{{\nu }_1+\lambda }{2}\right)\right]-\frac{\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)}{4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)}\phi \left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)\\ \le 2{\int }_{{\sigma }_1}^{\theta }{\int }_{{\nu }_1}^{\lambda }\phi \left(\tau ,r\right)drd\tau -2\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)\left[\phi \left(\frac{{\sigma }_1+\theta }{2},{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+\theta }{2},\lambda \right)\right]\\ \times {\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau -2\left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right)\left[\phi \left(\theta ,\frac{{\nu }_1+\lambda }{2}\right)+\phi \left({\sigma }_1,\frac{{\nu }_1+\lambda }{2}\right)\right]\\ \times {\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau -4f\left(\frac{{\sigma }_1+\theta }{2},\frac{{\nu }_1+\lambda }{2}\right)\\ \hfill \times \left(\theta -{\sigma }_1\right)\left(\lambda -{\nu }_1\right){\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau \le F\left(\theta ,\lambda \right).\end{array}$$

(30)

From the second inequality in (22), it is obvious that $F\left(\theta ,\lambda \right)\ge 0$. Hence, (23) is established. □

Remark 7.

Suppose that the assumptions of Theorem 8 are satisfied and $\theta ={\sigma }_2$, $\lambda ={\nu }_1$, then from (29) we obtain that

$$\begin{array}{c}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau ,r\right)drd\tau -\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)\hfill \\ \times \left[\frac{\phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)}{4}\right]{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau \\ \le \phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right){\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau \\ +\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)\left\{\frac{1}{2}\left[\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},{\nu }_1\right)+\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},{\nu }_2\right)\right]\right.\\ \hfill \left.+\frac{1}{2}\left[\phi \left({\sigma }_2,\frac{{\nu }_1+{\nu }_2}{2}\right)+\phi \left({\sigma }_1,\frac{{\nu }_1+{\nu }_2}{2}\right)\right]\right\}{\int }_0^1{h}_1\left(\tau \right)d\tau {\int }_0^1{h}_2\left(\tau \right)d\tau .\end{array}$$

(31)

3. Some Mappings Related to Hermite–Hadamard-Type Inequalities

Let us define two mappings on the rectangle $\left[0,1\right]\times \left[0,1\right]$

$$\begin{array}{cc}\hfill H\left(\tau ,s\right)& =\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}\hfill \\ \hfill & \times {\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2},s\lambda +\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}\right)d\lambda d\theta \hfill \end{array}$$

and

$$\begin{array}{cc}\hfill F\left(\tau ,s\right)& =\frac{1}{{\left({\sigma }_2-{\sigma }_1\right)}^2{\left({\nu }_2-{\nu }_1\right)}^2}\hfill \\ \hfill & \times {\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,sz+\left(1-s\right)w\right)d\lambda d\theta dzdw.\hfill \end{array}$$

We refer the interested readers to [6] for the properties of these two mappings for coordinated convex functions defined on a rectangle from the plane ${\mathbb{R}}^2$. We investigate which of these properties can be generalized for coordinated $\left(h_1,h_2\right)$-convex functions defined on a rectangle from the plane ${\mathbb{R}}^2$.

Theorem 9.

Let φ be coordinated $\left(h_1,h_2\right)$-convex functions defined on rectangle $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ from the plane ${\mathbb{R}}^2$ and $\left[0,1\right]\times \left[0,1\right]$. Then, the function H is coordinated $\left(h_1,h_2\right)$-convex on $\left[0,1\right]\times \left[0,1\right]$ and for all $\left(\tau ,s\right)\in \left[0,1\right]\times \left[0,1\right]$

$$H\left(0,0\right)\le \tau s{\mathcal{C}}_{1}H\left(\tau ,s\right),$$

(32)

where

 (i)

$\tau s{\mathcal{C}}_1=4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right),$ in general case or

 (ii)

$\tau s{\mathcal{C}}_1=min\left\{\begin{array}{c}4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right),\\ \frac{4{\int }_0^1{\int }_0^1{h}_1\left(\frac{{\sigma }_2-{\sigma }_1}{2}\tau \theta \right)h_2\left(\frac{{\nu }_2-{\nu }_1}{2}sz\right)dzd\theta }{{\int }_0^1{\int }_0^1{\int }_0^1{\int }_0^1{h}_1\left(\frac{{\sigma }_2-{\sigma }_1}{2}\tau \left(\lambda +\theta \right)\right)h_2\left(\frac{{\nu }_2-{\nu }_1}{2}s\left(w+z\right)\right)d\lambda d\theta dwdz}\end{array}\right\}$, $h_1,h_2$ satisfy (i) or (ii) of Theorem 7.

Proof. 

Let $\alpha$, $\beta$, ${\alpha }_1$, ${\beta }_1\in \left[0,1\right]$ with $\alpha +\beta =1$, ${\alpha }_1+{\beta }_1=1$ and $\left({\tau }_1,s_1\right)$, $\left({\tau }_2,s_2\right)\in \left[0,1\right]\times \left[0,1\right]$. Then, by using the fact that $\phi$ is a coordinated $\left(h_1,h_2\right)$-convex function on rectangle $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, we have

$$\begin{array}{c}H\left(\alpha {\tau }_1+\beta {\tau }_2,{\alpha }_1{s}_1+{\beta }_1{s}_2\right)=\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}\hfill \\ \times {\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\left(\alpha {\tau }_1+\beta {\tau }_2\right)\theta +\left(1-\left(\alpha {\tau }_1+\beta {\tau }_2\right)\right)\frac{{\sigma }_1+{\sigma }_2}{2},\right.\\ \left.\left({\alpha }_1{s}_1+{\beta }_1{s}_2\right)\lambda +\left(1-\left({\alpha }_1{s}_1+{\beta }_1{s}_2\right)\right)\frac{{\nu }_1+{\nu }_2}{2}\right)d\lambda d\theta \\ =\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left[\alpha \left({\tau }_1\theta +\left(1-{\tau }_1\right)\frac{{\sigma }_1+{\sigma }_2}{2}\right)\right.\\ +\beta \left({\tau }_2\theta +\left(1-{\tau }_2\right)\frac{{\sigma }_1+{\sigma }_2}{2}\right),{\alpha }_1\left(s_1\lambda +\left(1-s_1\right)\frac{{\nu }_1+{\nu }_2}{2}\right)\\ \left.+{\beta }_1\left(s_2\lambda +\left(1-s_2\right)\frac{{\nu }_1+{\nu }_2}{2}\right)\right]d\lambda d\theta \\ \le \frac{h_1\left(\alpha \right)h_2\left({\alpha }_1\right)}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\begin{array}{c}{\tau }_1\theta +\left(1-{\tau }_1\right)\frac{{\sigma }_1+{\sigma }_2}{2},\\ s_1\lambda +\left(1-s_1\right)\frac{{\nu }_1+{\nu }_2}{2}\end{array}\right)d\lambda d\theta \\ \hfill +\frac{h_1\left(\alpha \right)h_2\left({\beta }_1\right)}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\begin{array}{c}{\tau }_1\theta +\left(1-{\tau }_1\right)\frac{{\sigma }_1+{\sigma }_2}{2},\\ s_2\lambda +\left(1-s_2\right)\frac{{\nu }_1+{\nu }_2}{2}\end{array}\right)d\lambda d\theta \end{array}$$

$$\begin{array}{c}+\frac{h_1\left(\beta \right)h_2\left({\alpha }_1\right)}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\begin{array}{c}{\tau }_2\theta +\left(1-{\tau }_2\right)\frac{{\sigma }_1+{\sigma }_2}{2},\\ s_1\lambda +\left(1-s_1\right)\frac{{\nu }_1+{\nu }_2}{2}\end{array}\right)d\lambda d\theta \hfill \\ \hfill +\frac{h_1\left(\beta \right)h_2\left({\beta }_1\right)}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\begin{array}{c}{\tau }_2\theta +\left(1-{\tau }_2\right)\frac{{\sigma }_1+{\sigma }_2}{2},\\ s_2\lambda +\left(1-s_2\right)\frac{{\nu }_1+{\nu }_2}{2}\end{array}\right)d\lambda d\theta .\end{array}$$

It is thus proved that

$$\begin{array}{c}H\left(\alpha {\tau }_1+\beta {\tau }_2,{\alpha }_1{s}_1+{\beta }_1{s}_2\right)\hfill \\ \le h_1\left(\alpha \right)h_2\left({\alpha }_1\right)H\left({\tau }_1,s_1\right)+h_1\left(\alpha \right)h_2\left({\beta }_1\right)H\left({\tau }_1,s_2\right)\\ \hfill +h_1\left(\beta \right)h_2\left({\alpha }_1\right)H\left({\tau }_2,s_1\right)+h_1\left(\beta \right)h_2\left({\beta }_1\right)H\left({\tau }_2,s_2\right).\end{array}$$

Hence, it is proved that H is coordinated $\left(h_1,h_2\right)$-convex on $\left[0,1\right]\times \left[0,1\right]$.

By making use of the change of variables $u_1=\tau \theta +\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2}$ and $u_2=s\lambda +\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}$, we obtain

$$\begin{array}{c}H\left(\tau ,s\right)=\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}\hfill \\ \times {\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2},s\lambda +\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}\right)d\lambda d\theta \\ =\frac{1}{\tau s\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{\tau {\sigma }_1+\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2}}^{\tau {\sigma }_2+\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2}}{\int }_{sc+\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}}^{s{\nu }_2+\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}}\phi \left(u_1,u_2\right)d{u}_{2}d{u}_1\\ \hfill =\frac{1}{\left(u_1^U-u_1^L\right)\left(u_2^L-u_2^L\right)}{\int }_{u_1^L}^{u_1^U}{\int }_{u_2^L}^{u_2^L}\phi \left(u_1,u_2\right)d{u}_{2}d{u}_1,\end{array}$$

(33)

where $u_1^L=\tau {\sigma }_1+\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2}$, $u_1^U=\tau {\sigma }_2+\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2}$, $u_2^L=sc+\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}$ and $u_2^L=s{\nu }_2+\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}$.

Multiplying both sides of (33) by ${\mathcal{C}}_1$ and by Theorem 8, we obtain

$$\begin{array}{c}{\mathcal{C}}_{1}H\left(\tau ,s\right)=\frac{{\mathcal{C}}_1}{\left(u_1^U-u_1^L\right)\left(u_2^L-u_2^L\right)}{\int }_{u_1^L}^{u_1^U}{\int }_{u_2^L}^{u_2^L}\phi \left(u_1,u_2\right)d{u}_{2}d{u}_1\hfill \\ \hfill \ge \phi \left(\frac{u_1^L+u_1^U}{2},\frac{u_2^L+u_2^U}{2}\right)=\phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)=H\left(0,0\right),\end{array}$$

(34)

where ${\mathcal{C}}_1$ can be calculated according to Theorem 8 with the change of variables $u_1=\tau \theta +\left(1-\tau \right)\frac{{\sigma }_1+{\sigma }_2}{2}$, $u_2=s\lambda +\left(1-s\right)\frac{{\nu }_1+{\nu }_2}{2}$ and $u_1^L$, $u_1^U$, $u_2^L$, $u_2^L$ as given above. □

Remark 8.

If φ is coordinated convex on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, then we have the relation $H\left(0,0\right)\le H\left(\tau ,s\right)$ which has already been proven in [6]. If φ is coordinated $\left(s_1,s_2\right)$-convex on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]\subset {\left(0,\infty \right)}^2$, then we have the relation $H\left(0,0\right)\le \frac{\left(s_1+2\right)\left(s_2+2\right)}{\left(2^{s_1+1}-1\right)\left(2^{s_2+1}-1\right)}H\left(\tau ,s\right)$.

Theorem 10.

Let φ be coordinated $\left(h_1,h_2\right)$-convex functions defined on rectangle $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ from the plane ${\mathbb{R}}^2$ and $\left[0,1\right]\times \left[0,1\right]$. Then, the function F is symmetric with respect to $\frac{1}{2}$ and $\left(h_1,h_2\right)$-convex functions on $\left[0,1\right]\times \left[0,1\right]$. Moreover, the following inequalities hold

$$4h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)F\left(\tau ,s\right)\ge F\left(\frac{1}{2},\frac{1}{2}\right)$$

(35)

and

$$\tau s{\mathcal{C}}_{1}F\left(\tau ,s\right)\ge H\left(1-\tau ,1-s\right),$$

(36)

where ${\mathcal{C}}_1$ is defined as in the previous theorem.

Proof. 

We can observe that

$$\frac{\theta +\lambda }{2}=\frac{1}{2}\left[\tau \theta +\left(1-\tau \right)\lambda \right]+\frac{1}{2}\left[\left(1-\tau \right)\theta +\tau \lambda \right]$$

and

$$\frac{z+w}{2}=\frac{1}{2}\left[\tau z+\left(1-\tau \right)w\right]+\frac{1}{2}\left[\left(1-\tau \right)z+\tau w\right].$$

Applying the coordinated $\left(h_1,h_2\right)$-convexity of $\phi$ on rectangle $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, we obtain

$$\begin{array}{c}\phi \left(\frac{\theta +\lambda }{2},\frac{z+w}{2}\right)\le h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)\hfill \\ \times \left[\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,\tau z+\left(1-\tau \right)w\right)+\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,\left(1-\tau \right)z+\tau w\right)\right.\\ \hfill \left.+\phi \left(\left(1-\tau \right)\theta +\tau \lambda ,\tau z+\left(1-\tau \right)w\right)+\phi \left(\left(1-\tau \right)\theta +\tau \lambda ,\left(1-\tau \right)z+\tau w\right)\right].\end{array}$$

(37)

Integrating both sides of (37) over $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ with respect to $\lambda$ and $\theta$, and with respect to $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ with respect to w and z, we obtain

$$\begin{array}{c}\frac{1}{{\left({\sigma }_2-{\sigma }_1\right)}^2{\left({\nu }_2-{\nu }_1\right)}^2}\phi \left(\frac{\theta +\lambda }{2},\frac{z+w}{2}\right)\le h_1\left(\frac{1}{2}\right)h_2\left(\frac{1}{2}\right)\hfill \\ \times \left[{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,\tau z+\left(1-\tau \right)w\right)d\lambda d\theta dzdw\right.\\ +{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,\left(1-\tau \right)z+\tau w\right)d\lambda d\theta dzdw\\ +{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\left(1-\tau \right)\theta +\tau \lambda ,\tau z+\left(1-\tau \right)w\right)d\lambda d\theta dzdw\\ \hfill \left.+{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\left(1-\tau \right)\theta +\tau \lambda ,\left(1-\tau \right)z+\tau w\right)d\lambda d\theta dzdw\right].\end{array}$$

(38)

Since

$$\begin{array}{c}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,\tau z+\left(1-\tau \right)w\right)d\lambda d\theta dzdw\hfill \\ ={\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,\left(1-\tau \right)z+\tau w\right)d\lambda d\theta dzdw\\ ={\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\left(1-\tau \right)\theta +\tau \lambda ,\tau z+\left(1-\tau \right)w\right)d\lambda d\theta dzdw\\ \hfill ={\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\left(1-\tau \right)\theta +\tau \lambda ,\left(1-\tau \right)z+\tau w\right)d\lambda d\theta dzdw.\end{array}$$

Thus, (35) is established.

Let us now prove the inequality (36). Let $\lambda$ and w be fixed and consider

$$H_{\lambda ,w}\left(\tau ,s\right)=\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau \theta +\left(1-\tau \right)\lambda ,sz+\left(1-s\right)w\right)dzd\theta .$$

By making the substitutions $u_1=\tau \theta +\left(1-\tau \right)\lambda$ and $u_2=sz+\left(1-s\right)w$, we obtain that

$$H_{\lambda ,w}\left(\tau ,s\right)=\frac{1}{\left(u_1^U-u_1^L\right)\left(u_2^U-u_2^L\right)}{\int }_{u_1^L}^{u_1^U}{\int }_{u_2^L}^{u_2^U}\phi \left(u_1,u_2\right)d{u}_{2}d{u}_1,$$

where $u_1^L=\tau {\sigma }_1+\left(1-\tau \right)\lambda$, $u_1^U=\tau {\sigma }_2+\left(1-\tau \right)\lambda$, $u_2^L=sc+\left(1-s\right)w$ and $u_2^L=s{\nu }_2+\left(1-s\right)w$.

According to Theorem 8, we obtain that

$$\begin{array}{c}{\mathcal{C}}_1{H}_{\lambda ,w}\left(\tau ,s\right)=\frac{{\mathcal{C}}_1}{\left(u_1^U-u_1^L\right)\left(u_2^U-u_2^L\right)}{\int }_{u_1^L}^{u_1^U}{\int }_{u_2^L}^{u_2^U}\phi \left(u_1,u_2\right)d{u}_{2}d{u}_1\hfill \\ \ge \phi \left(\frac{u_1^L+u_1^U}{2},\frac{u_2^L+u_2^U}{2}\right)\\ \hfill =\phi \left(\tau \frac{{\sigma }_1+{\sigma }_2}{2}+\left(1-\tau \right)\lambda ,s\frac{{\nu }_1+{\nu }_2}{2}+\left(1-\tau \right)w\right).\end{array}$$

(39)

Integrating with respect to $\lambda$ over $\left[{\sigma }_1,{\sigma }_2\right]$ and with respect to w over $\left[{\nu }_1,{\nu }_2\right]$ and dividing by $\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)$, we obtain

$$\tau s{\mathcal{C}}_{1}F\left(\tau ,s\right)\ge H\left(1-\tau ,1-s\right),$$

which is the desired result. □

Remark 9.

If $\tau s{\mathcal{C}}_1>0$, then we have

$$F\left(\tau ,s\right)\ge \frac{1}{\tau s{\mathcal{C}}_1}H\left(1-\tau ,1-s\right)$$

Replacing τ by $1-\tau$ and s by $1-s$, we have

$$F\left(1-\tau ,1-s\right)\ge \frac{1}{\left(1-\tau \right)\left(1-s\right){\mathcal{C}}_1}H\left(\tau ,s\right).$$

Since F is symmetric with respect to $\frac{1}{2}$ on coordinates on $\left[0,1\right]\times \left[0,1\right]$, we obtain

$$F\left(\tau ,s\right)=F\left(1-\tau ,1-s\right).$$

Thus, we have

$$F\left(\tau ,s\right)\ge max\left\{\frac{1}{\tau s{\mathcal{C}}_1}H\left(1-\tau ,1-s\right),\frac{1}{\left(1-\tau \right)\left(1-s\right){\mathcal{C}}_1}H\left(\tau ,s\right)\right\}.$$

 (i)

If φ is coordinated convex on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, then

$$F\left(\tau ,s\right)\ge max\left\{H\left(1-\tau ,1-s\right),H\left(\tau ,s\right)\right\}.$$

 (ii)

If $h_1,h_2$ are multiplicative functions, then $\left(1-\tau \right)\left(1-s\right){\mathcal{C}}_1=\tau s{\mathcal{C}}_1$; hence, we obtain

$$F\left(\tau ,s\right)\ge \frac{1}{\tau s{\mathcal{C}}_1}max\left\{H\left(1-\tau ,1-s\right),H\left(\tau ,s\right)\right\}.$$

 (iii)

If φ is a coordinated $\left(s_1,s_2\right)$-convex function on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$, then

$$\begin{array}{c}F\left(\tau ,s\right)\ge \frac{\left(s_1+2\right)\left(s_2+2\right)}{\left(2^{s_1+1}-1\right)\left(2^{s_2+1}-1\right)}max\left\{H\left(1-\tau ,1-s\right),H\left(\tau ,s\right)\right\}\hfill \\ \hfill \ge 2^{s_1+s_2-2}max\left\{H\left(1-\tau ,1-s\right),H\left(\tau ,s\right)\right\}.\end{array}$$

4. Applications to Means

If f is a concave and $\left(h_1,h_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ with ${\int }_0^1{\int }_0^1{h}_1\left(u\right)h_2\left(v\right)dudv>0$, then the classical Hermite–Hadamard-type inequalities (4) and Theorems 6 and 7 give us

$$\begin{array}{c}\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\theta ,\lambda \right)d\lambda d\theta \hfill \\ \hfill \le \phi \left(\frac{{\sigma }_1+{\sigma }_2}{2},\frac{{\nu }_1+{\nu }_2}{2}\right)\le \mathcal{C}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(\tau ,r\right)drd\tau \end{array}$$

(40)

and

$$\begin{array}{c}\frac{1}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right){\int }_0^1{\int }_0^1{h}_1\left(u\right)h_2\left(v\right)dudv}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(u,v\right)dudv\hfill \\ \le \phi \left({\sigma }_1,{\nu }_1\right)+\phi \left({\sigma }_1,{\nu }_2\right)+\phi \left({\sigma }_2,{\nu }_1\right)+\phi \left({\sigma }_2,{\nu }_2\right)\\ \hfill \le \frac{4}{\left({\sigma }_2-{\sigma }_1\right)\left({\nu }_2-{\nu }_1\right)}{\int }_{{\sigma }_1}^{{\sigma }_2}{\int }_{{\nu }_1}^{{\nu }_2}\phi \left(u,v\right)dudv.\end{array}$$

(41)

If f is a concave and $\left(h_1,h_2\right)$-convex function on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ with ${\int }_0^1{\int }_0^1{h}_1\left(u\right)h_2\left(v\right)dudv>0$, then the inequalites (40) and (41) hold in the reversed direction.

Let the functions $\phi$ and $h_m$ and $h_n$ be defined as $h_m\left(\theta \right)={\theta }^m$, $h_n\left(\theta \right)=x^n$, $f\left(\theta ,\lambda \right)={\theta }^p{\lambda }^q$, $\theta ,\lambda >0$, $m,n,p,q\in \mathbb{R}$, then

(i)

the function $\phi$ is $\left(h_m,h_n\right)$-convex on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ if $p,q\in \left(-\infty ,0\right]\cup \left[1,\infty \right)$ and $m,n\le 1$ or $p,q\in \left(0,1\right)$ and $m\le p$, $n\le q$.

(ii)

the function $\phi$ is $\left(h_m,h_n\right)$-convex on the coordinates on $\left[{\sigma }_1,{\sigma }_2\right]\times \left[{\nu }_1,{\nu }_2\right]$ if $p,q\in \left(0,1\right)$ and $m,n\ge 1$ or $p,q>1$ and $m\ge p$, $n\ge q$.

Let us now consider the case when p, $q\in \left(0,1\right)$ and $0\le m\le p$, $0\le n\le q$, then we have the inequalities

$$\begin{array}{c}{L}_m^m\left({\sigma }_1,{\sigma }_2\right)L_n^n\left({\nu }_1,{\nu }_2\right)\le A^m\left({\sigma }_1,{\sigma }_2\right)A^n\left({\nu }_1,{\nu }_2\right)\hfill \\ \hfill \le \frac{\left(d-c\right)\left(b-a\right)(p+2)(q+2)}{\left(2^{p+1}-1\right)\left(2^{q+1}-1\right)}{L}_m^m\left({\sigma }_1,{\sigma }_2\right)L_n^n\left({\nu }_1,{\nu }_2\right)\end{array}$$

(42)

and

$$\begin{array}{c}\frac{(p+1)(q+1)L_m^m\left({\sigma }_1,{\sigma }_2\right)L_n^n\left({\nu }_1,{\nu }_2\right)}{4}\hfill \\ \hfill \le A\left({\sigma }_1^m{\nu }_1^n,{\sigma }_1^m{\nu }_2^n,{\sigma }_2^m{\nu }_1^n,{\sigma }_2^m{\nu }_2^m\right)\le L_m^m\left({\sigma }_1,{\sigma }_2\right)L_n^n\left({\nu }_1,{\nu }_2\right),\end{array}$$

(43)

where

$$A\left({\lambda }_1,{\lambda }_2\cdots {\lambda }_t\right)=\frac{{\sum }_{t=1}^n{\lambda }_t}{n}$$

and

$$L_s\left({\sigma }_1,{\nu }_1\right)={\left[\frac{{\nu }_1^{s+1}-{\sigma }_1^{s+1}}{\left(s+1\right)\left({\nu }_1-{\sigma }_1\right)}\right]}^{\frac{1}{s}},s\in \mathbb{R}\setminus \left\{0,-1\right\},{\sigma }_1,{\nu }_1>0.$$

If m, $n>1$ and $p\ge m$ and $q\ge n$, then the inequalities (42) and (43) hold in the reversed direction.

5. Conclusions

The research in this paper discusses some new inequalities of Hermite–Hadamard–Fejér type for coordinated $\left(h_1,h_2\right)$-convex functions on the rectangle from the plane ${\mathbb{R}}^2$. As a result, we also obtained new generalizations of Hermite–Hadamard-type inequalities already proved in a research by Dragomir [6]. In this study, we observed that there is no change in the properties associated with the integral mean of the function $\phi$ of two variables when the class of coordinated convex functions is extended to the class of coordinated $(h_1,h_2)$-convex functions. Particular cases of the obtained results have also been discussed. We have discussed some properties of functionals defined over $[0,1]\times [0,1]$ which are connected with the Hermite–Hadamard-type inequalities for $(h_1,h_2)$-convex functions and obtained new results for the inequalities containing these mappings. Particular cases of the inequalities contained in these mappings have also been considered. Some applications are given at the end of this research. The results in this study could inspire the mathematicians working in the field of mathematical inequalities related to coordinated convex functions and its generalizations.