Unfolding the Transmission Dynamics of Monkeypox Virus: An Epidemiological Modelling Analysis
Abstract
:1. Introduction
2. Methods
2.1. Mpox Outbreak Data
2.2. Mpoxv Model Description
2.3. Fundamental Properties of the Mpoxv Model
2.3.1. Positivity of Model Solutions
2.3.2. Invariant Region
3. Analytical Results
3.1. Disease-Free Equilibrium and Basic Reproduction Number
3.2. Basic Reproduction Number
3.3. Endemic Equilibrium and Its Stability
3.3.1. Endemic Equilibrium
3.3.2. Global Stability Analysis of the Endemic Equilibrium
3.4. Bifurcation Analysis
4. Numerical Results
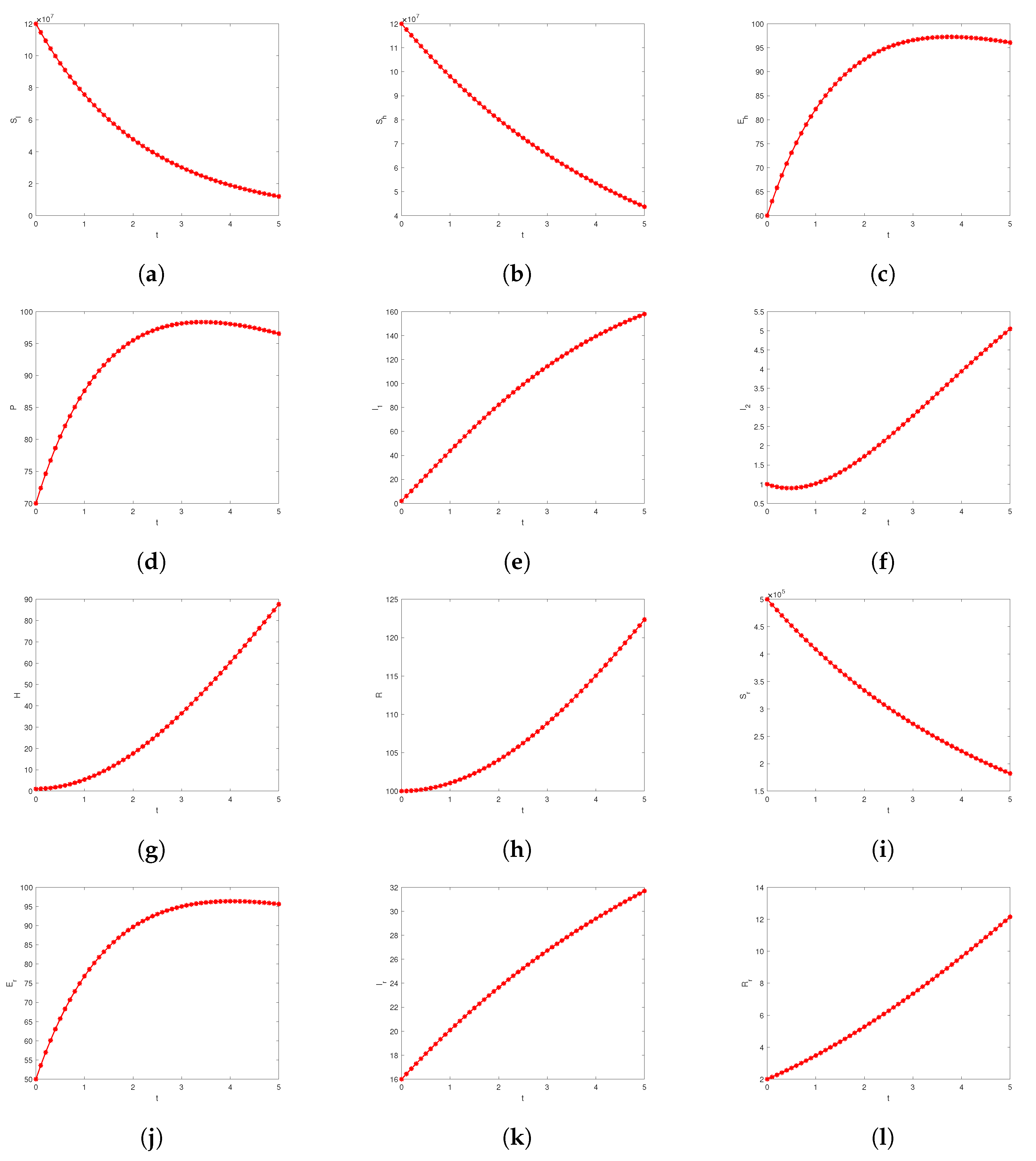
4.1. Model Prediction
4.2. Numerical Simulations
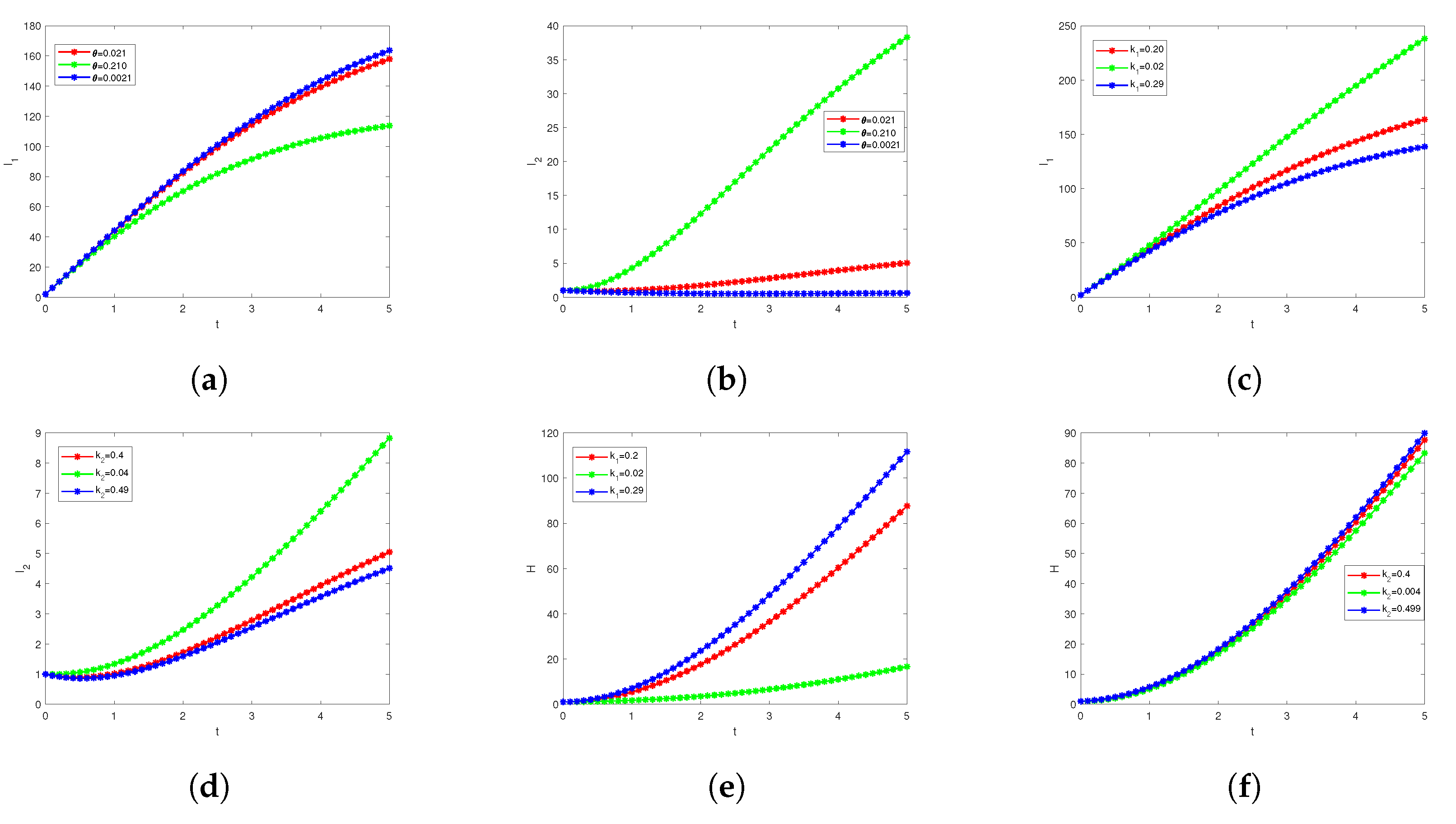
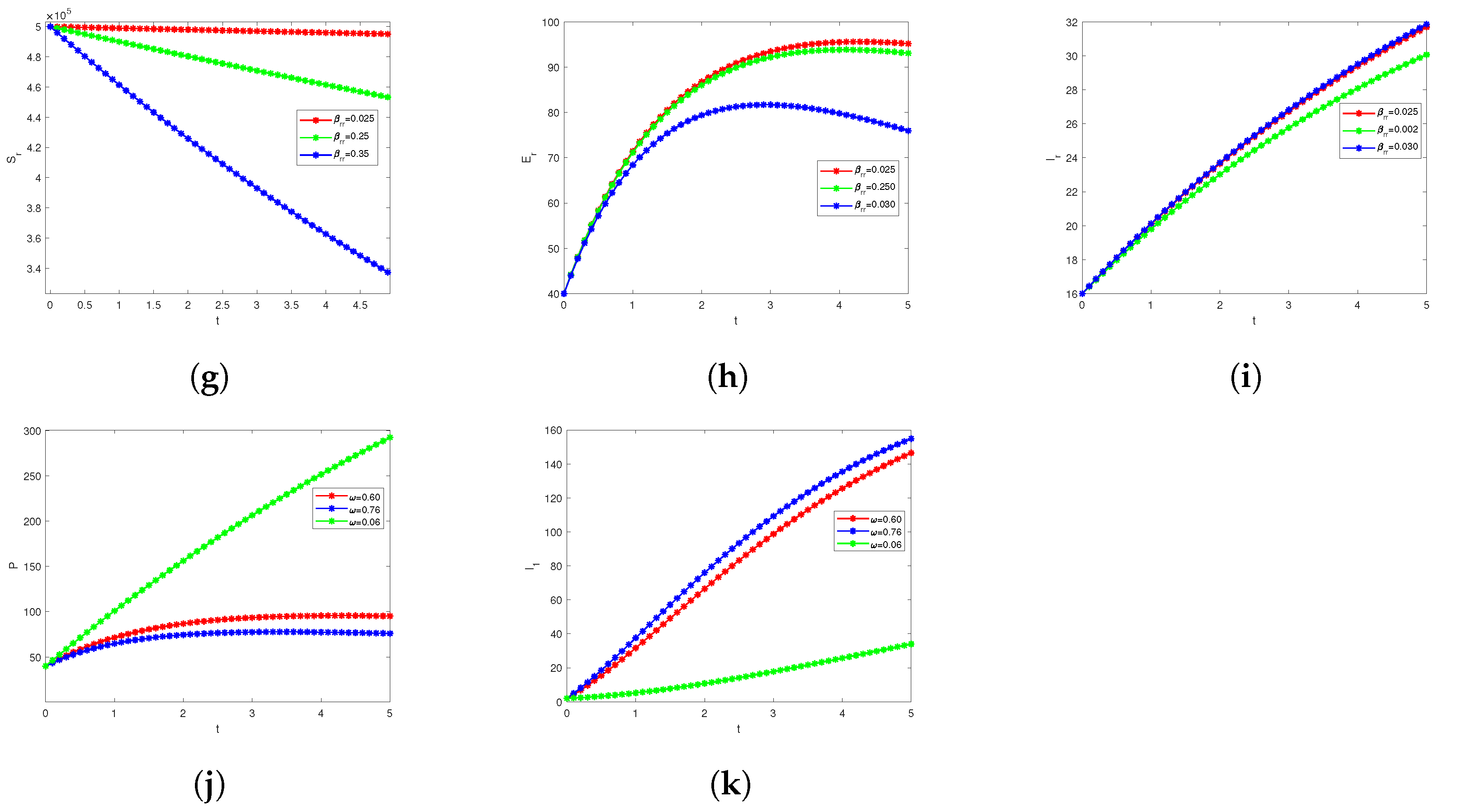
4.3. Sensitivity Analysis
5. Concluding Remarks
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sherrard-Smith, E.; Hogan, A.B.; Hamlet, A.; Watson, O.J.; Whittaker, C.; Winskill, P.; Ali, F.; Mohammad, A.B.; Uhomoibhi, P.; Maikore, I.; et al. The potential public health consequences of COVID-19 on malaria in Africa. Nat. Med. 2020, 26, 1411–1416. [Google Scholar] [CrossRef] [PubMed]
- World Health Organization. Monkeypox. Key Facts. 2022. Available online: https://www.who.int/news-room/fact-sheets/detail/monkeypox#:~:text=Monkeypox%20is%20usually%20a%20self,material%20contaminated%20with%20the%20virus (accessed on 12 October 2022).
- Musa, S.S.; Yusuf, A.; Bakare, E.A.; Abdullahi, Z.U.; Adamu, L.; Mustapha, U.T.; He, D. Unravelling the dynamics of Lassa fever transmission with differential infectivity: Modeling analysis and control strategies. Math. Biosci. Eng. 2022, 19, 13114–13136. [Google Scholar] [CrossRef] [PubMed]
- Asamoah, J.K.; Jin, Z.; Sun, G.Q. Non-seasonal and seasonal relapse model for Q fever disease with comprehen-sive cost-effectiveness analysis. Results Phys. 2021, 22, 103889. [Google Scholar] [CrossRef]
- Yuan, P.; Tan, Y.; Yang, L.; Aruffo, E.; Ogden, N.H.; Bélair, J.; Heffernan, J.; Arino, J.; Watmough, J.; Carabin, H.; et al. Assessing transmission risks and control strategy for monkeypox as an emerging zoonosis in a metropolitan area. J. Med. Virol. 2022, 95, e28137. [Google Scholar] [CrossRef]
- Kupferschmidt, K. Why monkeypox is mostly hitting men who have sex with men. Science 2022, 376, 1364–1365. [Google Scholar] [CrossRef]
- Du, Z.; Shao, Z.; Bai, Y.; Wang, L.; Herrera-Diestra, J.L.; Fox, S.J.; Ertem, Z.; Ertem, Z.; Lau, E.H.Y.; Cowling, B.J. Reproduction number of mon-keypox in the early stage of the 2022 multi-country outbreak. J. Trav. Med. 2022, 29, taac009. [Google Scholar] [CrossRef]
- Cowling, B.J.; Fang, V.J.; Riley, S.; Peiris, J.M.; Leung, G.M. Estimation of the serial interval of influenza. Epidemiology 2009, 20, 344. [Google Scholar] [CrossRef] [Green Version]
- Zhao, S. Estimating the time interval between transmission generations when negative values occur in the se-rial interval data: Using COVID-19 as an example. Math. Biosci. Eng. 2020, 17, 3512–3519. [Google Scholar] [CrossRef]
- Haider, N.; Guitian, J.; Simons, D.; Asogun, D.; Ansumana, R.; Honeyborne, I.; Velavan, T.P.; Ntoumi, F.; Valdoleiros, S.R.; Petersen, E.; et al. Increased outbreaks of monkeypox highlight gaps in actual disease burden in Sub-Saharan Africa and in animal reservoirs. Int. J. Infect. Dis. 2022, 122, 107–111. [Google Scholar] [CrossRef]
- Centers for Disease Control and Prevention. Monkeypox. 2022. Available online: https://www.cdc.gov/poxvirus/monkeypox/index.html (accessed on 29 November 2022).
- Endo, A.; Murayama, H.; Abbott, S.; Ratnayake, R.; Pearson, C.A.; Edmunds, W.J.; Fearon, E.; Funk, S. Heavy-tailed sexual con-tact networks and monkeypox epidemiology in the global outbreak. Science 2022, 2022, eadd4507. [Google Scholar]
- Akhmetzhanov, A.R.; Ponce, L.; Thompson, R.N. Emergence potential of monkeypox in the Western Pacific Region, July 2022. Int. J. Infect. Dis. 2022, 122, 829–831. [Google Scholar] [CrossRef] [PubMed]
- Antinori, A.; Mazzotta, V.; Vita, S.; Carletti, F.; Tacconi, D.; Lapini, L.E.; D’Abramo, A.; Cicalini, S.; Lapa, D.; Pittalis, S.; et al. Epidemiological, clinical and viro-logical characteristics of four cases of monkeypox support transmission through sexual contact, Italy, May 2022. Eurosurveillance 2022, 27, 2200421. [Google Scholar] [CrossRef] [PubMed]
- Najeeb, H.; Huda, Z. Monkeypox virus: A spreading threat for Pakistan? Ann. Med. Surg. 2022, 79, 103977. [Google Scholar] [CrossRef] [PubMed]
- Guo, Z.; Zhao, S.; Sun, S.; He, D.; Chong, K.C.; Yeoh, E.K. Estimation of the serial interval of monkeypox during the early outbreak in 2022. J. Med. Virol. 2022, 95, e28248. [Google Scholar] [CrossRef] [PubMed]
- Musa, S.S.; Abdullahi, Z.U.; Zhao, S.; Bello, U.M.; Hussaini, N.; Habib, A.G.; He, D. Transmission Dynamics of Monkeypox Virus in Nigeria during the Current COVID-19 Pandemic and Estimation of Effective Repro-duction Number. Vaccines 2022, 10, 2153. [Google Scholar] [CrossRef] [PubMed]
- Yuan, P.; Tan, Y.; Yang, L.; Aruffo, E.; Ogden, N.H.; Belair, J.; Arino, J.; Heffernan, J.; Watmough, J.; Carabin, H.; et al. Modelling vaccination and control strategies of outbreaks of monkeypox at gatherings. medRxiv 2022. [Google Scholar] [CrossRef]
- Ogoina, D.; Iroezindu, M.; James, H.I.; Oladokun, R.; Yinka-Ogunleye, A.; Wakama, P.; Otike-Odibi, B.; Usman, L.M.; Obazee, E.; Aruna, O.; et al. Clinical course and outcome of human monkeypox in Nigeria. Clin. Infect. Dis. 2020, 71, e210–e214. [Google Scholar] [CrossRef]
- Ogoina, D.; Izibewule, J.H.; Ogunleye, A.; Ederiane, E.; Anebonam, U.; Neni, A.; Oyeyemi, A.; Etebu, E.N.; Ihekweazu, C. The 2017 human monkey-pox outbreak in Nigeria—Report of outbreak experience and response in the Niger Delta University Teaching Hospital, Bayelsa State, Nigeria. PLoS ONE 2019, 14, e0214229. [Google Scholar] [CrossRef] [Green Version]
- Happi, C.; Adetifa, I.; Mbala, P.; Njouom, R.; Nakoune, E.; Happi, A.; Ndodo, N.; Ayansola, O.; Mboowa, G.; Bed-ford, T.; et al. Urgent need for a non-discriminatory and non-stigmatizing nomenclature for monkeypox virus. PLoS Biol. 2022, 20, e3001769. [Google Scholar] [CrossRef]
- Peter, O.J.; Kumar, S.; Kumari, N.; Oguntolu, F.A.; Oshinubi, K.; Musa, R. Transmission dynamics of Monkeypox virus: A mathematical modelling approach. Model. Earth Syst. Environ. 2022, 8, 3423–3434. [Google Scholar] [CrossRef]
- Nigeria Centre for Disease Control. Disease Situation Report. 2022. Available online: https://ncdc.gov.ng/diseases/sitreps (accessed on 1 December 2022).
- Musa, S.S.; Zhao, S.; Gao, D.; Lin, Q.; Chowell, G.; He, D. Mechanistic modelling of the large-scale Lassa fever epidemics in Nigeria from 2016 to 2019. J. Theoret. Biol. 2020, 2020, 110209. [Google Scholar] [CrossRef] [PubMed]
- Safi, M.A.; Gumel, A.B. Qualitative study of a quarantine/isolation model with multiple disease stages. Appl. Math. Comput. 2011, 218, 1941–1961. [Google Scholar] [CrossRef] [PubMed]
- Yang, C.; Wang, X.; Gao, D.; Wang, J. Impact of awareness programs on cholera dynamics: Two modeling ap-proaches. Bull. Math. Biol. 2017, 79, 2109–2131. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Roop, O.P.; Chinviriyasit, W.; Chinviriyasit, S. The effect of incidence function in backward bifurcation for malaria model with temporary immunity. Math. Biosci. 2015, 265, 47–64. [Google Scholar] [CrossRef]
- Musa, S.S.; Zhao, S.; Chan, H.S.; Jin, Z.; He, D.H. A mathematical model to study the 2014–2015 large-scale den-gue epidemics in Kaohsiung and Tainan cities in Taiwan, China. Math. Biosci. Eng. 2019, 16, 3841–3863. [Google Scholar] [CrossRef]
- Hussaini, N.; Okuneye, K.; Gumel, A.B. Mathematical analysis of a model for zoonotic visceral leishmaniasis. Infect. Dis. Model. 2017, 2, 455–474. [Google Scholar] [CrossRef]
- Van den Driessche, P.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for com-partmental models of disease transmission. Math. Bosci. 2002, 180, 29–48. [Google Scholar] [CrossRef]
- Van den Driessche, P. Reproduction numbers of infectious disease models. Infect. Dis. Model. 2017, 2, 288–303. [Google Scholar] [CrossRef]
- Diekmann, O.; Heesterbeek, J.A.; Metz, J.A. On the definition and the computation of the basic reproduction ratio R 0 in models for infectious diseases in heterogeneous populations. J. Math. Biol. 1990, 28, 365–382. [Google Scholar] [CrossRef] [Green Version]
- Usman, S.; Adamu, I.I. Modeling the transmission dynamics of the monkeypox virus infection with treatment and vaccination interventions. J. Appl. Math. Phys. 2017, 5, 2335. [Google Scholar] [CrossRef] [Green Version]
- LaSalle, J.P. The Stability of Dynamical Systems, Regional Conference Series in Applied Mathematics; SIAM: Philadephia, PA, USA, 1976. [Google Scholar]
- Musa, S.S.; Hussaini, N.; Zhao, S.; Daihai, H. Dynamical analysis of chikungunya and dengue co-infection model. Disc. Cont. Dyn. Syst.-B 2019, 22, 1–27. [Google Scholar] [CrossRef] [Green Version]
- Shuai, Z.; Van den Driessche, P. Global stability of infectious disease models using Lyapunov functions. SIAM J. Appl. Math. 2013, 73, 1513–1532. [Google Scholar] [CrossRef] [Green Version]
- Musa, S.S.; Baba, I.A.; Yusuf, A.; Sulaiman, T.A.; Aliyu, A.I.; Zhao, S.; He, D. Transmission dynamics of SARS-CoV-2: A modeling analysis with high-and-moderate risk populations. Res. Phys. 2021, 26, 104290. [Google Scholar] [CrossRef] [PubMed]
- Castillo-Chavez, C.; Song, B. Dynamical model of tuberculosis and their applications, Math. Biosci. Eng. 2004, 1, 361–404. [Google Scholar] [CrossRef]
- Carr, J. Applications of Centre Manifold Theory; Springer: New York, NY, USA, 1981. [Google Scholar]
- Statista, Global No.1 Data Platform. 2022. Available online: https://www.statista.com/ (accessed on 6 December 2022).
- Sengupta, P. The laboratory rat: Relating its age with humans. Int. J. Prev. Med. 2013, 4, 624–630. [Google Scholar]
- World Bank, Data, Population Website. Available online: https://data.worldbank.org/indicator/SP.POP.TOTL?locations=NG (accessed on 6 December 2022).
- Ojo, M.M.; Gbadamosi, B.; Benson, T.O.; Adebimpe, O.; Georgina, A.L. Modeling the dynamics of Lassa fever in Nigeria. J. Egyptian Math. Soc. 2021, 29, 1185. [Google Scholar] [CrossRef]
- Bankuru, S.V.; Kossol, S.; Hou, W.; Mahmoudi, P.; Rychtář, J.; Taylor, D. A game-theoretic model of Monkeypox to assess vaccination strategies. PeerJ 2020, 8, e9272. [Google Scholar] [CrossRef]
- Khan, A.; Naveed, M.; Dur-e-Ahmad, M.; Imran, M. Estimating the basic reproductive ratio for the Ebola out-break in Liberia and Sierra Leone. Infect. Dis. Pov. 2015, 4, 1–8. [Google Scholar]
- Gao, D.; Lou, Y.; He, D.; Porco, T.C.; Kuang, Y.; Chowell, G.; Ruan, S. Prevention and control of Zika as a mosqui-to-borne and sexually transmitted disease: A mathematical modeling analysis. Sci Rep. 2016, 6, 28070. [Google Scholar] [CrossRef] [Green Version]
- Zhao, S.; Stone, L.; Gao, D.; He, D. Modelling the large-scale yellow fever outbreak in Luanda, Angola, and the impact of vaccination. PLoS. Neglect. Trop. Dis. 2018, 12, e0006158. [Google Scholar] [CrossRef]
- Ghosh, I.; Tiwari, P.K.; Chattopadhyay, J. Effect of active case finding on dengue control: Implications from a mathematical model. J. Theor. Biol. 2019, 464, 50–62. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dubey, B.; Dubey, P.; Dubey, U.S. Dynamics of an SIR model with nonlinear incidence and treatment rate. Appl. Appl. Math. Int. J. (AAM) 2015, 10, 5. [Google Scholar]
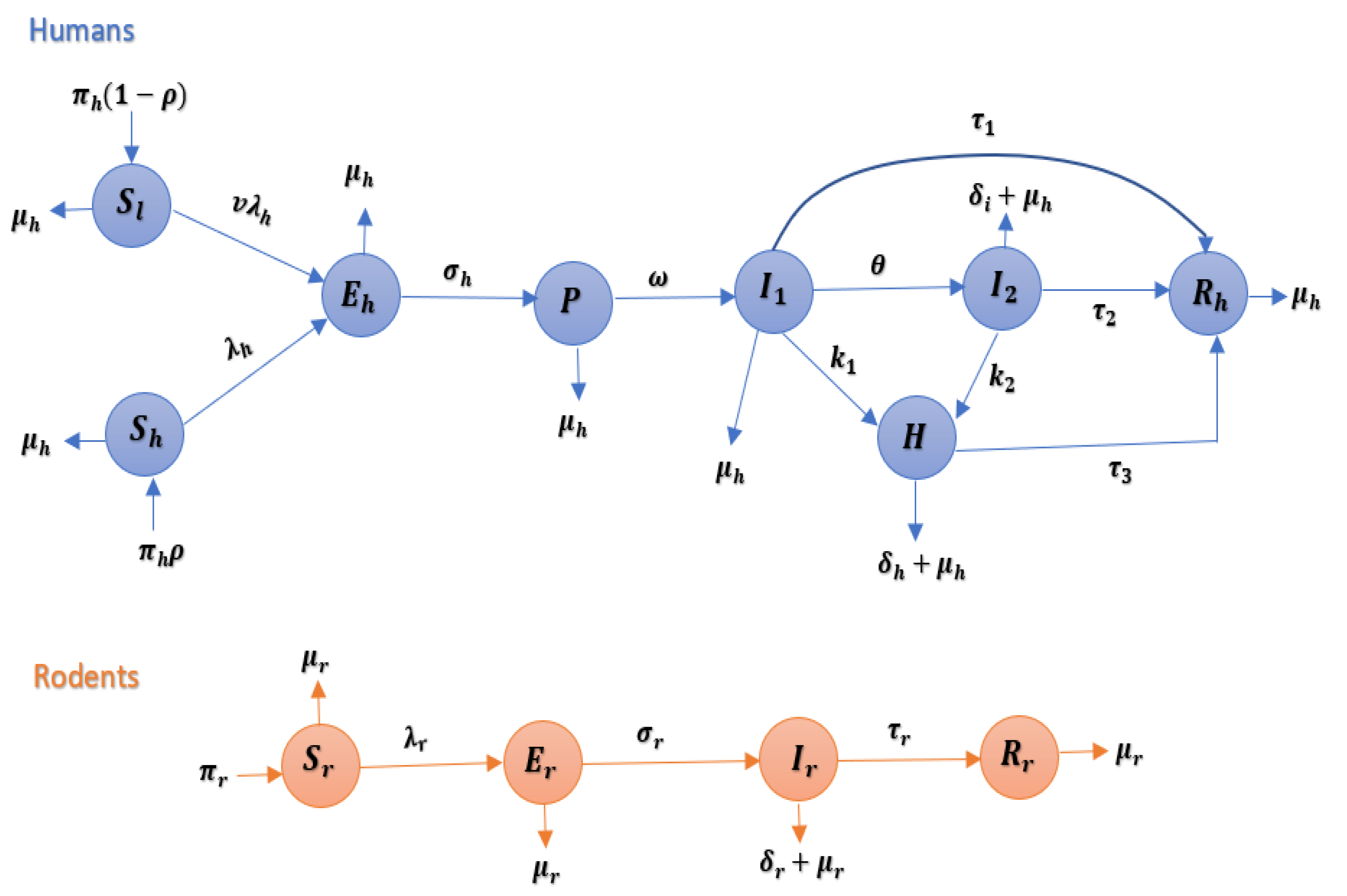


| Variable | Description |
|---|---|
| Overall human population | |
| Population of susceptible humans at high-risk | |
| Population of susceptible humans at low-risk | |
| Exposed humans | |
| P | Populations of infectious humans in the prodromal stage |
| Symptomatically mild infectious humans | |
| Symptomatically severe infectious humans | |
| H | Hospitalised humans |
| Recovered humans | |
| Total rodent population | |
| Susceptible rodents | |
| Exposed rodents | |
| Infectious rodents | |
| Recovered rodents | |
| Parameter | |
| Transmission rates/transmission probabilities | |
| Modification parameters | |
| Human recruitment rate (rodents) | |
| Fraction of newly recruited humans moving to | |
| v | Rate of reduction in infectiousness from |
| Progression rates | |
| Rate at which mpoxv progress from P to | |
| Progression rate from mild to severe disease | |
| Hospitalisation rates | |
| mpoxv-induced death rates | |
| Recovery rates | |
| Human (rodents) natural death rate |
| Parameter | Baseline (Range) | Units | Sources |
|---|---|---|---|
| – | Persons | [3,40] | |
| Rodents | Assumed | ||
| – | [3,40] | ||
| – | [41] | ||
| – | [3,24,42] | ||
| – | [3,43,44] | ||
| – | [45] | ||
| v | – | Dimensionless | Estimated |
| – | Estimated | ||
| – | Estimated | ||
| – | Estimated | ||
| – | Dimensionless | [5] | |
| – | Dimensionless | [5] | |
| – | [44] | ||
| – | [44] | ||
| – | [5] | ||
| – | Estimated | ||
| – | Estimated | ||
| – | Estimated | ||
| – | [5] | ||
| – | [5] | ||
| – | [5] | ||
| – | [44] | ||
| – | [5] | ||
| – | [5] | ||
| – | [44] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Shomrani, M.M.; Musa, S.S.; Yusuf, A. Unfolding the Transmission Dynamics of Monkeypox Virus: An Epidemiological Modelling Analysis. Mathematics 2023, 11, 1121. https://doi.org/10.3390/math11051121
Al-Shomrani MM, Musa SS, Yusuf A. Unfolding the Transmission Dynamics of Monkeypox Virus: An Epidemiological Modelling Analysis. Mathematics. 2023; 11(5):1121. https://doi.org/10.3390/math11051121
Chicago/Turabian StyleAl-Shomrani, Mohammed M., Salihu S. Musa, and Abdullahi Yusuf. 2023. "Unfolding the Transmission Dynamics of Monkeypox Virus: An Epidemiological Modelling Analysis" Mathematics 11, no. 5: 1121. https://doi.org/10.3390/math11051121
APA StyleAl-Shomrani, M. M., Musa, S. S., & Yusuf, A. (2023). Unfolding the Transmission Dynamics of Monkeypox Virus: An Epidemiological Modelling Analysis. Mathematics, 11(5), 1121. https://doi.org/10.3390/math11051121









