An Approach Using the Transfer Matrix Method (TMM) for Mandible Body Bone Calculus
Abstract
1. Introduction
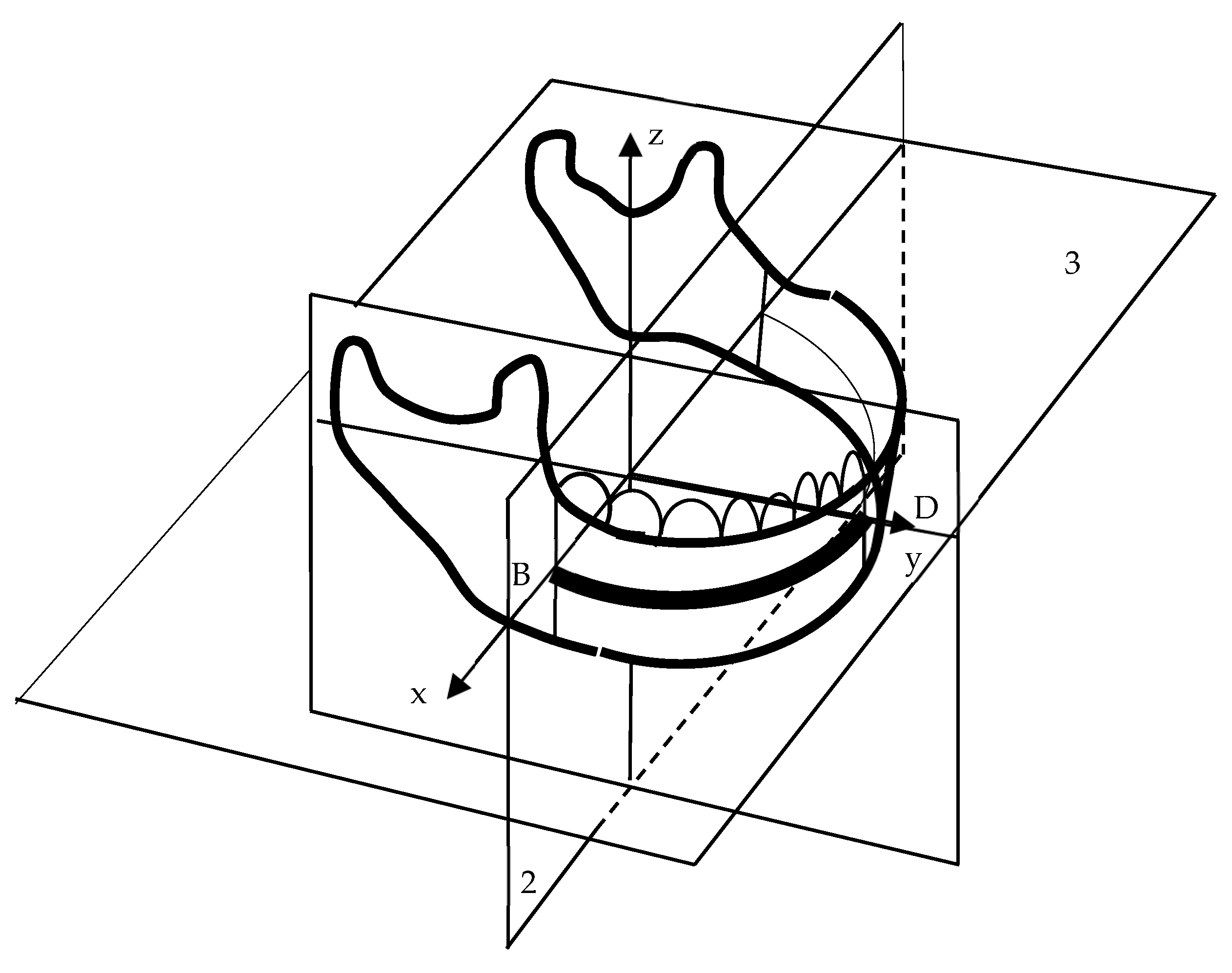
2. Mathematical Model for Mandible Body
3. Equations of a Plane Spring Loaded Perpendicular to Its Plane [1]
- .
- (-Mr)on—radial moment;
- (-Mt)on—tangential moment.
- A resultant with module .
- (-Mr + dMr) on ;
- (-Mt + dMt) on .
- γt (s) after tangent;
- γn (s) after normal;
- γt (s) ds;
- γn (s) ds;
- Projection on :dMt + Mr dψ + γt ds = 0,
- Projection on :dMr + Mt dψ + γn ds − Tds = 0,
- Projection on :where R is the radius of the spring curvature at a point in abscissa s, and we know thatd T = − p (s) ds.ds = R dψ.
- E—Longitudinal modulus of elasticity (Young’s modulus);
- G—Transverse modulus of elasticity;
- I—Moment of inertia around the horizontal axis;
- J—Torsional stiffness inertia.
4. Transfer Matrix of Spring Model Loaded Perpendicular to Its Plane
4.1. General Expression of the Transfer Matrix
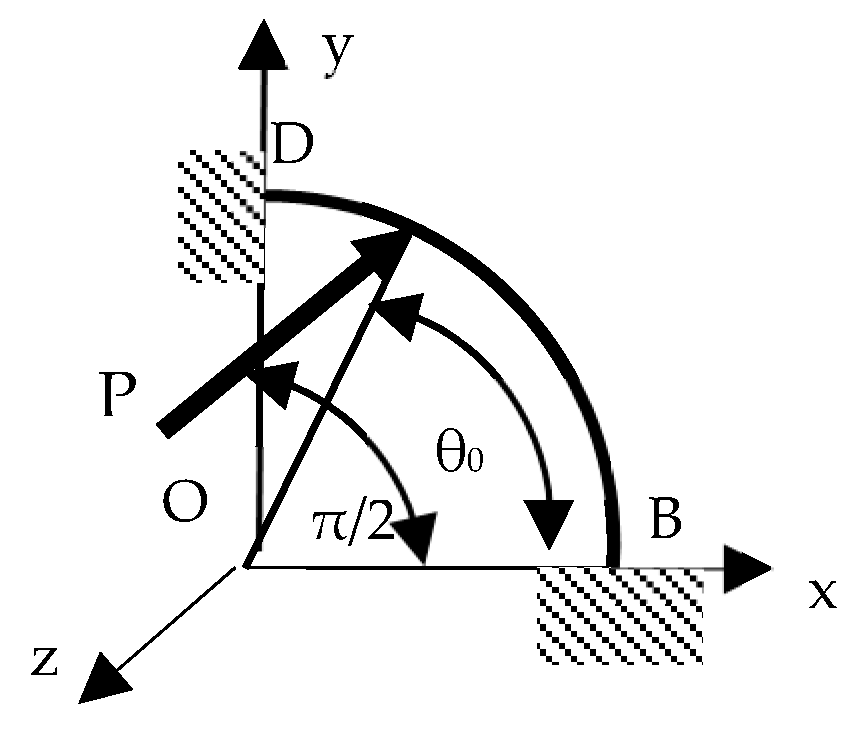
4.2. Calculus of Elements for the Vector Corresponding to an External Vertical Concentrate Load in Section at Angle θ = θ0
5. Application to a Model as a Quarter Circle for Mandible Body by TMM: Results
6. Discussion
Funding
Data Availability Statement
Conflicts of Interest
References
- Gery, P.-M.; Calgaro, J.A. Les Matrices-Transfer dans le calcul des Structures; Editions Eyrolles: Paris, France, 1987. [Google Scholar]
- Suciu, M.; Tripa, M.-S. Strength of Materials; ED. UTPRESS: Cluj-Napoca, Romania, 2021. [Google Scholar]
- Tripa, M.; Florescu, C.; Sorcoi, D.; Sorcoi, A.; Paunescu, D.; Suciu, M. About a Study for the Body of Mandible Bone by Transfer-Matrix Method. J. Multidiscip. Eng. Sci. Technol. 2020, 7, 13233–13237. Available online: www.jmest.org/wp-content/uploads/JMESTN42353644.pdf (accessed on 10 January 2022).
- Tripa, M.; Şolea, G.; Sorcoi, D.; Florescu, I.; Sorcoi, A.; Păunescu, D.; Bâlc, N.; Suciu, M. About buckling calculus of straight bars on elastic environment by Transfer-Matrix Method (TMM) for dental implants. MATEC Web Conf. 2018, 178, 4007. [Google Scholar] [CrossRef][Green Version]
- TRIPA, M.; Sorcoi, D.; Florescu, I.; Sorcoi, L.; Păunescu, D.; Suciu, M. Contribution on The Analytical Study of Dental Bridge by Similarity to a Beam by Transfer-Matrix Method (TMM); ATN: Cluj-Napoca, Romania, 2019. [Google Scholar]
- David, S.; Sârbu1, I.; Comăneanu, R.M.; Pătroi, D.N. Finite element method studies on the transmission of masticator forces to the bone substrate via titanium and zirconium implants. Ro J. Stomatol. 2018, 64. [Google Scholar] [CrossRef]
- Sirbu, D. Creating the bone supply for patients with severe atrophy of the mandible for rehabilitation prosthetic implants. R. J. Med. Stomatol. 2013, 3, 47–53. [Google Scholar]
- Traistaru, V.; Nitescu, M.; Ionescu, C.; David, M.; Bodnar, D.C.; Bodnar, T.; Comanescu, C.; Burlibasa, M. Study on the Position of the Mandibular Channel at Totally Edented Patients—Clinical Implications, Acta Medica Transilvanica (ISSN 2285-7079, ISSN-L 1453-1968), Sibiu, Romania. 2014, Volume II (III). Available online: https://www.amtsibiu.ro/index.php?option=com_content&view=article&id=1688:study-of-the-mandibular-canal-in-totally-edentulous-patients-clinical-implications&catid=30:nr-3-2014 (accessed on 3 January 2022).
- Ciapetti, G.; Ambrosio, L.; Marletta, G.; Baldini, N.; Giunti, A. Human bone marrow stromal cells: In vitro expansion and differentiation for bone engineering. Biomaterials 2006, 27, 6150–6160. [Google Scholar] [CrossRef] [PubMed]
- Botean, A.-I. Study of changing geometric characteristics in proximal femoral bone affected by osteoporosis in compliance with Singh index. Acta Tech. Napocensis. Ser. Appl. Math. Mech. 2016, 60, 3. [Google Scholar]
- Olaru, A.D.; Olaru, S.A.; Mihai, N.N. Proper Jacobian Pseudo Inverse Neural Network Matrix Method Applied to Robot Inverse Kinematics Controlling. Int. J. Mech. Eng. Robot. Res. 2016, 5, 2. [Google Scholar] [CrossRef]
- Matysiak, R.; Gegenwart, P.; Ochiai, A.; Steglich, F. Application of Numerical Quantum Transfer-Matrix Approach in the Randomly Diluted Quantum Spin Chains. In Parallel Processing and Applied Mathematics. PPAM 2017; Lecture Notes in Computer Science; Springer: Cham, Switzerland, 2018; pp. 359–367. [Google Scholar] [CrossRef]
- Abbasinasab, A.; Yanushkevich, S.N. Reliability evaluation of multivalued logic circuits via probabilistic transfer matrices. In Proceedings of the 25th IEEE Canadian Conference on Electrical and Computer Engineering (CCECE), Montreal, QC, Canada, 29 April–2 May 2012; 2012; pp. 1–4. [Google Scholar] [CrossRef]
- Liu, B.; Cai, L.; Bai, P.; Peng, W. Reliability evaluation for single event crosstalk via probabilistic transfer matrix. Microelectron. Reliab. 2012, 52, 1511–1514. [Google Scholar] [CrossRef]
- Zhou, J.; Pu, H. Analytical study on the evolutionary behavior of transfer matrix element moments in strongly coupled multimode systems. Opt. Express 2021, 29, 13987–14005. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, K.; Yonezawa, K.; Müller, A.; Avellan, F.; Tsujimoto, Y. Evaluation of a Dynamic Transfer Matrix for a Hydraulic Turbine. J. Fluids Eng. 2020, 142. [Google Scholar] [CrossRef]
- Jeng, S.-L.; Roy, R.; Chieng, W.-H. A Matrix Approach for Analyzing Signal Flow Graph. Information 2020, 11, 562. [Google Scholar] [CrossRef]
- BENTOSELA, F.; Cornean, H.; Fleury, B.H.; Marchetti, N. On the transfer matrix of a MIMO system. Math. Methods Appl. Sci. 2011, 34, 963–976. [Google Scholar] [CrossRef][Green Version]
- Mo, Z.; Song, G.; Hou, K.; Bolton, J.S. An iterative transfer matrix approach for estimating the sound speed and attenuation constant of air in a standing wave tube. J. Acoust. Soc. Am. 2022, 151, 4016–4027. [Google Scholar] [CrossRef] [PubMed]
- Wapenaar, K.; de Ridder, S.; Dukalski, M.; Reinicke, C. The propagator and transfer matrix for a 3D inhomogeneous dissipative acoustic medium, expressed in Marchenko focusing functions. In Second International Meeting for Applied Geoscience & Energy; Society of Exploration Geophysicists and American Association of Petroleum Geologists: Tulsa, OK, USA, 2022; 3694p, ISSN1 1052-3812. ISSN2 1949-4645. [Google Scholar] [CrossRef]
- Pillai, M.A.; Ebenezer, D.D.; Deenadayalan, E. Transfer matrix analysis of a duct with gradually varying arbitrary cross-sectional area. J. Acoust. Soc. Am. 2019, 146, 4435–4445. [Google Scholar] [CrossRef] [PubMed]
- Bernal, A.; Avendaño, J.; Torres, R.; García-Ravelo, J. Adaptable, transfer-matrix method for fixed-energy finite-width beams. Phys. Scr. 2021, 96, 035220. [Google Scholar] [CrossRef]
- Pérez-Alvarez, R.; Rodriguez-Coppola, H.; López-Gondar, J.; Lago-Izquierdo, M. Transfer Matrix in the Quasiclassical Approximation with Constant and Position-Dependent Mass. Resonant Tunneling. Phys. Status Solidi 1988, 145, 501–508. [Google Scholar] [CrossRef]
- Görlich, A. The Transfer Matrix in Four-Dimensional Causal Dynamical Triangulations. In Relativity and Gravitation; Springer: Cham, Switzerland, 2014; pp. 505–513. [Google Scholar] [CrossRef]
- Sarkar, S.; Padhy, A.; Nayak, C. Transfer matrix optimization of a one-dimensional photonic crystal cavity for enhanced absorption of monolayer graphene. Appl. Opt. 2022, 61, 8613. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suciu, M. An Approach Using the Transfer Matrix Method (TMM) for Mandible Body Bone Calculus. Mathematics 2023, 11, 450. https://doi.org/10.3390/math11020450
Suciu M. An Approach Using the Transfer Matrix Method (TMM) for Mandible Body Bone Calculus. Mathematics. 2023; 11(2):450. https://doi.org/10.3390/math11020450
Chicago/Turabian StyleSuciu, Mihaela. 2023. "An Approach Using the Transfer Matrix Method (TMM) for Mandible Body Bone Calculus" Mathematics 11, no. 2: 450. https://doi.org/10.3390/math11020450
APA StyleSuciu, M. (2023). An Approach Using the Transfer Matrix Method (TMM) for Mandible Body Bone Calculus. Mathematics, 11(2), 450. https://doi.org/10.3390/math11020450





