Fourth-Order Difference Scheme and a Matrix Transform Approach for Solving Fractional PDEs
Abstract
1. Introduction
2. Matrix Transform Technique
Discrete Scheme of 2D Case
3. Approximating an Integral Part with Lagrange Interpolation
4. Convergence and Stability Analysis
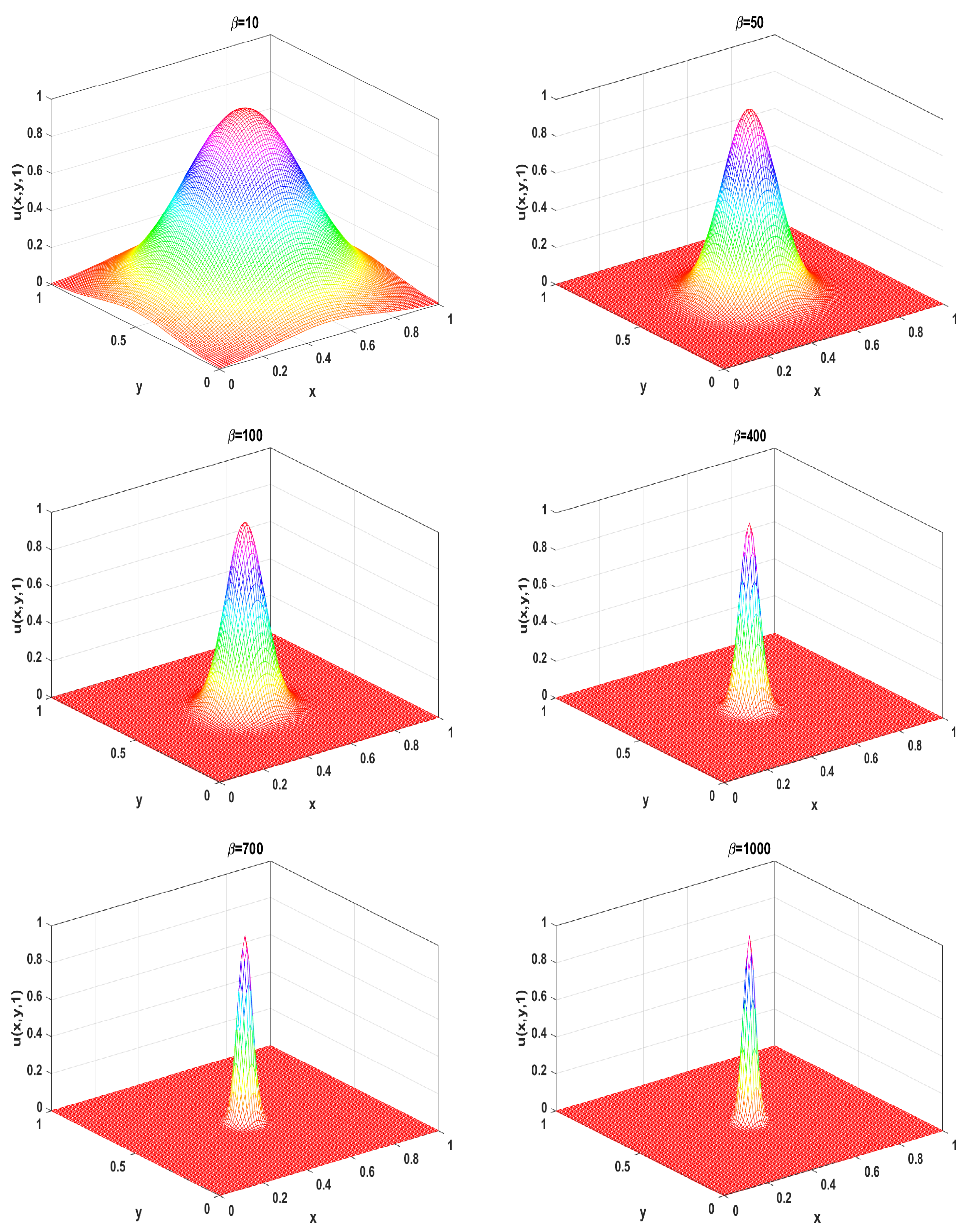
5. Numerical Validations
5.1. Example 1
5.2. Example 2
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar] [CrossRef]
- Giesekus, H. Theory of Viscoelasticity. VonR. M. Christensen. Academic Press, New York-London 1971. 1. Aufl., XI, 245 S., zahlr. Abb. geb. $ 13.50. Chem. Ing. Tech. CIT 1972, 44, 356. [Google Scholar] [CrossRef]
- Renardy, M. Mathematical Analysis of Viscoelastic Flows; SIAM: Philadelphia, PA, USA, 2000. [Google Scholar]
- Aziz, I.; Ain, Q.U. Numerical solution of partial integro-differential equations with weakly singular kernels. Adv. Math. Model. Appl. 2020, 5, 149–160. [Google Scholar]
- Qiao, L.; Xu, D.; Yan, Y. High-order ADI orthogonal spline collocation method for a new 2D fractional integro-differential problem. Math. Methods Appl. Sci. 2020, 43, 5162–5178. [Google Scholar] [CrossRef]
- Alavi, J.; Aminikhah, H. Orthogonal cubic spline basis and its applications to a partial integro-differential equation with a weakly singular kernel. Comput. Appl. Math. 2021, 40, 55. [Google Scholar] [CrossRef]
- Guo, J.; Xu, D. A Compact Difference Scheme for the Time-Fractional Partial Integro-Differential Equation with a Weakly Singular Kernel. Adv. Appl. Math. Mech. 2020, 12, 1261–1279. [Google Scholar] [CrossRef]
- Fakhar-Izadi, F. Fully spectral-Galerkin method for the one- and two-dimensional fourth-order time-fractional partial integro-differential equations with a weakly singular kernel. Numer. Methods Partial. Differ. Equ. 2020, 38, 160–176. [Google Scholar] [CrossRef]
- Sadeghi, B.; Maleki, M.; Almasieh, H. Space-time Muntz spectral collocation approach for parabolic Volterra integro-differential equations with a singular kernel. Int. J. Nonlinear Anal. Appl. 2022, 14, 153–162. [Google Scholar] [CrossRef]
- Ul Islam, S.; Ali, A.; Zafar, A.; Hussain, I. A Differential Quadrature Based Approach for Volterra Partial Integro-Differential Equation with a Weakly Singular Kernel. Comput. Model. Eng. Sci. 2020, 124, 915–935. [Google Scholar] [CrossRef]
- Chen, H.; Qiu, W.; Zaky, M.A.; Hendy, A.S. A two-grid temporal second-order scheme for the two-dimensional nonlinear Volterra integro-differential equation with weakly singular kernel. Calcolo 2023, 60, 13. [Google Scholar] [CrossRef]
- Chen, X.; Ding, D.; Lei, S.L.; Wang, W. An implicit-explicit preconditioned direct method for pricing options under regime-switching tempered fractional partial differential models. Numer. Algorithms 2021, 87, 939–965. [Google Scholar] [CrossRef]
- Chen, Y. Second-order IMEX scheme for a system of partial integro-differential equations from Asian option pricing under regime-switching jump-diffusion models. Numer. Algorithms 2022, 89, 1823–1843. [Google Scholar] [CrossRef]
- Qiu, W.; Xu, D.; Yang, X.; Zhang, H. The efficient ADI Galerkin finite element methods for the three-dimensional nonlocal evolution problem arising in viscoelastic mechanics. Discret. Contin. Dyn. Syst. B 2023, 28, 3079–3106. [Google Scholar] [CrossRef]
- Zhang, H.; Han, X.; Yang, X. Quintic B-spline collocation method for fourth order partial integro-differential equations with a weakly singular kernel. Appl. Math. Comput. 2013, 219, 6565–6575. [Google Scholar] [CrossRef]
- Santra, S.; Mohapatra, J. A novel finite difference technique with error estimate for time fractional partial integro-differential equation of Volterra type. J. Comput. Appl. Math. 2022, 400, 113746. [Google Scholar] [CrossRef]
- Sachs, E.; Strauss, A. Efficient solution of a partial integro-differential equation in finance. Appl. Numer. Math. 2008, 58, 1687–1703. [Google Scholar] [CrossRef]
- Baigereyev, D.; Omariyeva, D.; Temirbekov, N.; Yergaliyev, Y.; Boranbek, K. Numerical Method for a Filtration Model Involving a Nonlinear Partial Integro-Differential Equation. Mathematics 2022, 10, 1319. [Google Scholar] [CrossRef]
- Luo, Z.; Zhang, X.; Wang, S.; Yao, L. Numerical approximation of time fractional partial integro-differential equation based on compact finite difference scheme. Chaos Solitons Fractals 2022, 161, 112395. [Google Scholar] [CrossRef]
- Abdalla, M.; Boulaaras, S.; Akel, M. On Fourier–Bessel matrix transforms and applications. Math. Methods Appl. Sci. 2021, 44, 11293–11306. [Google Scholar] [CrossRef]
- Rani, D.; Mishra, V.; Cattani, C. Numerical inversion of Laplace transform based on Bernstein operational matrix. Math. Methods Appl. Sci. 2018, 41, 9231–9243. [Google Scholar] [CrossRef]
- Mahmoodi Darani, N. Hybrid collocation method for some classes of second-kind nonlinear weakly singular integral equations. Comput. Methods Differ. Equ. 2022, 11, 183–196. [Google Scholar] [CrossRef]
- Amin, A.Z.; Zaky, M.A.; Hendy, A.S.; Hashim, I.; Aldraiweesh, A. High-Order Multivariate Spectral Algorithms for High-Dimensional Nonlinear Weakly Singular Integral Equations with Delay. Mathematics 2022, 10, 3065. [Google Scholar] [CrossRef]
- Atta, A.; Youssri, Y. Advanced shifted first-kind Chebyshev collocation approach for solving the nonlinear time-fractional partial integro-differential equation with a weakly singular kernel. Comput. Appl. Math. 2022, 41, 381. [Google Scholar] [CrossRef]
- Li, H.; Ma, J. Piecewise Fractional Jacobi Polynomial Approximations for Volterra Integro-Differential Equations with Weakly Singular Kernels. Axioms 2022, 11, 530. [Google Scholar] [CrossRef]
- Liao, F.; Zhang, L.; Wang, T. Two energy-conserving and compact finite difference schemes for two-dimensional Schrödinger-Boussinesq equations. Numer. Algorithms 2020, 85, 1335–1363. [Google Scholar] [CrossRef]
- Vong, S.; Lyu, P.; Chen, X.; Lei, S.L. High order finite difference method for time-space fractional differential equations with Caputo and Riemann-Liouville derivatives. Numer. Algorithms 2016, 72, 195–210. [Google Scholar] [CrossRef]
- Dehghan, M.; Mohebbi, A.; Asgari, Z. Fourth-order compact solution of the nonlinear Klein-Gordon equation. Numer. Algorithms 2009, 52, 523–540. [Google Scholar] [CrossRef]
- Chen, C.M.; Liu, F.; Turner, I.; Anh, V. Numerical schemes and multivariate extrapolation of a two-dimensional anomalous sub-diffusion equation. Numer. Algorithms 2010, 54, 1–21. [Google Scholar] [CrossRef][Green Version]
- Wu, X.; Deng, W.; Barkai, E. Tempered fractional Feynman-Kac equation: Theory and examples. Phys. Rev. E 2016, 93, 032151. [Google Scholar] [CrossRef]
- Li, C.; Zeng, F. Numerical Methods for Fractional Calculus; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Caputo, M. Mean fractional-order-derivatives differential equations and filters. Ann. Dell’Universita Ferrara. 1995, 41, 73–84. [Google Scholar] [CrossRef]
- Yu, B. High-order compact finite difference method for the multi-term time fractional mixed diffusion and diffusion-wave equation. Math. Methods Appl. Sci. 2021, 44, 6526–6539. [Google Scholar] [CrossRef]
- Cui, M. Compact finite difference method for the fractional diffusion equation. J. Comput. Phys. 2009, 228, 7792–7804. [Google Scholar] [CrossRef]
- Khaksar-e Oshagh, M.; Shamsi, M. Direct pseudo-spectral method for optimal control of obstacle problem–an optimal control problem governed by elliptic variational inequality. Math. Methods Appl. Sci. 2017, 40, 4993–5004. [Google Scholar] [CrossRef]
- Marrero, J.A.; Hadj, D.A. Improving formulas for the eigenvalues of finite block-Toeplitz tridiagonal matrices. Appl. Math. Comput. 2020, 382, 125324. [Google Scholar] [CrossRef]
- Kaneko, H.; Xu, Y. Gauss-type quadratures for weakly singular integrals and their application to Fredholm integral equations of the second kind. Math. Comput. 1994, 62, 739–753. [Google Scholar] [CrossRef]
- Horn, R.A.; Johnson, C.R. Matrix Analysis; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
| h | -Order | -Order | ||
|---|---|---|---|---|
| − | − | |||
| h | -Order | -Order | ||
|---|---|---|---|---|
| − | − | |||
| h | Error | -Order | Error | -Order | ||
|---|---|---|---|---|---|---|
| − | − | |||||
| − | − | |||||
| − | − | |||||
| h | -Order | -Order | ||
|---|---|---|---|---|
| − | − | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salman, Z.I.; Tavassoli Kajani, M.; Mechee, M.S.; Allame, M. Fourth-Order Difference Scheme and a Matrix Transform Approach for Solving Fractional PDEs. Mathematics 2023, 11, 3786. https://doi.org/10.3390/math11173786
Salman ZI, Tavassoli Kajani M, Mechee MS, Allame M. Fourth-Order Difference Scheme and a Matrix Transform Approach for Solving Fractional PDEs. Mathematics. 2023; 11(17):3786. https://doi.org/10.3390/math11173786
Chicago/Turabian StyleSalman, Zahrah I., Majid Tavassoli Kajani, Mohammed Sahib Mechee, and Masoud Allame. 2023. "Fourth-Order Difference Scheme and a Matrix Transform Approach for Solving Fractional PDEs" Mathematics 11, no. 17: 3786. https://doi.org/10.3390/math11173786
APA StyleSalman, Z. I., Tavassoli Kajani, M., Mechee, M. S., & Allame, M. (2023). Fourth-Order Difference Scheme and a Matrix Transform Approach for Solving Fractional PDEs. Mathematics, 11(17), 3786. https://doi.org/10.3390/math11173786





