Neuroadaptive Asymptotic Tracking Control of Nonlinear Systems with Multiple Uncertainties
Abstract
1. Introduction
- (1)
- Without requiring definite bounds of exogenous disturbances, several novel control schemes that can reject matched uncertainties and, meanwhile, mismatched uncertainties are put forward;
- (2)
- By constructing a set of asymptotic filters, the asymptotic stability can be acquired without an “explosion of complexity”;
- (3)
- The synthesized controllers with simple control schemes can be easily applied to extensive plants without much complexity.
2. General Control Issue Formulation
3. Command Filtered Robust Adaptive Controller Design
3.1. NN Adaptation
3.2. Control Schemes Design
3.3. Main Results
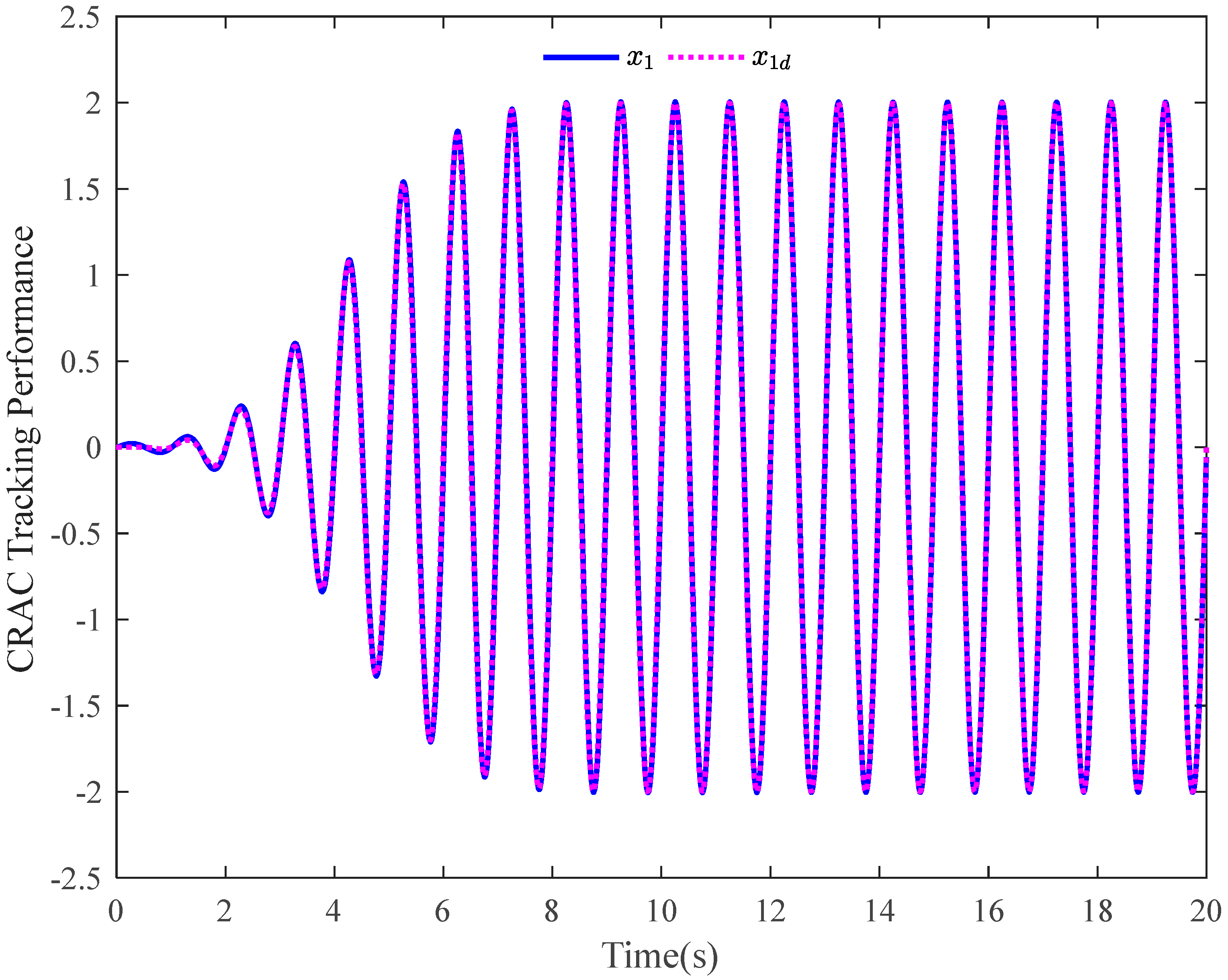
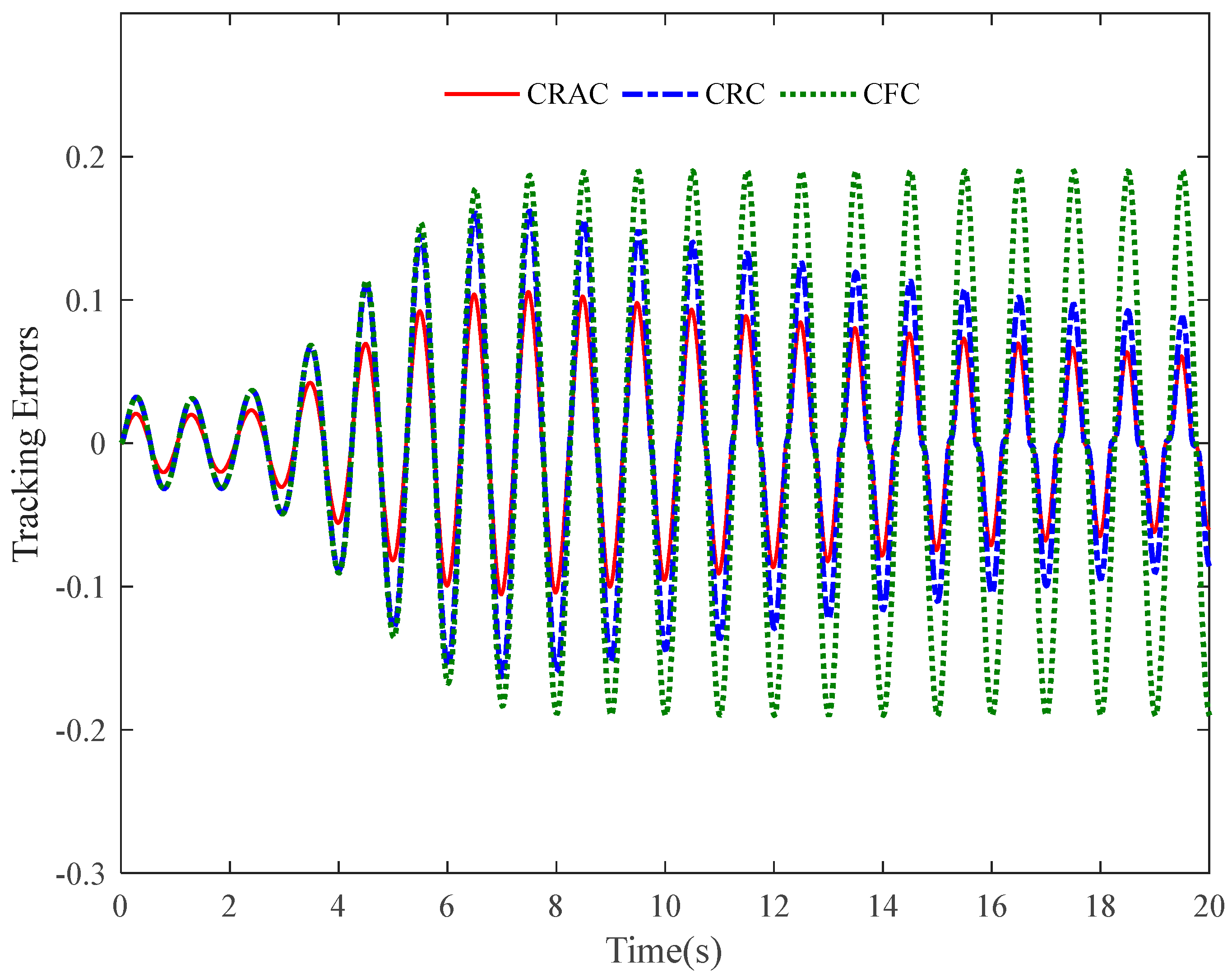
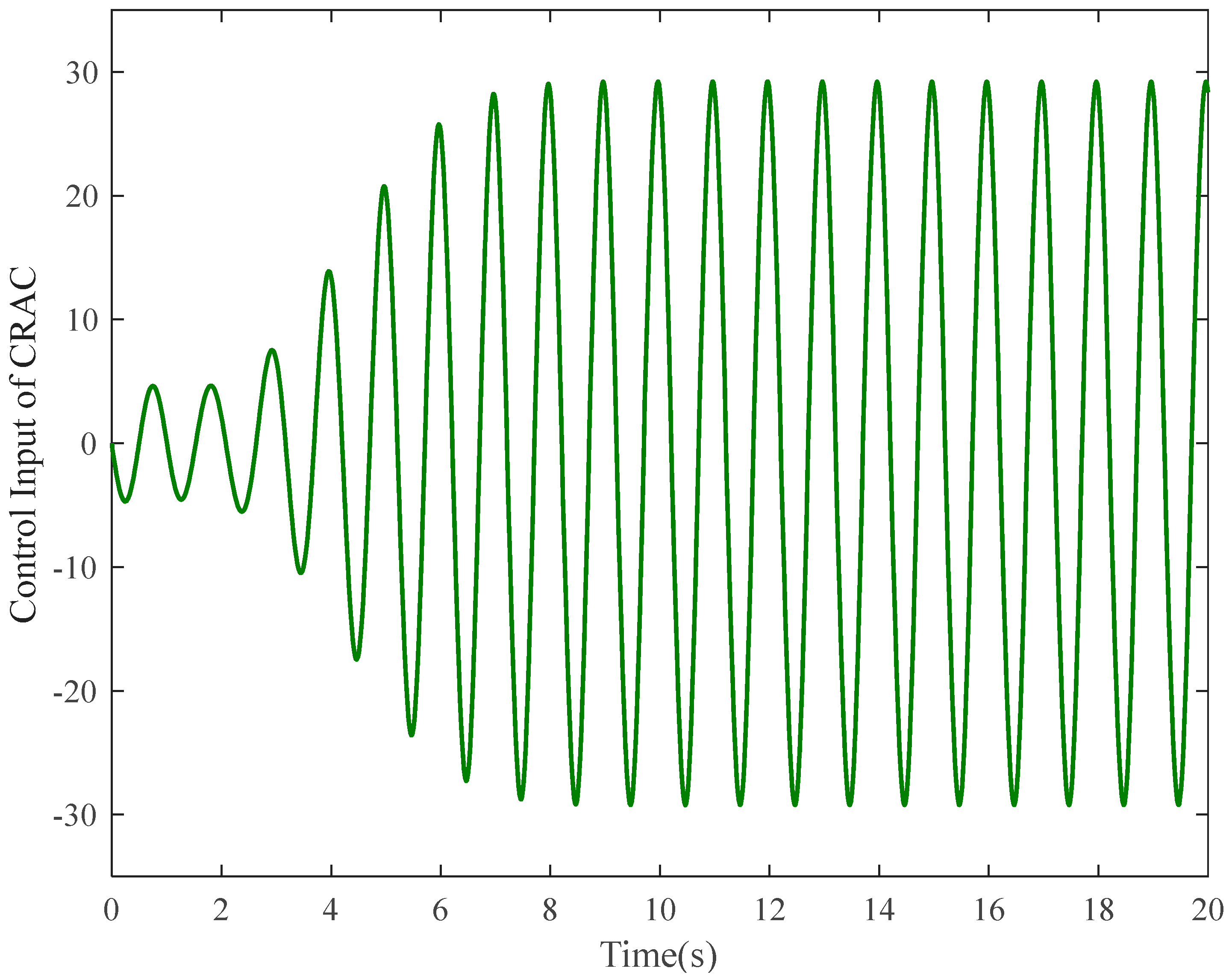
4. Verification Case
- (1)
- CRAC: This is the presented command filtered robust neuroadaptive controller with control scheme I. The control gains are adjusted as: γ1 = 100, γ2 = 100 and γ3 = 100. The adaptation gains are chosen as: κ1 = 5, κ2 = 1 and k2 = 10, k3 = 1. The design parameters of CFs are tuned as: wc1 = wc2 = 1.0 × 10−3. In addition, p1(t) = p2(t) = q2(t) = q3(t) = 100/(t2 + 0.1). The NN adaptation rate matrices are selected as Γ2 = 5.0 × 10−2I11×11 and 2.0 × 103I11×11. For , the center vector is evenly spaced in [−1, 1] × [−2, 2] and the widths for x1 and x2 are both 1. For , the center vector is evenly spaced in [−1, 1] × [−2, 2] × [−600, 600] and the widths for x1, x2 and x3 are 500, 500 and 1000, respectively.
- (2)
- CRC: This is the control scheme that is the same as CRAC but without NN compensation.
- (3)
- CFC: This is the control scheme that is same as CRAC but without NN compensation and the robust terms φ1s, φ2s, β2s and us.
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Yang, G.; Yao, J.; Ullah, N. Neuroadaptive control of saturated nonlinear systems with disturbance compensation. ISA Trans. 2022, 122, 49–62. [Google Scholar] [CrossRef] [PubMed]
- Yang, G.; Zhu, T.; Yang, F.; Cui, L.; Wang, H. Output feedback adaptive RISE control for uncertain nonlinear systems. Asian J. Control 2023, 25, 433–442. [Google Scholar] [CrossRef]
- Yao, B.; Tomizuka, M. Adaptive robust control of SISO nonlinear systems in a semi-strict feedback form. Automatica 1997, 33, 893–900. [Google Scholar] [CrossRef]
- Wang, X.S.; Su, C.Y.; Hong, H. Robust adaptive control of a class of nonlinear systems with unknown dead-zone. Automatica 2004, 40, 407–413. [Google Scholar] [CrossRef]
- Bechlioulis, C.P.; Rovithakis, G.A. Robust adaptive control of feedback linearizable MIMO nonlinear systems with prescribed performance. IEEE Trans. Autom. Control 2008, 53, 2090–2099. [Google Scholar] [CrossRef]
- Guo, L.; Cao, S. Anti-disturbance control theory for systems with multiple disturbances: A survey. ISA Trans. 2014, 53, 846–849. [Google Scholar] [CrossRef]
- Xu, Z.; Sun, C.; Hu, X.; Liu, Q.; Yao, J. Barrier Lyapunov function-based adaptive output feedback prescribed performance controller for hydraulic systems with uncertainties compensation. IEEE Trans. Ind. Electron. 2023; early access. [Google Scholar] [CrossRef]
- Chen, M.; Ge, S.S. Adaptive neural output feedback control of uncertain nonlinear systems with unknown hysteresis using disturbance observer. IEEE Trans. Ind. Electron. 2015, 62, 7706–7716. [Google Scholar] [CrossRef]
- Jiang, T.; Huang, C.; Guo, L. Control of uncertain nonlinear systems based on observers and estimators. Automatica 2015, 59, 35–47. [Google Scholar] [CrossRef]
- Chen, W.H.; Yang, J.; Guo, L.; Li, S. Disturbance-observer-based control and related methods—An overview. IEEE Trans. Ind. Electron. 2016, 63, 1083–1095. [Google Scholar] [CrossRef]
- Yang, G.; Yao, J. Nonlinear adaptive output feedback robust control of hydraulic actuators with largely unknown modeling uncertainties. Appl. Math. Model. 2020, 79, 824–842. [Google Scholar] [CrossRef]
- Tong, S.; Li, Y.; Sui, S. Adaptive fuzzy tracking control design for SISO uncertain nonstrict feedback nonlinear systems. IEEE Trans. Fuzzy Syst. 2016, 24, 1441–1454. [Google Scholar] [CrossRef]
- Zhang, Z.; Ju, H.P.; Shao, H.; Qi, Z. Exact tracking control of uncertain non-linear systems with additive disturbance. IET Control Theory Appl. 2015, 9, 736–744. [Google Scholar] [CrossRef]
- Zou, X.; Yang, G.; Hong, R.; Dai, Y. Chattering-free terminal sliding-mode tracking control for uncertain nonlinear systems with disturbance compensation. J. Vib. Control, 2023; early access. [Google Scholar] [CrossRef]
- Ying-Jeh, H.; Tzu-Chun, K.; Shin-Hung, C. Adaptive sliding-mode control for nonlinear systems with uncertain parameters. IEEE Trans. Syst. Man Cybern. Part B Cybern. 2008, 38, 534–539. [Google Scholar]
- Utkin, V.I.; Poznyak, A.S. Adaptive sliding mode control with application to super-twist algorithm: Equivalent control method. Automatica 2013, 49, 39–47. [Google Scholar] [CrossRef]
- Edwards, C.; Shtessel, Y.B. Adaptive continuous higher order sliding mode control. Automatica 2016, 65, 183–190. [Google Scholar] [CrossRef]
- Lee, J.; Chang, P.H.; Jin, M. Adaptive integral sliding mode control with time-delay estimation for robot manipulators. IEEE Trans. Ind. Electron. 2017, 64, 6796–6804. [Google Scholar] [CrossRef]
- Xian, B.; Dawson, D.M.; Queiroz, M.S.; Chen, J. A continuous asymptotic tracking control strategy for uncertain nonlinear systems. IEEE Trans. Autom. Control 2004, 49, 1206–1211. [Google Scholar] [CrossRef]
- Patre, P.M.; Mackunis, W.; Makkar, C.; Dixon, W.E. Asymptotic tracking for systems with structured and unstructured uncertainties. IEEE Trans. Control Syst. Technol. 2008, 16, 373–379. [Google Scholar] [CrossRef]
- Zhang, Z.; Xu, S.; Zhang, B. Asymptotic tracking control of uncertain nonlinear systems with unknown actuator nonlinearity. IEEE Trans. Autom. Control 2014, 59, 1336–1341. [Google Scholar] [CrossRef]
- Zhang, Z.; Xie, X.J. Asymptotic tracking control of uncertain nonlinear systems with unknown actuator nonlinearity and unknown gain signs. Int. J. Control 2014, 87, 2294–2311. [Google Scholar] [CrossRef]
- Liu, Y.H.; Li, H. Adaptive asymptotic tracking using barrier functions. Automatica 2018, 98, 239–246. [Google Scholar] [CrossRef]
- Liu, Y.H. Dynamic surface asymptotic tracking of a class of uncertain nonlinear hysteretic systems using adaptive filters. J. Frankl. Inst. 2018, 355, 123–140. [Google Scholar] [CrossRef]
- Cai, Z.; de Queiroz, M.S.; Dawson, D.M. Robust adaptive asymptotic tracking of nonlinear systems with additive disturbance. IEEE Trans. Autom. Control 2006, 51, 524–529. [Google Scholar] [CrossRef]
- Zheng, Y.; Wen, C.; Li, Z. Robust adaptive asymptotic tracking control of uncertain nonlinear systems subject to nonsmooth actuator nonlinearities. Int. J. Adapt. Control Signal Process. 2013, 27, 108–121. [Google Scholar] [CrossRef]
- Li, Y.X.; Yang, G.H. Adaptive asymptotic tracking control of uncertain nonlinear systems with input quantization and actuator faults. Automatica 2016, 72, 177–185. [Google Scholar] [CrossRef]
- Yang, G.; Yao, J.; Le, G.; Ma, D. High precise tracking control for a chain of integrator systems with modelling uncertainties. Trans. Inst. Meas. Control 2016, 39, 1710–1720. [Google Scholar] [CrossRef]
- Xu, B.; Sun, F. Composite intelligent learning control of strict-feedback systems with disturbance. IEEE Trans. Cybern. 2017, 48, 730–741. [Google Scholar] [CrossRef] [PubMed]
- Swaroop, D.; Hedrick, J.K.; Yip, P.P.; Gerdes, J.C. Dynamic surface control for a class of nonlinear systems. IEEE Trans. Autom. Control 2000, 45, 1893–1899. [Google Scholar] [CrossRef]
- Farrell, J.A.; Polycarpou, M.; Sharma, M.; Dong, W. Command fltered backstepping. IEEE Trans. Autom. Control 2009, 54, 1391–1395. [Google Scholar] [CrossRef]
- Yang, G.; Wang, H.; Chen, J.; Zhang, H. Command filtered robust control of nonlinear systems with full-state time-varying constraints and disturbances rejection. Nonlinear Dyn. 2020, 101, 2325–2342. [Google Scholar] [CrossRef]
- Liu, Y.H. Adaptive dynamic surface asymptotic tracking for a class of uncertain nonlinear systems. Int. J. Robust Nonlinear Control 2018, 28, 1233–1245. [Google Scholar] [CrossRef]
- Yang, G.; Yao, J.; Dong, Z. Neuroadaptive learning algorithm for constrained nonlinear systems with disturbance rejection. Int. J. Robust Nonlinear Control 2022, 32, 6127–6147. [Google Scholar] [CrossRef]
- Yang, G.; Wang, H.; Chen, J. Disturbance compensation based asymptotic tracking control for nonlinear systems with mismatched modeling uncertainties. Int. J. Robust Nonlinear Control 2021, 31, 2993–3010. [Google Scholar] [CrossRef]
- Wang, L.; Sun, W.; Su, S.F.; Wu, Y. Adaptive prescribed performance asymptotic tracking control for nonlinear systems with time-varying parameters. Int. J. Robust Nonlinear Control 2022, 32, 4535–4552. [Google Scholar] [CrossRef]
- Yan, B.; Niu, B.; Zhao, X.; Wang, H.; Chen, W.; Liu, X. Neural-network-based adaptive event-triggered asymptotically consensus tracking control for nonlinear nonstrict-feedback MASs: An improved dynamic surface approach. IEEE Trans. Neural Netw. Learn. Syst. 2022; early access. [Google Scholar] [CrossRef]
- Liu, Y.H.; Chen, L.L.; Zhou, Q.; Su, C.Y. Asymptotic output tracking control with prescribed transient performance of nonlinear systems in the presence of unknown dynamics. Int. J. Robust Nonlinear Control 2022, 32, 9363–9379. [Google Scholar] [CrossRef]
- Song, S.; Zhang, B.; Song, X.; Zhang, Z. Neuro-fuzzy-based adaptive dynamic surface control for fractional-order nonlinear strict-feedback systems with input constraint. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 3575–3586. [Google Scholar] [CrossRef]
- Yang, G. Asymptotic tracking with novel integral robust schemes for mismatched uncertain nonlinear systems. Int. J. Robust Nonlinear Control 2023, 33, 1988–2002. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, G. Neuroadaptive Asymptotic Tracking Control of Nonlinear Systems with Multiple Uncertainties. Mathematics 2023, 11, 2978. https://doi.org/10.3390/math11132978
Yang G. Neuroadaptive Asymptotic Tracking Control of Nonlinear Systems with Multiple Uncertainties. Mathematics. 2023; 11(13):2978. https://doi.org/10.3390/math11132978
Chicago/Turabian StyleYang, Guichao. 2023. "Neuroadaptive Asymptotic Tracking Control of Nonlinear Systems with Multiple Uncertainties" Mathematics 11, no. 13: 2978. https://doi.org/10.3390/math11132978
APA StyleYang, G. (2023). Neuroadaptive Asymptotic Tracking Control of Nonlinear Systems with Multiple Uncertainties. Mathematics, 11(13), 2978. https://doi.org/10.3390/math11132978





