Lump-Type Solutions, Lump Solutions, and Mixed Rogue Waves for Coupled Nonlinear Generalized Zakharov Equations
Abstract
1. Introduction
2. Lump Solution
3. Mixed Solutions of Soliton and Lump Waves
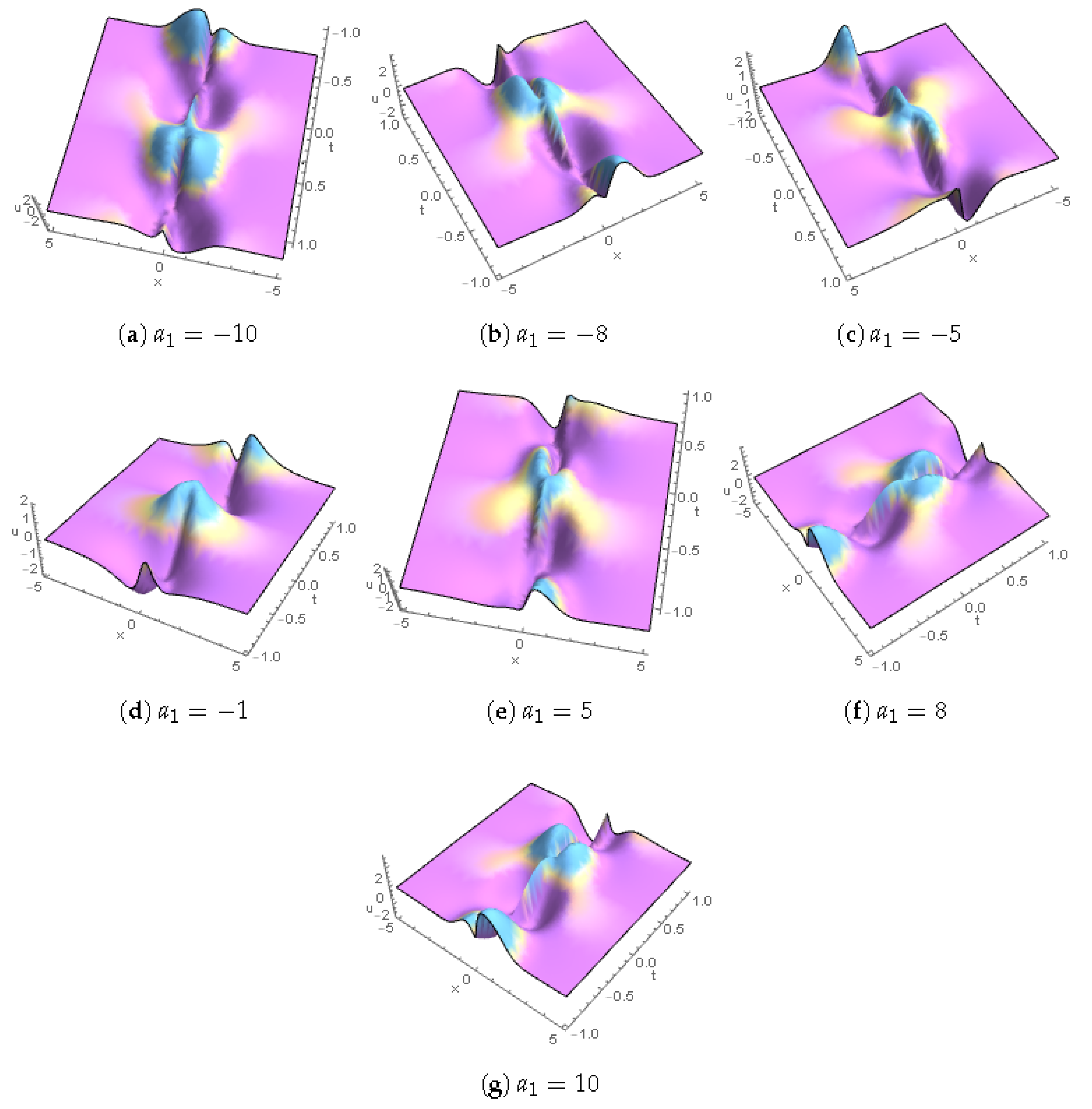
3.1. Lump One-Strip Soliton Interaction Solution
3.2. Lump Double-Strip Soliton Interaction Solution
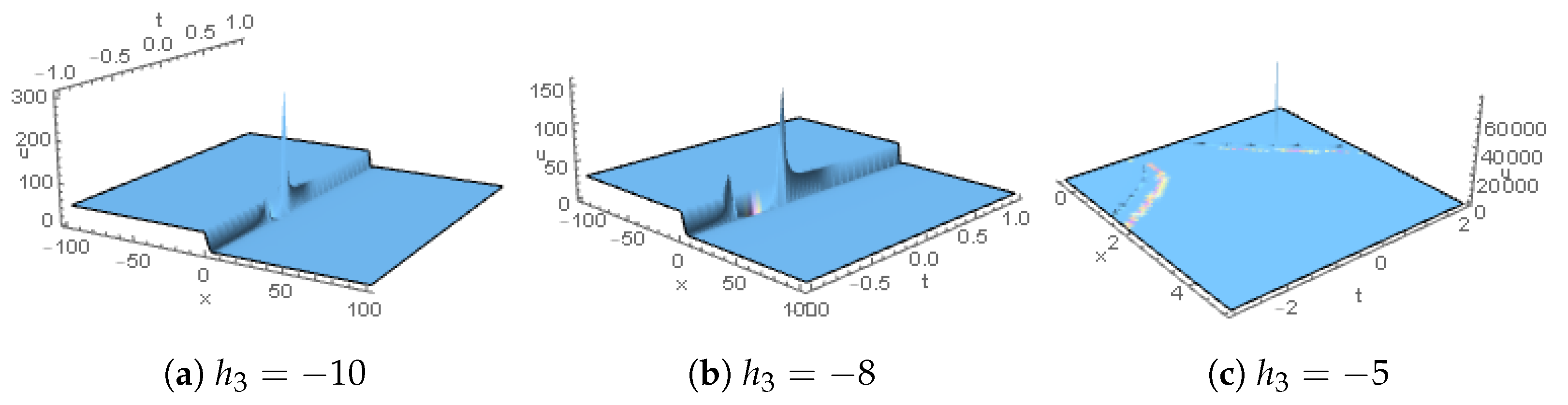
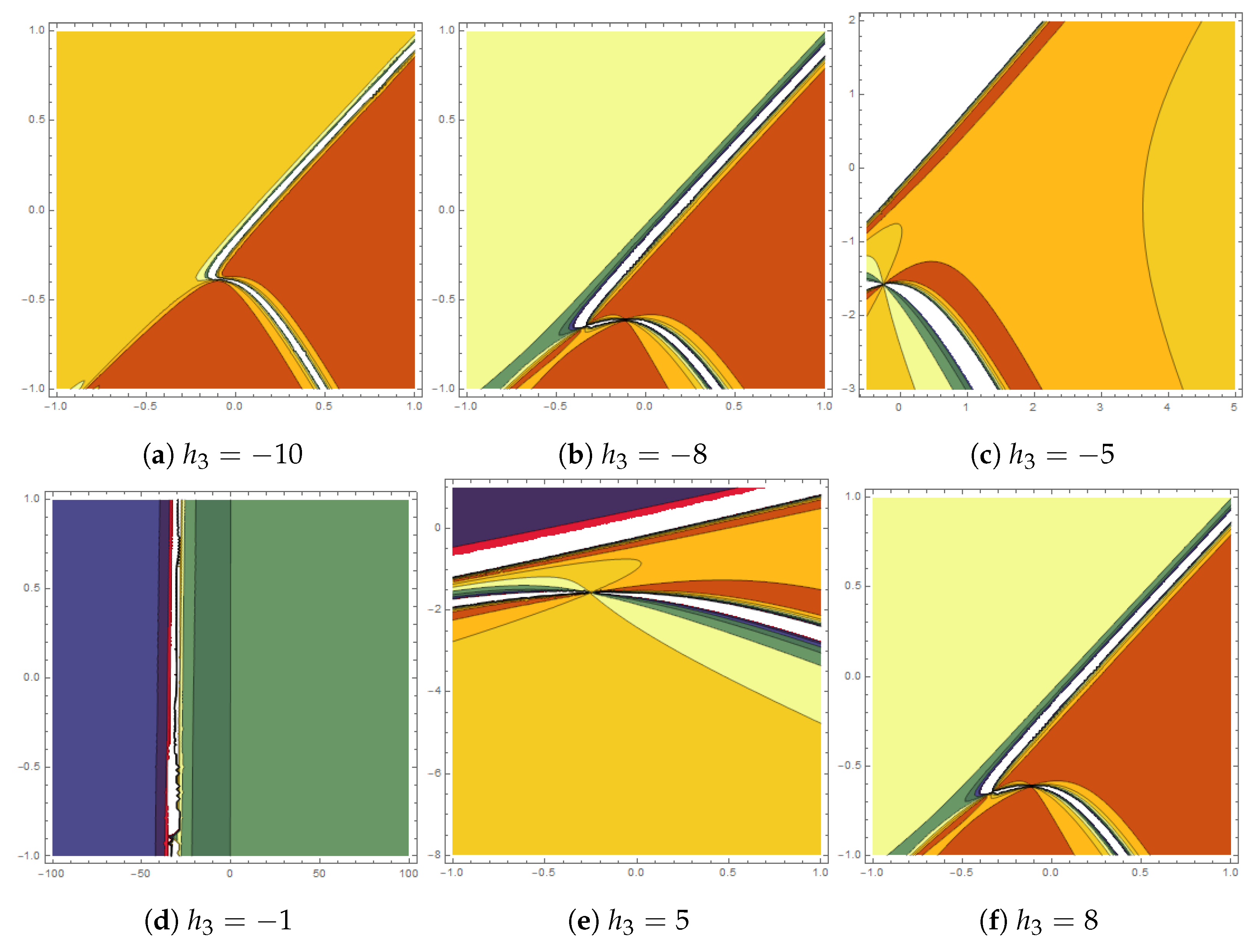
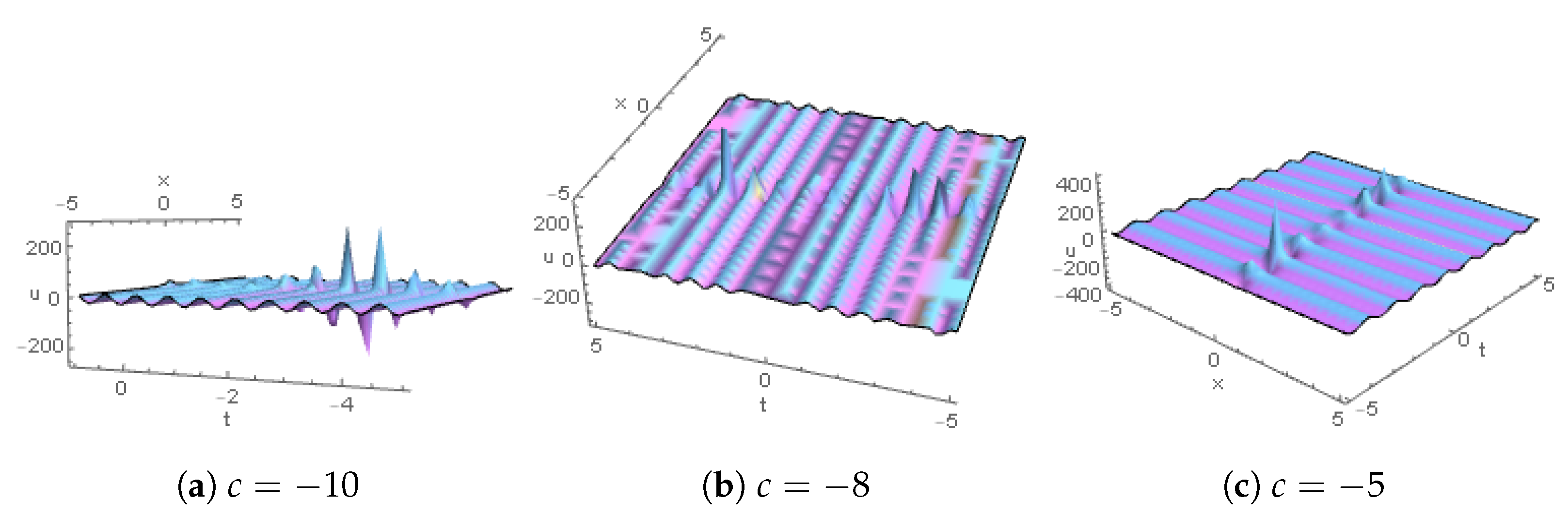
4. Lump Periodic Soliton Solution
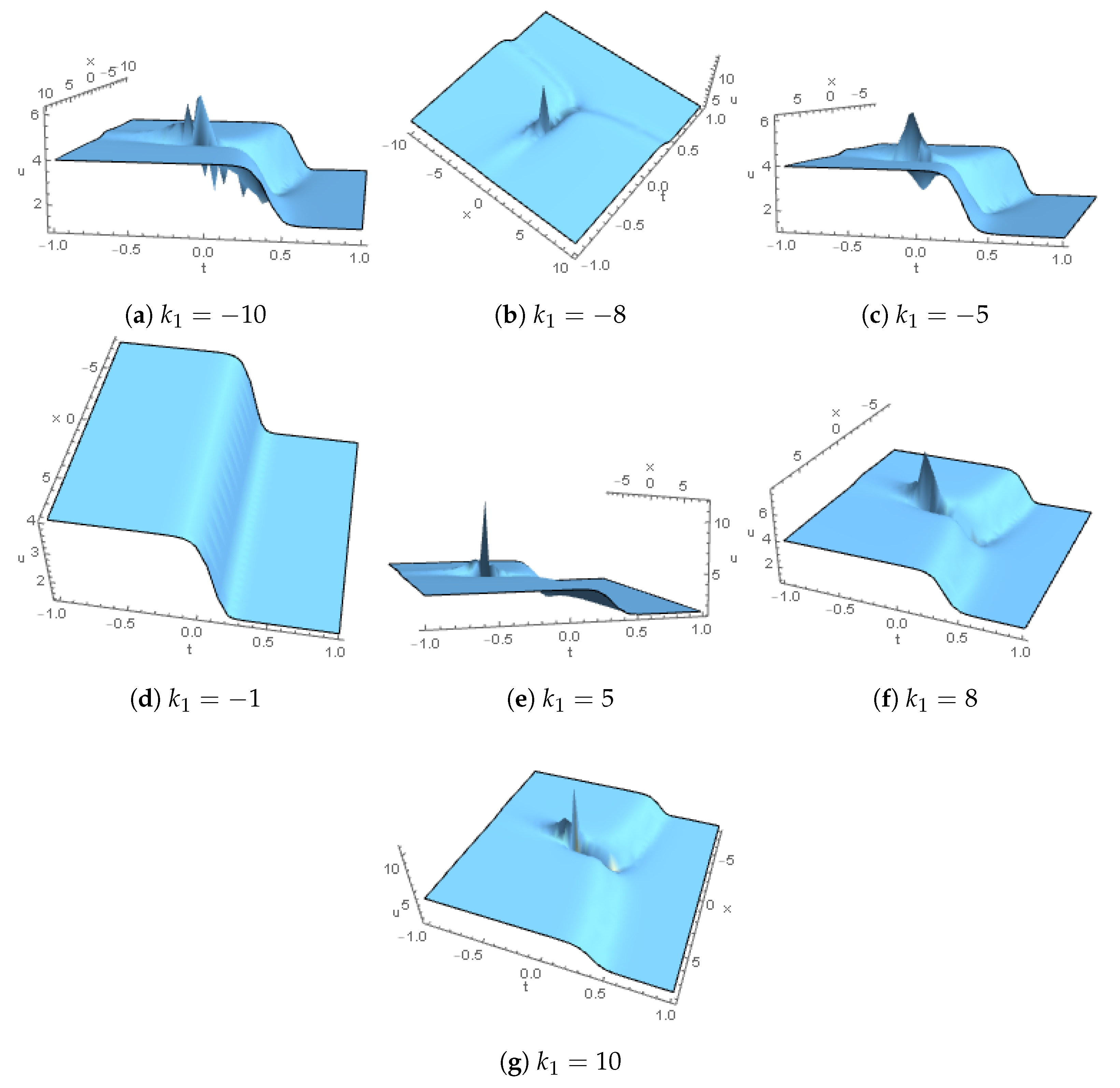
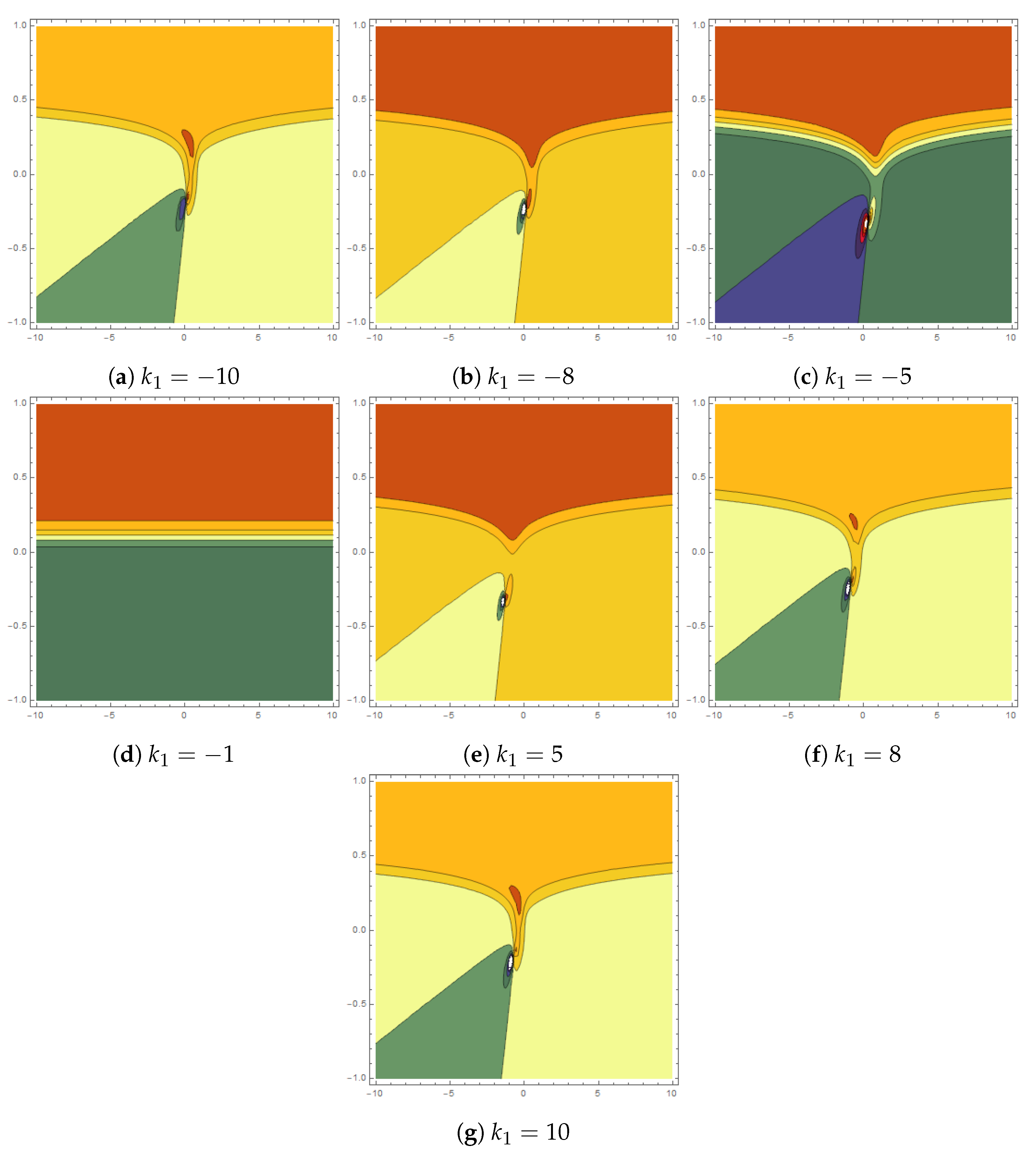
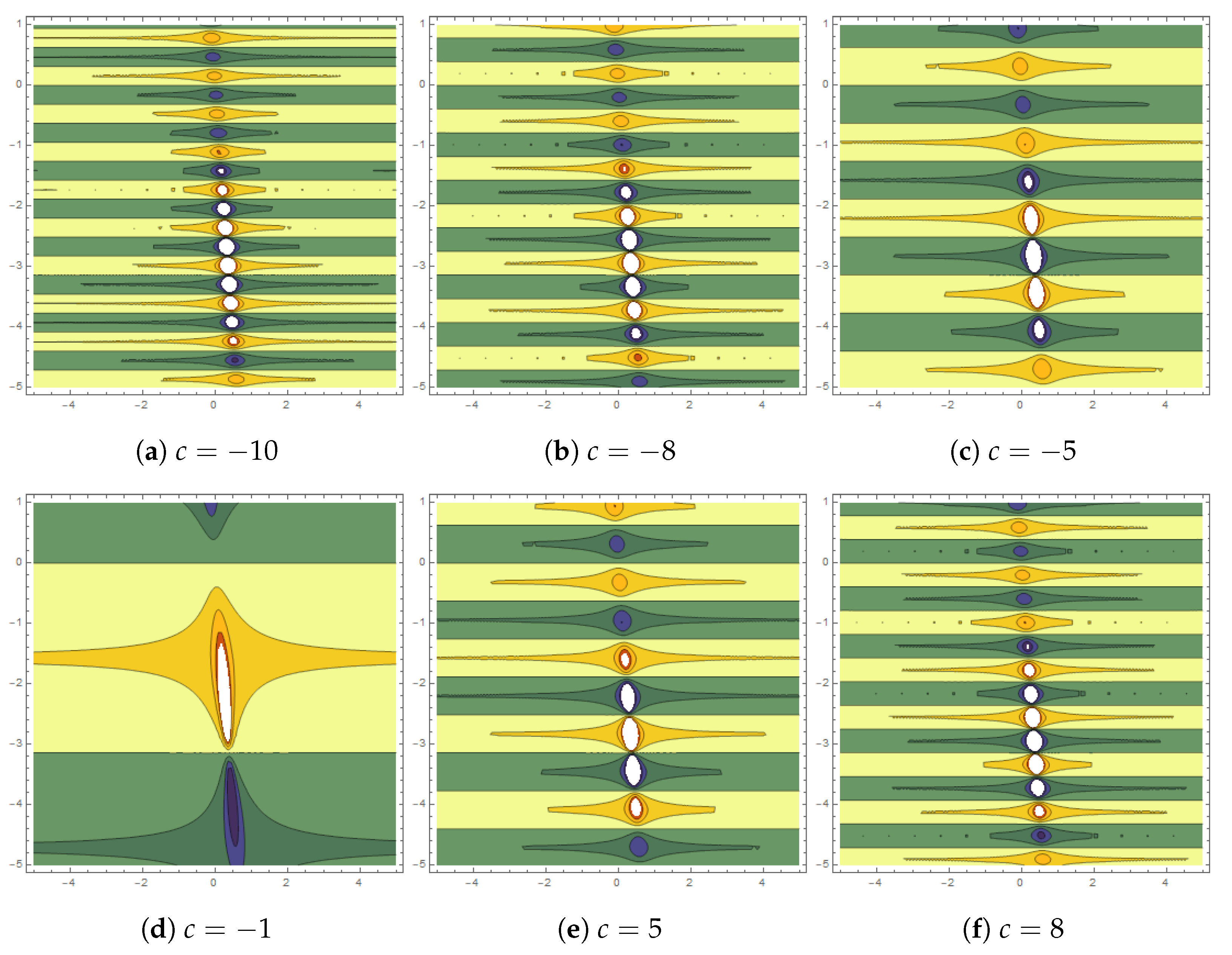
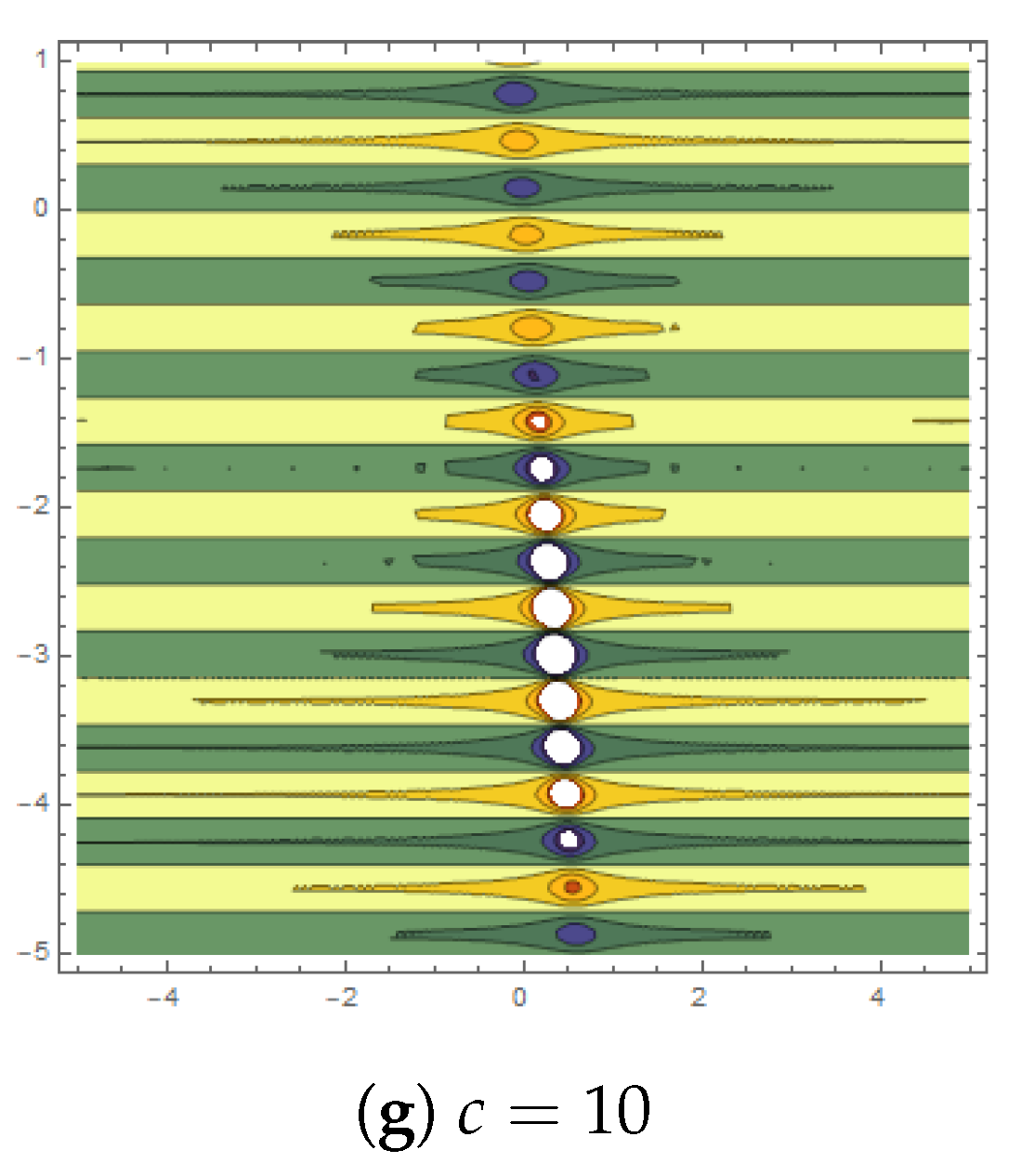
5. Rogue-Wave Solutions
6. Results and Discussion
7. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahmed, S.; Seadawy, A.R.; Rizvi, S.T. Study of breathers, rogue waves and lump solutions for the nonlinear chains of atoms. Opt. Quantum Electron. 2022, 54, 320. [Google Scholar] [CrossRef]
- Ding, Q.; Wong, P.J. A higher order numerical scheme for solving fractional Bagley-Torvik equation. Math. Methods Appl. Sci. 2022, 45, 1241–1258. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Rizvi, S.T.; Ahmed, S. Weierstrass and Jacobi elliptic, bell and kink type, lumps, Ma and Kuznetsov breathers with rogue wave solutions to the dissipative nonlinear Schrödinger equation. Chaos Solitons Fractals 2022, 160, 112258. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Ahmed, S.; Rizvi, S.T.; Ali, K. Various forms of lumps and interaction solutions to generalized Vakhnenko Parkes equation arising from high-frequency wave propagation in electromagnetic physics. J. Geom. Phys. 2022, 176, 104507. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Ahmed, S.; Rizvi, S.T.; Ali, K. Lumps, breathers, interactions and rogue wave solutions for a stochastic gene evolution in double chain deoxyribonucleic acid system. Chaos Solitons Fractals 2022, 161, 112307. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Rizvi, S.T.; Ahmed, S. Multiple lump, generalized breathers, Akhmediev breather, manifold periodic and rogue wave solutions for generalized Fitzhugh-Nagumo equation: Applications in nuclear reactor theory. Chaos Solitons Fractals 2022, 161, 112326. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Rizvi, S.T.; Ahmed, S.; Younas, M. Applications of lump and interaction soliton solutions to the Model of liquid crystals and nerve fibers. Encycl. Complex. Syst. Sci. 2022, 1–20. [Google Scholar]
- Bashir, A.; Seadawy, A.R.; Ahmed, S.; Rizvi, S.T. The Weierstrass and Jacobi elliptic solutions along with multiwave, homoclinic breather, kink-periodic-cross rational and other solitary wave solutions to Fornberg Whitham equation. Chaos Solitons Fractals 2022, 163, 112538. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Rizvi, S.T.R.; Ahmad, S.; Younis, M.; Baleanu, D. Lump, lump-one stripe, multiwave and breather solutions for the Hunter–Saxton equation. Open Phys. 2021, 19, 1–10. [Google Scholar] [CrossRef]
- Li, X.; Wong, P.J. Generalized Alikhanov’s approximation and numerical treatment of generalized fractional sub-diffusion equations. Commun. Nonlinear Sci. Numer. Simul. 2021, 97, 105719. [Google Scholar] [CrossRef]
- Ahmad, H.; Seadawy, A.R.; Khan, T.A. Numerical Solution of Korteweg-de Vries-Burgers Equation by the Modified Variational Iteration Algorithm-II arising in shallow water waves. Phys. Scr. 2020, 95, 45210. [Google Scholar] [CrossRef]
- Li, X.; Wong, P.J. gL1 Scheme for Solving a Class of Generalized Time-Fractional Diffusion Equations. Mathematics 2022, 10, 1219. [Google Scholar] [CrossRef]
- Soundararajan, R.; Subburayan, V.; Wong, P.J. Streamline Diffusion Finite Element Method for Singularly Perturbed 1D-Parabolic Convection Diffusion Differential Equations with Line Discontinuous Source. Mathematics 2023, 11, 2034. [Google Scholar] [CrossRef]
- Liu, Y.; Li, B.; Wazwaz, A.M. Novel high-order breathers and rogue waves in the Boussinesq equation via determinants. Int. J. Mod. Phys. 2020, 43, 3701–3715. [Google Scholar] [CrossRef]
- Younas, U.; Seadawy, A.R.; Younis, M.; Rizvi, S.T.R. Optical solitons and closed form solutions to (3+1)-dimensional resonant Schrodinger equation. Int. J. Mod. Phys. 2020, 34, 2050291. [Google Scholar] [CrossRef]
- Ghaffar, A.; Ali, A.; Ahmed, S.; Akram, S.; Baleanu, D.; Nisar, K.S. A novel analytical technique to obtain the solitary solutions for nonlinear evolution equation of fractional order. Adv. Differ. Equ. 2020, 1, 308. [Google Scholar] [CrossRef]
- Wang, M.; Li, X. Extended F-expansion method and periodic wave solutions for the generalized Zakharov equations. Phys. Lett. A 2005, 343, 48–54. [Google Scholar] [CrossRef]
- Jin, S.; Markowich, P.A.; Zheng, C. Numerical simulation of a generalized Zakharov system. J. Comput. Phys. 2004, 201, 376–395. [Google Scholar] [CrossRef]
- Bao, W.; Sun, F.; Wei, G.W. Numerical methods for the generalized Zakharov system. J. Comput. Phys. 2003, 190, 201–228. [Google Scholar] [CrossRef]
- Bhrawy, A.H. An efficient Jacobi pseudospectral approximation for nonlinear complex generalized Zakharov system. Appl. Math. Comput. 2014, 247, 30–46. [Google Scholar] [CrossRef]
- Zhang, J. Variational approach to solitary wave solution of the generalized Zakharov equation. Comput. Math. Appl. 2007, 54, 1043–1046. [Google Scholar] [CrossRef]
- Khan, Y.; Faraz, N.; Yildirim, A. New soliton solutions of the generalized Zakharov equations using He’s variational approach. Appl. Math. Lett. 2011, 24, 965–968. [Google Scholar] [CrossRef]
- Li, Y.Z.; Li, K.M.; Lin, C. Exp-function method for solving the generalized-Zakharov equations. Appl. Math. Comput. 2008, 205, 197–201. [Google Scholar] [CrossRef]
- Buhe, E.; Bluman, G.W. Symmetry reductions, exact solutions, and conservation laws of the generalized Zakharov equations. J. Math. Phys. 2015, 56, 101501. [Google Scholar] [CrossRef]
- Wu, Y. Variational approach to the generalized Zakharov equations. Int. J. Nonlinear Sci. Numer. Simul. 2009, 10, 1245–1248. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Rizvi, S.T.; Ashraf, M.A.; Younis, M.; Hanif, M. Rational solutions and their interactions with kink and periodic waves for a nonlinear dynamical phenomenon. Int. J. Mod. Phys. B 2021, 35, 2150236. [Google Scholar] [CrossRef]
- Wang, H. Lump and interaction solutions to the (2+1)-dimensional Burgers equation. Appl. Math. Lett. 2018, 85, 27–34. [Google Scholar] [CrossRef]
- Zhou, Y.; Manukure, S.; Ma, W.X. Lump and lump-soliton solutions to the Hirota Satsuma equation. Commun. Nonlinear Sci. Numer. Simul. 2019, 68, 56–62. [Google Scholar] [CrossRef]
- Wu, P.; Zhang, Y.; Muhammad, I.; Yin, Q. Lump, periodic lump and interaction lump stripe solutions to the (2+1)-dimensional B-type Kadomtsev–Petviashvili equation. Mod. Phys. Lett. B 2018, 32, 1850106. [Google Scholar] [CrossRef]
- Li, B.Q.; Ma, Y.L. multiple-lump waves for a (3+1)-dimensional Boiti–Leon–Manna–Pempinelli equation arising from incompressible fluid. Comput. Math. Appl. 2018, 76, 204–214. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Seadawy, A.R.; Rizvi, S.T.R.; Zahed, H. Lump-Type Solutions, Lump Solutions, and Mixed Rogue Waves for Coupled Nonlinear Generalized Zakharov Equations. Mathematics 2023, 11, 2856. https://doi.org/10.3390/math11132856
Seadawy AR, Rizvi STR, Zahed H. Lump-Type Solutions, Lump Solutions, and Mixed Rogue Waves for Coupled Nonlinear Generalized Zakharov Equations. Mathematics. 2023; 11(13):2856. https://doi.org/10.3390/math11132856
Chicago/Turabian StyleSeadawy, Aly R., Syed T. R. Rizvi, and Hanadi Zahed. 2023. "Lump-Type Solutions, Lump Solutions, and Mixed Rogue Waves for Coupled Nonlinear Generalized Zakharov Equations" Mathematics 11, no. 13: 2856. https://doi.org/10.3390/math11132856
APA StyleSeadawy, A. R., Rizvi, S. T. R., & Zahed, H. (2023). Lump-Type Solutions, Lump Solutions, and Mixed Rogue Waves for Coupled Nonlinear Generalized Zakharov Equations. Mathematics, 11(13), 2856. https://doi.org/10.3390/math11132856






