Aspects of Quantum Statistical Mechanics: Fractional and Tsallis Approaches
Abstract
1. Introduction
2. Generalized Statistical Mechanics
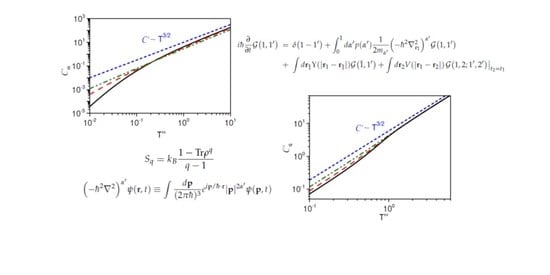
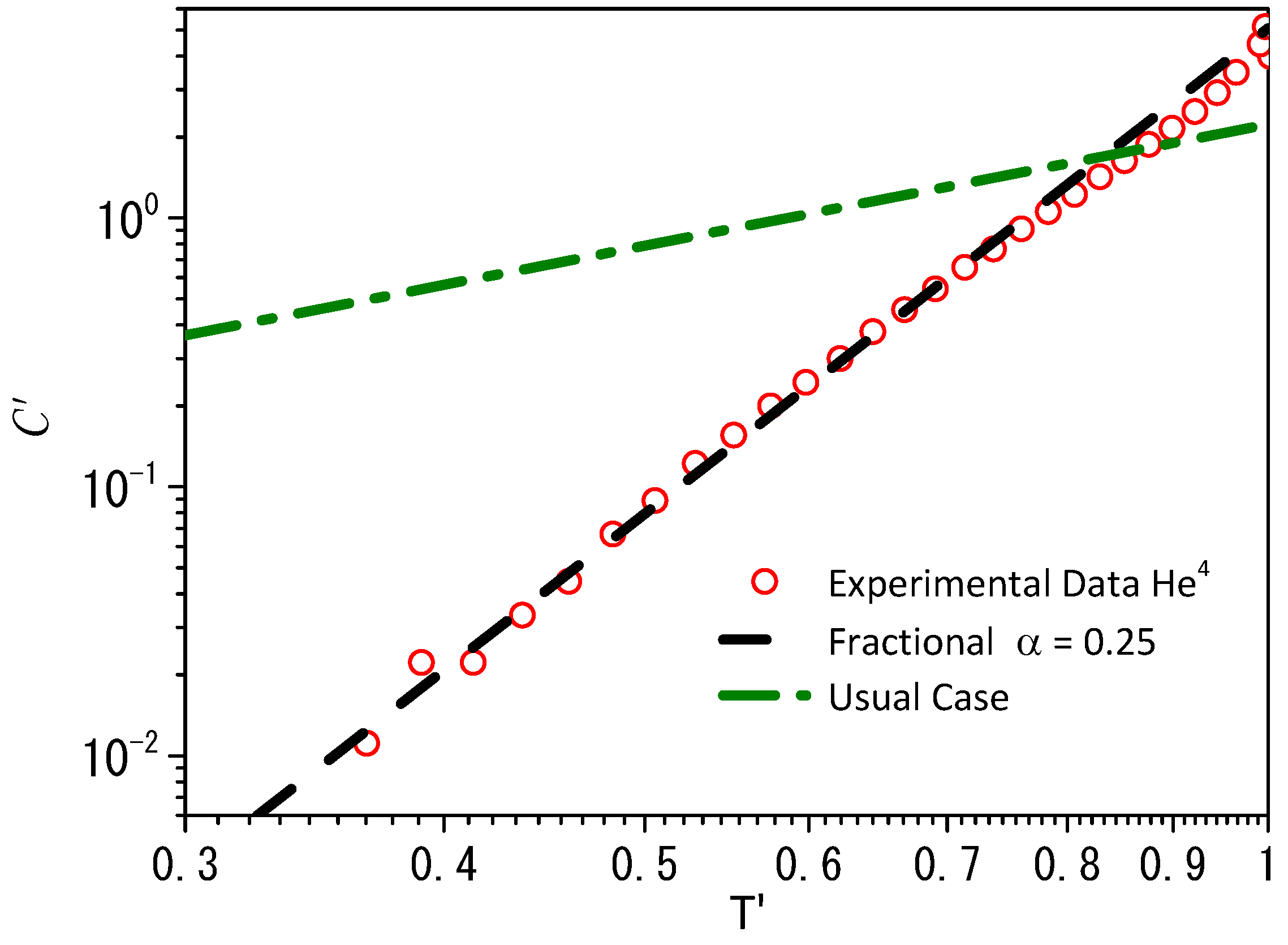
2.1. Fractional Dynamics and Statistical Mechanics
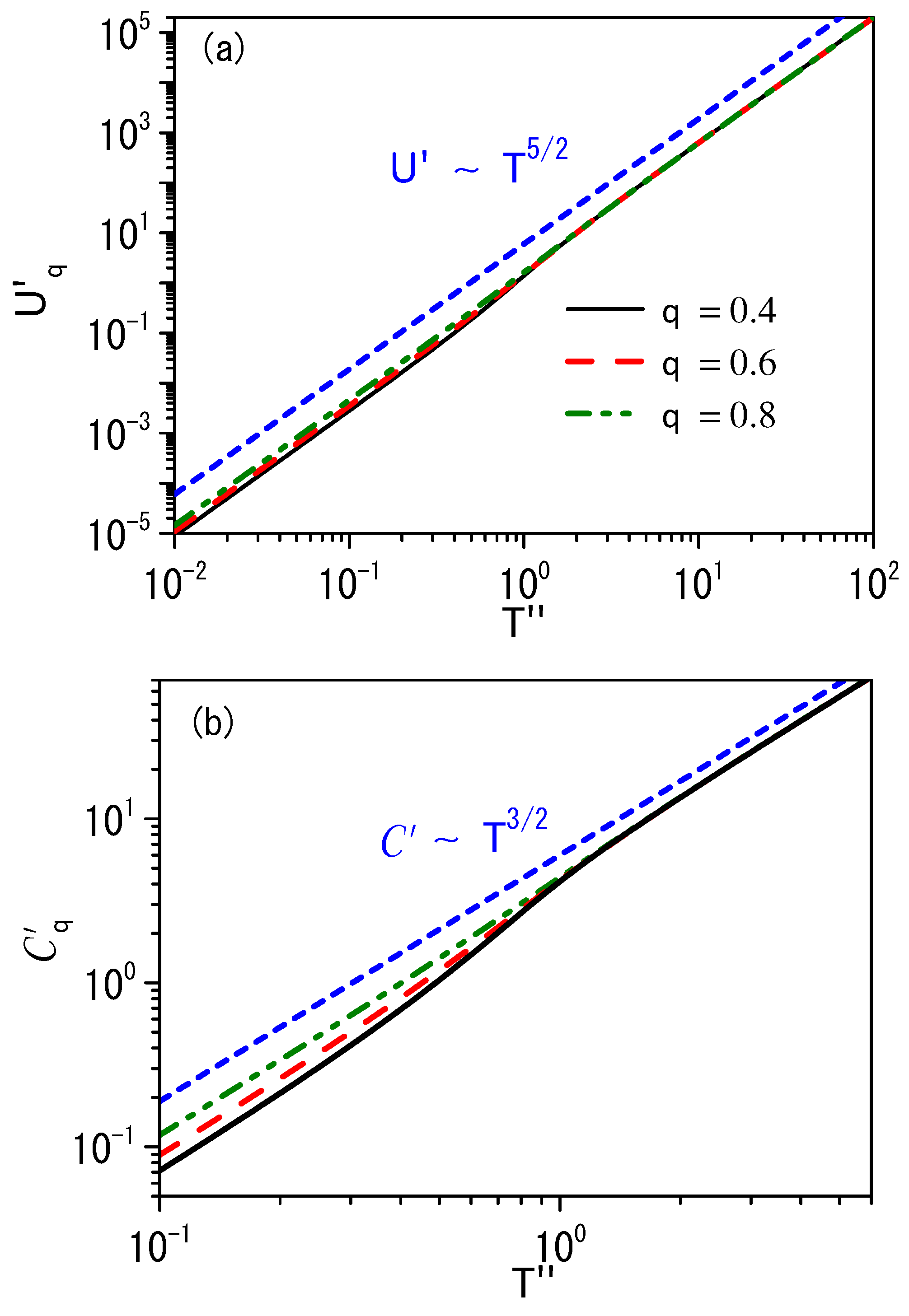
2.2. Tsallis Approach and Thermal Green Functions
2.3. Fractional-Generalized Entropy and Thermal Green Functions
3. Discussions and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Uffink, J. Boltzmann’s Work in Statistical Physics. In The Stanford Encyclopedia of Philosophy, Summer 2022 ed.; Zalta, E.N., Ed.; Metaphysics Research Lab, Stanford University: Stanford, CA, USA, 2022. [Google Scholar]
- Goldstein, S. Boltzmann’s approach to statistical mechanics. In Chance in Physics: Foundations and Perspectives; Springer: Berlin/Heidelberg, Germany, 2001; pp. 39–54. [Google Scholar]
- Klein, M.J. The development of Boltzmann’s statistical ideas. In Proceedings of the The Boltzmann Equation: Theory and Applications; Springer: Berlin/Heidelberg, Germany, 1973; pp. 53–106. [Google Scholar]
- Lebowitz, J.L. Statistical mechanics: A selective review of two central issues. Rev. Mod Phys. 1999, 71, S346. [Google Scholar] [CrossRef]
- Pathria, R.K. Statistical Mechanics; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Huang, K. Statistical Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Ourabah, K. Quasiequilibrium self-gravitating systems. Phys. Rev. D 2020, 102, 043017. [Google Scholar] [CrossRef]
- Jung, S.; Swinney, H.L. Velocity difference statistics in turbulence. Phys. Rev. E 2005, 72, 026304. [Google Scholar] [CrossRef] [PubMed]
- Defenu, N.; Donner, T.; Macrí, T.; Pagano, G.; Ruffo, S.; Trombettoni, A. Long-range interacting quantum systems. arXiv 2021, arXiv:2109.01063. [Google Scholar]
- Xiong, H.N.; Lo, P.Y.; Zhang, W.M.; Nori, F.; Feng, D.H. Non-Markovian dynamics impact on the foundations of statistical mechanics. arXiv 2013, arXiv:1311.1282. [Google Scholar]
- Speck, T.; Seifert, U. The Jarzynski relation, fluctuation theorems, and stochastic thermodynamics for non-Markovian processes. J. Stat. Mech: Theory Exp. 2007, 2007, L09002. [Google Scholar] [CrossRef]
- Pękalski, A.; Sznajd-Weron, K. Anomalous Diffusion: From Basics to Applications; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Klages, R. Microscopic Chaos, Fractals and Transport in Nonequilibrium Statistical Mechanics; World Scientific: Singapore, 2007. [Google Scholar]
- Tsallis, C.; Mendes, R.S.; Plastino, A.R. The role of constraints within generalized nonextensive statistics. Phys. A 1998, 261, 534–554. [Google Scholar] [CrossRef]
- Abe, S.; Okamoto, Y. Nonextensive Statistical Mechanics and Its Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2001; Volume 560. [Google Scholar]
- Lenzi, E.K.; Mendes, R.S.; da Silva, L.R. Statistical mechanics based on Renyi entropy. Phys. A 2000, 280, 337–345. [Google Scholar] [CrossRef]
- Glushak, P.A.; Markiv, B.B.; Tokarchuk, M.V. Zubarev’s nonequilibrium statistical operator method in the generalized statistics of multiparticle systems. Theor. Math. Phys. 2018, 194, 57–73. [Google Scholar] [CrossRef]
- Kaniadakis, G.; Lissia, M.; Scarfone, A.M. Two-parameter deformations of logarithm, exponential, and entropy: A consistent framework for generalized statistical mechanics. Phys. Rev. E 2005, 71, 046128. [Google Scholar] [CrossRef]
- Kaniadakis, G. Maximum entropy principle and power-law tailed distributions. Eur. Phys. J. B 2009, 70, 3–13. [Google Scholar] [CrossRef]
- Curado, E.M.F.; Cedeño, C.E.; Soares, I.D.; Tsallis, C. Relativistic gas: Lorentz-invariant distribution for the velocities. Chaos 2022, 32, 103110. [Google Scholar] [CrossRef]
- Megías, E.; Timóteo, V.S.; Gammal, A.; Deppman, A. Bose–Einstein condensation and non-extensive statistics for finite systems. Phys. A 2022, 585, 126440. [Google Scholar] [CrossRef]
- Rovenchak, A.; Krynytskyi, Y. Ideal Bose gas in steep one-dimensional traps. Low Temp. Phys. 2022, 48, 20–25. [Google Scholar] [CrossRef]
- Sigalotti, L.D.G.; Ramírez-Rojas, A.; Vargas, C.A. Tsallis q-Statistics in Seismology. Entropy 2023, 25, 408. [Google Scholar] [CrossRef]
- Wilk, G.; Włodarczyk, Z. Some Non-Obvious Consequences of Non-Extensiveness of Entropy. Entropy 2023, 25, 474. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Evangelista, L.R.; Lenzi, E.K. Fractional Diffusion Equations and Anomalous Diffusion; Cambridge University Press: Cambridge, UK, 2018. [Google Scholar]
- Evangelista, L.R.; Lenzi, E.K. An Introduction to Anomalous Diffusion and Relaxation; Springer Nature: Berlin/Heidelberg, Germany, 2023. [Google Scholar]
- Uchaikin, V.V.; Sibatov, R. Fractional Kinetics in Solids: Anomalous Charge Transport in Semiconductors, Dielectrics, and Nanosystems; World Scientific: Singapore, 2013. [Google Scholar]
- Zaslavsky, G.M. Chaos, fractional kinetics, and anomalous transport. Phys. Rep. 2002, 371, 461–580. [Google Scholar] [CrossRef]
- Lenzi, E.K.; Ribeiro, H.V.; Lenzi, M.K.; Evangelista, L.R.; Magin, R.L. Fractional diffusion with geometric constraints: Application to signal decay in magnetic resonance imaging (MRI). Mathematics 2022, 10, 389. [Google Scholar] [CrossRef]
- Magin, R.L.; Karaman, M.M.; Hall, M.G.; Zhu, W.; Zhou, X.J. Capturing complexity of the diffusion-weighted MR signal decay. Magn. Reson. Imaging 2019, 56, 110–118. [Google Scholar] [CrossRef] [PubMed]
- Magin, R.L. Models of diffusion signal decay in magnetic resonance imaging: Capturing complexity. Concept. Magn. Reson. A 2016, 45, e21401. [Google Scholar] [CrossRef]
- Magin, R.L.; Hall, M.G.; Karaman, M.M.; Vegh, V. Fractional calculus models of magnetic resonance phenomena: Relaxation and diffusion. Crit. Rev. Biomed. Eng. 2020, 48, 285–326. [Google Scholar] [CrossRef]
- Magin, R.L.; Karani, H.; Wang, S.; Liang, Y. Fractional order complexity model of the diffusion signal decay in MRI. Mathematics 2019, 7, 348. [Google Scholar] [CrossRef]
- Barbero, G.; Evangelista, L.R.; Lenzi, E.K. Time-fractional approach to the electrochemical impedance: The Displacement current. J. Electroanal. Chem. 2022, 920, 116588. [Google Scholar] [CrossRef]
- Bisquert, J.; Compte, A. Theory of the electrochemical impedance of anomalous diffusion. J. Electroanal. Chem. 2001, 499, 112–120. [Google Scholar] [CrossRef]
- Meilanov, R.P.; Magomedov, R.A. Thermodynamics in fractional calculus. J. Eng. Phys. Thermophy. 2014, 87, 1521–1531. [Google Scholar] [CrossRef]
- Magomedov, R.A.; Meilanov, R.R.; Meilanov, R.P.; Akhmedov, E.N.; Beybalaev, V.D.; Aliverdiev, A.A. To the fractal equation of state. Proc. J. Phys. Conf. Ser. 2017, 891, 012331. [Google Scholar] [CrossRef]
- Magomedov, R.A.; Meilanov, R.P.; Akhmedov, E.N.; Aliverdiev, A.A. Calculation of multicomponent compound properties using generalization of thermodynamics in derivatives of fractional order. Proc. J. Phys. Conf. Ser. 2016, 774, 012025. [Google Scholar] [CrossRef]
- Laskin, N. Fractional quantum mechanics. Phys. Rev. E 2000, 62, 3135. [Google Scholar] [CrossRef]
- Tarasov, V.E. Fractional statistical mechanics. Chaos 2006, 16, 033108. [Google Scholar] [CrossRef]
- Korichi, Z.; Meftah, M.T. Statistical mechanics based on fractional classical and quantum mechanics. J. Math. Phys. 2014, 55, 033302. [Google Scholar] [CrossRef]
- Tarasov, V.E. Nonlocal statistical mechanics: General fractional Liouville equations and their solutions. Phys. A 2023, 609, 128366. [Google Scholar] [CrossRef]
- Lenzi, E.K.; de Oliveira, B.F.; Astrath, N.G.C.; Malacarne, L.C.; Mendes, R.S.; Baesso, M.L.; Evangelista, L.R. Fractional approach, quantum statistics, and non-crystalline solids at very low temperatures. Eur. Phys. J. B 2008, 62, 155–158. [Google Scholar] [CrossRef]
- Lenzi, E.K.; Astrath, N.G.C.; Rossato, R.; Evangelista, L.R. Nonlocal effects on the thermal behavior of non-crystalline solids. Braz. J. Phys. 2009, 39, 507–510. [Google Scholar] [CrossRef]
- Rajagopal, A.K.; Mendes, R.S.; Lenzi, E.K. Quantum statistical mechanics for nonextensive systems: Prediction for possible experimental tests. Phys. Rev. Lett. 1998, 80, 3907. [Google Scholar] [CrossRef]
- Kadanoff, L.P.; Baym, G. Quantum Statistical Mechanics; CRC Press: Boca Raton, FL, USA, 1963. [Google Scholar]
- Sandev, T.; Petreska, I.; Lenzi, E.K. Time-dependent Schrödinger-like equation with nonlocal term. J. Math. Phys. 2014, 55, 092105. [Google Scholar] [CrossRef]
- Lenzi, E.K.; Evangelista, L.R.; Zola, R.S.; Petreska, I.; Sandev, T. Fractional Schrödinger equation and anomalous relaxation: Nonlocal terms and delta potentials. Mod. Phys. Lett. A 2021, 36, 2140004. [Google Scholar] [CrossRef]
- Lenzi, E.K.; Mendes, R.S.; Rajagopal, A.K. Green functions based on Tsallis nonextensive statistical mechanics: Normalized q-expectation value formulation. Phys. A 2000, 286, 503–517. [Google Scholar] [CrossRef]
- Lenzi, E.K.; Mendes, R.S.; Rajagopal, A.K. Quantum statistical mechanics for nonextensive systems. Phys. Rev. E 1999, 59, 1398. [Google Scholar] [CrossRef]
- Tsallis, C. Probabilistic and Stochastic Dynamical Foundations of Nonextensive Statistical Mechanics. In Introduction to Nonextensive Statistical Mechanics: Approaching a Complex World; Springer: Berlin/Heidelberg, Germany, 2023; pp. 139–227. [Google Scholar]
- Wei, Y.; Ma, X.; Feng, Z.; Zhang, Y.; Zhang, L.; Yang, H.; Qi, Y.; Meng, Z.Y.; Wang, Y.C.; Shi, Y.; et al. Nonlocal effects of low-energy excitations in quantum-spin-liquid candidate Cu3Zn(OH)6FBr. Chin. Phys. Lett. 2021, 38, 097501. [Google Scholar] [CrossRef]
- Kramers, H.C.; Wasscher, J.D.; Gorter, C.J. The specific heat of liquid helium between 0.25 and 1.9° K. Physica 1952, 18, 329–338. [Google Scholar] [CrossRef]
- Rovenchak, A. Ideal Bose-gas in nonadditive statistics. Low Temp. Phys. 2018, 44, 1025–1031. [Google Scholar] [CrossRef]
- Biró, T.S.; Shen, K.M.; Zhang, B.W. Non-extensive quantum statistics with particle–hole symmetry. Phys. A 2015, 428, 410–415. [Google Scholar] [CrossRef]
- Rahaman, M.; Bhattacharyya, T.; Alam, J. Thermal field theory of the Tsallis statistics. arXiv 2019, arXiv:1906.02893. [Google Scholar]
- Lenzi, E.K.; Lenzi, M.K.; Belich, H.; Lucena, L.S. Specific heat in the nonextensive statistics: Effective temperature and Lagrange parameter β. Phys. Lett. A 2002, 292, 315–319, Erratum in Phys. Lett. A 2002, 299, 318. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lenzi, E.K.; Evangelista, L.R.; da Silva, L.R. Aspects of Quantum Statistical Mechanics: Fractional and Tsallis Approaches. Mathematics 2023, 11, 2777. https://doi.org/10.3390/math11122777
Lenzi EK, Evangelista LR, da Silva LR. Aspects of Quantum Statistical Mechanics: Fractional and Tsallis Approaches. Mathematics. 2023; 11(12):2777. https://doi.org/10.3390/math11122777
Chicago/Turabian StyleLenzi, Ervin Kaminski, Luiz Roberto Evangelista, and Luciano Rodrigues da Silva. 2023. "Aspects of Quantum Statistical Mechanics: Fractional and Tsallis Approaches" Mathematics 11, no. 12: 2777. https://doi.org/10.3390/math11122777
APA StyleLenzi, E. K., Evangelista, L. R., & da Silva, L. R. (2023). Aspects of Quantum Statistical Mechanics: Fractional and Tsallis Approaches. Mathematics, 11(12), 2777. https://doi.org/10.3390/math11122777