Synchronization Analysis of Linearly Coupled Systems with Signal-Dependent Noises
Abstract
1. Introduction
2. Preliminaries
- If near , the system is locally stable near ;
- If as , and for all , then system is globally stable;
- If near , the system is locally unstable near .
3. Synchronization of Linear Multiagent Systems
- (1)
- A constant exists, such that ; noticing that , then the condition is equal to the existence of another constant , such that . Using Formulas (12)~(15), a constant exists, such thatwhere . We can see that if , the relation is satisfied near the synchronization manifold ; thus the synchronization manifold is locally stable. If and , then a exists, such that , which means that the synchronization manifold is globally stable.
- (2)
- A constant exists, such that . Similarly, this condition is equal to the existence of another constant , such that . Using Formulas (12)~(15), we havewhere . Then it is obvious that if , a exists which satisfies the relation . As a result, the synchronization manifold is locally unstable. □
4. Synchronization of Linearly Coupled Nonlinear Dynamical Systems
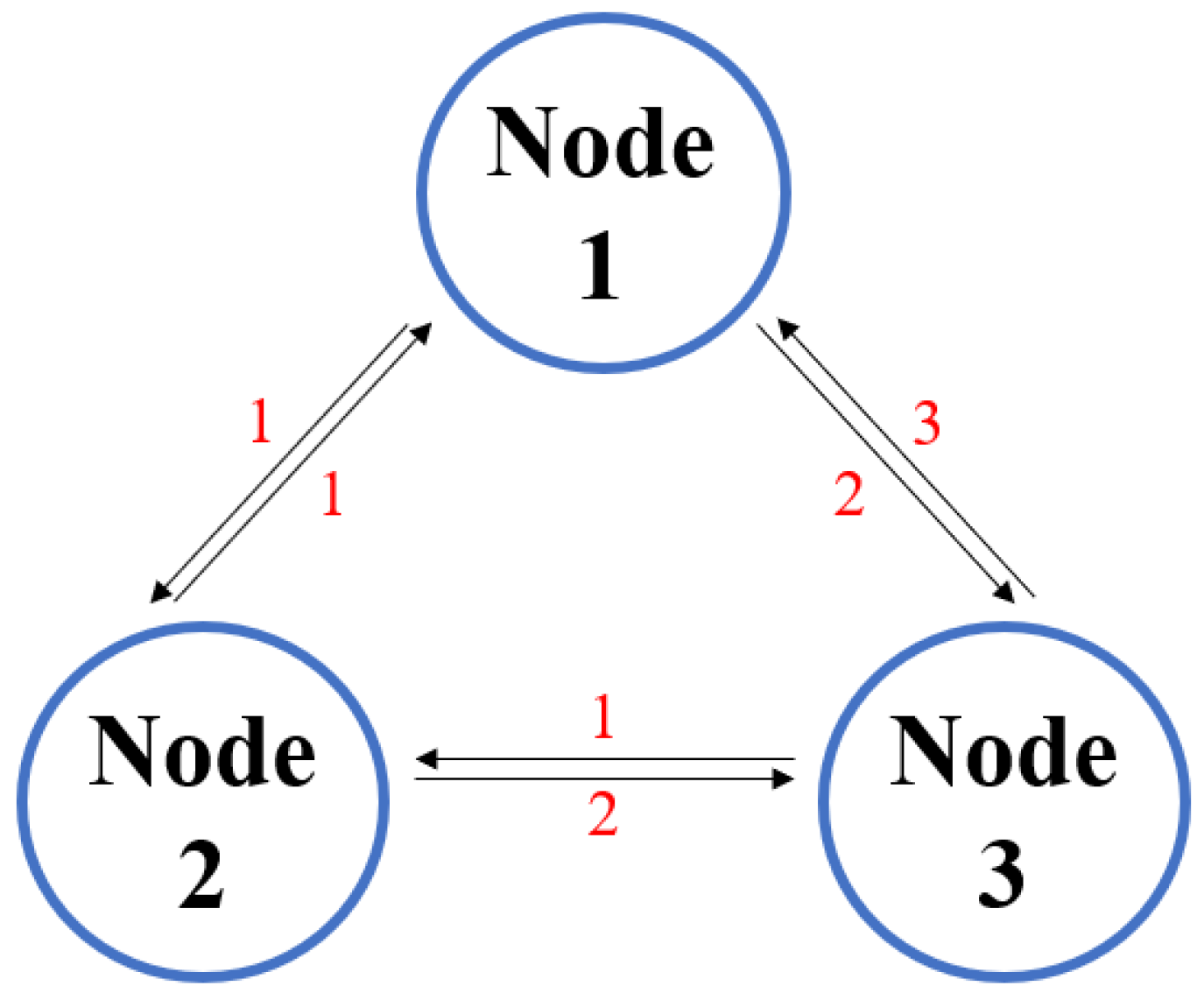
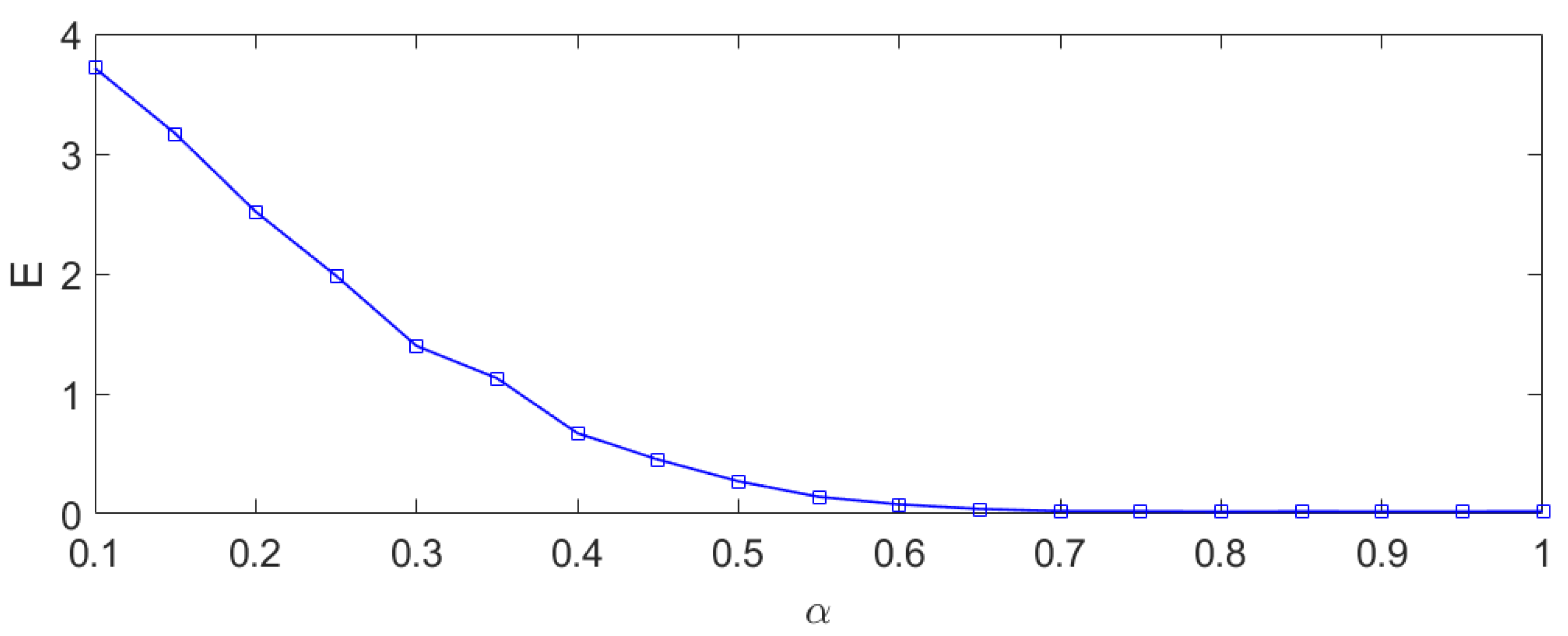
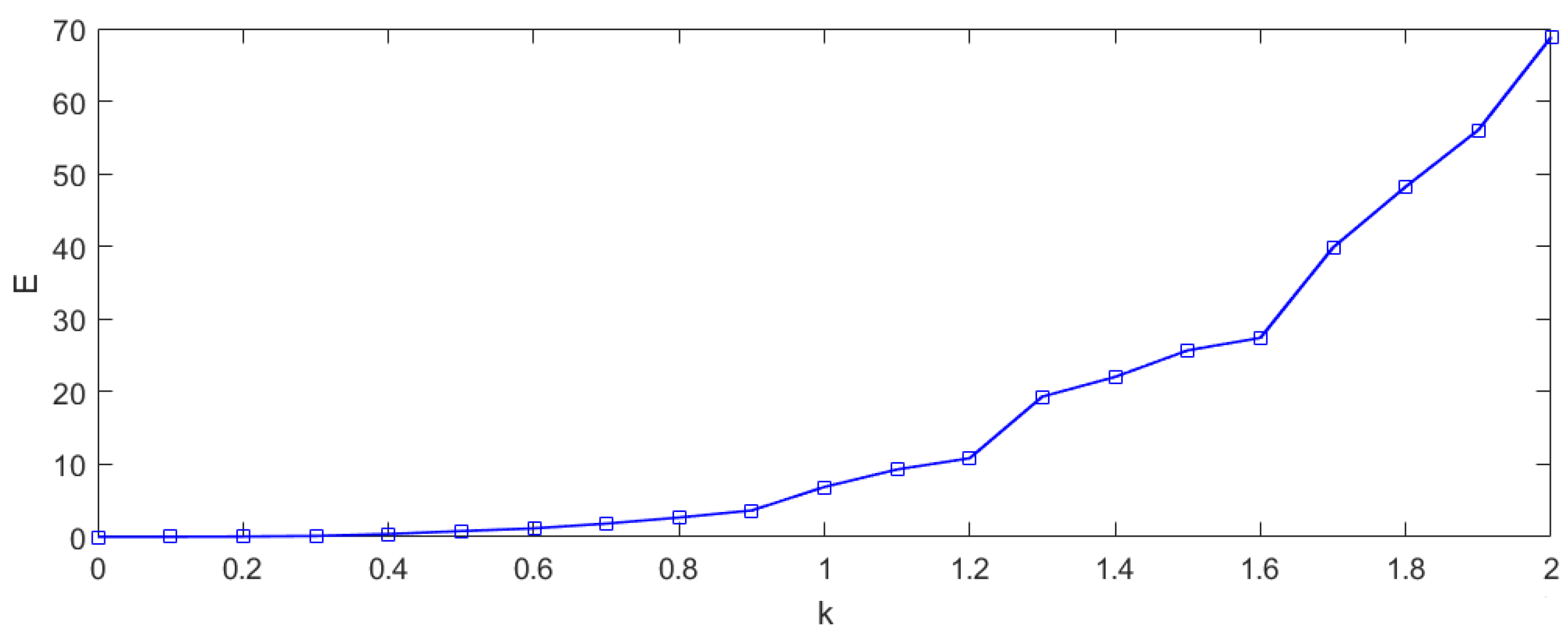
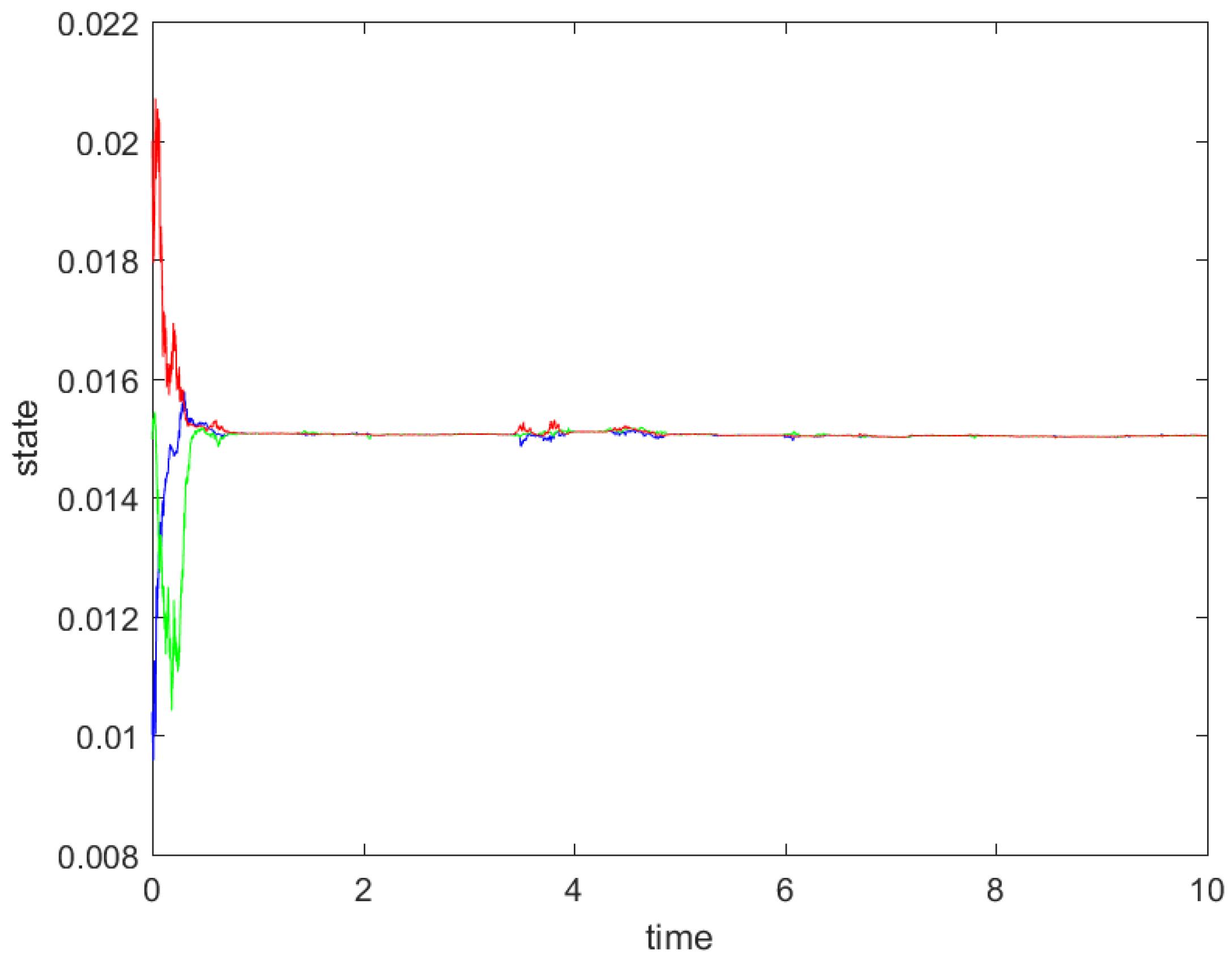
5. Numerical Experiments
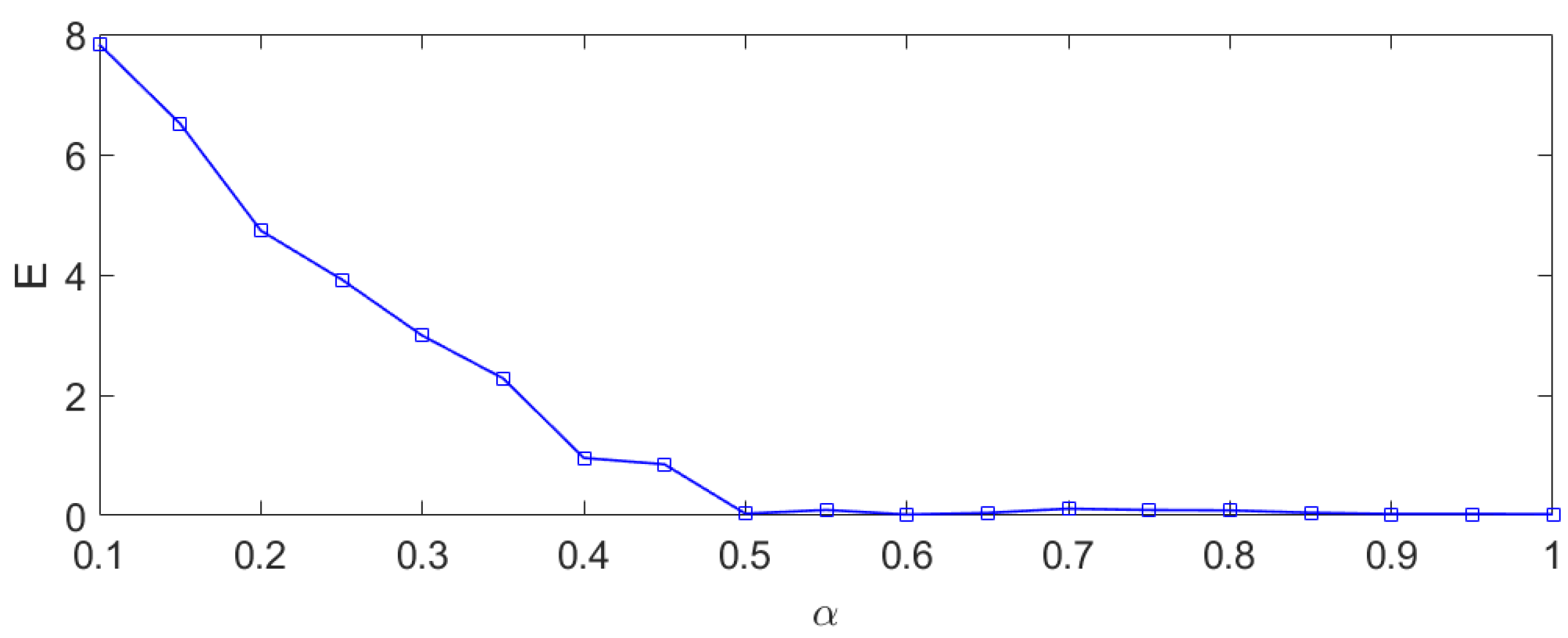
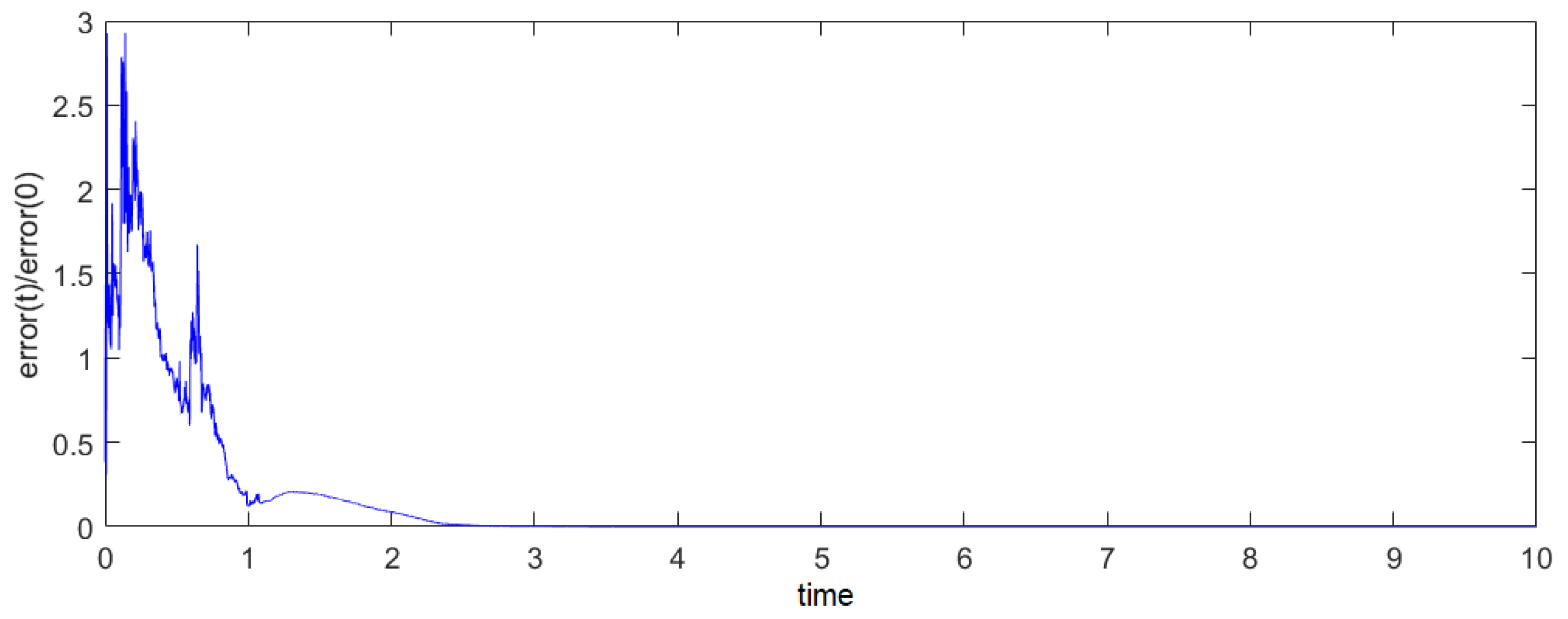
5.1. Simulation of Synchronization on the Multiagent System
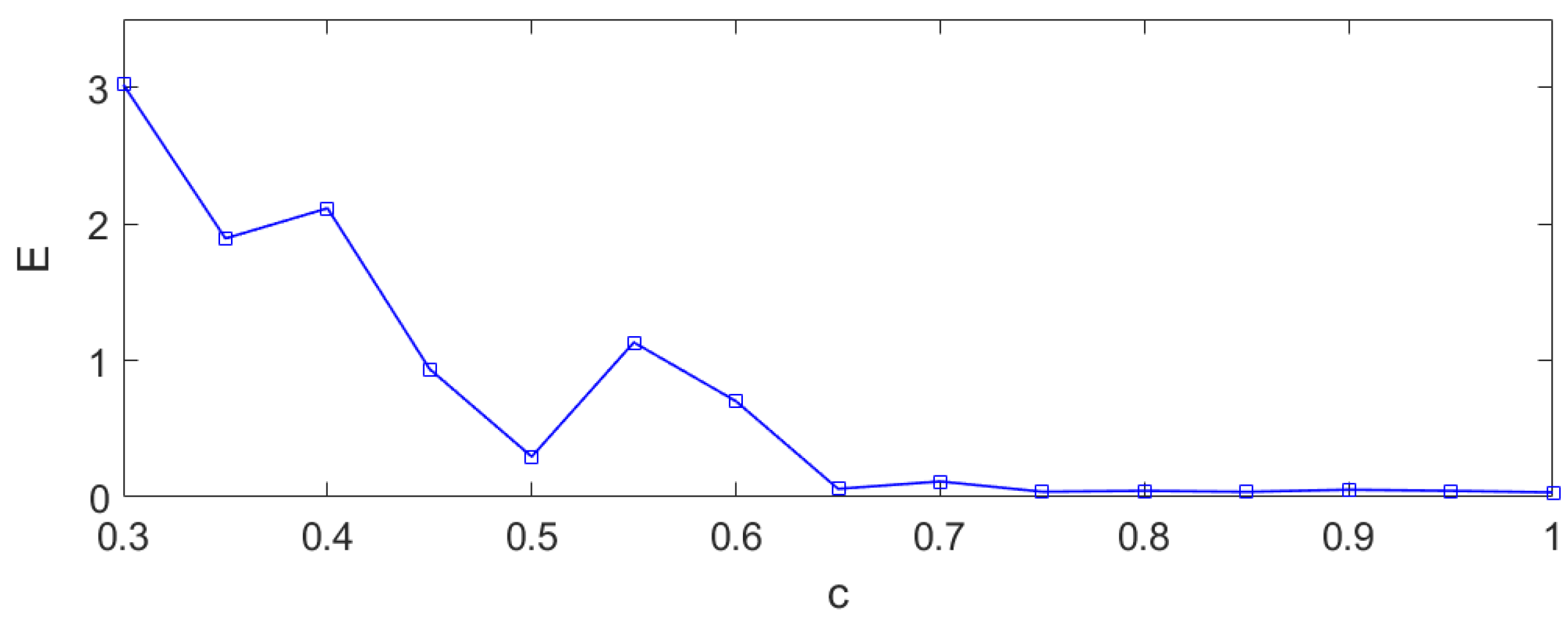
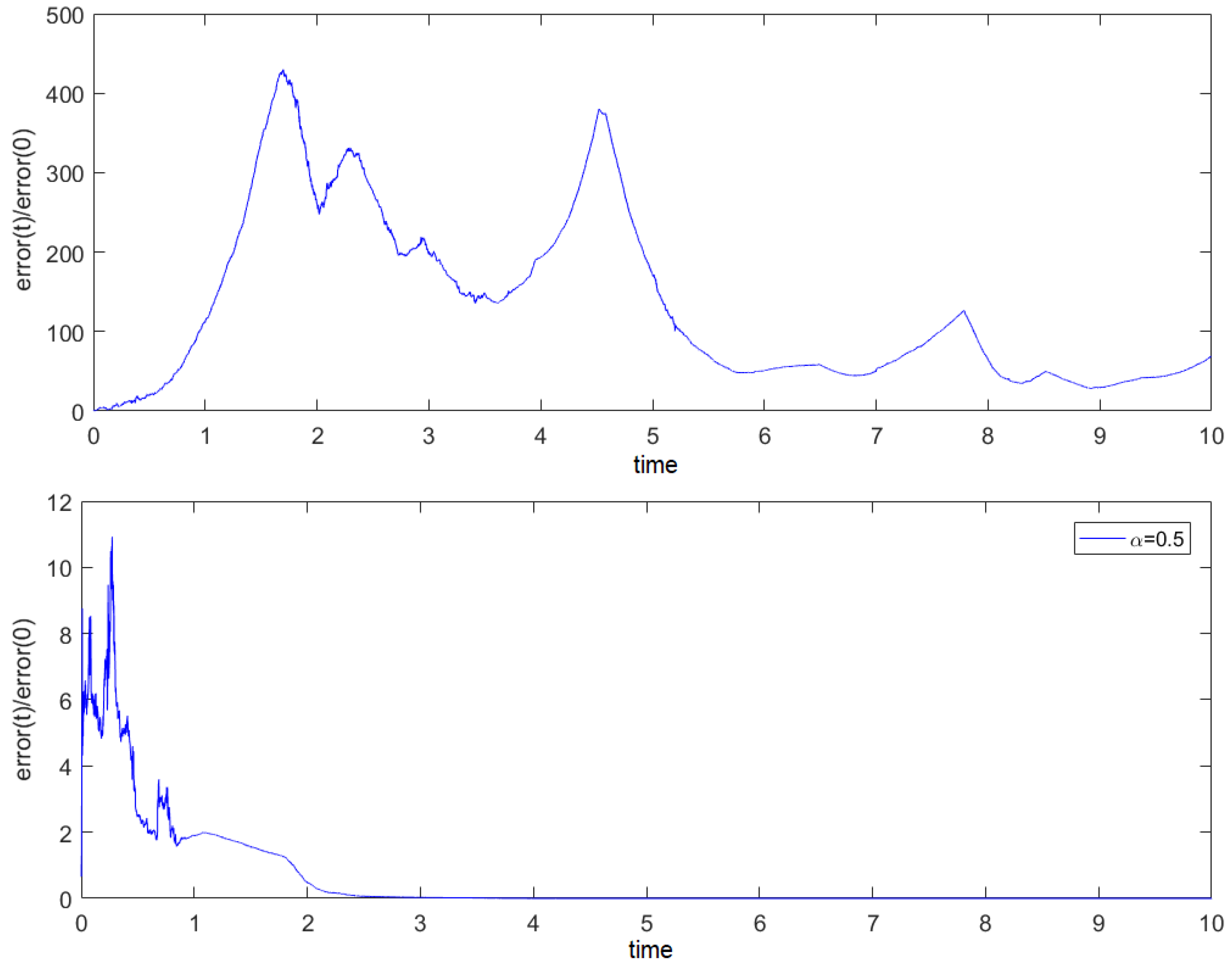
5.2. Simulation of Synchronization on the Linearly Coupled Nonlinear Dynamical Systems
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lu, W.; Chen, T. New approach to synchronization analysis of linearly coupled ordinary differential systems. Phys. D Nonlinear Phenom. 2006, 213, 214–230. [Google Scholar] [CrossRef]
- Lu, W.; Chen, T.; Chen, G. Synchronization analysis of linearly coupled systems described by differential equations with a coupling delay. Phys. D Nonlinear Phenom. 2006, 221, 118–134. [Google Scholar] [CrossRef]
- Yi, X.; Lu, W.; Chen, T. Achieving Synchronization in Arrays of Coupled Differential Systems with Time-Varying Couplings. Abstr. Appl. Anal. 2013, 2013, 134265. [Google Scholar] [CrossRef]
- Lu, W.; Chen, T. Synchronization of networks with time-varying couplings. Appl. Math. A J. Chin. Univ. 2013, 28, 438–454. [Google Scholar] [CrossRef]
- Yi, X.; Lu, W.; Chen, T. Synchronization analysis of coupled differential systems with time-varying couplings. In Proceedings of the 2013 25th Chinese Control and Decision Conference (CCDC), Guiyang, China, 25–27 May 2013; pp. 4640–4645. [Google Scholar]
- Wu, W.; Zhou, W.; Chen, T. Cluster Synchronization of Linearly Coupled Complex Networks Under Pinning Control. IEEE Trans. Circuits Syst. I Regul. Pap. 2009, 56, 829–839. [Google Scholar] [CrossRef]
- Zhao, Y.; Feng, J.; Wang, J. On Cluster Synchronization for Linearly Coupled Complex Networks. In Proceedings of the 2011 Seventh International Conference on Computational Intelligence and Security, Washington, DC, USA, 3–4 December 2011; pp. 300–304. [Google Scholar]
- Zhao, Y.; Feng, J.; Wang, J. Cluster Synchronization for Linearly Coupled Complex Networks with Identical and Nonidentical Nodes. Math. Probl. Eng. 2012, 2012, 534743. [Google Scholar] [CrossRef]
- Jing, J.; Chen, F.; Xiang, L. Event-triggered control for cluster synchronization of linearly coupled complex networks. In Proceedings of the 33rd Chinese Control Conference, Nanjing, China, 28–30 July 2014; pp. 1299–1304. [Google Scholar]
- Fang, Q.; Peng, J.; Cao, F. Synchronization and Control of Linearly Coupled Singular Systems. Math. Probl. Eng. 2013, 2013, 230741. [Google Scholar]
- Chen, T.; Liu, X.; Lu, W. Pinning Complex Networks by a Single Controller. IEEE Trans. Circuits Syst. I Regul. Pap. 2007, 54, 1317–1326. [Google Scholar] [CrossRef]
- Ping, T.; Chen, S. New results for exponential synchronization of linearly coupled ordinary differential systems. Chin. Phys. B 2017, 26, 050503. [Google Scholar]
- Jadbabaie, A.; Lin, J.; Morse, A. Coordination of groups of mobile autonomous agents using nearest neighbour rules. IEEE Transcat. Autom. Control 2003, 48, 988–1001. [Google Scholar] [CrossRef]
- Olfati-Saber, R.; Murray, R.M. Consensus problems in networks of agents with switching topology and time-delays. IEEE Transcat. Autom. Control 2004, 49, 1520–1533. [Google Scholar] [CrossRef]
- Cao, Y.; Ren, W. Optimal linear-consensus algorithms: An LQR perspective. IEEE Trans. Syst. Man Cybern. 2010, 40, 819–830. [Google Scholar]
- Saber, R.; Fax, J.; Murray, R. Consensus and cooperation in networked multi-agent systems. Proc. IEEE 2007, 95, 215–233. [Google Scholar] [CrossRef]
- Sepulchre, R. Consensus on nonlinear spaces. Annu. Rev. Control. 2012, 35, 56–64. [Google Scholar] [CrossRef]
- Dofler, F.; Bullo, F. Synchronization and transient stability in power networks and nonuniform Kuramoto oscillators. SIAM J. Control. Optim. 2012, 50, 1616–1642. [Google Scholar] [CrossRef]
- Dofler, F.; Chertkov, M.; Bullo, F. Synchronization in complex oscillator networks and smart grids. Proc. Natl. Acad. Sci. USA 2013, 110, 2005–2010. [Google Scholar] [CrossRef]
- Uhlhass, P.; Singer, W. Neural synchrony in brain disorder: Relevance for cognitive dysfunctions and pathophysiology. Neuron 2006, 52, 155–168. [Google Scholar] [CrossRef]
- Engel, A.; Fries, P.; Singer, W. Rapid feature selective neuronal synchronization through correlated latency shifting. Nat. Rev. Neurosci. 2001, 2, 704–716. [Google Scholar] [CrossRef]
- Palva, J.M.; Monto, S.; Kulashekhar, S.; Palva, S. Neuronal synchrony reveals working memory networks and predicts individual memory capacity. Proc. Natl. Acad. Sci. USA 2010, 107, 7580–7585. [Google Scholar] [CrossRef]
- Krinsky, V.I.; Biktashev, V.N.; Efimov, I.R. Autowaves principles for parallel image processing. Physica D 1991, 49, 247–253. [Google Scholar] [CrossRef]
- Perez-Manuzuri, V.; Perez-Villar, V.; Chua, L.O. Autowaves for image processing on a two-dimensional CNN array of excitable nonlinear circuits: Flat and wrinkled labyrinths. IEEE Trans. Circuits Syst. I 1995, 40, 174–181. [Google Scholar] [CrossRef]
- Lu, W.; Chen, T. Synchronization of coupled connected neural networks with delays. IEEE Trans. Circuits Syst. I 2004, 51, 2491–2503. [Google Scholar] [CrossRef]
- Lin, W.; He, Y. Complete synchronization of the noise-perturbed Chua’s circuits. Chaos Interdiscip. J. Nonlinear Sci. 2005, 15, 023705. [Google Scholar] [CrossRef] [PubMed]
- Lin, W. Using white noise to enhance synchronization of coupled chaotic systems. Chaos Interdiscip. J. Nonlinear Sci. 2006, 16, 013134. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Cao, J. Adaptive lag synchronization of unknown chaotic delayed neural networks with noise perturbation. Phys. Lett. A 2007, 364, 277–285. [Google Scholar] [CrossRef]
- Sun, Y.; Cao, J. Adaptive synchronization between two different noise-perturbed chaotic systems with fully unknown parameters. Phys. A Stat. Mech. Appl. 2007, 376, 253–265. [Google Scholar] [CrossRef]
- Zheng, S.; Jiong, R. Synchronization between two different chaotic systems with noise perturbation. Chin. Phys. B 2010, 19, 070513. [Google Scholar] [CrossRef]
- Sun, Y.; Li, W.; Ruan, J. Generalized outer synchronization between complex dynamical networks with time delay and noise perturbation. Commun. Nonlinear Sci. Numer. Simul. 2013, 18, 989–998. [Google Scholar] [CrossRef]
- Sun, Y.; Ruan, J. Synchronization in coupled time-delayed systems with parameter mismatch and noise perturbation. Chaos Interdiscip. J. Nonlinear Sci. 2009, 19, 043113. [Google Scholar] [CrossRef]
- Zhao, X.; Zhou, J.; Lu, J. Pinning Synchronization of Multiplex Delayed Networks with Stochastic Perturbations. IEEE Trans. Cybern. 2019, 49, 4262–4270. [Google Scholar] [CrossRef]
- Chen, Z. Complete and generalized synchronization in a class of noise perturbed chaotic systems. Chaos Interdiscip. J. Nonlinear Sci. 2007, 17, 023106. [Google Scholar] [CrossRef] [PubMed]
- Feng, J.; He, L.; Xu, C.; Francis, A.; Wu, G. Synchronizing the noise-perturbed Genesio chaotic system by sliding mode control. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 2546–2551. [Google Scholar]
- Kong, C.; Chen, S. Synchronization of noise-perturbed generalized Lorenz system by sliding mode control. Chin. Phys. B 2009, 18, 91. [Google Scholar]
- Zhang, Q.; Chen, S.; Hu, Y.; Wang, C. Synchronizing the noise-perturbed unified chaotic system by sliding mode control. Phys. A Stat. Mech. Appl. 2006, 371, 317–324. [Google Scholar] [CrossRef]
- Xiao, Y.; Xu, W.; Tang, S.; Li, X. Adaptive complete synchronization of the noise-perturbed two bi-directionally coupled chaotic systems with time-delay and unknown parametric mismatch. Appl. Math. Comput. 2009, 213, 538–547. [Google Scholar] [CrossRef]
- Song, B.; Park, J.H.; Wu, Z.; Zhang, Y. Global synchronization of complex networks perturbed by the Poisson noise. Appl. Math. Comput. 2012, 219, 3831–3839. [Google Scholar] [CrossRef]
- Serdar, C.; Yilmaz, U.; Ihsan, P. Simulation and circuit implementation of sprott case H chaotic system and its synchronization application for secure communication systems. J. Circuits Syst. Comput. 2013, 22, 1350022. [Google Scholar]
- Lu, J.; Wu, X.; Lu, J. Synchronization of a unified chaotic system and the application in secure communication. Phys. Lett. A 2002, 305, 365–370. [Google Scholar] [CrossRef]
- Shyam, S.; Ali, A.M. Synchronization of Randomly Multiplexed Chaotic Systems with Application to Communication. Phys. Rev. Lett. 2000, 85, 5456. [Google Scholar]
- Akgul, A.; Rajagopal, K.; Durdu, A.; Pala, M.A.; Boyaz, O.F.; Yildiz, M.Z. A simple fractional-order chaotic system based on memristor and memcapacitor and its synchronization application. Chaos Solitons Fractals 2021, 152, 111306. [Google Scholar] [CrossRef]
- Kocarev, L.; Parlitz, U. General Approach for Chaotic Synchronization with Applications to Communication. Phys. Rev. Lett. 1995, 74, 5028. [Google Scholar] [CrossRef] [PubMed]
- Luo, Q.; Ge, T.; Grabenhorst, F.; Feng, J.; Rolls, E.T. Attention-Dependent Modulation of Cortical Taste Circuits Revealed by Granger Causality with Signal-Dependent Noise. PLoS Comput. Biol. 2013, 9, e1003265. [Google Scholar] [CrossRef] [PubMed]
- Luo, Q.; Ma, Y.; Bhatt, M.A.; Montague, P.R.; Feng, J. The Functional Architecture of the Brain Underlies Strategic Deception in Impression Management. Front. Hum. Neurosci. 2017, 11, 513. [Google Scholar] [CrossRef] [PubMed]
- Harris, C.M.; Wolpert, D.M. Signal-dependent noise determines motor planning. Nature 1998, 394, 780–784. [Google Scholar] [CrossRef]
- Ishihara, A.K.; Doornik, J.V.; Ben-Menahem, S. Stochastic stability of a neural-net robot controller subject to signal-dependent noise in the learning rule. Int. J. Adapt. Control. Signal Process. 2010, 24, 445–466. [Google Scholar] [CrossRef]
- Jiang, Y.; Jiang, Z. A robust adaptive dynamic programming principle for sensorimotor control with signal-dependent noise. J. Syst. Sci. Complex. 2015, 28, 261–288. [Google Scholar] [CrossRef]
- Bian, T.; Jiang, Z. Adaptive optimal control for linear stochastic systems with additive noise. In Proceedings of the 34th Chinese Control Conference, Hangzhou, China, 28–30 July 2015; pp. 3011–3016. [Google Scholar]
- Horn, P.A.; Johnson, C.R. Matrix Analysis; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Minc, H. Nonnegative Matrices; Wiley: New York, NY, USA, 1988. [Google Scholar]
- Lu, W.; Chen, T. QUAD-Condition, Synchronization, Consensus of Multiagents, and Anti-Synchronization of Complex Networks. IEEE Trans. Cybern. 2021, 51, 3384–3388. [Google Scholar] [CrossRef] [PubMed]
- Mao, X. Stochastic Differential Equations and Applications; Elsevier: Amsterdam, The Netherlands, 1997. [Google Scholar]
- Lewis, F.L.; Zhang, H.; Hengster-Movric, K.; Das, A. Cooperative Control of Multi-Agent Systems: Optimal and Adaptive Design Approaches; Springer Science & Business Media: Berlin, Germany, 2013. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, Y.; Luo, Q.; Lu, W. Synchronization Analysis of Linearly Coupled Systems with Signal-Dependent Noises. Mathematics 2023, 11, 2328. https://doi.org/10.3390/math11102328
Ren Y, Luo Q, Lu W. Synchronization Analysis of Linearly Coupled Systems with Signal-Dependent Noises. Mathematics. 2023; 11(10):2328. https://doi.org/10.3390/math11102328
Chicago/Turabian StyleRen, Yanhao, Qiang Luo, and Wenlian Lu. 2023. "Synchronization Analysis of Linearly Coupled Systems with Signal-Dependent Noises" Mathematics 11, no. 10: 2328. https://doi.org/10.3390/math11102328
APA StyleRen, Y., Luo, Q., & Lu, W. (2023). Synchronization Analysis of Linearly Coupled Systems with Signal-Dependent Noises. Mathematics, 11(10), 2328. https://doi.org/10.3390/math11102328





