Polynomial Noises for Nonlinear Systems with Nonlinear Impulses and Time-Varying Delays
Abstract
:1. Introduction
2. Problem Description
3. Stochastic Suppression of Explosive Solution
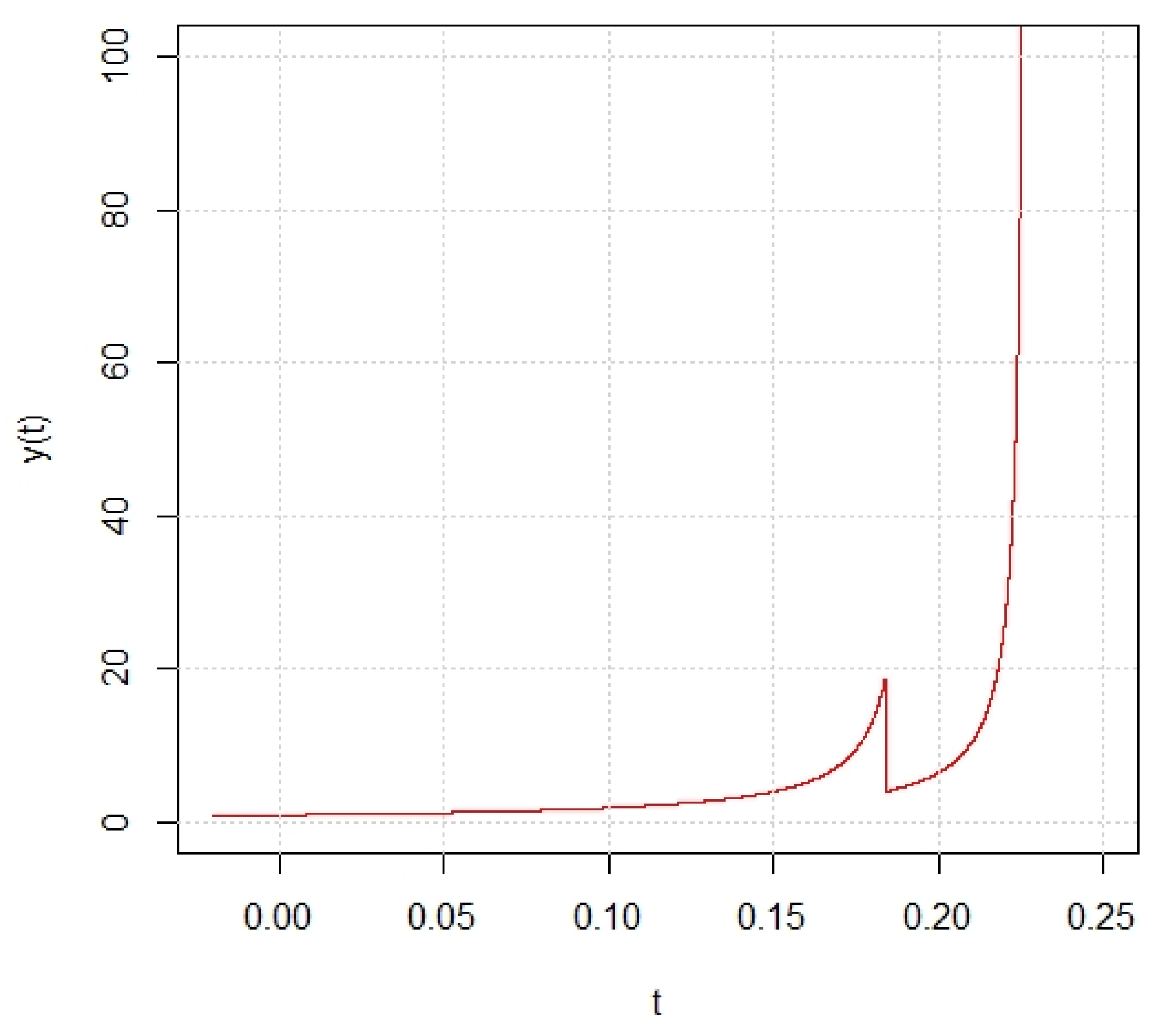
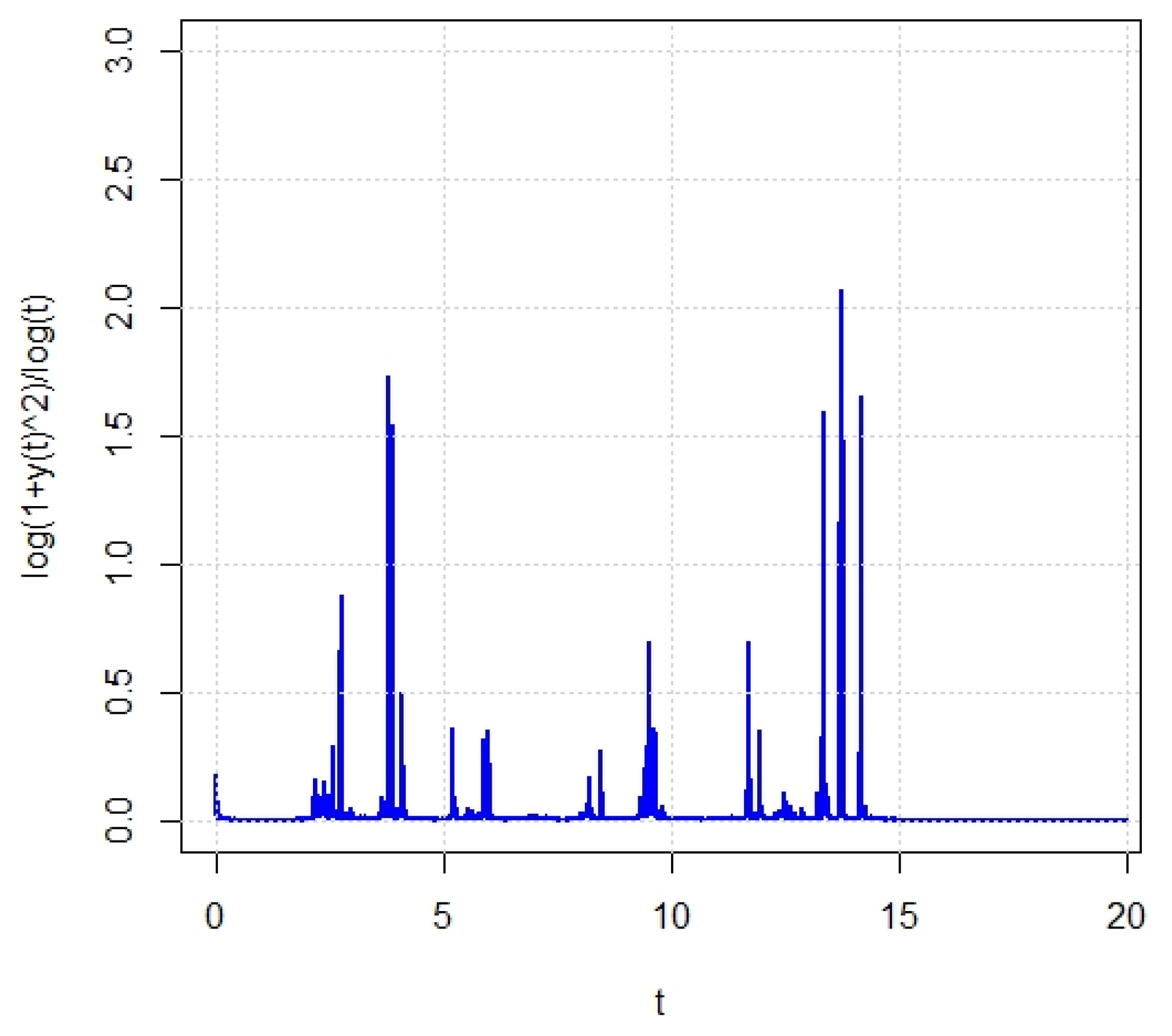
4. A Numeric Example
5. Conclusions and Future Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Proof of Theorem 1
Appendix B. Proof of Theorem 2
Appendix C. Proof of Theorem 3
References
- Khasminiskii, R.Z. Stochatic Stability of Differential Equations; Sijthoff and Noordhoff: Alphen aan den Rijn, The Netherlands, 1981. [Google Scholar]
- Arnold, L.; Crauel, H.; Wihstutz, V. Stabilization of Linear Systems by Noise. SIAM J. Control Optim. 1983, 21, 451–461. [Google Scholar] [CrossRef]
- Mao, X. Stochastic Differential Equations and Applications; Horwood: Chichester, UK, 1997. [Google Scholar]
- Mao, X. Exponential Stability of Stochastic Differential Equations; Dekker: New York, NY, USA, 1994. [Google Scholar]
- Appleby, J.A.D.; Mao, X. Stochatic stabilisation of functional differential equations. Syst. Control Lett. 2005, 54, 1069–1081. [Google Scholar] [CrossRef]
- Appleby, J.A.D.; Mao, X.; Rodkina, A. Stabilisation and destabilization of nonlinear differential equations by noise. IEEE Trans. Autom. Control 2008, 53, 638–691. [Google Scholar] [CrossRef] [Green Version]
- Mao, X.; Marion, G.; Renshaw, E. Environmental noise suppresses explosion in population dynamics. Stoch. Processes Appl. 2002, 97, 95–110. [Google Scholar] [CrossRef]
- Wu, F.; Hu, S. Suppression and stabilization of noise. Int. J. Control 2009, 82, 2150–2157. [Google Scholar] [CrossRef]
- Wu, F.; Hu, S. Stochastic suppression and stabilization of delay differential systems. Int. J. Robust Nonlinear Control 2011, 21, 488–500. [Google Scholar] [CrossRef]
- Yin, G.; Zhao, G.; Wu, F. Regularization and Stabilization of Randomly Switching Dynamic Systems. SIAM J. Appl. Math. 2012, 72, 1361–1382. [Google Scholar] [CrossRef]
- Deng, F.; Luo, Q.; Mao, X. Stochastic stabilization of hybrid differential equations. Automatica 2012, 48, 2321–2328. [Google Scholar] [CrossRef]
- Huang, L. Stochastic stabilization and destabilization of nonlinear differential equations. Syst. Control Lett. 2013, 62, 163–169. [Google Scholar] [CrossRef]
- Deng, F.; Luo, Q.; Mao, X.; Pang, S. Noise suppresses or expresses exponential growth. Syst. Control Lett. 2008, 57, 262–270. [Google Scholar] [CrossRef] [Green Version]
- Hu, G.; Liu, M.; Mao, X.; Song, M. Noise expresses exponential growth under regime switching. Syst. Control Lett. 2009, 58, 691–699. [Google Scholar] [CrossRef] [Green Version]
- Zhu, S.; Yang, Q.; Shen, Y. Noise further expresses exponential decay for globally exponentially stable time-varying delayed neural networks. Neural Netw. 2016, 77, 7–13. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Shen, Y. Noise suppresses explosive solutions of differential systems with coefficients satisfying the polynomial growth condition. Automatica 2012, 48, 619–624. [Google Scholar] [CrossRef]
- Feng, L.; Wu, Z.; Zheng, S. A note on explosion suppression for nonlinear delay differential systems by polynomial noise. Int. J. Gen. Syst. 2018, 47, 137–154. [Google Scholar] [CrossRef]
- Feng, L.; Li, S.; Song, R.; Li, Y. Suppression of explosion by polynomial noise for nonlinear differential systems. Sci. China Inf. Sci. 2018, 61, 136–146. [Google Scholar] [CrossRef]
- Dubkov, A.A.; Spagnolo, B. Verhulst model with Lévy white noise excitation. Eur. Phys. J. B 2008, 65, 361–367. [Google Scholar] [CrossRef] [Green Version]
- Spagnolo, B.; Dubkov, A.A.; Pankratov, A.L.; Pankratova, E.V.; Fiasconaro, A.; Ochab-Marcinek, A. Lifetime of metastable states and suppression of noise in interdisciplinary physical models. Acta Phys. Pol. B 2008, 38, 1925–1950. [Google Scholar]
- Dubkov, A.A.; Litovsky, I.A. Probabilistic characteristics of noisy Van der Pol type oscillator with nonlinear damping. J. Stat. Mech. Theory Exp. 2016, 2016, 054036. [Google Scholar] [CrossRef]
- Mikhaylov, A.N.; Guseinov, D.V.; Belov, A.I.; Korolev, D.S.; Shishmakova, V.A.; Koryazhkina, M.N.; Filatov, D.O.; Alonso, F.V.; Carollo, A.; Spagnolo, B.; et al. Stochastic resonance in a Metal-Oxide memristive device. Chaos Solitons Fractals 2021, 144, 110723. [Google Scholar] [CrossRef]
- Lakshmikantham, V.; Bainov, D.D.; Simeonov, P.S. Theory of Impulsive Differential Equations; World Scientific: Singapore, 1989. [Google Scholar]
- Feketa, P.; Klinshov, V.; Lücken, L. A survey on the modeling of hybrid behaviors: How to account for impulsive jumps properly. Commun. Nonlinear Sci. Numer. Simul. 2021, 103, 105955. [Google Scholar] [CrossRef]
- Yang, X.; Lu, J.; Ho, D.W.C.; Song, Q. Synchronization of uncertain hybrid switching and impulsive complex networks. Appl. Math. Model. 2018, 59, 379–392. [Google Scholar] [CrossRef]
- Yang, X.; Li, X.; Lu, J.; Cheng, Z. Synchronization of time-delayed complex networks with switching topology via hybrid actuator fault and impulsive effects control. IEEE Trans. Cybern. 2020, 50, 4043–4052. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Feng, L.; Wu, Z.; Park, J.H. Stability criteria of random delay differential systems subject to random impulses. Int. J. Robust Nonlinear Control 2021, 31, 6681–6698. [Google Scholar] [CrossRef]
- Peng, D.; Li, X.; Rakkiyappan, R.; Ding, Y. Stabilization of stochastic delayed systems: Event-triggered impulsive control. Appl. Math. Comput. 2021, 401, 126054. [Google Scholar] [CrossRef]
- Fu, X.; Li, X. LMI conditions for stability of impulsive stochastic Cohen–Grossberg neural networks with mixed delays. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 435–454. [Google Scholar] [CrossRef]
- Hu, W.; Zhu, Q. Stability analysis of impulsive stochastic delayed differential systems with unbounded delays. Syst. Control Lett. 2020, 136, 104606. [Google Scholar] [CrossRef]
- Peng, S.; Deng, F. New Criteria on pth Moment Input-to-State Stability of Impulsive Stochastic Delayed Differential Systems. IEEE Trans. Autom. Control 2017, 62, 3573–3579. [Google Scholar] [CrossRef]
- Cheng, P.; Deng, F.; Yao, F. Almost sure exponential stability and stochastic stabilization of stochastic differential systems with impulsive effects. Nonlinear Anal. Hybrid Syst. 2018, 30, 106–117. [Google Scholar] [CrossRef]
- Hao, S.; Zhang, C.; Feng, L.; Yan, S. Noises for Impulsive Differential Systems. IEEE Access 2019, 7, 138253–138259. [Google Scholar] [CrossRef]
- Feng, L.; Wu, Z.; Cao, J.; Zheng, S.; Alsaadi, F.E. Exponential stability for nonlinear hybrid stochastic systems with time varying delays of neutral type. Appl. Math. Lett. 2020, 107, 106468. [Google Scholar] [CrossRef]
- Shukla, A.; Patel, R. Existence and Optimal Control Results for Second-Order Semilinear System in Hilbert Spaces. Circuits Syst. Signal Process. 2021, 40, 4246–4258. [Google Scholar] [CrossRef]
- Singh, A.; Shukla, A.; Vijayakumar, V.; Udhayakumar, R. Asymptotic stability of fractional order (1,2] stochastic delay differential equations in Banach spaces. Chaos Solitons Fractals 2021, 150, 111095. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, L.; Wang, Q.; Zhang, C.; Gong, D. Polynomial Noises for Nonlinear Systems with Nonlinear Impulses and Time-Varying Delays. Mathematics 2022, 10, 1525. https://doi.org/10.3390/math10091525
Feng L, Wang Q, Zhang C, Gong D. Polynomial Noises for Nonlinear Systems with Nonlinear Impulses and Time-Varying Delays. Mathematics. 2022; 10(9):1525. https://doi.org/10.3390/math10091525
Chicago/Turabian StyleFeng, Lichao, Qiaona Wang, Chunyan Zhang, and Dianxuan Gong. 2022. "Polynomial Noises for Nonlinear Systems with Nonlinear Impulses and Time-Varying Delays" Mathematics 10, no. 9: 1525. https://doi.org/10.3390/math10091525
APA StyleFeng, L., Wang, Q., Zhang, C., & Gong, D. (2022). Polynomial Noises for Nonlinear Systems with Nonlinear Impulses and Time-Varying Delays. Mathematics, 10(9), 1525. https://doi.org/10.3390/math10091525





