A Comparative Study of Different Schemes Based on Bézier-like Functions with an Application of Craniofacial Fractures Reconstruction
Abstract
1. Introduction
- A comparison between the existing scheme-like techniques (mirroring, reference skull, thin plate spline, iterative closest point, radial basis functions, the technique using CAD/CAM) and the techniques using spline curves.
- A comparative analysis of techniques based on spline curves.
2. Theoretical Foundation
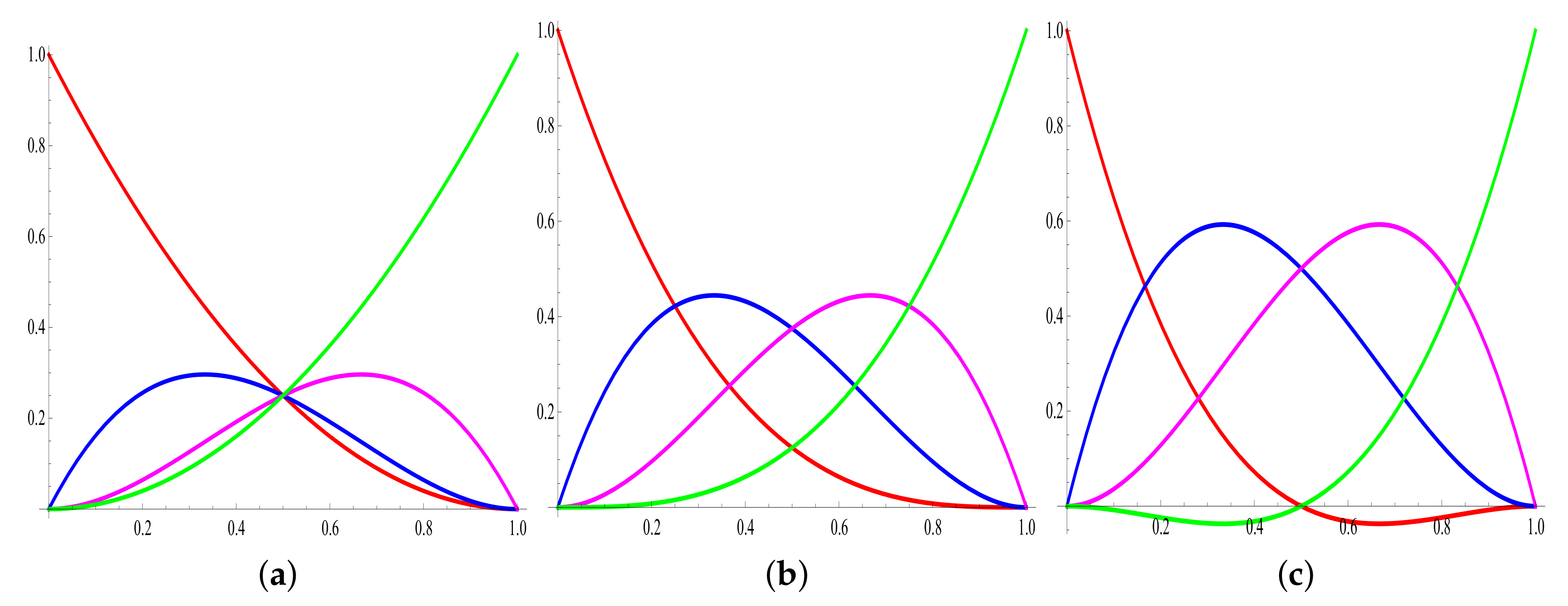
2.1. Bézier-like Functions
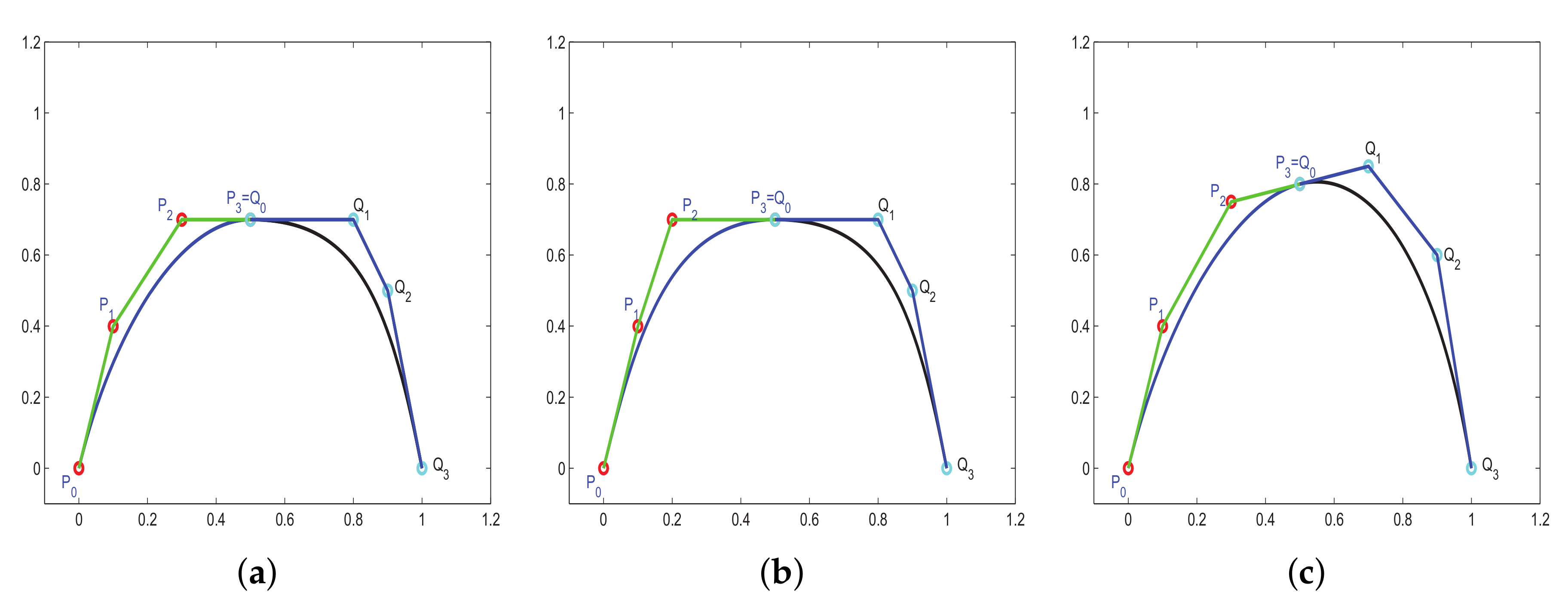
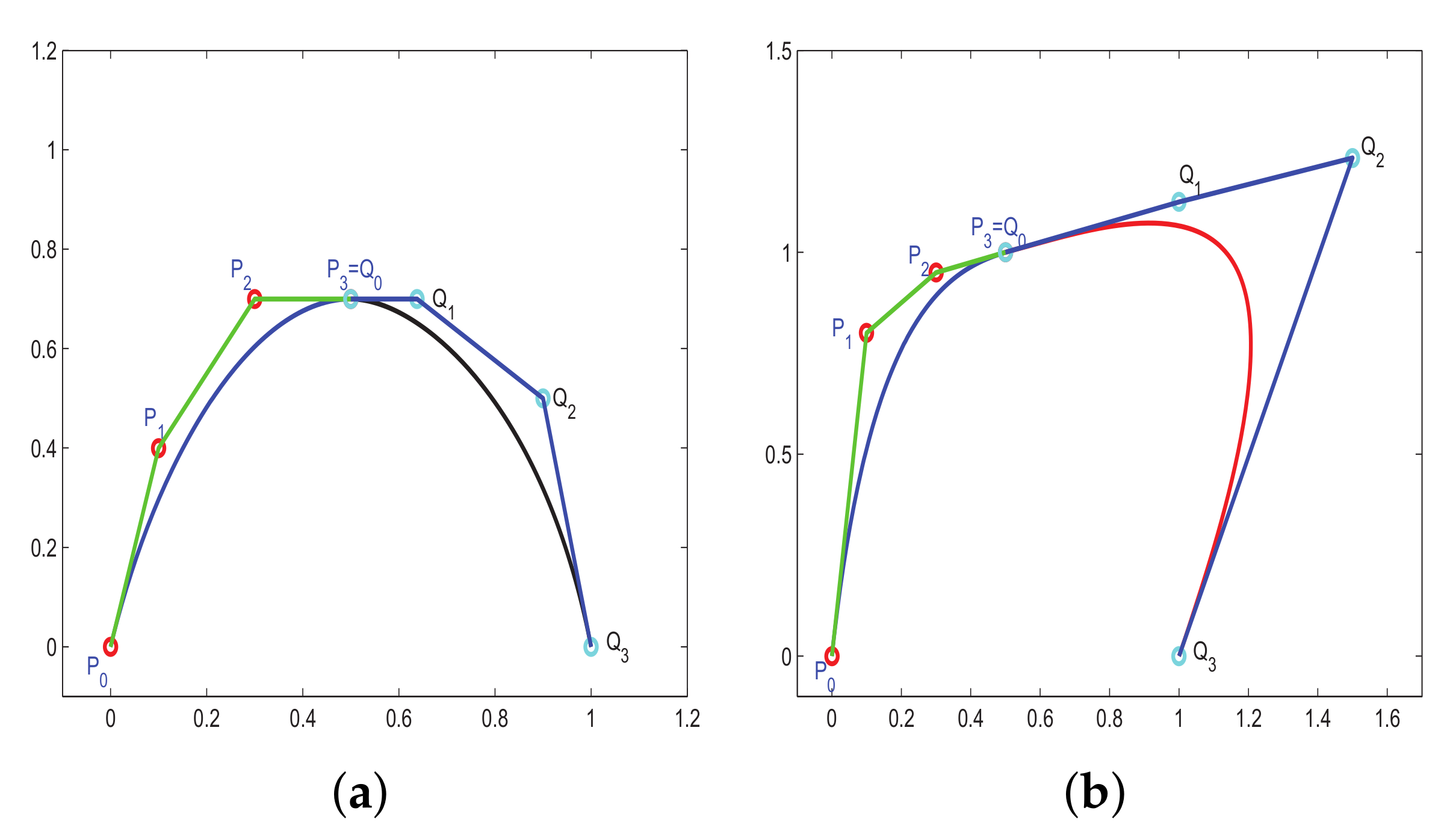
2.2. Curve Segment and Continuity
2.3. Parametric and Geometric Continuity
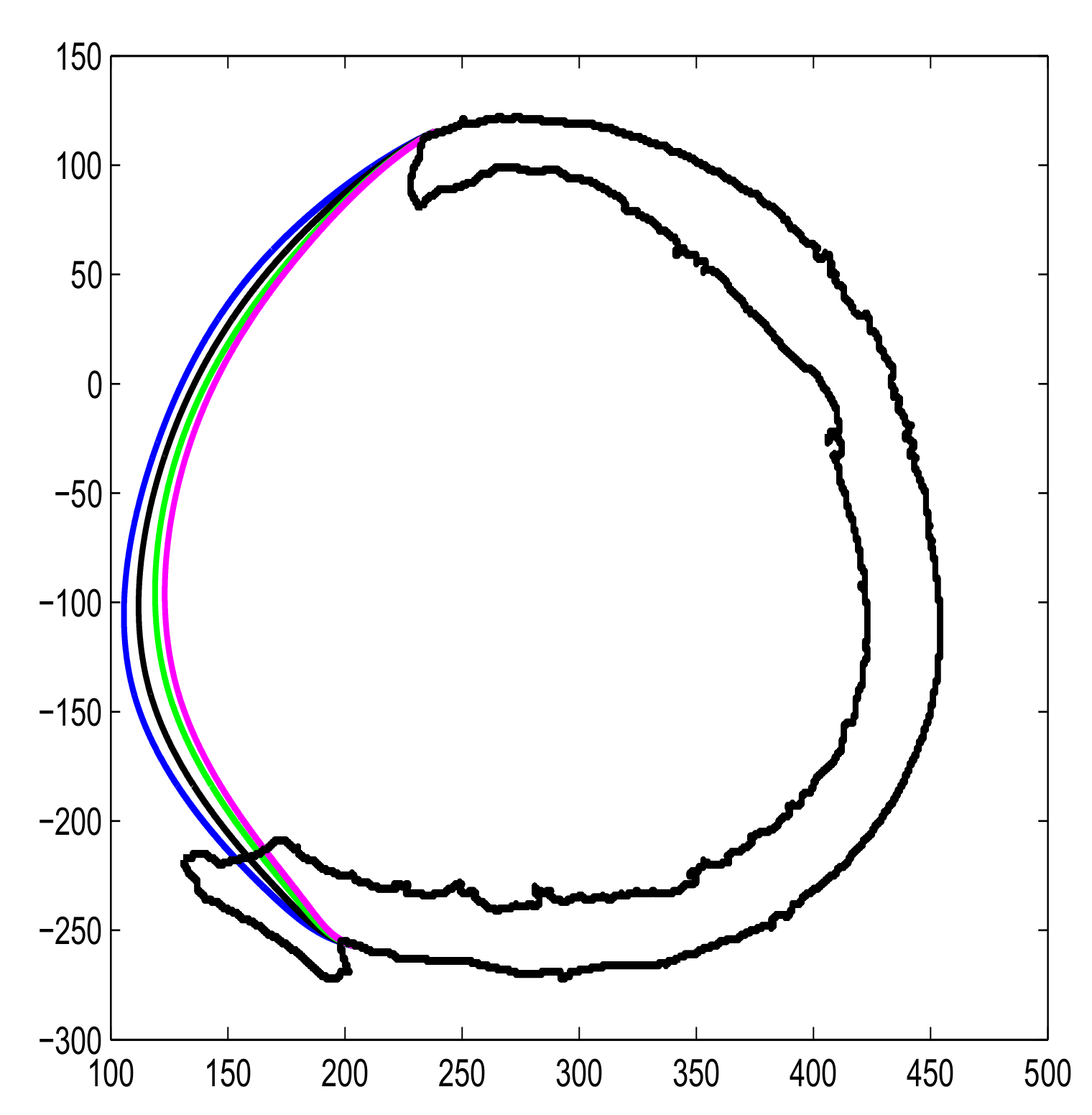
3. Comparative Analysis
3.1. Existing vs. Bézier-like Function Techniques
3.2. Comparison of Techniques Using Spline Curves
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
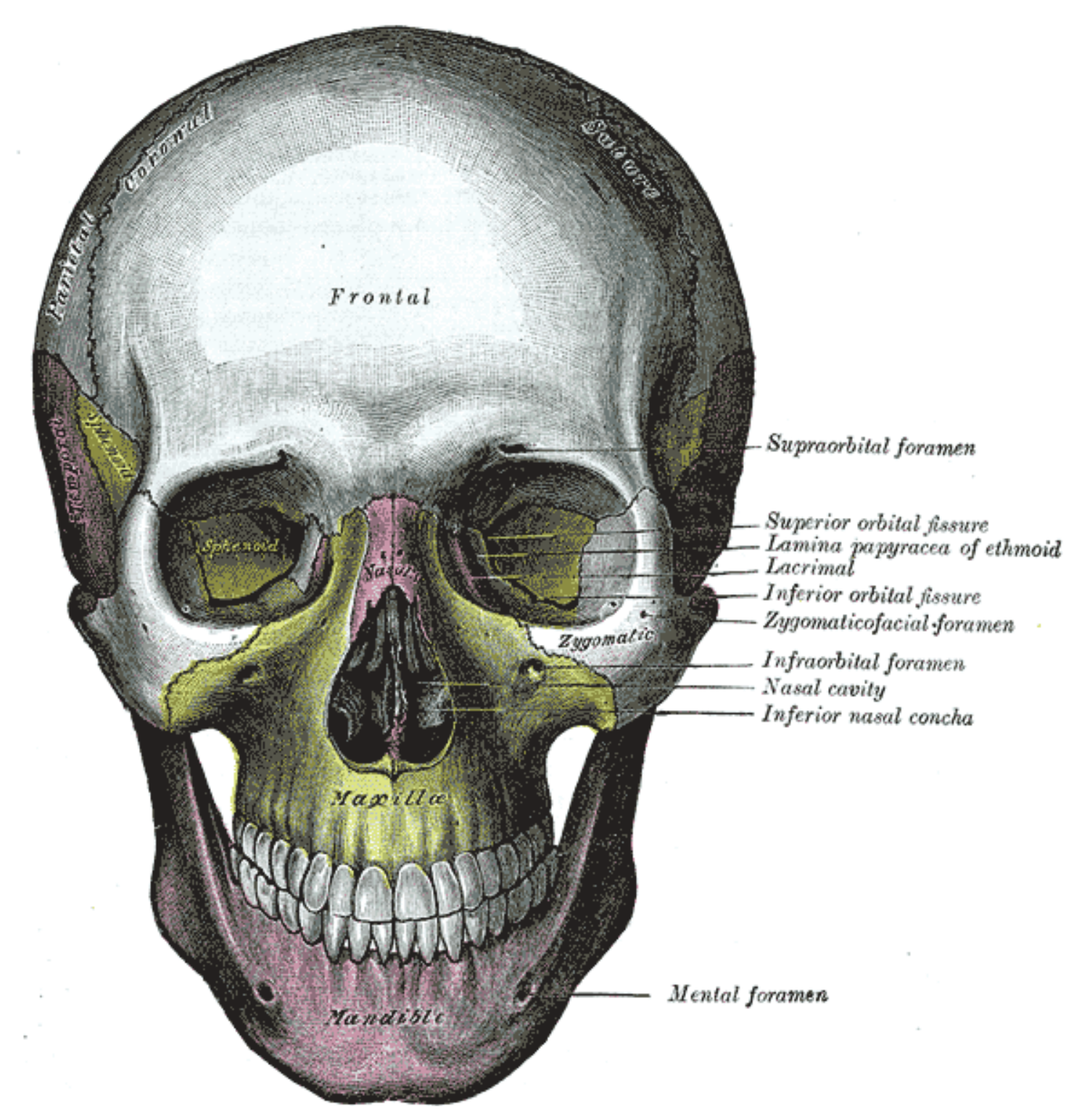
- Majeed, A.; Abbas, M.; Miura, K.T.; Kamran, M.; Nazir, T. Surface modeling from 2D contours with an application to craniofacial fracture construction. Mathematics 2020, 8, 1246. [Google Scholar] [CrossRef]
- Eufinger, H.; Wehmöller, M.; Machtens, E.; Heuser, L.; Harders, A.; Kruse, D. Reconstruction of craniofacial bone defects with individual alloplastic implants based on cad/cam-manipulated ct-data. J. Cranio-Maxillofac. Surg. 1995, 23, 175–181. [Google Scholar] [CrossRef]
- Müller, A.; Krishnan, K.G.; Uhl, E.; Mast, G. The application of rapid prototyping techniques in cranial reconstruction and preoperative planning in neurosurgery. J. Craniofac. Surg. 2003, 14, 899–914. [Google Scholar] [CrossRef] [PubMed]
- Majeed, A.; Abbas, M.; Qayyum, F.; Miura, K.T.; Misro, M.Y.; Nazir, T. Geometric modeling using new Cubic trigonometric B-Spline functions with shape parameter. Mathematics 2020, 8, 2102. [Google Scholar] [CrossRef]
- Sauret, V.; Linney, A.D.; Richards, R. Computer assisted surgery: The use of digital images in enabling computerized design and manufacture of titanium implants. Imaging 2002, 14, 464–471. [Google Scholar] [CrossRef]
- Min, K.J.; Dean, D. Highly accurate cad tools for cranial implants. In Proceedings of the 6th International Conference on Medical Image Computing and Computer-Assisted Intervention-MICCAI 2003, Montréal, QC, Canada, 15–18 November 2003; Randy, E.E., Terry, M.P., Eds.; Springer: Berlin/Heidelberg, Germany, 2003; pp. 99–107. [Google Scholar]
- Wu, T.; Engelhardt, M.; Fieten, L.; Popovic, A.; Radermacher, K. Anatomically constrained deformation for design of cranial implant: Methodology and validation. In Proceedings of the 9th International Conference on Medical Image Computing and Computer-Assisted Intervention–MICCAI 2006, Copenhagen, Denmark, 1–6 October 2006; Rasmus, L., Mads, N., Jon, S., Eds.; Springer: Berlin/Heidelberg, Germany, 2006; pp. 9–16. [Google Scholar]
- Shui, W.; Zhou, M.; Deng, Q.; Wu, Z.; Duan, F. 3D craniofacial reconstruction using reference skull-face database. In Proceedings of the 25th International Conference on Image and Vision Computing New Zealand (IVCNZ), Queenstown, New Zealand, 8–9 November 2010; pp. 1–7. [Google Scholar]
- Miyasaka, S.; Yoshino, M.; Imaizumi, K.; Seta, S. The computer-aided facial reconstruction system. Forensic Sci. Int. 1995, 74, 155–165. [Google Scholar] [CrossRef]
- Lee, T.Y.; Sum, Y.N.; Lin, Y.C.; Lin, L.; Lee, C. Three-dimensional facial model reconstruction and plastic surgery simulation. IEEE Trans. Inf. Technol. Biomed. 1999, 3, 214–220. [Google Scholar] [CrossRef] [PubMed]
- Majeed, A.; Mt Piah, A.R.; Gobithaasan, R.U.; Yahya, Z.R. Craniofacial Reconstruction Using Rational Cubic Ball Curves. PLoS ONE 2015, 10, e0122854. [Google Scholar] [CrossRef] [PubMed]
- Majeed, A.; Mt Piah, A.R.; Yahya, Z.R.; Abdullah, J.Y.; Rafique, M. Construction of occipital bone fracture using B-spline curves. Comput. Appl. Math. 2018, 37, 2877–2896. [Google Scholar] [CrossRef]
- Majeed, A.; Mt Piah, A.R. Maxillofacial Fracture Reconstruction Using GC2 Rational Cubic Ball Curves. In Proceedings of the 13th International Conference on Computer Graphics, Imaging and Visualization (CGiV), Beni Mellal City, Morocco, 29 March–1 April 2016; pp. 40–44. [Google Scholar]
- Majeed, A.; Rafique, M.; Abdullah, J.Y.; Rajion, Z.A. NURBS curves with the application of multiple bones fracture reconstruction. Appl. Math. Comput. 2017, 315, 70–84. [Google Scholar] [CrossRef]
- Archer, K.M. Craniofacial Reconstruction Using Hierarchical B-Spline Interpolation. Master’s Thesis, McGill University, Montreal, QC, Canada, 25 August 1997. [Google Scholar]
- Archer, K.M.; Coughlan, K.; Forsey, D.; Struben, S. Software tools for craniofacial growth and reconstruction. In Proceedings of the Graphics Interface, Vancouver, BC, Canada, 18–20 June 1998; pp. 73–81. [Google Scholar]
- Kang, L. Research on the Technique of Face Modeling Based on Skeletal Remains and Appliance to Forensic Facial Reconstruction. Master’s Thesis, Northwest University, Xi’an, China, 2006. [Google Scholar]
- Shui, W.; Zhou, M.; Wu, Z.; Deng, Q. An improved algorithm for craniofacial reconstruction based on landmarks registration. In Proceedings of the 2010 International Conference on Computer Application and System Modeling (ICCASM 2010), Taiyuan, China, 22–24 October 2010; Volume 5, pp. 498–502. [Google Scholar]
- Suetens, P.; Willems, G.; Vandermeulen, D.; De Greef, S.; Claes, P. Statistically deformable face models for cranio-facial reconstruction. J. Comput. Inf.-Technol. 2006, 1, 21–30. [Google Scholar]
- Turner, W.; Brown, R.; Kelliher, T.; Tu, P.; Taister, M.; Miller, K. A novel method of automated skull registration for forensic facial approximation. Forensic Sci. Int. 2005, 154, 149–158. [Google Scholar] [CrossRef] [PubMed]
- Claes, P.; Vandermeulen, D.; De Greef, S.; Willems, G.; Suetens, P. Craniofacial reconstruction using a combined statistical model of face shape and soft tissue depths: Methodology and validation. Forensic Sci. Int. 2006, 159, S147–S158. [Google Scholar] [CrossRef] [PubMed]
- Carr, J.C.; Beatson, R.K.; Cherrie, J.B.; Mitchell, T.J.; Fright, W.R.; McCallum, B.C.; Evans, T.R. Reconstruction and representation of 3D objects with radial basis functions. In Proceedings of the 28th Annual Conference on Computer Graphics and Interactive Techniques (SIGGRAPH’01), Los Angeles, CA, USA, 12–17 August 2001; pp. 67–76. [Google Scholar]
- Majeed, A.; Mt Piah, A.R.; Ridzuan, Y.Z. Surface Reconstruction from Parallel Curves with Application to Parietal Bone Fracture Reconstruction. PLoS ONE 2016, 11, e0149921. [Google Scholar] [CrossRef] [PubMed]
- Ali, J.M. An alternative derivation of said basis functions. Sains Malays. 1994, 23, 42–56. [Google Scholar]
- Ball, A.A. Consurf. part one: Introduction of the conic lofting tile. Comput. Aided Des. 1974, 6, 243–249. [Google Scholar] [CrossRef]
- Farin, G.; Josef, H.; Myung-Soo, K. Handbook of Computer Aided Geometric Design; Elsevier: Boston, MA, USA; London, UK; New York, NY, USA, 2002. [Google Scholar]
- Timmer, H.G. Alternative representation for parametric cubic curves and surfaces. Comput. Aided Des. 1980, 12, 25–28. [Google Scholar] [CrossRef]
- Majeed, A.; Yahya, Z.R.; Mt Piah, A.R.; Ahmad, M.Z. Reconstruction of Craniofacial Image using GC1 Cubic Ball Curves. Indian J. Sci. Technol. 2015, 8, 1–7. [Google Scholar] [CrossRef][Green Version]



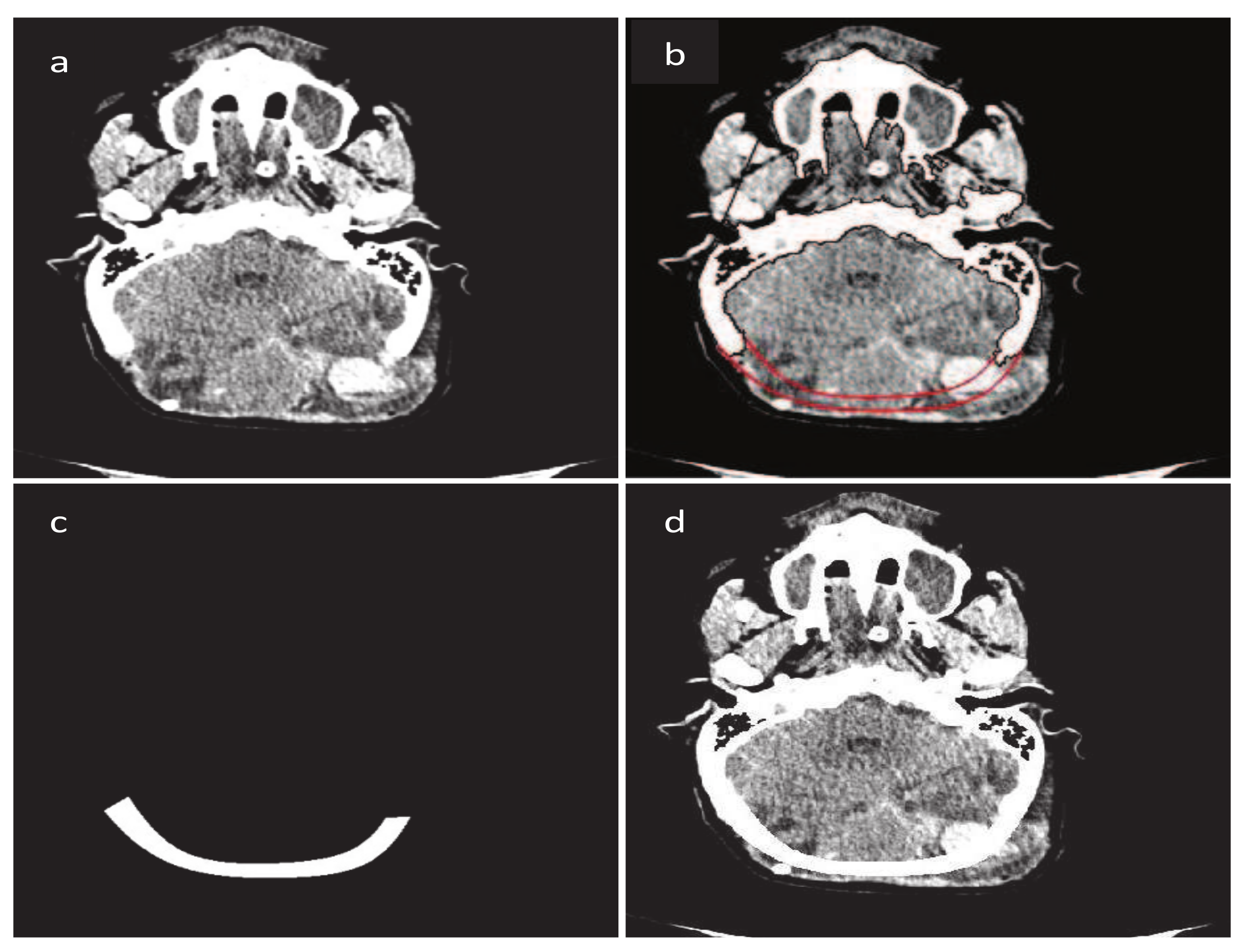
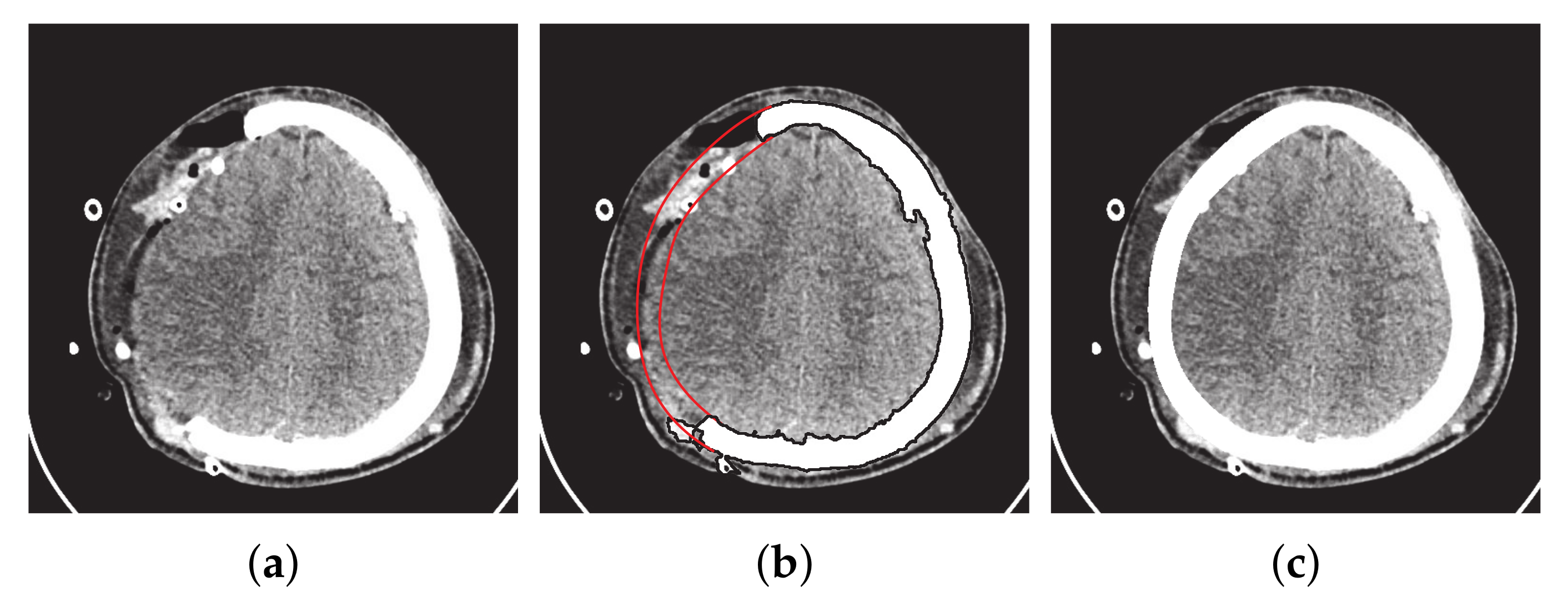
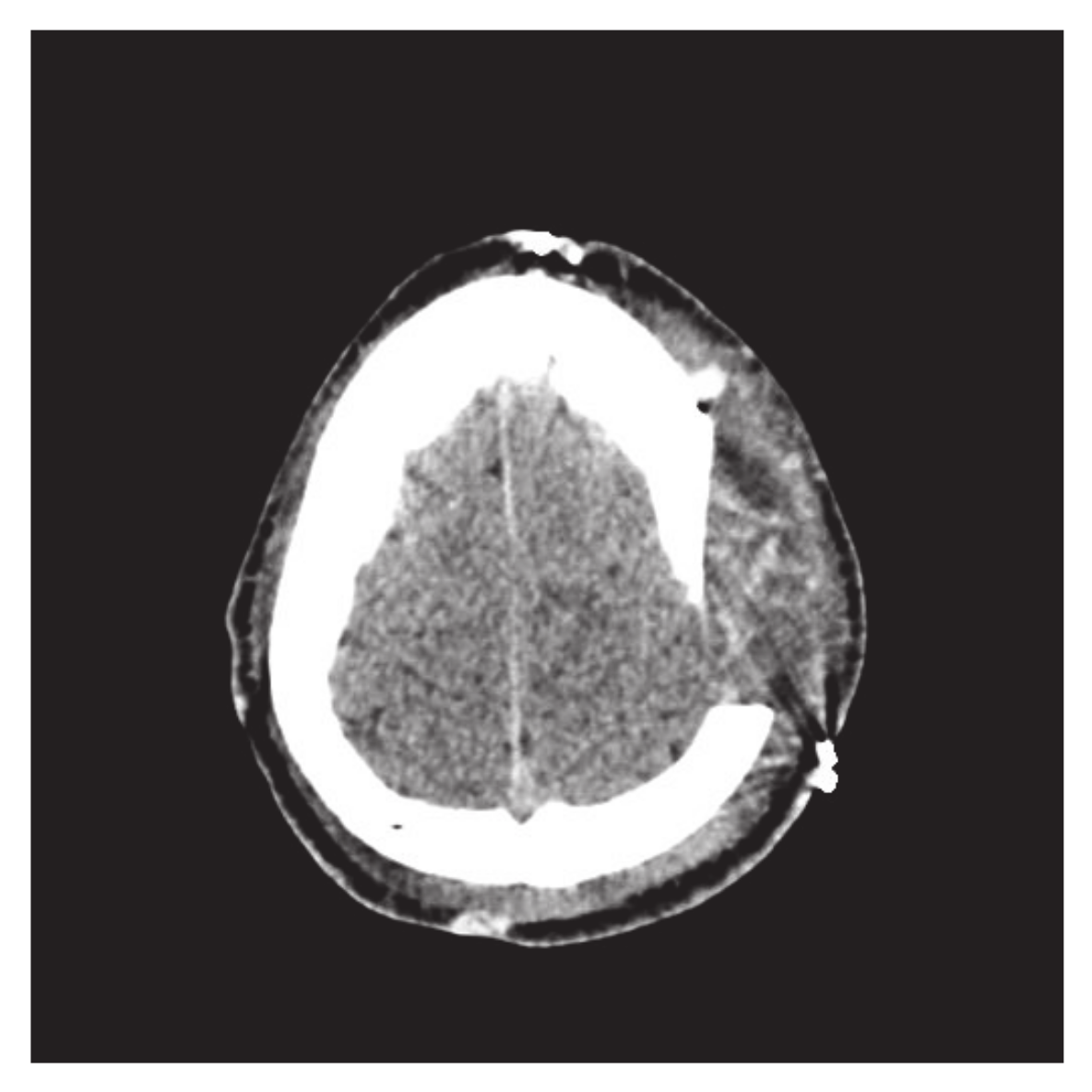
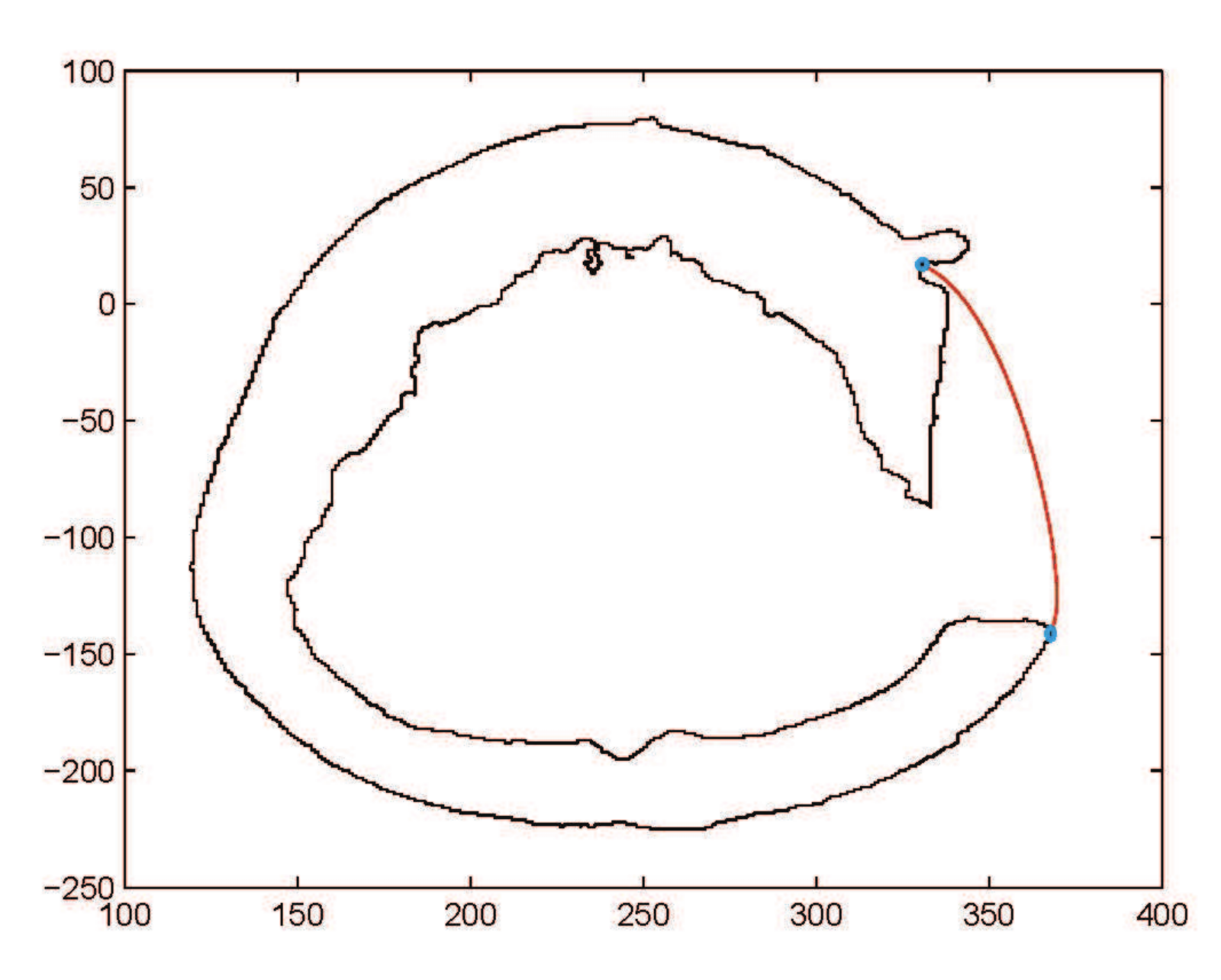
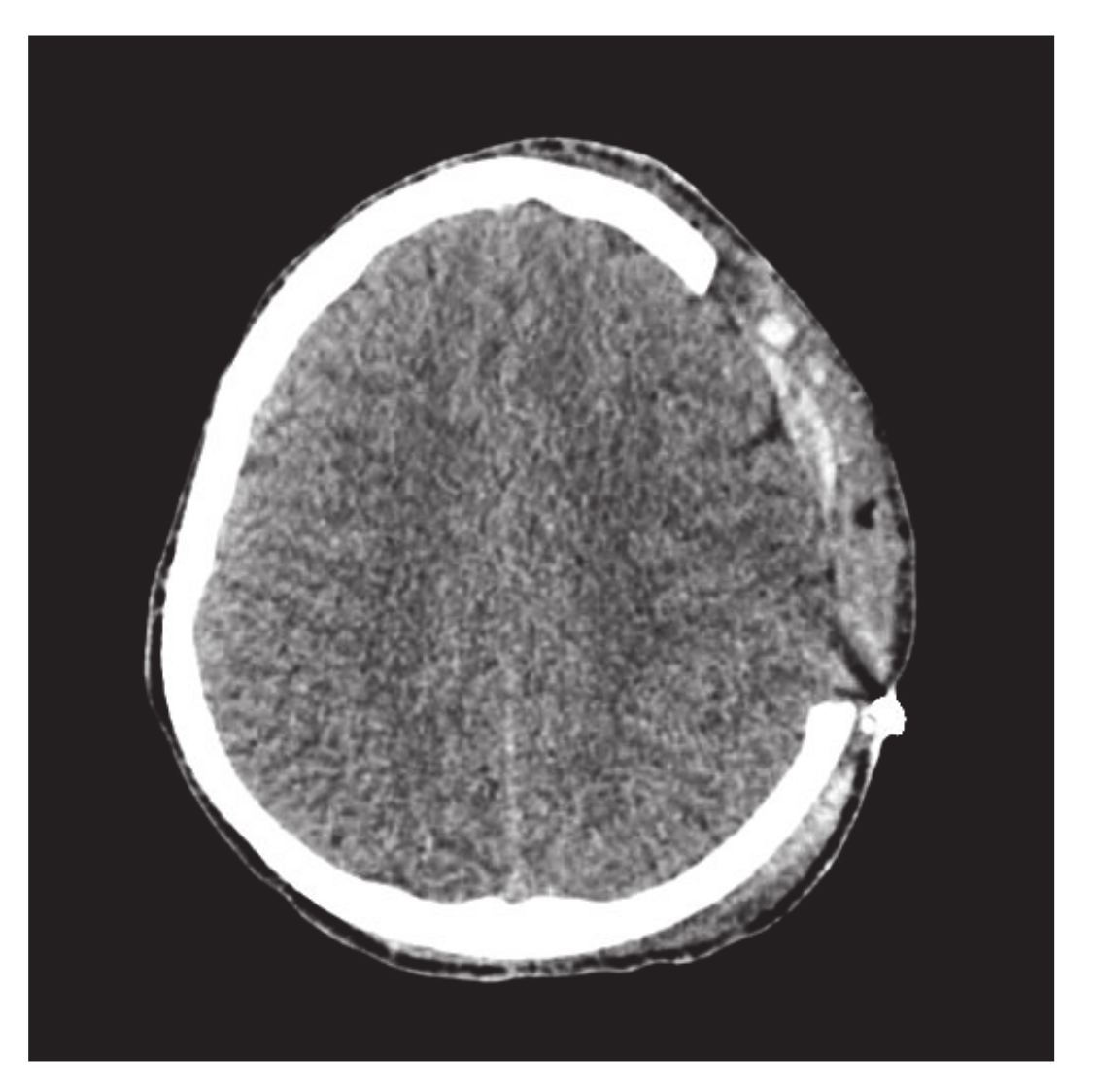
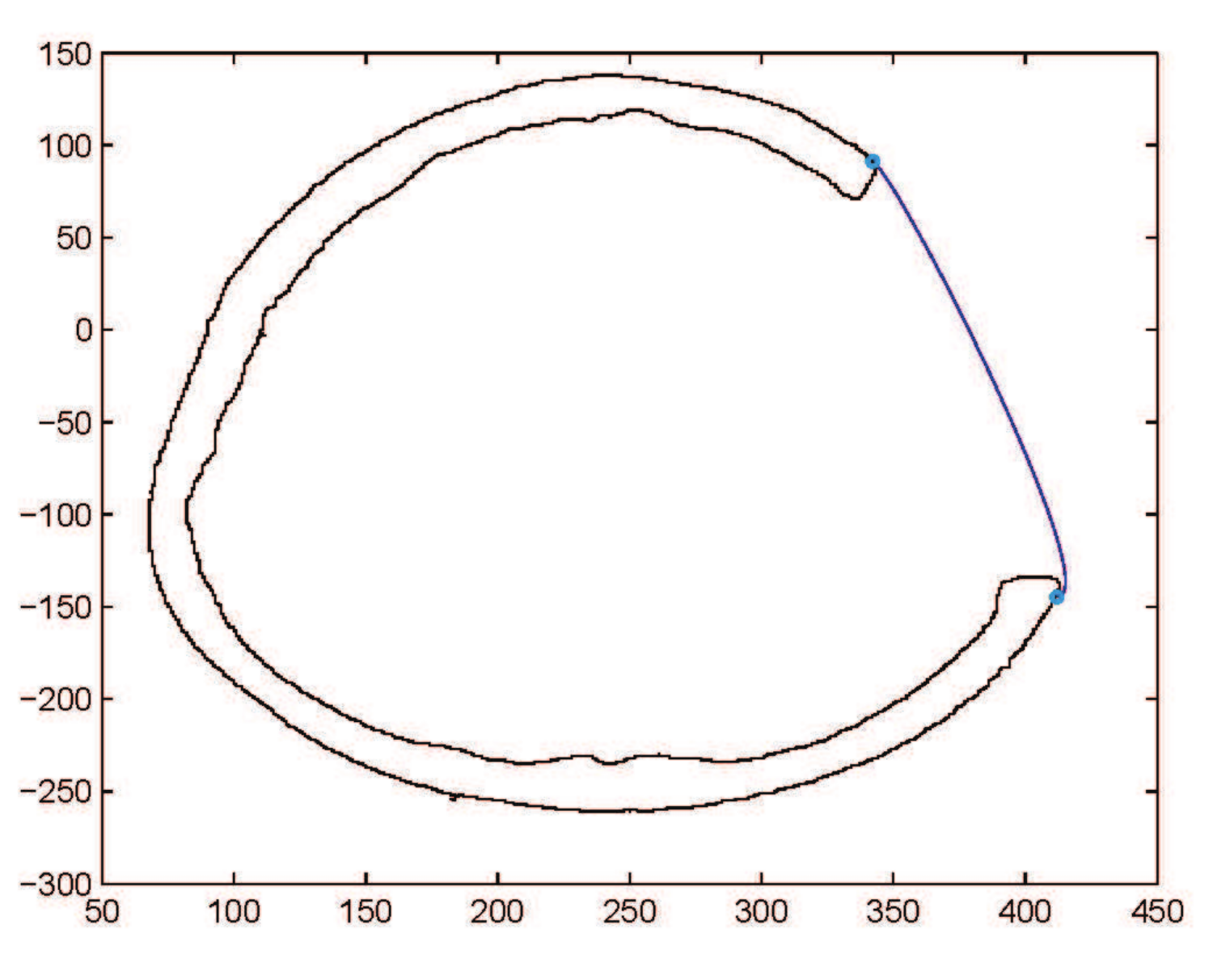
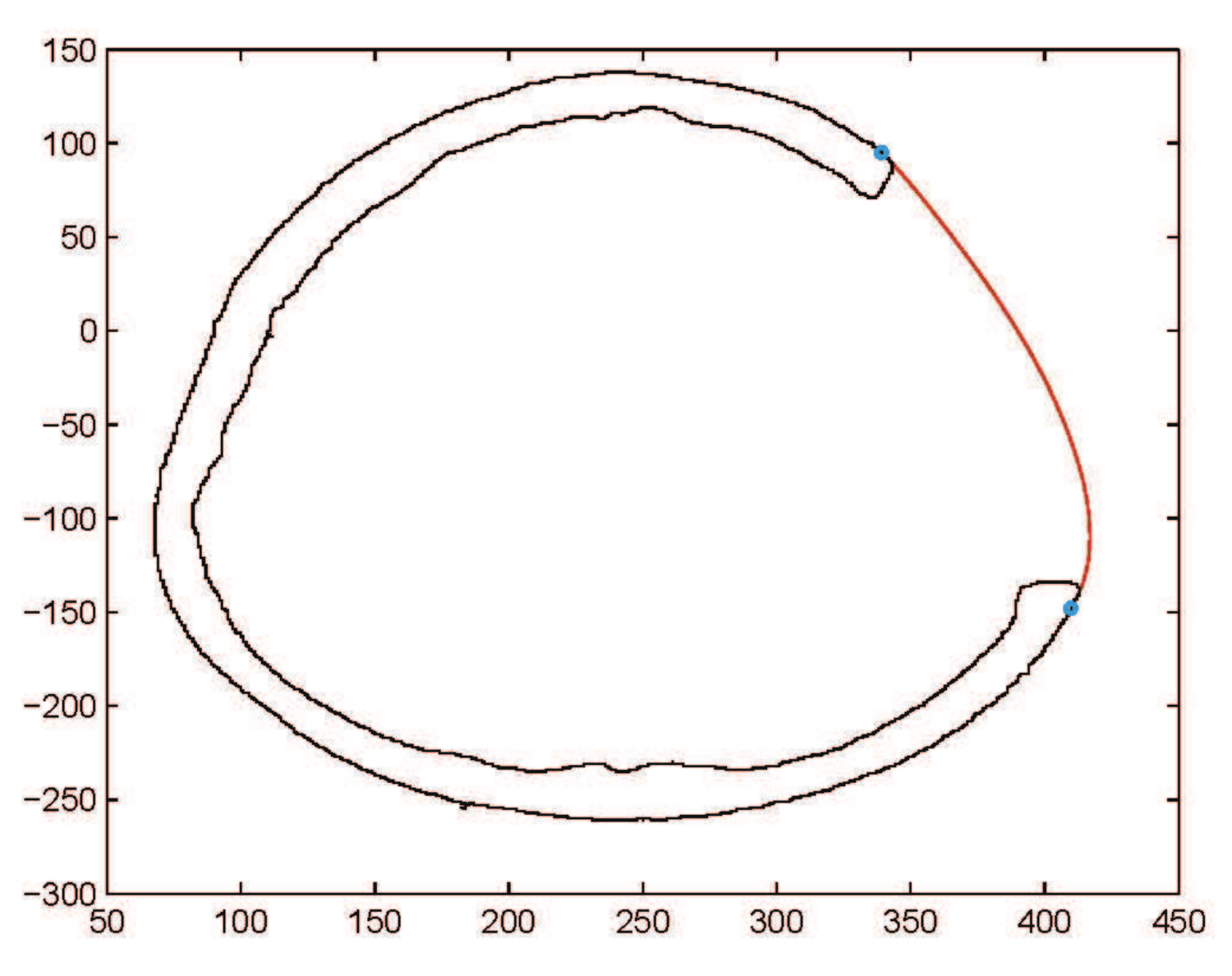
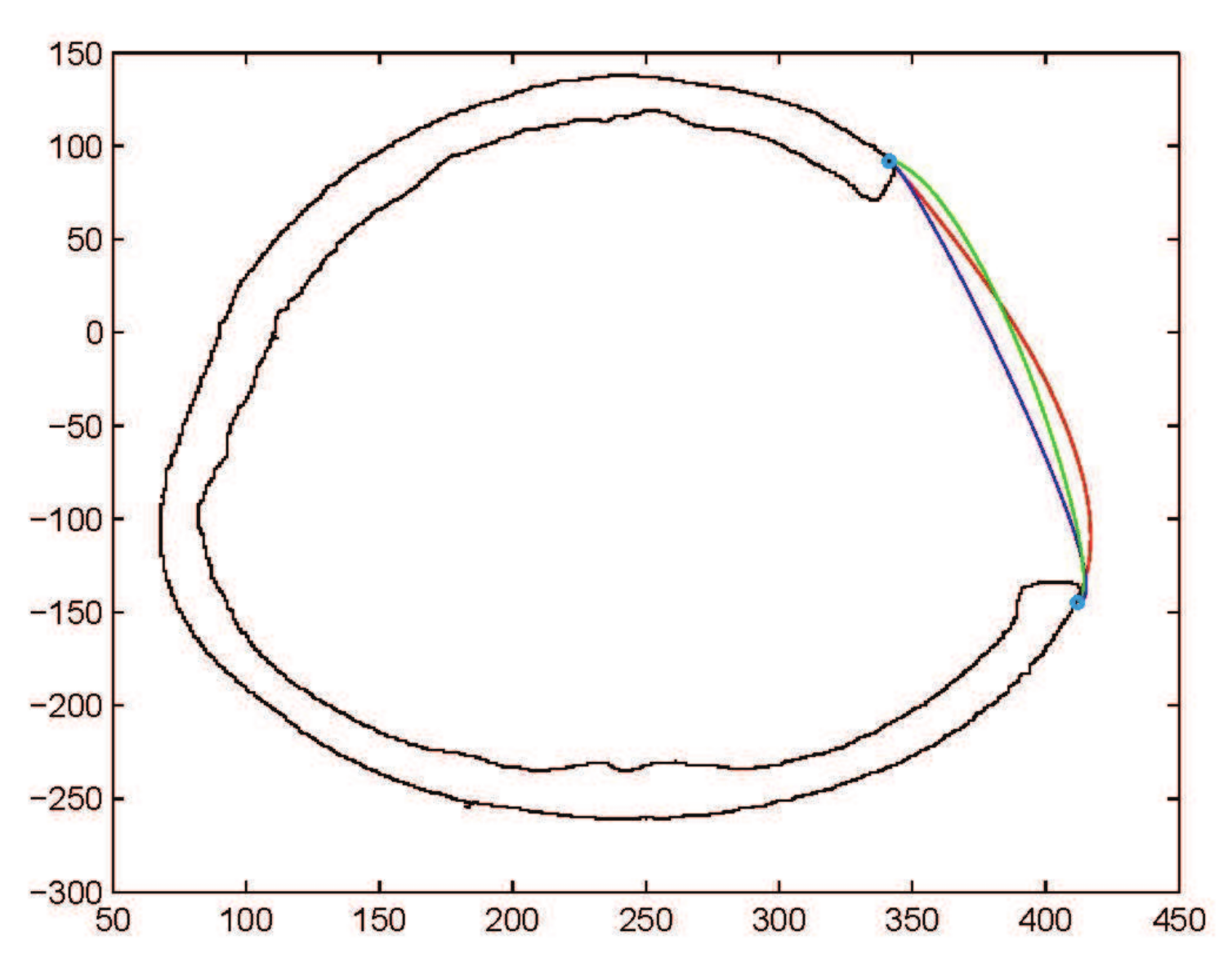
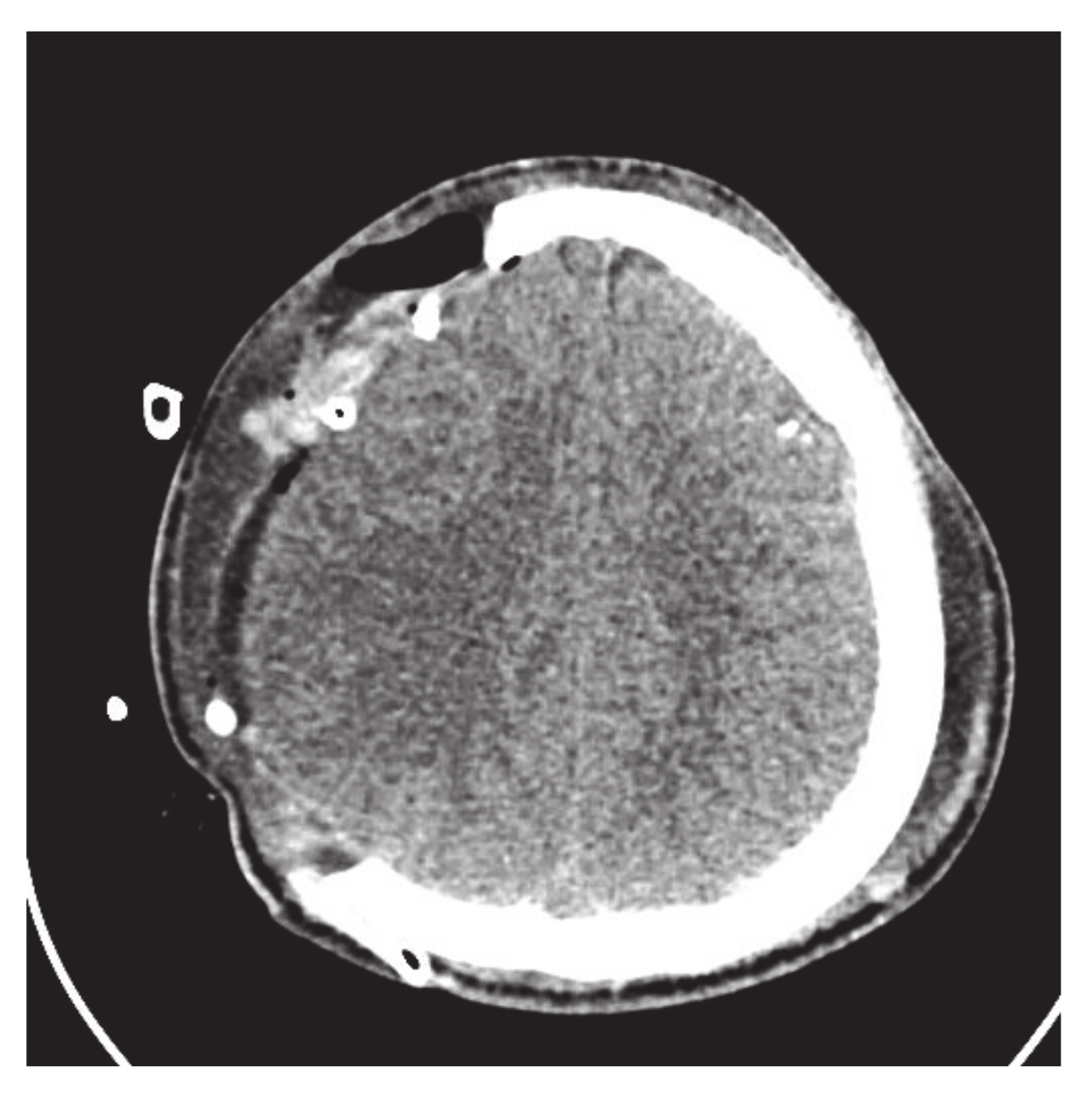


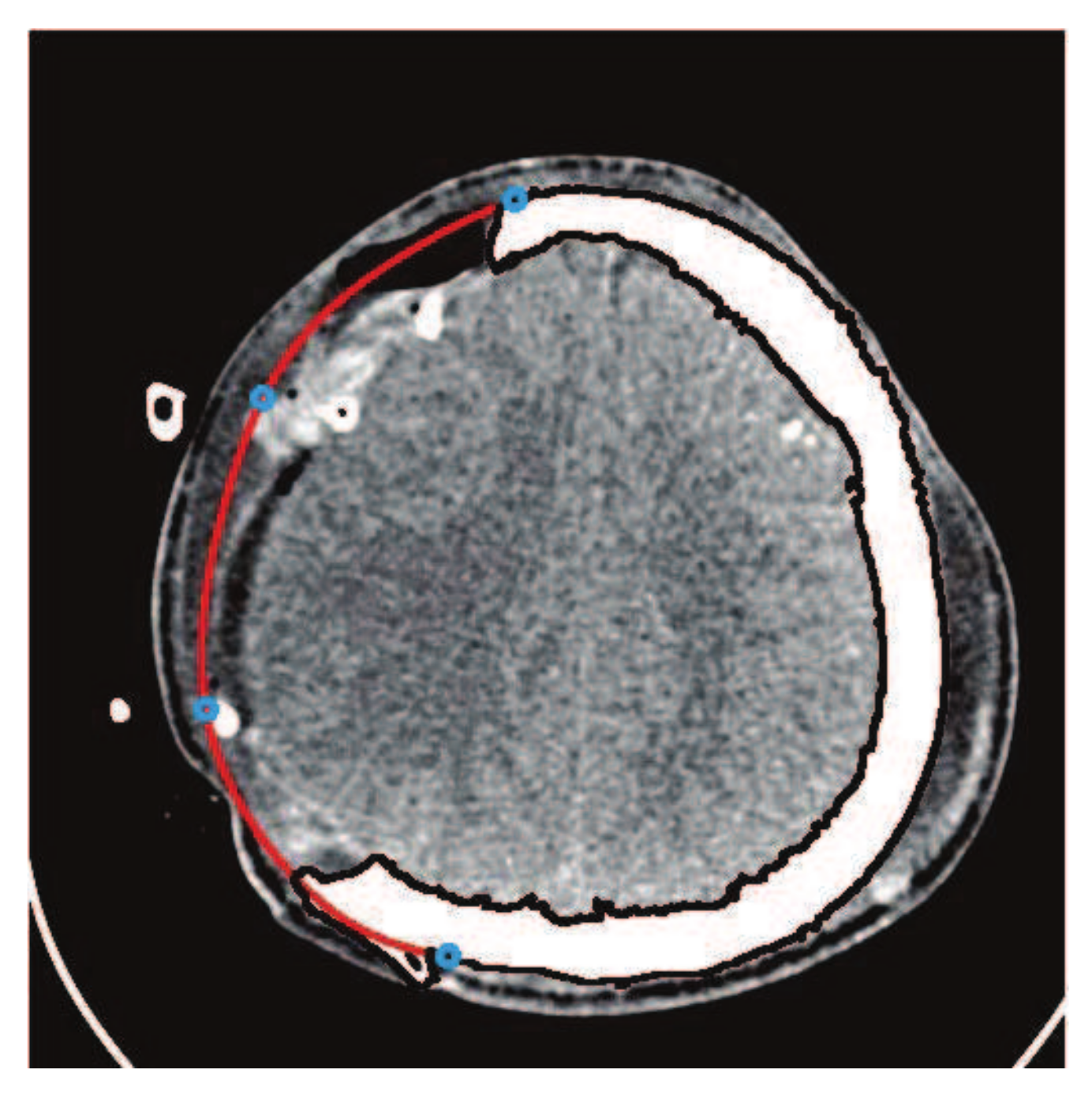
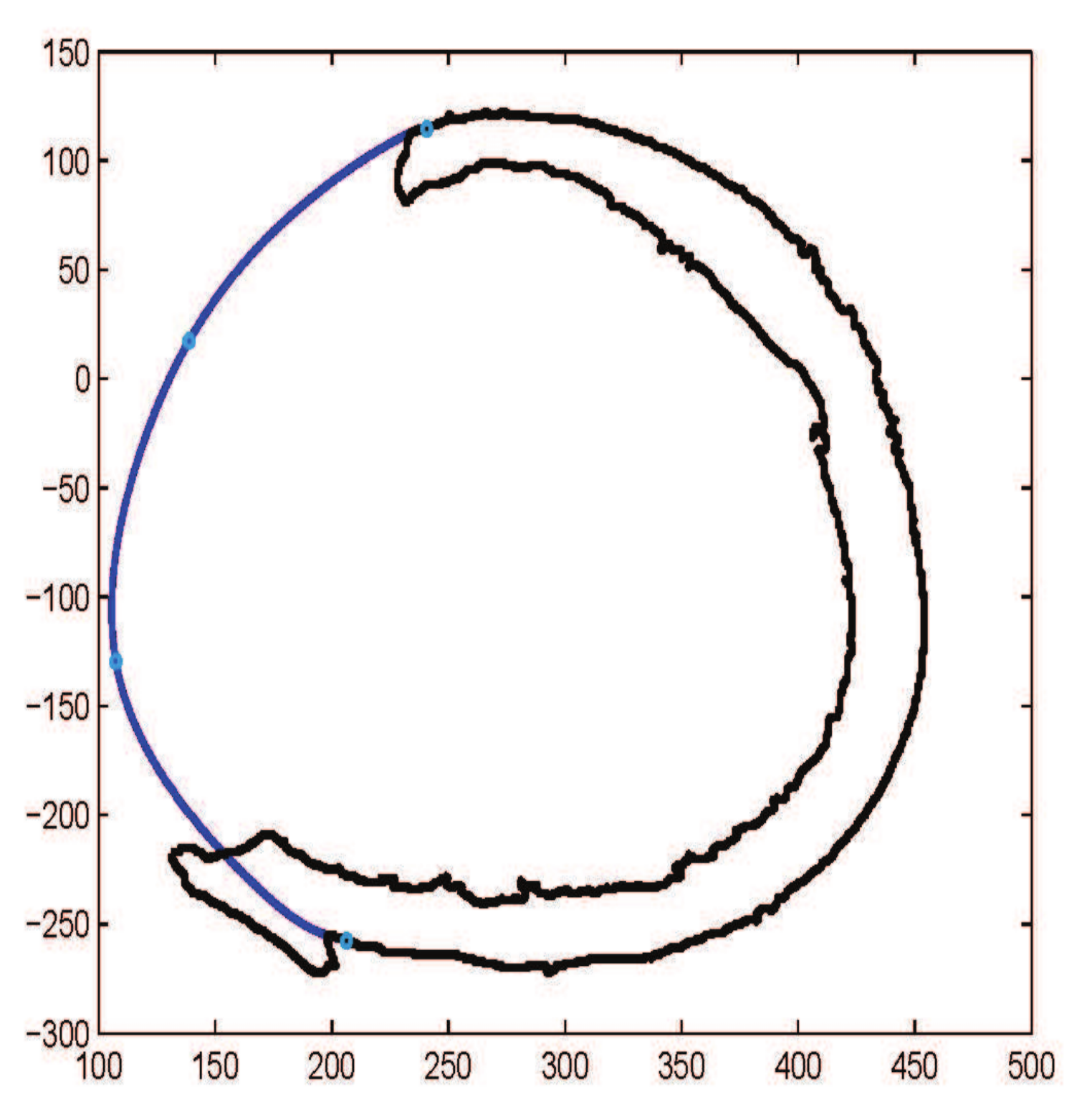
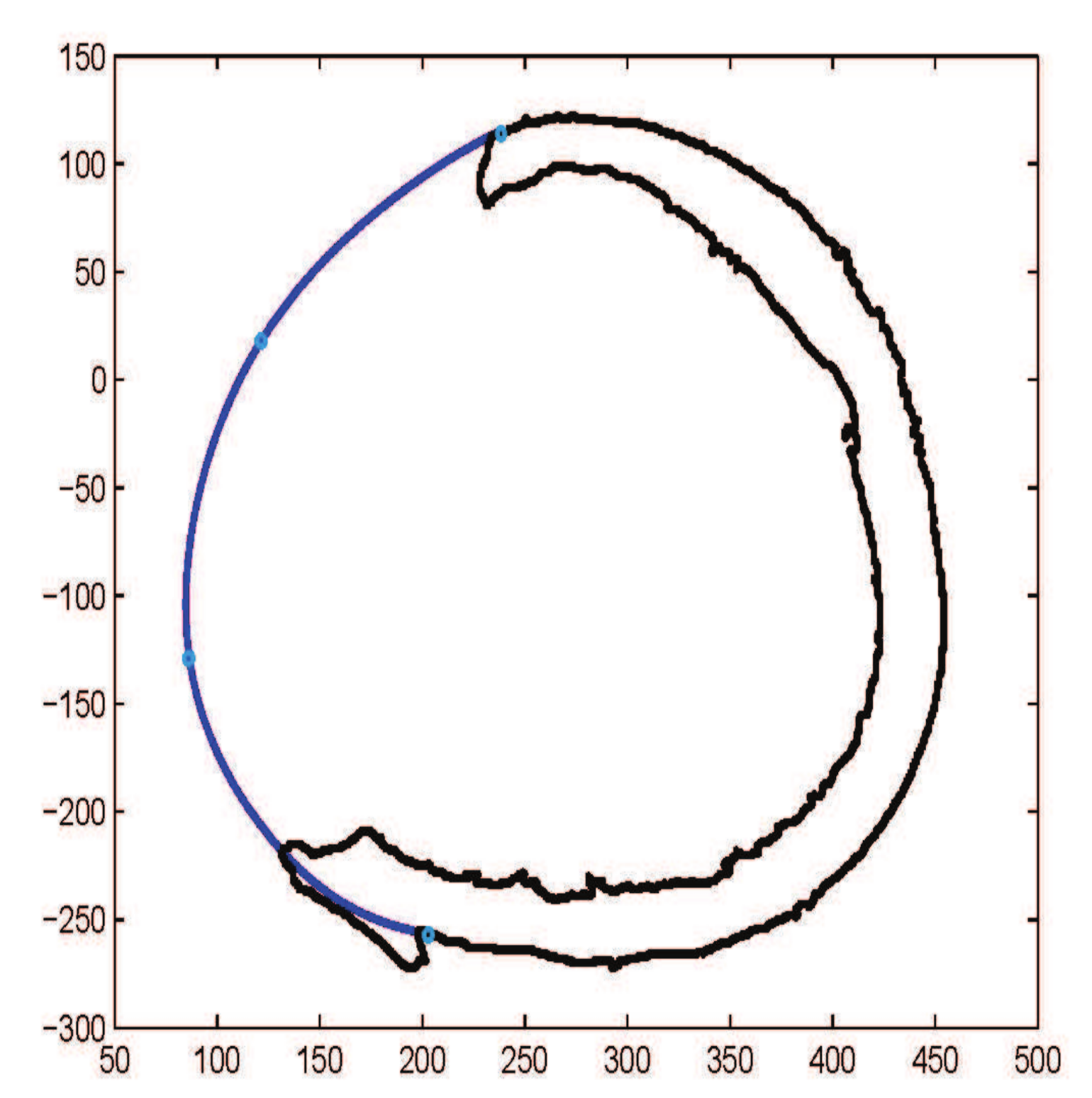
| Sr. No. | Parameter | Existing Methods | Methods Based on Bézier-like Functions |
|---|---|---|---|
| 1 | Technical staff | Refs. [2,3] used CAD/CAM process for craniofacial fracture reconstruction. It required technical staff/tools, which causes low efficiency and high cost | In this method, no need for staff/tools, reconstruction is based on patient CT scan DICOM data |
| 2 | Mirroring Method | Ref. [5] constructed the cranial part using the mirroring method. This method is not suitable for the patient with multiple bone fractures | The scheme based on spline functions is independent of mirroring and will work for any type of fractures. |
| 3 | Reference skull | Refs. [7,8] used adaptive deformation method for reconstruction. This scheme is dependent on reference skull | There is no need to take the reference skull using this method and reconstruction is based on CT scan data. |
| 4 | Thin plate spline | Ref. [19] proposed the thin plate spline and it is also based on the reference skull | Proposed method is independent of reference skull, construction will start directly using patient data. |
| 5 | Radial basis function | Radial basis functions have been used by [22]. In this method, authors consider the average thickness of bone and use a large number of data points | In this scheme, the user can attain the required thickness of the skull within a contour. |
| 6 | Iterative closest point | Ref. [20] used iterative closest point (ICP) algorithm to reconstruct the mandible bone fracture using non-fractured contour as a reference point | This scheme is independent of the reference skull. The reconstructed fractured part can be controlled and adjusted by shape parameters in the proposed method. The reconstructed implant is custom-made for each individual patient; thus, it is time saving and efficient. |
| Sr. No. | Curve | Number of Control Points Used | Number of Shape Parameters Used | Computational Time in Sec |
|---|---|---|---|---|
| 1 | Ball curve | 10 | 9 | 0.057 |
| 2 | Ball curve | 10 | 12 | 0.067 |
| 3 | B-spline curve | 6 | 0 | 0.034 |
| 4 | NURBS curve | 6 | 6 | 0.043 |
| Sr. No. | Bézier-like Curve | B-Spline | NURBS Curve |
|---|---|---|---|
| 1 | Interpolated curve | Approximated curve | Interpolated curve |
| 2 | Global control | Local control | Local control |
| 3 | Need to develop the continuity between two curve segments | No need to develop continuity; gives continuity | No need to develop continuity; gives continuity. |
| 4 | Need 4 control points for 1 curve segment | Need 4 control points for 1 curve segment | Need 4 control points for 1 curve segment |
| 5 | Need 7 and 10 control points for 2 and 3 curve segments, respectively | Need 5 and 6 control points for 2 and 3 curve segments, respectively | Need 5 and 6 control points for 2 and 3 curve segments, respectively |
| 6 | Need two shape parameter for 1 curve segment | No need for shape parameter | Need 4 weights (shape parameter for 1 segment) |
| 7 | needs 4 shape parameters for 2 curve segments, needs 6 and needs 8 | B-spline is independent of shape parameters. Control points are the only way to handle the curve | Need 5 control points for 2 consecutive curve segments |
| 8 | Need to find intermediate control points using different techniques | The control points can be evaluated by using knots and B-spline basis | The control points can be evaluated by using knots and b-spline basis |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Majeed, A.; Abbas, M.; Miura, K.T. A Comparative Study of Different Schemes Based on Bézier-like Functions with an Application of Craniofacial Fractures Reconstruction. Mathematics 2022, 10, 1269. https://doi.org/10.3390/math10081269
Majeed A, Abbas M, Miura KT. A Comparative Study of Different Schemes Based on Bézier-like Functions with an Application of Craniofacial Fractures Reconstruction. Mathematics. 2022; 10(8):1269. https://doi.org/10.3390/math10081269
Chicago/Turabian StyleMajeed, Abdul, Muhammad Abbas, and Kenjiro T. Miura. 2022. "A Comparative Study of Different Schemes Based on Bézier-like Functions with an Application of Craniofacial Fractures Reconstruction" Mathematics 10, no. 8: 1269. https://doi.org/10.3390/math10081269
APA StyleMajeed, A., Abbas, M., & Miura, K. T. (2022). A Comparative Study of Different Schemes Based on Bézier-like Functions with an Application of Craniofacial Fractures Reconstruction. Mathematics, 10(8), 1269. https://doi.org/10.3390/math10081269








