Modeling and Fatigue Characteristic Analysis of the Gear Flexspline of a Harmonic Reducer
Abstract
:1. Introduction
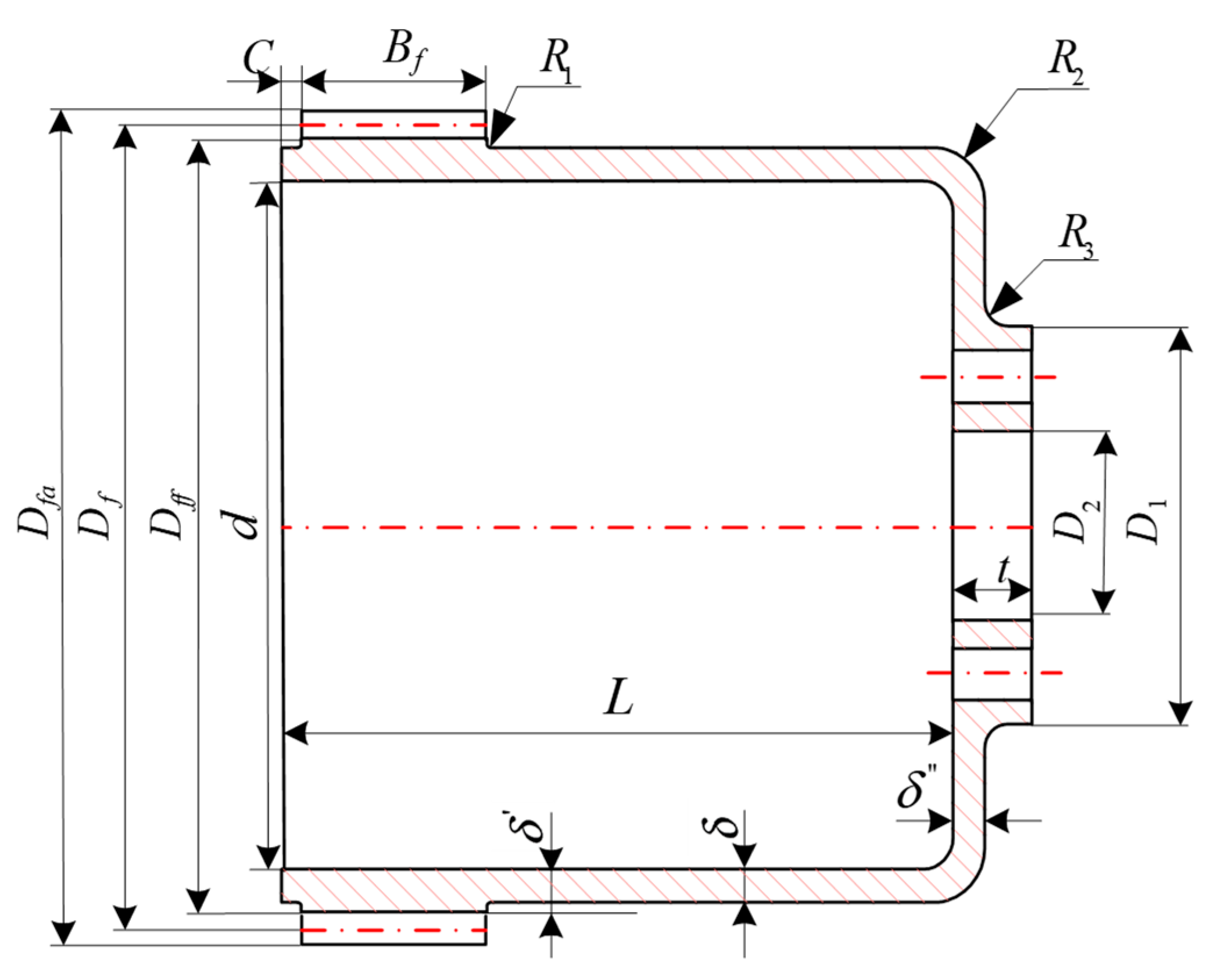
2. Structure Design and Model Establishment of a Harmonic Gear Reducer Flexible Wheel
3. Verification of the Finite Element Model
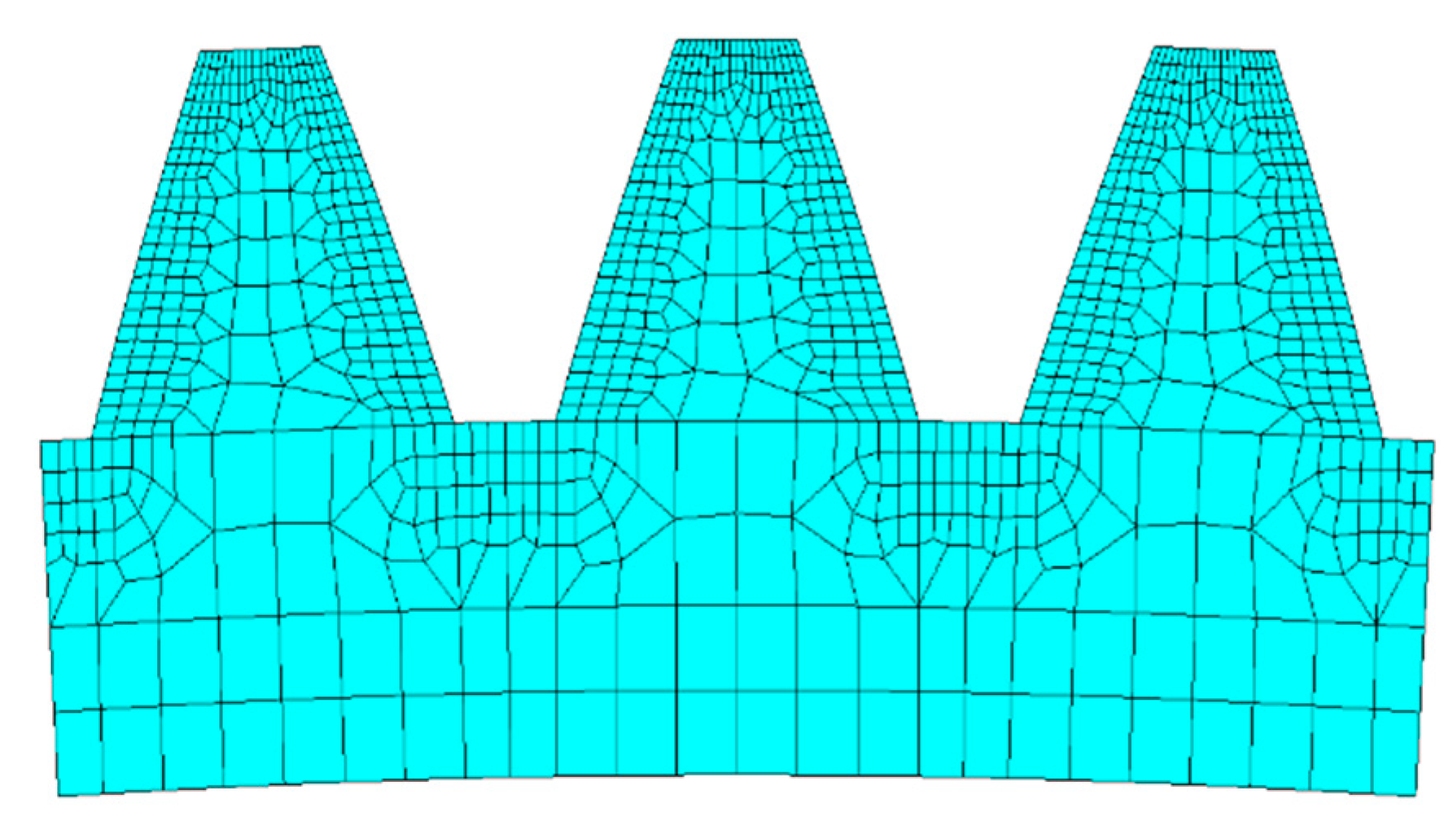
3.1. Simplification of the Mesh Model of the Flexspline Tooth Profile
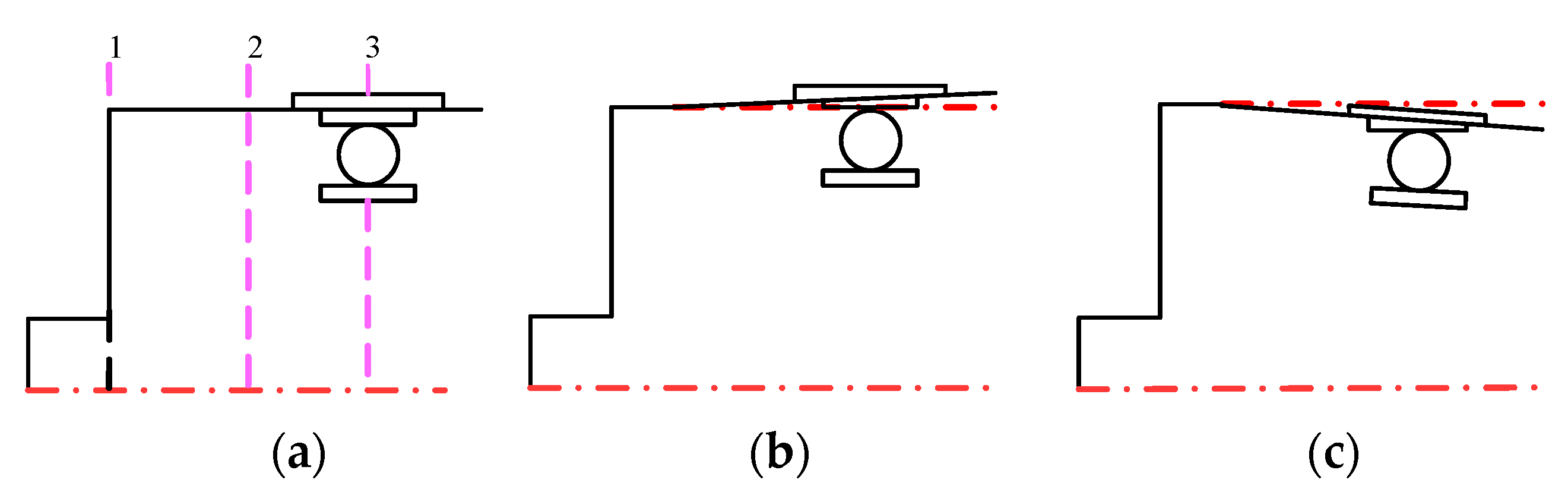
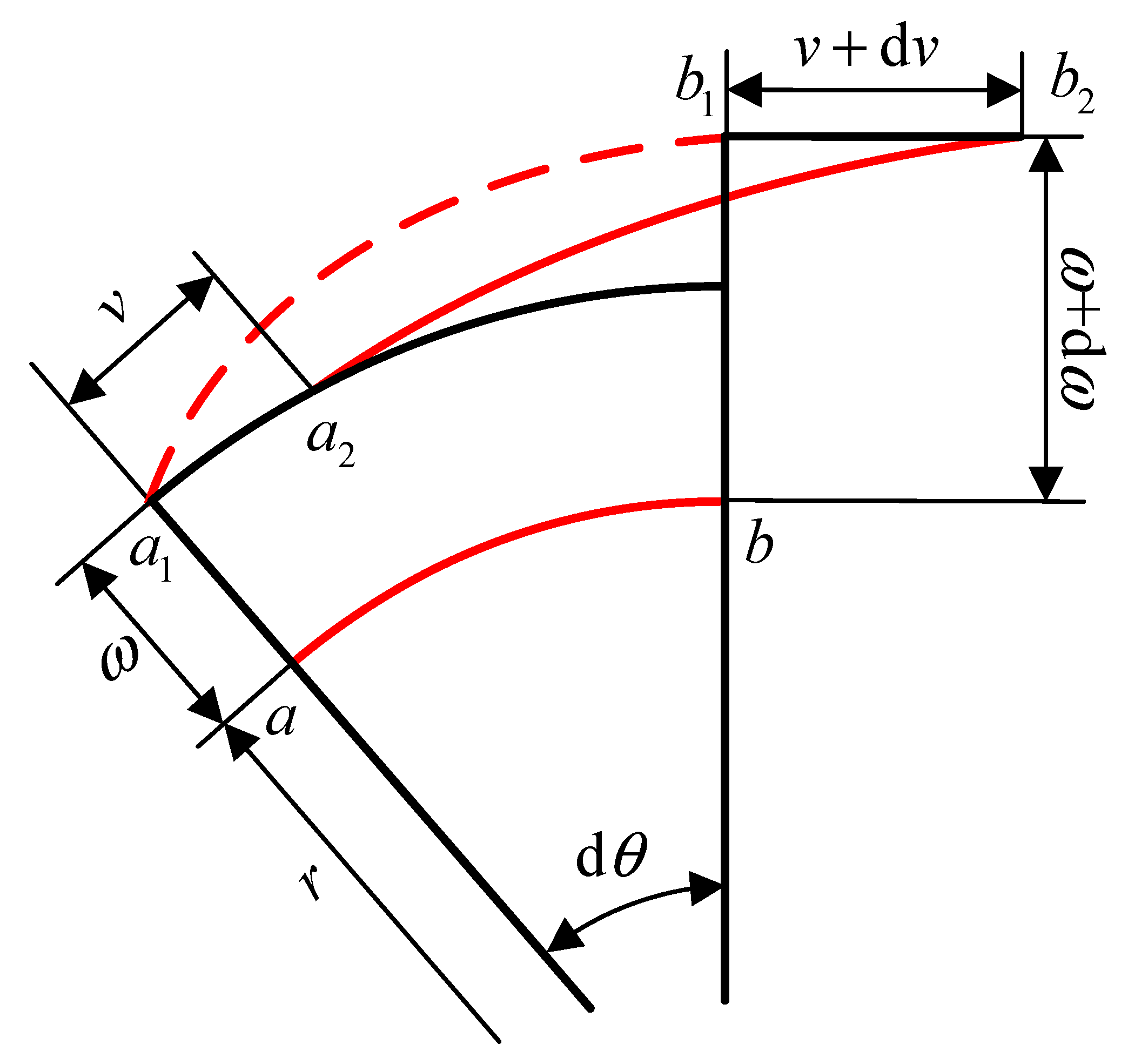
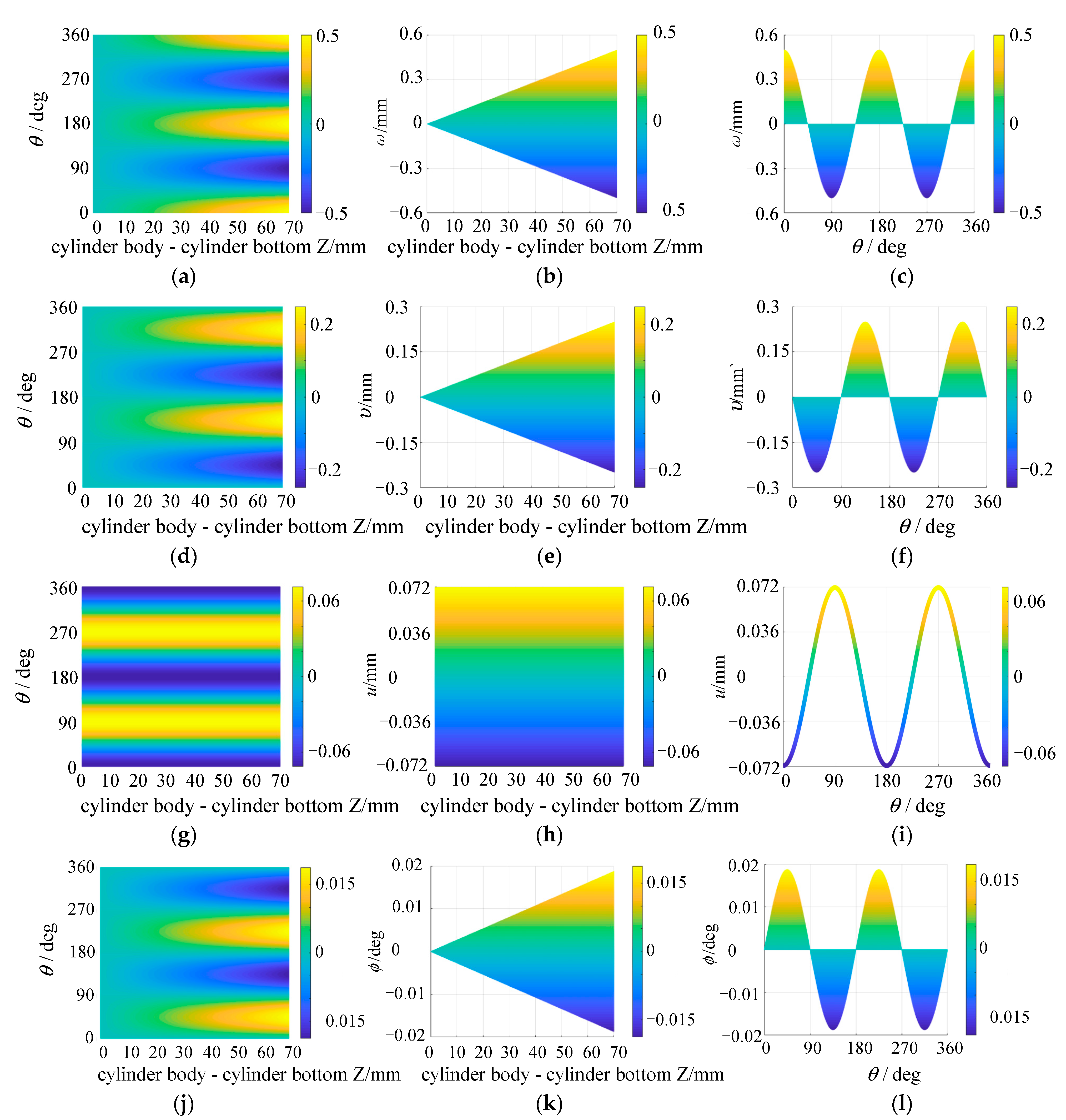
3.2. Mechanical Analysis of the Flexspline under Cosine Cam
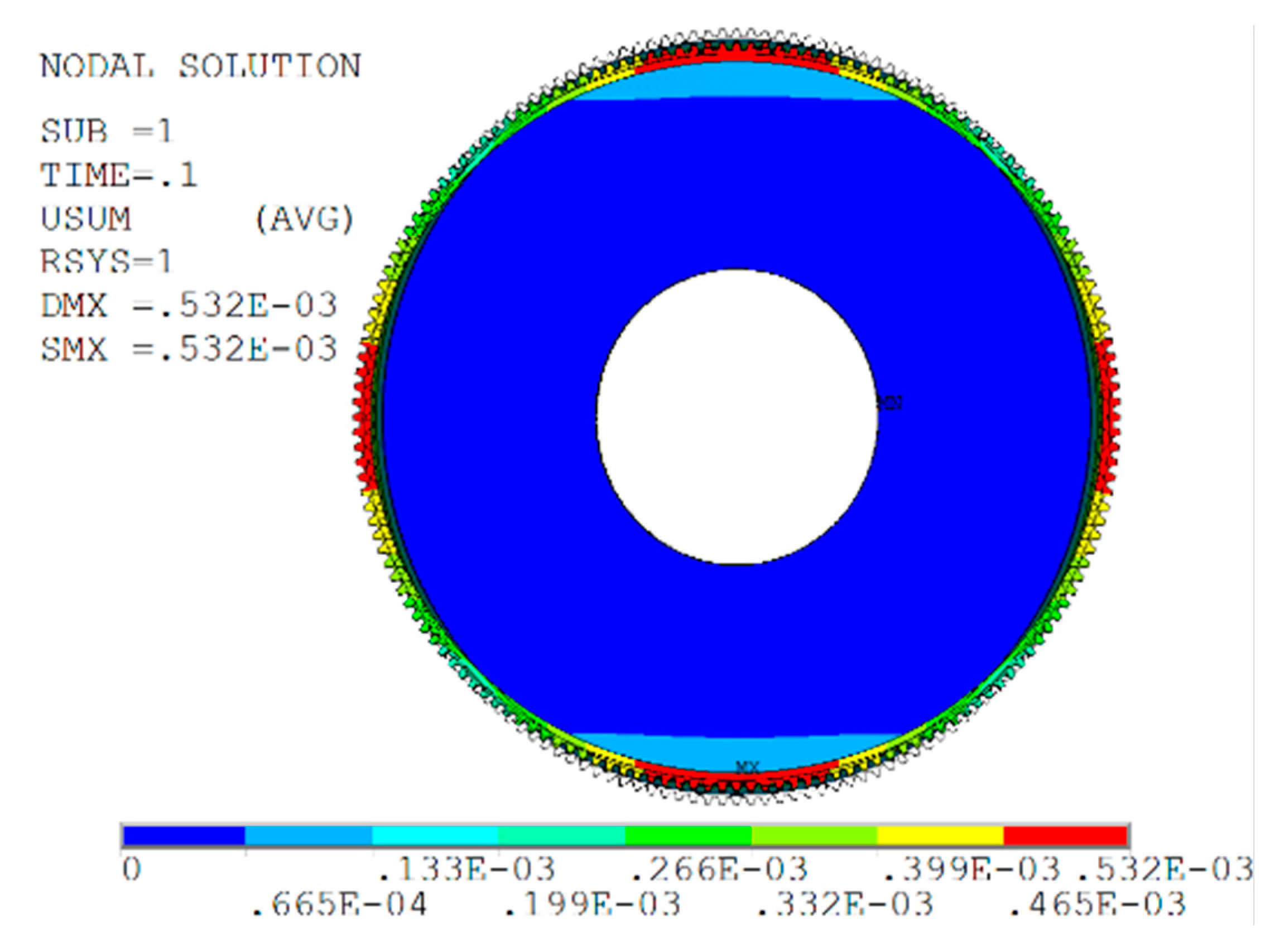
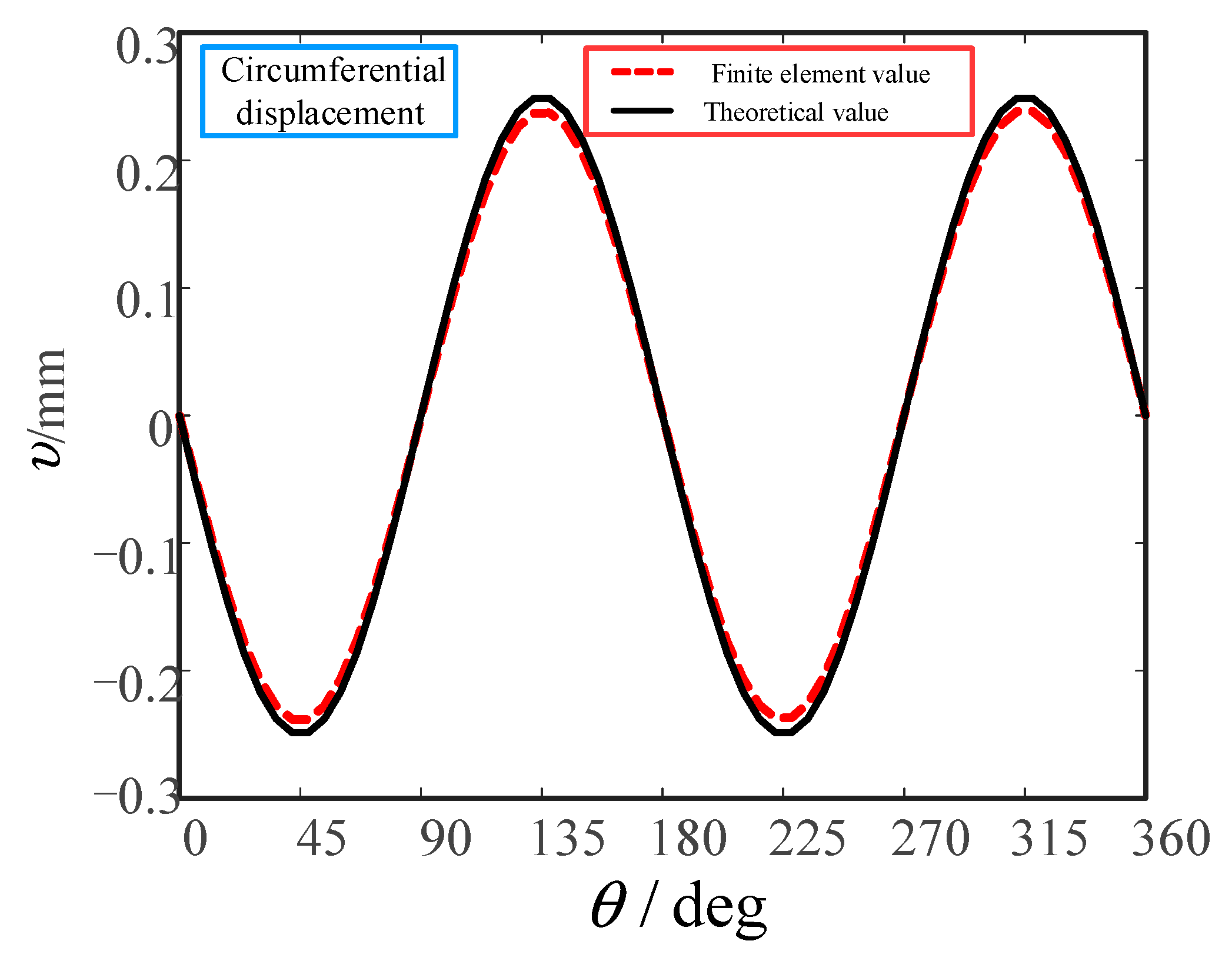
3.3. Comparison between Simulation Results and Theoretical Calculations
4. Sensitivity Calculation of Stress Characteristics and Fatigue Life Analysis of the Flexspline
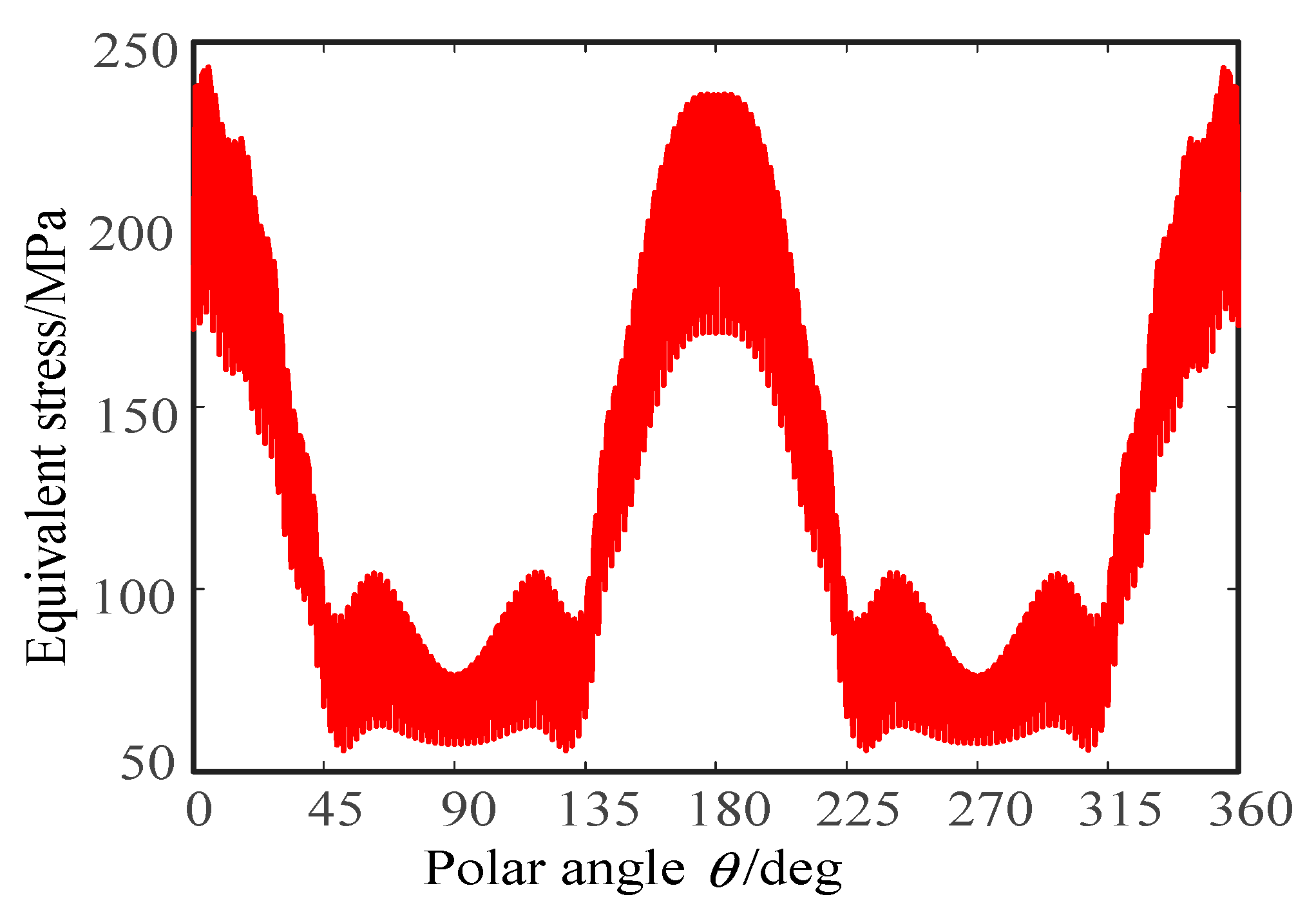
4.1. Calculation of Meshing Force of the Flexspline Teeth
4.2. Fatigue Life Analysis of the Flexspline
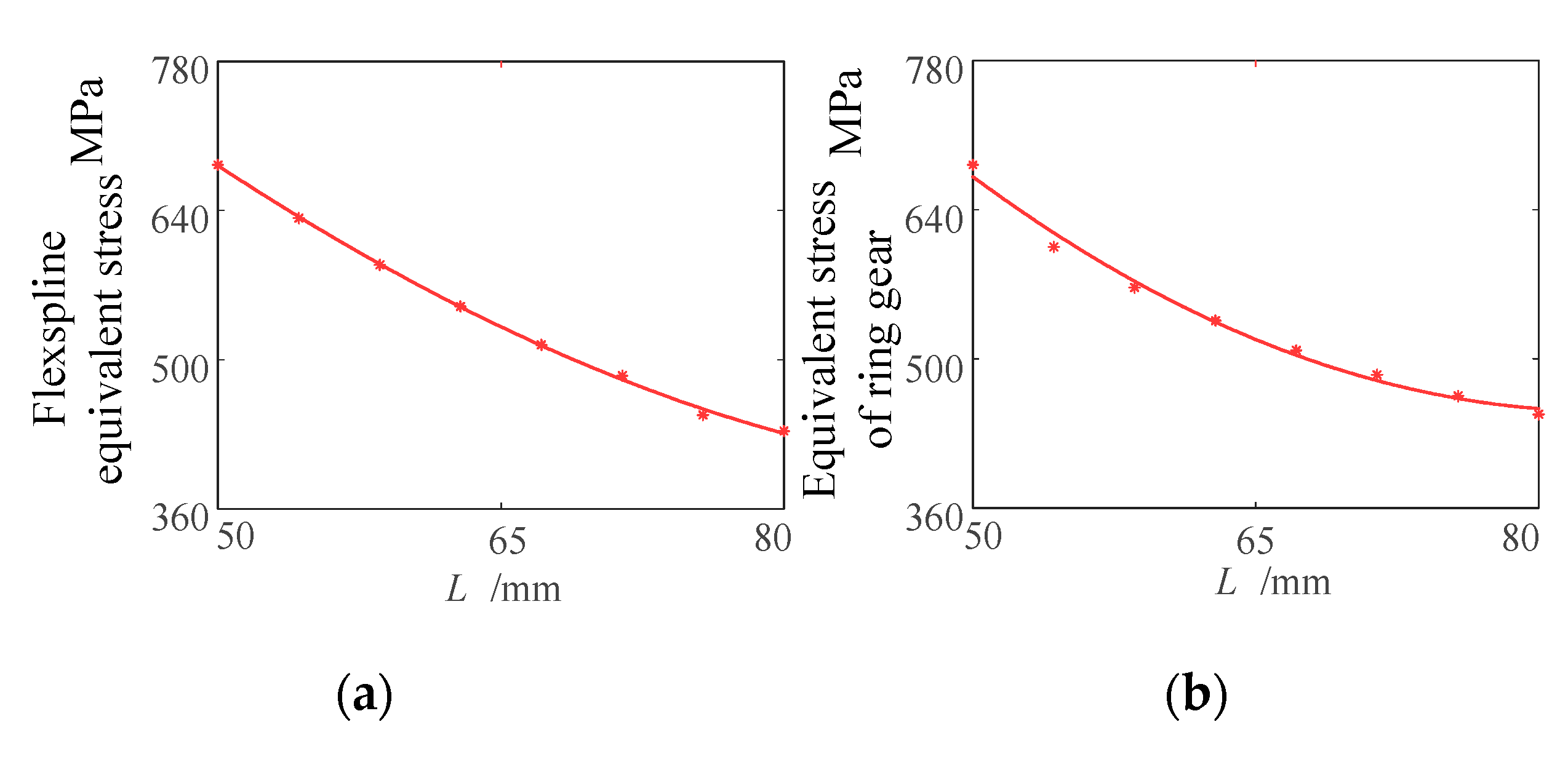
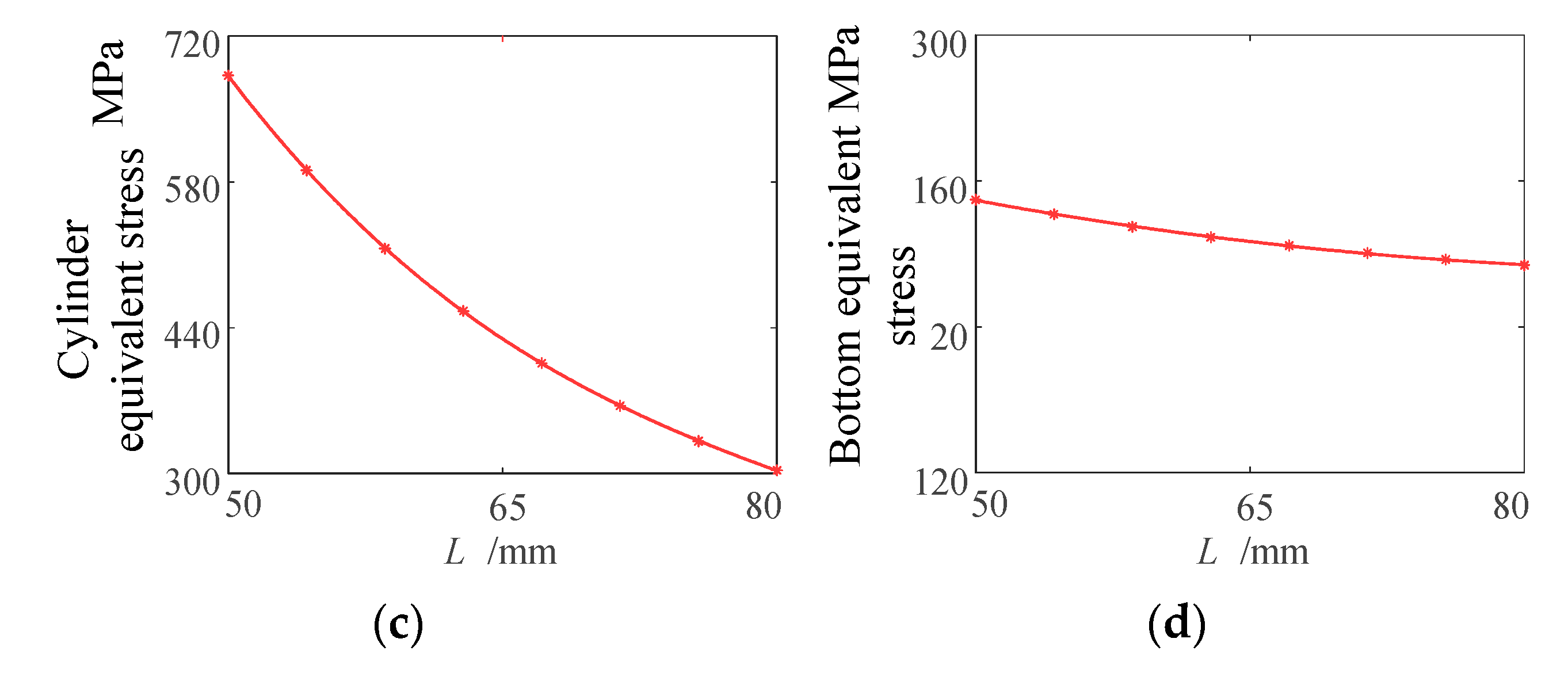
4.3. Analysis of the Influence of Cylinder Length on the Flexspline
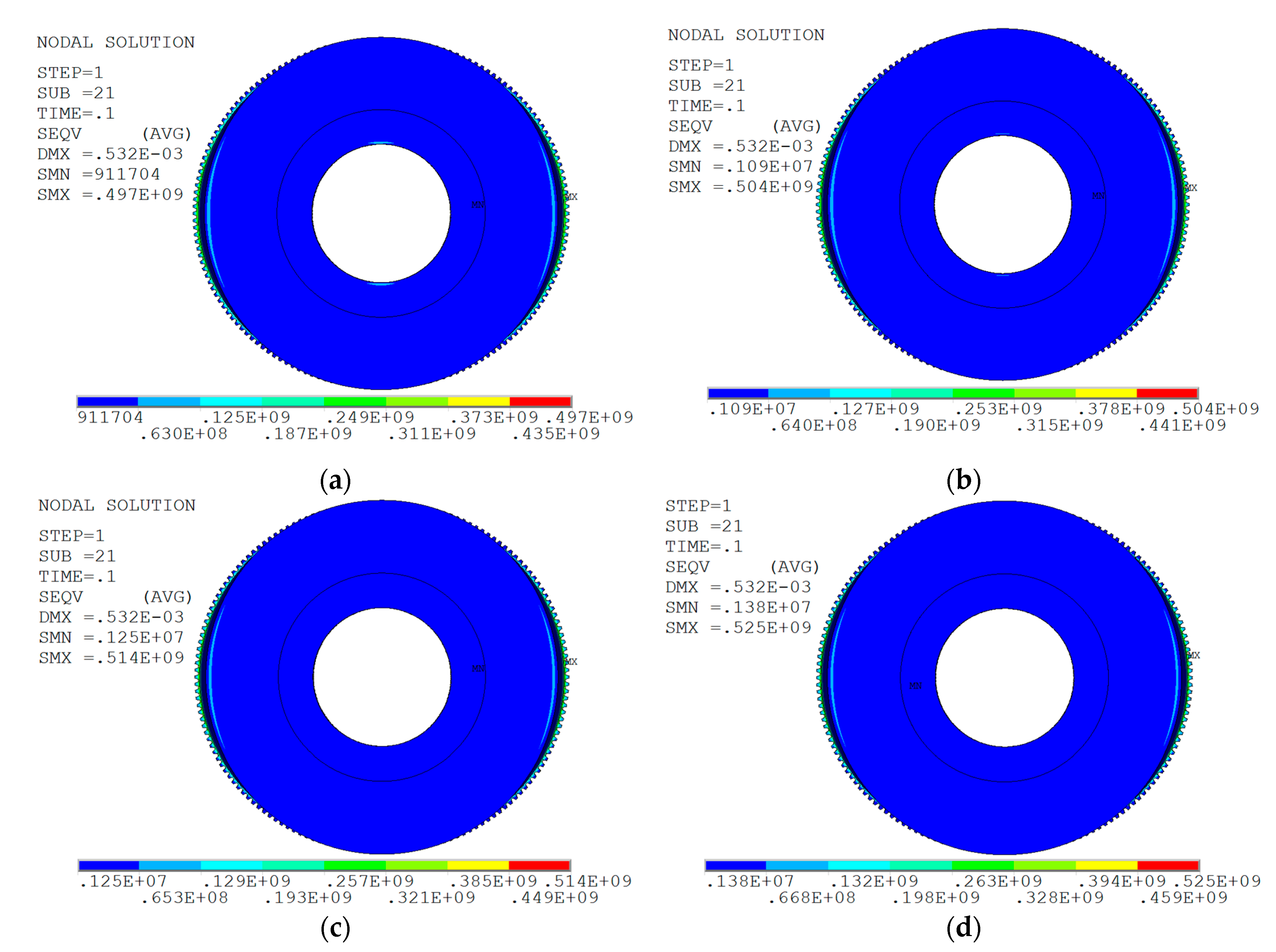
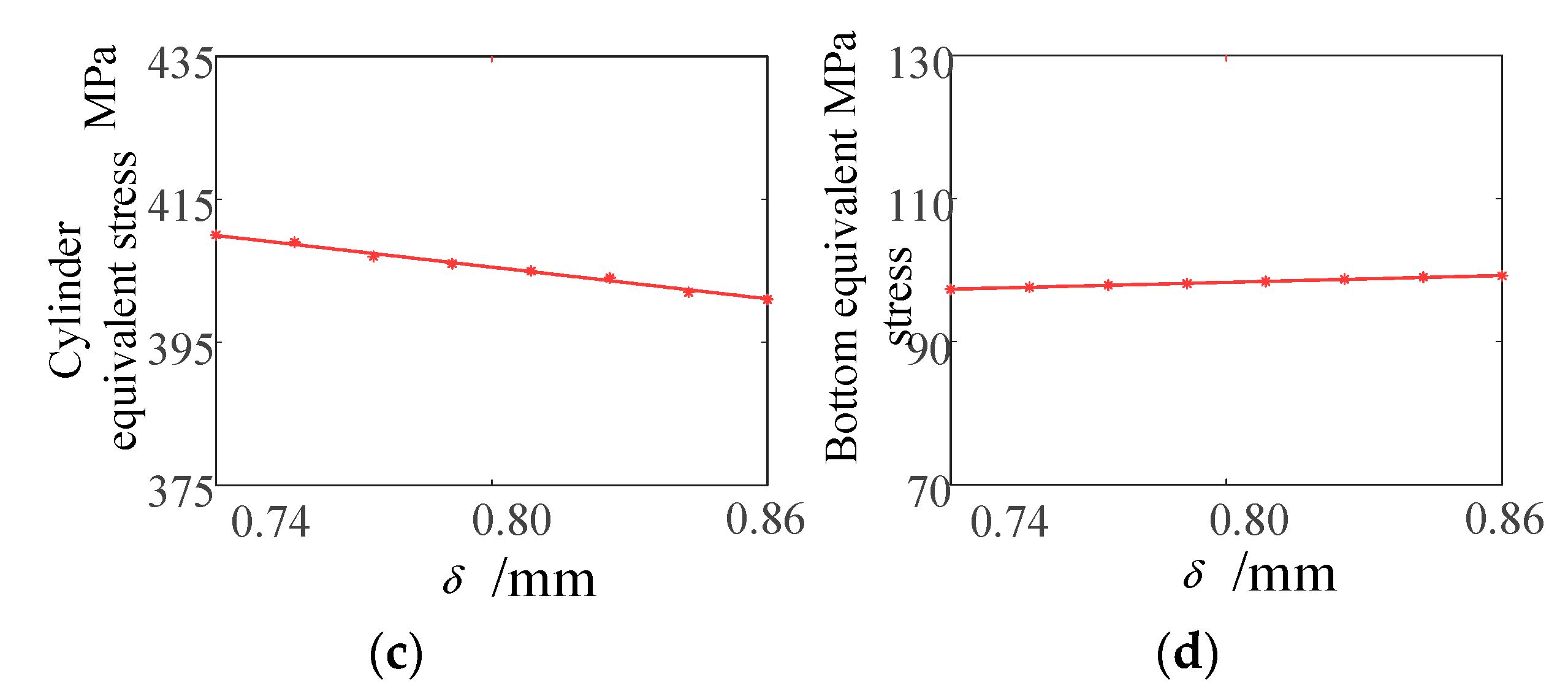
4.3.1. Analysis of the Influence of Cylinder Length on Flexspline Stress
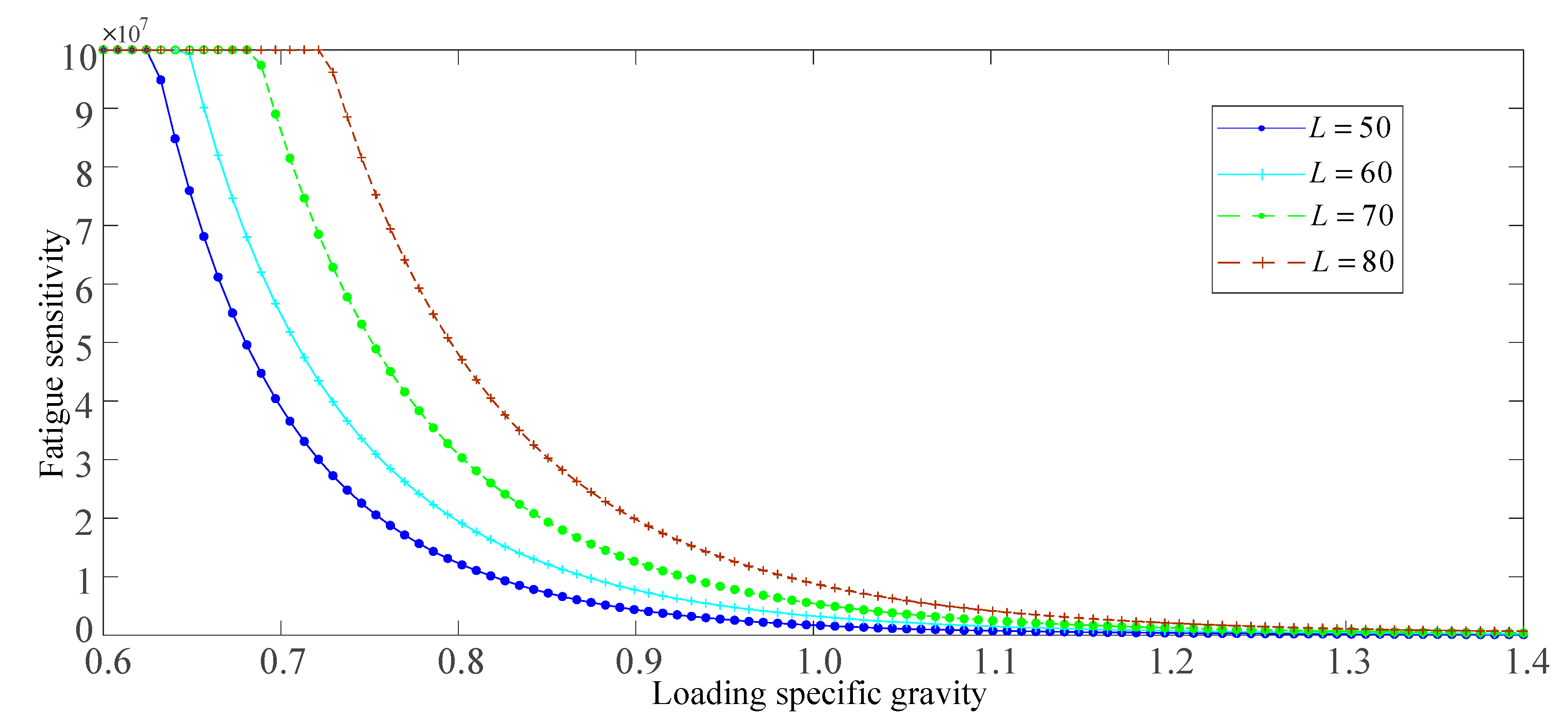
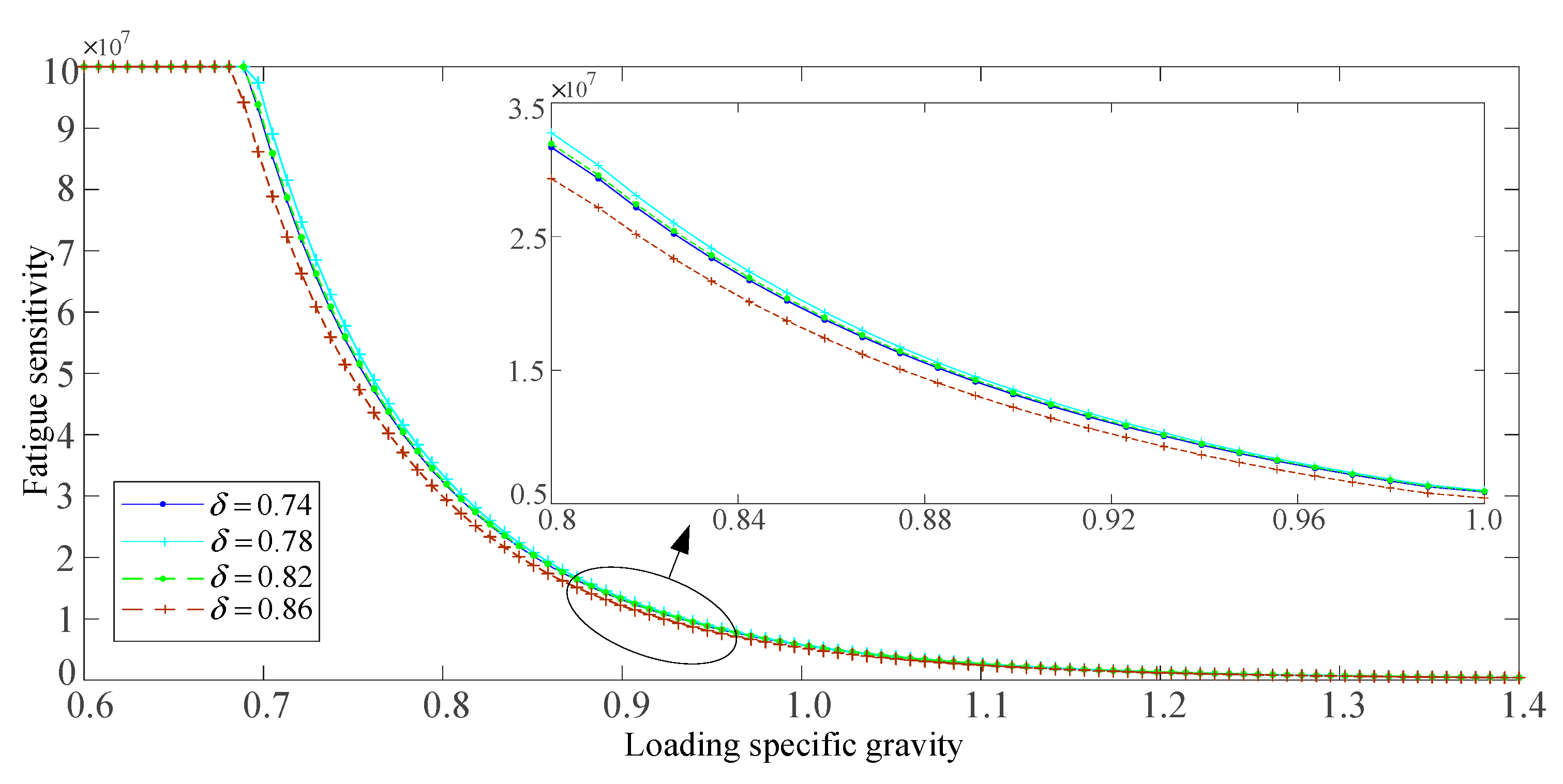
4.3.2. Influence of Cylinder Length on Fatigue Life
4.4. Analysis of the Influence of Smooth Cylinder Wall Thickness on the Flexspline
4.4.1. Analysis of the Influence of Smooth Cylinder Wall Thickness on Flexspline Stress
4.4.2. Influence of Wall Thickness of Smooth Cylinder on Fatigue Life
5. Conclusions
- (1)
- With the increase in the length of the cylinder, the maximum equivalent stress value of the flexspline decreases and the service life is evidently prolonged. Therefore, the stress condition of the flexspline can be improved by increasing the length of the cylinder, but the increase in the cylinder length will lead to an increase in volume and a decrease in torsional stiffness.
- (2)
- With the increase in smooth cylinder wall thickness, the maximum equivalent stress value of the flexspline body increases, but the increasing trend is not evident.
- (3)
- Compared with the wall thickness at the bottom of the cylinder and the wall thickness of the smooth cylinder, the length of the flexible cylinder has a greater impact on its fatigue life. The increase in flexible wheel cylinder length can significantly prolong its service life, but the increase in flexible wheel cylinder length will lead to an increase in volume and a decrease in torsional stiffness.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shen, Y.W.; Ye, Q.T. Theory and Design of Harmonic Gear Drive; Mechanical Industry Press: Beijing, China, 1985. [Google Scholar]
- Jia, H.; Li, J.; Xiang, G.; Wang, J.; Xiao, K.; Han, Y. Modeling and analysis of pure kinematic error in harmonic drive. Mech. Mach. Theory 2021, 155, 104122. [Google Scholar] [CrossRef]
- Li, X.; Song, C.; Yang, Y.; Zhu, C.; Liao, D. Optimal design of wave generator profile for harmonic gear drive using support function. Mech. Mach. Theory 2020, 152, 103941. [Google Scholar] [CrossRef]
- Li, S. Diaphragm stress analysis and fatigue strength evaluation of the flex-spline, a very thin-walled spur gear used in the strain wave gearing. Mech. Mach. Theory 2016, 104, 1–16. [Google Scholar] [CrossRef]
- Pacana, J.; Witkowski, W.; Mucha, J. FEM analysis of stressdistribution in the hermetic harmonic drive flexspline. Strength Mater. 2017, 49, 388–398. [Google Scholar] [CrossRef]
- Routh, B.; Maiti, R.; Ray, A.K.; Sobczyk, A. An investigation on secondary force contacts of tooth pairs in conventional harmonic drives with involute toothed gear set. Proc. Inst. Mech. Eng. 2015, 230, 622–638. [Google Scholar] [CrossRef]
- Kayabasi, O.; Erzincanli, F. Shape optimization of tooth profile of a flexspline for harmonic drive by finite element modeling. Mater. Des. 2007, 28, 441–447. [Google Scholar] [CrossRef]
- Ostapski, W.; Mukha, I. Stress State Analysis of Harmonic Drive Elements by FEM. Tech. Sci. 2007, 55, 115–123. [Google Scholar]
- Ostapski, W. Analysis of the Stress State in the Harmonic Drive Generator Flexspline System in Relationto Selected Structural Parameters and Manufacturing Deviations. Bull. Pol. Acad. Sci. Tech. Sci. 2010, 4, 693–698. [Google Scholar]
- Feng, F.; Wang, W.; Tang, L.N.; Fengfan, C.; Yanwei, B.; Shanghai Aerospace Equipments Manufacturer. Application and development trend of space high precision harmonic reducer. Mech. Transm. 2014, 38, 98–107. [Google Scholar]
- Leno, D.; Arzola, N.; Tovar, A. Statistical analysis of the influence of tooth geometry in the performance of a harmonic drive. J. Braz. Soc. Mech. Sci. Eng. 2015, 37, 723–735. [Google Scholar] [CrossRef] [Green Version]
- Gravagno, F.; Mucino, V.H.; Pennestrì, E. Influence of wave generator profile on the pure kinematic error and centrodes of harmonic drive. Mech. Mach. Theory 2016, 104, 100–117. [Google Scholar] [CrossRef]
- Zhang, C.; Shao, Z.; Zhu, Y.; Li, J. Austenite grain refinement and homogenization control of the flexspline for industrial robot harmonic drive. J. Mater. Eng. Perform. 2021, 30, 4393–4400. [Google Scholar] [CrossRef]
- Xiao, Q.; Han, X.; Jia, H. Dynamic optimum design and analysis of cam wave generator for harmonic gear drive. In Proceedings of the International Conference on Information and Automation, Shenzhen, China, 6–8 June 2011; pp. 315–319.
- Ye, N.H.; Deng, X.; He, Y.; Sun, Y. Mechanical analysis and fatigue life study of harmonic flexspline. J. Hunan Univ. Nat. Sci. Ed. 2018, 45, 18–25. [Google Scholar]
- Ivanov, M.H.; Shen, Y.W. Harmonic Gear Drive; National Defense Industry Press: Beijing, China, 1987. [Google Scholar]
- Ishikawa, S. Tooth Profile of Spline of Strain Wave. U.S. Patent 4,823,638, 25 April 1989. [Google Scholar]
- Ishikawa, S.; Kiyosawa, Y. Flexing Contact Type Gear Driveof Non-Profile-Shifted Two-Circular-Arc Composite Tooth Profile. U.S. Patent 5,458,023, 17 October 1995. [Google Scholar]
- Si, C.G.; Fan, Y.G.; Lin, Z.N.; Zhang, H.Y. Harmonic Gear Drive; National Defense Industry Press: Beijing, China, 1978. [Google Scholar]
- Rao, Z.G. Design of Planetary Transmission Mechanism; National Defense Industry Press: Beijing, China, 1980. [Google Scholar]
- Li, L.; Luo, Z.; He, F.; Sun, K.; Yan, X. An improved partial similitude method for dynamic characteristic of rotor systems based on Levenberg–Marquardt method. Mech. Syst. Signal Process. 2022, 165, 108405. [Google Scholar] [CrossRef]
- Chen, X.X.; Liu, Y.S.; Xing, J.Z.; Xu, W. Expansion and contraction deformation law of flexspline neutral layer in harmonic gear. J. Mech. Eng. 2014, 50, 189–196. [Google Scholar] [CrossRef]
- Gao, H.B.; Li, Z.G.; Deng, Z.Q. Sensitivity analysis of structural parameters of cup-shaped flexspline to flexspline stress based on ANSYS. Proc. Mech. Eng. Soc. 2010, 46, 1–7. [Google Scholar]
- John, S. Harmonic drives provide high torque without backlash. Assembly 2005, 48, 8–15. [Google Scholar]
- Zhao, S.B. Anti-Fatigue Design Manual; Machinery Industry Press: Beijing, China, 2015. [Google Scholar]
- Zhang, A.G.; Zhu, C.J.; Chen, M.C. Fatigue, Fracture and Injury; Southwest Jiaotong University Press: Chengdu, China, 2006. [Google Scholar]
- Wu, Y. Fracture and Fatigue; China University of Geosciences Press: Wuhan, China, 2008. [Google Scholar]
- Gao, Z.T. Fatigue Test Design and Data Processing; Beijing University of Aeronautics and Astronautics Press: Beijing, China, 1999. [Google Scholar]








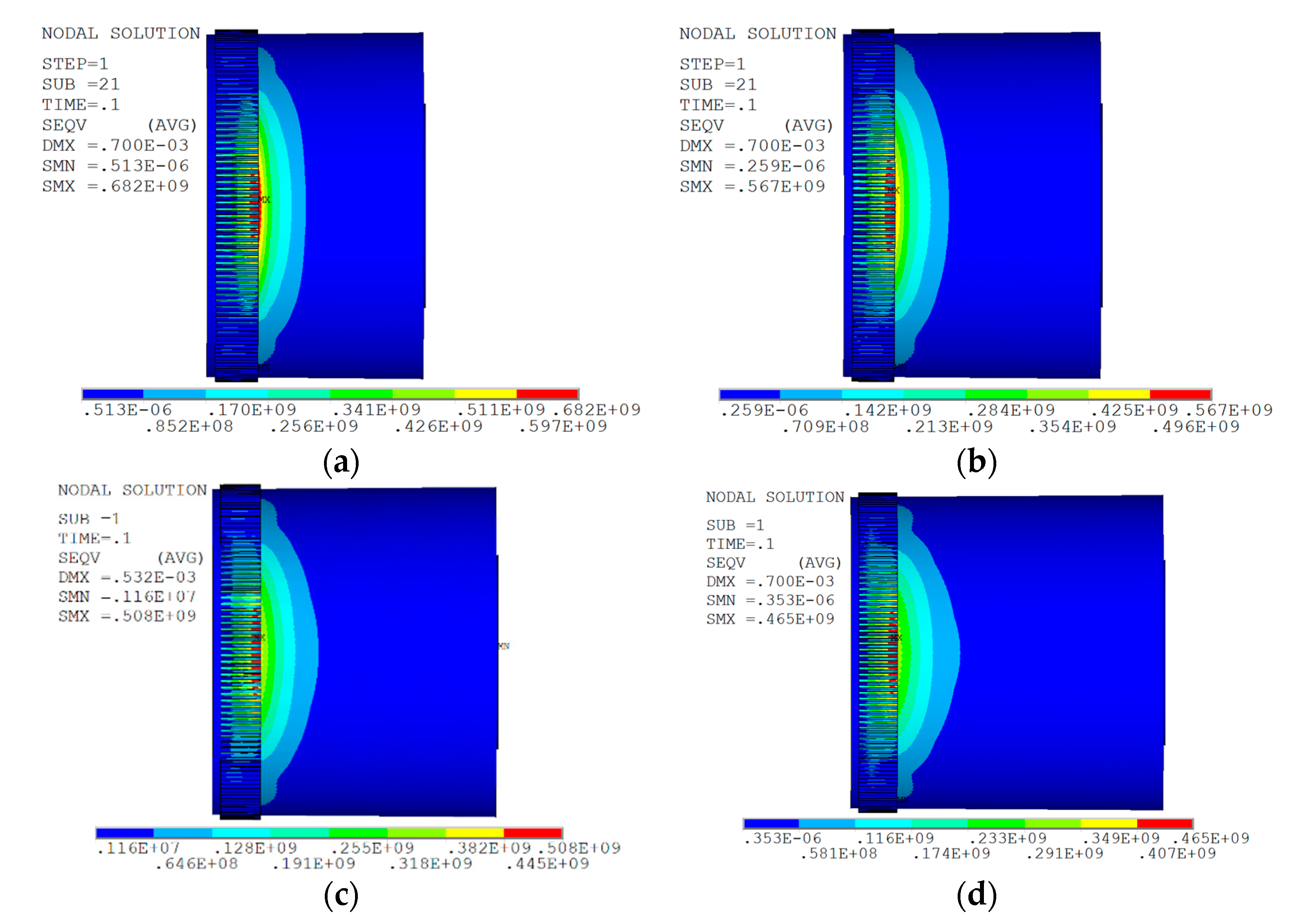

| T (N·m) | Q (MPa) | ||||||
|---|---|---|---|---|---|---|---|
| 0 | 6.6021 | 13.050 | 19.172 | 24.822 | 29.861 | 34.164 | |
| 70 | 37.623 | 40.162 | 41.709 | 42.229 | 41.709 | 40.162 | 37.623 |
| 34.164 | 29.861 | 24.822 | 19.172 | 13.050 | 6.6021 | 0 | |
| 0 | 7.5798 | 14.914 | 21.911 | 28.368 | 34.126 | 39.045 | |
| 80 | 43.002 | 45.890 | 47.668 | 48.262 | 47.668 | 45.890 | 43.002 |
| 39.045 | 34.126 | 28.368 | 21.911 | 14.914 | 7.5498 | 0 | |
| 0 | 8.4396 | 16.778 | 24.649 | 31.914 | 38.392 | 43.925 | |
| 90 | 48.377 | 51.637 | 53.626 | 54.295 | 53.626 | 51.637 | 48.377 |
| 43.925 | 38.392 | 31.914 | 24.649 | 16.788 | 8.4396 | 0 | |
| 0 | 9.4373 | 18.642 | 27.388 | 35.460 | 42.658 | 48.806 | |
| 100 | 53.752 | 57.375 | 59.585 | 60.328 | 59.585 | 57.375 | 53.752 |
| 48.806 | 42.658 | 35.406 | 27.388 | 18.642 | 9.4373 | 0 | |
| 0 | 10.381 | 20.506 | 30.127 | 39.006 | 46.924 | 53.687 | |
| 110 | 59.127 | 63.112 | 65.543 | 66.360 | 65.543 | 63.112 | 59.127 |
| 53.687 | 46.924 | 39.006 | 30.127 | 20.506 | 10.381 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Li, X.; Xu, J.; Guo, Y.; Li, B. Modeling and Fatigue Characteristic Analysis of the Gear Flexspline of a Harmonic Reducer. Mathematics 2022, 10, 868. https://doi.org/10.3390/math10060868
Yang H, Li X, Xu J, Guo Y, Li B. Modeling and Fatigue Characteristic Analysis of the Gear Flexspline of a Harmonic Reducer. Mathematics. 2022; 10(6):868. https://doi.org/10.3390/math10060868
Chicago/Turabian StyleYang, Hexu, Xiaopeng Li, Jinchi Xu, Yajing Guo, and Baitao Li. 2022. "Modeling and Fatigue Characteristic Analysis of the Gear Flexspline of a Harmonic Reducer" Mathematics 10, no. 6: 868. https://doi.org/10.3390/math10060868
APA StyleYang, H., Li, X., Xu, J., Guo, Y., & Li, B. (2022). Modeling and Fatigue Characteristic Analysis of the Gear Flexspline of a Harmonic Reducer. Mathematics, 10(6), 868. https://doi.org/10.3390/math10060868






